Fixed-time synchronization problem of coupled delayed discontinuous neural networks via indefinite derivative method

1.   Introduction

An artificial neural network is an important branch of a dynamic system, which has existed for more than a hundred years. From the initial basic research on the right-hand continuous differential equation ^[1,2,3], it has gradually developed into a comprehensive discipline, which not only includes the research on the theory of mathematics itself but also covers the applied research in many fields of applied engineering ^[4,5,6]. At the same time, due to the diversity of nature and the influence of many uncertain factors in practical engineering problems, the differential equation model needs to consider the effects of many conditions, such as time-delay ^[7], explosion ^[8], oscillation ^[9], impulse ^[10], Stochastic phenomenon ^[11] and so on, which will have an important impact on the existence, stability, periodicity and uniqueness of the model solution in the actual problems. In the specific field of artificial neural network systems, more and more scholars, whether in mathematics or engineering, take the model of discontinuous neural networks as the main research object. Due to the internal connection and interaction between different neurons in the neural network, the activation function of neurons in the considered time-delay model is discontinuous, and a series of coupling effects between neurons are studied to improve the general dynamic behavior of differential equations. At the same time, the synchronous stability dynamic behavior and control theory of neural network model are explored more deeply.

In dynamic systems, the neural network system is one of the disciplines most closely related to human body characteristics. The neural network system is based on the physiological structure characteristics of the human brain and corresponds to an organic topological dynamic system. In recent years, it has attracted countless experts and scholars to take neural network systems as the research object ^{[12,13,14,15,16]}. Corresponding to the discontinuous differential equation, the transmission between neural network signals are also usually a discontinuous process, so it is most practical to use discontinuous system to describe the model. This involves both Filippove functional differential inclusion theory and Forti's analysis tool ^[17,18,19]. The results of these right-hand discontinuous differential equations are mainly reflected in the conclusions of the existence ^[20], bifurcation ^[21], finite-time convergence ^[22], chaos ^[23], robustness ^[24] and uniqueness of the periodic solution of the system ^[25]. There are also many places worth studying in the development of artificial intelligence networks, big data cloud computing, machine learning and deep learning theory. On the other hand, the coupling relationship between different neurons in a general neural network is complex in practice, so the influence of the coupling relationship must be considered to make the neural network model more diversified and uncertain, and closer to the actual engineering state. Marcus and Westervelt pointed out in ^[26] that nonlinear coupling can change the stability state of neural networks, that is, the stability state of neural networks may change to an unstable state under the action of internal coupling. Therefore, after the internal coupling is introduced into the model, in order to maintain the stability state of the neural network model, more in-depth theoretical methods and more novel analysis tools are needed, such as optimizing the parameters of the model under the pattern recognition theory, and global optimization of neurons through the training method of machine learning. It is not only a challenge to the mathematical theory of functional differential inclusion framework, but also a need for more rigorous environmental analysis in the application of dynamic behavior results and learning methods of engineering technology models.

In engineering applications, the synchronization phenomenon plays a very important role in the neural network nodes, especially in some models with an external force or internal coupling relationships. If we ignore the influence of external force and only consider the coupling relationship between internal neurons, this synchronization is called self-synchronization, which is more extensive and ideal in practical engineering. Unfortunately, because every single node is interrelated, the synchronization phenomenon of this coupled neural network model with switching is not easy to design. With the development of sensor technology and computer technology, people have done a lot of empirical research on complex networks to understand their evolution laws (such as scale-free characteristics). In addition to exploring the basic mechanism of the statistical characteristics of these complex networks, researchers have also made substantial progress in the synthesis and analysis of dynamic behavior on complex networks.

The key point is to design suitable coupling laws and rules and obtain some coupling functions so that the states of all nodes in the considered system can be synchronized. Research shows that neural network synchronization is of great significance to control various more practical networks. For example, speed synchronization is the premise to solve the cluster control problem of a multi-agent dynamic systems ^[27]. Linear transformation technology is used to transform the flight problem of multiple aircraft formation into a synchronization problem ^[28]. The synchronization phenomenon itself is an important collective behavior in a neural network ^[29,30]. Synchronization can generally be divided into two types: local synchronization and global synchronization. Global synchronization is more widely used in practical applications because it can synchronize any given initial state. The methods adopted by researchers to achieve global synchronization are also diverse. For example, Lu in the article ^[31] introduced the concepts of the distance between node states and synchronization manifold, and proposed a new global synchronization research method of the coupled neural network system. In reference ^[32], the coupled linear system is studied by state-feedback control. For non-strongly connected networks or problems without directed spanning trees, the literature ^[33,34,35] studied the synchronous traction control theory of complex networks, which can be used for the characteristics of network topology such as strong connection, undirected network connection, scale-free characteristic network or stochastic network, and can realize global traction synchronous control under the stability framework.

The research on the FTS problem of nonlinear systems is still in its infancy, and its application lags behind. It is urgent and of great significance to further develop and study the FTS. We urgently need to establish some effective FTS criteria. Generally speaking, it is almost impossible to obtain the accurate time value of the FTS issue, so an important index of studying FTS is to estimate its stability time with high precision. In addition to establishing some standards to gain FTS for nonlinear dynamic systems, i. The important problem is how to optimally estimate the fixed convergence time.

In short, the synchronization problem of complex networks in engineering, especially considering the late start of the research on the coupling problem between neurons, the functional differential inclusion theory applied to it is not very complete, the specific application is relatively one-sided, and there are no new methods and tools, but it itself is a hot issue with a wide range of applications. This also urgently needs the efforts of scholars to further improve and develop the application of functional differential equation theory in complex neural networks, further explore and study the general dynamic behavior of discontinuous neural network systems and better realize the FTS of the system.

Based on the above statements, this brief provides a state-feedback control scheme and adaptive control scheme for FTS of the CDNNs with indefinite derivative. The main contributions of this paper are summarized as follows:

$\diamond$ This paper comprehensively considers a class of CDNNs system, and the activation function is discontinuous, which is more complex than the previous literature models.

$\diamond$ We consider the variable coefficient nonautonomous system, and use the Lyapunov method of indefinite derivative, it is state-dependent and further expands the convergence accuracy of the system. The restriction that the $V$-function must be negative definite is broken.

$\diamond$ By designing an appropriate controller and considering the FTS problem of CDNNs, this paper can ensure that the system can achieve synchronization faster and the settling time is shorter.

2.   Model description and some preliminaries

In order to get our main results, this part gives some basic definitions, lemmas and assumptions, which can be obtained from some basic references ^{[36,37,38,39,40,41]}.

In this section we introduce a mathematical model of CDNNs as follows:

where $x(t) = (x_{1}(t), x_{2}(t), \ldots, x_{n}(t))^{T}\in {\mathbb R}^{n}$ is the state variable of the $i$th neuron at time $t$; $D = \mathrm{diag}(d_{1}, d_{2}, \ldots, d_{n})$ denotes the self-inhibition with $d_{i} > 0$ and when disconnected from the network and input, the neuron will reset its potential to the resting state in the isolated state; $A = (a_{ij})_{n\times n}$ and $B = (b_{ij})_{n\times n}$ represent the non-delay connection strength and the delayed connection strength of $j$th neuron on the $i$th neuron, respectively; $f(x(t)) = (f_{1}(x_{1}(t)), \ldots, f_{n}(x_{2}(t)), \ldots, f_{n}(x_{n}(t)))^{T}$ expresses the activation function of $j$th neuron. Moreover, $I = (I_{1}, I_{2}, \ldots, I_{n})^{T}$ is a input vector, and $\tau(t)$ denotes to the time-varying transmission delay with $0\leq\tau(t)\leq\tau$.

Now in this brief one presents that the activation function $f_{i}(\cdot)$ is piecewise for $i\in1, 2, \ldots, n$, then we can gain the solution of model (2.1) as follows:

where $\mathbb{F}(x)\triangleq K[f(x)] = (K[f_{1}(x)], K[f_{2}(x)], \ldots, K[f_{n}(x)])$, which $K[f_{i}(x)] = \big[\min\{f_{i}(x^{-}), f_{i}(x^{+})\}, \max\{f_{i}(x^{-}), f_{i}(x^{+})\}\big]$.

By the framework of Filippov ^[39] and the measurable selection theorem ^[40]. If $x(t)$ is a continuous solution of (2.1) in $[-\tau, T)$, and $x$ is absolutely continuous on any compact subinterval of $[0, T)$. Then we can have

where $\gamma(t)\in K[f(x(t))]$ is a measurable function.

Definition 2.1 (see ^[36]). If the CDNNs (2.1) is finite-time stabilized to the equilibrium point $x^{*}$, for the solution of CDNNs (2.1) $x(t)$, there exists a time $T^{*}(x_{0}, t_{0})$ such that $\lim_{t\rightarrow T^{*}}\|x(t)-x^{*}\| = 0$ and $\|x(x)-x^{*}\|\equiv0$ for all $t > T^{*}$. Moreover, if the time $T^{*}(x_{0}, t_{0})$ which is bounded on any initial state point $x_{0}\in {\mathbb R}^{n}$, the equilibrium point of CDNNs (2.1) is said to be fixed-time stabilized, that is, there exists a constant $T_{\max} > 0$ such that $T^{*}(x_{0}, t_{0})\leq t_{0}+T_{\max}$, which $T^{*}(x_{0}, t_{0})$ is called settling time.

The aim of this brief is to solve the FTS issue of considered system. Before that, we give the following basic assumption of CDNNs.

Hypothesis. For $f\in\mathcal{G}_{\mathcal{D}}$, there exist nonnegative constants $\alpha$ and $\beta$ such that

Definition 2.2 (see ^[4]). If the function $\psi: {\mathbb R}_{+}\rightarrow {\mathbb R}_{+}$ is continuous and strictly increasing with $\psi(0) = 0$, $\psi$ is called a $\mathcal{K}$-function and it can be expressed as $\psi\in\mathcal{K}$. Moreover, if $\lim_{s\rightarrow +\infty}\psi(s) = +\infty$ holds, the function $\psi$ is called a $\mathcal{K}_{\infty}$-function with $\psi\in\mathcal{K}_{\infty}$.

Lemma 2.3 (C-Regular see ^[40]). The function $V: {\mathbb R}^{n}\rightarrow {\mathbb R}^{n}$ is C-Regular if and only if $V(x)$ satisfies:

1) regularity in ${\mathbb R}^{n}$;

2) $V(x) > 0$ for $x\neq0$ and $V(0) = 0$;

3) $V(x)\rightarrow +\infty$ as $\|x\|\rightarrow +\infty$.

Lemma 2.4 (Chain Rule see ^[40]). $V(x)$ is C-regular and for $x(t)$ denotes absolute continuity function in $[t_{0}, +\infty)\rightarrow {\mathbb R}^{n}$, then $V(x(t))$ is differentiable for a.e. $t\geq t_{0}$, and $\frac{\mathrm{d}V(x(t))}{\mathrm{d}t} = < \mathcal{L}(t), \frac{\mathrm{d}x(t)}{\mathrm{d}t} >$, $\mathcal{L}(t)\in\partial V(x(t))$.

Lemma 2.5 (see ^[33]). If the graph $\mathcal{F}$ is undirected, and the adjacency matrix is $D = [d_{ij}]$ and the corresponding Laplacian matrix is $L$, for arbitrary $x = (x_{1}, x_{2}, \ldots, x_{n})\in {\mathbb R}^{n}$, one can obtain

Lemma 2.6 (see ^[41]). For the positive numbers $\lambda_{1}, \lambda_{2}, \ldots, \lambda_{n}$ and $0 < \theta < \vartheta$, then one can have

Remark 2.7. The nonlinear activation function $f$ in this brief is different from the previous literature on neural network dynamics, because our system may no longer satisfy the conventional Lipschitz condition. Different from the previous researches, the biggest difference in our model is that we recognize that the activation function of neurons is discontinuous. In addition, the internal system of neurons is not an independent system, they affect and connect with each other. The nonlinear coupling relationship considered in this paper makes the system closer to engineering practice and more complex, which generalizes the conclusion in the previous literature.

3.   Main results

In this section, we consider the FTS problem for $N$ identical discontinuous CDNNs (2.1) with nonlinear coupling. Firstly, one takes the system (2.1) as the driving system, the corresponding $N$ response system equation is described as follows:

where $y_{i}(t) = (y_{i1}(t), y_{i2}(t), \ldots, y_{in}(t))^{T}\in {\mathbb R}^{n}(i = 1, 2, \ldots, N)$ are the state variable of the $i$th neuron; $m$ is the coupling strength, $\phi$ is the nonlinear coupling function. Matrix $C = [c_{ij}]$ denotes the adjacency matrix of subsystems, which corresponding Laplacian matrix is represented as $L$, and all of them are applicable to undirected weighted networks. $u_{i}(t)$ is the controller.

Then subtracting (2.1) from (3.1), we can get the following error dynamical systems:

where $i = 1, 2, \ldots, N$, $e_{i}(t) = y_{i}(t)-x(t)$, $\widetilde{f}_{i}(t) = f(y_{i}(t))-f(x(t))$, $\widetilde{f}_{i}(t-\tau(t)) = f(y_{i}(t-\tau(t)))-f(x(t-\tau(t)))$.

Then according to the theories of set-valued maps and differential inclusions, we can have

or, through the Filippov regularization discussed above, for a.e. $t\in[0, T)$, there exist measurable functions $\widetilde{\gamma}_{j}(t)\in K[f(y_{i}(t))]$, $\gamma^{*}_{j}(t)\in K[f(x(t))]$, one can have

where $\gamma_{i}(t) = \widetilde{\gamma}_{i}(t)-\gamma^{*}_{i}(t)$.

For the sake of convenience, we denote $A = (a_{ij})_{k, l = 1}^{n}$, $B = (b_{ij})_{k, l = 1}^{n}$ and $a^{\max} = \max_{1\leq k, l\leq n}|a_{kl}|$, $b^{\max} = \max_{1\leq k, l\leq n}|b_{kl}|$.

Moreover, the nonlinear coupling function $\phi$ in this brief can be described as:

where $\Xi = (1, 1, \ldots, 1)^{T}$, $\mathrm{SIGN}(\nu) = \mathrm{diag}(\mathrm{sign}(\nu_{1}), \mathrm{sign}(\nu_{2}), \cdots, \mathrm{sign}(\nu_{n}))$; when $\mathrm{sign}(\nu_{i}) = 1$ with $\nu_{i} > 0$; $\mathrm{sign}(\nu_{i}) = 0$ with $v_{i} = 0$; $\mathrm{sign}(v_{i}) = -1$ with $v_{i} < 0$; and the controller is designed by:

where $0 < \theta < 1$, $\vartheta > 1$, and $Q(t)$ is a $K_{\infty}$-function.

Then the main results in this paper are given by the following theorems.

Theorem 3.1. If the activation function $f$ of the system is assumed as the above Hypothesis. The response system (3.1) with nonlinear coupling (3.3) and novel controller (3.4) synchronize to the drive system (2.1) in a fixed-time if the following two equals hold for every $i\in {\mathbb N}$:

(H) $d^{\min} > \alpha a^{\max}+n\alpha b^{\max}$ and $r_{i} > n\beta(a^{\max}+b^{\max})$.

Proof. In order to get the fixed time result, the proof needs to be divided into the following two steps:

Step 1: Firstly, choose a non-negative function $V(t, e)$ as follows:

Taking the derivative of $V(t, e)$ along the trajectories of (3.2)

According to the Hypothesis of function $f$, one can have

According to lemma 2.5, we know that for adjacency and symmetric matrix $C = [c_{ij}]_{n\times n}$ in undirected topological graph, there exists a Laplace matrix $L$ corresponding to it, then

The same as (3.8), one can have

Combining the above Eqs (3.6)–(3.9), the Eq (3.5) can be reduced to

Recalling the inequality lemma 2.6 and by the assumption of this theorem, one can get $\sum\limits^{N}_{i = 1}\sum\limits^{n}_{k = 1}|e_{ik}(t)|^{1+\theta}\geq \big(\sum\limits^{N}_{i = 1}\sum\limits^{n}_{k = 1}|e_{ik}(t)|^{2}\big)^{\frac{1+\theta}{2}} = V^{\frac{1+\theta}{2}}(t, e)$ and $\sum\limits^{N}_{i = 1}\sum\limits^{n}_{k = 1}|e_{ik}(t)|^{1+\vartheta}\geq n^{\frac{1}{\theta}-\frac{1}{\vartheta}}\big(\sum\limits^{N}_{i = 1}\sum\limits^{n}_{k = 1}|e_{ik}(t)|^{2}\big)^{\frac{1+\vartheta}{2}} = n^{\frac{1}{\theta}-\frac{1}{\vartheta}}V^{\frac{1+\vartheta}{2}}(t, e)$, then the Eq (3.5) can be reduced to

where $\breve{p} = \min_{1\leq i\leq N}\{p_{i}\}$ and $\breve{q} = \min_{1\leq i\leq N}\{q_{i}\}\cdot n^{\frac{1}{\theta}-\frac{1}{\vartheta}}$.

Step 2: Then we will show the FTS of the drive-response error system (3.2) and give the settling time $T^{*}(e_{0}, t_{0})$, which is bounded without $e_{0}$ by new indefinite derivative method.

Because $0 < \frac{1+\theta}{2} < 1$ and $\frac{1+\vartheta}{2} > 1$, multiplying both sides of (3.11) by $V^{-\frac{1+\theta}{2}}(t, e)$, one can have

let $W(t, e) = V^{\frac{1-\theta}{2}}(t, e)$, then we can expand the inequality (3.12) as

multiplying both sides of (3.13) by $e^{-\int^{t}_{t^{*}}Q(\varsigma)\mathrm{d}\varsigma}$, for a.e. $t\geq t^{*}$ we get

Integrate both sides of (3.14) from $t^{*}$ to $t$ and according to the definition of $K_{\infty}$-function, which implies $\int^{t}_{t_{0}}Q(s)\mathrm{d}s\leq -\lambda(t-t_{0})+M$, then one can get

which implies

Moreover, from (3.11) it's easily to see that $\frac{\mathrm{d}V(t, e)}{\mathrm{d}t}\leq Q(t)V(t, e)$, which yields for $t^{*} > t_{0}$

Combined with (3.16) and (3.17), it is easy to see $V(t, e(t))\equiv0$ if the following inequality holds:

which yield

where $t^{*}$ is a priori condition to make inequality (3.17) true, it is not difficult to find that when $t_{0}$ and $e_{0}$ are determined, one can find the corresponding $t^{*}$, then according to (3.16)–(3.19) and the fact $e^{\int^{t}_{t^{*}}Q(\varsigma)\mathrm{d}\varsigma} > 0$, when $t\geq T^{*}(t_{0}, e_{0}) = t^{*}+\frac{1}{\lambda}\ln\Big(1+\frac{2\lambda e^{M}\Upsilon}{(1-\theta)\breve{p}}\Big)$, one can obtain $V(t, e(t))\equiv0$. Then the FTS can be finally realized and the settling time can be estimated by $T^{*}(t_{0}, e_{0})$.

The corresponding adaptive controller of (3.4) can be designed as

where $\Delta_{i} = \mathrm{diag}(\zeta_{i1}, \zeta_{i2}, \ldots, \zeta_{in})$, $\Pi_{i} = \mathrm{diag}(\pi_{i1}, \pi_{i2}, \ldots, \pi_{in})$, $\Lambda_{i} = \mathrm{diag}(\epsilon_{i1}, \epsilon_{i2}, \ldots, \epsilon_{in})$, $\Xi = (1, 1, \ldots, 1)^{T}$ and for $k = 1, 2, \ldots, n$ the controller rules of $\zeta_{ik}$ $\pi_{ik}$ and $\epsilon_{ik}$ satisfy:

where $p_{ik}$, $q_{ik}$ and $r_{ik}$ are adaptive coefficients need to be determined.

Theorem 3.2. If the condition of theorem 3.1 holds and the activation function $f$ of the system is assumed as the above Hypothesis. The response system (3.1) with nonlinear coupling (3.3) can synchronize to the drive system (2.1) in a fixed-time by adding the adaptive controller (3.20) and choosing the suitable adaptive coefficients.

Proof. We construct the following Lyapunov function:

Taking the derivative of $V(t, e)$ along the trajectories of (3.2)

By using the proof of theorem 3.1, the above Eq (3.21) can be simplification and consolidation as

Recalling the inequalities (3.6)–(3.9) and the assumption of this theorem, one can reduce $\dot{V}(t, e)$ to

where $\breve{p} = \min_{1\leq i\leq N, 1\leq k\leq n}\{p_{ik}\}$ and $\breve{q} = \min_{1\leq i\leq N, 1\leq k\leq n}\{q_{ik}\}\cdot n^{\frac{1}{\theta}-\frac{1}{\vartheta}}$.

Then according to the Step 2 of the theorem 3.1, the FTS of the drive-response error system can be achieved. Moreover, the settling time $T^{*}(t_{0}, e_{0})$ can be estimated by (3.19).

Remark 3.3. The key to the proof in this paper is that there is a non-negative $Q(t)$ term. Compared with the literature ^[4], the assumption of $Q(t)$ only needs to make $m$ and $\lambda$ nonnegative, which expands the scope of $Q(t)$ and obtains more general results, which shows that this paper generalizes the previous conclusions.

Remark 3.4. Unlike the fixed time stability theorem in previous literature ^[14,25,36], the derivative of $V(t)$ designed in this paper is not required to be non-negative, but an indefinite derivative. The system still achieves FTS in this case. Moreover, the conclusion can be applied to both discontinuous systems and continuous systems by omitting the conventional $V$-function condition, therefore, our conclusion is more general than the existing literature.

Remark 3.5. There are many interesting changes in the internal topological relationship of a neural network, such as clustering, multilayer-NN, leader-follower, impulse and other models. The relatively novel methods in this paper can be applied to the above types. In fact, once the derivative of $V(x)$ function does not need to meet the negative qualitative conditions, the selectable range of $V(x)$ function will be expanded. As a general conclusion, the results of this paper provide a wide application of Lyapunov theory.

4.   Simulation examples

In this part, in order to gain the validity of our proposed method, we introduce two numerical examples and simulations to prove its effectiveness.

Example 4.1. For $i = 1, 2, 3, 4, 5$, the three-dimensional model and its coefficients can be described as follows:

where $m = 1$, $D = \begin{pmatrix} 9 & 0 & 0 \\ 0 & 9 & 0 \\ 0 & 0 & 9 \end{pmatrix}$; $A = \begin{pmatrix} 1 & 2 & 0 \\ 2 & 1 & 0 \\ 0 & -1 & 1 \end{pmatrix}$; $B = \begin{pmatrix} 2 & -1 & 0 \\ 0 & 2 & -2 \\ -2 & 0 & 2 \end{pmatrix}$; The discontinuous activation function $f$ is expressed as follows:

Let $\alpha = 1$, $\beta = 0.4$, one can easily see that the Hypothesis in this paper of $f$ holds.

Moreover, we randomly select a relationship between different neurons of coupling network, and its topology rule and the corresponding adjacency matrix can be shown as follows:

and the corresponding Laplace matrix $\lambda_{\min}(L) = 0$.

Now we choose the controller

with $Q(t) = \frac{1}{1+t^{2}}$, $p_{i} = q_{i} = 1$, $r_{i} = 5$ for every $i\in {\mathbb N}$, and $\theta = 0.5$, $\vartheta = 2$, one can check that:

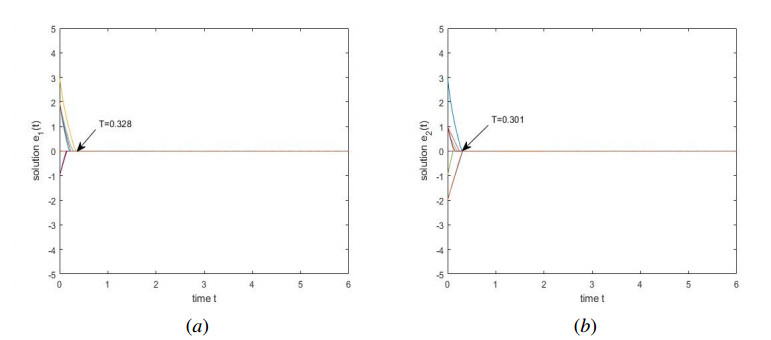
Then the assumptions of theorem 3.1 hold, the CDNNs (4.1) with nonlinear coupled can achieve FTS by adding the suitable controller. The simulation results are shown in Figure 1(a). Moreover, the settling time can be estimated as $T^{*}(t_{0}, e^{0}) = 0.328$. A numerical example verifies our theoretical analysis and research results.

Example 4.2. Reconsider the model (4.1) i.e., let $N = 8$, and its topology rule and the corresponding adjacency matrix can be shown as follows:

And the corresponding Laplace is $L$, one can see that $\lambda_{\min}(L) = 0$, $\alpha = 1$ and $\beta = 0.4$. Then Now we choose the adaptive controller (3.20) with $Q(t) = \frac{1}{1+t^{2}}$, $p_{i} = q_{i} = 2$, $r_{i} = 4$ for every $i\in {\mathbb N}$, and $\theta = 0.5$, $\vartheta = 2$, then we can check the conditions of Theorem 3.2 are satisfied. According to theorem 3.2, the CDNNs (4.1) with nonlinear coupled can achieve FTS. The simulation results can be shown in Figure 1(b). Moreover, the settling time is estimated as $T^{*}(t_{0}, e^{0}) = 0.301$. $

5.   Conclusions

This paper briefly introduces the FTS of complex CDNNs with discontinuous systems and time-delay. The main methods are based on Lyapunov functional and undirected topological graph theory, the new state-feedback controller and the corresponding adaptive controller schemes are established in the form of vectors. We especially use the use the method of indefinite derivative to solve the FTS problem of the CDNNs, which is independent of the initial value. The restriction that $V$-function must be negative definite is broken. Finally, we verify the correctness and effectiveness of the experimental and theoretical methods in this paper through experimental analysis and numerical simulation. In addition, our control method and the calculation technique of indefinite derivative established are relatively novel in this brief, and they can be extended to many fields, such as interconnected fuzzy neural networks ^[42], multi-agent systems ^[43], uncertain impulsive static neural networks ^[44], stochastic information constraints ^[45].

Funding

This work is supported by the National Natural Science Foundation of China (11501054, 11801042), Scientific Research Foundation of Hunan Provincial Education Department (19B064, 21B0771), and Training Program for Excellent Young Innovators of Changsha (kq20106072).

Conflict of interest

All authors declare that they have no conflict of interest.