Study of numerical treatment of functional first-kind Volterra integral equations

Hassanein Falah; Parviz Darania; Saeed Pishbin; Hassanein Falah; Parviz Darania; Saeed Pishbin

doi:10.3934/math.2024846

AIMS Mathematics

2024, Volume 9, Issue 7: 17414-17429. doi: 10.3934/math.2024846

Previous Article Next Article

Research article

Study of numerical treatment of functional first-kind Volterra integral equations

Department of Mathematics, Faculty of Sciences, Urmia University, P. O. Box 165, Urmia, Iran

Received: 26 March 2024 Revised: 27 April 2024 Accepted: 30 April 2024 Published: 21 May 2024
MSC : 45L05, 65R20

First-kind Volterra integral equations have ill-posed nature in comparison to the second-kind of these equations such that a measure of ill-posedness can be described by $\nu$ -smoothing of the integral operator. A comprehensive study of the convergence and super-convergence properties of the piecewise polynomial collocation method for the second-kind Volterra integral equations (VIEs) with constant delay has been given in ^[1]. However, convergence analysis of the collocation method for first-kind delay VIEs appears to be a research problem. Here, we investigated the convergence of the collocation solution as a research problem for a first-kind VIE with constant delay. Three test problems have been fairly well-studied for the sake of verifying theoretical achievements in practice.

Keywords:

Citation: Hassanein Falah, Parviz Darania, Saeed Pishbin. Study of numerical treatment of functional first-kind Volterra integral equations[J]. AIMS Mathematics, 2024, 9(7): 17414-17429. doi: 10.3934/math.2024846

Related Papers:

[1]	Faheem Khan, Tayyaba Arshad, Abdul Ghaffar, Kottakkaran Sooppy Nisar, Devendra Kumar . Numerical solutions of 2D Fredholm integral equation of first kind by discretization technique. AIMS Mathematics, 2020, 5(3): 2295-2306. doi: 10.3934/math.2020152
[2]	Sima Karamseraji, Shokrollah Ziari, Reza Ezzati . Approximate solution of nonlinear fuzzy Fredholm integral equations using bivariate Bernstein polynomials with error estimation. AIMS Mathematics, 2022, 7(4): 7234-7256. doi: 10.3934/math.2022404
[3]	Xiaopeng Yi, Chongyang Liu, Huey Tyng Cheong, Kok Lay Teo, Song Wang . A third-order numerical method for solving fractional ordinary differential equations. AIMS Mathematics, 2024, 9(8): 21125-21143. doi: 10.3934/math.20241026
[4]	Sunyoung Bu . A collocation methods based on the quadratic quadrature technique for fractional differential equations. AIMS Mathematics, 2022, 7(1): 804-820. doi: 10.3934/math.2022048
[5]	Younes Talaei, Sanda Micula, Hasan Hosseinzadeh, Samad Noeiaghdam . A novel algorithm to solve nonlinear fractional quadratic integral equations. AIMS Mathematics, 2022, 7(7): 13237-13257. doi: 10.3934/math.2022730
[6]	Ziqiang Wang, Kaihao Shi, Xingyang Ye, Junying Cao . Higher-order uniform accurate numerical scheme for two-dimensional nonlinear fractional Hadamard integral equations. AIMS Mathematics, 2023, 8(12): 29759-29796. doi: 10.3934/math.20231523
[7]	Adil Owaid Jhaily, Saeed Sohrabi, Hamid Ranjbar . On the numerical solution of highly oscillatory Fredholm integral equations using a generalized quadrature method. AIMS Mathematics, 2025, 10(3): 5631-5650. doi: 10.3934/math.2025260
[8]	Ishtiaq Ali . Long time behavior of higher-order delay differential equation with vanishing proportional delay and its convergence analysis using spectral method. AIMS Mathematics, 2022, 7(4): 4946-4959. doi: 10.3934/math.2022275
[9]	Waleed Mohamed Abd-Elhameed, Youssri Hassan Youssri . Spectral tau solution of the linearized time-fractional KdV-Type equations. AIMS Mathematics, 2022, 7(8): 15138-15158. doi: 10.3934/math.2022830
[10]	Ziqiang Wang, Qin Liu, Junying Cao . A higher-order numerical scheme for system of two-dimensional nonlinear fractional Volterra integral equations with uniform accuracy. AIMS Mathematics, 2023, 8(6): 13096-13122. doi: 10.3934/math.2023661

Abstract

1. Introduction

The exploration of delay differential equations (DDEs) to represent optical devices commenced in 1979, with Ikeda's anticipation of chaos in an optically bistable device ^[2,3]. As the majority of the suggested models featured a delay significantly surpassing other time scales of the device, mathematicians became intrigued by the limit of substantial delays. Special focus was dedicated to the initial Hopf bifurcation, which destabilizes the fundamental steady state. For further detail, see ^[4,5] and references therein.

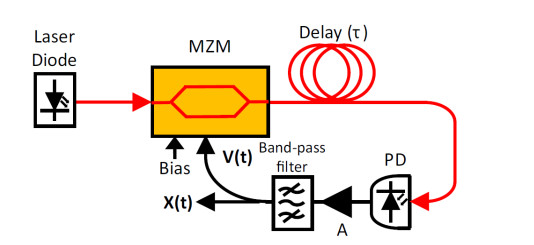
An optoelectronic oscillator (OEO) is a self-contained system with the ability to generate a microwave electromagnetic wave characterized by high spectral purity and minimal electronic noise. The diagram in Figure 1 illustrates the schematic representation of the experimental configuration for an OEO. From ^[4,5], mathematically, OEOs can be characterized by a pair of first-order delay differential equations in the following structure:

$\begin{equation} \left\{ \begin{array}{rllrrrrr} \tau_L \frac{dy(t)}{dt} = &-(1+\frac{\tau_L}{\tau_H})y(t)-x(t)-\beta \cos^2(y(t-\tau)+\phi), &t\in (0, T], &\\ \tau_H\frac{d x(t)}{dt} = &y(t), &t\in (0, T], &\\ y(t) = &\Phi(t), &t\in [-\tau, 0], \end{array}\right. \end{equation}$

(1.1)

Figure 1. Plot of the experimental setup.

DownLoad: Full-Size Img PowerPoint

with an initial condition

$\begin{equation} x(0) = x_0, \end{equation}$

(1.2)

where $\Phi(t)$ is a given function and $x, y$ are unknown functions in which $y$ denotes the normalized output signal that signifies the voltage applied to the modulator. The parameter $\beta$ is dimensionless and characterizes the feedback strength of the loop. $\tau$ represents the overall delay of the feedback signal, and $\phi$ is the bias point of the modulator. Additionally, $\tau_L$ and $\tau_H$ serve as time constants describing the characteristics of the low-pass and high-pass filters, respectively.

System (1.1) is equivalent to the following integrodifferential equation:

$\begin{equation} \begin{array}{rllrrrrr} \tau_L \frac{dy(t)}{dt} = &-x(0)-(1+\frac{\tau_L}{\tau_H})y(t)-\frac{1}{\tau_H} \int_{0}^{t}y(s)ds-\beta \cos^2(y(t-\tau)+\phi), &t\in (0, T], \end{array} \end{equation}$

(1.3)

By using mathematical theorems and changing the appropriate variables of the equation, the Volterra integral equation (VIE) of the delayed type can be written in the following form:

$\begin{equation} \tau_Ly(t) = \hat{g}(t)+ \int_{0}^{t}\hat{k}_1(t, s, y(s))ds+ \int_{-\tau}^{t-\tau}\hat{k}_2(t, s, y(s))ds, \quad t\in I = (0, T], \end{equation}$

(1.4)

where

$\begin{equation} \begin{array}{rllrrrrr} \hat{k}_1(t, s, y(s)) = &-(1+\frac{\tau_L}{\tau_H})y(s)-\frac{1}{\tau_H} \int_{0}^{s}y(z)dz, &\\ \hat{k}_2(t, s, y(s)) = &-\beta \cos^2(y(s)+\phi), &\\ \hat{g}(t) = &-tx_0+\tau_L\Phi(0). \end{array} \end{equation}$

(1.5)

Now, in Eq (1.5), suppose that the low-pass constant is a very small number $\tau_L\rightarrow 0$ , then we have

$\begin{equation} 0 = g(t)+ \int_{0}^{t}k_1(t, s, y(s))ds+ \int_{-\tau}^{t-\tau}k_2(t, s, y(s))ds, \quad t\in I = (0, T], \end{equation}$

(1.6)

where

$\begin{equation} \begin{array}{rllrrrrr} k_1(t, s, y(s)) = &-y(s)-\frac{1}{\tau_H} \int_{0}^{s}y(z)dz, &\\ k_2(t, s, y(s)) = &\hat{k}_2(t, s, y(s)), &\\ g(t) = &-tx_0. \end{array} \end{equation}$

(1.7)

According to the complexities of the analytical solution of this type of equation, the researchers analyzed a number of numerical methods for the approximate solution of this equation. However, since these equations are inherently ill-posed, some numerical methods are not suitable for studying the numerical solutions of this equation. Nevertheless, these equations pose mathematical challenges for analysis, and a significant portion of the current comprehension of their potential solutions primarily stems from thorough computer simulations. This is especially true in optics, where the intricacies of optical and optoelectronic feedback are scrutinized extensively, facilitating systematic comparisons between experimental findings and theoretical predictions. Equations (1.4) and (1.6) are nonlinear, and their kernels $k_i$ and $\hat{k}_i, \; i = 1, 2$ can be linearized by using linearization methods such as Newton's method.

Here, we study an approximate numerical solution of the first-kind linear VIEs with constant delay $\tau > 0$ . More precisely, we consider

$\begin{equation} g(t) = \int_0^{t}k_1(t, s)y(s)ds+\int_0^{t-\tau}{k}_{2}(t, s)y(s)ds, \quad t\in I = (0, T], \end{equation}$

(1.8)

with

$y(t) = \phi(t), \; \; \; t\in[-\tau, 0].$

Also, $g(t)$ is a known function and the kernel functions $k_1(t, s)$ , $k_2(t, s)$ are defined within the domains $D = \{(t, s): 0 \leq s \leq t \leq T\}$ , $D_{\tau} = \{(t, s): -\tau \leq s \leq t-\tau, t \in I\}$ , respectively. The presence of a nonzero delay $t-\tau$ leads to the emergence of the initial discontinuity points ${\xi_{\mu}}$ , which are determined through a recursive process

$\xi_{\mu} = \mu \tau, \; \; \; \mu = 0, 1, \ldots,$

and the initial discontinuity points remain a finite number in any bounded interval $I$ . The solution to the Eq (1.8) exhibits a lack of continuity dependence on the provided functions $k_1, k_2$ , and $g$ . From ^[1], we have smoothing property in solutions of delay VIEs of the second kind, but this is no longer true for solutions of delay VIEs of the first-kind. The jump discontinuity in the solution at $t = 0$ results in a similar discontinuity at the subsequent primary discontinuity point $\xi_{1}$ , and this discontinuity persists at other points. Now, we can summarize the regularity result for the unique solution of the Eq (1.8) as:

Theorem 1.1. ^[1] Let

$1)$ $k_{1}(., .) \in C^1(D), k_{2}(., .)\in C^1(D_{\tau}),$ and $g\in C^{1}(I)$ .

$2)$ For all $t\in I$ , we have $| k_{1}(t, t)|\geq k_0 > 0.$

For any initial function $\phi(t)\in C[-\tau, 0]$ , the Eq (1.8) has a unique solution denoted by $y$ , where $y \in C(\xi_{\mu}, \xi_{\mu+1}]$ for $\mu = 0, \cdots, M$ . This solution $y$ stays bounded at $t = 0$ if, and only if,

$\int_{ -\tau}^{0}k_{2}(0, s)\phi(s)\mathrm{d}s = -g(0).$

The great contributions of recent developments in the conceptually promising and challenging areas of the VIEs are all gathered in the milestone book due to Brunner ^[1]. Without any exaggeration, a great deal of tribute should be paid to Volterra as the founder of VIEs with delays. In other words, some well-formulated models of Volterra functional integral equations, namely, in population dynamics, evolutionary phenomena, and mechanics of continua, date back to Volterra's efforts in his early studies ^[1,6,7,8]. Many authors ^{[1,9,10,11,12,13,14,15,16]} have studied the approximate solutions of delay IEs. For the ill-posed Volterra equation, the efficient local regularization methods preserve the causal structure of the Volterra problem. In ^[17], the author introduced new local regularization method for general finitely smoothing VIEs and investigate convergence of the resulting method. The multistep collocation method was applied to first kind VIEs in ^[18]. Convergence conditions of the proposed multistep method were analyzed and the corresponding convergence order was described. Brunner ^[1,10] analyzed the properties of global convergence and local super-convergence of piecewise polynomial collocation for VIEs with constant delay. First-kind VIEs have ill-posed nature in comparison to the second-kind of integral equations. Also, this type of equation, especially their delay type, arise in some mathematical modeling processes, and as far as we know, there are few works available in the literature on the numerical solution of delay first-kind VIEs in comparision of the second-kind of these equations. Therefore, choosing an appropriate numerical method so that its convergence is guaranteed can be useful. Piecewise polynomial collocation methods can be used for different types of integral equations, but it is very necessary to investigate the convergence of the methods. The novelty of this paper is the investigation of the convergence analysis of the proposed numerical methods with more details. We will show that the choice of collocation points can be effective in the convergence of the method such that incorrect selection of these points can lead to a decrease in the order of convergence or even divergence. It is known that, for second-kind VIEs, the collocation solution converges to the precise solution for every selection of collocation parameters $c_i$ with $0 \leq c_1 < \cdots < c_m \leq 1$ . However, this property no longer holds for first-kind VIEs. It is necessary to distinguish between two scenarios, $c_m = 1$ and $c_m < 1$ , and investigate convergence analysis based on the nontrivial eigenvalues of the obtained matrices.

The following is the paper's outline. In Section 2, we use the polynomial spline collocation method to (1.8). Convergence analysis is given in Section 3. We consider some numerical examples in Section 4.

2. Polynomial spline collocation

For some $M \geq 1$ , assume that $T = \xi_{M}$ , and

$I_{h}: = \bigcup \limits_{\mu = 0}^M I_{h}^{(\mu)}, \qquad I_{h}^{(\mu)}: = \{t_{n}^{(\mu)}\; :\; \xi_{\mu} = t_{0}^{(\mu)} < t_{1}^{(\mu)} < \cdots < t_{N}^{(\mu)} = \xi_{\mu+1}\},$

where $h_{n}^{(\mu)} = t_{n+1}^{(\mu)}-t_{n}^{(\mu)}, \mu = 0, \ldots, M$ ( $M\geq 1$ ). The collocation points are chosen as follows:

$\mathcal{X}_{h}: = \bigcup\limits_{\mu = 0}^{M} \mathcal{X}_{h}^{(\mu)},$

where

$\mathcal{X}_{h}^{(\mu)} = \{t_{n, i}^{(\mu)} = t_{n}^{(\mu)}+c_{i}h_{n}^{(\mu)} : 0 < c_{1} < \cdots < c_{m} \leq 1, \; (0 \leq n \leq N-1)\}.$

After that, the solution obtained through collocation denoted as $u$ for Eq (1.8) is given by:

$\begin{equation} g(t) = \int_0^{t}k_{1}(t, s)u(s)ds+ \int_0^{t-\tau}{k}_{2}(t, s)u(s)ds, \qquad t \in \mathcal{X}_{h}, \end{equation}$

(2.1)

with

$u(t) = \phi(t), \quad t\in[-\tau, 0].$

On each subinterval $\sigma_{n} = (t_{n}^{(\mu)}, t_{n+1}^{(\mu)}]$ , we have

$\begin{equation} u_{h}(t_{n}^{(\mu)}+\rho h_{n}^{(\mu)}) = \sum\limits_{j = 1}^{m}L_{j}(\rho)U_{n, j}^{(\mu)}, \; \; \; U_{n, j}^{(\mu)} = u_{h}(t_{n, j}^{(\mu)}), \end{equation}$

(2.2)

where $L_j(\rho)$ denotes canonical polynomials of Lagrange for the collocation parameters $\{c_j\}$ . Now, let

$\ \pmb U_{l}^{(\eta)} = [U_{l, 1}^{(\eta)}, \ldots , U_{l, m}^{(\eta)}]^T, \qquad \pmb g_{n}^{(\mu)} = [g(t_{n, 1}^{(\mu)}), \ldots , g(t_{n, m}^{(\mu)})]^T,$

$\pmb K_{2, n}^{(0)} = [\int_0^{t_{n, 1}^{(0)}-\tau}{k}_{2}(t_{n, 1}^{(0)}, s)\phi(s)ds, \ldots , \int_0^{t_{n, m}^{(0)}-\tau}{k}_{2}(t_{n, m}^{(0)}, s)\phi(s)ds],$

$\Big(\pmb{\mathcal{K}}_{\alpha, n, \lambda}^{c_{a, b}, (\mu, \kappa)}\Big)_{i, j} = \int_{0}^{c_{a, b}}k_{\alpha}(t_{n, i}^{(\mu)}, t_{\lambda}^{(\kappa)}+\rho h_{\lambda}^{(\kappa)})L_{j}(\rho)\mathrm{d}\rho, \alpha = 1, 2, c_{a, 0} = c_{a}, \; c_{0, b} = 1.$

Inserting (2.2) into (2.1), a linear system is derived for the vector of unknowns $\pmb U_{n}^{(\mu)}\; (n = 0, \ldots, N-1)$ in two different cases:

Case I: For $\mu = 0$ , we have

$\begin{equation} \pmb g_{n}^{(0)} = \sum\limits_{l = 0}^{n-1}h_l^{(0)}\pmb{\mathcal{K}}_{1, n, l}^{c_{0, b}, (0, 0)} \pmb U_{l}^{(0)}+h_n^{(0)}\pmb{\mathcal{K}}_{1, n, n}^{c_{i, 0}, (0, 0)} \pmb U_{n}^{(0)}+\pmb K_{2, n}^{(0)}. \end{equation}$

(2.3)

Case II: For $\mu = 1, 2, \ldots, M$ , we have

$\begin{equation} \begin{array}{rlr} \pmb g_{n}^{(\mu)} = \sum\limits_{\eta = 0}^{\mu-1}\sum\limits_{l = 0}^{N-1}h_l^{(\eta)}\pmb{\mathcal{K}}_{1, n, l}^{c_{0, b}, (\mu, \eta)} \pmb U_{l}^{(\eta)}+ \sum\limits_{l = 0}^{n-1}h_l^{(\mu)}\pmb{\mathcal{K}}_{1, n, l}^{c_{0, b}, (\mu, \mu)} \pmb U_{l}^{(\mu)} +h_n^{(\mu)}\pmb{\mathcal{K}}_{1, n, n}^{c_{i, 0}, (\mu, \mu)}\pmb U_{n}^{(\mu)}\\ + \sum\limits_{\eta = 0}^{\mu-2}\sum\limits_{l = 0}^{N-1}h_l^{(\eta)} \pmb{\mathcal{K}}_{2, n, l}^{c_{0, b}, (\mu, \eta)} \pmb U_{l}^{(\eta)} + \sum\limits_{l = 0}^{n-1}h_l^{(\mu-1)}\pmb{\mathcal{K}}_{2, n, l}^{c_{0, b}, (\mu, \mu-1)} \pmb U_{l}^{(\mu-1)} +h_n^{(\mu-1)}\pmb{\mathcal{K}}_{2, n, n}^{c_{i, 0}, (\mu, \mu-1)}\pmb U_{n}^{(\mu-1)}. \end{array} \end{equation}$

(2.4)

3. Convergence analysis

In this section, using interpolation error, we study convergence analysis of the proposed numerical method.

Let

$\begin{equation} g(t) = (\mathcal{V}y)(t)+(\mathcal{V}_{\tau}y)(t), \end{equation}$

(3.1)

where

$\begin{equation} (\mathcal{V}y)(t) = \int_{0}^{t}k_{1}(t, s)y(s)ds, \end{equation}$

(3.2)

$\begin{equation} (\mathcal{V}_{\tau}y)(t) = \int_{0}^{t-\tau}k_{2}(t, s)y(s)ds. \end{equation}$

(3.3)

Consider the collocation equation as

$\begin{equation} g(t) = (\mathcal{V}u_{h})(t)+(\mathcal{V}_{\tau}u_{h})(t). \end{equation}$

(3.4)

Now, for $n = 0, \ldots, N-1$ and $\mu = 0, \ldots, M$ , we set $h = \max h_n^{(\mu)}$ . Using Peano's theorem on the representation of the interpolation (Theorem 1.8.1 from ^[1]), we write

$\begin{equation} y(t_{n}^{(\mu)}+vh) = \sum\limits_{j = 1}^{m}L_{j}(v)Y_{n, j}^{(\mu)}+h^{m}R_{m, n}^{(\mu)}(v), \; \; Y_{n, j}^{(\mu)} = y(t_{n, j}^{(\mu)}), \quad v\in [0, 1]. \end{equation}$

(3.5)

Here, we have

$\begin{equation*} R_{m, n}^{(\mu)}(v): = \int_{0}^{1}k_{m}(v, z)y^{(m)}(t_{n}^{(\mu)}+zh)dz, \end{equation*}$

and

$k_{p}(s, x) = \dfrac{1}{(p-1)!}\{(s-x)_{+}^{p-1}-\sum\limits_{k = 1}^{m}L_{k}(s)(c_{k}-x)_{+}^{p-1}\}, \quad x\in [0, 1].$

Therefore, it follows that

$\begin{equation*} u_{h}(t_{n}^{(\mu)}+vh) = \sum\limits_{j = 1}^{m}L_{j}(v)U_{n, j}^{(\mu)}, \quad v\in(0, 1]. \end{equation*}$

The collocation error, denoted as $e_{h} = y-u_{h}$ , is governed by the equations:

$\begin{equation} (\mathcal{V}e_{h})(t_{n, i}^{(\mu)})+(\mathcal{V}_{\tau}e_{h})(t_{n, i}^{(\mu)}) = 0, \quad i = 1, 2, \ldots, m, \quad n = 0, 1, \ldots , N-1, \end{equation}$

(3.6)

and has the local expression

$\begin{equation} e_{h}(t_{n}^{(\mu)}+vh) = \sum\limits_{j = 1}^{m}L_{j}(v)\varepsilon_{n, j}^{(\mu)}+h^{m}R_{m, n}^{(\mu)}(v), \; \; \varepsilon_{n, j}^{(\mu)} = Y_{n, j}^{(\mu)}-U_{n, j}^{(\mu)}, \quad v\in(0, 1], \end{equation}$

(3.7)

which satisfies the Eq (3.6).

In Eq (3.6), for $\mu = 0, 1, \ldots, M$ , using (3.7), we have

$\begin{equation} \begin{array}{lr} (\mathcal{V}e_{h})(t_{n, i}^{(\mu)}) = h \sum\limits_{v = 0}^{\mu-1}\sum\limits_{l = 0}^{N-1}\int_{0}^{1}k_{1}(t_{n, i}^{(\mu)}, t_{l}^{(v)}+zh) e_{h}(t_{l}^{(v)}+zh)dz\\ \qquad \qquad \qquad +h \sum\limits_{l = 0}^{n-1}\int_{0}^{1}k_{1}(t_{n, i}^{(\mu)}, t_{l}^{(\mu)}+zh)e_{h}(t_{l}^{(\mu)}+zh)dz\\ \qquad \qquad \qquad + h\int_{0}^{c_{i}}k_{1}(t_{n, i}^{(\mu)}, t_{n}^{(\mu)}+zh)e_{h}(t_{n}^{(\mu)}+zh)dz, \end{array} \end{equation}$

(3.8)

and

$\begin{equation} (\mathcal{V}_{\tau}e_{h})(t_{n, i}^{(\mu)}) = \left\{ \begin{array}{lr} 0, \qquad\qquad \qquad \; \; \; \; \; \; \; \; \; \; \; \; \; \; \; \; \; \; \; \; \; \; \; \; \; \; \; \; \; \; \; \; \; \; \; \mu = 0, \\ \\ h \sum\limits_{v = 0}^{\mu-2}\sum\limits_{l = 0}^{N-1}\int_{0}^{1}k_{2}(t_{n, i}^{(\mu)}, t_{l}^{(v)}+zh) e_{h}(t_{l}^{(v)}+zh)dz\\ +h \sum\limits_{l = 0}^{n-1}\int_{0}^{1}k_{2}(t_{n, i}^{(\mu)}, t_{l}^{(\mu-1)}+zh)e_{h}(t_{l}^{(\mu-1)}+zh)dz\\ + h\int_{0}^{c_{i}}k_{2}(t_{n, i}^{(\mu)}, t_{n}^{(\mu-1)}+zh)e_{h}(t_{n}^{(\mu-1)}+zh)dz, \quad \mu = 1, \cdots, M. \end{array}\right. \end{equation}$

(3.9)

Now, for $\mu = 0$ on the first macro-interval $(\xi_{0}, \xi_{1}]$ , considering (3.9) and using Eq (3.6), for $n = 0, 1, \ldots, N-1$ and $i = 1, \ldots, m$ , we obtain:

$(\mathcal{V}e_{h})(t_{n, i}^{(0)}) = 0.$

Then, by using the convergence results of the spline collocation method for the classical first kind VIEs from Theorem 2.4.2 in the ^[1], we have

$||e_h||_{\infty}\leq \left \{ \begin{array}{cc}\mathcal{C}h^{m}, \; \; \; & \quad if \quad \lambda\in [-1, 1), \\ \mathcal{C} h^{m-1}, &if \quad\quad \lambda = 1, \end{array}\right.$

in which $\mathcal{C}$ is a positive constant and

$\lambda = (-1)^m\prod\limits_{i = 1}^m\frac{1-c_i}{c_i}.$

For $\mu\geq 1$ , by (3.6), to differentiate the (continuous) error equation, we resort to its discrete analogous, which is

$\begin{equation} \dfrac{1}{h}[(\mathcal{V}e_{h})(t_{n, i}^{(\mu)})-(\mathcal{V}e_{h})(t_{n-1, m}^{(\mu)})]+\dfrac{1}{h} [(\mathcal{V}_{\tau}e_{h})(t_{n, i}^{(\mu)})-(\mathcal{V}_{\tau}e_{h})(t_{n-1, m}^{(\mu)})] = 0, \quad i = 1, 2, \ldots , m. \end{equation}$

(3.10)

By using (3.8) and (3.9), we have

$\begin{equation} \begin{array}{lr} \int_{0}^{c_{i}}k_{1}(t_{n, i}^{(\mu)}, t_{n}^{(\mu)}+zh)e_{h}(t_{n}^{(\mu)}+zh)dz = \int_{0}^{c_{m}}k_{1}(t_{n-1, m}^{(\mu)}, t_{n-1}^{(\mu)}+zh)e_{h}(t_{n-1}^{(\mu)}+zh)dz\\ - \sum\limits_{l = 0}^{n-1}\int_{0}^{1}k_{1}(t_{n, i}^{(\mu)}, t_{l}^{(\mu)}+zh)e_{h}(t_{l}^{(\mu)}+zh)dz+ \sum\limits_{l = 0}^{n-2}\int_{0}^{1}k_{1}(t_{n-1, m}^{(\mu)}, t_{l}^{(\mu)}+zh)e_{h}(t_{l}^{(\mu)}+zh)dz\\ - \sum\limits_{v = 0}^{\mu-1}\sum\limits_{l = 0}^{N-1}\int_{0}^{1}k_{1}(t_{n, i}^{(\mu)}, t_{l}^{(v)}+zh) e_{h}(t_{l}^{(v)}+zh)dz+ \sum\limits_{v = 0}^{\mu-1}\sum\limits_{l = 0}^{N-1}\int_{0}^{1}k_{1}(t_{n-1, m}^{(\mu)}, t_{l}^{(v)}+zh) e_{h}(t_{l}^{(v)}+zh)dz\\ - \int_{0}^{c_{i}}k_{2}(t_{n, i}^{(\mu)}, t_{n}^{(\mu-1)}+zh)e_{h}(t_{n}^{(\mu-1)}+zh)dz+\int_{0}^{c_{m}}k_{2}(t_{n-1, m}^{(\mu)}, t_{n-1}^{(\mu-1)}+zh)e_{h}(t_{n-1}^{(\mu-1)}+zh)dz\\ - \sum\limits_{l = 0}^{n-1}\int_{0}^{1}k_{2}(t_{n, i}^{(\mu)}, t_{l}^{(\mu-1)}+zh)e_{h}(t_{l}^{(\mu-1)}+zh)dz+ \sum\limits_{l = 0}^{n-2}\int_{0}^{1}k_{2}(t_{n-1, m}^{(\mu)}, t_{l}^{(\mu-1)}+zh)e_{h}(t_{l}^{(\mu-1)}+zh)dz\\ - \sum\limits_{v = 0}^{\mu-2}\sum\limits_{l = 0}^{N-1}\int_{0}^{1}k_{2}(t_{n, i}^{(\mu)}, t_{l}^{(v)}+zh) e_{h}(t_{l}^{(v)}+zh)dz+ \sum\limits_{v = 0}^{\mu-2}\sum\limits_{l = 0}^{N-1}\int_{0}^{1}k_{2}(t_{n-1, m}^{(\mu)}, t_{l}^{(v)}+zh) e_{h}(t_{l}^{(v)}+zh)dz. \end{array} \end{equation}$

(3.11)

Because of the supposed regularity of the kernels $k_{p}(t, s), p = 1, 2$ , we have

$\begin{equation} \begin{array}{lr} k_{p}(t_{n, i}^{(\mu)}, t_{l}^{(q)}+sh)-k_{p}(t_{n-1, m}^{(\mu)}, t_{l}^{(q)}+sh)\\ \quad = c_{i}hk_{p, t}(t_n^{(\mu)}, t_{l}^{(q)}+sh)+(1-c_{m})hk_{p, t}(t_n^{(\mu)}, t_{l}^{(q)}+sh)+\mathcal{O}(h), \quad p = 1, 2, \end{array} \end{equation}$

(3.12)

where $k_{p, t}(, ) = \dfrac{\partial k_{p}}{\partial t}$ and the first unspecified arguments in the partial derivatives of $k_{p}, p = 1, 2$ , are those that arise from Taylor's remainder terms. Now, without sacrificing generality, we consider two cases:

Case I): $c_{m} = 1$ .

Considering (3.12), for $i = 1, \ldots, m$ , Eq (3.11) reduces to

$\begin{equation} \begin{array}{rlllllllr} \int_{0}^{c_{i}}k_{1}(t_{n, i}^{(\mu)}, t_{n}^{(\mu)}+zh)e_{h}(t_{n}^{(\mu)}+zh)dz = &- \int_{0}^{c_{i}}k_{2}(t_{n, i}^{(\mu)}, t_{n}^{(\mu-1)}+zh)e_{h}(t_{n}^{(\mu-1)}+zh)dz\\ &-c_ih \sum\limits_{l = 0}^{n-1}\int_{0}^{1}k_{1, t}(t_{n}^{(\mu)}, t_{l}^{(\mu)}+zh)e_{h}(t_{l}^{(\mu)}+zh)dz\\ & -c_ih \sum\limits_{v = 0}^{\mu-1}\sum\limits_{l = 0}^{N-1}\int_{0}^{1}k_{1, t}(t_{n}^{(\mu)}, t_{l}^{(v)}+zh) e_{h}(t_{l}^{(v)}+zh)dz\\ & -c_ih \sum\limits_{l = 0}^{n-1}\int_{0}^{1}k_{2, t}(t_{n}^{(\mu)}, t_{l}^{(\mu-1)}+zh)e_{h}(t_{l}^{(\mu-1)}+zh)dz\\ & -c_ih \sum\limits_{v = 0}^{\mu-2}\sum\limits_{l = 0}^{N-1}\int_{0}^{1}k_{2, t}(t_{n}^{(\mu)}, t_{l}^{(v)}+zh) e_{h}(t_{l}^{(v)}+zh)dz. \end{array} \end{equation}$

(3.13)

Using (3.7), we arrive at

$\begin{equation} \begin{array}{rllllllrlr} \pmb{\mathcal{K}}_{1, n, n}^{c_{i, 0}, (\mu, \mu)}\pmb \varepsilon_{n}^{(\mu)} = & -h \sum\limits_{l = 0}^{n-1}\pmb{C}\pmb{\mathcal{K}}_{\{1, t\}, n, l}^{c_{0, b}, (\mu, \mu)} \pmb \varepsilon_{l}^{(\mu)} -h \sum\limits_{\eta = 0}^{\mu-1}\sum\limits_{l = 0}^{N-1} \pmb{C}\pmb{\mathcal{K}}_{\{1, t\}, n, l}^{c_{0, b}, (\mu, \eta)} \pmb \epsilon_{l}^{(\eta)}-\pmb{\mathcal{K}}_{2, n, n}^{c_{i, 0}, (\mu, \mu-1)}\pmb \varepsilon_{n}^{(\mu-1)}\\ &-h \sum\limits_{l = 0}^{n-1}\pmb{C}\pmb{\mathcal{K}}_{\{2, t\}, n, l}^{c_{0, b}, (\mu, \mu-1)} \pmb \varepsilon_{l}^{(\mu-1)} -h \sum\limits_{\eta = 0}^{\mu-2}\sum\limits_{l = 0}^{N-1}\pmb{C}\pmb{\mathcal{K}}_{\{2, t\}, n, l}^{c_{0, b}, (\mu, \eta)} \pmb \varepsilon_{l}^{(\eta)} +\pmb {O}(h^m), \end{array} \end{equation}$

(3.14)

where $\pmb{C} = \mbox{diag}\big(c_1, \ldots, c_m\big)$ , $\pmb \varepsilon_{l}^{(\alpha)} = [\varepsilon_{l, 1}^{(\alpha)}, \ldots, \varepsilon_{l, m}^{(\alpha)}]^T$ , and the meaning of the matrices $\pmb{\mathcal{K}}_{1, n, n}^{c_{i, 0}, (\mu, \mu)}, \ldots, \pmb{\mathcal{K}}_{\{2, t\}, n, l}^{c_{0, b}, (\mu, \eta)}$ are clear from Section 2. Since $|k_1(t, t)| \geq k_0 > 0$ for all $t\in I$ , if $h$ is small enough, the matrix's inverse on the left side exists and is bounded. It follows from Gronwall's inequality and upper bounds of $\Vert\pmb \varepsilon_{n}^{(\alpha)}\Vert (\alpha = 0, \ldots, \mu - 1)$ in the previous steps that

$||e_h||_{\infty}\leq \mathcal{C}h^{m}.$

Case II): $c_{m} < 1$ .

To express the main ideas without resorting to complex notation, we can presume that $k_{p}(t, s) = 1$ or we can employ the Taylor series expansion $k_{p}$ as:

$\begin{equation} k_{p}(t_{n, i}^{(\mu)}, t_{l}^{(q)}+sh) = k_{p}(t_{n}^{(\mu)}, t_{l}^{(q)})+\pmb {O}(h), \end{equation}$

(3.15)

The error Eq (3.11) can then be written as:

$\begin{equation} \begin{array}{ll} {\sum\limits_{j = 1}^{m}(\int_{0}^{c_{i}}}L_{j}(s)ds) \varepsilon_{n, j}^{(\mu)} = &- {\sum\limits_{j = 1}^{m}(\int_{c_m}^{1}}L_{j}(s)ds)\varepsilon_{n-1, j}^{(\mu)}- {\sum\limits_{j = 1}^{m}(\int_{0}^{c_{i}}}L_{j}(s)ds) \varepsilon_{n, j}^{(\mu-1)}\\ &- {\sum\limits_{j = 1}^{m}(\int_{c_m}^{1}}L_{j}(s)ds)\varepsilon_{n-1, j}^{(\mu-1)}+\pmb {O}(h^m). \end{array} \end{equation}$

(3.16)

Now, in Eq (3.16), we set $m\times m$ matrices $\pmb P$ and $\pmb Q$ as:

$\pmb P = \begin{bmatrix} \int_{0}^{c_{1}}L_{1}(s)ds & \cdots & \int_{0}^{c_{1}}L_{m}(s)ds\\ \\ \int_{0}^{c_{2}}L_{1}(s)ds & \cdots & \int_{0}^{c_{2}}L_{m}(s)ds\\ \\ \vdots & \vdots & \vdots\\ \\ \int_{0}^{c_{m}}L_{1}(s)ds & \cdots & \int_{0}^{c_{m}}L_{m}(s)ds \end{bmatrix}, \qquad \pmb Q = \begin{bmatrix} \int_{c_{m}}^{1}L_{1}(s)ds & \cdots & \int_{c_{m}}^{1}L_{m}(s)ds\\ \\ \int_{c_{m}}^{1}L_{1}(s)ds & \cdots & \int_{c_{m}}^{1}L_{m}(s)ds\\ \\ \vdots & \vdots & \vdots\\ \\ \int_{c_{m}}^{1}L_{1}(s)ds & \cdots & \int_{c_{m}}^{1}L_{m}(s)ds \end{bmatrix},$

then, we get

$\begin{align*} \pmb P\pmb \varepsilon_{n}^{(\mu)} = -\pmb Q \varepsilon_{n-1}^{(\mu)}-\pmb P\pmb \varepsilon_{n}^{(\mu-1)}-\pmb Q \varepsilon_{n-1}^{(\mu-1)}+\pmb O(h^m), \end{align*}$

where $\pmb P$ is not singular and $\pmb Q$ has rank one. We have the following difference equations:

$\begin{equation} \pmb \varepsilon_{n}^{(\mu)} = -\pmb \Omega \varepsilon_{n-1}^{(\mu)}-\pmb \varepsilon_{n}^{(\mu-1)}-\pmb \Omega \varepsilon_{n-1}^{(\mu-1)}+\pmb O(h^m), \end{equation}$

(3.17)

where $\pmb \Omega = \pmb P^{-1}\pmb Q$ .

Now, we consider the following lemma:

Lemma 3.1. Assume that $0 < c_1 < \cdots < c_m < 1$ . Subsequently, the nontrivial eigenvalue $\lambda$ of the rank-one matrix $\pmb \Omega$ is:

$\lambda = (-1)^m\prod\limits_{i = 1}^m\frac{1-c_i}{c_i}.$

Proof. See Lemma 2.4.3 of Reference ^[1] (pp. 126). □

According to the basic principles outlined in the difference equations theory in ^[19], the solutions to the system of first-order difference equations (3.17) exhibit uniform boundedness if, and only if, $|\lambda|\leq1$ . Note that $\pmb \Omega$ is a diagonalizable matrix; therefore, a matrix exists, denoted as $\Upsilon$ , which $\pmb \Omega = \Upsilon \Psi \Upsilon^{-1}$ with $\Psi = \text{diag}(\lambda, 0, \cdots, 0)$ . Multiplying (3.17) by $\Upsilon^{-1}$ and defining $\pmb Z_n^{(\mu)} = \Upsilon^{-1}\pmb \varepsilon_n^{(\mu)}$ yields:

$\begin{equation} \pmb Z_{n}^{(\mu)} = -\Psi \pmb Z_{n-1}^{(\mu)}- \pmb Z_{n}^{(\mu-1)}-\Psi \pmb Z_{n-1}^{(\mu-1)}+\pmb O(h^m). \end{equation}$

(3.18)

Using Lemma 6 from ^[20], Lemma 2.4.4 from ^[1], and the upper bounds of $\Vert\pmb \varepsilon_{n}^{(\alpha)}\Vert (\alpha = 0, \ldots, \mu - 1)$ in the previous steps, if $\lambda\in[-1, 1)$ , then

$\begin{equation} \|\pmb \varepsilon_n^{(\mu)}\|_1\leq \mathcal{C}h^{m}. \end{equation}$

(3.19)

If $\lambda = 1$ , then,

$\begin{equation} \|\pmb \varepsilon_n^{(\mu)}\|_1\leq \mathcal{C}h^{m-1}. \end{equation}$

(3.20)

Now, the following theorem summarizes our findings.

Theorem 3.1. Assume that for $d \geq m$ , the given functions in (1.8) satisfy:

$k_{1}(., .) \in C^{d+1}(D), k_{2}(., .)\in C^{d+1}1(D_{\tau}), g\in C^{d+1}(I), \phi(t)\in C^d[-\tau, 0],$

and for all $t\in I$ , $| k_{1}(t, t)|\geq k_0 > 0.$ Also, let $u_h\in S_{m-1}^{-1}(\Pi_N)$ be the collocation approximation of the solution $y$ in the Eq (1.8). If $c_m = 1$ , the approximate solution $u_h$ converges to $y$ and the following order of convergence holds:

$||y-u_h||_{\infty}\leq \mathcal{C}h^{m}.$

If $c_m < 1$ , the collocation approximation $u_h$ converges to $y$ if, and only if,

$-1\leq\lambda = (-1)^m\prod\limits_{i = 1}^m\frac{1-c_i}{c_i}\leq 1.$

Furthermore, the following order of convergence holds:

$||y-u_h||_{\infty}\leq \left \{ \begin{array}{cc}\mathcal{C}h^{m}, \; \; \; & \quad if \quad \lambda\in [-1, 1), \\ \mathcal{C}h^{m-1}, &if \quad\quad \lambda = 1, \end{array}\right.$

as $h\rightarrow 0$ with $Nh\leq \text{const}.$

4. Numerical examples

We will give three examples in this section to demonstrate the convergence results. Mathematica $^\circledR$ software is used to perform all calculations.

Example 1. Consider the first-kind VIEs with constant delay as:

$\begin{equation} \left\{ \begin{array}{rllllcc} f(t) = & \int_0^{t}e^{s-t}y(s)ds+ \int_0^{t-\frac{1}{4}}t\sin (s ) y(s)ds, &&t\in (0, 1], \\ y(t) = &\cos t+2, &&t\in[-\frac{1}{4}, 0], \end{array}\right. \end{equation}$

(4.1)

where $f(t)$ such that the exact solution is: $y(t) = \cos (t)+2.$

For the numerical solution of (4.1), we choose $m = 2, 3$ . For $m = 2$ , we utilize the Gauss collocation parameters (i.e., the zeros of $P_m(2s - 1)$ in which $P_m$ implies the Legendre polynomial of degree $m$ ), the Radau II collocation parameters (i.e., the roots of $P_{m-1}(2s - 1)- P_{m}(2s - 1)$ ), and four sets of random collocation parameters, $c_1 = \frac{1}{2}, c_2 = 1$ ; $c_1 = \frac{1}{4}, c_2 = \frac{5}{6};$ $c_1 = \frac{1}{3}, c_2 = \frac{2}{3}$ ; $c_1 = \frac{1}{6}, c_2 = \frac{1}{2}$ , respectively. We use the Gauss collocation parameters for $m = 3$ , the Radau II collocation parameters, and four sets of random collocation parameters, $c_1 = \frac{1}{2}, c_2 = \frac{2}{3}, c_3 = 1$ ; $c_1 = \frac{1}{3}, c_2 = \frac{1}{2}, c_3 = \frac{2}{3}$ ; $c_1 = \frac{1}{2}, c_2 = \frac{2}{3}, c_3 = \frac{8}{9}$ ; $c_1 = \frac{1}{9}, c_2 = \frac{1}{3}, c_3 = \frac{1}{2}$ , respectively.

– show the maximum errors and the orders of convergence for various values of $m$ and $N$ at grid points. Also, – show the orders of convergence from the maximum errors at the grid points, which confirm the theoretical results of Theorem 3.1. Note that the collocation parameter $(\frac{1}{9}, \frac{1}{3}, \frac{1}{2})$ in the Table 2, does not meet the following condition:

$-1\leq (-1)^m\prod\limits_{i = 1}^m\frac{1-c_i}{c_i}\leq 1,$

Table 1. Orders of convergence for

$m = 2$ in Example 1.

$N$	Gauss Radau II	$\quad(\frac{1}{2}, 1)\qquad\quad(\frac{1}{4}, \frac{5}{6})$	$\quad(\frac{1}{3}, \frac{2}{3})$
$16$	$0.732\qquad\qquad 1.91$	$\quad1.88\qquad\qquad 1.41$	$\quad0.610$
$32$	$0.891\qquad\qquad 1.96$	$\quad1.95\qquad\qquad 1.71$	$\quad0.846$

| Show Table

DownLoad: CSV

Table 2. Maximum errors

$||y-u||_{\infty}$ for

$m = 3$ in Example 1.

$N$	Gauss Radau II	$(\frac{1}{2}, \frac{2}{3}, 1)\qquad(\frac{1}{3}, \frac{1}{2}, \frac{2}{3})$	$(\frac{1}{2}, \frac{2}{3}, \frac{8}{9})\qquad(\frac{1}{9}, \frac{1}{3}, \frac{1}{2})$
$8$	$2.39e-5\quad 3.18e-5$	$1.67e-5\qquad 5.47e-6$	$1.72e-5\qquad9.33e-4$
$16$	$2.70e-6\quad 4.89e-6$	$2.65e-6\qquad 3.31e-7$	$2.81e-6\qquad1.17e-2$
$32$	$3.21e-7\quad 6.74e-7$	$3.70e-7\qquad 3.77e-8$	$3.95e-7\qquad4.58e+1$

| Show Table

DownLoad: CSV

Table 3. Orders of convergence for

$m = 3$ in Example 1.

$N$	Gauss Radau II	$(\frac{1}{2}, \frac{2}{3}, 1)\qquad(\frac{1}{3}, \frac{1}{2}, \frac{2}{3})$	$(\frac{1}{2}, \frac{2}{3}, \frac{8}{9})$
$16$	$3.14\qquad\qquad 2.70$	$\quad2.65\qquad\qquad 3.85$	$\quad2.61$
$32$	$3.07\qquad\qquad 2.85$	$\quad2.84\qquad\qquad 3.13$	$\quad2.83$

| Show Table

DownLoad: CSV

Figure 2. Graph illustrating the convergence rate in Example 1.

DownLoad: Full-Size Img PowerPoint

Figure 3. Graph illustrating the convergence rate in Example 1.

DownLoad: Full-Size Img PowerPoint

Figure 4. Graph illustrating the convergence rate in Example 2.

DownLoad: Full-Size Img PowerPoint

then, the convergence of the proposed collocation method does not hold.

Example 2. Consider the first-kind VIEs with constant delay as:

$\begin{equation} -\frac{3}{8}-t = \int_0^{t}y(s)ds+ \int_0^{t-\frac{1}{2}}(t+ s+1) y(s)ds, \; \; t\in (0, 1], \\\\ \end{equation}$

(4.2)

where the exact solution is a discontinuous function as follows:

$y(t) = \left\{ \begin{array}{lll} 1, & \frac{-1}{2}\leq t\leq 0, \\\\ -1-3t, & 0 < t\leq \frac{1}{2}, \\\\ \frac{1}{8}(-11+60t^2), & \frac{1}{2} < t\leq 1. \end{array}\right.$

The maximum errors and the orders of convergence have been reported for various values of $N$ and $m = 2$ in Tables 4 and 5.

Table 4. Maximum errors

$||y-u||_{\infty}$ for

$m = 2$ in Example 2.

$N$	Gauss Radau II	$\quad(\frac{1}{2}, 1)\qquad\quad(\frac{1}{4}, \frac{5}{6})$	$\quad(\frac{1}{3}, \frac{2}{3})$
$8$	$7.97e-2\quad 6.01e-3$	$4.88e-3\qquad 2.32e-2$	$8.02e-2$
$16$	$3.94e-2\quad 1.62e-3$	$1.22e-3\qquad 5.89e-3$	$3.96e-2$
$32$	$1.96e-2\quad 4.06e-4$	$3.05e-4\qquad 1.47e-3$	$1.96e-2$

| Show Table

DownLoad: CSV

Table 5. Orders of convergence for

$m = 2$ in Example 2.

$N$	Gauss Radau II	$\quad(\frac{1}{2}, 1)\qquad\quad(\frac{1}{4}, \frac{5}{6})$	$\quad(\frac{1}{3}, \frac{2}{3})$
$16$	$1.01\qquad\qquad 2.00$	$\quad 2.00\qquad\qquad 1.97$	$\quad 1.01$
$32$	$1.00\qquad\qquad 2.00$	$\quad 2.00\qquad\qquad 1.99$	$\quad 1.00$

| Show Table

DownLoad: CSV

Example 3. As an applied test problem, consider the Eq (1.3) with $\phi = \frac{\pi}{2}, \; \tau_L = 0, \; \tau_H = 1, \; \tau = 1, \; \beta = 0.2, \; x(0) = 0$ , and $\Phi(t) = t - 0.2\sin^2 (-1.1694)$ . The maximum errors for various values of $m$ and $N$ at grid points, are listed in Table 6.

Table 6. Maximum errors

$||y-u||_{\infty}$ for

$m = 2, 3$ in Example 3.

$N$	$\quad m=2, \quad(\frac{1}{2}, 1)$	$\qquad m=3, \quad(\frac{1}{2}, \frac{2}{3}, 1)$
$8$	$\qquad1.17e-4$	$\qquad \qquad4.11e-6$
$16$	$\qquad 3.09e-5$	$\qquad\qquad 5.34e-7$
$32$	$\qquad7.92e-6$	$\qquad \qquad 6.80e-8$

| Show Table

DownLoad: CSV

5. Conclusions

Convergence analysis of the piecewise polynomial collocation method for the first-kind delay VIEs was investigated. We showed that the choice of collocation points $c_i$ can be effective in the convergence of the method such that incorrect selection of these points can lead to a decrease in the order of convergence or even divergence. Also, some examples were considered so that their solutions had a different degree of smoothness to demonstrate the effectiveness of the proposed numerical method. As for our future study, we will analyze approximate methods to the numerical solution of the delay weakly singular integral-algebraic equations (IAEs) with nonvanishing delay.

Author contributions

Mr Hassanein wrote some part of the text, prepared the results, and did the formal analysis. Dr Pishbin wrote the code in Mathematica software, completed the validation process, and reviewed and edited the contents. Dr Darania wrote part of the text about the convergence analysis of the method and edited the contents. All authors discussed the results and revised the draft. All authors have read and approved the final version of the manuscript for publication.

Use of AI tools declaration

The authors declare they have not used Artificial Intelligence (AI) tools in the creation of this article.

Acknowledgments

The authors gratefully acknowledge the anonymous referees for their careful reading of the manuscript and constructive comments.

Conflict of interest

All authors declare that they have no conflicts of interest.

References

[1]	H. Brunner, Collocation methods for Volterra integral and related functional equations, Cambridge: Cambridge University Press, 2004. https://doi.org/10.1017/CBO9780511543234
[2]	K. Ikeda, Multiple-valued stationary state and its instability of the transmitted light by a ring cavity system, Opt. Commun., 30 (1979), 257–261. https://doi.org/10.1016/0030-4018(79)90090-7 doi: 10.1016/0030-4018(79)90090-7
[3]	K. Ikeda, H. Daido, O. Akimoto, Optical turbulence: Chaotic behavior of transmitted light from a ring cavity, Phys. Rev. Lett., 45 (1980), 709. https://doi.org/10.1103/PhysRevLett.45.709 doi: 10.1103/PhysRevLett.45.709
[4]	M. Peil, M. Jacquot, Y. K. Chembo, L. Larger, T. Erneux, Routes to chaos and multiple time scale dynamics in broadband bandpass nonlinear delay electro-optic oscillators, Phys. Rev. E., 79 (2009), 026208. https://doi.org/10.1103/PhysRevE.79.026208 doi: 10.1103/PhysRevE.79.026208
[5]	L. Weicker, G. Friart, T. Erneux, Two distinct bifurcation routes for delayed optoelectronic oscillators, Phys. Rev. E., 96 (2017), 032206. https://doi.org/10.1103/PhysRevE.96.032206 doi: 10.1103/PhysRevE.96.032206
[6]	J. Belair, Population models with state-dependent delays, In: Mathematical population dynamics, New York: Marcel Dekker, 1991,165–176. https://doi.org/10.1201/9781003072706-13
[7]	K. L. Cooke, An epidemic equation with immigration, Math. Biosci., 29 (1976), 135–158. https://doi.org/10.1016/0025-5564(76)90033-X doi: 10.1016/0025-5564(76)90033-X
[8]	H. W. Hethcote, P. van den Driesschew, Two SIS epidemiologic models with delays, J. Math. Biol., 40 (2000), 3–26. https://doi.org/10.1007/s002850050003 doi: 10.1007/s002850050003
[9]	A. Bellour, M. Bousselsal, A Taylor collocation method for solving delay integral equations, Numer. Algor., 65 (2014), 843–857. https://doi.org/10.1007/s11075-013-9717-8 doi: 10.1007/s11075-013-9717-8
[10]	H. Brunner, Iterated collocation methods for Volterra integral equations with delay arguments, Math. Comp., 62 (1994), 581–599. https://doi.org/10.2307/2153525 doi: 10.2307/2153525
[11]	H. Brunner, Y. Yatsenko, Spline collocation methods for nonlinear Volterra integral equations with unknown delay, J. Comput. Appl. Math., 71 (1996), 67–81. https://doi.org/10.1016/0377-0427(95)00228-6 doi: 10.1016/0377-0427(95)00228-6
[12]	F. Calio, E. Marchetti, R. Pavani, About the deficient spline collocation method for particular differential and integral equations with delay, Rend. Sem. Mat. Univ. Pol. Torino, 61 (2003), 287–300.
[13]	I. Ali, H. Brunner, T. Tang, Spectral methods for pantograph-type differential and integral equations with multiple delays, Front. Math. China, 4 (2009), 49–61. https://doi.org/10.1007/s11464-009-0010-z doi: 10.1007/s11464-009-0010-z
[14]	V. Horvat, On collocation methods for Volterra integral equations with delay arguments, Math. Commun., 4 (1999), 93–109.
[15]	Q. Y. Hu, Multilevel correction for discrete collocation solutions of Volterra integral equations with delay arguments, Appl. Numer. Math., 31 (1999), 159–171. https://doi.org/10.1016/S0168-9274(98)00127-5 doi: 10.1016/S0168-9274(98)00127-5
[16]	M. Khasi, F. Ghoreishi, M. Hadizadeh, Numerical analysis of a high order method for state-dependent delay integral equations, Numer. Algor., 66 (2014), 177–201. https://doi.org/10.1007/s11075-013-9729-4 doi: 10.1007/s11075-013-9729-4
[17]	P. K. Lamm, Full convergence of sequential local regularization methods for Volterra inverse problems, Inverse Probl., 21 (2005), 785. https://doi.org/10.1088/0266-5611/21/3/001 doi: 10.1088/0266-5611/21/3/001
[18]	T. T. Zhang, H. Liang, Multistep collocation approximations to solutions of first-kind Volterra integral equations, Appl. Numer. Math., 130 (2018), 171–183. https://doi.org/10.1016/j.apnum.2018.04.005 doi: 10.1016/j.apnum.2018.04.005
[19]	S. N. Elaydi, An introduction to difference equations, New York: Springer, 2005. https://doi.org/10.1007/0-387-27602-5
[20]	E. Hairer, C. Lubich, S. P. Nørset, Order of convergence of one-step methods for Volterra integral equations of the second kind, SIAM J. Numer. Anal., 20 (1983), 569–579. https://doi.org/10.1137/0720037 doi: 10.1137/0720037

Reader Comments

Your name:*

Email:*
© 2024 the Author(s), licensee AIMS Press. This is an open access article distributed under the terms of the Creative Commons Attribution License (http://creativecommons.org/licenses/by/4.0)

通讯作者: 陈斌, bchen63@163.com

1.
沈阳化工大学材料科学与工程学院沈阳 110142

AIMS Mathematics

1.8 3.4

Metrics

Article views(916) PDF downloads(37) Cited by(0)

Preview PDF

Download XML

Export Citation

Article outline

Show full outline

Figures and Tables

Figures(4) / Tables(6)

AIMS Mathematics

Study of numerical treatment of functional first-kind Volterra integral equations

Related Papers:

Abstract

1. Introduction

2. Polynomial spline collocation

3. Convergence analysis

4. Numerical examples

5. Conclusions

Author contributions

Use of AI tools declaration

Acknowledgments

Conflict of interest

References

Reader Comments

通讯作者: 陈斌, bchen63@163.com

Metrics

Figures and Tables

Other Articles By Authors

Catalog

AIMS Mathematics

Study of numerical treatment of functional first-kind Volterra integral equations

Related Papers:

Abstract

1. Introduction

2. Polynomial spline collocation

3. Convergence analysis

4. Numerical examples

5. Conclusions

Author contributions

Use of AI tools declaration

Acknowledgments

Conflict of interest

References

Reader Comments

通讯作者: 陈斌, bchen63@163.com

Metrics

Figures and Tables

Other Articles By Authors

Related pages

Tools

Export File

Citation

Format

Content

Catalog