Coupled systems of nonlinear sequential proportional Hilfer-type fractional differential equations with multi-point boundary conditions

1.   Introduction

Dynamical modeling systems and their computational analysis have been the interest of researchers in science and engineering. Recently, fractional operators have been receiving a lot of attention for their ability to generalize the scope of the classical works. Singular and non-singular kernels and local and non-local kernels, are studied in fractional calculus (FC). Readers can explore the impressive works in the field of FC. We highlight some useful works as follows: a fractional order COVID-19 model and simulations ^[1], fractional order sine-Gordon equations ^[2], fractional order nonlinear models ^[3], and imperfect testing disease models ^[4,5], which can be studied in the literature.

The infectious disease known as tuberculosis (TB) is typically brought on by the bacteria known as Mycobacterium TB. Although TB most frequently infects the lungs, the disease can manifest in other places of the body as well. Latent TB is the term used when an infection does not manifest any symptoms. This occurs in the majority of cases. About 10% are reinfected if treatment is not received, and approximately half of those who are afflicted will pass away due to the active disease that was caused by the latent infection that eventually became active. Active TB often presents itself with a cough that brings up blood-tinged mucus, night sweats, fever, and a reduction in body weight. Other symptoms may accompany these as well. Because of the significant weight loss that was associated with the disease in the past, it was historically known as consumption. There is a wide variety of symptoms that can be brought on by an infection in another organ. People who have active TB in their lungs can pass the disease on to others through the air when they cough, spit, speak, or sneeze. This is how the disease is transmitted. Latent TB does not contribute to the spread of the infection. Active infection happens more frequently in population who already have HIV or AIDS, or are smokers. Chest X-rays, as well as microscopic analysis and the culture of fluids in a body, are utilized in the diagnostic process for active tuberculosis. The tuberculin skin test, also known as the TST, or a blood is used to diagnose latent TB. One of the most interconnected important public health issues is TB. TB is a leading cause of deaths in HIV-positive individuals, according to ^[6]. Those who are infected with Mycobacterium TB and also have HIV or AIDS have a tenfold increased risk of developing TB compared to those who are HIV-uninfected ^[7]. Because of how the disease expresses itself in a unique way, TB is usually challenging to diagnose ^[8]. Additionally, the presence of TB makes HIV infection worse through a number of processes, some of which include an enhanced viral structure and an CD4/CD8 cell growth ^[9]. Because of the drug complications, close toxic effects characteristics and immune recovery inflammatory condition, and a high medication burden that may affect compliance, co-infection of TB and HIV, makes it difficult to handle. This is because of the similarities in toxicity traits between the two conditions.

Moreover, there has been indication that prompt diagnosis of TB reduces the risk of disease and death in individuals who have been infected with the condition, and successful treatments are regularly used. One of the most significant challenges to global health that the world's nations have been confronted with over the passed 30 years has been the spread of infection of various groups that have the HIV-TB diseases. These illnesses are two of the leading causes of death around the world, and one of the reasons for this is that HIV infection weakens the immune system of humans.

Even though the mathematical modeling of biological processes is not a novel concept in the realm of scientific fields, the COVID-19 pandemic which has significantly affected the entire world has enhanced its usage. This is the case despite the fact that the mathematical modeling of biological processes has been an interest of researchers for quite some time ^[10]. Co-infection of HIV and TB disorders is typical and had been mathematically estimated and examined by applied researchers in numerous works. As ^[11] shows, some of the research included the dynamics of HIV-TB co-infection as well as integrated treatment strategies. Using such a model, different combination therapy strategies were examined, simulating HIV-TB co-infection. They also tried combining different medicines that were given at different periods. Mycobacterium TB has been shown to worsen the clinical record in HIV infected individuals; ultimately, dealing with TB in HIV infected individuals may have a substantial impact on the disease spread. A model of a host with co-infections of TB and HIV was constructed by Kirschner et al. ^[12]. It was the first time anyone had attempted to understand how TB impacts the characteristics of HIV-infected people. It is believed that TB, which is the most prevalent and severe opportunistic infection that affects HIV-positive people, is the primary cause of more than half of the cases that develop into AIDS syndrome in underdeveloped countries.

Zhao et al. ^[13], considered the following TB model with consideration of the age factor in the susceptible class:

with

In this model there are four compartments including the susceptible group $"S(t)"$, $"E(t)"$ which represents the exposed but not yet actively infected individuals, $"I(t)"$ which represents the infected people among the population and the recovered people which are grouped in the class $"R(t)"$. For better interpretation of the age factor in the infection spread, the susceptible portion has been divided into three subclasses: child $"\mathrm{S}_1 (t)"$, middle-aged people $"\mathcal{S}_2 (t)"$, and elders $"\mathrm{S}_3 (t)"$. The death rate of the recovered people is denoted by $"d"$. Death caused by TB is expressed in terms of the rate of $"\mu"$ which is added to $"d"$. The annual birth in the whole population is $"A"$, $"m_1"$ and $"m_2"$ are the rates at which the child susceptibles become middle age susceptibles and the susceptibles from the middle age group transition to the elderly, respectively, $"\vartheta_1"$, $"\vartheta_2"$ and $"\vartheta_3"$, are the death rates for the child, middle-aged people and elderly susceptible groups, respectively $"p"$ is the rate of fast-spreading infection cases; $"v"$ is the rate at which the dormant organisms are re-activated in TB patients, $d_1, \, d_2, \, d_3$ are the death rates for the child, the middle-aged and the subclass of the elderly susceptible, $"d"$ is the natural death rate, $"\mu"$ is the rate of death due to TB, $"\gamma"$ represents the rate of regaining health, and $"\eta"$ is the rate of TB treatment.

FC is used in so many fields of science and engineering because of its theoretical outcomes, numerical computations and experimental results. The use of FC-theory chaotic problems for the investigation of previously unknown and novel forms of chaotic behavior in dynamical systems can be seen in ^[14,15]. In mathematical physics, FC has numerous applications ^[16,17]. Bio-mathematics ^[18,19,20] and engineering ^[21,22] problems have recently become target applications for FC. Regarding dynamical systems, smoking models can be seen in previous works ^[23,24]. Non-oscillatory solutions for nabla forced equations were studied in ^[25]. Asymptotic and oscilatory criteria for nonlinear differential equations were considered in ^[26]. Impulsive fractional differential equations (FDEs) with piecewise Caputo's FDEs were investigated for the existence and uniqueness of solutions (EUS) with applications in ^[27,28,29].

Further, Atangana and Araz studied a new subject of operators known as piecewise operators ^[30]. The exponential and Mittag-Lefler kernels fail to address the crossover behavior of a case problem. Scientists are utilizing this approach to analyze the crossover behaviors in dynamical systems. In the study of disease dynamics, this type of piecewise operator is extensively applied. For example, Dengue virus transmission within populations was considered for piecewise operators by Ahmad et al. ^[31]. One can see more related and useful works on piecewise operators in ^[32,33,34]. For more details on numerical methods and their applications the readers may study in ^[35,36].

In light of these merits, we give the notion of the piecewise modified Atangana-Baleanu-Caputo (mABC) fractional derivative and apply it to a TB model. This novel operator is a combination of the classical derivative and the recently developed modified Atangana-Baleanu operator in the Caputo's sense. For this combination, we have considered the splitting of an interval $[0, t_2]$ for $t_2\in\mathbb{R}^+$, such that, the classical derivative is applied in the first portion $[0, t_1]$ while the second differential operator is applied in the interval $[t_1, t_2]$. As a result, we have the piecewise mABC operator. Its corresponding integral is also given accordingly. This new operator is then applied to a TB model for the study of crossover behavior. The existence of solutions and stability of solutions are studied for the nonlinear piecewise mABC TB model. A numerical scheme for the simulations is presented and applied to the available data. We shall explore the model (1.1) in the following fashion, employing the piecewise differential operator in the case of the classical and mABC derivatives.

In the piecewise TB model (1.2), the $\mathrm{P}CC$ represents piecewise the classical and mABC derivatives.

In order to make the calculations easier, we rewrite TB model (1.2) in piecewise form, as follows

where, $\mathbb{G}_1 = A-d_1\mathrm{S}_1-m_1\mathrm{S}_1-\vartheta_1\mathrm{S}_1I, \mathbb{G}_2 = m_1\mathrm{S}_1-d_2\mathcal{S}_2-\vartheta_2\mathcal{S}_2I-m_2\mathcal{S}_2,$$\mathbb{G}_3 = m_2\mathcal{S}_2-d_3\mathrm{S}_3-\vartheta_3\mathrm{S}_3I, \mathbb{G}_4 = (1-p)[\vartheta_1\mathrm{S}_1+\vartheta_2\mathcal{S}_2I+\vartheta_3S-3I]-vE-dE,$$\mathbb{G}_5 = p(\vartheta_1\mathrm{S}_1I+\vartheta_2\mathcal{S}_2I+\vartheta_3\mathrm{S}_3I)-(d+\gamma+\mu)I+\eta R+vE, \mathbb{G}_6 = \gamma I-dR-\eta R$.

2.   Preliminaries

In this section, the fundamental definitions associated with the mABC calculus are explained. Additionally, the piecewise operators are defined with respect to both the classical and mABC operators. These definitions and findings will be put to use in the future to obtain existence results and implement a numerical scheme for the simulations of the dynamics of the piecewise model (1.2)

Definition 2.1. ^[37,38] For $\varrho\in(0, 1)$, and $f\in L^1(0, T)$, the mABC derivative is given as follows

One can easily obtain that $^{mABC}D^{\varrho}_0 C = 0$ ^[37].

Definition 2.2. ^[37,38] The modified AB-integral for $\varrho\in(0, 1)$, and $f\in L^1(0, T)$, is defined by

Lemma 2.3. ^[37] For $f'\in L^1(0, \infty)$, and $\varrho\in(0, 1)$, we have

Definition 2.4. ^[30] The piecewise integral for a differentiable $\mathbf{h}(\mathsf{t})$, is given by:

Definition 2.5. Consider a differentiable function $\mathbf{h}(\mathsf{t})$; then, the piecewise derivative (PD) of $\mathbf{h}(\mathsf{t})$ is

Definition 2.6. For a differentiable function $\mathbf{h}(\mathsf{t})$, the piecewise integration of the classical and mAB-integral is given by

where $^{\mathrm{P}F}_{0}\mathbb{I}_{\mathsf{t}}\mathbf{h}(\mathsf{t})$ is integer order integration in $0 < \mathsf{t}\leq \mathsf{t}_1$ and in $\mathsf{t}_1 < \mathsf{t}\leq \mathsf{t}_2$; it is an mAB fractional integral.

Lemma 2.7. The piecewise DE for $t\in (0, T]$ is given by

has the following solution

3.   Qualitative analysis

In this section, we focus on EUS for the piecewise model (1.2). To accomplish this, we can make use of Lemma 2.7. Furthermore, in order to provide further clarification, we can also write the aforementioned model in the following form; for which, one can be benefit from ^[39,40,41].

The solution of

is

where

Let us take $\infty > t_2\geq t > t_1 > 0$ and the set $E = {C{^6}}[0, T]$ as a Banach space with

for $\mathrm{g}(t) = (\mathrm{g}_1, \mathrm{g}_2, \ldots, \mathrm{g}_6)$.

The presumptions are as follows:

(C1) $\exists$ $L_{\mathrm{g}} > 0$; $\forall$ $G, \ \bar{\mathrm{g}}\in {E}$ we arrive at

(C2) $\exists$ $C_{ \mathbb{G}} > 0$ & $M_{ \mathbb{G}} > 0,$;

Theorem 3.1. With the assumption of $(C2)$ and the piecewise function $\mathbb{G}$, the TB model (3.1) has a solution.

Proof. Considering a closed subset $\mathfrak{B}$ of $E$,

Assume that $\mathbb{T}: {\mathfrak{B}} \rightarrow {\mathfrak{B}}$ and from (5.1), we have the following

For the $\mathrm{g}\in {B}$, we have

Hence, $\mathbb{T}(\mathfrak{B})\subset \mathfrak{B}$. Thus, we have that $\mathbb{T}$ is a closed and complete. Now, to show that the operator $\mathbb{T}$ is completely continuous, we assume that $t_n > t_m \in [0, t_1]$, which gives us the following:

Thanks to (3.3), when $t_m\rightarrow t_n$, we have that

This implies equicontinuity of $\mathbb{T}$ in $[0, t_1]$. For $t_i, t_j \in [t_1, T]$, we have

If $t_n\rightarrow \mathsf{t}_m$, then

This demonstrates that $\mathbb{T}$ is equally continuous over the interval $[t_1, t_2]$. As a result, we proved the equicontinuity of the operator $T$. Thus, by the Arzel'a-Ascoli and Schauder theorems TB model (3.1) has a solution. □

Theorem 3.2. Let us assume that $(C1)$ holds true and $\mathbb{L}_g\big(\frac{1-\varpi}{B(\varpi)}+ \frac{1}{B(\varpi)}\frac{(t_2-t_1)^{\varpi}}{\Gamma(\varpi)}\big) < 1$; then, the TB system with the piecewise operator given by (3.1) has a unique solution.

Proof. We already discussed the continuity of $\mathbb{T}: \mathfrak{B}\rightarrow \mathfrak{B}$. Furthermore, for $g, \, \bar{\mathrm{g}} \in \mathfrak{B}$ on $[0, t_1]$, we have

From (3.5), we have

This implies that $\mathbb{T}$ is a contraction and by Banach's contraction principle, (3.1) has a unique solution. Moreover, for $t\in[t_1, t_2]$, we have

From (3.7), we have

So, $\mathbb{T}$ is a contraction. By the help of Banach's theorem, the model has a unique solution. □

4.   Hyers-Ulam-Stability

Here, we give definition for the Hyers-Ulam (HU) stability and derive it for the proposed model.

Definition 4.1. The piecewise model (1.2) is HU-stable if for $\alpha > 0$, and

there is $\overline{\mathrm{g}}\in Z$ with $\mathcal{H} > 0$, a constant, satisfying that

Also, for a nondecreasing function $\Phi:[0, \infty)\rightarrow R^{+}$, if we have

where $\Phi(0) = 0$, then the model (1.2) is generalized HU-stable.

Lemma 4.2. Consider the function

The solution of (4.4) is

It is easy to obtain the following

Theorem 4.3. With the help of lemma 4.2 and $\frac{L_{f}}{1-M_{f}} < 1$, the solution of (1.2) is HU-stable as well as gHU- stable.

Proof. Let us assume that $\mathrm{g}$ is a solution of the model (1.2) and consider an approaximate solution $\overline{\mathrm{g}}$ of (1.2), for $t\in\mathrm{B}$, implies that

Upon further simplification, we get

Case 2: for $t\in\mathrm{B}$, we have

for $\bar{\delta}_g = \frac{L_g}{\alpha}\big(\frac{1-\varrho}{B(\varpi)} + \frac{1}{\Gamma(\varpi)B(\varpi)}\big)$. Since, $\mathrm{g}, \, \, \bar{\mathrm{g}}$ are solutions of (1.2), this implies that these are the fixed points (FPs) of $\mathbb{T}$, or mathematically, $\mathbb{T}(\mathrm{g}) = g$, and $\mathbb{T}(\bar{\mathrm{g}}) = \bar{\mathrm{g}}$. Thus, from (4.7), we have

Also, we have

With the use of (4.7), (4.8), and (4.10), we have

For $\mathcal{H} = \frac{1}{1-\bar{\delta}_g}$. Thus, (1.2) is HU-stable. Also, with a replacement of $\alpha$ by $\Phi(\alpha)$, (4.4), implies that

From $\Phi(0) = 0$, we have that (1.2) is gHU-stable. □

5.   Numerical scheme

Here, we aim to produce a numerical Scheme for (1.2). The scheme has been applied to obtain computational results. To demonstrate this, consider the following:

At $t = t_{n+1}$, we deduce for (5.1) that

Now, (5.1) can be expressed by using Lagrange's interpolation polynomial (LIP), we have

6.   Numerical simulations and discussion

Mycobacterium TB affects the brain, kidneys, and spine, but mostly the lungs. TB is one of the biggest infectious disease killers globally, and it spreads through coughs and sneezes. TB symptoms might be moderate and may not present until the disease progresses. Coughing, chest discomfort, fever, and weariness are common. Night sweats, weight loss, and appetite loss may occur. Untreated TB can cause lung damage, organ failure, and death ^[42]. A chest X-ray, TST, and sputum cultures can diagnose TB. TB treatment requires months of medication. Even if symptoms improve, patients must finish the medications to prevent the development of antibiotic-resistant germs. Early diagnosis, treatment, immunization, and infection control measures like hospital isolation and masks can prevent TB from spreading. TB remains a public health issue, especially in low- and middle-income nations, despite advances. The COVID-19 pandemic has disrupted TB detection and treatment, increasing infections in some nations. TB impacts millions of individuals globally. Early diagnosis, treatment, and prevention are crucial to minimizing the disease's burden, and more research is needed to find better medicines and vaccines to fight this global health issue.

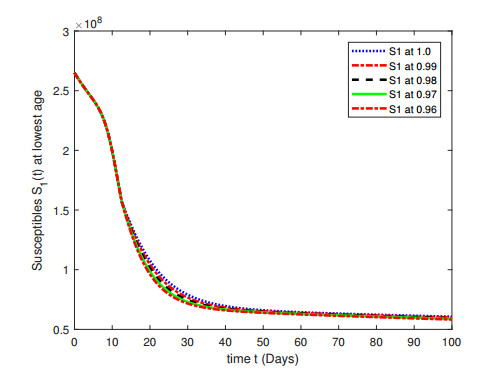
Here, we present the application of the computational scheme for the (1.2) with the parametric values given by $A = 1.623*10^7, \, \mu = 0.0025, p = 0.05, \, \gamma = 0.496, \, \eta = 0.00341, \, \phi = 0.9, \, d = 0.007, \, d_1 = 0.0017, \, d_2 = 0.0023, \, ,\, \nu = 6, \, \vartheta_2 = 5.11*10^{-10}, \, \vartheta_1 = 1.268*10^{-10}, \, \vartheta_3 = 2.553*10^{-9}, \, \, d_3 = 0.0367, m_1 = 0.079, \, m_2 = 0.0067$, and $, \, \xi = 0.51$. For the fractional orders $\varphi = 1.0, \, \varphi = 0.99, \, \varphi = 0.98, \, \varphi = 0.97$ and $, \, \varphi = 0.96$, with the initial conditions given by $\mathrm{S}_1(0) = 26504*10^4, \, \mathcal{S}_2(0) = 94197*10^4, \, \mathrm{S}_3(0) = 10055*10^4, \, \, E(0) = 970279*0.121, \, \, I(0) = 1259308,$ and $\, R(0) = 776223$ as given in ^[13]. Figure 1 shows the dynamics of the susceptible group $\mathrm{S}_1(t)$.

Here we describe the simulations that are shown in the figures. Thus, the differential operator and integral operator that we propose have been derived from a combination of classical and mABC operators. As such, we have assumed a study period of 100 days, that is, $I = [0,100]$. This interval was divided into two sub-intervals. The first one is $I_1 = [0, 35]$ and the second one is $[35,100]$. Thus, $t_1 = 35$ and $t_2 = 100$. Figure 1 presents the results of simulations for the $S_1(t)$ dynamics for the crossover behaviour is presented in which is for the younger most susceptible population, which exhibited a decrease. The role of the modified derivative in the graph is apparent. Because it is closer to the classical case that is $1$.

There is a slight higher rate of decrease. And beyond $t_1$, the cross over behaviour is well established. We have presumed the orders of the derivative to be $1.0, \, 0.99, \, 0.98, \, 0.97, \, 0.96$. This class is a bit sensitive to the cross over behaviour as compared to the results in Figure 2, and Figure 3. Both of these graphs show a sudden decrease of the population of the susceptible classes. This decrease in population indicates a transition of individuals to the exposed group, the results of which are given in the Figure 4. In this class, the role of the derivative is very much apparent. In the early days there is a rise in the dynamics of the class and then we have a slight and gradual decrease in the population. This decrease is clearly reflected in the change in population of the infected group, as shown in Figure 5. There is an increase in the population of the recovered class in the $[t_1, t_2]$, as shown in Figure 6. Here, as the order decreases, there is an increase in the population of the group. The cross over behavior of the recovered class can be seen in Figure 6.

7.   Conclusions

In this paper, we introduced a piecewise differential operator which is a combination of the classical derivative and the mABC fractional derivative. Its corresponding integral is also given. The new operator was applied to a TB model for the study of its dynamics. We have observed very nice interpretations of the dynamics in the two sub intervals. For the numerical simulations, we considered the the interval of study as $[0,100]$ in days. This interval was divided into sub-intervals as $I_1 = [0, t_1)$ and $[t_1, t_2]$, where $t_1 = 25$ and $t_2 = 100$. In the $I_1$ interval, we have considered the classical case while in the second interval $I_2$, we have the mABC operators. This work is an interpretation of the dynamics of crossover behavior of TB under the two types of operators.

The EUS with a piecewise derivative was investigated for the TB-infection model. HU-stability based on the nonlinear analysis was used to show the stability of the solutions. To approximate the solution to the stated issue, the piecewise LIP was used. The computations for the proposed infection model have been given for various fractional orders. In the simulation aspect, we have observed that beyond $t_1$, the cross over behaviour is well established. We have presumed the orders of the derivative to be $1.0, \, 0.99, \, 0.98, \, 0.97, \, 0.96$. Figures 2 and 3 show sudden decreases in the population of the susceptible classes. These decreases in population are included as a component of the population that is exposed, and its details are presented in Figure 4. Within this context, the function of the derivative is made abundantly clear. After an initial period in which there is an increase in the population of the exposed group, there is a minor and steady drop in the overall population of the class. This drop is likely the one that was reflected in the infected group shown in Figure 5. There is also increase in the number of people belonging to the recovered class during the time period $[t_1, t_2]$. In this situation, the population of the group is growing at a rate that is disproportionately faster than the order's decline.

Use of AI tools declaration

The authors declare that they have not used Artificial Intelligence tools in the creation of this article.

Acknowledgments

Hasib Khan and Jehad Alzabut express their sincere thanks to Prince Sultan University. Jehad Alzabut, also expresses his gratitude to OSTİM Technical University for their endless support. The fifth author would like to thank Azarbaijan Shahid Madani University.

Conflict of interest

The authors declare no conflict of interest.

Author statement

Hasib Khan: Conceptualization, Methodology, Writing- Original draft preparation, Software; Jehad Alzabut: Conceptualization, Methodology, Software, Writing-Original draft preparation; J.F. Gómez Aguilar: Conceptualization, Methodology, Writing- Original draft preparation, Software, Supervision; Praveen Agarwal: Methodology, Software, Writing-Original draft preparation. All authors read and approved the final manuscript.