Exploring analytical results for (2+1) dimensional breaking soliton equation and stochastic fractional Broer-Kaup system

1.   Introduction

A differential equation whose coefficients are random is known as a stochastic differential equation. They could be random functions or random constants, but their statistical features should be disclosed, just as the coefficients are in regular equations. As a result, a random function will be the solution of the equation, and the challenge is to identify its statistical characteristics. In the past ten years or so, it has become evident how crucial stochastic differential equations are to solving many issues in the sciences. Systems of differential equations have historically been used to construct mathematical simulations of dynamic processes in engineering, biology, and physics ^[1,2,3]. Model confirmation and future research into dynamic processes associated with initial value problems depend on concerns about the presence and uniqueness of solutions.

The initial value problem is transformed into a corresponding integral equation problem by using approximation strategies, fixed point theory, or methods of exact solution ^[4,5,6]. For mathematical reasons, the normalized Wiener process ^[7] is used to define stochastic processes as integral equations, to explain the impact of random environmental instabilities.

The escalating interest in fractional-order differential equations over recent decades has revolutionized a myriad of scientific fields, including processing, biophysics, wave theory, quantum mechanics, biology, ecology, fractional stochastic systems, control processing, and viscoelastic systems. This interest stems from their superior ability to model complex behaviors and phenomena with greater accuracy than traditional differential equations. Specifically, Katugampola's work in 2014 ^[8] introduced an innovative approach to generalized fractional derivatives, significantly enhancing the mathematical framework for fractional calculus. Following this, Mohamed, Faeza, and Nidal in 2022 ^[9] developed a computational technique for studying analytical solutions to the fractional Modified KDV-Zakharov-Kuznetsov equation, demonstrating the potential of fractional-order differential equations in providing deeper insights into complex systems. Further expanding the scope, Faeza and Mohamed in 2021 ^[10] explored advanced analytical wave solutions in both (2+1)- and (3+1)-dimensional spaces, showcasing the versatility and depth fractional-order differential equations bring to modeling wave dynamics. Additionally, Mohamed and Faeza in 2022 ^[11] discussed the significant advantages of applying differential equations to solve complex problems in mathematical physics, highlighting the importance of symbolic computation in understanding such intricate systems ^[12,15]. Collectively, these pivotal contributions illustrate the transformative impact and broad applicability of fractional-order differential equations in enhancing our understanding and modeling of complex scientific phenomena ^[16,17,18].

The necessity of investigating SBSE using some random force appears to be more pressing. Because of the significance of this hydrodynamic model, which describes waves in shallow water and plasma physics, various extremely complex physical phenomena can be described more comprehensively and accurately by these analytical stochastic solutions ^{[19,20,21,22]}. Given the importance of the Broer-Kaup equations, some researchers have developed exact analytical solutions to this system, which is solved by many methods, including improved Jacobi elliptic function methods ^[23,24] and sine-cosine methods ^[25,26]. The Broer-Kaup equations ^[27,28] describe the unidirectional diffusion of wave propagation according to the Navier-Stokes equations ^[29,30].

The primary objective of our work is to obtain analytical stochastic solutions for SBSE and the SFBK equations in the existence of a stochastic period. To accomplish this, we utilize the first integral method ^[31,32]. These equations have significant relevance in various fields such as the hydrodynamic wave classic of shallow-water waves, plasma physics, and fluid dynamics, as they describe important phenomena in these domains ^[33,34]. By obtaining analytical stochastic solutions, we can provide a more extensive and crucial understanding of these highly complex physical phenomena.

Additionally, our obtained solutions extend previous results reported in ^{[26,31,33,35]}, further contributing to the existing body of knowledge. We also examine the effects of the Wiener process on the analytical solutions of these equations using Mable tools to generate graphical representations ^[36,37,38]. Through the first integral method, we derive explicit expressions of solutions for the equations. These solutions encompass trigonometric, exponential, hyperbolic, and solitary wave solutions, expanding upon previous findings ^[39,40,41]. Given the significance of the Broer-Kaup equations in modeling the bidirectional propagation of long waves in shallow water ^[17], it becomes imperative to investigate the SBSE equation with the inclusion of a random force. This equation is particularly relevant in describing waves in shallow water and plasma physics, employing a hydrodynamic wave model ^[18]. With the introduction of analytical stochastic solutions, we can comprehensively and accurately describe a wide range of intricate physical phenomena ^{[42,43,44,45]}.

Furthermore, the results obtained in this study demonstrate the effectiveness and flexibility of the proposed method in finding analytical solutions for complex problems. The impact of noise on the solution of SBSE and the system of SFBK equations is clearly demonstrated, indicating the broad applicability of the proposed method ^[1]. Revolutionizing our comprehension of intricate physical phenomena, this study presents a groundbreaking exploration into analytical stochastic solutions for the SBSE and the SFBK. By employing the first integral method ^[19,20], we delve into the intricacies of these mathematical models, uncovering new exact solutions that significantly contribute to our understanding of complex phenomena ^[1].

The application of the first integral method allows us to not only extend previous results but also unveil novel insights into the (2+1) SBSE equation and the SFBK system of equations. This comprehensive investigation leads to the discovery of fresh analytical solutions, encompassing trigonometric, exponential, hyperbolic, and solitary wave solutions. Recent advancements in fractional-order differential equations have significantly impacted diverse scientific fields, as evidenced by the pioneering works of Bai and Zhao (2010) ^[21] on algebraic methods for Broer-Kaup-Kupershmidt equations, Mohammed et al. (2023) ^[22] on the effects of noise in soliton solutions, Al-Askar et al. (2022) ^[23] on the Wiener process's impact on soliton equations, Yıldırım and Yaşar (2018) ^[24] on breaking soliton equations, Feng (2002) ^[25] on the first-integral method for nonlinear wave equations, Wazwaz (2010) ^[26] on integrable soliton equations, and Khalil et al. (2014) ^[27] on a new fractional derivative definition. These studies collectively enhance our understanding of complex systems through novel mathematical frameworks, computational techniques, and analytical solutions, marking significant strides in applied mathematics, physics, and beyond, demonstrating the broad applicability and transformative potential of fractional calculus in modern scientific research.

The significance of these findings lies in their broad applicability across diverse fields. The proposed method not only demonstrates its efficiency but also showcases remarkable flexibility in providing solutions to intricate problems. As we venture into uncharted territories of mathematical modeling, this research serves as a foundation for future explorations, promising to enhance our understanding of complex physical phenomena and contribute to advancements in various scientific disciplines.

The remainder of this paper is organized as follows. In Section 2, we present the New First Integral Method. Section 3 shows the applications for our main results. In Section 4, we present schematic illustrations. Conclusions are given in Section 5.

2.   New first integral method

Nonlinear partial differential equations are generally defined as follows:

by using the wave transforms for Eq (1) of the form

such that

Equation (1) can be transformed into ordinary differential equations as

The idea of the first integral method is to suppose new independent variables as

Then, we get a system of ordinary differential equations as

It is challenging to calculate the first integral for the autonomous system described by Eq (6). However, we can overcome this difficulty by applying the division theorem based on the fundamental theory of differential equations ^[28]. The division theorem states that if $\Lambda (X, Y)$ and $P(X, Y)$ are polynomials of two variables $X$ and $Y$ in $\mathbb{C}[X, Y]$, where $P(X, Y)$ is irreducible in $\mathbb{C}[X, Y]$, and if $\Lambda (X, Y)$ vanishes at any zero point of $P(X, Y)$, then there exists a polynomial $Q(X, Y)$ in $\mathbb{C}[X, Y]$ such that $\Lambda (X, Y) = P(X, Y)\cdot Q(X, Y)$ ^[28]. This theorem enables us to directly solve the system using the division theorem, providing a valuable approach for finding the desired solutions.

3.   Applications

In this part, we search for the applicability of the first integral method to solving important nonlinear stochastic equations with two types of derivatives: fractional and partial.

3.1.   Stochastic (2+1)-dimensional breaking soliton equation

The formula for the SBSE ^[29] is

where $\phi(x, y, t)$ is a real stochastic function of $x, y, \; \mathrm{a}\mathrm{n}\mathrm{d}\; t$, $W = W\left(t\right)$ a standard Wiener process that represents the Brownian motion, and σ is the noise intensity.

To obtain the wave equation of the SBSE, we employ the next wave transformation:

where $\rho$ is a real function, and ${\theta }_{i}$ for all $i = \mathrm{1, 2}, 3$ are real factors. Note that

where $\frac{1}{2}{\sigma }^{2}dt$ is the Itô correction factor. Substituting (8) into (7) and using (9), we attain the resulting ordinary differential equation:

Remembering that $\rho$ is the deterministic function and applying the operation of taking the expectation with respect to the variable t to both sides of the Eq (10), we obtain

But for any normal Gaussian process $Z$, such that $\mathrm{E}\left[\mathrm{e}\mathrm{b}\mathrm{Z}\right] = {e}^{\frac{{b}^{2}}{2}Var\left(Z\right)}$, given that $Var\left(Z\right) = t$ for a standard Brownian motion where $Var\left(W\right(t\left)\right) = t$, we have

It follows from Eq (12) that $\sigma W\left(t\right)$ is distributed like $\sqrt{t}Z$, so Eq (11) becomes

By integrating Eq (13) and simplifying, we get

Let $U\left(\zeta \right) = {\mathrm{\rho }}^{\mathrm{{'}}}\left(r\right)$, where $r = \zeta = x+y+ct$. Substituting in Eq (14), we obtain

By utilizing Eq (5) and comparing with Eq (6), we get a system of ordinary differential equations:

After that, we apply the division theorem to find the first integral of Eq (16). There are non-trivial solutions to Eq (16), where $\Lambda (x, y) = \sum_{i = 0}^{M}{a}_{i}\left(x\right){y}^{i} = 0.$ Then, it is an irreducible polynomial. $\mathbb{C}\left[X, Y\right].$

Here, Eq (17) is called the first integral method (FIM), and ${a}_{i}\left(X\right)(i = \mathrm{0, 1}, 2, \dots M)$are polynomials where ${a}_{M}\left(X\right)\ne 0$. There exists a polynomial $\alpha \left(X\right)+\beta \left(X\right)Y$ in $\mathbb{C}\left[X, Y\right]$. That is,

Now, $M = 1$ in Eq (18) gives

According to the new first integration method ^[25], by equating the coefficients ${Y}^{i}\left(i = \mathrm{2, 1}, 0\right)$we obtain

and

where

and

Since ${a}_{i}\left(x\right) (i = 0, 1)$ are polynomials, from Eq (20), we deduce that ${a}_{1}\left(x\right)$ is a constant, and $\beta \left(x\right) = 0$. For simplification, taking ${a}_{1}\left(x\right) = 1$ we have

By Eqs (20) and (21), we conclude that $\alpha \left(X\right) = AX+B$. Then, we find

Integrating Eq (19) with ${a}_{1}\left(x\right)$ and ${a}_{0}\left(x\right)$ and ignoring the constant of integration, we get ${a}_{0}\left(X\right) = \sqrt{2{X}^{3}-c{X}^{2}}$. Substituting in Eq (17), we obtain

Combining Eq (22) with Eq (16), we have two cases:

Case 1: Let $\mathrm{c} > 0.\; \mathrm{I}\mathrm{f}\; \mathrm{c} = {a}^{2}$, then Eq (16) has the solution

Case 2: Let $\mathrm{c} < 0.\; \mathrm{I}\mathrm{f}\; \mathrm{c} = -{a}^{2}$, then Eq (16) has the solution

Then, from Eqs (15), (23), and (24), we find

Also,

Combining with Eq (14), we get

Then, the general analytical stochastic solutions of Eq (1) are

where ${\zeta }_{0}, {\zeta }_{1}, \; \mathrm{a}\mathrm{n}\mathrm{d}\; {\zeta }_{2}$are constants of integration.

3.2.   Stochastic fractional Broer-Kaup equations

Now, we study the following system of SFBK equations:

where $\phi(x, t)$ is a stochastic function representing horizontal velocity, the stochastic function $\varphi (x, t)$ is the height that deviates from the balance position of fluid, the derivative ${D}_{x}^{\alpha }$is conformable ^[30], $W\left(t\right)$ is a standard Wiener process representing Brownian motion, and σ is the noise intensity.

The Broer-Kaup equations refer to a pair of interconnected nonlinear partial differential equations that provide a mathematical framework for modeling the simultaneous propagation of long waves in shallow water. The Eq (31) can be regarded as a broader representation of the Korteweg-de Vries equation, a widely recognized mathematical model utilized to study solitons and various other nonlinear wave phenomena. Extensive research has been conducted on the Broer-Kaup equations, employing diverse analytical and numerical techniques. These investigations have revealed the presence of intricate and diverse dynamics within the system, including solitary waves, periodic waves, multi-peakons, and interactions. There are two distinct physical consequences that arise from the investigation of the soliton-bearing Sine-Gordon equation in connection with the Broer-Kaup equations. On the one hand, this research has the potential to yield novel perspectives on the mathematical characteristics and solutions of the Broer-Kaup equations, encompassing aspects such as integrability, symmetry, conservation laws, and bifurcations.

The SFBK equations with $\sigma = 0$ are used to model the bidirectional propagation of long waves in shallow water ^[1].

The wave transforms for the SFBK equations are given by

Here, $c$ is a constant, while $\phi\; \mathrm{a}\mathrm{n}\mathrm{d}\; \varphi$ are functions. Now, putting Eq (32) in Eq (31), we get

Taking the expectation E(·) for Eq (33), we get

Since $W\left(t\right)$ is a normal distribution, $\mathrm{E}\left({e}^{\sigma W\left(t\right)}\right) = {e}^{{\sigma }^{2}/2t}$. Now, Eq (34) becomes

Integrating Eq (35) and setting the integration constants equal to zero, we get

Now, eliminating $\varphi$ Eq (36), we get

We need to apply the first integral method in the form

where $T\left(U\right(\zeta), {U}^{{'}}(\zeta \left)\right)$ is a polynomial in $U\; \mathrm{a}\mathrm{n}\mathrm{d}\; {U}^{{'}},$ and $R\left(U\right(\zeta \left)\right)$ is a polynomial with real coefficients.

Now, with Eq (31) we find $T\left(U\right(\zeta), {U}^{{'}}(\zeta \left)\right) = 0$, and$R\left(U\left(\zeta \right)\right) = {U}^{3}\left(\zeta \right)+2c{U}^{2}\left(\zeta \right)+({c}^{2}-1)U\left(\zeta \right)$, so Eq (15) changes and becomes

Using Eqs (5) and (6), Eq (39) is equivalent to the two-dimensional autonomous system

Now, we apply the division theorem to seek the first integral to Eq (40), the nontrivial solution to Eq (40), where $\Lambda \left(x, y\right) = \sum_{i = 0}^{M}{a}_{i}\left(x\right){y}^{i} = 0$, which is an irreducible polynomial in the complex domain $C[X, Y]$. Thus,

${a}_{i}\left(X\right)(i = 0, \; 1, \; 2, \dots, M)$are polynomials, and ${a}_{M}\left(X\right)\ne 0$. Equation (41) is called the first integral method. There exists a polynomial $\alpha \left(X\right)+\beta \left(X\right)Y$ in $C\left[X, Y\right]$such that

Now, when $M = 1$, Eq (42) gives

By equalizing the coefficients ${Y}^{i}\left(i = 2, \; 1, \; 0\right)$, we have

and

where

and

Since ${a}_{i}\left(x\right)(i = 0, 1)$ are polynomials, from (44), we deduce that ${a}_{i}\left(x\right)$ is a constant, and $\beta \left(x\right) = 0$. For simplicity, take ${a}_{1}\left(x\right) = 1$. We have

By Eqs (44) and (46), we conclude that $\alpha \left(X\right) = AX+B$. Then, we find

Substituting ${a}_{1}\left(x\right)$ and ${a}_{0}\left(x\right)$ into Eq (45) and setting all coefficients of ${X}^{i}(i = 2, 1, 0)$ to zero, we get many groups of values for constants, as

Using the above groups of values for constants in Eq (41) and combining with Eq (40), respectively, we obtain

By the above solutions we get the new solutions of Eq (31) with Eq (32) as follows:

When $c = 0$,

When $c = 3$,

When $c = -3$,

When $c = 0$,

When $c = -3$,

When $c = 3$,

If $M = 2$, Eq (42) gives

According to the new first integration method, by equating the coefficients ${Y}^{i}\left(i = 3, \; 2, \; 1, \; 0\right)$, we have

and

where

and

Since ${a}_{i}\left(x\right)(i = 0, 1, 2)$ are polynomials, from (54), we deduce that ${a}_{2}\left(X\right)$ is a constant, and $\beta \left(x\right) = 0$. For simplification, taking ${a}_{2}\left(x\right) =$ 1, we have

By Eqs (54) and (56), we conclude that $\alpha \left(X\right) = AX+B$, where $A, B$ are constants. Then, we find

where $d$ is the constant of integration.

Substituting ${a}_{2}\left(X\right)$, ${a}_{1}\left(X\right)$, and ${a}_{0}\left(X\right)$ into Eq (55) and setting all coefficients of ${X}^{i}(i = 5, 4, 3, 2, 1, 0)$ to zero, we get six sets of constant values:

Putting the above in Eq (41) and combining with Eq (40), respectively, we obtain

The exact solution to Eq (31) with Eq (32) is as follows:

When $c = 0$,

When $c = 0$,

When $c = 3$,

When $c = -3$,

When $c = -3$,

When $c = 3$,

We do not think that the solutions obtained when $M = 2$ are the same as the solutions when $M = 1$. The only difference is the arrangement of the solutions.

Remark 1. Some published solutions to this problem may be re-derived from Eqs (29) and (30):

● Equations (21) and (27) in ^[26] are particular solutions with $a = 1$.

● Equations (60) and (61) in ^[31] are particular solutions with$\sigma = 0$.

Equation (29) in ^[32] is a particular integral of Eq (47). There are more special cases of equations. Relations (47) and (57), with$\sigma = 0$, and Eqs (50) and (58) with $\sigma = 0$ and ${C}_{1} = 0$, can be found in ^[33].

These are all the prior results known to the authors.

Remark 2. Finding exact solutions to stochastic fractional equations is a complex task due to the combined challenges of fractional calculus and stochastic processes. Exact solutions are often difficult to obtain, and research in this area is ongoing. However, there are some fundamental principles and techniques that can be used. Here are a few principles along with relevant sources:

Laplace transform Method for fractional equations: The Laplace transform can be extended to fractional calculus, enabling the transformation of fractional differential equations into algebraic equations. However, this method might not directly address stochastic terms ^[34].

Fractional differential equations and special functions: Fractional differential equations can sometimes be mapped to special functions like the Mittag-Leffler function, which is a generalization of the exponential function ^[35].

Integral transform methods for fractional equations: Techniques like the Laplace, Fourier, and Mellin transforms can be adapted for solving fractional equations. However, incorporating stochastic terms into these transforms is challenging ^[36].

Fractional stochastic calculus: Combining fractional calculus with stochastic processes requires specialized tools from fractional stochastic calculus ^[37].

It is important to note that exact solutions for stochastic fractional equations are often limited due to the inherent complexity and randomness involved. Researchers frequently rely on numerical methods, Monte Carlo simulations, or approximations to analyze and understand the behavior of such equations.

4.   Graphical simulations and discussion

The impact of noise on the data or system might manifest in several manners, influencing the characteristics and dynamics of these solutions. One potential issue that can arise in the numerical integration, interpolation or differentiation of sinc functions is the introduction of errors due to noise. These mistakes have the potential to result in solutions that are either erroneous or unstable. The presence of noise can have an impact on both the convergence and stability of the iterative methods employed for solving the linear systems that result from the discretization of integral equations. In addition, the presence of noise can have an impact on the selection of optimal parameters for the SBSE and SFBK methods. These factors include the number and placement of collocation points, the width of sinc windows, and the regularization value.

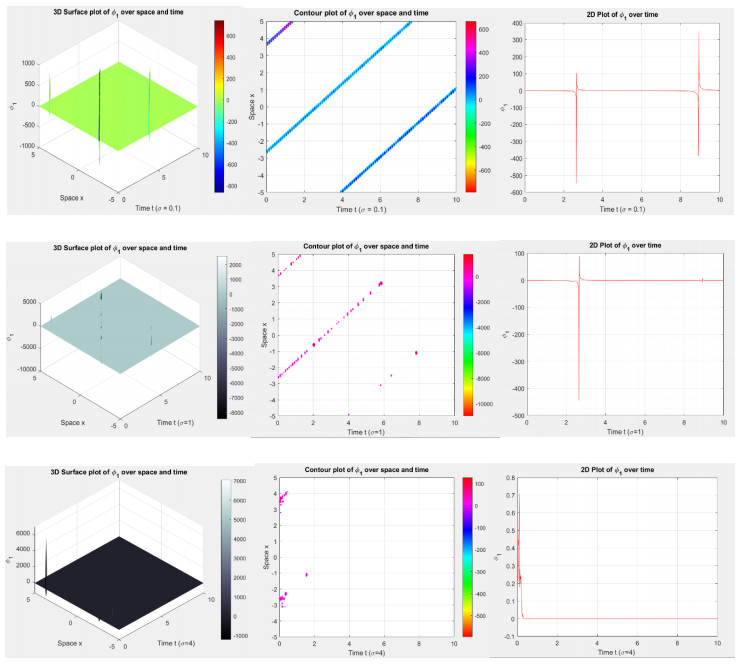
We validate at this point the influence of the Wiener process on the analytical explanations of the SBSE (7) and the SFBK (31). In the following are certain diagrams of the performance of these results. We plot the solutions (29) and (52) for several intensities of noise. In Figure 1, we plot the solution ${\phi}_{1}\left(x, y, t\right)$ in Eq (29) with parameters$a = 2$, ${\zeta }_{0} = 1$, and ${\zeta }_{2} = 1$ using MATLAB at $y = 1$, for $x\in \left[\mathrm{0, 5}\right]$ and $t\in \left[\mathrm{0, 5}\right]$. In Figures 2 and 3 we plot ${\phi}_{6}\left(x, t\right)$ and ${\varphi }_{6}\left(x, t\right)$in Eq (52) with parameters $c = 3\text{and}{C}_{1} = 1$, for different values of $\sigma$ and $\alpha$. When we examine the surface at $\sigma = 0.1$ in Figure 1, we find that there is some fluctuation and that it is not entirely flat. However, when the noise is considered, and its intensity is increased by a factor of $\sigma = \mathrm{1, 4}$, As noise intensifies, the solution's surface smooths out, yet it also develops minor, intricate patterns. This demonstrates that the Wiener process has an effect on the solutions and helps stabilize them. Figure 2 shows the effect of fractional order. If $\sigma = 0.1$, we can see that the surface expands when α is increasing. Figure 3 shows that the surface is greatly flatter when noise is added, and noise strength is increased from $\sigma = \mathrm{1, 4}$. Also, it shows that the Wiener process influences the solutions and aids in stabilizing them.

The deterministic breaking soliton equation (7) (with$\sigma = 0.1$) describes the propagation of shallow water waves, with different dispersion relations. The performance of these waves' changes with particular outer effects (random fluctuations) is considered in Eq (29) as exposed in Figure 1 with σ = 0. As we explain before, the external influence has an impact on the waves and makes them stable with$\sigma \ne 0$. The deterministic Broer-Kaup equation (31) (with$\sigma = 0.1$) describes the propagation of shallow water waves, with different dispersion relations. The conduct of these waves' changes with specific outer influence (random fluctuations) is reflected in Eq (52) as shown in Figure 1 with σ = 0.1. As we explicated earlier, outer effects have an influence on the waves and make them stable with$\sigma \ne 0\; \mathrm{w}\mathrm{i}\mathrm{t}\mathrm{h}\alpha$. The soliton equation (7) and Broer-Kaup equation (31) describe shallow water waves with different dispersion relations. Figure 1 shows the dampening effect of a stochastic driving term.

The three plots we have shared graphically illustrate the effect of the fractional order α on the solution ${\phi}_{6}\left(x, t\right)$ of a given differential equation. Here is how the fractional order α appears to affect the solution, based on the plots: For a smaller value of α, the plot is more localized around the origin, and the peaks of the waveform are closer to the center. This could indicate that a lower fractional order results in more localized and possibly higher frequency behavior of the solution. As α increases, the solution spreads out, and the waveform becomes less localized. This could suggest that a higher fractional order leads to solutions that are more distributed over the domain and possibly have a lower frequency. The amplitude of the solutions also changes significantly with α. For the lowest value of α, the solution's amplitude is the smallest. As α increases, so does the amplitude, reaching a maximum for the highest value of α shown. The physical meaning of these observations would depend on the context of the differential equation. The fractional order in differential equations can represent the effect of memory or hereditary properties of a material or process. In the context of wave propagation, for example, different values of α might model how various media affect the speed, dispersion, and absorption of waves. Lower orders might represent media with high dispersion or damping, while higher orders could represent more inertial or less dispersive media.

In the context of wave models, parameters like the fractional order α, wave amplitude factor ${C}_{1}$, and noise intensity $\sigma$ crucially influence the characteristics of wave solutions. The fractional order α can dictate the wave speed and dispersion, with higher values potentially leading to slower wave propagation and increased dispersion, affecting the wave's phase and spreading. The amplitude factor ${C}_{1}$ typically determines the height and steepness of the wave, where larger values may result in higher and sharper wave peaks, thus influencing the wave profile's overall shape. Lastly, the noise intensity $\sigma$ introduces stochastic fluctuations, which can cause variations in both the phase and amplitude of the wave.

5.   Conclusions

This study marks a significant advancement in the field of hydrodynamic wave analysis by delving into the SBSE and the SFBK. Utilizing the first integral method, we have derived explicit solutions, including trigonometric, exponential, hyperbolic, and solitary wave solutions, thus bridging a critical gap in the existing literature. Our findings not only extend but also enrich the understanding of shallow-water wave behaviors in the presence of stochastic influences, offering a novel perspective that was previously unexplored. The introduction of analytical solutions to the SBSE and SFBK equations under stochastic conditions represents a notable departure from conventional studies, which have primarily focused on deterministic approaches. The ability to capture wave behavior under varying degrees of randomness ($\sigma = 0$ and $\sigma \ne 0$) underscores the originality and utility of our research, positioning it as a foundational piece for future investigations in this domain. Moreover, our work distinguishes itself by demonstrating that the first integral method, while not universally applicable to all degrees of polynomial solutions, is a robust tool for uncovering new insights into complex wave dynamics. The exploration of solutions beyond polynomial degree $M > 1$and the identification of novel solutions not previously reported in the literature (^{[12,13,14,23,28,29]}) further underscore the innovative nature of our research. As we look to the future, our study opens the door to a broad spectrum of applications and investigations, ranging from fractional models in cancer research to complex equations governing atmospheric and oceanic phenomena. The path laid by our research invites further exploration into fractional models such as the fractional cancer model, fractional coupled Hirota-Satsuma and KdV equations, Navier-Stokes equations, and beyond. This not only demonstrates the broad applicability and relevance of our findings but also highlights the ongoing need for innovative analytical methods in addressing the multifaceted challenges presented by stochastic and fractional dynamics in natural sciences. Our research contributes a novel, comprehensive framework for understanding and modeling the intricate behaviors of shallow-water waves under stochastic and fractional conditions. By addressing significant gaps in the current literature and introducing fresh analytical solutions, this work sets a new benchmark for future studies in hydrodynamic wave analysis and related fields, ensuring its place as a pivotal reference for researchers seeking to advance the boundaries of knowledge in this crucial area of study. Some previously reported results were referenced from ^[46,47,48]. In future research, the focus will be on solving new fractional models such as the fractional cancer model ^[49], fractional coupled Hirota-Satsuma and KdV equations ^[50], Navier-Stokes ^[51], and others ^{[52,53,54,55]}.

Use of AI tools declaration

The authors declare they have not used artificial intelligence (AI) tools in the creation of this article.

Acknowledgments

The authors extend their appreciation to the Deanship of Postgraduate Studies and Scientific Research at Majmaah University for funding this research work.

Conflict of interest

The authors declare no conflict of interest.