Distance spectrum of some zero divisor graphs

1.   Introduction

In this paper, all graphs considered are simple, finite and connected. Consider a graph $G = (V(G), E(G))$, where $V(G) = \{v_1, v_2, \dots, v_n\}$ is the vertex set and $E(G)$ is the edge set. The neighborhood $N_G(v)$ of $v\in V(G)$ is the set of vertices adjacent to $v$. The degree $d_v$ of a vertex $v$ is the number of elements in the set $N_G(v)$. The length of the shortest path connecting two vertices, $u$ and $v$, is known as the distance $d_G(u, v)$ between them. The vertices of $G$ index the distance matrix $D(G) = (d_{uv})$ of $G$, where $d_{uv} = d_G(u, v)$. Since $D(G)$ is a real symmetric matrix, the eigenvalues $\psi_1 \geq \psi_2 \geq \dots\geq \psi_n$ of $D(G)$ are all real and they are referred to as the distance eigenvalues of $G$. The distance spectral radius of $G$ is the largest eigenvalue $\psi_1$ of $D(G)$ and the distance spectrum of $G$ is the multiset of all the eigenvalues of $D(G)$. The distance energy of $D(G)$ is defined as

The distance spectrum and the distance energy of $G$ have been thoroughly investigated thus far; for further information on their history and development, see ^{[5,6,13,14,15,22,30,31,32,34]} and the references therein.

For a Hermitian matrix $M$, the inertia of $M$ is a triplet $(e_{1}(M), e_{2}(M), e_{3}(M)),$ where $e_{1}(M), e_{2}(M)$ and $e_{3}(M)$ are the number of positive, zero and negative eigenvalues of $M$, respectively. Let $\mathcal{R}$ be a commutative ring with identity different from one. If we can locate $v\neq 0\in \mathcal{R}$ such that $u\cdot v = 0$, then $u\neq 0\in \mathcal{R}$ is known as the zero divisor of $\mathcal{R}$. The set of all non-zero zero divisors of $\mathcal{R}$ is denoted by $Z^*(\mathcal{R})$. The $\Gamma(\mathcal{R})$ is said to be a zero divisor graph if $a, b \in V(\Gamma(\mathcal{R})) = Z^*(\mathcal{R})$ and $(a, b) \in E(\Gamma(\mathcal{R}))$ if and only if $a$. $b = 0$.

In recent years, the zero divisor graph has received a great deal of interest. When Beck ^[7] first introduced the zero divisor graphs of commutative rings in 1988, he considered the set of vertices as zero divisors including $0$. Later, Anderson and Livingston ^[2] modified the definition of zero divisor graphs and considered only non-zero zero divisors as vertices. Young ^[33] was the first to discuss the adjacency eigenvalues of zero divisor graphs. In ^[18,19], the spectral theory was developed further. A number of topological indices as well as the energy and the Laplacian energy of zero divisor graphs along with their corresponding line graphs of commutative rings were provided by Singh and Bhat ^[28,29]. For more literature on zero divisor graphs, see ^{[1,3,11,17,20,24,25,27]} and the references cited therein. For notations/terminology, the readers are referred to ^[9,12,21].

The rest of the paper is organized as follows. In Section 2, we discuss the distance spectrum of $\Gamma(\mathbb{Z}_t[x]/\langle x^4 \rangle)$, $\Gamma(\mathbb{Z}_{t^2}[x] / \langle x^2 \rangle)$ and $\Gamma(\mathbb{F}_{t}[u] / \langle u^3 \rangle)$, respectively. We also find the inertia of the distance matrices of the said graphs. In Section 3, we give the sharp bounds for the distance energy of these graphs while providing the closed formula for the distance energy of $\Gamma(\mathbb{F}_{t}[u] / \langle u^3 \rangle)$.

2.   Distance spectrum of zero divisor graphs

A factor ring is formed by the set of all cosets $\mathcal{R}/\mathcal{I} = \{\mathcal{I} + a; a \in \mathcal{R} \}$, where $\mathcal{I}$ is the ideal of $\mathcal{R}$. Consider $\mathbb{Z}_n[x] = \{a_nx^n + \dots + a_1x + a_0 | a_i \in \mathbb{Z}_n\}$ to be a polynomial of a commutative ring.

We start by considering the finite commutative ring $\mathbb{Z}_t[x] / \langle x^4 \rangle$, where $t$ is any prime. Then, $\Gamma(\mathbb{Z}_t[x]/\langle x^4 \rangle)$ is a zero divisor graph with $(t^3 - 1)$ zero divisors of $(\mathbb{Z}_t[x]/\langle x^4 \rangle) / \{0\}$ considered to be vertices and $\frac{1}{2}(t-1)(3t^3 - t^2 - 2t - 2)$ edges. This graph structure was originally defined by Johnson and Sankar in ^[16] and was modified with typo correction by Rather in ^[23]. For the sake of completeness, we write the details of this graph structure. The vertex set of $\Gamma(\mathbb{Z}_t[x] / \langle x^4 \rangle)$ can be written as

It is clear to see that $D = \Big\{ x^{3}, 2x^{3}, 3x^{3}, \dots, (t-2)x^{3}, (t-1)x^{3} \Big\}$ and hence $|D| = t-1.$ Likewise, $|E| = t(t-1)$ and the cardinality of $F$ is $t(t^{2}-t).$ Further, for any two elements say $a_{i}x^{3}$, $a_{j}x^{3}$ in $D,$ we obtain

Every element of $D$ is therefore adjacent to every other element that is contained inside $D$. Thus, they form a clique of cardinality $t-1$. Now, if $b_{i}x^{3}+a_{j}x^{2}, b_{j}x^{3}+a_{i}x^{2} \in E,$ we see that

This shows that a clique of size $t^{2}-t$ is formed by the vertices in $E$, meaning that every vertex in $E$ is connected to every other vertex in $E$. Again for $c_{i} x^{3} +b_{i} x^{2} +a_{i} x \in F$ and $c_{j} x^{3} +b_{j} x^{2} +a_{j} x \in F,$ we have

Therefore, the vertices in $F$ form an independent set of cardinality $t^{3}-t^{2}$ because they are not adjacent to each other. Again, for $a_{i} x^{3} \in D$ and $b_{i}x^{3} + a_{j} x^{2}\in E,$ we see that

Every vertex in $D$ is therefore connected to every vertex in $E$. Similarly, if $a_{i} x^{3}\in D$ and $c_{i} x^{3} + b_{i} x^{2} + a_{j} x\in F,$ we have

This means that every vertex in $D$ is adjacent to every vertex in $F$. Finally, if $b_{i} x^{3} + a_{i} x^{2} \in E$ and $c_{j} x^{3} + b_{j} x^{2} + a_{j} x \in F,$ then

As a result, it can be concluded that no vertex in $F$ is adjacent to any of the vertices in $E$. This gives us the structure of $\Gamma(\mathbb{Z}_t[x]/\langle x^4 \rangle)$ completely. We use an example (the same as described in ^[16]) to demonstrate this method.

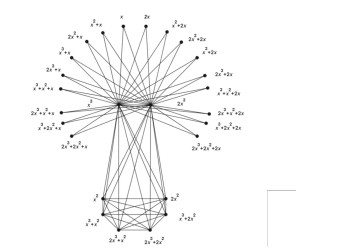
Example 1. For $\mathcal{R}\cong \mathbb{Z}_{3}[x]/\langle x^{4} \rangle$, the vertex set of $G \cong \Gamma \big(\mathbb{Z}_{3}[x]/\langle x^{4} \rangle \big)$ is as follows

Figure 1 displays the graphical representation of $G$. It can be seen that the two vertices in $D$ have degree $25$ and are dominating vertices (that is, adjacent to all vertices). In $E$, the degree of each vertex is $7$, and in $F$, it is $2$.

We now examine the distance spectrum of $\Gamma (\mathbb{Z}_{t}[x]/\langle x^{4} \rangle)$, but first we need to mention some known results.

For $\alpha = 0$ in Proposition 11 of ^[10], the following conclusions are drawn.

Lemma 1. ^[10] Suppose that $G$ is a connected graph of order $n$ and $S$ is a subset of the vertex set $V(G)$ such that $N_G(x) = N_G(y)$ for any $x, y\in S$, where $|S| = t$. Then

(i) If $S$ is an independent set, then $-2$ is an eigenvalue of $D(G)$ with multiplicity at least $t-1$.

(ii) If $S$ is a clique, then $-1$ is an eigenvalue of $D(G)$ with multiplicity at least $t-1$.

The distance spectrum of the zero divisor graph of $\mathbb{Z}_{t}[x]/\langle x^{4} \rangle$ is provided by the following theorem.

Theorem 1. Consider a zero divisor graph $G\cong \Gamma(\mathbb{Z}_{t}[x]/\langle x^{4} \rangle)$ with any prime $t$. Then the distance spectrum of $G$ consists of the eigenvalues $-1$ and $-2$ having multiplicities $t^{2}-3$ and $t^{3}-t^{2}-1$, respectively. The remaining distance eigenvalues of $G$ can be obtained as the zeros of the following polynomial

Proof. We label the vertices of $G$ from the elements in $D,$ then the elements in $E$ and finally in $F.$ According to this vertex labelling, the distance matrix of $G$ is given by

Here, $I$ is the identity matrix and $J$ is the matrix whose each entry is $1$. Given that a clique of size $t-1$ is formed by the vertices in $D$ and that each of these vertices of $D$ has the same neighbors, the hypothesis of Lemma 1 is satisfied. Consequently, $G$ has a distance eigenvalue of $-1$ having multiplicity of $t-2$, and the corresponding eigenvectors are

With the similar idea, the vertices in $E$ form a clique and satisfy the hypothesis of Lemma 1, so it follows that $-1$ is the distance eigenvalue of $G$ having multiplicity $t^{2}-t-1$. The associated eigenvectors of the distance eigenvalue $-1$ are

Hence, $-1$ is the distance eigenvalue of $G$, where the multiplicity of $-1$ is $t^{2}-t-1+t-2 = t^{2}-3$. Moreover, it can be observed from the structure of the zero divisor graph that the vertices of $F$ constitute an independent set, where each of these vertices of $F$ has the same neighbors. Hence, using Lemma 1, it can be seen that $-2$ is the distance eigenvalue of $G$ having multiplicity $t^{3}-t^{2}-1$ and its corresponding eigenvectors are

Consequently, this method gives us $t^{3}-t^{2}-1+t^{2}-3 = t^{3}-4$ distance eigenvalues. Next, we have to look for the other three remaining eigenvalues of $D(G).$ For $i = 1, 2, 3, \dots, t^{3}-1,$ consider $X$ to be the eigenvector of $D(G)$, where $x_{i} = X(v_{i})$. Hence, it can be deduced (refer to ^[9]) that each component of $X$ that corresponds to a vertex in $D$ is equal to $x_{1}$, components of $X$ that correspond to vertices in $E$ are equal to $x_{2}$ and components of $X$ that correspond to vertices in $F$ are denoted by $x_{3}$. Thus, with $X = \Big(\underbrace{x_{1}, x_{1}, \dots, x_{1}}_{t-1}, \underbrace{x_{2}, x_{2}, \dots, x_{2}}_{t^{2}-t}, \underbrace{x_{3}, x_{3}, \dots, x_{3}}_{t^{3}-t^{2}}\Big)^T$, the eigenequation $D(G)X = \lambda X$ gives us

The coefficient matrix of the right hand side of the above system of equations is

From (2.3), we obtain the characteristic polynomial of $P$ as follows

The zeros of the polynomial $f(\lambda)$ are the remaining three distance eigenvalues of $G$. □

Since the zeros of $f(\lambda)$ cannot be explicitly found, so we will approximate them with the help of intermediate value theorem. Let $\lambda_1 \geq \lambda_2 \geq \lambda_3$ be the zeros of (2.4), then with the manual calculations, we have

Thus, with the above observation and intermediate value theorem, it follows that

Based on the above theorem and observation, Theorem 1 gives us the following result.

Corollary 1. The inertia of $D(\Gamma(\mathbb{Z}_{t}[x]/\langle x^{4} \rangle))$ is $\begin{cases} (1, 0, t^{3}-2)\; \mathit{\text{if}}\; \lambda_{2} < 0, \\ (2, 0, t^{3}-3)\; \mathit{\text{if}}\; \lambda_{2} > 0. \end{cases}$

Next, we consider the finite commutative ring $\mathbb{Z}_{t^2}[x] / \langle x^2 \rangle$, where $t \geq 3$ is any prime. Then, the zero divisor graph $G\cong \Gamma(\mathbb{Z}_{t^2}[x]/\langle x^2 \rangle)$ of order $(t^3 - 1)$ and size $\frac{1}{2}(2t^5 - 2t^4 - t^3 -t^2 +2)$ can be obtained by the following procedure, see ^[26].

Here,

Now, we divide the vertex set as follows

Clearly $|D| = (t-1), |E| = 2t(t-1)$ and $|F| = t(t-1)^2.$ Additionally, $E$ and $F$ are subdivided by

Here, we made some slight corrections to the subdivision of the set $F$ (for more details, see ^[26]). It is clear to see that if $b_itx, b_jtx \in D \implies (b_itx)(b_jtx) = b_ib_jt^2x^2\equiv 0 \mod x^2$, then all of the vertices in $D$ are adjacent to one another and if $b_itx \in D, b_jt, a_jx, b_jtx+ b_jt \in E \implies b_ib_jt^2x \equiv 0 \mod t^2, b_ia_jtx^2 \equiv 0 \mod x^2, b_ib_jt^2x^2 + b_ib_jt^2x \equiv 0 \mod t^2$, subsequently each vertex in $D$ is connected to each vertex in $E$. Finally, if $b_itx \in D, a_jx+b_jt \in F \implies (b_itx)(a_jx+b_jt) = b_ia_jtx^2 + b_ib_jt^2x \equiv 0 \mod x^2$ or $\mod t^2$, consequently each vertex in $D$ is adjacent to each vertex in $F$.

Furthermore, if $a_ix, a_jx \in E_1 \implies (a_ix)(a_jx) = a_ia_jx^2 \equiv 0 \mod x^2$, then all vertices in $E_1$ are adjacent to one another. The same is true for $E_2$. However, if $a_ix \in E_1, b_jt \in E_2 \implies (a_ix)(b_jt) = a_ib_jtx \not\equiv 0 \mod x^2$, therefore there isn't a vertex in $E_1$ that is adjacent to a vertex in $E_2$.

Last, if $a_ix +t, a_jx + t(t-1) \in F_1 \implies (a_ix + t)(a_jx + t(t-1)) = a_ia_jx^2 + (a_i+a_j)t^2x + t^2(t-1) \equiv 0 \mod x^2$ or $\mod t^2$, then $a_ix + t$ is adjacent to $a_jx + t(t-1)$ in $F_1$ but no two $a_ix +t$ or $a_jx + t(t-1)$ has zero product by modulo $x^2$ or $t^2$ in $F_1$. The same is true for $F_2, F_3, \dots, F_{t-1}$.

An illustration (pictorial representation) of the above construction for $\mathbb{Z}_{t^2}[x] / \langle x^2 \rangle$ with $t = 3$ is given as below.

Now, we will give the distance spectrum of $\Gamma(\mathbb{Z}_{t^2}[x] / \langle x^2 \rangle)$ in the following result.

Theorem 2. Consider a zero divisor graph $G\cong \Gamma(\mathbb{Z}_{t^2}[x] / \langle x^2 \rangle)$ with any prime $t \geq 3$. Then the distance spectrum of $G$ consists of the eigenvalues $-1$, $-2$ and $t^{2}-t-2$ with multiplicities $2t^{2}-t-4$, $t^{3}-2t^{2}+1$ and $t-2$, respectively. The other distance eigenvalues of $G$ are $-(t^2 - t + 1)$ and the zeros of the polynomial given below

Proof. Beginning with the vertices in $D,$ we label the vertices in $E_1$, $E_2$, $F_1, F_2, \dots, F_{t-1}$ respectively. According to this vertex labelling, the distance matrix of $G$ is given by

Here, $I$ is the identity matrix, $J$ is the matrix whose each entry is $1$, $A_{1j} = J_{(t-1)\times (t^{2}-t)},$ for $j = 4, 5, \dots, t+2,$ $A_{ii} = 2(J_{(t^{2}-t)}-I_{(t^{2}-t)})$, for $i = 4, 5, \dots, t+2$, $A_{ij} = 2\big(J_{(t^{2}-t)\times (t^{2}-t) }\big),$ for $i = 2, 3,$ and $j = 4, 5, \dots, t+2,$ $A_{ij} = J_{(t^{2}-t)\times (t^{2}-t)}, i = 4, 5, \dots, t+2, j = 4, 5, \dots, t+2$ except $i\neq j.$

Given that a clique of size $t-1$ ($t^{2}-t$) is formed by the vertices of $D$ ($E_{1}$ and $E_{2}$) in $G$ and that each of these vertices of $D$ ($E_{1}$ and $E_{2}$) has the same neighbors, the hypothesis of Lemma 1 is satisfied. Hence, with the similar analysis as in Theorem 1, the distance spectrum of $G$ comprises of the eigenvalue $-1$ having multiplicity $2(t^{2}-t-1)+t-2 = 2t^{2}-t-4$. Also, the elements in $F_1$ ($F_2, F_3, \dots, F_{t-1}$) form an independent set, with each vertex in $F_1$ ($F_2, F_3 \dots, F_{t-1}$) having the same neighbourhood. Lemma 1 thus indicates that $-2$ is the eigenvalue of $D(G)$, where the multiplicity of $-2$ is $(t-1)(t^{2}-t-1) = t^{3}-2t^{2}+1$. Hence, with this method, we have obtained $t^{3}-2t^{2}+1+2t^{2}-t-4 = t^3 -t -3$ distance eigenvalues.

Next, with eigenvector

the coefficient matrix of the eigenequation $D(G)X = \lambda X$ is

It is easy to verify that $t^{2}-t-2$ is the eigenvalue of $P$ having multiplicity $t-2$ and corresponding eigenvectors are $X_{i} = \big(0, 0, 0, -1, x_{i1}, x_{i2}, \dots x_{i(t-2)}\big)^T$ where $x_{ij} = \begin{cases} 1 & \text{if}\; i = j\\ 0 & \text{if}\; i\neq j \end{cases}$, for $j = 1, 2, \dots, t-2$ and $i = 1, 2, \dots, t-2$. The other four distance eigenvalues of $G$ are the eigenvalues of the following matrix

From (2.6), we obtain the characteristic polynomial of $P^{\prime}$ as follows

It is clear that $-(t^2 - t + 1)$ is a zero of the above polynomial. The following polynomial gives the remaining three distance eigenvalues of $G$

□

Next, we approximate the zeros of $g(\lambda)$ given in Eq (2.7). Let $\lambda_1 \geq \lambda_2 \geq \lambda_3$ be the zeros of (2.7), then it is easy to verify the following

Thus, by intermediate value theorem, it follows that $\lambda_1 \in (t^2 -2, t^4 - 2)$, $\lambda_2 \in (-1, t-2)$ and $\lambda_3 \in (-t^3, -t)$.

Based on the above theorem and observation, we have the following consequence of Theorem 2.

Corollary 2. The inertia of $D(\Gamma(\mathbb{Z}_{t^2}[x] / \langle x^2 \rangle))$ is $\begin{cases} (t-1, 0, t^{3} -t)\; \mathit{\text{if}}\; \lambda_{2} < 0, \\ (t, 0, t^{3} -t -1)\; \mathit{\text{if}}\; \lambda_{2} > 0. \end{cases}$

Let $t$ be an odd prime. The ring $\mathbb{F}_{t}[u] / \langle u^3 \rangle$ is defined as a characteristic $t$ ring subject to restrictions $u^3 = 0$. The ring isomorphism $\mathbb{F}_{t}[u] / \langle u^3 \rangle \cong \mathbb{F}_t + u\mathbb{F}_t + u^2\mathbb{F}_t$ is obvious to see. An element $a + ub + u^2c$ is unit if and only if $a \neq 0$.

Now, we will discuss the zero divisor graph $\Gamma(\mathbb{F}_{t}[u] / \langle u^3 \rangle)$ of order $(t^2 - 1)$ and size $\frac{1}{2}(2t^3 - 3t^2 - t +2)$ which can be obtained by the following procedure, see ^[4].

The vertex set $V(\Gamma(\mathbb{F}_{t}[u] / \langle u^3 \rangle)) = D \cup E \cup F$, where

As $u^3 = 0$, every vertex of $D$ is adjacent with every vertex of $E$, every vertex of $E$ is adjacent with every vertex of $F$ and any two distinct vertices of $E$ are adjacent.

Example 2. For $t = 3$, the vertex set of $\Gamma(\mathbb{F}_{3}[u] / \langle u^3 \rangle)$ is given as

The diagram of $\Gamma(\mathbb{F}_{3}[u] / \langle u^3 \rangle)$ is shown in Figure 3, where the number of vertices is $8$ and the number of edges is $13$.

Now, we will give the distance spectrum of $\Gamma(\mathbb{F}_{t}[u] / \langle u^3 \rangle)$ in the following result.

Theorem 3. Consider a zero divisor graph $G\cong \Gamma(\mathbb{F}_{t}[u] / \langle u^3 \rangle)$ with odd prime $t$. Then the distance spectrum of $G$ consists of the eigenvalues $-1$ and $-2$ having multiplicities $t-2$ and $t^2 - t -2$, respectively. The remaining distance eigenvalues of $G$ can be obtained as the zeros of the following

which are $-2$ and $\frac{1}{2}\big(2t^2 -t -4 \pm \sqrt{t}\sqrt{4t^3-8t^2+t+4}\big)$.

Proof. We label the vertices of $G$ from the elements in $D,$ then the elements in $E$ and finally in $C.$ According to this vertex labelling, the distance matrix of $G$ is given by

where $I$ is the identity matrix and $J$ is the matrix whose each entry is $1$.

Given that an independent set of size $t-1$ is formed by the vertices of $D$ and that each of these vertices of $D$ has the same neighborhood, the hypothesis of Lemma 1 is satisfied. Consequently, $G$ has a distance eigenvalue of $-2$ having multiplicity $t-2$. Moreover, it can be observed from the structure of the zero divisor graph that the vertices in $E$ form a clique and satisfy the hypothesis of Lemma 1, so it follows that $-1$ is a distance eigenvalue of $G$ having multiplicity $t-2$. With the similar idea, the vertices in $F$ constitute an independent set, with each vertex having the same neighborhood. Hence, it follows from Lemma 1 that $-2$ is the distance eigenvalue of $G$ having multiplicity $t^{2}-2t$. Therefore, the distance eigenvalue $-2$ of $G$ has multiplicity $t^{2}-2t+t-2 = t^{2}-t-2$. Following the same procedure as Theorem 1, we can find the corresponding eigenvectors.

Consequently, we have obtained $t^{2}-t-2+t-2 = t^{2}-4$ distance eigenvalues using this method. Next, we have to look for the other three remaining eigenvalues of $D(G).$ For $i = 1, 2, 3, \dots, t^{2}-1,$ consider $X$ to be the eigenvector of $D(G)$, where $x_{i} = X(v_{i})$. Hence, it can be deduced (refer to ^[9]) that each component of $X$ that corresponds to a vertex in $D$ is denoted by $x_{1}$, components of $X$ that correspond to vertices in $E$ are equal to $x_{2}$ and the components of $X$ that correspond to vertices in $F$ are denoted by $x_{3}$. Thus, with $X = \Big(\underbrace{x_{1}, x_{1}, \dots, x_{1}}_{t-1}, \underbrace{x_{2}, x_{2}, \dots, x_{2}}_{t-1}, \underbrace{x_{3}, x_{3}, \dots, x_{3}}_{(t-1)^2}\Big)^T$, the eigenequation $D(G)X = \lambda X$ gives us

The coefficient matrix of the right hand side of the above system of equations is

From (2.10), we obtain the characteristic polynomial of $P$ as follows

The zeros of the polynomial $h(\lambda)$ are the remaining three distance eigenvalues of $G$. It is clear that $\lambda_1 = -2$, $\lambda_2 = \frac{1}{2}\big(2t^2 -t -4 + \sqrt{t}\sqrt{4t^3-8t^2+t+4}\big)$ and $\lambda_3 = \frac{1}{2}\big(2t^2 -t -4 - \sqrt{t}\sqrt{4t^3-8t^2+t+4}\big)$ are the three zeros of the above polynomial. □

Based on the above theorem, we have the following consequence of Theorem 3.

Corollary 3. The inertia of $D(\Gamma(\mathbb{F}_{t}[u] / \langle u^3 \rangle))$ is $\begin{cases} (1, 0, t^{2}-2)\; \mathit{\text{if}}\; \lambda_{3} < 0, \\ (2, 0, t^{2}-3)\; \mathit{\text{if}}\; \lambda_{3} > 0. \end{cases}$

3.   Distance energy of zero divisor graphs

From Theorem 1, the distance spectrum of $G\cong \Gamma(\mathbb{Z}_{t}[x]/\langle x^{4} \rangle)$ consists of the eigenvalue $-1$ with multiplicity $t^{2}-3$, $-2$ with multiplicity $t^{3}-t^{2}-1$ and the eigenvalues of $P$ given in (2.3). The eigenvalues of $P$ lie in the intervals

By definition of the distance energy, we have

where $E(P)$ is the energy of $P$. With the above calculations of $\lambda_{i}$'s, we get the following bounds for the distance energy of $G$

and

Next, we establish more general sharp bounds for the distance energy of the said graph.

Consider the set of positive real numbers $\{f_{1}, f_{2}, f_{3}, \dots, f_{s}\}$. We define $\Pi_{n}$ to be the average of products of $n$-element subset of $\{f_{1}, f_{2}, f_{3}, \dots, f_{s}\},$ that is

The Maclaurin symmetric mean inequality ^[8] establishes a relationship among $\Pi_{i}$'s as follows

with equalities holding if and only if $f_{1} = f_{2} = \dots = f_{s}.$ Now, from (3.1), we have

We will find bounds for $E(P).$ Using Maclaurin inequality (3.2) on the set

we have

that is,

that is,

where $tr(P^{2})$ is the trace of $P^{2}.$ From (2.3), the trace of $P^{2}$ is

When we substitute it in the above expression, we get

Thus by (3.3), we get

where the equality holds if and only if the equality holds in (3.4), that is, $|\lambda_{1}(P)| = |\lambda_{2}(P)| = |\lambda_{3}(P)|.$ Further, the second inequality of (3.2) gives

that is equivalent to

that is,

that is,

From (2.3), the determinant of $P$ is $det(P) = -t^6+5t^5-8t^4+7t^3-t^2-4.$ Therefore, from (3.3), we have

Equality holds if and only if $|\lambda_{1}(P)| = |\lambda_{2}(P)| = |\lambda_{3}(P)|.$

We make the above observation precise in the following result.

Theorem 4. Consider a zero divisor graph $G \cong \Gamma(\mathbb{Z}_{t}[x]/\langle x^{4} \rangle)$ with any prime $t$. Then

and

where equality holds if and only if $|\lambda_{1}(P)| = |\lambda_{2}(P)| = |\lambda_{3}(P)|.$

Next, from Theorem 2, the distance spectrum of $G\cong \Gamma(\mathbb{Z}_{t^2}[x] / \langle x^2 \rangle)$ consists of the eigenvalue $-1$ with multiplicity $2t^{2}-t-4$, $-2$ with multiplicity $t^{3}-2t^{2}+1$, $t^2 -t -2$ with multiplicity $t-2$ and the eigenvalues of $P^{\prime}$ given in (2.6). The spectrum of $P^{\prime}$ consists of $-(t^2-t+1)$ and the three eigenvalues which lie in the intervals

By definition of the distance energy, we have

With the above calculations of $\lambda_{i}$'s, we get the following bounds for the distance energy of $G$

and

To establish more general sharp bounds for the distance energy of $\Gamma(\mathbb{Z}_{t^2}[x] / \langle x^2 \rangle)$, from (3.6), we have

Now, we will determine bounds for $E(P^{\prime}).$ Using Maclaurin inequality (3.2) on the set

we have

that is,

that is,

Here, $tr(P^{\prime})^{2}$ denotes the trace of $(P^{\prime})^{2}.$ Now, (2.6) gives the trace of $(P^{\prime})^{2}$ as follows

When we substitute it in the above expression, we get

So, (3.7) gives

where the equality holds if and only if equality holds in (3.8), that is, $|\lambda_{1}(P^{\prime})| = |\lambda_{2}(P^{\prime})| = |\lambda_{3}(P^{\prime})|.$ Now, similar to (3.5), we have

From (2.6), the determinant of $P^{\prime}$ is $det(P^{\prime}) = 2t^8 - 16t^7+49t^6-77t^5+63t^4-23t^3-3t^2+3t+4.$ Therefore, from (3.7), we have

Equality holds if and only if $|\lambda_{1}(P^{\prime})| = |\lambda_{2}(P^{\prime})| = |\lambda_{3}(P^{\prime})|.$ Similar to Theorem 4, we have the following result for $G\cong \Gamma(\mathbb{Z}_{t^2}[x] / \langle x^2 \rangle)$.

Theorem 5. Consider a zero divisor graph $G \cong \Gamma(\mathbb{Z}_{t^2}[x] / \langle x^2 \rangle)$ with any prime $t\geq3$. Then

and

where equality holds if and only if $|\lambda_{1}(P^{\prime})| = |\lambda_{2}(P^{\prime})| = |\lambda_{3}(P^{\prime})|.$ Also, $det(P^{\prime}) = 2t^8 - 16t^7+49t^6-77t^5+63t^4-23t^3-3t^2+3t+4$.

The last result gives the closed formula for the distance energy of $\Gamma(\mathbb{F}_{t}[u] / \langle u^3 \rangle)$.

Theorem 6. Consider a zero divisor graph $G \cong \Gamma(\mathbb{F}_{t}[u] / \langle u^3 \rangle)$ with odd prime $t$. Then

Proof. From Theorem 3, the result is evident.□

4.   Conclusions

In this paper, we discussed the distance spectrum of zero divisor graphs like $\Gamma(\mathbb{Z}_t[x]/\langle x^4 \rangle)$ with any prime $t$, $\Gamma(\mathbb{Z}_{t^2}[x] / \langle x^2 \rangle)$ with any prime $t \geq 3$ and $\Gamma(\mathbb{F}_{t}[u] / \langle u^3 \rangle)$ with any odd prime $t$, where $\mathbb{Z}_{t}$ is an integer modulo ring and $\mathbb{F}_{t}$ is a field. We also presented the formulas for the inertia of the distance matrices of the aforementioned graphs. Furthermore, we provide the closed formula for the distance energy of $\Gamma(\mathbb{F}_{t}[u] / \langle u^3 \rangle)$ while giving the sharp bounds for the distance energy of $\Gamma(\mathbb{Z}_t[x]/\langle x^4 \rangle)$ and $\Gamma(\mathbb{Z}_{t^2}[x] / \langle x^2 \rangle)$. As for future work, we suggest finding the distance spectrum and the distance energy for the zero divisor graph $\Gamma(\mathbb{Z}_{st}[x]/\langle x^2 \rangle)$ with any prime $2 < s < t$ (for more details, see ^[26]).

Author contributions

Fareeha Jamal: Conceptualization, Methodology, Software, Validation, Formal analysis, Investigation, Resources, Data curation, Writing-original draft, Writing-review and editing, Project administration; Muhammad Imran: Conceptualization, Validation, Formal analysis, Resources, Writing-review and editing, Visualization, Supervision, Project administration, Funding acquisition. All authors have read and approved the final version of the manuscript for publication.

Use of AI tools declaration

The authors declare they have not used Artificial Intelligence (AI) tools in the creation of this article.

Acknowledgment

This research is supported by UPAR grant of United Arab Emirates University (UAEU) via Grant No. G00003739.

Conflict of interest

The authors declare that they have no competing interests.