Precise large deviations for aggregate claims in a two-dimensional compound dependent risk model

1.   Introduction

Partial differential equations and fractional partial differential equations have developed in a variety of scientific disciplines ^[1,2,3,4]. The popularity of FPDEs, on the other hand, has recently increased as a result of their extraordinary capacity to offer more accurate simulations for a variety of physical phenomena. The integration of non-local memory effects and long-range interactions through fractional derivatives, which take into account the complexity present in many complex systems, is the cause of this increased accuracy ^[5,6,7,8,9]. FPDEs are a strong tool for modeling and analyzing a wide range of phenomena, including heat conduction, wave propagation, image processing and data analysis. They have a wide range of applications in physics, biology, economics and engineering. As a result, the study of FPDEs has developed into a booming research area, stimulating the identification of conceptual foundations and novel methods for resolving these equations, enabling new applications in a variety of real-world situations ^{[10,11,12,13]}.

The solution of FPDEs may be handled in two ways: Numerical and analytical techniques. The finite difference approach ^[14,15], finite element method ^[16], this Monte Carlo method ^[17], shooting method ^[18] and the adaptive moving mesh and uniform mesh methods ^{[19,20,21,22,23]} are examples of numerical methodologies that rely on numerical algorithms and computational simulations to approximate the solution. Analytical methods, on the other hand, use algebraic and calculus techniques to solve issues and produce accurate solutions. Analytical procedures are frequently preferred over numerical methods because they provide this more thorough knowledge of the problem and its underlying behavior. Analytical approaches are very useful when dealing with issues that have simple geometries or equations that can be solved in closed form, increasing efficiency in such situations ^[24,25,26].

As a result, several researchers have explored distinct FPDEs with diverse analytical methodologies. The Laplace Adomian decomposition technique ^[27], (G'/G)-expansion technique ^[28,29,30], perturbation methods ^[31], direct algebraic methods ^[32], variational iteration method ^[33], exp-function approach ^[34,35], auxiliary equation method ^[36], Jacobian elliptic function method ^[37], Riccati mapping method ^[38], Darboux transformation method ^[39], Hirota bilinear method ^[40] and modified extended DAM (mEDAM) ^[41] are some widely used analytical methods. For example, in groundwater modeling, Al-Mdallal et al. employed the Laplace transform approach to solve a FPDE ^[42]. Jiang et al. used a variable separation approach to solve the multi-term time-fractional diffusion-wave equation in a finite domain in another work ^[43]. Similarly, Zheng solved the nonlinear fractional Sharma-Tasso-Olver problem by using the exp-function technique ^[44]. Finally, Khan et al. used the (G'/G)-expansion approach to achieve accurate solutions for FPDEs ^[45]. Overall, these analytical strategies have been shown to be helpful in addressing various types of FPDEs in a variety of scientific and technical domains.

For its accuracy and effectiveness in solving FPDEs, the DAM stands out as a very strong and effective analytical strategy. The DAM differs from previous transformation-based systems in that it can convert FPDEs directly into a system of nonlinear equations without the need for a linearization phase. Using a recommended series-based solution derived from an ordinary differential equation (ODE) solution, the FPDE is first transformed into a nonlinear ODE (NODE), which is then transformed into a system of algebraic equations. The DAM is distinguished by three variants: simple DAM ^[46], extended DAM (EDAM) ^[47] and mEDAM ^[48,49,50]. An enhanced version of the DAM known as the mEDAM, has shown to be extremely efficient in handling a variety of FPDE forms. As a result, the DAM offers a simple, effective, and precise solution to FPDEs, emphasizing its potential for significant contributions in a range of fields of science and technology ^[51,52,53].

The generalized modified Caamassa-Holm (CH) and Degasperis-Procesi (DP) equations were initially presented and studied by Wazwaz ^[54]. These modifications to the DP equation were created by Wazwaz ^[54] as a tool for identifying discrepancies in the physical makeup of the generated solution. It is important to remember that the standard DP equation has multi-peakon solutions. The characteristics of these peakon solutions are altered to bell-shaped solutions in the updated DP equation, though. When examining shallow water dynamics, this equation is applicable, practical and integrable. The fractional modified DP (MDP) equation is a nonlinear FPDE that is created by fusing the MDP equation with fractional derivatives. A versatile equation with uses in fluid dynamics, oceanography and image processing is the fractional MDP equation. The equation has been applied to fluid dynamics to study interactions with submerged objects, characterize wave packet propagation and mimic wave behavior in shallow water. The equation has been applied to oceanography to analyze wave dynamics, interactions between waves and currents and soliton formation. The equation has been applied to image processing to create algorithms for object recognition, image segmentation and edge detection. Because standard derivatives are unable to simulate events with non-local repercussions and long-range dependencies, the inclusion of fractional derivatives in the equation is crucial. Particularly fractional derivatives capture the non-local interactions and memory effects that are prevalent in many real-world systems. The fractional generalized MDP and CH equation has the following mathematical form:

where $u = u(x, t)$, $0 < \alpha, \beta \leq 1$, $\mu$ is a constant and $t \geq 0$. In this study we have solved (1.1) with $\mu = 3$ thus (1.1) becomes the fractional MDP equation given by

To model compressible fluid flow in a medium, the fractional gas dynamics equation is utilized. Aeronautical engineering, combustion research, and materials science are all fields that utilize the equation. Its solutions have been applied in the simulation of shock waves, turbulent flows, and other complex fluid phenomena. The use of fractional derivatives in the equation results in a more accurate representation of the system's dynamics, making it a useful tool for understanding the behavior of compressible fluid flow under a variety of conditions ^[55,56,57]. The fractional gas dynamics equation has the following mathematical form ^[45]:

Both the fractional MDP and fractional gas dynamics equations have been treated analytically and numerically in the literature. For example, Dubey et al. in ^[58] investigated the time-fractional MDP equation with a Caputo fractional derivative by using the Sumudu transform and q-homotopy analysis approach. In ^[59], Zhang et al. offered a detailed comparison of two strong analytical approaches for generating series solutions to fractional DP equations, namely the homotopy perturbation transform method and the Aboodh Adomian decomposition method. Similarly, Das and Kumar successfully used the differential transform approach to derive approximate analytical solutions to the nonlinear fractional gas dynamics equation ^[60]. Finally, Khan et al. used the (G'/G)-expansion approach to achieve accurate solutions for fractional gas dynamics equations ^[45].

However, the fundamental goal and novelty of this research is to enhance the area of nonlinear science by introducing the revolutionary mEDAM approach, which results in the discovery of a slew of new solitary wave solution families for both the fractional MDP and fractional gas dynamics equations. This accomplishment not only broadens current knowledge, it also goes deeper into the complexities of these mathematical models. Furthermore, our research attempted to completely examine the wave behavior of these solitary waves in both models and develop relevant linkages between the wave dynamics and the underlying mathematical formulations, providing insight into the fundamental relationships driving these systems. These combined objectives have our research to make major contributions to the understanding and practical uses of soliton waves in a variety of scientific disciplines.

Because of its widespread acceptance and well-established mathematical qualities, the authors elected to employ Caputo's fractional derivative in this study. Caputo's fractional derivative is well known for retaining the physical meaning of standard derivatives, making it more appropriate for modeling real-world events and systems. It has also improved behavior for non-smooth functions and produces consistent results across a wide range of applications. Here, the authors establish consistency with current literature and increase the credibility and comparability of their findings within the scientific community by employing Caputo's fractional derivative. This operator is defined as follows ^[61]:

where $f(x, t)$ is a sufficiently smooth function. The following two properties of this derivative will be utilized while transforming targeted FPDEs into NODEs:

where $f(x)$ and $g(x)$ are differentiable functions and $j$ is a real number.

2.   The methodology of the modified mEDAM

In this section, we present the methodology of the mEDAM. Consider the FPDE of the following form ^[49,50,62]:

where $y$ is a function of $v_1, v_2, v_3, \ldots, v_n$ and $t$.

To solve Eq (2.1), we follow the following steps:

(1) First we perform variable transformation $y(t, v_1, v_2, v_3, \dots, v_n) = Y(\xi)$, $\xi = \xi(t, v_1, v_2, v_3, \dots, v_n)$, where $\xi$ can be defined in various ways. This transformation converts Eq (2.1) into a nonlinear ODE of the form

where the derivatives of $Y$ in Eq (2.2) are with respect to $\xi$. The constants of integration can then be obtained by integrating Eq (2.2) one or more times.

(2) We then suppose the following solution to Eq (2.2):

where $a_\rho (\rho = -m_1, …, 0, 1, 2, …, m_2)$ are constants to be calculated, and $Q(\xi)$ satisfies the following ODE:

where $A\neq0$ or $1$ and $a, b$ and $c$ are constants.

(3) The positive integers $m_1$ and $m_2$ given in Eq (2.3) are calculated by finding the homogeneous balance between the highest order derivative and the largest nonlinear term in Eq (2.2).

(4) After that, we plug Eq (2.3) into Eq (2.4) or the equation generated by integrating Eq (2.4) and gather all terms of $(Q(\xi))$ in the same order. We then set each coefficient of the following polynomial as equal to zero, yielding a system of algebraic equations for $a_\rho (\rho = -m_1, ..., 0, 1, 2, ..., m_2)$ and other parameters in the system of algebraic equations.

(5) We use Maple to solve this system of algebraic equations.

(6) Finally, we retrieve the unknown values and plug them into Eq (2.3) along with $Q(\xi)$ (i.e., the solution of Eq (2.4)), which gives us the analytical solutions to Eq (2.1). We can generate the following families of solutions by using the generic solution of Eq (2.4).

Family 1. When $Z < 0 \ \ \text{and} \ \ c\neq0$,

and

Family 2. When $Z > 0 \ \ \text{and} \ \ c\neq0$,

and

Family 3. When $ac > 0$ and $b = 0$,

and

Family 4. When $ac > 0$ and $b = 0$,

and

Family 5. When $c = a$ and $b = 0$,

and

Family 6. When $c = -a$ and $b = 0$,

and

Family 7. When $Z = 0$,

Family 8. When $b = \lambda$, $a = n\lambda (n\neq0)$ and $c = 0$,

Family 9. When $b = c = 0$,

Family 10. When $b = a = 0$,

Family 11. When $a = 0$, $b\neq0$ and $c\neq0$,

and

Family 12. When $b = \lambda$, $c = n\lambda(n\neq0)$ and $a = 0$,

Here, $p, q > 0$, and they are called the deformation parameters while $Z = b^2-4ac$. The generalized trigonometric and hyperbolic functions are defined as follow:

Similarly,

3.   Implementation of the method

In this section, we utilize our suggested this improved mEDAM approach to address the targeted problems.

3.1.   Problem 1

First, the equation for the fractional MDP stated in Eq (1.2) is taken into account. In order to transform Eq (1.2) into a nonlinear ODE, we utilize the following complex transformation:

which results in the following

integrating Eq (3.2) with respect to the wave variable $\xi$ and the constant of integration to zero, yields:

We balance the linear and nonlinear terms of the greatest order, which we may do by putting $m_1 = m_2$. When we attempt $m_1 = m_2 = 1$, however, the system of algebraic equations generated via Eq (3.3) only has trivial solutions. As a result, we choose this $m_1 = m_2 = 2$ instead. By replacing $m_1 = m_2 = 2$ into Eq (2.3), we get the following series solution for Eq (3.3):

By substituting Eq (3.3) into Eq (3.2) and equating the coefficients of $(G \left(\xi \right))^i$ to zero for $i = -6, -5, ..., 0, 1, ..., 6$, we obtain a system of nonlinear algebraic equations. We can solve this system for the unknowns $d_{-2}$, $d_{-1}$, $d_0$, $d_1$, $d_2$, $k_1$ and $k_2$ by using Maple. The solution produces four sets of answers:

Case 1.

Case 2.

Case 3.

Case 4.

If we consider Case 1, we obtain the following sets of traveling wave solutions:

Family 1. When $Z < 0$ and $a$, $b$, $c$ are nonzero, the corresponding family of solitary wave solutions for Eq (1.2) is given as follows:

and

Family 2. When $Z > 0$ and $a$, $b$, $c$ are nonzero and the corresponding family of solitary wave solutions for Eq (1.2) is given as follow:

and

Family 3. When $ac > 0$ and $b = 0$, the corresponding family of solitary wave solutions for Eq (1.2) is given as follow:

and

Family 4. When $ac > 0$ and $b = 0$, the corresponding family of solitary wave solutions for Eq (1.2) is given as follow:

and

Family 5. If $c = a$ and $b = 0$, the corresponding family of solitary wave solutions for Eq (1.2) is given as follows:

and

Family 6. When $c = -a$ and $b = 0$, the corresponding family of solitary wave solutions for Eq (1.2) is given as follows:

and

Family 7. If $a = 0$, $b \neq 0$ and $c\neq0$, the corresponding family of solitary wave solutions for Eq (1.2) is given as follows:

and

Family 8. If $b = \lambda$, $c = n\lambda$ (where $n\neq0$) and $a = 0$, the corresponding family of solitary wave solutions for Eq (1.2) is given as follows:

where $\xi = \frac{\sqrt {- \left(4\, ac-{b}^{2} \right) ^{-1}} x^\beta}{\ln \left(A \right) \Gamma(\beta+1)}- \frac{5\, \sqrt {- \left(4\, ac-{b}^{2} \right) ^{-1}} t^\alpha}{2 \ln \left(A \right) \Gamma(\alpha+1)}$.

Now by assuming Case 2, we obtain the following families of solutions:

Family 9. If $Z < 0$ and $a$, $b$, $c$ are nonzero then the corresponding family of solitary wave solutions for Eq (1.2) is given as follows:

and

Family 10. If $Z > 0$ and $a$, $b$, $c$ are nonzero then the corresponding family of solitary wave solutions for Eq (1.2) is given as follows:

and

Family 11. When $ac > 0$ and $b = 0$, this the corresponding family of solitary wave solutions for Eq (1.2) is given as follows:

and

Family 12. When $ac > 0$ and $b = 0$, the corresponding family of solitary wave solutions for Eq (1.2) is given as follows:

and

Family 13. If $c = a$ and $b = 0$, this the corresponding family of solitary wave solutions for Eq (1.2) is given as follows:

and

Family 14. If $c = -a$ and $b = 0$, then the corresponding family of solitary wave solutions for Eq (1.2) is given as follows:

and

Family 15. If $b = \lambda$, $a = n\lambda$ (where $n\neq0$) and $c = 0$, then the corresponding family of solitary wave solutions for Eq (1.2) is given as follows:

where $\xi = \frac{\sqrt {- \left(4\, ac-{b}^{2} \right) ^{-1}} x^\beta}{\ln \left(A \right) \Gamma(\beta+1)}- \frac{5\, \sqrt {- \left(4\, ac-{b}^{2} \right) ^{-1}} t^\alpha}{2 \ln \left(A \right) \Gamma(\alpha+1)}$.

If we consider Case 3, we obtain the following sets of traveling wave solutions:

Family 16. If $Z < 0$ and $a$, $b$, $c$ are nonzero then the corresponding family of solitary wave solutions for Eq (1.2) is given as follows:

and

Family 17. If $Z > 0$ and $a$, $b$, $c$ are nonzero then the corresponding family of solitary wave solutions for Eq (1.2) is given as follows:

and

Family 18. When $ac > 0$ and $b = 0$, the corresponding family of solitary wave solutions for Eq (1.2) is given as follows:

and

Family 19. When $ac > 0$ and $b = 0$, the corresponding family of solitary wave solutions for Eq (1.2) is given as follows:

and

Family 20. If $c = a$ and $b = 0$, the corresponding family of solitary wave solutions for Eq (1.2) is given as follows:

and

Family 21. When $c = -a$ and $b = 0$, the corresponding family of solitary wave solutions for Eq (1.2) is given as follows:

and

Family 22. If $a = 0$, $b \neq 0$ and $c\neq0$, the corresponding family of solitary wave solutions for Eq (1.2) is given as follows:

and

Family 23. If $b = \lambda$, $c = n\lambda$ (where $n\neq0$) and $a = 0$, the corresponding family of solitary wave solutions for Eq (1.2) is given as follows:

where $\xi = \frac{ \left(25\, i\pm \left(\sqrt {5}\sqrt {3} \right) \right) \sqrt {5} x^\beta}{10 \sqrt {i \left(4\, ac-{b}^{2} \right) \left(25\, i\pm \left(\sqrt {5}\sqrt {3} \right) \right) } \ln \left(A \right)\Gamma(\beta+1)}$

$+{\frac { \left(25\, i\pm\left(\sqrt {5}\sqrt {3} \right) \right) \sqrt {5} \left(-739+27\, i\pm\left(\sqrt {5}\sqrt {3} \right) \right) t^\alpha}{40 \left(73+15\, i\pm \left(\sqrt {5}\sqrt {3} \right) \right) \sqrt {i \left(4\, ac-{b}^{2} \right) \left(25\, i\pm \left(\sqrt {5}\sqrt {3} \right) \right) }\ln \left(A \right) \Gamma(\alpha+1) }}$.

Assuming Case 4, we obtain the following families of solutions:

Family 24. If $Z < 0$ and $a$, $b$, $c$ are nonzero then the corresponding family of solitary wave solutions for Eq (1.2) is given as follows:

and

Family 25. If $Z > 0$ and $a$, $b$, $c$ are nonzero then the corresponding family of solitary wave solutions for Eq (1.2) is given as follows:

and

Family 26. When $ac > 0$ and $b = 0$, the corresponding family of solitary wave solutions for Eq (1.2) is given as follows:

and

Family 27. When $ac > 0$ and $b = 0$, the corresponding family of solitary wave solutions for Eq (1.2) is given as follows:

and

Family 28. If $c = a$ and $b = 0$, then the corresponding family of solitary wave solutions for Eq (1.2) is given as follows:

and

Family 29. If $c = -a$ and $b = 0$, then the corresponding family of solitary wave solutions for Eq (1.2) is given as follows:

and

Family 30. If $b = \lambda$, $a = n\lambda$ (where $n\neq0$) and $c = 0$, then the corresponding family of solitary wave solutions for Eq (1.2) is given as follows:

where $\xi = \frac{ \left(25\, i\pm \left(\sqrt {5}\sqrt {3} \right) \right) \sqrt {5} x^\beta}{10 \sqrt {i \left(4\, ac-{b}^{2} \right) \left(25\, i\pm \left(\sqrt {5}\sqrt {3} \right) \right) } \ln \left(A \right)\Gamma(\beta+1)}$

$+{\frac { \left(25\, i\pm\left(\sqrt {5}\sqrt {3} \right) \right) \sqrt {5} \left(-739+27\, i\pm\left(\sqrt {5}\sqrt {3} \right) \right) t^\alpha}{40 \left(73+15\, i\pm \left(\sqrt {5}\sqrt {3} \right) \right) \sqrt {i \left(4\, ac-{b}^{2} \right) \left(25\, i\pm \left(\sqrt {5}\sqrt {3} \right) \right) }\ln \left(A \right) \Gamma(\alpha+1) }}$.

3.2.   Problem 2

Consider the fractional gas dynamics equation given by Eq (1.3). In order to convert Eq (1.3) into a NODE, we apply the following complex transformation:

This yields

If we balance the highest order linear term $U'$ with the nonlinear term $U^2$, we obtain that $m_1 = m_2 = 1$. Substituting $m_1 = m_2 = 1$ into Eq (2.3), we can obtain a series form solution for Eq (3.138) as

By substituting Eq (3.139) into Eq (3.138), we can obtain a system of nonlinear algebraic equations by equating the coefficients of $(G\left(\xi\right))^i$ for $i = -3, ..., 0, ..., 3$ to zero. Solving this system for the unknown $d_{-1}$, $d_0$, $d_1$, $k_1$ and $k_2$ by using Maple, we obtain the following two sets of solutions:

Case 1.

Case 2.

Assuming Case 1, we can obtain the following families of solutions

Family 1. If $Z < 0$ and $a$, $b$, $c$ are nonzero then the corresponding family of solitary wave solutions for Eq (1.3) is given as follows:

and

Family 2. If $Z > 0$ and $a$, $b$, $c$ are nonzero then the corresponding family of solitary wave solutions for Eq (1.3) is given as follows:

and

Family 3. If $ac > 0$ and $b = 0$, then the corresponding family of solitary wave solutions for Eq (1.3) is given as follows:

and

Family 4. If $ac < 0$ and $b = 0$, then the corresponding family of solitary wave solutions for Eq (1.3) is given as follows:

and

Family 5. If $c = a$ and $b = 0$, then the corresponding family of solitary wave solutions for Eq (1.3) is given as follows:

and

Family 6. If $c = -a$ and $b = 0$, then the corresponding family of solitary wave solutions for Eq (1.3) is given as follows:

and

Family 7. If $b = \lambda$, $a = n\lambda(n\neq0)$, and $c = 0$, then the corresponding family of solitary wave solutions for Eq (1.3) is given as follows:

where $\xi = \frac{\sqrt {- \left(-{b}^{2}+4\, ac \right) ^{-1}} \left(\ln \left(A \right) \right) ^{-1} t^\alpha}{\Gamma(\alpha+1)}$.

By assuming Case 2, we can derive the following families of solutions:

Family 8. When $Z < 0$ and $a$, $b$, $c$ are nonzero then the corresponding family of solitary wave solutions for Eq (1.3) is given as follows:

and

Family 9. When $Z > 0$ and $a$, $b$, $c$ are nonzero then the corresponding family of solitary wave solutions for Eq (1.3) is given as follows:

and

Family 10. When $ac > 0$ and $b = 0$ then the corresponding family of solitary wave solutions for Eq (1.3) is given as follows:

and

Family 11. When $ac < 0$ and $b = 0$ then the corresponding family of solitary wave solutions for Eq (1.3) is given as follows:

and

Family 12. When $c = a$ and $b = 0$, the corresponding family of solitary wave solutions for Eq (1.3) is given as follows:

and

Family 13. When $c = -a$ and $b = 0$, the corresponding family of solitary wave solutions for Eq (1.3) is given as follows:

and

Family 14. If $a = 0$, $b\neq0$ and $c\neq0$, then the corresponding family of solitary wave solutions for Eq (1.3) is given as follows:

and

Family 15. If $b = \lambda$, $c = n\lambda \ \ (n\neq0)$ and $a = 0$, then the corresponding family of solitary wave solutions for Eq (1.3) is given as follows:

where $\xi = \frac{\sqrt {- \left(-{b}^{2}+4\, ac \right) ^{-1}} \left(\ln \left(A \right) \right) ^{-1} t^\alpha}{\Gamma(\alpha+1)}$.

4.   Discussion and graphs

We discovered solitary wave solutions for the fractional MDP and fractional gas dynamics equations by using a unique mEDAM approach in this work. Our findings contain a variety of essential features, such as periodic waves, hyperbolic waves, singular waves, singular kink waves, shock waves and solitons, among others. Periodic waves are distinguished by their consistent amplitude and wavelength oscillations that are continuous and regular. Hyperbolic waves, on the other hand, are more complicated in shape and this distinguished by steep, concave or convex profiles. A singular wave is a wave that has a singularity or a concentrated energy distribution. Kink waves, on the other hand, are distinguished by abrupt discontinuities in the wave profile. Solitons, on the other hand, are self-reinforcing solitary waves that keep their shape and speed as they travel across a medium without dispersing or losing energy.

The fundamental goal of our research was to enhance nonlinear science by introducing the revolutionary mEDAM approach, which resulted in the discovery of a slew of new solitary wave solution families for both the fractional MDP and fractional gas dynamics equations. This accomplishment not only broadens current knowledge, also goes deeper into the complexities of these mathematical models. Furthermore, our research to examine the wave behavior of these solitary waves in both models in depth and to develop significant linkages between the wave dynamics and the underlying mathematical formulations, giving insight into the fundamental interconnections that drive these systems. These combined goals have allowed our research to make substantial contributions to the understanding and practical uses of soliton waves in a variety of scientific disciplines. The relationship between these waves and the solved FPDEs is fascinating. The fractional MDP equation is a nonlinear dispersive wave equation that models complex wave propagation. The equation's fractional structure allows it to replicate waves with nonlocal interactions, making it an effective tool for modeling complex wave phenomena. The fractional gas dynamics equation, on the other hand, is a model that depicts the mobility of gas in a fluid medium.

It is critical to recognize that the fractional gas dynamics equation is time dependent but not space-dependant. As a result, while the wave profile does not change in space, it does change over time. This is due to the fractional order derivative of the equation, which creates a memory effect and allows the wave to recall information about its prior behavior. Thus, the equation may be utilized to forecast wave occurrences including long-range interactions and memory effects.

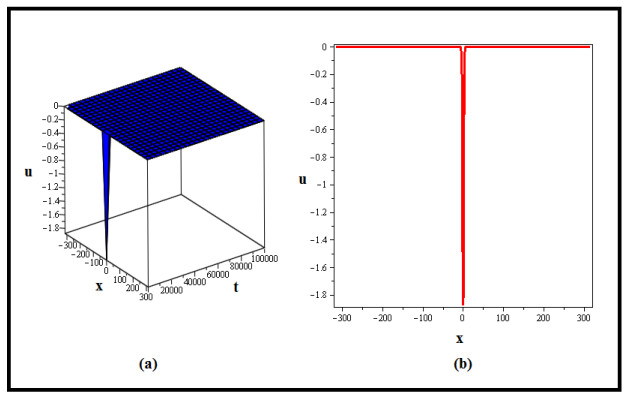
Remark 1. Figure 1 illustrates a singular kink wave profile. The fractional MDP equation is known for backing singular kink wave solutions, which are fascinating wave dynamics phenomena. These isolated kinks are caused by localized wave structures with abrupt, non-smooth characteristics. They appear in the context of the fractional MDP equation due to the interaction of nonlinearities and fractional derivatives, resulting in the development of these separate solitary waves. In this model, studying singular kink waves can provide valuable insights 1) into how fractional calculus influences wave behavior and 2) the emergence of complex localized structures in various physical systems, providing a deeper understanding of the equation's behaviour in applications such as fluid dynamics and oceanography.

Remark 2. In Figure 2, (a) depicts a singular wave (which is formed by the combination of two shock waves that propagate in opposite directions with a common asymptote) while (b) shows a singular kink wave profile. Singular waves, particularly shock waves, in gas dynamics equations provide critical insights into the behavior of compressible fluids and the propagation of disturbances. These waves, which are distinguished by sudden changes in fluid characteristics, shed light on the phenomena of gas compression and the rarefaction found in barriers or flow shifts. Their importance lies in understanding high-speed flow physics, particularly in supersonic and hypersonic contexts, through aspects such as shock wave formation, wave propagation governed by Rankine-Hugoniot relations, strength and speed determination influenced by multiple factors, energy dissipation and heat transfer mechanisms. Furthermore, precise solitary wave modelling is critical in engineering and aerospace applications to optimize designs and assure safety in high-speed transportation systems.

Similarly, within the fractional gas dynamics equation, singular kink wave solutions reflect highly localized, abrupt changes in the density or pressure profiles of compressible flows. The complicated interplay between the nonlinear components and fractional derivatives in the equation causes these peculiar bends. In conventional gas dynamics, they are akin to shock waves, but with fractional influences controlling their generation and behavior. Investigating singular kink waves in the fractional gas dynamics equation yields valuable insights 1) into how fractional calculus affects the dynamics of compressible flows and 2) the formation of sharp, non-smooth wave structures, providing a deeper understanding of wave phenomena in the context of gas dynamics, particularly in scenarios involving rarefaction waves and other complex wave interactions.

5.   Conclusions

The research resulted in the creation of the mEDAM, a ground breaking approach for generating solitary wave solutions for both the fractional MDP and fractional gas dynamics equations with Caputo's derivatives. This method uses complex transformations and series-based solutions, as well as generalized hyperbolic and trigonometric functions, to build families of solitary wave solutions. The study makes an important addition to nonlinear science, with applications ranging from fluid dynamics to plasma physics to nonlinear optics. These answers give essential insights into the behavior of soliton waves in these systems. In terms of future work, we want to adapt and apply the mEDAM approach to different FPDEs that use varied and modern derivative operators. This extension intends to improve our understanding of wave behavior across FPDEs and open up new paths for practical applications.

Use of AI tools declaration

The authors declare that they have not used Artificial Intelligence (AI) tools in the creation of this article.

Funding

This work was supported by Princess Nourah bint Abdulrahman University Researchers Supporting Project number (PNURSP2023R183), Princess Nourah bint Abdulrahman University, Riyadh, Saudi Arabia. This work was supported by the Deanship of Scientific Research, the Vice Presidency for Graduate Studies and Scientific Research, King Faisal University, Saudi Arabia (Grant No. 4271).

Acknowledgments

Princess Nourah bint Abdulrahman University Researchers Supporting Project number (PNURSP2023R183), Princess Nourah bint Abdulrahman University, Riyadh, Saudi Arabia. This work was supported by the Deanship of Scientific Research, the Vice Presidency for Graduate Studies and Scientific Research, King Faisal University, Saudi Arabia (Grant No. 4271).

Conflict of interest

The authors declare that they have no competing interests.