The goal of this work is to study the well-posedness and the asymptotic behavior of solutions of a triple beam system commonly known as the Rao-Nakra beam model. We consider the effect of Gurtin-Pipkin's thermal law on the outer layers of the beam system. Using standard semi-group theory for linear operators and the multiplier method, we establish the existence and uniqueness of weak global solution, as well as a stability result.
Citation: Soh Edwin Mukiawa. Well-posedness and stabilization of a type three layer beam system with Gurtin-Pipkin's thermal law[J]. AIMS Mathematics, 2023, 8(12): 28188-28209. doi: 10.3934/math.20231443
| [1] | Adel M. Al-Mahdi, Maher Noor, Mohammed M. Al-Gharabli, Baowei Feng, Abdelaziz Soufyane . Stability analysis for a Rao-Nakra sandwich beam equation with time-varying weights and frictional dampings. AIMS Mathematics, 2024, 9(5): 12570-12587. doi: 10.3934/math.2024615 |
| [2] | Adel M. Al-Mahdi . Long-time behavior for a nonlinear Timoshenko system: Thermal damping versus weak damping of variable-exponents type. AIMS Mathematics, 2023, 8(12): 29577-29603. doi: 10.3934/math.20231515 |
| [3] | Khaled zennir, Djamel Ouchenane, Abdelbaki Choucha, Mohamad Biomy . Well-posedness and stability for Bresse-Timoshenko type systems with thermodiffusion effects and nonlinear damping. AIMS Mathematics, 2021, 6(3): 2704-2721. doi: 10.3934/math.2021164 |
| [4] | Cyril Dennis Enyi, Soh Edwin Mukiawa . Dynamics of a thermoelastic-laminated beam problem. AIMS Mathematics, 2020, 5(5): 5261-5286. doi: 10.3934/math.2020338 |
| [5] | Yonghui Zou, Xin Xu, An Gao . Local well-posedness to the thermal boundary layer equations in Sobolev space. AIMS Mathematics, 2023, 8(4): 9933-9964. doi: 10.3934/math.2023503 |
| [6] | Tijani A. Apalara, Aminat O. Ige, Cyril D. Enyi, Mcsylvester E. Omaba . Uniform stability result of laminated beams with thermoelasticity of type Ⅲ. AIMS Mathematics, 2023, 8(1): 1090-1101. doi: 10.3934/math.2023054 |
| [7] | Said Mesloub, Hassan Altayeb Gadain, Lotfi Kasmi . On the well posedness of a mathematical model for a singular nonlinear fractional pseudo-hyperbolic system with nonlocal boundary conditions and frictional damping terms. AIMS Mathematics, 2024, 9(2): 2964-2992. doi: 10.3934/math.2024146 |
| [8] | Yellamma, N. Manjunatha, Umair Khan, Samia Elattar, Sayed M. Eldin, Jasgurpreet Singh Chohan, R. Sumithra, K. Sarada . Onset of triple-diffusive convective stability in the presence of a heat source and temperature gradients: An exact method. AIMS Mathematics, 2023, 8(6): 13432-13453. doi: 10.3934/math.2023681 |
| [9] | Noelia Bazarra, José R. Fernández, Jaime E. Muñoz-Rivera, Elena Ochoa, Ramón Quintanilla . Analytical and numerical analyses of a viscous strain gradient problem involving type Ⅱ thermoelasticity. AIMS Mathematics, 2024, 9(7): 16998-17024. doi: 10.3934/math.2024825 |
| [10] | Osama Moaaz, Ahmed E. Abouelregal, Fahad Alsharari . Lateral vibration of an axially moving thermoelastic nanobeam subjected to an external transverse excitation. AIMS Mathematics, 2023, 8(1): 2272-2295. doi: 10.3934/math.2023118 |
The goal of this work is to study the well-posedness and the asymptotic behavior of solutions of a triple beam system commonly known as the Rao-Nakra beam model. We consider the effect of Gurtin-Pipkin's thermal law on the outer layers of the beam system. Using standard semi-group theory for linear operators and the multiplier method, we establish the existence and uniqueness of weak global solution, as well as a stability result.
In the present work, we consider the Rao-Nakra (three layer) beam system, where the top and the bottom layers of the beam are subjected to Gurtin-Pipkin's thermal law, namely
| {ρ1h1utt−E1h1uxx−k(−u+v+αwx)+δ1θx=0, in (0,π)×R+,ρ3h3vtt−E3h3vxx+k(−u+v+αwx)−δ1θ+δ2ϑx=0, in (0,π)×R+,ρhwtt+EIwxxxx−αk(−u+v+αwx)x+δ3wt=0, in (0,π)×R+,ρ4θt−β1∫+∞0g1(s)θxx(x,t−s)ds+δ1(uxt+vt)=0, in (0,π)×R+,ρ5ϑt−β2∫+∞0g2(s)ϑxx(x,t−s)ds+δ2vxt=0, in (0,π)×R+ | (1.1) |
with the following boundary conditions:
| {ux(0,t)=vx(0,t)=w(0,t)=wxx(0,t)=θ(0,t)=ϑ(0,t)=0, t≥0,u(π,t)=v(π,t)=w(π,t)=wxx(π,t)=θx(π,t)=ϑx(π,t)=0, t≥0, | (1.2) |
and the initial data
| {u(x,0)=u0(x), v(x,0)=v0(x), w(x,0)=w0(x),x∈(0,π),ut(x,0)=u1(x), vt(x,0)=v1(x), wt(x,0)=w1(x),x∈(0,π),θ(x,−t)=θ0(x,t),ϑ(x,−t)=ϑ0(x,t),x∈(0,π), t>0. | (1.3) |
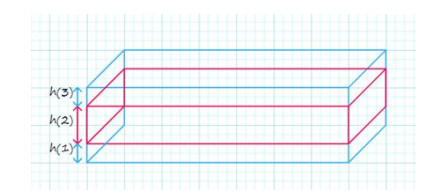
The relaxation functions g1 and g2 are positive non-increasing functions to be specified later. The stabilization of Rao-Nakra beam systems has gathered much interest from researchers recently, and a great number of results have been established. The Rao-Nakra beam model is a beam system that takes into account the motion of two outer face plates (assumed to be relatively stiff) and a sandwiched compliant inner core layer, see [1,2,3,4,5] for Rao-Nakra, Mead-Markus and multilayer plates or sandwich models. The basic equations of motion of the Rao-Nakra model are derived thanks to the Euler-Bernoulli beam assumptions for the outer face plate layers, the Timoshenko beam assumptions for the sandwich layer and a "no slip" assumption for the motion along the interface. Suppose h(j), j=1,2,3 is the thickness of each layer in the beam of length π, see Figure 1 and h=h(1)+h(2)+h(3) the total thickness of the beam.
Assuming the Kirchhoff hypothesis is imposed on the outer layers of beam and in addition, there is a continuous, piecewise linear displacements through the cross-sections, Liu et al. [6] gave a detailed derivation of following laminated beam system:
| {ρ1h1utt−E1h1uxx−τ=0,ρ1I1y1tt−E1I1y1xx−h12τ+G1h1(wx+y1)=0,ρhwtt+EIwxxxx−G1h1k(wx+y1)x−G3h3(wx+y3)x−h2τx=0,ρ3h3vtt−E3h3vxx+τ=0,ρ3I3y3tt−E3I3y3xx−h32τ+G3h3(wx+y3)=0, | (1.4) |
where x∈(0,π),t>0, (u,y1),(v,y3) represent longitudinal displacement and shear angle of the bottom and top layers plates. The transverse displacement of the beam is represented by w, and τ is the shear stress of the core layer. Also, for j=1,2,3 (from bottom to top layer), Ej,Gj,Ij,ρj>0 are Young's modulus, shear modulus, moments of inertia and density respectively for each layer. Moreover, in (1.4)3, we have that ρh=ρ1h1+ρ2h2+ρ3h3 and EI=E1I1+E3I3. By neglecting the rotary inertia in top and bottom layers of the beam, we obtain ρ1I1=ρ3I3=0 in (1.4)4 and (1.4)5. Furthermore, if we neglect the transverse shear, this leads to the Euler-Bernoulli hypothesis wx+y1=wx+y3=0. Assuming that the core layer consists of a material that is linearly elastic with the stress-strain relationship τ=2G2ε, where the shear strain ε is defined by
| ε=12h2(−u+v+αwx) where α=h2+h1+h22. |
Thus, we arrive at the following Rao-Nakra beam model [1], given by
| {ρ1h1utt−E1h1uxx−k(−u+v+αwx)=0,ρ3h3vtt−E3h3vxx+k(−u+v+αwx)=0,ρhwtt+EIwxxxx−αk(−u+v+αwx)x=0, | (1.5) |
where k=G2h2, G2=E22(1+ν) and −1<ν<12 is the Poisson ratio. Furthermore, when the extensional motion of the outer layers is neglected, system (1.4) takes the form of the two-layer laminated beam system derived by Hansen and Spies [7]. Li et al. [8] showed that system (1.5) is unstable if only one of the equations is damped. When two of the three equations in (1.5) were damped, the authors in [8] proved a polynomial stability. For recent results in literature, Méndez et al. [9] considered (1.5) with with Kelvin-Voigt damping and studied the well-posedness, lack of exponential decay and polynomial decay. Feng and Özer [10] looked at a nonlinearly damped Rao-Nakra beam system and established the global attractor with finite fractal dimension. Feng et al. [11] studied the stability of Rao-Nakra sandwich beam with time-varying weight and time-varying delay. Mukiawa et al. [12] considered (1.5) with viscoelastic damping on the first equation and heat conduction govern by Fourier's law and proved the well-posedness and a general decay result. Also, Raposo et al. [13] coupled (1.5) with Maxwell-Cattaneo heat conduction established the well-posedness. For more results related Rao-Nakra beam system with frictional, delay or thermal damping, see [14,15,16,17,18,19,20] and the references therein.
An interesting tool used by Mathematician in stabilizing beam models such as the Laminated and Timoshenko beam systems is the Gurtin-Pipkin's thermal law, see [21], with constitutive equation
| βq(t)+∫∞0g(s)θx(x,t−s)ds=0, | (1.6) |
where θ=θ(x,t) is the temperature difference, q=q(x,t) is the heat flux, β is a coupling constant coefficient and the relaxation g is a summable convex L1([0,+∞)) function with unit mass. For results related to (1.6), Dell'Oro and Pata [22] studied
| {ρ1utt−k(ux+v)x=0, in (0,π)×R+,ρ2vtt−bvxx+k(ux+v)+δθx=0, in (0,π)×R+,ρ3θt−1β∫∞0h(s)θxx(x,t−s)ds+δvxt=0, in (0,π)×R+ | (1.7) |
and proved an exponential stability result if and only if χh=0, where
| χh=(ρ1kρ3−βh(0))(ρ1k−ρ2b)−βh(0)ρ1δ2kbρ3. |
For similar results with Gurtin-Pipkin's thermal law, see [23,24,25,26,27,28] and references therein. As clearly elaborated in [22], the Fourier's and Cattaneo's (second sound) thermal law can be recovered from (1.6) by defining the memory function g in (1.6) as
| gδ(s)=1δh(sδ), δ>0 | (1.8) |
and
| gτ(s)=βτe−sβτ, τ>0 | (1.9) |
respectively. A closely related thermal law to the Gurtin-Pipkin's thermal law is the Coleman-Gurtin's heat conduction law, see [29], with constitutive equation given by
| βq(t)+(1−η)θx+η∫∞0μ(s)θx(x,t−s)ds=0, η∈(0,1), | (1.10) |
where η=1 and η=0 correspond to the Gurtin-Pipkin's and Fourier thermal laws, respectively. This entails replacing (1.7)3 with
| ρ3θt−(1−η)βθxx−ηβ∫∞0μ(s)θxx(x,t−s)ds+δvxt=0, in (0,π)×R+. | (1.11) |
We should note here that systems govern by Coleman-Gurtin's thermal lawa (1.10) gain additional dissipation from the term −(1−η)βθxx and thus less difficult to handle compare to systems with Gurtin-Pipkin's thermal law (1.6).
Our main focus of this paper is to investigate the well-posedness and the asymptotic behavior of solutions of system (1.1)–(1.3). We mote here that, the rotational inertia term wxxtt which should be in (1.1)3 of the original models is neglected in the present model. However, the result in this paper is not affected by the absent of this term. Also, since the thermal coupling in system (1.1)–(1.3) is not strong enough to achieve exponential stability, a viscous damping term wt is added to (1.1)3. The rest of work is organized as follows: In Section 2, we state some assumptions and set up our problem (1.1)–(1.3) in appropriate spaces. In Section 3, we prove the existence and uniqueness result for the system (1.1)–(1.3). In Section 4, we study the asymptotic behavior of solution of system (1.1)–(1.3).
For the relaxation functions g1 and g2, we assume the following:
Assumption (A0):
(a0) g1,g2:[0,+∞)⟶(0,+∞) are non-increasing C2([0,+∞)) and convex summable functions satisfying
| lims→+∞gi(s)=0 and ∫+∞0gi(s)ds=1, i=1,2. | (2.1) |
(b0) There exists ξi>0, i=1,2 such that
| −gi″ | (2.2) |
By setting
| \begin{equation} \mu_1(s) = -g_1'(s) \ {\rm and}\ \mu_2(s) = -g_2'(s), \end{equation} | (2.3) |
assumption (A_0) ensues the following:
Assumption (A_1) :
( a_1 ) \mu_1, \mu_2:[0, +\infty)\longrightarrow (0, +\infty) are non-increasing C^1([0, +\infty)) and convex summable functions satisfying
| \begin{equation} \mu_{0i} = \int_0^{+\infty}\mu_i(s)ds = g_i(0) > 0, \ {\rm and}\ \int_0^{+\infty}s\mu_i(s)ds = 1, \ i = 1, 2. \end{equation} | (2.4) |
( b_1 ) There exists \xi_i > 0, \ i = 1, 2 such that
| \begin{equation} \mu_i'(s)\leq-\xi_i \mu_i(s), \ \forall s\geq 0, \ i = 1, 2. \end{equation} | (2.5) |
Due to the work of Dafermos [30], we define new functions for the relative past history of \theta and \vartheta as follows:
| \begin{equation} \nonumber \sigma, \zeta: (0, \pi)\times \mathbb{R}_{+}\times \mathbb{R}_{+}\rightarrow \mathbb{R}_{+}, \end{equation} |
define by
| \begin{equation} \sigma = \sigma(x, t, s): = \int_{t-s}^t\theta(x, r)dr\ \ {\rm and}\ \zeta = \zeta(x, t, s): = \int_{t-s}^t\vartheta(x, r)dr. \end{equation} | (2.6) |
On account of the boundary conditions (1.2), we have
| \sigma(0, t, s) = \sigma_x(\pi, t, s) = \zeta(0, t, s) = \zeta_x(\pi, t, s) = 0, |
and routine calculation gives
| \begin{equation} \left\{ \begin{split} &\sigma_t +\sigma_s-\theta = 0, \ & {\rm in}\ (0, \pi)\times (\mathbb{R}_{+}\times \mathbb{R}_{+}, \\ & \zeta_t +\zeta_s-\vartheta = 0, \ & {\rm in}\ (0, \pi)\times \mathbb{R}_{+}\times \mathbb{R}_{+}, \\ &\sigma(x, t, 0) = \zeta(x, t, 0) = 0, \ & {\rm in}\ (0, \pi)\times \mathbb{R}_{+}, \\ &\sigma(x, 0, s) = \int_0^s\theta_0(x, r)dr: = \sigma_0(x, s), \ & {\rm in}\ (0, \pi)\times \mathbb{R}_{+}, \\ & \zeta(x, 0, s) = \int_0^s\vartheta_0(x, r)dr: = \zeta_0(x, s), \ & {\rm in}\ (0, \pi)\times \mathbb{R}_{+}, \end{split} \right. \end{equation} | (2.7) |
where \sigma_0 and \zeta_0 represent the history of \theta and \vartheta respectively. Also, using direct computations, we have
| \begin{equation} \begin{aligned} &\int_0^{+\infty}g_1(s) \theta_{xx}(x, t-s)ds \\ = &\lim\limits_{a\rightarrow +\infty}g_1(s)\int_{t-s}^t\theta_{xx}(x, r)dr \bigg|_{s = 0}^{s = a}- \int_0^{+\infty}g_1'(s)\int_{t-s}^t\theta_{xx}(x, r)drds \\ = & \int_0^{+\infty}\mu_1(s)\sigma_{xx}(x, t, s)ds. \end{aligned} \end{equation} | (2.8) |
Similarly, we get
| \begin{equation} \begin{aligned} \int_0^{+\infty}g_2(s)&\vartheta_{xx}(x, t-s)ds = \int_0^{+\infty}\mu_2(s)\zeta_{xx}(x, t, s)ds. \end{aligned} \end{equation} | (2.9) |
On account of (2.6)–(2.9), system (1.1)–(1.3) takes the form
| \begin{equation} \left\{ \begin{split} &\rho_1h_1 u_{tt} -E_1h_1u_{xx} -k(-u+v+\alpha w_x)+\delta_1\theta_x = 0, &{\rm in} \ (0, \pi)\times \mathbb{R}_{+}, \\ &\rho_3h_3 v_{tt} -E_3h_3v_{xx} +k(-u+v+\alpha w_x)-\delta_1\theta+\delta_2 \vartheta_x = 0, &{\rm in} \ (0, \pi)\times \mathbb{R}_{+}, \\ &\rho h w_{tt} +EIw_{xxxx}-\alpha k(-u+v+\alpha w_x)_x + \delta_3 w_t = 0, & {\rm in} \ (0, \pi)\times \mathbb{R}_{+}, \\ &\rho_4\theta_{t} -\beta_1\int_0^{+\infty}\mu_1(s)\sigma_{xx}(x, t, s)ds+\delta_1(u_{xt}+v_t) = 0, & {\rm in} \ (0, \pi)\times \mathbb{R}_{+}, \\ &\sigma_t +\sigma_s-\theta = 0, & {\rm in}\ (0, \pi)\times \mathbb{R}_{+}\times \mathbb{R}_{+}, \\ &\rho_5\vartheta_{t} -\beta_2\int_0^{+\infty}\mu_2(s)\zeta_{xx}(x, t, s)ds+\delta_2v_{xt} = 0, & {\rm in} \ (0, \pi)\times \mathbb{R}_{+}, \\ &\zeta_t +\zeta_s-\vartheta = 0, & {\rm in}\ (0, \pi)\times \mathbb{R}_{+}\times \mathbb{R}_{+} \end{split} \right. \end{equation} | (2.10) |
with the boundary conditions
| \begin{equation} \left\{ \begin{split} &u_x(0, t) = v_x(0, t) = w(0, t) = w_{xx}(0, t) = \theta(0, t) = \vartheta(0, t), \ & t\geq 0, \\ &u(\pi, t) = v(\pi, t) = w(\pi, t) = w_{xx}(\pi, t) = \theta_x(\pi, t) = \vartheta_x(\pi, t) = 0, \ & t\geq 0, \\ &\sigma(0, t, s) = \sigma_x(\pi, t, s) = \zeta(0, t, s) = \zeta_x(\pi, t, s) = 0, \ & s, t\in\mathbb{R}_{+}, \\ &\sigma(x, t, 0) = \zeta(x, t, 0) = 0, \ & x\in (0, \pi), t\in\mathbb{R}_{+}\\ \end{split} \right. \end{equation} | (2.11) |
and the initial data
| \begin{equation} \left\{ \begin{split} & u(x, 0) = u_0(x), \ v(x, 0) = v_0(x), \ w(x, 0) = w_0(x), & x\in (0, \pi), \\ &u_t(x, 0) = u_1(x), \ v_t(x, 0) = v_1(x), \ w_t(x, 0) = w_1(x), & x\in (0, \pi), \\ &\theta(x, -t) = \theta_0(x, t), \vartheta(x, -t) = \vartheta_0(x, t) & x\in (0, \pi), \ t > 0, \\ &\sigma(x, 0, s) = \sigma_0(x, s), \ \zeta(x, 0, s) = \zeta_0(x, s), &\ x\in (0, \pi), s > 0. \end{split} \right. \end{equation} | (2.12) |
Setting \Psi = (u, \varphi, v, \psi, w, \phi, \theta, \sigma, \vartheta, \zeta)^T , with \varphi = u_t , \psi = v_t and \phi = w_t . Then, the semi-group formulation of system (2.10)–(2.12) is given by the Cauchy problem
| \begin{equation} (P)\begin{cases} & \Psi_t+\mathcal{A}\Psi = 0, \\ & \Psi(0) = \Psi_0, \end{cases} \end{equation} | (2.13) |
where \Psi_0 = (u_0, u_1, v_0, v_1, w_0, w_1, \theta_0, \sigma_0, \vartheta_0, \zeta_0)^T and the linear operator \mathcal{A} is defined by
| \mathcal{A}\Psi = \begin{pmatrix} -\varphi\\ \\ -\dfrac{E_1}{\rho_1}u_{xx}-\dfrac{k}{\rho_1h_1}(-u+v+\alpha w_x)+\dfrac{\delta_1}{\rho_1h_1}\theta_x\\ \\ -\psi\\ -\dfrac{E_3}{\rho_3}v_{xx} +\dfrac{k}{\rho_3h_3}(-u+v+\alpha w_x)-\frac{\delta_1}{\rho_3h_3}\theta+\frac{\delta_2}{\rho_3h_3}\vartheta_x\\ -\phi\\ \\ \dfrac{EI}{\rho h}w_{xxxx}-\dfrac{\alpha k}{\rho h}(-u+v+\alpha w_x)_x+\dfrac{\delta_3}{\rho h}\phi\\ \\ -\frac{\beta_1}{\rho_4} \int_0^{+\infty}\mu_1(s)\sigma_{xx}(x, s)ds+ \dfrac{\delta_1}{\rho_4}\left( \varphi_x+\psi\right) \\ \\ \sigma_s-\theta\\ \\ -\frac{\beta_2}{\rho_5} \int_0^{+\infty}\mu_2(s)\zeta_{xx}(x, s)ds+ \dfrac{\delta_2}{\rho_5}\psi_x\\ \\ \zeta_s-\vartheta \end{pmatrix}. |
Let \langle, \rangle and \|.\| denote the inner product and the norm in L^2(0, \pi) respectively and we define following Sobolev spaces:
| \begin{align*} H^1_a: = &\{\varpi\in H^1(0, \pi)/ \varpi(0) = 0\}, \ H^1_b: = \{\varpi\in H^1(0, \pi)/ \varpi(\pi) = 0\}, \\ H^2_a: = &\{\varpi\in H^2(0, \pi)/ \varpi_x\in H^1_a\}, \ H^2_b: = \{\varpi\in H^2(0, \pi)/ \varpi_x\in H^1_b\}, \\ H^2_* : = & H^2(0, \pi)\cap H^1_0(0, \pi), \end{align*} |
where H^2_* is equip with the inner product
| \langle\varpi, \hat{\varpi} \rangle_{H^2_*} = \langle \varpi_{xx}, \hat{\varpi}_{xx}\rangle |
and norm
| \|\varpi\|^2_{H^2_* } = \|\varpi_{xx}\|^2. |
It is easy to check that (H^2_*, \|.\|^2_{H^2_*}) is a Banach space and the norm \|.\|^2_{H^2_* } is equivalent to the usual norm in H^2(0, \pi). Next, we introduce the weighted-Hilbert space of H_a^{1}(0, \pi) -real valued functions on (0, +\infty) by
| \begin{align*} &L^2_{\mu}: = L^2_{\mu}\left( \mathbb{R}_{+};H_a^{1}(0, \pi)\right), \end{align*} |
where
| \begin{align*} &L^2_{\mu}\left( \mathbb{R}_{+};H_a^{1}(0, \pi)\right) = \left\lbrace \varpi: \mathbb{R}_{+}\longrightarrow H_a^{1}(0, \pi) / \int_0^{+\infty} \mu(s)\|\varpi_{x}(s)\|^2ds < \infty\right\rbrace, \end{align*} |
and equip them with the inner product
| \begin{align*} &\left(\varpi, \hat{\varpi}\right)_{L^2_{\mu}}: = \int_0^{+\infty} \mu(s)\langle\varpi_{x}(s), \hat{\varpi}_{x}(s)\rangle ds, \end{align*} |
and norm
| \begin{align*} &\|\varpi\|^2_{L^2_{\mu}} = \int_0^{+\infty} \mu(s)\|\varpi_{x}(s)\|^2ds. \end{align*} |
Also, we define
| \begin{equation*} \mathcal{D}\left(L^2_{\mu}\right) : = \left\lbrace \varpi\in L^2_{\mu} / \varpi_s\in L^2_{\mu}\ \ {\rm and}\ \lim\limits_{s\rightarrow 0}\|\varpi_x(s)\| = 0\right\rbrace. \end{equation*} |
Now, we introduce the phase space of our problem given by
| \begin{equation*} \mathcal{H}: = H^1_b\times L^2 \times H^1_b\times L^2\times H^2_*\times L^2\times L^2\times L^2_{\mu_1}\times L^2\times L^2_{\mu_2} \end{equation*} |
and equipped it with the inner product
| \begin{align*} &\langle (u, \varphi, v, \psi, w, \phi, \theta, \sigma, \vartheta, \zeta), (\hat{u}, \hat{\varphi}, \hat{ v}, \hat{\psi}, \hat{w}, \hat{\phi}, \hat{\theta}, \hat{\sigma}, \hat{\vartheta}, \hat{\zeta})\rangle_{\mathcal{H}}\\ : = & E_1h_1 \langle u_x, \hat{u}_x\rangle + \rho_1h_1\langle \varphi, \hat{\varphi}\rangle + k \langle (-u+v+\alpha w_x), (-\hat{u}+\hat{v}+\alpha\hat{w}_x)\rangle\\ & + E_3h_3 \langle v_x, \hat{v}_x\rangle + \rho_3h_3\langle \psi, \hat{\psi}\rangle + EI\langle w_{xx}, \hat{w}_{xx}\rangle + \rho h \langle \phi, \hat{\phi}\rangle +\rho_4\langle \theta, \hat{\theta}\rangle\\ &+\beta_1\langle \sigma, \hat{\sigma}\rangle_{L^2_{\mu_1}} + \rho_5\langle \vartheta, \hat{\vartheta}\rangle+ \beta_2\langle \zeta, \hat{\zeta}\rangle_{L^2_{\mu_2}} \end{align*} |
and norm
| \begin{align*} \|\Psi\|^2_{\mathcal{H}} = & \| (u, \varphi, v, \psi, w, \phi, \theta, \sigma, \vartheta, \zeta)\|^2_{\mathcal{H}}\\ : = & E_1h_1 \|u_x\|^2+ \rho_1h_1\|\varphi\|^2+ k\|(-u+v+\alpha w_x)\|^2 \\ &+ E_3h_3\|v_x\|^2+ \rho_3h_3\|\psi\|^2 +EI\|w_{xx}\|^2 +\rho h\|\phi\|^2 \\ &+\rho_4\|\theta\|^2 +\beta_1\|\sigma\|^2_{L^2_{\mu_1}}+\rho_5\|\vartheta\|^2 +\beta_2 \|\zeta\|^2_{L^2_{\mu_2}}, \end{align*} |
for any \Phi = (w, \varphi, v, \psi, u, \phi, \theta, \sigma, \vartheta, \zeta)^T \in \mathcal{H} .
The domain of the linear operator \mathcal{A} in (2.13) is defined as follows:
| \begin{equation*} \mathcal{D}(\mathcal{A}): = \left\{(u, \varphi, v, \psi, w, \phi, \theta, \sigma, \vartheta, \zeta) \in \mathcal{H}\left| \begin{split} &u, v\in H^2_b\cap H^1_b, \ \varphi, \psi \in H^1_b, \\ & w\in H^4\cap H^2_*, \ \phi\in H^2_*, \\ & \sigma\in \mathcal{D}\left(L^2_{\mu_1}\right), \ \theta\in H^1_a, \\ & \zeta\in \mathcal{D}\left(L^2_{\mu_2}\right), \ \vartheta \in H^1_a, \\ & (-u+v+\alpha w_x)\in H^1_a\cap H^1_b, \\ & \int_0^{+\infty} \mu_1(s)\sigma(s) ds\in H^2\cap H^1_a, \\ &\int_0^{+\infty} \mu_2(s)\zeta(s) ds\in H^2\cap H^1_a, \\ &w_{xx}(0) = w_{xx}(\pi) = 0. \end{split}\right.\right\}. \end{equation*} |
Remark 2.1. (1) Due to (2.5), we can deduce that
| \begin{equation} \langle -\varpi_s, \varpi\rangle_{L^2_{\mu_i}} \leq -\frac{\xi_i}{2}\|\varpi\|^2_{L^2_{\mu_i}}, \ \ \forall\ \varpi\in \mathcal{D}\left(L^2_{\mu_i}\right), \ i = 1, 2. \end{equation} | (2.14) |
(2) Using Hölder's and Young's inequalities, we have that
| \begin{equation} \int_0^{+\infty}\mu_i(s)\|\varpi_{x}(s)\| ds\leq \sqrt{g_i(0)}\|\varpi\|_{L^2_{\mu_i}}, \ i = 1, 2. \end{equation} | (2.15) |
In this section, we establish the existence and uniqueness of global weak solution to the system (2.10)–(2.12).
Lemma 3.1. The linear operator \mathcal{A}:\mathcal{D}(\mathcal{A})\subset\mathcal{H}\to \mathcal{H} defined in (2.13) is monotone.
Proof. Let \Psi = (u, \varphi, v, \psi, w, \phi, \theta, \sigma, \vartheta, \zeta) \in\mathcal{D}(\mathcal{A}) , then using integration by parts and the boundary conditions (2.11), we have
| \begin{align*} \langle \mathcal{A}\Psi, \Psi\rangle_{\mathcal{H}} = &\delta_3\|\phi\|^2+ \beta_1 \int_0^{+\infty}\mu_1(s)\langle \sigma_{xs}(s), \sigma_{x}(s)\rangle ds + \beta_2 \int_0^{+\infty}\mu_2(s)\langle \zeta_{xs}(s), \zeta_{x}(s)\rangle ds \\ = & \delta_3\|\phi\|^2 +\frac{\beta_1}{2} \int_0^{+\infty}\mu_1(s)\frac{d}{ds}\left(\|\sigma_{x}(s)\|^2\right) ds +\frac{\beta_2}{2} \int_0^{+\infty}\mu_2(s)\frac{d}{ds}\left(\|\zeta_{x}(s)\|^2\right) ds\\ = & \delta_3\|\phi\|^2- \frac{\beta_1}{2} \int_0^{+\infty}\mu_1'(s)\|\sigma_{x}(s)\|^2 ds + \frac{\beta_1}{2}\lim\limits_{a\rightarrow +\infty}\mu_1(s)\|\sigma_{x}(s)\|^2\bigg\vert_{s = 0}^{s = a}\\ &- \frac{\beta_2}{2} \int_0^{+\infty}\mu_2'(s)\|\zeta_{x}(s)\|^2 ds + \frac{\beta_2}{2}\lim\limits_{a\rightarrow +\infty}\mu_2(s)\|\zeta_{x}(s)\|^2\bigg\vert_{s = 0}^{s = a} . \end{align*} |
From (2.5) and (2.6), we obtain
| \lim\limits_{a\rightarrow +\infty}\mu_1(s)\|\sigma_{x}(s)\|^2\bigg\vert_{s = 0}^{s = a} = \lim\limits_{a\rightarrow +\infty}\mu_2(s)\|\zeta_{x}(s)\|^2\bigg\vert_{s = 0}^{s = a} = 0. |
Therefore,
| \begin{align*} \langle \mathcal{A}\Psi, \Psi\rangle_{\mathcal{H}} = &\delta_3\|\phi\|^2- \frac{\beta_1}{2} \int_0^{+\infty}\mu_1'(s)\|\sigma_{x}(s)\|^2 ds - \frac{\beta_2}{2} \int_0^{+\infty}\mu_2'(s)\|\zeta_{x}(s)\|^2 ds\geq 0. \end{align*} |
Therefore, \mathcal{A} is monotone.
Lemma 3.2. The linear operator \mathcal{A}:\mathcal{D}(\mathcal{A})\subset\mathcal{H}\to \mathcal{H} defined in (2.13) maximal, that is \mathfrak{R}(I+\mathcal{A}) = \mathcal{H}.
Proof. Given F = (k^1, k^2, k^3, k^4, k^5, k^6, k^7, k^8, k^9, k^{10})\in\mathcal{H}, we look for a unique solution
| \Psi = (u, \varphi, v, \psi, w, \phi, \theta, \sigma, \vartheta, \zeta) \in\mathcal{D}(\mathcal{A}) |
such that \Psi solves the stationary problem
| \begin{equation} \Psi+\mathcal{A}\Psi = F. \end{equation} | (3.1) |
System (3.1) is equivalent to
| \begin{equation} \begin{cases} \begin{aligned} & u-\varphi = k^1, &\ {\rm in}\ H^1_b, \\ &\rho_1h_1\varphi- E_1h_1 u_{xx}-k (-u+v+\alpha w_x)+\delta_1\theta_x = \rho_1h_1 k^2, & \ {\rm in}\ L^2, \\ &v-\psi = k^3, &\ {\rm in}\ H^1_b, \\ &\rho_3 h_3 \psi-E_3 h_3 v_{xx} + k (-u+v+\alpha w_x)-\delta_1\theta+\delta_2\vartheta_x = \rho_3 h_3 k^4, &\ {\rm in}\ L^2, \\ &w-\phi = k^5, &\ {\rm in}\ H^2_*, \\ &(\rho h+\delta_3)\phi+ EIw_{xxxx}- \alpha k(-u+v+\alpha w_x)_x = \rho h k^6, &\ {\rm in}\ L^2, \\ &\rho_4 \theta-\beta_1 \int_0^{+\infty}\mu_1(s)\sigma_{xx}(x, s)ds+ \delta_1\left( \varphi_x+\psi\right) = \rho_4 k^7, &\ {\rm in}\ L^2, \\ &\sigma+\sigma_s-\theta = k^8, &\ {\rm in}\ L^2_{\mu_1}\\ &\rho_5\vartheta-\beta_2 \int_0^{+\infty}\mu_2(s)\zeta_{xx}(x, s)ds+ \delta_2 \psi_x = \rho_5 k^9, &\ {\rm in}\ L^2, \\ &\zeta+\zeta_s-\vartheta = k^{10}, &\ {\rm in}\ L^2_{\mu_2}. \end{aligned} \end{cases} \end{equation} | (3.2) |
By multiplying (3.2)_8 and (3.2)_{10} by e^{r} and integrating the results over (0, s), we arrive at
| \begin{equation} \begin{aligned} &\sigma(s) = \left( 1-e^{-s}\right)\theta + \int_0^s e^{r-s}k^8(r)dr, \\ &\zeta(s) = \left( 1-e^{-s}\right)\vartheta+ \int_0^s e^{r-s}k^{10}(r)dr. \end{aligned} \end{equation} | (3.3) |
From (3.2)_1 , (3.2)_3 and (3.2)_5 , we get
| \begin{equation} u-k^1 = \varphi, \ \ v-k^3 = \psi \ {\rm and}\ \ w-k^5 = \phi, \end{equation} | (3.4) |
respectively. Substituting (3.4) and (3.3) into (3.2)_2 , (3.2)_4, (3.2)_6, (3.2)_7 and (3.2)_9 leads to
| \begin{equation} \begin{cases} \begin{aligned} &\rho_1h_1u- E_1h_1 u_{xx}-k (-u+v+\alpha w_x)+\delta_1\theta_x = \underbrace{\rho_1h_1(k^1+ k^2)}_{f^1}, & \ {\rm in}\ L^2, \\ &\rho_3 h_3 v-E_3 h_3 v_{xx} + k (-u+v+\alpha w_x)-\delta_1\theta+\delta_2\vartheta_x = \underbrace{\rho_3 h_3 (k^3+k^4)}_{f^2}, &\ {\rm in}\ L^2, \\ &\rho h w+ EIw_{xxxx}- \alpha k(-u+v+\alpha w_x)_x = \underbrace{\delta_3 k^5+\rho h (k^5+k^6)}_{f^3}, &\ {\rm in}\ L^2, \\ &\rho_4 \theta-C_{\beta_1, \mu_1}\theta_{xx}+ \delta_1\left( u_x+v\right) \\ = &\underbrace{\delta_1\left( k^1_x+k^3\right) +\rho_4 k^7+\beta_1\int_0^{+\infty}\mu_1(s)\left(\int_0^s e^{r-s}k_{xx}^8(r)dr \right)ds}_{f^4}, &\ {\rm in}\ H^{-1}, \\ &\rho_5\vartheta-C_{\beta_2, \mu_2}\vartheta_{xx}+ \delta_2v_x\\ = &\underbrace{\delta_2k_x^3+\rho_5 k^9+\beta_2\int_0^{+\infty}\mu_2(s)\left(\int_0^s e^{r-s}k_{xx}^{10}(r)dr \right)ds}_{f^5}, &\ {\rm in}\ H^{-1}, \end{aligned} \end{cases} \end{equation} | (3.5) |
where
| C_{\beta_i, \mu_i} = \beta_i \int_0^{+\infty} \mu_i(s)\left(1-e^{-s} \right)ds > 0, \ i = 1, 2. |
Now, we observe that last terms in f^4 and f^ 5 are in H^{-1}(0, \pi) . Indeed, since k^8\in L^2_{\mu_1} , we have for any
| \varpi\in H^{1}_a(0, \pi), \ {\rm with} \ \|\varpi_x\|\leq 1, |
that
| \begin{align*} \left|\langle \int_0^{+\infty} \mu_1(s)\left(\int_0^s e^{r-s}k_{xx}^8(r)dr\right)ds, \varpi \rangle \right| = &\left|\langle \int_0^{+\infty} \mu_1(s)\left(\int_0^s e^{r-s}k_{x}^8(r)dr\right)ds, \varpi_x \rangle \right|\\ \leq& \int_0^{+\infty} \mu_1(s)e^{-s}\left(\int_0^s e^{r}\|k_{x}^8(r)\| dr\right)ds \\ = &\int_0^{+\infty} e^{r}\|k_{x}^8(r)\| \left(\int_r^{+\infty} e^{-s} \mu_1(s) ds\right)dr\\ = &\leq \int_0^{+\infty}\mu_1(r) e^{r}\|k_{x}^8(r)\| \int_r^{+\infty} e^{-s} ds dr\\ = &\int_0^{+\infty}\mu_1(r) \|k_{x}^8(r)\| dr < \infty. \end{align*} |
In the same way, we get that
| \int_0^{+\infty}\mu_2(s)\left(\int_0^s e^{r-s}k_{xx}^{10}(r)dr \right)ds \in H^{-1}(0, \pi). |
Next, we consider the Banach space \mathbb{H}: = H^1_b \times H^1_b \times H^2_*\times L^2 \times L^2 and equip it with the norm
| \begin{align*} \|(u, v, w, \theta, \vartheta)\|^2_{\mathbb{H}} = &\rho_1h_1\|u\|^2 +E_1h_1\|u_x\|^2+ k \|(-u+v+ \alpha w_x)\|^2+\rho_3 h_3\|v\|^2+E_3 h_3\|v_x\|^2\\ &+\rho h\|w\|^2 + EI\|w _{xx}\|^2+\rho_4\|\theta\|^2+\rho_5\|\vartheta\|^2. \end{align*} |
On the account of the weak formulation of (3.5), we consider the bilinear form \mathcal{B} on \mathbb{H}\times\mathbb{H} and linear form \mathcal{L} on \mathbb{H} , define as follows:
| \begin{align*} &\mathcal{B}((u, v, w, \theta, \vartheta), (u^*, v^*, w^*, \theta^*, \vartheta^*))\\ \quad : = &\rho_1h_1\langle u, u^*\rangle +E_1h_1\langle u_{x}, u^*_x\rangle +k\langle (-u+v+ \alpha w_x), (-u^*+v^*+\alpha w^*_x)\rangle \\ &+\rho_3 h_3\langle v, v^*\rangle +E_3 h_3\langle v_x, v^*_x\rangle +\rho h\langle w, w^*\rangle+ EI\langle w_{xx}, w_{xx}^* \rangle \\ &+\rho_4\langle\theta, \theta^* \rangle+ C_{\eta, \beta_1, \mu_1}\langle\theta_x, \theta_x^*\rangle +\rho_5\langle\vartheta, \vartheta^* \rangle + C_{\eta, \beta_2, \mu_2}\langle \vartheta_x, \vartheta_x^* \rangle, \end{align*} |
and
| \begin{align*} \mathcal{L} ((u^*, v^*, w^*, \theta^*, \vartheta^*)) : = &\langle \rho_1h_1(k^1+ k^2), u^*\rangle + \langle \rho_3 h_3(k^3+k^4), v^*\rangle + \langle \delta_3 k^5+\rho h (k^5+k^6), u^*\rangle\\ & + \langle\delta_1\left( k^1_x+k^3\right) +\rho_4 k^7, \theta^*\rangle + \langle \beta_1\int_0^{+\infty}\mu_1(s)\left(\int_0^s e^{r-s}k_{x}^8(r)dr \right)ds, \theta^*_x\rangle\\ &+\langle \delta_2k_x^3+\rho_5 k^9, \vartheta^* \rangle + \langle \beta_2\int_0^{+\infty}\mu_2(s)\left(\int_0^s e^{r-s}k_{x}^{10}(r)dr \right)ds, \vartheta^*_x \rangle, \end{align*} |
for every (u, v, w, \theta, \vartheta), \ (u^*, v^*, w^*, \theta^*, \vartheta^*)\in \mathbb{H} . Routine computations, using Cauchy-Schwarz, Young's and Poincaré's inequalities shows that \mathcal{B} is a bounded and coercive bilinear form on \mathbb{H}\times\mathbb{H} , and \mathcal{L} is a bounded linear form on \mathbb{H} . Therefore, using Lax-Milgram theorem, there exists a unique (u, v, w, \theta, \vartheta)\in \mathbb{H} such that
| \mathcal{B}((u, v, w, \theta, \vartheta), (u^*, v^*, w^*, \theta^*, \vartheta^*)) = \mathcal{L}((u^*, v^*, w^*, \theta^*, \vartheta^*)), \ \forall\ (u^*, v^*, w^*, \theta^*, \vartheta^*)\in \mathbb{H}. |
From (3.4) , it follows that
| \varphi\in H^1_b, \ \psi\in H^1_b\ and \ \phi\in H^2_*. |
Then, using standard regularity theory, it follows from (3.5) , that
| u, v\in H^2_b\cap H^1_b, \ w\in H^4\cap H^2_*, \ \ \theta, \vartheta\in H^2\cap H^1_a. |
Since u, v\in H^1_b, w, k^6\in H^2_* and k^6\in L^2 , it easy to see from (3.5)_3 that w satisfy
| w_{xx}(0) = w_{xx}(\pi) = 0. |
Also, from (3.3), substituting \theta and \vartheta , we see that
| \sigma\in\mathcal{D}(L^2_{\mu_1}), \ \ \zeta \in\mathcal{D}(L^2_{\mu_2}). |
Finally, from (3.2)_7 and (3.2)_9 , using regularity theory, we get that
| \int_0^{+\infty}\mu_1(s)\sigma(s) ds, \int_0^{+\infty} \mu_2(s)\zeta(s) ds\in H^2\cap H^1_a. |
Thus, \Psi = (u, \varphi, v, \psi, w, \phi, \theta, \sigma, \vartheta, \zeta) \in\mathcal{D}(\mathcal{A}) and satisfies (3.1). That is, the operator \mathcal{A} is maximal.
Theorem 3.1. Suppose \Psi_0 = (u_0, u_1, v_0, v_1, w_0, w_1, \theta_0, \sigma_0, \vartheta_0, \zeta_0)\in\mathcal{H} is given and condition (A_1) holds, then the Cauchy problem (2.13) has a unique weak global solution
| \Psi\in\mathcal{C}([0, +\infty), \mathcal{H}). |
Furthermore, if \Psi_0 = (u_0, u_1, v_0, v_1, w_0, w_1, \theta_0, \sigma_0, \vartheta_0, \zeta_0)\in\mathcal{D}(\mathcal{A}) , then the solution is in the class
| \Psi\in\mathcal{C}([0, \infty), \mathcal{D}(\mathcal{A}))\cap \mathcal{C}^1([0, \infty), \mathcal{H}). |
Proof. On account of Lemmas 3.1 and 3.2 applying the Hille-Yosida theorem, we have that \mathcal{A} is a generator of a C_0 -semigroup of contractions \mathcal{S}(t) = e^{\mathcal{A}t}, \ t\geq 0, on \mathcal{H}. By the semigroup theory for linear operators (Pazy [31]), we get that
| \Psi(t) = \mathcal{S}(t)\Psi_0, \ t\geq0, |
on \mathcal{H} is a unique solution satisfying problem (2.13).
In this section, we study the stability of solution of (2.10)–(2.12). The energy functional associated to the solution \Psi = (u, u_t, v, v_t, w, w_t, \theta, \sigma, \vartheta, \zeta) of system (2.10)–(2.12) is defined by
| \begin{equation} \begin{aligned} \mathcal{E}(t) = & \frac{1}{2}\left[ \rho_1 h_1 \|u_t\|^2 + \rho_3 h_3\|v_t\|^2 + \rho h\| w_t\|^2 + E_1 h_1 \|u_x\|^2+ E_3 h_3 \|v_x\|^2+ EI\|w_{xx}\|^2 \right]\\ &+\dfrac{1}{2}\left[k \|(-u+v+\alpha w_x)\|^2 +\rho_4\|\theta\|^2 +\beta_1\|\sigma\|^2_{L^2_{\mu_1}}+\rho_5\|\vartheta\|^2 +\beta_2\|\zeta\|^2_{L^2_{\mu_2}}\right], \ \ \forall \ t\geq 0. \end{aligned} \end{equation} | (4.1) |
Lemma 4.1. Under the conditions of Theorem 3.1, the energy functional (4.1) satisfies
| \begin{equation} \begin{aligned} \mathcal{E}'(t)& = -\delta_3\|w_t\|^2+\frac{\beta_1}{2}\int_0^{+\infty}\mu_1'(s)\|\sigma_x(s)\|^2ds +\frac{\beta_2}{2}\int_0^{+\infty}\mu_2'(s)\|\zeta_x(s)\|^2ds\leq 0, \forall t\geq 0. \end{aligned} \end{equation} | (4.2) |
Proof. Multiplication in L^2(0, \pi) the Eq (2.10)_1, (2.10)_2, (2.10)_3 , (2.10)_4 and (2.10)_6 by u_t, v_t, w_t , \theta and \vartheta respectively, follow by multiplying (2.10)_5 and (2.10)_7 by \sigma and \zeta in L^2_{\mu_1} and L^2_{\mu_2} respectively, then using integration by parts and the boundary conditions (2.11), we have
| \begin{equation} \frac{1}{2}\frac{d}{dt}\left[\rho_1h_1\|u_t\|^2 +E_1h_1\|u_x\|^2 \right]-\langle k(-u+v+\alpha w_x), u_t \rangle -\delta_1\langle \theta, u_{xt} \rangle = 0, \end{equation} | (4.3) |
| \begin{equation} \begin{aligned} \frac{1}{2}\frac{d}{dt}\left[\rho_3h_3 \|v_t\|^2 + E_3h_3\|v_x\|^2 \right]& +\langle k(-u+v+\alpha w_x), v_t \rangle -\delta_1\langle\theta, v_{t}\rangle-\delta_2\langle \vartheta, v_{xt}\rangle = 0, \end{aligned} \end{equation} | (4.4) |
| \begin{equation} \frac{1}{2}\frac{d}{dt}\left[\rho h\|w_t\|^2 + EI\|w_{xx}\|^2 \right] + \langle k(-u+v+\alpha w_x), \alpha w_{xt} \rangle + \delta_3\|w_t\|^2 = 0, \end{equation} | (4.5) |
| \begin{equation} \frac{1}{2}\frac{d}{dt}\left[\rho_4\|\theta\|^2 \right]+\beta_1\int_0^{+\infty}\mu_1(s)\langle\sigma_x(s), \theta_x(t) \rangle ds + \delta_1\langle \theta, (u_{xt}+v_t)\rangle = 0, \end{equation} | (4.6) |
| \begin{equation} \frac{1}{2}\frac{d}{dt}\left[\beta_1 \| \sigma\|^2_{L^2_{\mu_1}}\right]-\frac{\beta_1}{2}\int_0^{+\infty}\mu_1'(s)\|\sigma_x(s)\|^2ds - \beta_1\int_0^{+\infty}\mu_1(s)\langle\sigma_x(s), \theta_x(t)\rangle ds = 0, \end{equation} | (4.7) |
| \begin{equation} \frac{1}{2}\frac{d}{dt}\left[\rho_5\|\vartheta\|^2 \right]+\beta_2\int_0^{+\infty}\mu_2(s)\langle\zeta_x(s), \vartheta_x(t) \rangle ds + \delta_2\langle\vartheta, v_{xt}\rangle = 0, \end{equation} | (4.8) |
and
| \begin{equation} \frac{1}{2}\frac{d}{dt}\left[\beta_2 \| \zeta\|^2_{L^2_{\mu_2}}\right]-\frac{\beta_2}{2}\int_0^{+\infty}\mu_2'(s)\|\zeta_x(s)\|^2ds - \beta_1\int_0^{+\infty}\mu_2(s)\langle\zeta_x(s), \vartheta_x(t)\rangle ds = 0. \end{equation} | (4.9) |
Addition of (4.3)–(4.9) leads to
| \begin{equation} \begin{aligned} \mathcal{E}'(t) = -\delta_3\|w_t\|^2+\frac{\beta_1}{2}\int_0^{+\infty}\mu_1'(s)\|\sigma_x(s)\|^2ds +\frac{\beta_2}{2}\int_0^{+\infty}\mu_2'(s)\|\zeta_x(s)\|^2ds\leq 0. \end{aligned} \end{equation} | (4.10) |
Therefore, the energy \mathcal{E} is non-increasing and bounded above by \mathcal{E}(0) . Also, the computations here are done for regular solution. However, the result remains true for weak solution by density argument.
Lemma 4.2. Let \Psi = (u, u_t, v, v_t, w, w_t, \theta, \sigma, \vartheta, \zeta)\in \mathcal{H} be the solution of system (2.10)–(2.12) given by Theorem 3.1, then the functional G_1 defined by
| G_1(t) = \rho_1 h_1\langle u_t, u \rangle + \rho_3h_3\langle v_t, v \rangle + \rho h\langle w_t, w \rangle + \frac{\delta_3}{2}\| w\|^2 |
satisfies the estimate
| \begin{equation} \begin{aligned} G_1'(t)\leq &-\frac{E_1h_1}{2}\|u_x\|^2 -\frac{E_3h_3}{2}\|v_x\|^2 -EI\|w_{xx}\|^2- k \|(-u+v+\alpha w_x)\|^2\\ &+\rho_1 h_1 \|u_{t}\|^2 + \rho_3h_3\|v_t\|^2 + \rho h\|w_t\|^2 + C\|\theta\|^2+C\|\vartheta\|^2, \ \forall\ t\geq 0. \end{aligned} \end{equation} | (4.11) |
Proof. Differentiation of G_1 gives
| \begin{align*} G_1'(t) = &\rho_1 h_1\langle u_{tt}, u \rangle + \rho_3h_3\langle v_{tt}, v \rangle + \rho h\langle w_{tt}, w \rangle + \delta_3 \langle w_t, w\rangle\\ &+\rho_1 h_1 \|u_{t}\|^2 + \rho_3h_3\|v_t\|^2 + \rho h\|w_t\|^2. \end{align*} |
Using Eq (2.10)_1 , (2.10)_2 and (2.10)_3 , then applying integration by parts over (0, \pi) and making use of the boundary conditions (2.11) leads to
| \begin{equation} \nonumber \begin{aligned} G_1'(t) = &- E_1h_1 \|u_x\|^2 - E_3h_3\|v_x\|^2 -EI\|w_{xx}\|^2- k \|(-u+v+\alpha w_x)\|^2\\ &+ \delta_1\langle u_x, \theta \rangle +\delta_1\langle v, \theta \rangle+ \delta_2\langle v_x, \vartheta \rangle + \rho_1 h_1 \|u_{t}\|^2 + \rho_3h_3\|v_t\|^2 + \rho h\|w_t\|^2. \end{aligned} \end{equation} |
Applying Young's and Poincaré's inequalities, we obtain
| \begin{equation} \nonumber \begin{aligned} G_1'(t)\leq & -\frac{E_1h_1}{2}\|u_x\|^2 -\frac{E_3h_3}{2}\|v_x\|^2 -EI\|w_{xx}\|^2- k \|(-u+v+\alpha w_x)\|^2\\ &+ \rho_1 h_1 \|u_{t}\|^2 + \rho_3h_3\|v_t\|^2 + \rho h\|w_t\|^2 + C\|\theta\|^2+ C\|\vartheta\|^2. \end{aligned} \end{equation} |
Lemma 4.3. Let \Psi = (u, u_t, v, v_t, w, w_t, \theta, \sigma, \vartheta, \zeta)\in \mathcal{H} be the solution of system (2.10)–(2.12) given by Theorem 3.1, then the functional G_2 defined by
| G_2(t) = -\rho_1h_1\rho_4 \langle \theta , \widehat{u}_t(t)\rangle, \ {\rm where}\ \widehat{u}_t(t) = \int_0^x u_t(y, t)dy dx |
satisfies, for any \epsilon_1 > 0 and \epsilon_2 > 0, the the estimate
| \begin{align} G_2'(t)\leq&-\frac{\rho_1h_1\delta_1}{2}\|u_t\|^2 + \epsilon_1 \|u_x\|^2 +\epsilon_2 \|(-u+v+\alpha w_x) \|^2 \\ &+ C\|v_{t}\|^2 + C\| \sigma\|^2_{L^2_{\mu_1}} + C\left(1+\frac{1}{\epsilon_1}+\frac{1}{\epsilon_2} \right) \|\theta\|^2, \ \forall\ t\geq 0. \end{align} | (4.12) |
Proof. Differentiation of G_2 , using (2.10)_1 and (2.10)_4 , integration by parts and boundary conditions (2.11), we arrive at
| \begin{align} G'_2(t) = & -\rho_1h_1\rho_4\langle \theta, \widehat{u}_{tt}(t) \rangle-\rho_1h_1\rho_4 \langle \theta_t, \widehat{u}_t(t) \rangle\\ = &-\rho_1h_1\delta_1\|u_t\|^2 -\rho_4E_1h_1\langle\theta, u_x \rangle + \rho_1h_1\delta_1 \langle v_t, \widehat{u}_t(t) \rangle \\ & -\rho_4k \langle \theta, \widehat{(-u+v+\alpha w_x)}\rangle +\rho_3\delta_1\|\theta\|^2\\ &+ \rho_1h_1 \beta_1\langle u_t, \int_0^{+\infty} \mu_1(s)\sigma_x(., t, s)ds \rangle. \end{align} |
Using Cauchy-Schwarz, Young's and Poincaré's inequalities yields
| \begin{align} G'_2(t)\leq& -\rho_1h_1\delta_1\|u_t\|^2 + \epsilon_1\|u_x\|^2 + \frac{(\rho_4E_1h_1)^2}{4\epsilon_1}\|\theta\|^2+ \frac{3\rho_1h_1\delta_1}{4}\|v_t\|^2 \\ &+ \frac{\rho_1h_1\delta_1}{4}\|u_t\|^2 + \epsilon_2 \|(-u+v+\alpha w_x)\|^2 +\frac{(\rho_4k)^2}{4\epsilon_2}\|\theta\|^2 \\ &+\rho_3\delta_1\|\theta\|^2 + \frac{\rho_1h_1\delta_1}{4}\|u_t\|^2+\frac{3\rho_1h_1\beta_1^2}{4\delta_1} \| \sigma\|^2_{L^2_{\mu_1}}. \end{align} |
Thus, we obtain (4.12).
Lemma 4.4. Let \Psi = (u, u_t, v, v_t, w, w_t, \theta, \sigma, \vartheta, \zeta)\in \mathcal{H} be the solution of system (2.10)–(2.12) given by Theorem 3.1, then the functional G_3 defined by
| G_3(t) = -\rho_3h_3\rho_5 \langle \vartheta, \widehat{v}_t(t)\rangle, \ {\rm where}\ \widehat{v}_t(t) = \int_0^x v_t(y, t)dy |
satisfies, for any \epsilon_3 > 0 and \epsilon_4 > 0, the estimate
| \begin{align} G_3'(t)\leq&-\frac{\rho_3h_3\delta_2}{2}\|v_t\|^2 + \epsilon_3 \|v_x\|^2 +\epsilon_4 \|(-u+v+\alpha w_x) \|^2 \\ &+ C\|\theta\|^2 + C\| \zeta\|^2_{L^2_{\mu_2}} + C\left(1+\frac{1}{\epsilon_3}+\frac{1}{\epsilon_4} \right) \|\vartheta\|^2, \ \forall\ t\geq 0. \end{align} | (4.13) |
Proof. Differentiation of G_3 , using (2.10)_2 and (2.10)_5 , integration by parts and boundary conditions (2.11), we arrive at
| \begin{align} G'_3(t) = & -\rho_3h_3\rho_5\langle \vartheta, \widehat{v}_{tt}(t)\rangle-\rho_3h_3\rho_5\langle \vartheta_t, \widehat{v}_t(t)\rangle\\ = &-\rho_3h_3\delta_2\|v_t\|^2 -\rho_5E_3h_3\langle\vartheta, v_x \rangle - \rho_5\delta_1\langle \vartheta, \widehat{\theta}(t)\rangle +\rho_5k \langle \vartheta, \widehat{(-u+v+\alpha w_x)}\rangle \\ & +\rho_5\delta_2\|\vartheta\|^2+ \rho_3h_3 \beta_2 \langle v_t, \int_0^{+\infty} \mu_2(s)\zeta_x(., t, s)ds\rangle. \end{align} |
Applying Cauchy-Schwarz, Young's and Poincaré's inequalities, we have
| \begin{align} G'_3(t)\leq& -\rho_3h_3\delta_2\|v_t\|^2 + \epsilon_3\|v_x\|^2 + \frac{(\rho_5E_3h_3)^2}{4\epsilon_3}\|\vartheta\|^2+ \frac{\rho_5\delta_1}{2}\|\theta\|^2 \\ &+ \frac{\rho_5\delta_1}{2}\|\vartheta\|^2 + \epsilon_4 \|(-u+v+\alpha w_x)\|^2 +\frac{(\rho_5k)^2}{4\epsilon_4}\|\vartheta\|^2 \\ &+\rho_5\delta_2\|\vartheta\|^2 + \frac{\rho_3h_3\delta_2}{4}\|v_t\|^2+\frac{3\rho_3h_3\beta_2^2}{4\delta_2} \| \zeta\|^2_{L^2_{\mu_2}}. \end{align} |
Hence, we get (4.13).
Lemma 4.5. Let \Psi = (u, u_t, v, v_t, w, w_t, \theta, \sigma, \vartheta, \zeta)\in \mathcal{H} be the solution of system (2.10)–(2.12) given by Theorem 3.1, then the functional G_4 defined by
| G_4(t) = -\rho_4\langle \theta, \int_0^{+\infty}\mu_1(s)\sigma(., t, s) ds\rangle, |
satisfies, for any \epsilon_5 > 0 and \epsilon_6 > 0, the estimate
| \begin{align} G_4'(t)\leq& -\frac{\rho_4g_1(0)}{2}\|\theta\|^2 + \epsilon_5 \|u_t\|^2 + \epsilon_6 \|v_t\|^2- C\int_0^{+\infty}\mu_1'(s)\|\sigma_x(s)\|^2 ds \end{align} | (4.14) |
| \begin{align} &+C\left( 1+\frac{1}{\epsilon_5}+\frac{1}{\epsilon_6}\right)\|\sigma\|^2_{L^2_{\mu_1}}, \ \forall\ t\geq 0. \end{align} | (4.15) |
Proof. Differentiating G_4 with respect to t , using (2.10)_4 and (2.10)_5 , integration by parts and the boundary conditions (2.11) and recalling (2.4), we get
| \begin{align} G'_4(t) = & -\rho_4\langle \theta_t, \int_0^{+\infty}\mu_1(s) \sigma(., t, s) ds\rangle- \rho_4\langle \theta, \int_0^{+\infty}\mu_1(s) \sigma_t(., t, s) ds\rangle\\ = &-\rho_4g_1(0) \|\theta\|^2 + \beta_1\left \|\int_0^{+\infty}\mu_1(s)\sigma_x(., t, s) ds\right\|^2 \\ &-\delta_1\langle u_t, \int_0^{+\infty}\mu_1(s)\sigma_x(., t, s) ds\rangle + \delta _1\langle v_t, \int_0^{+\infty}\mu_1(s)\sigma_x(., t, s) ds\rangle\\ & +\rho_4\langle \theta, \int_0^{+\infty}\mu_1(s)\sigma_s(., t, s) ds\rangle. \end{align} |
Making use of Cauchy-Schwarz and Young's inequalities, we have
| \begin{equation} \beta_1\left \|\int_0^{+\infty}\mu_1(s)\sigma_x(., t, s) ds\right\|^2 \leq C\|\sigma\|^2_{L^2_{\mu_1}}, \end{equation} | (4.16) |
| \begin{equation} \left | -\delta_1\langle u_t, \int_0^{+\infty}\mu_1(s)\sigma_x(., t, s) ds\rangle\right | \leq \epsilon_5 \|u_t\|^2 + \frac{C}{\epsilon_5}\|\sigma\|^2_{L^2_{\mu_1}}, \ {\rm for\ any}\ \epsilon_5 > 0, \end{equation} | (4.17) |
| \begin{equation} \left |\delta _1\langle v_t, \int_0^{+\infty}\mu_1(s)\sigma_x(., t, s) ds\rangle\right | \leq \epsilon_6 \|v_t\|^2 + \frac{C}{\epsilon_6}\|\sigma\|^2_{L^2_{\mu_1}}, \ {\rm for\ any}\ \epsilon_6 > 0. \end{equation} | (4.18) |
Also, using integration by parts with respect to s , we get
| \begin{equation} \begin{aligned} &\left | \rho_4\langle \theta, \int_0^{+\infty}\mu_1(s)\sigma_s(., t, s) ds\rangle \right |\\ = &\left | -\rho_4\langle \theta, \int_0^{+\infty}\mu_1(s)\sigma'(., t, s) ds\rangle \right |\\ \leq & C\|\theta\|\left( -\int_0^{+\infty}\mu_1'(s)\|\sigma_x\|^2 ds\right)^{\frac{1}{2}} \\ \leq & \frac{\rho_4g_1(0)}{2}\|\theta\|^2 - C\int_0^{+\infty}\mu_1'(s)\|\sigma_x(s)\|^2 ds. \end{aligned} \end{equation} | (4.19) |
On account of (4.16)–(4.19), we obtain
| \begin{align*} G_4'(t)\leq & -\frac{\rho_4g_1(0)}{2}\|\theta\|^2 + \epsilon_5 \|u_t\|^2 + \epsilon_6 \|v_t\|^2- C\int_0^{+\infty}\mu_1'(s)\|\sigma_x(s)\|^2 ds\\ &+C\left( 1+\frac{1}{\epsilon_5}+\frac{1}{\epsilon_6}\right) \|\sigma\|^2_{L^2_{\mu_1}}. \end{align*} |
Lemma 4.6. Let \Psi = (u, u_t, v, v_t, w, w_t, \theta, \sigma, \vartheta, \zeta)\in \mathcal{H} be the solution of system (2.10)–(2.12) given by Theorem 3.1, then the functional G_5 defined by
| G_5(t) = -\rho_5\langle \vartheta, \int_0^{+\infty}\mu_2(s)\zeta(., t, s) ds\rangle, |
satisfies for any \epsilon_7 > 0 , the estimate
| \begin{align} G_5'(t)(t)\leq -\frac{\rho_5 g_2(0)}{2}\|\vartheta\|^2+ \epsilon_7 \|v_t\|^2 - C\int_0^{+\infty}\mu_2'(s)\|\zeta_x(s)\|^2 ds + C\left(1+\frac{1}{\epsilon_7}\right) \| \zeta\|^2_{L^2_{\mu_2}} , \ \forall\ t\geq 0. \end{align} | (4.20) |
Proof. Differentiation of G_5 with respect to t , using (2.10)_6 and (2.10)_7 , integration by parts and the boundary conditions (2.11), and recalling (2.4), we get
| \begin{align} G'_5 = & -\rho_5\langle \vartheta_t, \int_0^{+\infty}\mu_2(s) \zeta(., t, s) ds\rangle- \rho_5\langle\vartheta, \int_0^{+\infty}\mu_2(s) \zeta_t(., t, s) ds\rangle\\ = &-\rho_5g_1(0) \|\vartheta\|^2 + \beta_2 \left\|\int_0^{+\infty}\mu_2(s)\zeta_x(., t, s) ds \right\|^2\\ & -\delta_2\langle v_t, \int_0^{+\infty}\mu_2(s)\zeta_x(., t, s) ds\rangle +\rho_5\langle \vartheta, \int_0^{+\infty}\mu_2(s)\zeta_s(., t, s) ds\rangle. \end{align} |
Using similar estimations as in (4.16)–(4.19) leads to (4.20).
The main stability result of this work is the following:
Theorem 4.1. Let \Psi_0 = (u_0, u_1, v_0, v_1, w_0, w_1, \theta_0, \sigma_0, \vartheta_0, \zeta_0)\in\mathcal{D}(\mathcal{A}) be given. Suppose condition (A_1) holds, then the energy functional \mathcal{E}(t) defined in (4.1) decays exponentially. That is, there exists positive constants M and \lambda such that
| \begin{equation} \mathcal{E}(t)\leq M e^{-\lambda t}, \ \forall t\geq 0. \end{equation} | (4.21) |
Proof. We set
| \begin{equation} L(t): = N \mathcal{E}(t) + N_1G_1(t) + N_2 G_2(t) + N_3G_3(t)+ N_4G_4(t)+ N_5G_5(t), \ \ t\geq 0, \end{equation} | (4.22) |
for some N, N_1, N_2, N_3, N_4, N_5 > 0 to be specified later. Direct computations, applying Young's, Cauchy-Schwarz and Poincaré's inequalities gives
| \begin{equation} \tilde{b}_1\mathcal{E}(t)\leq L(t)\leq \tilde{b}_2\mathcal{E}(t), \ \ t\geq 0, \end{equation} | (4.23) |
for some positive constants \tilde{b}_1 and \tilde{b}_2. Now, using Lemmas 4.1 and 4.2 - 4.6, we get
| \begin{equation} \begin{aligned} L'(t)\leq & - \left[\frac{\rho_1h_1\delta_1}{2}N_2-\rho_1 h_1 N_1-\epsilon_5 N_4\right] \|u_t\|^2-\left[\delta_3 N- \rho h N_1 \right] \|w_t\|^2 \\ &- \left[ \frac{\rho_3h_3\delta_2}{2}N_3-\rho_3 h_3 N_1- CN_2-\epsilon_6N_4-\epsilon_7N_5 \right]\|v_t\|^2\\ &-\left[\frac{E_1h_1}{2}N_1- \epsilon_1N_2\right]\|u_x\|^2 -\left[\frac{E_3h_3}{2}N_1-\epsilon_3 N_3 \right] \|v_x\|^2 -EIN_1 \|w_{xx}\|^2 \\ &- \left[kN_1-\epsilon_2N_2 -\epsilon_4 N_3 \right] \|(-u+v+\alpha w_x)\|^2 \\ &-\left[\frac{\rho_4g_1(0)}{2}N_4-CN_1-CN_2\left(1+\frac{1}{\epsilon}_1+\frac{1}{\epsilon_2}\right)-CN_3\right] \|\theta\|^2\\ &+\left[CN_2+CN_4\left(1+\frac{1}{\epsilon_5}+\frac{1}{\epsilon_6}\right) \right] \| \sigma\|^2_{L^2_{\mu_1}} -\left[ \frac{\beta_1}{2}N-CN_4\right] \int_0^{+\infty}\mu'_1(s)\|\sigma_x(s)\|^2ds\\ &-\left[\frac{\rho_5g_2(0)}{2}N_5-CN_1-CN_3\left(1+\frac{1}{\epsilon_3}+\frac{1}{\epsilon}_4\right)\right] \|\vartheta\|^2\\ &+\left[CN_3+CN_5\left(1+\frac{1}{\epsilon_7}\right) \right] \| \zeta\|^2_{L^2_{\mu_2}} -\left[ \frac{\beta_2}{2}N-CN_5\right] \int_0^{+\infty}\mu'_2(s)\|\zeta_x(s)\|^2ds. \end{aligned} \end{equation} | (4.24) |
From (2.5), we have that
| \mu_i(s)\leq -\frac{1}{\xi_i}\mu'_i(s), \ \ i = 1, 2. |
Also, by choosing
| \begin{equation} \nonumber N_1 = 1, \epsilon_1 = \frac{E_1h_1}{4N_2}, \ \epsilon_2 = \frac{k}{4N_2}, \ \epsilon_3 = \frac{E_3h_3}{4N_3}, \ \epsilon_4 = \frac{k}{4N_3}, \end{equation} |
| \begin{equation} \nonumber \epsilon_5 = \frac{\rho_1h_1\delta_1}{4N_4}, \ \ \epsilon_6 = \frac{\rho_3h_3\delta_2}{8N_4}, \ \ \epsilon_7 = \frac{\rho_3h_3\delta_2}{8N_5}, \end{equation} |
then (4.24) takes the form
| \begin{equation} \begin{aligned} L'(t)\leq & - \left[\frac{\rho_1h_1\delta_1}{4}N_2-\rho_1 h_1\right] \|u_t\|^2- \left[ \frac{\rho_3h_3\delta_2}{4}N_3- CN_2-\rho_3 h_3 \right]\|v_t\|^2 \\ &-\left[\delta_3 N- \rho h \right] \|w_t\|^2-\frac{E_1h_1}{4}\|u_x\|^2 -\frac{E_3h_3}{4} \|v_x\|^2 \\ & -EI\|w_{xx}\|^2-\frac{k}{2} \|(-u+v+\alpha w_x)\|^2\\ &-\left[\frac{\rho_4g_1(0)}{2}N_4-CN_2\left(1+\frac{4N_2}{E_1h_1}+\frac{4N_2}{k}\right)-CN_3-C\right] \|\theta\|^2\\ &-\left[ \frac{\beta_1\xi_1}{2}N-C\xi_1N_4- \left( CN_2+CN_4\left(1+\frac{4N_4}{\rho_1h_1\delta_1}+\frac{8N_4}{\rho_3h_3\delta_2}\right)\right) \right]\| \sigma\|^2_{L^2_{\mu_1}}\\ &-\left[\frac{\rho_5g_2(0)}{2}N_5-CN_3\left(1+\frac{4N_3}{E_3h_3}+\frac{4N_3}{k}\right)-C\right] \|\vartheta\|^2\\ &-\left[\frac{\beta_2\xi_2}{2}N-C\xi_2N_5-\left(CN_3+CN_5\left(1+\frac{8N_5}{\rho_3h_3\delta_2}\right) \right) \right] \| \zeta\|^2_{L^2_{\mu_2}}. \end{aligned} \end{equation} | (4.25) |
Next, we specified the rest of the parameters. First, we choose N_2 large such that
| \begin{equation} \nonumber \frac{\rho_1h_1\delta_1}{4}N_2-\rho_1 h_1 > 0. \end{equation} |
Second, we select N_3 large enough such that
| \begin{equation} \nonumber \frac{\rho_3h_3\delta_2}{4}N_3- CN_2-\rho_3 h_3 > 0. \end{equation} |
Thirdly, we choose N_4 and N_5 large enough such that
| \begin{equation} \nonumber \frac{\rho_4g_1(0)}{2}N_4-CN_2\left(1+\frac{4N_2}{E_1h_1}+\frac{4N_2}{k}\right)-CN_3-C > 0, \end{equation} |
and
| \begin{equation} \nonumber \ \frac{\rho_4h_2(0)}{2}N_5-CN_3\left(1+\frac{8N_3}{k}+\frac{4N_3}{b}\right)-C > 0. \end{equation} |
Finally, we choose N very large so that (4.23) remain valid and
| \delta_3 N- \rho h > 0, \ \frac{\beta_1\xi_1}{2}N-C\xi_1N_4-\left( CN_2+CN_4\left(1+\frac{4N_4}{\rho_1h_1\delta_1}+\frac{8N_4}{\rho_3h_3\delta_2}\right)\right) > 0, |
| \frac{\beta_2\xi_2}{2}N-C\xi_2N_5-\left(CN_3+CN_5\left(1+\frac{8N_5}{\rho_3h_3\delta_2}\right) \right) > 0. |
Thus, we obtain
| \begin{equation} \begin{aligned} L'(t)\leq &-\gamma_0\left[ \|u_t\|^2 + \|v_t\|^2 + \| w_t\|^2 + \|u_x\|^2+ \|v_x\|^2+ \|w_{xx}\|^2\right] \\ &-\gamma_0\left[ \|(-u+v+\alpha w_x)\|^2+|\theta\|^2 +\|\sigma\|^2_{L^2_{\mu_1}}+\|\vartheta\|^2 +\|\zeta\|^2_{L^2_{\mu_2}}\right] \end{aligned} \end{equation} | (4.26) |
for some \gamma_0 > 0. Recalling (4.1), it follows from (4.26) that
| \begin{equation} L'(t)\leq -\gamma_1\mathcal{E}(t), \ \forall \ t\geq 0, \end{equation} | (4.27) |
for some \gamma_1 > 0. Using (4.23), we obtain
| \begin{equation} L'(t)\leq -\gamma_2 L(t), \ \forall\ t\geq 0, \end{equation} | (4.28) |
for some \gamma_2 > 0. Integrating (4.28) over (0, t) yields for some \gamma_3 > 0
| \begin{equation} L(t)\leq L(0)e^{-\gamma_3 t}, \ \forall\ t\geq 0. \end{equation} | (4.29) |
Hence, the exponential estimate of the energy functional \mathcal{E}(t) in (4.21) follows from (4.29) by using (4.23). This completes the proof.
In this work, we investigated the the effect of Gurtin-Pipkin's thermal law on the outer layers of the Rao-Nakra beam model. Using standard semi-group theory for linear operators and the multiplier method, the well-posedness and a stability result of solutions of the triple beam system have been established.
The author declares he has not used Artificial Intelligence (AI) tools in the creation of this article.
The author acknowledges the technical and financial support from the Ministry of education and the University of Hafr Al Batin, Saudi Arabia. This research work was funded by Institutional fund projects \# IFP-A-2022-2-1-04.
The author declares no potential conflict of interest.
| [1] |
Y. V. K. S. Rao, B. C. Nakra, Vibrations of unsymmetrical sandwich beams and plates with viscoelastic cores, J. Sound Vibr., 34 (1974), 309–326. https://doi.org/10.1016/S0022-460X(74)80315-9 doi: 10.1016/S0022-460X(74)80315-9

|
| [2] |
D. J. Mead, S. Markus, The forced vibration of a three-layer, damped sandwich beam with arbitrary boundary conditions, J. Sound Vibr., 10 (1969), 163–175. https://doi.org/10.1016/0022-460X(69)90193-X doi: 10.1016/0022-460X(69)90193-X

|
| [3] |
M. J. Yan, E. H. Dowell, Governing equations for vibrating constrained-layer damping sandwich plates and beams, J. Appl. Mech., 39 (1972), 1041–1047. https://doi.org/10.1115/1.3422825 doi: 10.1115/1.3422825

|
| [4] |
S. W. Hansen, Several related models for multilayer sandwich plates, Math. Models Methods Appl. Sci., 14 (2004), 1103–1132. https://doi.org/10.1142/S0218202504003568 doi: 10.1142/S0218202504003568

|
| [5] |
A. Ö. Özer, S. W. Hansen, Uniform stabilization of a multilayer Rao-Nakra sandwich beam, Evolution Equ. Control Theory, 2 (2013), 695–710. https://doi.org/10.3934/eect.2013.2.695 doi: 10.3934/eect.2013.2.695

|
| [6] |
Z. Liu, S. A. Trogdon, J. Yong, Modeling and analysis of a laminated beam, Math. Comput. Model., 30 (1999), 149–167. https://doi.org/10.1016/S0895-7177(99)00122-3 doi: 10.1016/S0895-7177(99)00122-3

|
| [7] |
S. W. Hansen, R. D. Spies, Structural damping in a laminated beam due to interfacial slip, J. Sound Vibr., 204 (1997), 183–202. https://doi.org/10.1006/jsvi.1996.0913 doi: 10.1006/jsvi.1996.0913

|
| [8] |
Y. F. Li, Z. Y. Liu, Y. Wang, Weak stability of a laminated beam, Math. Control Relat. Fields, 8 (2018), 789–808. https://doi.org/10.3934/mcrf.2018035 doi: 10.3934/mcrf.2018035

|
| [9] |
T. Q. Méndez, V. C. Zannini, B. W. Feng, Asymptotic behavior of the Rao-Nakra sandwich beam model with Kelvin-Voigt damping, Math. Mech. Solids, 2023. https://doi.org/10.1177/10812865231180535 doi: 10.1177/10812865231180535

|
| [10] |
B. W. Feng, A. Ö. Özer, Long-time behavior of a nonlinearly-damped three-layer Rao-Nakra sandwich beam, Appl. Math. Optim., 87 (2023), 19. https://doi.org/10.1007/s00245-022-09931-7 doi: 10.1007/s00245-022-09931-7

|
| [11] |
B. W. Feng, C. A. Raposo, C. A. Nonato, A. Soufyane, Analysis of exponential stabilization for Rao-Nakra sandwich beam with time-varying weight and time-varying delay: Multiplier method versus observability, Math. Control Relat. Fields, 13 (2023), 631–663. https://doi.org/10.3934/mcrf.2022011 doi: 10.3934/mcrf.2022011

|
| [12] |
S. E. Mukiawa, C. D. Enyi, J. D. Audu, Well-posedness and stability result for a thermoelastic Rao-Nakra beam model, J. Therm. Stresses, 45 (2022), 720–739. https://doi.org/10.1080/01495739.2022.2074931 doi: 10.1080/01495739.2022.2074931

|
| [13] |
C. A. Raposo, O. P. V. Villagran, J. Ferreira, E. Pişkin, Rao-Nakra sandwich beam with second sound, Part. Differ. Equ. Appl. Math., 4 (2021), 100053. https://doi.org/10.1016/j.padiff.2021.100053 doi: 10.1016/j.padiff.2021.100053

|
| [14] |
Z. Y. Liu, B. P. Rao, Q. Zheng, Polynomial stability of the Rao-Nakra beam with a single internal viscous damping, J. Differ. Equ., 269 (2020), 6125–6162. https://doi.org/10.1016/j.jde.2020.04.030 doi: 10.1016/j.jde.2020.04.030

|
| [15] |
S. W. Hansen, O. Y. Imanuvilov, Exact controllability of a multilayer Rao-Nakra plate with free boundary conditions, Math. Control Relat. Fields, 1 (2011), 189–230. https://doi.org/10.3934/mcrf.2011.1.189 doi: 10.3934/mcrf.2011.1.189

|
| [16] |
S. W. Hansen, O. Imanuvilov, Exact controllability of a multilayer Rao-Nakra plate with clamped boundary conditions, ESAIM Control Optim. Calc. Var., 17 (2011), 1101–1132. https://doi.org/10.1051/cocv/2010040 doi: 10.1051/cocv/2010040

|
| [17] | S. W. Hansen, R. Rajaram, Simultaneous boundary control of a Rao-Nakra sandwich beam, in: Proceedings of the 44th IEEE Conference on Decision and Control, 2005, 3146–3151. https://doi.org/10.1109/CDC.2005.1582645 |
| [18] | S. W. Hansen, R. Rajaram, Riesz basis property and related results for a Rao-Nakra sandwich beam, Conf. Publ., 2005 (2005), 365–375. |
| [19] |
R. Rajaram, Exact boundary controllability result for a Rao-Nakra sandwich beam, Syst. Control Lett., 56 (2007), 558–567. https://doi.org/10.1016/j.sysconle.2007.03.007 doi: 10.1016/j.sysconle.2007.03.007

|
| [20] |
C. A. Raposo, Rao-Nakra model with internal damping and time delay, Math. Morav., 25 (2021), 53–67. https://doi.org/10.5937/MatMor2102053R doi: 10.5937/MatMor2102053R

|
| [21] |
M. E. Gurtin, A. C. Pipkin, A general theory of heat conduction with finite waves peeds, Arch. Rational Mech. Anal., 31 (1968), 113–126. https://doi.org/10.1007/BF00281373 doi: 10.1007/BF00281373

|
| [22] |
F. Dell'Oro, V. Pata, On the stability of Timoshenko systems with Gurtin-Pipkin thermal law, J. Differ. Equ., 257 (2014), 523–548. https://doi.org/10.1016/j.jde.2014.04.009 doi: 10.1016/j.jde.2014.04.009

|
| [23] |
A. Fareh, Exponential stability of a Timoshenko type thermoelastic system with Gurtin-Pipkin thermal law and frictional damping, Commun. Fac. Sci. Univ. Ank. Ser. A1 Math. Stat., 71 (2022), 95–115. https://doi.org/10.31801/cfsuasmas.847038 doi: 10.31801/cfsuasmas.847038

|
| [24] |
W. J. Liu, W. F. Zhao, On the stability of a laminated beam with structural damping and Gurti-Pipkin thermal law, Nonlinear Anal. Model. Control, 26 (2021), 396–418. https://doi.org/10.15388/namc.2021.26.23051 doi: 10.15388/namc.2021.26.23051

|
| [25] |
T. A. Apalara, O. B. Almutairi, Well-posedness and exponential stability of swelling porous with Gurtin-Pipkin thermoelasticity, Mathematics, 10 (2022), 1–17. https://doi.org/10.3390/math10234498 doi: 10.3390/math10234498

|
| [26] |
M. Khader, B. Said-Houari, On the decay rate of solutions of the Bresse system with Gurtin-Pipkin thermal law, Asymptot. Anal., 103 (2017), 1–32. https://doi.org/10.3233/ASY-171417 doi: 10.3233/ASY-171417

|
| [27] |
D. Hanni, B. W. Feng, K. Zennir, Stability of Timoshenko system coupled with thermal law of Gurtin-Pipkin affecting on shear force, Appl. Anal., 101 (2022), 5171–5192. https://doi.org/10.1080/00036811.2021.1883591 doi: 10.1080/00036811.2021.1883591

|
| [28] |
F. Dell'Oro, On the stability of Bresse and Timoshenko systems with hyperbolic heat conduction, J. Differ. Equ., 281 (2021), 148–198. https://doi.org/10.1016/j.jde.2021.02.009 doi: 10.1016/j.jde.2021.02.009

|
| [29] |
B. D. Coleman, M. E. Gurtin, Equipresence and constitutive equations for rigid heat conductors, Z. Angew. Math. Phys., 18 (1967), 199–208. https://doi.org/10.1007/BF01596912 doi: 10.1007/BF01596912

|
| [30] |
C. M. Dafermos, An abstract Volterra equation with applications to linear viscoelasticity, J. Differ. Equ., 7 (1970), 554–569. https://doi.org/10.1016/0022-0396(70)90101-4 doi: 10.1016/0022-0396(70)90101-4

|
| [31] | A. Pazzy, Semigroups of linear operators and application to partial differential equations, New York: Springer, 1983. https://doi.org/10.1007/978-1-4612-5561-1 |
| 1. | Mohammed M. Al-Gharabli, Shadi Al-Omari, Adel M. Al-Mahdi, Genni Fragnelli, Stabilization of a Rao–Nakra Sandwich Beam System by Coleman–Gurtin’s Thermal Law and Nonlinear Damping of Variable-Exponent Type, 2024, 2024, 2314-4785, 1, 10.1155/2024/1615178 | |
| 2. | Djellali Fayssal, Victor R. Cabanillas Zannini, Adel M. Al-Mahdi, EXPONENTIAL STABILIZATION OF LAMINATED BEAMS WITH GURTIN–PIPKIN THERMAL LAW THE CASE OF EQUAL SPEEDS, 2024, 36, 0897-3962, 10.1216/jie.2024.36.183 | |
| 3. | Hasan Almutairi, Soh Edwin Mukiawa, On the uniform stability of a thermoelastic Timoshenko system with infinite memory, 2024, 9, 2473-6988, 16260, 10.3934/math.2024787 | |
| 4. | Aissa Guesmia, Study of the well-posedness and decay rates for Rao–Nakra sandwich beam models subject to a single internal infinite memory and Dirichlet–Neumann boundary conditions, 2025, 44, 2238-3603, 10.1007/s40314-024-03033-6 | |
| 5. | Soh Edwin Mukiawa, Johnson D. Audu, Salim A. Messaoudi, Stabilization of a coupled bridge system with past history and gurtin-pipkin’s heat conduction, 2025, 1607-3606, 1, 10.2989/16073606.2025.2457677 |