The goal of this paper is to provide analytical assessments to a fluid flowing in a porous medium with a non-linear diffusion linked to a degenerate diffusivity. The viscosity term is formulated with an Eyring-Powell law, together with a non-homogeneous diffusion typical of porous medium equations (as known in the theory of partial differential equations). Further, the equation is supplemented with an absorptive reaction term of Darcy-Forchheimer, commonly used to model flows in porous medium. The work starts by analyzing regularity, existence and uniqueness of solutions. Afterwards, the problem is transformed to study travelling wave kind of solutions. An asymptotic expansion is considered with a convergence criteria based on the geometric perturbation theory. Supported by this theory, there exists an exponential decaying rate in the travelling wave profile. Such exponential behaviour is validated with a numerical assessment. This is not a trivial result given the degenerate diffusivity induced by the non-linear diffusion of porous medium type and suggests the existence of regularity that can serve as a baseline to construct numerical or energetic approaches.
Citation: José Luis Díaz Palencia, Saeed ur Rahman, Antonio Naranjo Redondo. Analysis of travelling wave solutions for Eyring-Powell fluid formulated with a degenerate diffusivity and a Darcy-Forchheimer law[J]. AIMS Mathematics, 2022, 7(8): 15212-15233. doi: 10.3934/math.2022834
| [1] | S. Rahman, J. L. Díaz Palencia, J. Roa González . Analysis and profiles of travelling wave solutions to a Darcy-Forchheimer fluid formulated with a non-linear diffusion. AIMS Mathematics, 2022, 7(4): 6898-6914. doi: 10.3934/math.2022383 |
| [2] | José L. Díaz . Existence, uniqueness and travelling waves to model an invasive specie interaction with heterogeneous reaction and non-linear diffusion. AIMS Mathematics, 2022, 7(4): 5768-5789. doi: 10.3934/math.2022319 |
| [3] | W. Abbas, Ahmed M. Megahed . Powell-Eyring fluid flow over a stratified sheet through porous medium with thermal radiation and viscous dissipation. AIMS Mathematics, 2021, 6(12): 13464-13479. doi: 10.3934/math.2021780 |
| [4] | Bengisen Pekmen Geridönmez . Numerical investigation of ferrofluid convection with Kelvin forces and non-Darcy effects. AIMS Mathematics, 2018, 3(1): 195-210. doi: 10.3934/Math.2018.1.195 |
| [5] | Ghazala Akram, Maasoomah Sadaf, Mirfa Dawood, Muhammad Abbas, Dumitru Baleanu . Solitary wave solutions to Gardner equation using improved tan$ \left(\frac{\Omega(\Upsilon)}{2}\right) $-expansion method. AIMS Mathematics, 2023, 8(2): 4390-4406. doi: 10.3934/math.2023219 |
| [6] | Feiting Fan, Xingwu Chen . Dynamical behavior of traveling waves in a generalized VP-mVP equation with non-homogeneous power law nonlinearity. AIMS Mathematics, 2023, 8(8): 17514-17538. doi: 10.3934/math.2023895 |
| [7] | Dagmar Medková . Classical solutions of the Dirichlet problem for the Darcy-Forchheimer-Brinkman system. AIMS Mathematics, 2019, 4(6): 1540-1553. doi: 10.3934/math.2019.6.1540 |
| [8] | Yellamma, N. Manjunatha, Umair Khan, Samia Elattar, Sayed M. Eldin, Jasgurpreet Singh Chohan, R. Sumithra, K. Sarada . Onset of triple-diffusive convective stability in the presence of a heat source and temperature gradients: An exact method. AIMS Mathematics, 2023, 8(6): 13432-13453. doi: 10.3934/math.2023681 |
| [9] | Maxime Krier, Julia Orlik . Solvability of a fluid-structure interaction problem with semigroup theory. AIMS Mathematics, 2023, 8(12): 29490-29516. doi: 10.3934/math.20231510 |
| [10] | S. R. Mishra, Subhajit Panda, Mansoor Alshehri, Nehad Ali Shah, Jae Dong Chung . Sensitivity analysis on optimizing heat transfer rate in hybrid nanofluid flow over a permeable surface for the power law heat flux model: Response surface methodology with ANOVA test. AIMS Mathematics, 2024, 9(5): 12700-12725. doi: 10.3934/math.2024621 |
The goal of this paper is to provide analytical assessments to a fluid flowing in a porous medium with a non-linear diffusion linked to a degenerate diffusivity. The viscosity term is formulated with an Eyring-Powell law, together with a non-homogeneous diffusion typical of porous medium equations (as known in the theory of partial differential equations). Further, the equation is supplemented with an absorptive reaction term of Darcy-Forchheimer, commonly used to model flows in porous medium. The work starts by analyzing regularity, existence and uniqueness of solutions. Afterwards, the problem is transformed to study travelling wave kind of solutions. An asymptotic expansion is considered with a convergence criteria based on the geometric perturbation theory. Supported by this theory, there exists an exponential decaying rate in the travelling wave profile. Such exponential behaviour is validated with a numerical assessment. This is not a trivial result given the degenerate diffusivity induced by the non-linear diffusion of porous medium type and suggests the existence of regularity that can serve as a baseline to construct numerical or energetic approaches.
K1: Permeability of the medium.
K2: Inertial permeability.
L: Fluid characteristic length.
M: Hartmann number.
MHD: Magnetohydrodynamics.
PME: Porous Medium Equation.
Re: Reynolds.
U0: Fluid characteristic velocity.
ρ: Fluid density.
μ: Fluid viscosity.
ν: Fluid kinematic viscosity.
B0: Applied magnetic field.
σ: Electrical conductivity in the medium.
Any kind of material exhibiting pores filled by a flowing fluid leads to a physical interpretation beyond the Newtonian description related with viscosity and diffusion. Currently, flow in porous medium is a source of research, not only because of purely mathematical interests, but also because of their applications in electrochemical, mechanical, geophysical, metallurgical and chemical processes. In 1856, the observations made by Henry Darcy [1] associated to fluid flow in porous medium led to the formulation of a physical law to describe the basic dynamic experienced. Such law was intended to describe the fluid principles for low Reynolds numbers, typical of flows in this kind of porous medium. Afterwards, Forchheimer in 1901 [2] and Jaeger in 1956 [3] provided an extension of Darcy's law to account for turbulent flows at higher Reynolds numbers. Based on these works, the Darcy's law was renamed as Darcy-Forchheimer's law by Mustak in [4] and [5].
It shall be noted that the above mentioned researchers considered the viscosity and diffusive terms as provided by the classical Newtonian description. Nonetheless and since then, several researchers have tried to extend the viscosity formulation to increase the modelling accuracy beyond the Newtonian scope. As a set of representative examples of the described non-Newtonian fluid models, the reader is referred to the works [6,7,8,9] together with references therein. The non-Newtonian fluids are classified into several classes. It is relevant to highlight that efforts have been made to describe diffusion phenomena based on the kinetic theory of liquids rather than on experimental or intuitive principles related with viscosity. Such efforts were conducted with success leading to a kind of non-Newtonian fluid known as Eyring-Powell. The use of the kinetic theory of liquids to describe viscosity and diffusive mechanisms makes the Eyring-Powell fluid particularly attractive to model flows given by electrically conducting particles arising in Magnetohydrodynamics (MHD). In a set of interesting analysis, Akbar et al. [10] obtained the numerical results for two dimensional MHD Erying-Powell fluid over stretching surface. Hina et al. [11] analyzed the heat transfer for MHD Erying-Powell fluid under the slip effect at the boundary. Bhatti et al. [12] considered two dimensional MHD Erying-Powell fluid under permeable surface and analyzed the heat transfer by converting the equations into nonlinear ordinary equations and that are solved based on numerical principles. Other interesting results in non-Newtonian fluids are given in the references [13,14,15,16,17,18], but particularly there are some recent achievements in this regards to highlight: In [19] a modified Eyring-Powell model is introduced to improve predictions related with the Eyring-Powell parameter on flow velocity. In [20], the authors discuss the adequacy of Eyring-Powell models for viscous dissipation in a flow past a convectively heated stretching sheet. Furthermore, in [21] and [22], an Eyring-Powell fluid is considered to model Coriolis effects over a non-uniform surfaces together with a discussion on the adequacy of the formulation. Finally, the reader is referred to [23] and [24] for discussions on other types of fluids and other types of analysis based on purely numerical schemes respectively.
Other techniques have been of help to describe flows under non-Newtonian approaches. For instance, some kinds of solutions known as travelling and solitary waves have provided interesting results from analytical and numerical perspective. In this regard, the reader is referred to the set of studies [25,26,27].
As a main research question driving the present study, it shall be mentioned the idea of searching for appropriate regularity conditions even when the Eyring-Powell viscosity term is formulated with a degenerate diffusion. Specially but not limited, the question of finding an exponential profile of solution.
Let us start by considering a one dimensional Darcy-Forchheimer flow with a diffusion term given by an Erying-Powell principle. The velocity vector is then V=(v1,0,0) where v1(y,t) is the velocity profile in the x−direction that is dependant on the transversal direction y. The geometry under study is given by an open porous domain where the flow is developed through the x−direction and is initially distributed by an initial condition in the y−direction. In addition, the diffusion is further modified to account for a nonlinear term of the form ∂2vm1∂y2 (refer to [28] and [29] for a complete discussion about this kind of non-linearity and to [14,15,16] for different applications of particular diffusion terms). Note that the basic homogeneous problem
| ∂v1∂t=∂2vm1∂y2,m>1, | (1.1) |
is well known as porous medium equation. The PME is a nonlinear parabolic equation with degenerate diffusivity. Indeed, the diffusivity is given by D(v1)=mvm−11 that is null whenever v1=0. This degeneracy leads to the loss of a positive principle for null (or slightly null) solutions, and hence to the loss of regularity as understood for gaussian diffusion problems.
Based on the exposed principles, the equation under study emerges from the MHD theory and reads
| ∂v1∂t=−1ρdPdx+(ν+1βd1ρ)∂2vm1∂y2−12βd31ρ(∂v1∂y)2∂2v1∂y2−(σB20ρ+νK1)v1−v21K2, | (1.2) |
where m>1, P is the pressure field acting along the x−direction and β and d1 are the characteristic constants of the Eyring-Powell fluid model.
To account for a single general equation, introduce the following non-dimensional quantities (see [30]) as follows
| v∗1=v1U0,x∗=xL,y∗=yL,t∗=U0tL,P∗=PρU20,A1=1νβρd1ϵ=12βρd31L3,A2=LνU0K1,A3=LK2,A4=Um−10Re, | (1.3) |
where L is a characteristic length that can be given by the pores dimension. After replacement of the set of expressions in (1.3) into the Eq (1.2) (ignoring * for simplicity)
| ∂v1∂t=−1ρdPdx+A4(1+A1)∂2vm1∂y2−ϵ(∂v1∂y)2∂2v1∂y2−(M2Re+A2)v1−A3v21, | (1.4) |
where Re=U0Lν is the Reynolds number and and M=B0√Lσρ refers to the Hartmann number. Making the differentiation of Eq (1.4) with respect to x
| −1ρdPdx=A5. |
After using the value of dPdx in Eq (1.4)
| ∂v1∂t=A5+A4(1+A1)∂2vm1∂y2−ϵ(∂v1∂y)2∂2v1∂y2−(M2Re+A2)v1−A3v21. | (1.5) |
In addition, consider the following initial generalized condition
| v1(y,0)=v0(y)∈L1(R)∩L∞(R), | (1.6) |
where v0(y) is the initial velocity that represents any kind of non-homogeneous distribution with the only requirement to be bounded in L∞−norm and under a finite flowing mass quantity, i.e., a bound in L1−norm. In addition, note that this initial condition allows modelling any kind of irregularities or discontinuities that may exist in the porous medium involved.
Given the degeneracy in the diffusivity introduced by the non-linear term ∂2vm1∂y2 and given the generalized initial velocity distribution, solutions are analyzed based on a weak formulation. To this end, assume the existence of a test function Ω∈C∞(R) with compact support (or at least with a decreasing behaviour over R) such that for 0<τ<t<T
| ∫Rv1(t)Ω(t)dy=∫Rv1(τ)Ω(τ)dy+∫tτ∫Rv1(t)∂Ω∂sdyds+A5∫tτ∫RΩdyds+A4(1+A1)∫tτ∫Rvm1∂2Ω∂y2dyds+ϵ3∫tτ∫R(∂v1∂y)3∂Ω∂y−(M2Re+A2)∫tτ∫Rv1Ωdyds−A3∫tτ∫RΩv21dyds. | (2.1) |
Given a finite r0, admit the following ball Br centered in r0 and with radium r>>r0, such that the following equation is defined
| v1(t)∂Ω∂s+A5Ω+A4(1+A1)vm1∂2Ω∂y2+ϵ3(∂v1∂y)3∂Ω∂y−(M2Re+A2)v1Ω−A3Ωv21=0, | (2.2) |
in Br×[0,T], with the following boundary like and initial conditions
| 0<∂Ω∂y=Ω<<1,v1(y,0)=v0(y)∈L1(R)∩L∞(R). | (2.3) |
Firstly, the following theorem expresses the regularity properties of any solution to (1.5), i.e., solutions exist globally.
Theorem 2.1. Given v0(y)∈L1(R)∩L∞(R), then any non-negative solution (v1≥0) to (1.2) is bounded for all(y,t)∈Br×[0,T] with r>>1.
Proof. Consider λ∈R+, the following supporting cut-off function is defined as
| ψλ∈C∞0, 0≤ψλ≤1,ψλ=1inBr−λ,ψλ=0inR−Br−λ, |
such that
| |∂ψλ∂λ|=Ccλ. |
Multiplying Eq (2.2) by ψλ and integrating over Br×[τ,T]
| ∫tτ∫Brv1∂Ω∂sψλdyds+A5∫tτ∫RΩψλdyds=−A4(1+A1)∫tτ∫Rvm1∂2Ω∂y2ψλdyds−ϵ3∫tτ∫R(∂v1∂y)3∂Ω∂yψ5dyds+(M2Re+A2)∫tτ∫Rv1Ωψλdyds+A3∫tτ∫RΩv21ψλdyds. | (2.4) |
Based on some results in [28] and [29], for some large r>>r0>1 the following holds
| ∫tτv1ds≤∫tτvm1ds≤C1(τ)r2mm−1, | (2.5) |
and
| ∫tτv21ds≤C1(τ)r4. | (2.6) |
Considering the spatial variable y close to ∂Br, it can be assumed y∼r. Then
| ∫tτ(∂v1∂y)3ds≤8m3m−1C31(τ)r3m+3m−1. | (2.7) |
After using the expression (2.4) and after integration in the nonlinear diffusion term, the following holds
| −A4(1+A1)∫tτ∫Brvm1∂2Ω∂y2ψλdyds≤A4(1+A1)C1(τ)r2mm−1((∂Ω∂yψλ)∂Br−∫Br∂Ω∂y∂ψλ∂ydyds). |
Since r>>1 and since Ω is sufficiently small and regular in the asymptotic approach, it is possible to choose Ω in such a way ∂Ω∂yψλ<<1 in the proximity of the boundary ∂Br. Then, the above expression becomes
| −A4(1+A1)∫tτ∫Brvm1∂2Ω∂y2ψλdyds≤−A4(1+A1)C1(τ)∫Brr2mm−1∂Ω∂y∂ψλ∂ydyds≤A4(1+A1)C1(τ)∫Brr2mm−1∂Ω∂y∂ψλ∂ydyds. | (2.8) |
Based on (2.7), the following integral holds
| −ϵ3∫Br∫tτ(∂v1∂y)3∂Ω∂yψλdyds≤8ϵm33(m−1)3C31(τ)∫Brr3m+3m−1Ω∂ψλ∂ydy. | (2.9) |
After using the expressions (2.5), (2.6), (2.8) and (2.9) into (2.4), the following holds
| ∫tτ∫Brv1∂Ω∂sψλdyds+A5∫tτ∫RΩψλdyds≤A4(1+A1)C1(τ)∫Brr2mm−1∂Ω∂y∂ψλ∂ydy+8ϵm33(m−1)3C31(τ)∫Brr3m+3m−1Ω∂ψλ∂ydy+(M2Re+A2)C1(τ)∫Brr2mm−1Ωψλdy+A3C1(τ)∫Brr4Ωψλdy≤A4(1+A1)C1(τ)∫Brr2mm−1∂Ω∂yCcλdy+8ϵm33(m−1)3C31(τ)∫Brr3m+3m−1ΩCcλdy+(M2Re+A2)C1(τ)∫Brr2mm−1Ωψλdy+A3C1(τ)∫Brr4Ωψλdy, |
which implies that
| ∫tτ∫Brv1∂Ω∂sdyds+A5∫tτ∫RΩdyds≤A4(1+A1)CcC1(τ)∫Brrm+1m−1∂Ω∂ydy+8ϵm33(m−1)3CcC31(τ)∫Brr2m+4m−1Ωdy+(M2Re+A2)C1(τ)∫Brr2mm−1Ωdy+A3C1(τ)∫Brr4Ωdy. | (2.10) |
Consider a test function Ω∈C∞(R) of the form
| Ω(r,s)=e−ls(1+r2)−α. | (2.11) |
Choose α in a way that the expression (2.10) is convergent, therefore
| ∫tτ∫Brv1∂Ω∂sdyds+A5∫tτ∫RΩdyds≤2A4(1+A1)CcC1(τ)∫Bre−lsr2m−1−2αdy+8ϵm33(m−1)3CcC31(τ)∫Bre−lsr2m+4m−1−2αdy+(M2Re+A2)C1(τ)∫Bre−lsr2mm−1−2αdy+A3C1(τ)∫Bre−lsr4−2αdy. | (2.12) |
For convergence, take α>m+2m−1 and r→∞, so that the expression (2.12) becomes
| 0≤∫tτ∫Brv1∂Ω∂sdyds+A5∫tτ∫RΩdyds≤Υ, | (2.13) |
where Υ is a suitable value obtained for each Br and is sufficiently small for r→∞; hence, a finite value of Υ holds. Note that Ω and v1 are not negative, then the integrals in the expression (2.13) are finite in τ<s<t≤T, which shows that the solution v1 is bounded in R×[0,T].
Now, it is the aim to show additional regularity results, in particular that ∂v1∂y is bounded.
Theorem 2.2. Assume that v1(y) is a non-negative solution to Eq (1.5), then ∂v1∂y is bounded for all(y,t)∈R×[0,T].
Proof. Multiplying Eq (1.5) by v1 and applying integration by parts, the following holds
| 12ddt∫R|v1|2dy=A5∫Rv1dy−mA4(1+A1)∫Rvm−11(∂v1∂y)2dy+ϵ3∫R(∂v1∂y)4dy−(M2Re+A2)∫Rv21dy−A3∫Rv31dy, |
which implies that
| ∫R(∂v1∂y)2[ϵ3(∂v1∂y)2−mA4(1+A1)vm−11]dy=12ddt∫R|v1|2dy−A5∫Rv1dy+(M2Re+A2)∫Rv21+A3∫Rv31dy. |
Now, applying the integration done
| ∫t0∫R(∂v1∂y)2[ϵ3(∂v1∂y)2−mA4(1+A1)vm−11]dyds=12∫R|v1|2dy−∫R|v0(y)|2dy−A5∫t0∫Rv1dyds+(M2Re+A2)∫t0∫Rv21dyds+A3∫Rv31dyds. | (2.14) |
The Theorem 2.1 established the bound properties of non-negative solutions, as a consequence the right hand side of Eq (2.14) is bounded. Choose a finite bounding constant A5 such that
| ∫t0∫R(∂v1∂y)2[ϵ3(∂v1∂y)2−mA4(1+A1)vm−11]dyds≤A5, |
leading to conclude on the bound of ∂v1∂y.
The degeneracy introduce by the non-linear diffusion term may lead to the loss of uniqueness of non-negative solutions. Hence, a result about uniqueness of solutions is required to be shown.
Theorem 2.3. Let us consider two non-negative solutions to the Eq (1.5), v11(y,t) andv12(y,t). Then, if both solutions have the same initial distribution, uniqueness holds, i.e., v11(y,t)=v12(y,t).
Proof. First of all, define a test function Ψ(y,t)∈C∞(QT), where QT=R×[0,T]. The weak formulations for both solutions v11 and v22 are given as
| ∫Rv11(y,t)Ψ(y,t)dy=∫Rv11(y,0)Ψ(y,0)dy+∫t0∫Rv11∂Ψ∂sdyds+A4(1+A1)∫t0∫Rvm11∂2Ψ∂y2dyds+A5∫t0∫RΨdydt+ϵ3∫t0∫R(∂v11∂y)4∂Ψ∂ydyds−(M2Re+A2)∫t0∫Rv11Ψdydt−A3∫t0∫Rv211Ψdydt, | (2.15) |
and
| ∫Rv12(y,t)Ψ(y,t)dy=∫Rv12(y,0)Ψ(y,0)dy+∫t0∫Rv12∂Ψ∂sdyds+A4(1+A1)∫t0∫Rvm12∂2Ψ∂y2dyds+A5∫t0∫RΨdydt+ϵ3∫t0∫R(∂v12∂y)4∂Ψ∂ydyds−(M2Re+A2)∫t0∫Rv12Ψdydt−A3∫t0∫Rv212Ψdydt. | (2.16) |
Assume v11≥v12. After substraction, the following holds
| ∫R(v11−v12)(y,t)Ψ(y,t)dy=∫t0∫R(v11−v12)(y,t)∂Ψ∂sdyds+A4(1+A1)∫t0∫R(vm11−vm12)(y,t)∂2Ψ∂y2dyds+ϵ3∫t0∫R((∂v11∂y)4−(∂v12∂y)4)∂Ψ∂ydyds−(M2Re+A2)∫t0∫R(v11−v12)(y,t)Ψ(y,t)dydt−A3∫t0∫R(v211−v212)(y,t)Ψ(y,t)dydt. | (2.17) |
For v11(y,t)>v12(y,t), the following holds
| vm11−vm12≤mvm−111(v11−v12)≤mCm−12(v11−v12), | (2.18) |
where C2=max(y,t)∈QT(v11). Now, consider
| ((∂v11∂y)4−(∂v12∂y)4)=(∂v11∂y−∂v12∂y)(∂v11∂y+∂v12∂y)((∂v11∂y)2+(∂v12∂y)2)≤C3(∂v11∂y−∂v12∂y), | (2.19) |
where C3 is a suitable bounding constant that can be defined supported by the regularity results shown in Theorem 2.2.
Based on the expressions (2.18) and (2.19), the Eq (2.17) becomes
| ∫R(v11−v12)(y,t)Ψ(y,t)dy=∫t0∫R(v11−v12)(y,t)∂Ψ∂sdyds+(mCm−12A4(1+A1)−ϵC33)∫t0∫R(v11−v12)(y,t)∂2Ψ∂y2dyds−(M2Re+A2−2A3C2)∫t0∫R(v11−v12)(y,t)Ψdyds. | (2.20) |
Assume the following smooth shape test function defined as
| Ψ(y,s)=e−as(1+y2)−γ1, | (2.21) |
where γ1 can be chosen such that
| ∫RΨ(y,t)dy<∞. | (2.22) |
For simplicity
| ∫RΨ(y,t)dy=1. | (2.23) |
For |y|→∞, the integral mass shall be null. Then, for R>>1
| ∫||y|−R|→∞Ψ(y,t)dy=0. | (2.24) |
In the asymptotic approximation |y|→∞
| |y|2γ1|y|→0, | (2.25) |
then
| γ1>12. | (2.26) |
Now, define
| ∫Rψ(y,s)dy=e−as∫R1(1+y2)γ1dy=e−asχ(y), | (2.27) |
where
| χ(y)=∫y→∞1(1+y2)γ1dy. | (2.28) |
The integral (2.28) is finite under the condition for γ1 in (2.26).
Now, differentiate (2.21) with respect to y, so that
| ∂2Ψ∂y2=4γ1(γ1+1)y2e−as(1+y2)γ1+2−2γ1(1+y2)γ1+1≤4γ1(γ1+1)e−as(1+y2)γ1=4γ1(γ1+1)Ψ. |
After integration
| ∫R∂2Ψ∂y2≤C4(γ1)Ψ=C4(γ1)e−asχ(y), |
where C4(γ1)=4γ1(γ1+1).
Now, the intention is to assess each of the integrals involved in the right hand side of Eq (2.20)
| ∫t0∫R(v11−v12)(y,t)∂Ψ∂sdyds=−a∫t0∫R(v11−v12)(y,t)Ψdyds≤a∫t0∫R(v11−v12)(y,t)Ψdyds≤asup|v11−v12|χ(y)∫t0e−asds=asup|v11−v12|χ(y)(1−e−as). | (2.29) |
| (mCm−12A4(1+A1)−ϵC33)∫t0∫R(v11−v12)(y,t)∂2Ψ∂y2dyds≤(mCm−12A4(1+A1)−ϵC33)sup|v11−v12|C4(γ1)χ(y)∫t0∫RΨ(y,t)dyds=(mCm−12A4(1+A1)−ϵC33)sup|v11−v12|C4(γ1)χ(y)∫t0e−asds=(mCm−12A4(1+A1)−ϵC33)sup|v11−v12|C4(γ1)χ(y)(1−e−at), | (2.30) |
and similarly
| −(M2Re+A2−2A3C2)∫t0∫R(v11−v12)(y,t)Ψdyds≤(M2Re+A2−2A3C2)sup|v11−v12|χ(y)(1−e−at). | (2.31) |
Combining the assessments in (2.29), (2.30) and (2.31) with the Eq (2.20), the following holds
| ∫R(v11−v12)(y,t)Ψ(y,t)dy≤asup|v11−v12|χ(y)(1−e−as)+(mCm−12A4(1+A1)−ϵC33)sup|v11−v12|C4(γ1)χ(y)(1−e−at)+(M2Re+A2−2A3C2)sup|v11−v12|χ(y)(1−e−at). | (2.32) |
Since |χ(y)|<∞ and for t>0, with sup|v11−v12|→0
| ∫R(v11−v12)(y,t)Ψ(y,t)dy≤0, |
which implies that v11≤v12. This result contradicts the initial assumption v11≥v12. As a consequence, the only compatible result is to consider v11=v12, leading to conclude on solutions uniqueness.
The travelling wave profiles of solutions are given as per the following definitions: v1(y,t)=k(ζ), where ζ=y−a1t, a1 denotes travelling wave speed and the profile k:R→(0,∞) belongs to L∞(R). The Eq (1.5) is then transformed in terms of the travelling wave profile as
| −a1k′=A5+A4(1+A1)(km)″ | (3.1) |
Let us introduce the following new variables
| \begin{eqnarray} Y = k(\zeta){\rm{, }} \, \, \, \, \, Z = \left(k^{m}\right)', \end{eqnarray} | (3.2) |
such that the Eq (3.1) can be analyzed in a phase space driven by the following set of equations
| \begin{eqnarray} Y'& = &\frac{1}{m}Y^{1-m}Z\\ Z'& = &\frac{m^{3}\left[-\frac{a_{1}}{m}Y^{1-m}Z +\frac{\epsilon(1-m)}{m^{3}}Y^{2-3m}Z^{3} -A_{5}+\left(\frac{M^{2}}{R_{e}}+A_{2}\right)Y +A_{3}Y^{2}\right]}{m^{3}+A_{4}\left(1+A_{1}\right)- \epsilon Y^{3-3m}Z^{2}}. \end{eqnarray} | (3.3) |
Making Y' = 0 and Z' = 0 to determine the critical points, the following is obtained
| \begin{eqnarray} Y^{2}+\left(\frac{M^{2}}{A_{3}R_{e}}+\frac{A_{2}}{A_{3}}\right)Y -\frac{A_{5}}{A_{3}} = 0. \end{eqnarray} | (3.4) |
with solutions
| \begin{eqnarray} Y_{1} = -\frac{1}{2}\left(\frac{M^{2}}{R_{e}}+\frac{A_{2}}{A_{3}}\right) +\frac{1}{2}\sqrt{\left(\frac{M^{2}}{A_{3}R_{e}}+\frac{A_{2}}{A_{3}}\right)^{2} +\frac{4A_{5}}{A_{3}}} \end{eqnarray} | (3.5) |
and
| \begin{eqnarray} Y_{2} = -\frac{1}{2}\left(\frac{M^{2}}{R_{e}}+\frac{A_{2}}{A_{3}}\right) -\frac{1}{2}\sqrt{\left(\frac{M^{2}}{A_{3}R_{e}}+\frac{A_{2}}{A_{3}}\right)^{2} +\frac{4A_{5}}{A_{3}}}. \end{eqnarray} | (3.6) |
Consequently, the system critical points are given by (Y_{1}, 0) and (Y_{2}, 0) . The next intention is to characterize such critical points together with the bundles of orbits in their proximity.
The Geometric perturbation theory is used to show the asymptotic behavior of specifically defined manifolds in the proximity of the system critical points. Firstly, assume the following manifold I_0 defined as
| \begin{equation*} \begin{split} I_{0} = [ \, \, (Y,Z) \, \, / \, \, \, & Y' = \frac{1}{m}Y^{1-m}Z; \\& Z' = \frac{m^{3}\left[-\frac{a_{1}}{m}Y^{1-m}Z +\frac{\epsilon(1-m)}{m^{3}}Y^{2-3m}Z^{3} -A_{5}+\left(\frac{M^{2}}{R_{e}}+A_{2}\right)Y +A_{3}Y^{2}\right]}{m^{3}+A_{4}\left(1+A_{1}\right)- \epsilon Y^{3-3m}Z^{2}} \, \, ], \end{split} \end{equation*} |
with the system critical points (Y_{1}, 0) and (Y_{2}, 0).
A perturbed manifold I_{\beta} close to I_{0} in the critical point (Y_{1}, 0) is defined as
| \begin{eqnarray} I_{\beta} = \left[(Y,Z) \, \, / \, \, Y' = \beta Z; \, \, Z' = C_{5}\beta\left(Y-Y_{2}\right)\right], \end{eqnarray} | (3.7) |
where \beta denotes a perturbation parameter close to the equilibrium \left(Y_{1}, 0\right) and C_{5} is a suitable constant obtained after root factorization. For the coming assessments, assume Y_{3} = Y-Y_{2} .
The intention is to apply the Fenichel invariant manifold theorem [31] as formulated in [32] and [33]. To this end, it shall be shown that I_{0} is a normally hyperbolic manifold, i.e., the eigenvalues associated to I_{0} in the linear frame proximal to the critical points, and transversal to the tangent space, have non-zero real part. This is shown based on the following equivalent linear flow associated to I_{\beta}.
| \begin{eqnarray*} \begin{bmatrix} Y'_{3}\\ Z' \end{bmatrix} = \begin{bmatrix} 0 & \beta\\ C_{5}\beta & 0 \end{bmatrix} \begin{bmatrix} Y_{3}\\ Z \end{bmatrix} . \end{eqnarray*} |
The related eigenvalues are both real. Consequently, I_{0} is a hyperbolic manifold. Now, it is shown that the manifold I_{\beta} is locally invariant under the flow (3.3), so that the manifold I_{0} can be shown as an asymptotic approach to I_{\beta}. For this basis, consider the functions
| \begin{eqnarray*} \psi^{I_0}& = & \frac{m^{3}\left[-\frac{a_{1}}{m}Y^{1-m}Z +\frac{\epsilon(1-m)}{m^{3}}Y^{2-3m}Z^{3} -A_{5}+\left(\frac{M^{2}}{R_{e}}+A_{2}\right)Y +A_{3}Y^{2}\right]}{m^{3}+A_{4}\left(1+A_{1}\right)- \epsilon Y^{3-3m}Z^{2}}, \\ \psi^{I_\beta}& = & C_{5}\beta Y_{3}, \end{eqnarray*} |
which are C^{i}\left(R\times\left[0, \delta_{1}\right]\right), \, i \geq 1 , in the proximity of the critical point \left(Y_{1}, 0\right). In this case, \delta_{1} is determined based on the following flows that are considered to be measurable a.e. in R
| \begin{eqnarray*} \left\|\psi^{I_{\beta}} -\psi^{I_{0}}\right\|\leq \left\| C_{5}\beta Y_{3} - \varepsilon_0 \right\|\leq C_{5}\beta \left\|Y_{3}\right\|\leq\delta_{1}\beta, \end{eqnarray*} |
where \varepsilon_0 is the infinitesimal distance between the critical point (Y_1, 0) and the flow \psi^{I_{0}} , which exists given the normal hyperbolic condition of I_0 . As the solutions have been shown to be bounded, it is possible to conclude on a finite \delta_{1} . So the distance between the manifolds holds the normal hyperbolic condition for \delta_{1}\in\left(0, \infty\right) and \beta sufficiently small in the proximity of the critical point \left(Y_{1}, 0\right).
Now, consider a perturbed manifold I_{\gamma} associated to I_{0} , but for the critical point (Y_{2}, 0) and defined as
| \begin{eqnarray} I_{\gamma} = \left[Y,Z/Y' = \gamma Z; Z' = C_{6}\gamma\left(Y-Y_{1}\right)\right], \end{eqnarray} | (3.8) |
where \gamma denotes a perturbation parameter in the proximity of the equilibrium \left(Y_{2}, 0\right) and C_{6} is a suitable constant obtained after root factorization. Suppose Y_{4} = Y-Y_{1} , then the following flow associated to I_{\gamma} is given by
| \begin{eqnarray*} \begin{bmatrix} Y'_{4}\\ Z' \end{bmatrix} = \begin{bmatrix} 0 & \gamma\\ C_{6}\gamma & 0 \end{bmatrix} \begin{bmatrix} Y_{4}\\ Z \end{bmatrix} . \end{eqnarray*} |
The associated eigenvalues are both real, leading to state that I_{0} is, indeed, a hyperbolic manifold. Now, it is shown that the manifold I_{\gamma} is locally invariant under the flow (3.3), so that the manifold I_{0} can be shown as an asymptotic approach to I_{\gamma}. For this basis consider the functions
| \begin{eqnarray*} \psi^{I_0}& = & \frac{m^{3}\left[-\frac{a_{1}}{m}Y^{1-m}Z +\frac{\epsilon(1-m)}{m^{3}}Y^{2-3m}Z^{3} -A_{5}+\left(\frac{M^{2}}{R_{e}}+A_{2}\right)Y +A_{3}Y^{2}\right]}{m^{3}+A_{4}\left(1+A_{1}\right)- \epsilon Y^{3-3m}Z^{2}}, \\ \psi^{I_\gamma}& = & C_{6}\gamma Y_{4}, \end{eqnarray*} |
which are C^{i}\left(R\times\left[0, \delta_{1}\right]\right), \, i \geq 1 , in the proximity of the critical point \left(Y_{2}, 0\right). In this case, \delta_{1} is determined based on the following flows that are considered to be measurable a.e. in R:
| \begin{eqnarray*} \left\|\psi^{I_{\gamma}} -\psi^{I_{0}}\right\|\leq \left\| C_{6}\gamma Y_{4} - \varepsilon_1 \right\|\leq C_{6}\gamma \left\|Y_{4}\right\|\leq\delta_{1}\beta, \end{eqnarray*} |
where \varepsilon_1 is the infinitesimal distance between the critical point (Y_2, 0) and the flow \psi^{I_{0}} , which exists given the normal hyperbolic condition of I_0 . As the solutions are bounded, \delta_{1} is finite. Hence, the distance between the manifolds holds the normal hyperbolic condition for \delta_{1}\in\left(0, \infty\right) and \gamma sufficiently small, in the proximity of the critical point \left(Y_{2}, 0\right).
Since the manifold I_{0} has been shown to satisfy the normal hyperbolic condition under the flow (3.3), it is possible to obtain asymptotic profiles operating in the linearized flows I_\beta and I_\gamma .
For this purpose, consider the Eq (3.3) such that a family of trajectories in the phase plane (Y, Z) can be obtained by
| \begin{eqnarray} \frac{dZ}{dY} = \frac{m^{4}\left[-\frac{a_{1}}{m}Y^{1-m}Z +\frac{\epsilon(1-m)}{m^{3}}Y^{2-3m}Z^{3} -A_{5}+\left(\frac{M^{2}}{R_{e}}+A_{2}\right)Y +A_{3}Y^{2}\right]}{Y^{1-m}Z\left[m^{3}+A_{4}\left(1+A_{1}\right)- \epsilon Y^{3-3m}Z^{2}\right]} = H(Y,Z).\\ \end{eqnarray} | (3.9) |
For Y \gg 1 , the function H > 0 while for 0 < Y \ll 1, the function H < 0 . In addition, as the function H is singular for Z = 0 , a trajectory in the proximity of the critical condition (Y_{1}, 0) shall require
| \begin{eqnarray} -\frac{a_{1}}{m}Y_{1}^{1-m}Z_{1} +\frac{\epsilon(1-m)}{m^{3}}Y_{1}^{2-3m}Z_{1}^{3} -A_{5}+\left(\frac{M^{2}}{R_{e}}+A_{2}\right)Y_{1} +A_{3}Y_{1}^{2} = 0. \end{eqnarray} | (3.10) |
After using the Eq (3.3) into Eq (3.10), the following holds
| \begin{eqnarray} \epsilon(1-m)\left(Y_{1}'\right)^{3} -a_{1}Y_{1}Y_{1}' -A_{5}Y_{1}+\left(\frac{M^{2}}{R_{e}}+A_{2}\right)Y_{1}^{2} +A_{3}Y_{1}^{3} = 0. \end{eqnarray} | (3.11) |
Typically, the flow velocity in a porous medium permits the hypothesis U_0 \ll 1 . In addition, in the assumption of a sufficiently large characteristic length, L \gg 1 , the parameter \epsilon \ll 1 (see (1.3)) for typical fluid densities. As a consequence, such \epsilon is considered to construct a solution as an asymptotic expansion in terms of the mentioned parameter \epsilon , understood as a perturbation and as given in (1.3). The selection of this parameter permits to account for a physical nuisance as \epsilon is related with some of the constants involved in the fluid problem. Then, the following expansion holds for suitable regular functions Y_{1j}, \, j = 0, 1, 2...
| \begin{eqnarray} Y_{1} = Y_{10}+\epsilon Y_{11}+\epsilon^{2} Y_{12}+... \end{eqnarray} | (3.12) |
After using (3.12) into (3.11), the following holds
| \begin{eqnarray} \epsilon(1-m)\left(Y_{10}'+\epsilon Y_{11}'+\epsilon^{2} Y_{12}'+...\right)^{3} & = &a_{1}\left(Y_{10}+\epsilon Y_{11}+\epsilon^{2} Y_{12}+...\right) \left(Y_{10}'+\epsilon Y_{11}'+\epsilon^{2} Y_{12}'+...\right)\\ &+&A_{5}\left(Y_{10}+\epsilon Y_{11}+\epsilon^{2} Y_{12}+...\right)\\ &-&\left(\frac{M^{2}}{R_{e}}+A_{2}\right)\left(Y_{10}+\epsilon Y_{11}+\epsilon^{2} Y_{12}+...\right)^{2}\\ &-&A_{3}\left(Y_{10}+\epsilon Y_{11}+\epsilon^{2} Y_{12}+...\right)^{3}. \end{eqnarray} | (3.13) |
Comparing the terms of orders \epsilon^{0} and \epsilon^{1}
| \begin{eqnarray} Y_{10}' = -\frac{A_{5}}{a_{1}} +\left(\frac{M^{2}}{R_{e}a_{1}}+\frac{A_{2}}{a_{1}}\right)Y_{10} +\frac{A_{3}}{a_{1}}\left(Y_{10}\right)^{2}, \end{eqnarray} | (3.14) |
and
| \begin{eqnarray} Y_{11}'+\left(\frac{Y_{10}'}{Y_{10}} +\frac{A_{5}}{a_{1}Y_{10}} -\frac{2M^{2}}{R_{e}a_{1}}+\frac{2A_{2}}{a_{1}}-\frac{3A_{3}Y_{10}}{a_{1}} \right)Y_{11} = \frac{(1-m)\left(Y_{10}'\right)^{3}}{a_{1}Y_{10}}. \end{eqnarray} | (3.15) |
The standard resolution of (3.14) leads to
| \begin{eqnarray} Y_{10}& = &\frac{1}{1-e^{-\frac{2A_{3}b}{a_{1}}\zeta}} \Big[\left(\frac{M^{2}}{2A_{3}R_{e}}+\frac{A_{2}}{2A_{3}}\right) +\sqrt{\left(\frac{M^{2}}{2A_{3}R_{e}}+\frac{A_{2}}{2A_{3}}\right)^{2}+\frac{A_{5}}{A_{3}}}\\ &+&\left(\left(\frac{M^{2}}{2A_{3}R_{e}}+\frac{A_{2}}{2A_{3}}\right) -\sqrt{\left(\frac{M^{2}}{2A_{3}R_{e}}+\frac{A_{2}}{2A_{3}}\right)^{2}+\frac{A_{5}}{A_{3}}}\right) e^{-\frac{2A_{3}\sqrt{\left(\frac{M^{2}}{2A_{3}R_{e}}+\frac{A_{2}}{2A_{3}}\right)^{2}+\frac{A_{5}}{A_{3}}}}{a_{1}}\zeta}\Big].\\ \end{eqnarray} | (3.16) |
Differentiate (3.16) to get
| \begin{eqnarray} Y_{10}'-\frac{\left(\frac{M^{2}}{A_{3}R_{e}} +\frac{A_{2}}{A_{3}}\right)\frac{2A_{3}\sqrt{\left(\frac{M^{2}}{2A_{3}R_{e}}+\frac{A_{2}}{2A_{3}}\right)^{2}+\frac{A_{5}}{A_{3}}}} {a_{1}}e^{-\frac{2A_{3}\sqrt{\left(\frac{M^{2}}{2A_{3}R_{e}}+\frac{A_{2}}{2A_{3}}\right)^{2}+\frac{A_{5}}{A_{3}}}}{a_{1}}\zeta}} {\left(1-e^{-\frac{2A_{3}\sqrt{\left(\frac{M^{2}}{2A_{3}R_{e}}+\frac{A_{2}}{2A_{3}}\right)^{2}+\frac{A_{5}}{A_{3}}}}{a_{1}}\zeta}\right)^{2}}. \end{eqnarray} | (3.17) |
Upon substitution of (3.16) and (3.17) in (3.15), it holds
| \begin{eqnarray} Y_{11}'&+&\left[-\frac{2abe^{-b\zeta}}{\left(1-e^{-b\zeta}\right)\left(c+de^{-b\zeta}\right)} +\frac{A_{5}\left(1-e^{-b\zeta}\right)}{a_{1}\left(c+de^{-b\zeta}\right)} +f -\frac{3A_{3}\left(c+de^{-b\zeta}\right)}{a_{1}\left(1-e^{-b\zeta}\right)}\right]Y_{11}\\ & = &-\frac{(1-m)8a^{3}b^{3}e^{-3b\zeta}}{a_{1}\left(c+de^{-b\zeta}\right)\left( 1-e^{-b\zeta}\right)^{5}}, \end{eqnarray} | (3.18) |
where a = \left(\frac{M^{2}}{2A_{3}R_{e}}+\frac{A_{2}}{2A_{3}}\right), \, \, \, \, \, \, b = \frac{2A_{3}\sqrt{\left(\frac{M^{2}}{2A_{3}R_{e}}+\frac{A_{2}}{2A_{3}}\right)^{2}+\frac{A_{5}}{A_{3}}}}{a_{1}}, \, \, \, \, \, \, \, c = \left(\frac{M^{2}}{2A_{3}R_{e}}+\frac{A_{2}}{2A_{3}}\right)+ \sqrt{\left(\frac{M^{2}}{2A_{3}R_{e}}+\frac{A_{2}}{2A_{3}}\right)^{2}+\frac{A_{5}}{A_{3}}}, \, \, \, \, \, \, d = \left(\frac{M^{2}}{2A_{3}R_{e}}+\frac{A_{2}}{2A_{3}}\right)- \sqrt{\left(\frac{M^{2}}{2A_{3}R_{e}}+\frac{A_{2}}{2A_{3}}\right)^{2}+\frac{A_{5}}{A_{3}}} \, \, and f = -\frac{2M^{2}}{R_{e}a_{1}}+\frac{2A_{2}}{a_{1}}.
After solving the Eq (3.18)
| \begin{eqnarray} Y_{11} = \frac{\left(1-e^{-b\zeta}\right)^{C_{3}}} {\left(c+de^{-b\zeta}\right)^{C_{1}}e^{C_{2}\zeta}} \left[\frac{c^{C_{1}-1}e^{(C_{2}-1)\zeta}}{C_{2}-3} +\frac{c^{C_{1}}[cC_{3}+C_{1}d-d+5]e^{(C_{2}-b-3)\zeta}}{C_{2}-b-3}+...\right], \end{eqnarray} | (3.19) |
where C_{1} = \frac{2a}{d-c}- \frac{2A_{3}A_{5}(c+d)}{a_{1}bcd} , C_{2} = \frac{A_{5}}{c}+\frac{3(2A_{3}a+a_{1}b)}{2a_{1}}+f and C_{3} = \frac{2a}{d-c}-\frac{12aA_{3}}{a_{1}^{2}b} .
Introducing the expressions (3.16) and (3.19) into (3.12), the following holds
| \begin{eqnarray} Y_{1}& = &\frac{1}{1-e^{-\frac{2A_{3}b}{a_{1}}\zeta}} \Big[\left(\frac{M^{2}}{2A_{3}R_{e}}+\frac{A_{2}}{2A_{3}}\right) +\sqrt{\left(\frac{M^{2}}{2A_{3}R_{e}}+\frac{A_{2}}{2A_{3}}\right)^{2}+\frac{A_{5}}{A_{3}}}\\ &+&\left(\left(\frac{M^{2}}{2A_{3}R_{e}}+\frac{A_{2}}{2A_{3}}\right) -\sqrt{\left(\frac{M^{2}}{2A_{3}R_{e}}+\frac{A_{2}}{2A_{3}}\right)^{2}+\frac{A_{5}}{A_{3}}}\right) e^{-\frac{2A_{3}\sqrt{\left(\frac{M^{2}}{2A_{3}R_{e}}+\frac{A_{2}}{2A_{3}}\right)^{2}+\frac{A_{5}}{A_{3}}}}{a_{1}}\zeta}\Big].\\ &+&\frac{\epsilon\left(1-e^{-b\zeta}\right)^{C_{3}}} {\left(c+de^{-b\zeta}\right)^{C_{1}}e^{C_{2}\zeta}} \left[\frac{c^{C_{1}-1}e^{(C_{2}-1)\zeta}}{C_{2}-3} +\frac{c^{C_{1}}[cC_{3}+C_{1}d-d+5]e^{(C_{2}-b-3)\zeta}}{C_{2}-b-3}+...\right]+O(\epsilon^{2}),\\ \end{eqnarray} | (3.20) |
which implies that
| \begin{eqnarray} &\,&v_{1}(y,t) = \frac{1}{1-e^{-\frac{2A_{3}b}{a_{1}}(y-a_{1}t)}} \Big[\left(\frac{M^{2}}{2A_{3}R_{e}}+\frac{A_{2}}{2A_{3}}\right) +\sqrt{\left(\frac{M^{2}}{2A_{3}R_{e}}+\frac{A_{2}}{2A_{3}}\right)^{2}+\frac{A_{5}}{A_{3}}}\\ &+&\left(\left(\frac{M^{2}}{2A_{3}R_{e}}+\frac{A_{2}}{2A_{3}}\right) -\sqrt{\left(\frac{M^{2}}{2A_{3}R_{e}}+\frac{A_{2}}{2A_{3}}\right)^{2}+\frac{A_{5}}{A_{3}}}\right) e^{-\frac{2A_{3}\sqrt{\left(\frac{M^{2}}{2A_{3}R_{e}}+\frac{A_{2}}{2A_{3}}\right)^{2}+\frac{A_{5}}{A_{3}}}}{a_{1}}(y-a_{1}t)}\Big]\\ &+&\frac{\epsilon\left(1-e^{-b(y-a_{1}t)}\right)^{C_{3}}} {\left(c+de^{-b(y-a_{1}t)}\right)^{C_{1}}e^{C_{2}(y-a_{1}t)}} \Big[\frac{c^{C_{1}-1}e^{(C_{2}-1)(y-a_{1}t)}}{C_{2}-3} +\frac{c^{C_{1}}[cC_{3}+C_{1}d-d+5]e^{(C_{2}-b-3)(y-a_{1}t)}}{C_{2}-b-3}\\ &+&...\Big] +O(\epsilon^{2}). \end{eqnarray} | (3.21) |
The Eq (3.21) shows the existence of an exponential decaying under the travelling wave profile asymptotic expansion. This results is not trivial in this case given the degeneracy in the diffusivity under the non-linear diffusion of porous medium kind.
Now, the intention is to show that the defined supporting manifold I_{\beta} preserves the exponential behavior shown in the proximity of the associated critical point. For this purpose, consider the expression (3.7), so that
| \begin{eqnarray} \frac{dZ}{dY} = \frac{C_{5}(Y-Y_{2})}{Z}. \end{eqnarray} | (3.22) |
After solving (3.22)
| \begin{eqnarray} Z = -\sqrt{C_{5}}(Y-Y_{2}). \end{eqnarray} | (3.23) |
Introducing the expression (3.7) into (3.23), the following holds
| \begin{eqnarray} Y' = -\beta\sqrt{C_{5}}(Y-Y_{2}), \end{eqnarray} | (3.24) |
such that a solution is
| \begin{eqnarray} Y = Y_{2}+e^{-\beta\sqrt{C_{5}}\zeta}, \end{eqnarray} | (3.25) |
which implies that
| \begin{eqnarray} v_{1}(y,t) = \frac{1}{2}\left(\frac{M^{2}}{R_{e}}+\frac{A_{2}}{A_{3}}\right) +\frac{1}{2}\sqrt{\left(\frac{M^{2}}{A_{3}R_{e}}+\frac{A_{2}}{A_{3}}\right)^{2} +\frac{4A_{5}}{A_{3}}}+e^{-\beta \sqrt {C_{5}}(y-a_{1}t)} \end{eqnarray} | (3.26) |
This last expression shows the conservation of the exponential profile in the proximity of the critical point kept by the asymptotic manifold I_{\beta}.
The existence and exact assessments of an orbit in the proximity of the critical point (Y_{2}, 0) follows the same procedure as discussed above for the point (Y_{1}, 0) . Indeed, a trajectory in the phase plane is given as
| \begin{eqnarray} \epsilon(1-m)\left(Y_{2}'\right)^{3} -a_{1}Y_{2}Y_{2}' -A_{5}Y_{2}+\left(\frac{M^{2}}{R_{e}}+A_{2}\right)Y_{2}^{2} +A_{3}Y_{2}^{3} = 0. \end{eqnarray} | (3.27) |
Solving the Eq (3.27) leads to obtain
| \begin{eqnarray} Y_{2}& = &\frac{1}{1-e^{-\frac{2A_{3}b}{a_{1}}\zeta}} \Big[\left(\frac{M^{2}}{2A_{3}R_{e}}+\frac{A_{2}}{2A_{3}}\right) +\sqrt{\left(\frac{M^{2}}{2A_{3}R_{e}}+\frac{A_{2}}{2A_{3}}\right)^{2}+\frac{A_{5}}{A_{3}}}\\ &+&\left(\left(\frac{M^{2}}{2A_{3}R_{e}}+\frac{A_{2}}{2A_{3}}\right) -\sqrt{\left(\frac{M^{2}}{2A_{3}R_{e}}+\frac{A_{2}}{2A_{3}}\right)^{2}+\frac{A_{5}}{A_{3}}}\right) e^{-\frac{2A_{3}\sqrt{\left(\frac{M^{2}}{2A_{3}R_{e}}+\frac{A_{2}}{2A_{3}}\right)^{2}+\frac{A_{5}}{A_{3}}}}{a_{1}}\zeta}\Big] \\ &+&\frac{\epsilon\left(1-e^{-b\zeta}\right)^{C_{3}}} {\left(c+de^{-b\zeta}\right)^{C_{1}}e^{C_{2}\zeta}} \left[\frac{c^{C_{1}-1}e^{(C_{2}-1)\zeta}}{C_{2}-3} +\frac{c^{C_{1}}[cC_{3}+C_{1}d-d+5]e^{(C_{2}-b-3)\zeta}}{C_{2}-b-3}+...\right]+O(\epsilon^{2}),\\ \end{eqnarray} | (3.28) |
where a = \left(\frac{M^{2}}{2A_{3}R_{e}}+\frac{A_{2}}{2A_{3}}\right), b = \frac{2A_{3}\sqrt{\left(\frac{M^{2}}{2A_{3}R_{e}}+\frac{A_{2}}{2A_{3}}\right)^{2}+\frac{A_{5}}{A_{3}}}}{a_{1}},
c = \left(\frac{M^{2}}{2A_{3}R_{e}}+\frac{A_{2}}{2A_{3}}\right)+ \sqrt{\left(\frac{M^{2}}{2A_{3}R_{e}}+\frac{A_{2}}{2A_{3}}\right)^{2}+\frac{A_{5}}{A_{3}}},
d = \left(\frac{M^{2}}{2A_{3}R_{e}}+\frac{A_{2}}{2A_{3}}\right)- \sqrt{\left(\frac{M^{2}}{2A_{3}R_{e}}+\frac{A_{2}}{2A_{3}}\right)^{2}+\frac{A_{5}}{A_{3}}} ,
f = -\frac{2M^{2}}{R_{e}a_{1}}+\frac{2A_{2}}{a_{1}}, C_{1} = \frac{2a}{d-c}- \frac{2A_{3}A_{5}(c+d)}{a_{1}bcd} , C_{2} = \frac{A_{5}}{c}+\frac{3(2A_{3}a+a_{1}b)}{2a_{1}}+f and C_{3} = \frac{2a}{d-c}-\frac{12aA_{3}}{a_{1}^{2}b} .
As a consequence, a solution is given by the following expression
| \begin{eqnarray} &&v_{1}(y,t) = \frac{1}{1-e^{-\frac{2A_{3}b}{a_{1}}(y-a_{1}t)}} \Big[\left(\frac{M^{2}}{2A_{3}R_{e}}+\frac{A_{2}}{2A_{3}}\right) +\sqrt{\left(\frac{M^{2}}{2A_{3}R_{e}}+\frac{A_{2}}{2A_{3}}\right)^{2}+\frac{A_{5}}{A_{3}}}\\ &+&\left(\left(\frac{M^{2}}{2A_{3}R_{e}}+\frac{A_{2}}{2A_{3}}\right) -\sqrt{\left(\frac{M^{2}}{2A_{3}R_{e}}+\frac{A_{2}}{2A_{3}}\right)^{2}+\frac{A_{5}}{A_{3}}}\right) e^{-\frac{2A_{3}\sqrt{\left(\frac{M^{2}}{2A_{3}R_{e}}+\frac{A_{2}}{2A_{3}}\right)^{2}+\frac{A_{5}}{A_{3}}}}{a_{1}}(y-a_{1}t)}\Big]\\ &+&\frac{\epsilon\left(1-e^{-b(y-a_{1}t)}\right)^{C_{3}}} {\left(c+de^{-b(y-a_{1}t)}\right)^{C_{1}}e^{C_{2}(y-a_{1}t)}} \Big[\frac{c^{C_{1}-1}e^{(C_{2}-1)(y-a_{1}t)}}{C_{2}-3} +\frac{c^{C_{1}}[cC_{3}+C_{1}d-d+5]e^{(C_{2}-b-3)(y-a_{1}t)}}{C_{2}-b-3}\\ &+&...\Big] +O(\epsilon^{2}). \end{eqnarray} | (3.29) |
Again, the intention now is to show that the defined supporting manifold I_{\gamma} preserves the exponential behavior in the proximity of the critical point (Y_2, 0) . For this purpose, consider the expression (3.8) so that
| \begin{eqnarray} \frac{dZ}{dY} = \frac{C_{5}(Y-Y_{1})}{Z}. \end{eqnarray} | (3.30) |
After solving (3.30), the following holds
| \begin{eqnarray} Z = -\sqrt{C_{5}}(Y-Y_{1}). \end{eqnarray} | (3.31) |
Introducing the expression (3.8) into (3.31)
| \begin{eqnarray} Y_{1}' = -\gamma\sqrt{C_{6}}(Y-Y_{1}), \end{eqnarray} | (3.32) |
such that a solution is given by
| \begin{eqnarray} Y = Y_{1}+e^{-\gamma\sqrt{C_{6}}\zeta}, \end{eqnarray} | (3.33) |
which implies that
| \begin{eqnarray} v_{1}(y,t) = -\frac{1}{2}\left(\frac{M^{2}}{R_{e}}+\frac{A_{2}}{A_{3}}\right) +\frac{1}{2}\sqrt{\left(\frac{M^{2}}{A_{3}R_{e}}+\frac{A_{2}}{A_{3}}\right)^{2} +\frac{4A_{5}}{A_{3}}}+e^{-\gamma \sqrt {C_{6}}(y-a_{1}t)}. \end{eqnarray} | (3.34) |
This last expression permits to show the conservation of the exponential profile in the proximity of the critical point under study by the asymptotic manifold I_{\gamma}.
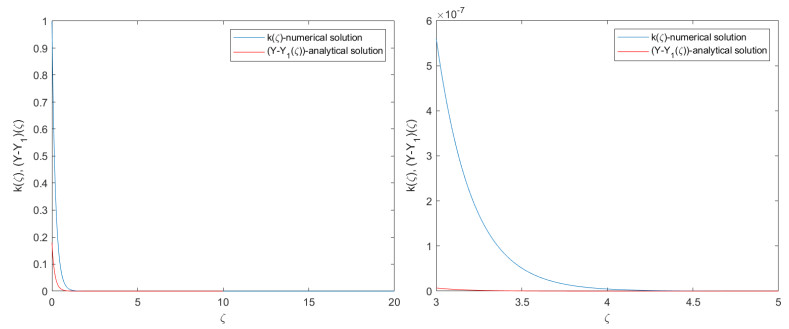
According to expressions (3.25) and (3.33), the analytical assessments have provided an exponential tail in the proximity of the system critical points. To validate the assessments, it is the intention to compare such analytical exponential tail with a numerical simulation of the Eq (2.1). Note that the numerical exploration is not intended to introduce a wide parametrical analysis with each of the involved fluid constants, on the contrary the objective is to provide evidences on the accuracy of the analytical process followed. In addition, for the validation exercise the expression (3.33) has been considered, under the form Y-Y_{1} = e^{-\gamma\sqrt{C_{6}}\zeta}. The numerical assessment is executed with the bvp4c in Matlab (refer to [34] for further details on this software tool).
The R_e number has been considered as a free parameter to establish the accuracy of solutions. In addition, the following particular values in the other fluid constant have been considered for the sake of simplicity, but with no impact in the conclusions
| \begin{equation} 0 < A_5 \ll 1, U_0 = 1, A_1 = 1, 0 < \epsilon \ll 1, M = 1, \nu = 1, K_1 = 1, K_2 = 1. \end{equation} | (4.1) |
Note that L = 1000 as the domain of integration has been considered as (-500,500) . This domain has been selected after some numerical trials and provides an efficient compromise between low impact of the collocation methods at the borders and a moderate computational time. In addition, the global absolute error allowed is of 10^{-4} .
Note that the R_e in porous media is typically low, hence the considered values are R_e = 1; 100 .
As it can be noticed from Figures 1 and 2, the analytical asymptotic solution (3.33) based on the geometric perturbation theory and the numerical solution to (2.1) fit an exponential behaviour with an order deficiency in the decreasing rate. Nonetheless, solutions are very close for increasing values of \zeta , this is in the asymptotic approach where the analytical solution has been obtained. This is not a trivial conclusion for the degenerate diffusion given in (2.1) and represents a finding to remark.

The set of assessments and discussions presented in this paper have provided evidences on the regularity of solutions to a kind of fluid flow with degenerate diffusivity and formulated with Darcy-Forchheimer porosity principles and Eyring-Powell viscosity terms. Afterwards, asymptotic travelling waves solutions have been explored based on a perturbation technique. Particularly, the Geometric Perturbation Theory has shown that an exponential decreasing rate holds even when the equation discussed presents a degenerate diffusivity. Furthermore, two linearized manifolds in the proximity of the critical points have been shown to hold and an exponential behaviour has been obtained accordingly. Eventually, a numerical exercise has been introduced to account for the validation of the analytical assessments done.
The authors declare that there are not any conflict of interests.
| [1] | H. Darcy, Les fontaines publiques de la ville de dijon, Paris: Dalmont, 1856. |
| [2] | P. Forchheimer, Wasserbewegung durch Boden, Zeitschrift des Vereines Deutscher Ingeneieure, 45 (1901), 1782–1788. |
| [3] | C. Jaeger, Engineering fluid mechanics, Edinburgh: Blackie and Son, 1956. |
| [4] | M. Muskat, The flow of homogeneous fluids through porous media, New York: McGrawHill Book Company, 1937. |
| [5] |
J. C. Ward, Turbulent flow in porous media, Journal of the Hydraulics Division, 5 (1964), 1–12. https://doi.org/10.1061/JYCEAJ.0001096 doi: 10.1061/JYCEAJ.0001096

|
| [6] |
D. Pal, H. Mondal, Hydromagnetic convective diffusion of species in Darcy-Forchheimer porous medium with nonuniform heat source/sink and variable viscosity, Int. Commun. Heat Mass, 39 (2012), 913–917. https://doi.org/10.1016/j.icheatmasstransfer.2012.05.012 doi: 10.1016/j.icheatmasstransfer.2012.05.012

|
| [7] |
R. U. Haq, F. A. Soomro, T. Mekkaouic, Q. M. Al-Mdallal, MHD natural convection flow enclosure in a corrugated cavity filled with a porous medium, Int. J. Heat Mass Tran., 121 (2018), 1168–1178. https://doi.org/10.1016/j.ijheatmasstransfer.2018.01.063 doi: 10.1016/j.ijheatmasstransfer.2018.01.063

|
| [8] |
T. Hayat, T. Muhammad, S. Al-Mezal, S. J. Liao, Darcy-Forchheimer flow with variable thermal conductivity and Cattaneo-Christov heat flux, International Journal of Numerical Methods for Heat and Fluid Flow, 26 (2016), 2355–2369. https://doi.org/10.1108/HFF-08-2015-0333 doi: 10.1108/HFF-08-2015-0333

|
| [9] |
N. A. Ganesh, A. K. A. Hakeem, B. Ganga, Darcy-Forchheimer flow of hydromagnetic nanofluid over a stretching/shrinking sheet in a thermally stratifed porous medium with second order slip, viscous and ohmic dissipations effects, Ain Shams Eng. J., 9 (2018), 939–951. https://doi.org/10.1016/j.asej.2016.04.019 doi: 10.1016/j.asej.2016.04.019

|
| [10] |
N. S. Akbar, A. Ebaid, Z. Khan, Numerical analysis of magnetic feld effects on Eyring-Powell fluid flow towards a stretching sheet, J. Magn. Magn. Mater., 382 (2015), 355–358. https://doi.org/10.1016/j.jmmm.2015.01.088 doi: 10.1016/j.jmmm.2015.01.088

|
| [11] |
S. Hina, MHD peristaltic transport of Eyring-Powell fluid with heat/mass transfer, wall properties and slip conditions, J. Magn. Magn. Mater., 404 (2016), 148–158. https://doi.org/10.1016/j.jmmm.2015.11.059 doi: 10.1016/j.jmmm.2015.11.059

|
| [12] |
M. Bhatti, T. Abbas, M. Rashidi, M. Ali, Z. Yang, Entropy generation on MHD Eyring-Powell nanofluid through a permeable stretching surface, Entropy, 18 (2016), 224. https://doi.org/10.3390/e18060224 doi: 10.3390/e18060224

|
| [13] |
A. Ara, N. A. Khan, H. Khan, F. Sultan, Radiation effect on boundary layer flow of an Eyring–Powell fluid over an exponentially shrinking sheet, Ain Shams Eng. J., 5 (2014), 1337–1342. https://doi.org/10.1016/j.asej.2014.06.002 doi: 10.1016/j.asej.2014.06.002

|
| [14] |
T. Hayat, Z. Iqbal, M. Qasim, S. Obaidat, Steady flow of an Eyring Powell fluid over a moving surface with convective boundary conditions, Int. J. Heat Mass Tran., 55 (2012), 1817–1822. https://doi.org/10.1016/j.ijheatmasstransfer.2011.10.046 doi: 10.1016/j.ijheatmasstransfer.2011.10.046

|
| [15] |
T. Hayat, M. Awais, S. Asghar, Radiative effects in a threedimensional flow of MHD Eyring-Powell fluid, Journal of the Egyptian Mathematical Society, 21 (2013), 379–384. https://doi.org/10.1016/j.joems.2013.02.009 doi: 10.1016/j.joems.2013.02.009

|
| [16] |
M. Jalil, S. Asghar, S. M. Imran, Self similar solutions for the flow and heat transfer of Powell-Eyring fluid over a moving surface in parallel free stream, Int. J. Heat Mass Tran., 65 (2013), 73–79. https://doi.org/10.1016/j.ijheatmasstransfer.2013.05.049 doi: 10.1016/j.ijheatmasstransfer.2013.05.049

|
| [17] |
J. A. Khan, M. Mustafa, T. Hayat, M. A. Farooq, A. Alsaedi, S. J. Liao, On model for three-dimensional flow of nanofluid: an application to solar energy, J. Mol. Liq., 194 (2014), 41–47. https://doi.org/10.1016/j.molliq.2013.12.045 doi: 10.1016/j.molliq.2013.12.045

|
| [18] |
A. Riaz, R. Ellahi, S. M. Sait, Role of hybrid nanoparticles in thermal performance of peristaltic flow of Eyring–Powell fluid model, J. Therm. Anal. Calorim., 143 (2021), 1021–1035. https://doi.org/10.1007/s10973-020-09872-9 doi: 10.1007/s10973-020-09872-9

|
| [19] |
A. S. Oke, Theoretical analysis of modified Eyring Powell fluid flow, J. Taiwan Inst. Chem. Eng., 132 (2022), 104152. https://doi.org/10.1016/j.jtice.2021.11.019 doi: 10.1016/j.jtice.2021.11.019

|
| [20] |
A. S. Oke, W. N. Mutuku, Significance of viscous dissipation on MHD Eyring–Powell flow past a convectively heated stretching sheet, Pramana-J. Phys., 95 (2021), 199. https://doi.org/10.1007/s12043-021-02237-3 doi: 10.1007/s12043-021-02237-3

|
| [21] |
A. S. Oke, Coriolis effects on MHD flow of MEP fluid over a non-uniform surface in the presence of thermal radiation, Int. Commun. Heat Mass, 129 (2021), 105695. https://doi.org/10.1016/j.icheatmasstransfer.2021.105695 doi: 10.1016/j.icheatmasstransfer.2021.105695

|
| [22] | A. S. Oke, W. N. Mutuku, Significance of Coriolis force on Eyring-Powell flow over a rotating non-uniform surface, Appl. Appl. Math., 16 (2021), 36. |
| [23] |
J. K. Kigio, W. N. Mutuku, A. S. Oke, Analysis of volume fraction and convective heat transfer on MHD Casson nanofluid over a vertical plate, Fluid Mechanics, 7 (2021), 1–8. https://doi.org/1.10.11648/j.fm.20210701.11 doi: 10.11648/j.fm.20210701.11

|
| [24] | A. S. Oke, Heat and mass transfer in 3D MHD flow of EG-based ternary hybrid nanofluid over a rotating surface, Arab. J. Sci. Eng., 2022, in press. https://doi.org/10.1007/s13369-022-06838-x |
| [25] | J. D. Murray, Mathematical biology, Berlin, Heidelberg: Springer, 1993. https://doi.org/10.1007/978-3-662-08542-4 |
| [26] | J. Smoller, Shock waves and reactiondiffusion equations, New York: Springer, 1994. https://doi.org/10.1007/978-1-4612-0873-0 |
| [27] | A. Champneys, G. Hunt, J. Thompson. Localization and solitary waves in solid mechanics, World Scientifc, 1999. https://doi.org/10.1142/4137 |
| [28] | A. De Pablo, Estudio de una ecuación de reacción-difusión, Doctoral Thesis, Universidad Autónoma de Madrid, 1989. |
| [29] |
A. de Pablo, J. L. Vázquez, Travelling waves and finite propagation in a reaction-diffusion equation, J. Differ. Equations, 93 (1991), 19–61. https://doi.org/10.1016/0022-0396(91)90021-Z doi: 10.1016/0022-0396(91)90021-Z

|
| [30] |
N. T. M. Eldabe, A. A. Hassan, M. A. Mohamed, Effect of couple stresses on the MHD of a non-Newtonian unsteady flow between two parallel porous plates, Zeitschrift für Naturforschung A, 58 (2003), 204–210. https://doi.org/10.1515/zna-2003-0405 doi: 10.1515/zna-2003-0405

|
| [31] |
N. Fenichel, Persistence and smoothness of invariant manifolds for flows, Indiana Univ. Math. J., 21 (1971), 193–226. https://doi.org/10.1512/IUMJ.1972.21.21017 doi: 10.1512/IUMJ.1972.21.21017

|
| [32] |
M. E. Akveld, J. Hulshof, Travelling wave solutions of a fourth-order semilinear diffusion equation, Appl. Math. Lett., 11 (1998), 115–120. https://doi.org/10.1016/S0893-9659(98)00042-1 doi: 10.1016/S0893-9659(98)00042-1

|
| [33] | C. K. R. T. Jones, Geometric singular perturbation theory, In: Dynamical systems, Berlin, Heidelberg: Springer, 1995, 44–118. https://doi.org/10.1007/BFb0095239 |
| [34] | H. Enright, P. H. Muir, A Runge-Kutta type boundary value ODE solver with defect control, Teh. Rep. 267/93, University of Toronto, Dept. of Computer Sciences, Toronto, Canada, 1993. |
| 1. | Pawan Shrestha, Durga Jang KC, Ramjee Sharma, Saranya Shekar, On the Construction of Traveling Water Waves with Constant Vorticity and Infinite Boundary, 2023, 2023, 1687-0425, 1, 10.1155/2023/6317674 | |
| 2. | Yong Zhang, Huan-He Dong, Yong Fang, Rational and Semi-Rational Solutions to the (2 + 1)-Dimensional Maccari System, 2022, 11, 2075-1680, 472, 10.3390/axioms11090472 | |
| 3. | M. Venkata Subba Rao, Kotha Gangadhar, Ali J. Chamkha, MHD Eyring-Powell fluid flow over a stratified stretching sheet immersed in a porous medium through mixed convection and viscous dissipation, 2023, 0228-6203, 1, 10.1080/02286203.2023.2296678 | |
| 4. | Abey Sherif Kelil, Appanah Rao Appadu, On the Numerical Solution of 1D and 2D KdV Equations Using Variational Homotopy Perturbation and Finite Difference Methods, 2022, 10, 2227-7390, 4443, 10.3390/math10234443 | |
| 5. | Manoj Kumar, Santosh Kumar Singh, New Exact Solutions of a Second Grade MHD Flow Through Porous Media Using Travelling Wave Method, 2024, 1016-2526, 471, 10.52280/pujm.2023.55(11-12))05 |