Solitary wave solutions to Gardner equation using improved tan$\left(\frac{\Omega(\Upsilon)}{2}\right)$-expansion method

1.   Introduction

Differential equations are very useful in many fields of science, including applied sciences and mathematical physics. Partial differential equations (PDEs) are widely employed in engineering to understand the behavior of physical systems through mathematical models. Many efficient approaches for identifying the solutions of nonlinear PDEs have been developed in recent years, such as: Hirotäs bilinear method ^[1], exp$(-\Phi(\xi))$-expansion method ^[2,3], generalized exponential rational function method ^[4], auxiliary equation method ^[5], first integral method ^[6], homotopy analysis method ^[7,8], tanh-method ^[9], transformed rational function method ^[10], residual power series method ^[11,12], extended direct algebraic method ^[13] and many other powerful mathematical techniques. Nonlinear PDEs are studied extensively because they help to understand the propagation of waves in many areas of mathematical physics, fluid mechanics and electromagnetic theory ^[14,15]. In addition, soliton solutions also have an effective contribution in fields of engineering and nonlinear optics ^{[2,16,18,19,20,21]}.

The traveling wave solutions of nonlinear PDEs equation are essential to explore and interpret various real life physical phenomena. The significance of the traveling wave solutions of nonlinear evolution equations has motivated many researches to investigate exact traveling wave solutions using effective and reliable mathematical techniques. The traveling wave solutions include solitary and other kinds of wave solutions. In particular, solitons are of great significance due to their useful applications in various areas of science and engineering ^[22,23].

This study aims to investigate soliton and other traveling wave solutions of Gardner equation, which is an integrable nonlinear partial differential. The Gardner equation was originally proposed by Clifford Gardner in $1968$ ^[24]. This equation is frequently referred to as combined Korteweg-de Vries-modified Korteweg-de Vries (KdV-mKdV) equation since it can be generalized to Korteweg-de Vries (KdV) equation. Gardner's equation has a wide range of applications in research, including quantum field theory and hydrodynamics ^[25,26,27].

In this work, solitary wave solutions of Gardner equation (GE) are retrieved by utilizing the improved tan$\left(\frac{\Omega(\Upsilon)}{2}\right)$-expansion method. This technique is a recently developed direct technique which provides a variety of traveling wave solutions for a wide class of nonlinear evolution equations ^{[28,29,30,31]}.

A soliton is an autonomous wave that diffuses while maintaining its shape and velocity. The nonlinear integrable KdV equation can be written, as

where $r(x, t)$ in Eq (1.1) is the appropriate field variable and $l$, $m$ are real constants, also $x$ is representing the spatial variable and $t$ is indicating the temporal variable. The solitary waves are generated due to nonlinear term $rr_{x}$ and the linear dispersion $r_{xxx}$. The Gardener equation with constant coefficients ^[32,33] is considered in the form

where $\delta$ is a nonzero constant. Eq $(1.2)$ is also called the combined KdV-mKdV equation. A higher order nonlinear term was added to the Eq (1.1) to generate the Gardner equation. Like KdV equation, Equation(1.2) is also an integrable equation.

2.   Improved tan$\left(\frac{\Omega(\Upsilon)}{2}\right)$-expansion method

Step 2.1. The nonlinear partial differential equation (NLPDE) for $r(x, t)$ is considered in the form

By the aid of transformation $\Upsilon = \kappa(x-\varpi t)$, Eq (2.1) can be converted into an ordinary differential equation, as

where $\varpi$ is to be evaluated later and $\Upsilon$ is a wave variable.

Step 2.2. The solitary wave solution of Eq (2.2) is supposed, as

where $G_{j} (0 \leq j \leq p)$ and $H_{j} (1 \leq j \leq p)$ are constants to be determined later. Also, $G_{p}\neq0, H_{p}\neq0$ and $\Omega = \Omega(\Upsilon)$ satisfy the ordinary differential equation (ODE),

Following are the special wave solutions for Eq (2.4).

Family 2.1. For $q_{0}^{2}+q_{1}^{2}-q_{2}^{2} < 0$ and $q_{1}-q_{2}\neq0$,

Family 2.2. For $q_{0}^{2}+q_{1}^{2}-q_{2}^{2} > 0$ and $q_{1}-q_{2}\neq0$,

Family 2.3. For $q_{0}^{2}+q_{1}^{2}-q_{2}^{2} > 0$, $q_{1}\neq0$ and $q_{2} = 0$,

Family 2.4. For $q_{0}^{2}+q_{1}^{2}-q_{2}^{2} < 0$, $q_{2}\neq0$ and $q_{1} = 0$,

Family 2.5. For $q_{0}^{2}+q_{1}^{2}-q_{2}^{2} > 0$, $q_{1}-q_{2}\neq0$ and $q_{0} = 0$,

Family 2.6. For $q_{0} = 0$ and $q_{2} = 0$,

Family 2.7. For $q_{1} = 0$ and $q_{2} = 0$,

Family 2.8. For $q_{0}^{2}+q_{1}^{2} = q_{2}^{2}$,

Family 2.9. For $q_{0} = q_{1} = q_{2} = il_{0}$,

Family 2.10. For $q_{0} = q_{2} = il_{0}$ and $q_{1} = -il_{0}$,

Family 2.11. For $q_{2} = q_{0}$,

Family 2.12. For $q_{0} = q_{2}$,

Family 2.13. For $q_{2} = -q_{0}$,

Family 2.14. For $q_{1} = -q_{2}$,

Family 2.15. For $q_{1} = 0$, $q_{0} = q_{2}$,

Family 2.16. For $q_{0} = 0$ and $q_{1} = q_{2}$,

Family 2.17. For $q_{0} = 0$, $q_{1} = -q_{2}$,

Family 2.18. For $q_{0} = 0$ and $q_{1} = 0$,

where $q_{0}, \; q_{1}$, $q_{2}$ and $G_{0}, \; G_{j}, \; H_{j}\; (j = 1, 2, ....p)$ are to be evaluated. Homogeneous balance principle is used to find the value of $p$ by considering highest order derivatives and highest non-linear terms occurring in Eq (2.2). If $p$ is not an integer, then suitable transformation is implemented.

Step 2.3. Once the value of $p$ is obtained, Eq (2.3) is substituted into Eq (2.2). By gathering the coefficients of $\tan\left(\frac{\Omega(\Upsilon)}{2}\right)^{j}$, $\cot\left(\frac{\Omega(\Upsilon)}{2}\right)^{j}$ $(j = 0, 1, 2, ...)$ and setting each coefficient equal to zero, a set of algebraic equations for $G_{0}, \; G_{j}, \; H_{j}\; (j = 1, 2, .\:.\:.p)$, $q_{0}, \; q_{1}, \; q_{2}$ and $q$ can be obtained.

Step 2.4. The set of over determined equations are solved and the values of $G_{0}, \; G_{1}, \; H_{1}, ..., \; G_{p}, \; H_{p}, \; \varpi$ and $q$ are substituted in Eq (2.3).

3.   Exact soliton solutions of Gardner equation

Consider the integrable nonlinear Gardner equation given by Eq (1.2). Substituting the wave transformation,

into Eq (1.2) yields an ordinary differential equation, as

Implementing the homogeneous balance principle the value of positive integer is obtained, as $p = 1$. The trial solution becomes

Substituting Eq (3.3) and Eq (2.4) into Eq (3.2), the following set of algebraic equations can be derived for $q_{0}, q_{1}, q_{2}, \kappa, \varpi,$ $G_{0}, G_{1}$ and $H_{1}$ by collecting the terms with the same order of $\tan\left(\frac{\Omega(\Upsilon)}{2}\right)$ and setting every coefficient of all the polynomials equal to zero.

Following are the solutions obtained by solving the system of algebraic equation.

Set 3.1. $\kappa = \kappa, \; \varpi = \frac{1}{\delta^{2}}, \; G_{0} = -\frac{1}{2\delta^{2}}, \; G_{{1}} = \pm\frac{1}{4}{\frac {2\, q_{{1}}\kappa\, \delta -\sqrt {4\, {\delta }^{2}{\kappa}^{2}{q_{{1}}}^{2}-1}}{{\delta }^{2}}}, \; H_{{1}} = \pm\frac{1}{4}{\frac {2\, q_{{1}}\kappa\, \delta +\sqrt {4\, {\delta}^{2}{\kappa}^{2}{q_{{1}}}^{2}-1}}{{\delta }^{2}}}, \; q_{{0}} = 0, \\q_{{1}} = q_{{1}}, \; q_{{2}} = \frac{1}{2}{\frac {\sqrt {4\, {\delta }^{2}{\kappa}^{2}{q_{{1}}}^{2}-1}}{\delta \, \kappa}},$

where $q_{0}$, $q_{1}$, $q_{2}$ are arbitrary constants.

Using Eq (3.4) and Families 2.2, 2.5 and 2.18 respectively, yields the following solutions:

Set 3.2. $\kappa = \kappa, \; \varpi = \frac{1}{\delta^{2}}, \; G_{0} = \frac{1}{2}{\frac {-1+\sqrt {1+ \left(-{q_{{1}}}^{2}+{q_{{2}}}^{2} \right) {\delta }^{2}{\kappa}^{2}}}{{\delta }^{2}}}, \; G_{{1}} = 0, \; H_{{1}} = \mp\frac{1}{2}{\frac {\kappa\, \left(q_{{1}}+q_{{2}} \right) }{\delta }},$ $\; q_{{0}} = \mp{\frac {\sqrt {1+ \left(-{q_{{1}}}^{2}+{q_{{2}}}^{2} \right) {\delta }^{2}{\kappa}^{2}}}{\kappa\, \delta }}, \; q_{{1}} = q_{{1}}, \; q_{{2}} = q_{2},$

where $q_{0}$, $q_{1}$, $q_{2}$ are arbitrary constants.

The following solutions are determined by using Eq (3.8) and Families 2.2, 2.3, 2.6, 2.7 and 2.11–2.14, respectively.

Set 3.3. $\kappa = \kappa, \; \varpi = \frac{1}{\delta^{2}}, \; G_{0} = \frac{1}{2}{\frac {-1+\sqrt {1+ \left(-{q_{{1}}}^{2}+{q_{{2}}}^{2} \right) {\delta }^{2}{\kappa}^{2}}}{{\delta }^{2}}}, \; G_{{1}} = \mp \frac{1}{2}{\frac {\kappa\, \left(q_{{1}}-q_{{2}} \right) }{\delta }}, \; H_{{1}} = 0, \;$ $q_{{0}} = \mp{\frac {\sqrt {1+ \left(-{q_{{1}}}^{2}+{q_{{2}}}^{2} \right) {\delta }^{2}{\kappa}^{2}}}{\kappa\, \delta }}, \; q_{{1}} = q_{{1}}, \; q_{{2}} = q_{2},$

where $q_{0}$, $q_{1}$, $q_{2}$ are arbitrary constants.

Using Eq (3.17) and Families 2.2, 2.3, 2.6, 2.7, 2.11–2.14, respectively, yields the following solution:

Set 3.4. $\kappa = \kappa, \; \varpi = {\frac {1+ \left({q_{{1}}}^{2}-{q_{{2}}}^{2} \right) {\kappa}^{2}{\delta }^{2}}{{\delta }^{2}}}, \; G_{0} = -\frac{1}{\delta^{2}}, \; G_{{1}} = \mp\frac{1}{2}{\frac {\kappa\, \left(q_{{1}}-q_{{2}} \right) }{\delta }}, \; H_{{1}} = \mp\frac{1}{2}{\frac {\kappa\, \left(q_{{1}}+q_{{2}} \right) }{\delta }}, \; q_{{0}} = \mp\frac{1}{\kappa \delta}, \\q_{{1}} = q_{{1}}, \; q_{{2}} = q_{2},$

where $q_{0}$, $q_{1}$, $q_{2}$ are arbitrary constants.

Using Eq (3.26) and Families 2.1–2.4, 2.8–2.10, 2.13–2.15, respectively, gives the following solutions:

Set 3.5. $\kappa = \kappa, \; \varpi = -2\, {\frac { \left(1+ \left({q_{{1}}}^{2}-{q_{{2}}}^{2} \right) {\kappa}^{2}{\delta }^{2} \right) \left(-\frac{1}{2}+ \left({q_{{1}}} ^{2}-{q_{{2}}}^{2} \right) {\kappa}^{2}{\delta }^{2} \right) }{{\delta }^{2} }}, \; G_{0} = {q_{{1}}}^{2}{\kappa}^{2}-{q_{{2}}}^{2}{\kappa}^{2}, \; G_{{1}} = \mp\frac{1}{2}{\frac {\kappa\, \left(q_{{1}}-q_{{2}} \right) }{\delta }}, \\ H_{{1}} = \mp\frac{1}{2}{\frac {\kappa\, \left(q_{{1}}+q_{{2}} \right) }{\delta }}, \; q_{{0}} = \mp{\frac {1+ \left(2\, {q_{{1}}}^{2}-2\, {q_{{2}}}^{2} \right) { \delta }^{2}{\kappa}^{2}}{\kappa\, \delta }}, \; q_{{1}} = q_{{1}}, \; q_{{2}} = q_{2},$

where $q_{0}$, $q_{1}$, $q_{2}$ are arbitrary constants.

Using Eq (3.37) and Families 2.1–2.3, 2.9, 2.10, 2.13 and 2.14, respectively, yields the following solutions:

where $\Upsilon = \kappa(x-\varpi t).$

4.   Graphical illustration

Some of the obtained soliton solutions are graphically represented in this section. Kink solitons, dark-bright solitons, bright solitons, singular solitons and periodic wave solutions are retrieved.

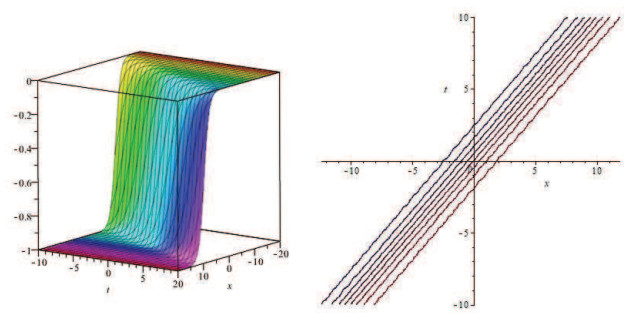
The $3$D-graph and contour plot for the solution $R_{4}(\Upsilon)$ are shown in Figure 1. The solution $R_{4}(\Upsilon)$ is derived from Family $2.2$ of solution Set $2.2$ as defined by Eq $(3.9)$. The obtained graphs show a kink soliton solution. Kink soliton is a type of solitary wave that ascend or descend from one asymptotic state to another. The contour graph is also included along with surface graph to illustrate the wave structure corresponding to the obtained solution.

The graphical illustration of $R_{23}(\Upsilon)$ is presented in Figure 2. The solution $R_{23}(\Upsilon)$ is given by Equation $(3.30)$ using the values of Set $2.4$ for Family $2.4$. The graphs in Figure 2 show a bright soliton. The surface graph shows a localized intensity peak above the continuous wave background which means that there is a temporary increase in the wave amplitude.

Figure 3 shows the $3$D plot and the corresponding contour plot of $R_{20}(\Upsilon)$ given by Eq $(3.27)$. The graph of $R_{12}(\Upsilon)$ given by Eq $(3.18)$ is illustrated in Figure 4. Figure 5 provides the graphical illustration of $R_{30}(\Upsilon)$ given by Eq $(3.38)$. Figure 6 shows the graph of a dark-bright soliton which is graphical illustration of the solution $R_{3}(\Upsilon)$ expressed by Eq $(3.7)$. Similarly, Figures 7–9 presents the graphical illustrations for the solutions presented by Eq $(3.35)$, Eq $(3.6)$ and Eq $(3.32)$, respectively.

It can be easily observed that improved tan$\left(\frac{\Omega(\Upsilon)}{2}\right)$ technique is a spectacular technique as compared to many other direct techniques as it gave abundant soliton solutions. Using this technique, kink, singular, bright and dark-bright soliton solutions have been retrieved in this paper. This method is clearly more powerful than many other methods, such as: the tanh-method ^[34], the $\frac{G'}{G}$ expansion method ^[35] and the generalized exponential rational function method, the Jacobi elliptic solution method ^[36], because the improved tan$\left(\frac{\Omega(\Upsilon)}{2}\right)$ method retrieved many more new solutions than the previously mentioned techniques.

5.   Conclusions

In this study, the soliton and other solitary wave solutions of the constant-coefficient Gardner equation are investigated using tan$\left(\frac{\Omega(\Upsilon)}{2}\right)$-expansion method. A variety of precise closed form traveling wave solutions have been constructed including bright solitons, dark-bright solitons, kink solitons and periodic wave solutions. Some of the obtained solutions are illustrated using graphical simulations for suitable choice of parameters. The wave profile corresponding to the obtained solutions is depicted through 3D-surface graphs and corresponding 2D-contour plots. Comparison of the obtained results with those available in the literature depict the efficacy and productivity of the improved tan$\left(\frac{\Omega(\Upsilon)}{2}\right)$ technique. Mathematical computations and simulations were obtained using Maple software. The reported results may be helpful in further explorations of the nonlinear physical problems governed by the Gardner equation in fluid dynamics, plasma physics and other fields. The improved tan$\left(\frac{\Omega(\Upsilon)}{2}\right)$ technique will be useful for the analytic study of a large class of nonlinear PDEs that are widely used in engineering, physics and other sciences.

Conflict of interest

The authors declare no conflicts of interest.