Modulational instability, multiple Exp-function method, SIVP, solitary and cross-kink solutions for the generalized KP equation

Junjie Li; Gurpreet Singh; Onur Alp İlhan; Jalil Manafian; Yusif S. Gasimov; Junjie Li; Gurpreet Singh; Onur Alp İlhan; Jalil Manafian; Yusif S. Gasimov

doi:10.3934/math.2021441

AIMS Mathematics

2021, Volume 6, Issue 7: 7555-7584. doi: 10.3934/math.2021441

Previous Article Next Article

Research article

Modulational instability, multiple Exp-function method, SIVP, solitary and cross-kink solutions for the generalized KP equation

1.
School of Applied Mathematics, Xiamen University of Technology, Xiamen 361024, China
2.
Department of Mathematics, Sant Baba Bhag Singh University, Jalandhar(INDIA)-144030
3.
Department of Mathematics, Faculty of Education, Erciyes University, 38039-Melikgazi-Kayseri, Turkey
4.
Department of Applied Mathematics, Faculty of Mathematical Sciences, University of Tabriz, Tabriz, Iran
5.
Natural Sciences Faculty, Lankaran State University, 50, H. Aslanov str., Lankaran, Azerbaijan
6.
Azerbaijan University, J. Hajibeyli, 71, AZ1007, Baku, Azerbaijan
7.
Baku State University, Institute for Physical Problems, Z.Khalilov, 23, AZ1148, Baku, Azerbaijan
8.
Baku State University, Institute of Mathematics and Mechanics, ANAS, B.Vahabzade, 9, AZ1148, Baku, Azerbaijan

Received: 03 February 2021 Accepted: 25 April 2021 Published: 08 May 2021
MSC : 35A20, 35A24, 35A25, 35B10, 70K50

The multiple Exp-function method is employed for seeking the multiple soliton solutions to the generalized (3+1)-dimensional Kadomtsev-Petviashvili (gKP) equation, where contains one-wave, two-wave, and triple-wave solutions. The periodic wave including (exponential, $\cosh$ hyperbolic, and $\cos$ periodic), cross-kink containing (exponential, $\sinh$ hyperbolic, and $\sin$ periodic), and solitary containing (exponential, $\tanh$ hyperbolic, and $\tan$ periodic) wave solutions are obtained. In continuing, the modulation instability is engaged to discuss the stability of obtained solutions. Also, the semi-inverse variational principle is applied for the gKP equation with four major cases. The physical phenomena of these received multiple soliton solutions are analyzed and demonstrated in figures by choosing the specific parameters. By means of symbolic computation these analytical solutions and corresponding rogue waves are obtained with the help of Maple software. Via various three-dimensional, curve, and density charts, dynamical characteristics of these waves are exhibited.

Keywords:

multiple Exp-function method,
generalized Kadomtsev-Petviashvili equation,
modulation instability,
semi-inverse variational principle

Citation: Junjie Li, Gurpreet Singh, Onur Alp İlhan, Jalil Manafian, Yusif S. Gasimov. Modulational instability, multiple Exp-function method, SIVP, solitary and cross-kink solutions for the generalized KP equation[J]. AIMS Mathematics, 2021, 6(7): 7555-7584. doi: 10.3934/math.2021441

Related Papers:

[1]	Boyu Wang . A splitting lattice Boltzmann scheme for (2+1)-dimensional soliton solutions of the Kadomtsev-Petviashvili equation. AIMS Mathematics, 2023, 8(11): 28071-28089. doi: 10.3934/math.20231436
[2]	Jalil Manafian, Onur Alp Ilhan, Sizar Abid Mohammed . Forming localized waves of the nonlinearity of the DNA dynamics arising in oscillator-chain of Peyrard-Bishop model. AIMS Mathematics, 2020, 5(3): 2461-2483. doi: 10.3934/math.2020163
[3]	Cheng Chen . Hyperbolic function solutions of time-fractional Kadomtsev-Petviashvili equation with variable-coefficients. AIMS Mathematics, 2022, 7(6): 10378-10386. doi: 10.3934/math.2022578
[4]	Abeer S. Khalifa, Hamdy M. Ahmed, Niveen M. Badra, Jalil Manafian, Khaled H. Mahmoud, Kottakkaran Sooppy Nisar, Wafaa B. Rabie . Derivation of some solitary wave solutions for the (3+1)- dimensional pKP-BKP equation via the IME tanh function method. AIMS Mathematics, 2024, 9(10): 27704-27720. doi: 10.3934/math.20241345
[5]	Wafaa B. Rabie, Hamdy M. Ahmed, Taher A. Nofal, Soliman Alkhatib . Wave solutions for the (3+1)-dimensional fractional Boussinesq-KP-type equation using the modified extended direct algebraic method. AIMS Mathematics, 2024, 9(11): 31882-31897. doi: 10.3934/math.20241532
[6]	Gulnur Yel, Haci Mehmet Baskonus, Wei Gao . New dark-bright soliton in the shallow water wave model. AIMS Mathematics, 2020, 5(4): 4027-4044. doi: 10.3934/math.2020259
[7]	Jamilu Sabi'u, Sekson Sirisubtawee, Surattana Sungnul, Mustafa Inc . Wave dynamics for the new generalized (3+1)-D Painlevé-type nonlinear evolution equation using efficient techniques. AIMS Mathematics, 2024, 9(11): 32366-32398. doi: 10.3934/math.20241552
[8]	Xiaoli Wang, Lizhen Wang . Traveling wave solutions of conformable time fractional Burgers type equations. AIMS Mathematics, 2021, 6(7): 7266-7284. doi: 10.3934/math.2021426
[9]	A. K. M. Kazi Sazzad Hossain, M. Ali Akbar . Solitary wave solutions of few nonlinear evolution equations. AIMS Mathematics, 2020, 5(2): 1199-1215. doi: 10.3934/math.2020083
[10]	Zhe Ji, Yifan Nie, Lingfei Li, Yingying Xie, Mancang Wang . Rational solutions of an extended (2+1)-dimensional Camassa-Holm- Kadomtsev-Petviashvili equation in liquid drop. AIMS Mathematics, 2023, 8(2): 3163-3184. doi: 10.3934/math.2023162

Abstract

1. Introduction

Nonlinear evolution equations (NLEEs) play an important part in the study of nonlinear science, particular in plasma physics, quantum field theory, nonlinear wave propagation and nonlinear optical fibers so that it attracted the attention of a large number of scholars. The extended auxiliary equation technique ^[1], the Bernoulli's equation approach ^[2], the Exp-function technique ^[3], the homotopy analysis technique ^[4], the homotopy perturbation technique ^[5], the improved $tan(\phi/2)$ -expansion technique (^[6,7]), the Hirota's bilinear technique ^{[8,9,10,11,12,13,14,15]}, the He's variational principle ^[16,17], the binary Darboux transformation ^[18], the Lie group analysis ^[19,20], the Bäcklund transformation method ^[21], optimal galerkin-homotopy asymptotic method applied ^[22], and the multiple rogue waves method (^[23,24]) have been proposed to solve NLEEs. By using these approaches, various exact solutions including soliton solution, lump solution, rogue wave solution, periodic solution, interaction solution, rational solution and high-order rational solution were obtained (^[25,26]).

In this paper, we mainly consider the following dynamical model, which can be used to describe some interesting (3+1)-dimensional waves of physics, namely, the generalized Kadomtsev-Petviashvili (gKP) equation ^[27]. That is

$\begin{equation} (\Psi_{t}+6\Psi\Psi_x+\Psi_{xxx})_x +a\Phi_{yy} = 0, \end{equation}$

(1.1)

and also above equation is integrable. Author of ^[28] introduced the modification of KP (mKP) equation ^[29] given

$\begin{equation} 4\Psi_{t}-6\Psi^2\Psi_x+\Psi_{xxx}+6\Psi_x\partial_{x}^{-1}\Psi_y +\Psi_{x}^{-1}\Phi_{yy} = 0. \end{equation}$

(1.2)

The generalized KP (gKP) equation has been researched by some scholars ^[30,31,32] in which is given as

$\begin{equation} (\Psi_{t}+\alpha\Psi_x +\beta\Psi\Psi_x+\gamma\Psi\Psi_{xxt})_x+\Psi_{yy} = 0. \end{equation}$

(1.3)

The another type of gKP equation is given in ^[33] as below

$\begin{equation} \Psi_{xxxy}+3(\Psi_x\Psi_y)_x+\Psi_{tx}+\Psi_{ty}+\Psi_{tz}-\Psi_{zz} = 0. \end{equation}$

(1.4)

We first present the bilinear form for Eq (1.4), by taking the following first-order logarithmic transformation

$\begin{equation} \Psi = 2(\ln f)_{x}, \end{equation}$

(1.5)

then, Eq (1.4) is turned into the bilinear form

$\begin{equation} \left(D_x^3 D_y+D_xD_t+D_yD_t+D_zD_t-D_z^2\right)\mathfrak{f}. \mathfrak{f} = 0, \end{equation}$

(1.6)

in which $D_t, D_x, D_y$ and $D_z$ are Hirota's bilinear frames. Cao ^[34] investigated the generalized B-type KP equation as follows

$\begin{equation} \Psi_{xxxy}+3(\Psi_x\Psi_y)_x-3\Psi_{xz}-\Psi_{ty} = 0. \end{equation}$

(1.7)

Guan et al. ^[35] derived a (3+1)-dimensional gKP equation in below form

$\begin{equation} \Psi_{xxxy}+3(\Psi_x \Psi_{y})_x+\alpha\Psi_{xxxz}+3\alpha(\Psi_x \Psi_{z})_x+\lambda_1\Psi_{xt}+\lambda_2\Phi_{yt}+\lambda_3\Phi_{zt}+ \omega_1\Phi_{xz} +\omega_2\Phi_{yz}+\omega_3\Phi_{zz} = 0, \end{equation}$

(1.8)

and some lump soliton solutions have been constructed using the Hirota bilinear method in ^[36]. Via transformation $\Psi = 2(\ln f)_{x},$ the bilinear form of equation (1.8) reads:

$\begin{equation} \left(D_x^3 D_y+\alpha D_x^3 D_z+\lambda_1D_xD_t+\lambda_2D_yD_t+\lambda_3D_zD_t+\omega_1D_xD_z+ \omega_2D_yD_z+\omega_3D_z^2\right)\mathfrak{f}. \mathfrak{f} = 0. \end{equation}$

(1.9)

Most classical test functions for solving NLPDEs by using the several particular functions can be constructed via Hirota bilinear technique. In other words, Hirota operator covers most of the classical hypothesis function method. For example, the fractional generalized CBS-BK equation ^[37], the generalized Bogoyavlensky-Konopelchenko equation ^[38], the (2+1)-dimensional asymmetrical Nizhnik-Novikov-Veselov equation ^[39], and the (2+1)-dimensional generalized variable-coefficient KP-Burgers-type equation ^[40]. Diverse kinds of studies on solve NLPDEs were perused via mighty authors in which some of them can be stated, for instance, multivariate rogue wave to some PDEs ^[41], interaction lump solutions the gKP equation ^[42], the (1+1)-dimensional coupled integrable dispersionless equations ^[43]. Therefore, we embark on the new research topic of constructing the analytic solutions of nonlinear PDEs by exploring the bilinear method. According to recent studies, we can obtain some of the new exact analytic solutions of nonlinear PDEs by way of constructing their corresponding bilinear differential equations in ^{[44,45,46,47]}. Here, we will study the multiple Exp-function method (MEFM) for determining the multiple soliton solutions (MSSs). The MEFM employed by some of powerful authors for various nonlinear equations have been surveyed in more studies in ^{[31,48,49,50]}. Authors of ^[51] utilized the reduced differential transform method for solving partial differential equations. Also, Yu and Sun ^[52] studied the dimensionally reduced generalized KP equations by help of Hirita bilinear method and obtained of lump solutions.

The outline of our paper is as follows: the multiple Exp-function scheme has been summarized in section 2. In sections 3, the KP equation, will investigate to finding 1-wave, 2-wave, and three-wave solutions. The Sections 4-6 devote to determined the periodic, cross-kink, and solitary wave solutions. Moreover, in Section 7, the modulation instability analysis is investigated. Finally in Section 8, the SIVP technique is considered with four cases for finding the solitary, bright, dark and singular wave solutions. A few of conclusions and outlook will be given in the final section.

2. Multiple Exp-function method

This method was summarized and improved for achieving the analytic solutions of NLPDEs:

Step 1. Assume a nonlinear PDE is given in general frame as follows

$\begin{equation} \mathcal{N}(x, y, t, \Psi , \Psi _{x}, \Psi _{y}, \Psi _{z}, \Psi _{t}, \Psi _{xx}, \Psi _{tt}, ...) = 0. \end{equation}$

(2.1)

Take the novel variables $\xi _{i} = \xi _{i}(x, y, z, t), 1\leq i\leq n$ , by differentiable frames:

$\begin{equation} \xi _{i, x} = \alpha _{i}\xi _{i}, \ \ \xi _{i, y} = \beta _{i}\xi _{i}, \ \ \xi _{i, z} = \gamma _{i}\xi _{i}, \ \ \xi _{i, t} = -\delta _{i}\xi _{i}, \ \ 1\leq i\leq n, \end{equation}$

(2.2)

where $\alpha _{i}, \beta _{i}, \gamma _{i}, 1\leq i\leq n$ , are unfound amounts. It noted that one can get as the following function

$\begin{equation} \xi _{i} = \varpi _{i}e^{\theta _{i}}, \ \ \theta _{i} = \alpha _{i}x+\beta _{i}y+\gamma _{i}z-\delta _{i}t, \ \ 1\leq i\leq n, \end{equation}$

(2.3)

where $\varpi _{i}, 1\leq i\leq n$ , unspecified amounts.

Step 2. Assuming the solution of the Eq (2.1) is function of variables $\xi _{i}, 1\leq i\leq n$ :

$\begin{equation} \Psi = \frac{\Delta (\xi _{1}, \xi _{2}, ..., \xi _{n})}{\Omega (\xi _{1}, \xi _{2}, ..., \xi _{n})}, \ \ \Delta = \sum\limits_{r, s = 1}^{n}\sum\limits_{i, j = 0}^{M}\Delta _{rs, ij}\xi _{r}^{i}\xi _{s}^{j}, \ \ \Omega = \sum\limits_{r, s = 1}^{n}\sum\limits_{i, j = 0}^{N}\Omega _{rs, ij}\xi _{r}^{i}\xi _{s}^{j}, \ \ \end{equation}$

(2.4)

in which $\Delta _{rs, ij}$ and $\Omega _{rs, ij}$ are amounts to be remained. Replacing Eq (2.4) into Eq (2.1) can be achieved the below form as:

$\begin{equation} \Psi = \frac{\Delta (\varpi _{1}e^{\alpha _{1}x+\beta _{1}y+\gamma _{1}z-\delta _{1}t}, ..., \varpi _{n}e^{\alpha _{n}x+\beta _{n}y+\gamma _{n}z-\delta _{n}t})}{\Omega (\varpi _{1}e^{\alpha _{1}x+\beta _{1}y+\gamma _{1}z-\delta _{1}t}, ..., \varpi _{n}e^{\alpha _{n}x+\beta _{n}y+\gamma _{n}z-\delta _{n}t})}, \end{equation}$

(2.5)

and also we have

$\begin{eqnarray} \Delta _{t} & = &\sum\limits_{i = 1}^{n}\Delta _{\xi _{i}}\xi _{i, t}, \ \ \Omega _{t} = \sum\limits_{i = 1}^{n}\Omega _{\xi _{i}}\xi _{i, t}, \ \ \Delta _{x} = \sum\limits_{i = 1}^{n}\Delta _{\xi _{i}}\xi _{i, x}, \ \ \Omega _{x} = \sum\limits_{i = 1}^{n}\Omega _{\xi _{i}}\xi _{i, x}, \ \ \Delta _{y} = \sum\limits_{i = 1}^{n}\Delta _{\xi _{i}}\xi _{i, y}, \ \\ \ \Delta _{z} & = &\sum\limits_{i = 1}^{n}\Delta _{\xi _{i}}\xi _{i, z}, \rm{ \ }\Omega _{y} = \sum\limits_{i = 1}^{n}\Omega _{\xi _{i}}\xi _{i, y}, \ \ \Omega _{z} = \sum\limits_{i = 1}^{n}\Omega _{\xi _{i}}\xi _{i, z}, \ \end{eqnarray}$

(2.6)

$\ \ \ \Psi _{t} = \frac{\Omega \sum\limits_{i = 1}^{n}\Delta _{\xi _{i}}\xi _{i, t}-\Delta \sum\limits_{i = 1}^{n}\Omega _{\xi _{i}}\xi _{i, t}}{\Omega ^{2}}, \ \ \Psi _{x} = \frac{\Omega \sum\limits_{i = 1}^{n}\Delta _{\xi _{i}}\xi _{i, x}-\Delta \sum\limits_{i = 1}^{n}\Omega _{\xi _{i}}\xi _{i, x}}{\Omega ^{2}},$

$\Psi _{y} = \frac{\Omega \sum\limits_{i = 1}^{n}\Delta _{\xi _{i}}\xi _{i, y}-\Delta \sum\limits_{i = 1}^{n}\Omega _{\xi _{i}}\xi _{i, y}}{\Omega ^{2}}, \ \ \Psi _{z} = \frac{ \Omega \sum\limits_{i = 1}^{n}\Delta _{\xi _{i}}\xi _{i, z}-\Delta \sum\limits_{i = 1}^{n}\Omega _{\xi _{i}}\xi _{i, z}}{\Omega ^{2}}.$

3. MSSs for the (3+1) gKP equation

3.1. Option I: 1-wave solution

The one-wave function of the solution will be reduced as below form

$\begin{equation} \Psi = \frac{2\Delta _{1}}{\Omega _{1}}, \ \ \Omega _{1} = 1+\rho _{1}+\rho _{2}\ e^{\alpha _{1}x+\beta _{1}y+\gamma _{1}z-\delta _{1}t}, \ \ \Delta _{1} = \sigma _{1}+\sigma _{2}\ e^{\alpha _{1}x+\beta _{1}y+\gamma _{1}z-\delta _{1}t}, \end{equation}$

(3.1)

in which $\rho _{1}, \rho _{2}, \sigma _{1}$ and $\sigma _{2}$ are unspecified amounts. Substituting (3.1) into Eq (1.8), the below cases will be concluded as:

Case I:

$\begin{equation} \alpha _{1} = \alpha _{1}, \ \ \beta _{1} = \beta _{1}, \ \ \rho _{1} = \rho _{1}, \ \ \rho _{2} = \ \ {\frac{\sigma _{{2}}\left( \rho _{{1}}+1\right) }{\sigma _{{1}}} \ \ } , \ \ \sigma _{1} = \sigma _{1}, \ \ \sigma _{{2}} = \sigma _{2}, \ \ \delta _{{1} } = \delta _{1}, \ \ \gamma _{1} = \gamma _{1}, \ \ \gamma _{2} = \gamma _{2}. \end{equation}$

(3.2)

Case II:

$\begin{equation} \alpha _{1} = \alpha _{1}, \ \ \beta _{1} = - \ \ {\frac{\alpha \, {\alpha _{{1}}} \ \ ^{3}\gamma _{{1}}-\alpha _{{1}}\delta _{{1}}\lambda _{{1}}+\alpha _{{1} }\gamma _{{1}}\omega _{{1}}-\delta _{{1}}\gamma _{{1}}\lambda _{{3}}+{\gamma _{{1}}} ^{2}\omega _{{3}}} \ \ {{\alpha _{{1}}} ^{3}-\delta _{{1}}\lambda _{{2} }+\gamma _{{1}}\omega _{{2}}} }, \ \ \rho _{1} = -1, \ \ \rho _{2} = \rho _{2}, \ \ \end{equation}$

(3.3)

$\sigma _{1} = \sigma _{1}, \ \ \sigma _{{2}} = \sigma _{2}, \ \ \delta _{{1} } = \delta _{1}, \ \ \gamma _{1} = \gamma _{1}, \ \ \gamma _{2} = \gamma _{2}.$

Case III:

$\begin{equation} \alpha _{1} = \ \ {\frac{\gamma _{{1}}\left( \alpha \, \delta _{{1}}\lambda _{{2} }-\alpha \, \gamma _{{1}}\omega _{{2}}-\delta _{{1}}\lambda _{{3}}+\gamma _{{1 }}\omega _{{3}}\right) }{\delta _{{1}}\lambda _{{1}}-\gamma _{{1}}\omega _{{1 }}} }, \ \ \beta _{1} = -\alpha \gamma _{1}, \ \ \rho _{1} = \rho _{1}, \ \ \rho _{2} = \rho _{2}, \ \ \end{equation}$

(3.4)

$\sigma _{1} = \sigma _{1}, \ \ \sigma _{{2}} = \sigma _{2}, \ \ \delta _{{1} } = \delta _{1}, \ \ \ \ \gamma _{1} = \gamma _{1}, \ \ \gamma _{2} = \gamma _{2}.$

Case IV:

$\begin{eqnarray} \alpha _{1} & = &\vartheta , \ \ \beta _{1} = \beta _{1}, \ or\ \ \beta _{1} = -\alpha \gamma _{1}, \ \ \ \rho _{1} = -1, \ \ \rho _{2} = \rho _{2}, \ \ \sigma _{1} = \sigma _{1}, \ \ \\ \sigma _{{2}} & = &\sigma _{2}, \ \delta _{{1}} = \frac{\vartheta ^{3}+\gamma _{1}\omega _{2}}{\lambda _{2}}, \ \ \gamma_1 = \gamma_1, \ \ \gamma_2 = \gamma_2, \ \end{eqnarray}$

(3.5)

in which $\vartheta$ , solves the equation $\lambda_1\vartheta ^4+(\gamma _1\lambda _3-\alpha \gamma _1\lambda_2) {\vartheta }^{3}+(\gamma _1\lambda _1\omega _2-\gamma_1\lambda _2\omega _1) \vartheta -\gamma _1 ^2\omega _3\lambda _2+\gamma_1^2\lambda _3\omega_2 = 0.$

For example, the 1-wave solution for Case III will be considered as

$\begin{equation} \Psi = 2\frac{\sigma _{1}+\sigma _{2}e^{ \ \ {\frac{\gamma _{{1}}\left( \alpha \, \delta _{{1}}\lambda _{{2}}-\alpha \, \gamma _{{1}}\omega _{{2}}-\delta _{{1 }}\lambda _{{3}}+\gamma _{{1}}\omega _{{3}}\right) }{\delta _{{1}}\lambda _{{ 1}}-\gamma _{{1}}\omega _{{1}}} \ \ }x-\alpha \gamma _{1}y+\gamma _{1}z-\delta _{1}t}}{1+\rho _{1}+\rho _{2}e^{ \ \ {\frac{\gamma _{{1}}\left( \alpha \, \delta _{ {1}}\lambda _{{2}}-\alpha \, \gamma _{{1}}\omega _{{2}}-\delta _{{1}}\lambda _{{3}}+\gamma _{{1}}\omega _{{3}}\right) }{\delta _{{1}}\lambda _{{1} }-\gamma _{{1}}\omega _{{1}}} \ \ }\ \ x-\alpha \gamma _{1}y+\gamma _{1}z-\delta _{1}t}}. \end{equation}$

(3.6)

3.2. Option II: 2-wave solutions

The two-wave function of the solution will be reduced as below form

$\begin{equation} \Psi = \frac{2\Delta _{2}}{\Omega _{2}}, \end{equation}$

(3.7)

$\begin{equation} \Omega _{2} = 1+\sigma _{1}e^{\alpha _{1}x+\beta _{1}y+\gamma _{1}z-\delta _{1}t}+\sigma _{2}e^{\alpha _{2}x+\beta _{2}y+\gamma _{2}z-\delta _{2}t}+\sigma _{1}\sigma _{2}\sigma _{12}e^{(\alpha _{1}+\alpha _{2})x+(\beta _{1}+\beta _{2})y+(\gamma _{1}+\gamma _{2})z-(\delta _{1}+\delta _{2})t}, \ \ \end{equation}$

(3.8)

$\Delta _{2} = \rho _{1}e^{\alpha _{1}x+\beta _{1}y+\gamma _{1}z-\delta _{1}t}+\rho _{2}e^{\alpha _{2}x+\beta _{2}y+\gamma _{2}z-\delta _{2}t}+\rho _{1}\rho _{2}\rho _{12}e^{(\alpha _{1}+\alpha _{2})x+(\beta _{1}+\beta _{2})y+(\gamma _{1}+\gamma _{2})z-(\delta _{1}+\delta _{2})t}.$

Substituting (3.7) in terms of (3.8) into Eq (1.8), the below cases will be resulted as:

Case I:

$\begin{eqnarray} \alpha _{1} & = &0, \ \ \alpha _{2} = \alpha _{2}, \ \ \beta _{1} = \beta _{1}, \ \ \beta _{2} = -\alpha \gamma _{2}, \ \ \delta _{{1}} = \ \ {\frac{\gamma _{{1}}\left( \beta _{{1}}\omega _{{2}}+\omega _{{3}}\gamma _{{1}}\right) }{\beta _{{1} }\lambda _{{2}}+\gamma _{{1}}\lambda _{{3}}} }, \ \ \\ \delta _{{2}} & = & \ \ {\frac{\gamma _{{2}}\left( \alpha \, \gamma _{{2}}\omega _{{ \ 2}}-\alpha _{{2}}\omega _{{1}}-\gamma _{{2}}\omega _{{3}}\right) }{\alpha \, \gamma _{{2}}\lambda _{{2}}-\alpha _{{2}}\lambda _{{1}}-\gamma _{{2} }\lambda _{{3}}} }, \gamma _{1} = \gamma _{1}, \ \ \gamma _{2} = \gamma _{2}, \ \ \rho _{1} = 0, \ \ \rho _{2} = \rho _{2}, \end{eqnarray}$

(3.9)

$\ \ \rho _{12} = \rho _{12}, \ \ \sigma _{1} = \sigma _{1}, \ \ \sigma _{2} = \sigma _{2}, \ \ \sigma _{12} = 1.$

Case II:

$\begin{eqnarray} \alpha _{1} & = &\alpha _{1}, \ \ \alpha _{2} = 0, \ \ \beta _{1} = -\alpha \gamma _{1}, \ \ \beta _{2} = \beta _{2}, \ \ \delta _{{1}} = \ \ {\frac{\gamma _{{1}}\left( \alpha \, \gamma _{{1}}\omega _{{\ 2}}-\alpha _{{1}}\omega _{{1}}-\omega _{{3} }\gamma _{{1}}\right) }{\alpha \, \gamma _{{1}}\lambda _{{2}}-\alpha _{{1} }\lambda _{{1}}-\gamma _{{1}}\lambda _{{3}}} }, \\ \ \ \delta _{{2}} & = & \ \ {\frac{\gamma _{{2}}\left( \beta _{{2}}\omega _{{2} }+\gamma _{{2}}\omega _{{3}}\right) }{\beta _{{2}}\lambda _{{2}}+\gamma _{{2} }\lambda _{{3}}} }, \gamma _{1} = \gamma _{1}, \ \ \gamma _{2} = \gamma _{2}, \ \ \rho _{1} = 0, \ \end{eqnarray}$

(3.10)

$\ \rho _{2} = \rho _{2}, \ \ \rho _{12} = \rho _{12}, \ \ \sigma _{1} = \sigma _{1}, \ \ \sigma _{2} = \sigma _{2}, \ \ \sigma _{12} = 1.$

Case III:

$\begin{eqnarray} \alpha _{1} & = &\alpha _{1}, \ \ \alpha _{2} = \alpha _{2}, \ \ \beta _{1} = -\alpha \gamma _{1}, \ \ \beta _{2} = -\alpha \gamma _{2}, \ \ \delta _{{1} } = \ \ {\frac{\gamma _{{1}}\left( \alpha \, \gamma _{{1}}\omega _{{\ 2}}-\alpha _{{ 1}}\omega _{{1}}-\omega _{{3}}\gamma _{{1}}\right) }{\alpha \, \gamma _{{1} }\lambda _{{2}}-\alpha _{{1}}\lambda _{{1}}-\gamma _{{1}}\lambda _{{3}}} }, \ \\ \ \delta _{{2}} & = & \ \ {\frac{\gamma _{{2}}\left( \alpha \, \gamma _{{2}}\omega _{ {\ 2}}-\alpha _{{2}}\omega _{{1}}-\gamma _{{2}}\omega _{{3}}\right) }{\alpha \, \gamma _{{2}}\lambda _{{2}}-\alpha _{{2}}\lambda _{{1}}-\gamma _{{2} }\lambda _{{3}}} }, \gamma _{1} = \gamma _{1}, \ \ \gamma _{2} = \gamma _{2}, \ \ \rho _{1} = 0, \end{eqnarray}$

(3.11)

$\ \ \rho _{2} = \rho _{2}, \ \ \rho _{12} = \rho _{12}, \ \ \sigma _{1} = \sigma _{1}, \ \ \sigma _{2} = \sigma _{2}, \ \ \sigma _{12} = 1.$

Case IV:

$\begin{eqnarray} \alpha _{1} & = &0, \ \ \alpha _{2} = \alpha _{2}, \ \ \beta _{1} = \beta _{1}, \ \ \beta _{2} = -\alpha \gamma _{2}, \ \ \delta _{{1}} = \ \ {\frac{\gamma _{{1}}\left( \beta _{{1}}\omega _{{2}}+\omega _{{3}}\gamma _{{1}}\right) }{\beta _{{1} }\lambda _{{2}}+\gamma _{{1}}\lambda _{{3}}} }, \ \\ \ \delta _{{2}} & = & \ \ {\frac{\gamma _{{2}}\left( \alpha \, \gamma _{{2}}\omega _{ {\ 2}}-\alpha _{{2}}\omega _{{1}}-\gamma _{{2}}\omega _{{3}}\right) }{\alpha \, \gamma _{{2}}\lambda _{{2}}-\alpha _{{2}}\lambda _{{1}}-\gamma _{{2} }\lambda _{{3}}} }, \gamma _{1} = \gamma _{1}, \ \ \gamma _{2} = \gamma _{2}, \ \ \rho _{1} = \rho _{1}, \ \ \rho _{2} = 0, \end{eqnarray}$

(3.12)

$\ \ \rho _{12} = \rho _{12}, \ \ \sigma _{1} = \sigma _{1}, \ \ \sigma _{2} = \sigma _{2}, \ \ \sigma _{12} = 1.$

Case V:

$\begin{eqnarray} \alpha _{1} & = &\alpha _{1}, \ \ \alpha _{2} = \alpha _{2}, \ \ \beta _{1} = -\alpha \gamma _{1}, \ \ \beta _{2} = -\alpha \gamma _{2}, \ \ \delta _{{1} } = \ \ {\frac{\gamma _{{1}}\left( \alpha \, \gamma _{{1}}\omega _{{\ 2}}-\alpha _{{ 1}}\omega _{{1}}-\omega _{{3}}\gamma _{{1}}\right) }{\alpha \, \gamma _{{1} }\lambda _{{2}}-\alpha _{{1}}\lambda _{{1}}-\gamma _{{1}}\lambda _{{3}}} }, \\ \ \ \delta _{{2}} & = & \ \ {\frac{\gamma _{{2}}\left( \alpha \, \gamma _{{2}}\omega _{{\ 2}}-\alpha _{{2}}\omega _{{1}}-\gamma _{{2}}\omega _{{3}}\right) }{ \alpha \, \gamma _{{2}}\lambda _{{2}}-\alpha _{{2}}\lambda _{{1}}-\gamma _{{2} }\lambda _{{3}}} }, \gamma _{1} = \gamma _{1}, \ \ \gamma _{2} = \gamma _{2}, \ \ \rho _{1} = \rho _{1}, \ \ \rho _{2} = 0, \end{eqnarray}$

(3.13)

$\ \ \rho _{12} = \rho _{12}, \ \ \sigma _{1} = \sigma _{1}, \ \ \sigma _{2} = \sigma _{2}, \ \ \sigma _{12} = 1.$

Case VI:

$\begin{eqnarray} \alpha _{1} & = &\alpha _{1}, \ \ \alpha _{2} = \alpha _{2}, \ \ \beta _{1} = -{ \frac{\alpha \, \alpha _{{1}}\gamma _{{2}}} \ \ {\alpha _{{2}}} }, \ \ \beta _{2} = -\alpha \gamma _{2}, \ \ \delta _{{1}} = \ \ {\frac{\alpha _{{1}}\gamma _{{2} }\left( \alpha \, \gamma _{{\ 2}}\omega _{{2}}-\alpha _{{2}}\omega _{{1} }-\gamma _{{2}}\omega _{{3}}\right) }{\alpha _{{2}}\left( \alpha \, \gamma _{{ 2}}\lambda _{{2}}-\alpha _{{2}}\lambda _{{1}}-\gamma _{{2}}\lambda _{{3} }\right) }}, \ \\ \ \delta _{{2}} & = & \ \ {\frac{\gamma _{{2}}\left( \alpha \, \gamma _{{2}}\omega _{ {\ 2}}-\alpha _{{2}}\omega _{{1}}-\gamma _{{2}}\omega _{{3}}\right) }{\alpha \, \gamma _{{2}}\lambda _{{2}}-\alpha _{{2}}\lambda _{{1}}-\gamma _{{2} }\lambda _{{3}}} }, \gamma _{1} = \gamma _{1}, \ \ \gamma _{2} = \gamma _{2}, \ \ \rho _{1} = \rho _{1}, \ \ \rho _{2} = 0, \end{eqnarray}$

(3.14)

$\ \ \rho _{12} = \rho _{12}, \ \ \sigma _{1} = \sigma _{1}, \ \ \sigma _{2} = \sigma _{2}, \ \ \sigma _{12} = \sigma _{12}.$

Case VII:

$\begin{eqnarray} \alpha _{1} & = &\alpha _{1}, \ \ \alpha _{2} = \alpha _{2}, \ \ \beta _{1} = -{ \frac{\alpha \, \alpha _{{1}}\gamma _{{2}}} \ \ {\alpha _{{2}}} }, \ \ \beta _{2} = -\alpha \gamma _{2}, \ \ \delta _{{1}} = \ \ {\frac{\alpha _{{1}}\gamma _{{2} }\left( \alpha \, \gamma _{{\ 2}}\omega _{{2}}-\alpha _{{2}}\omega _{{1} }-\gamma _{{2}}\omega _{{3}}\right) }{\alpha _{{2}}\left( \alpha \, \gamma _{{ 2}}\lambda _{{2}}-\alpha _{{2}}\lambda _{{1}}-\gamma _{{2}}\lambda _{{3} }\right) }}, \\ \ \ \delta _{{2}} & = & \ \ {\frac{\gamma _{{2}}\left( \alpha \, \gamma _{{2}}\omega _{{\ 2}}-\alpha _{{2}}\omega _{{1}}-\gamma _{{2}}\omega _{{3}}\right) }{ \alpha \, \gamma _{{2}}\lambda _{{2}}-\alpha _{{2}}\lambda _{{1}}-\gamma _{{2} }\lambda _{{3}}} }, \gamma _{1} = \ \ {\frac{\alpha _{{1}}\gamma _{{2}}} \ \ {\alpha _{{2} }}} \ \ , \ \ \gamma _{2} = \gamma _{2}, \ \ \rho _{1} = \rho _{1}, \ \ \rho _{2} = \rho _{2}, \end{eqnarray}$

(3.15)

$\ \ \rho _{12} = \rho _{12}, \ \ \sigma _{1} = 0, \ \ \sigma _{2} = \sigma _{2}, \ \ \sigma _{12} = \sigma _{12}.$

Case VIII:

$\begin{eqnarray} \alpha _{1} & = &\frac{1}{2}\alpha _{2}, \ \ \alpha _{2} = \alpha _{2}, \ \ \beta _{1} = -\frac{1}{2}\, \alpha \, \gamma _{{2}}, \ \ \beta _{2} = -\alpha \gamma _{2}, \ \ \delta _{{1}} = \frac{1}{2}\, \ \ {\frac{\gamma _{{2}}\left( \alpha \, \gamma _{{2}}\omega _{{2}}-\alpha _{{2}}\omega _{{1}}-\gamma _{{2}}\omega _{{3}}\right) }{\alpha \, \gamma _{{2}}\lambda _{{2}}-\alpha _{{2}}\lambda _{{ 1}}-\gamma _{{2}}\lambda _{{3}}} }, \ \\ \ \delta _{{2}} & = & \ \ {\frac{\gamma _{{2}}\left( \alpha \, \gamma _{{2}}\omega _{ {\ 2}}-\alpha _{{2}}\omega _{{1}}-\gamma _{{2}}\omega _{{3}}\right) }{\alpha \, \gamma _{{2}}\lambda _{{2}}-\alpha _{{2}}\lambda _{{1}}-\gamma _{{2} }\lambda _{{3}}} }, \gamma _{1} = \frac{1}{2}\gamma _{2}, \ \ \gamma _{2} = \gamma _{2}, \ \ \rho _{1} = \rho _{1}, \ \end{eqnarray}$

(3.16)

$\ \rho _{2} = \rho _{2}, \ \ \rho _{12} = \rho _{12}, \ \ \sigma _{1} = \sigma _{1}, \ \ \sigma _{2} = \sigma _{2}, \ \ \sigma _{12} = 0.$

For instance, the 2-wave solution for Case I will be taken as

$\begin{equation} \Psi _{1} = \left. 2\, \rho _{{2}}{\mathrm{e}^{- \ \ {\frac{t\gamma _{{2}}\left( \alpha \, \gamma _{{\ 2}}\omega _{{2}}-\alpha _{{2}}\omega _{{1}}-\gamma _{{2} }\omega _{{3}}\right) }{\alpha \, \gamma _{{2}}\lambda _{{2}}-\alpha _{{2} }\lambda _{{1}}-\gamma _{{2}}\lambda _{{3}}} \ \ }+x\alpha _{{2}}-y\alpha \, \gamma _{{2}}+z\gamma _{{2}}} \ \ }\right/ \left( 1+\sigma _{{1}}{\mathrm{e}^{-{ \frac{t\gamma _{{1}}\left( \beta _{{1}}\omega _{{2}}+\omega _{{3}}\gamma _{{1 }}\right) }{\beta _{{1}}\lambda _{{2}}+\gamma _{{1}}\lambda _{{3}}} \ \ }+y\beta _{{1}}+z\gamma _{{1}}} \ \ }+\right. \end{equation}$

(3.17)

$\left. \sigma _{{2}}{\mathrm{e}^{- \ \ {\frac{t\gamma _{{2}}\left( \alpha \, \gamma _{{2}}\omega _{{2}}-\alpha _{{2}}\omega _{{1}}-\gamma _{{2}}\omega _{{3}}\right) }{\alpha \, \gamma _{{2}}\lambda _{{2}}-\alpha _{{2}}\lambda _{{ 1}}-\gamma _{{2}}\lambda _{{3}}} \ \ }+x\alpha _{{2}}-y\alpha \, \gamma _{{2} }+z\gamma _{{2}}} \ \ }+\sigma _{{1}}\sigma _{{2}}{\mathrm{e}^{- \ \ {\frac{t\gamma _{{ 1}}\left( \beta _{{1}}\omega _{{2}}+\omega _{{3}}\gamma _{{1}}\right) }{ \beta _{{1}}\lambda _{{2}}+\gamma _{{1}}\lambda _{{3}}} \ \ }- \ \ {\frac{t\gamma _{{2} }\left( \alpha \, \gamma _{{2}}\omega _{{2}}-\alpha _{{2}}\omega _{{1} }-\gamma _{{2}}\omega _{{3}}\right) }{\alpha \, \gamma _{{2}}\lambda _{{2} }-\alpha _{{2}}\lambda _{{1}}-\gamma _{{2}}\lambda _{{3}}} \ \ }+x\alpha _{{2} }+y\beta _{{1}}-y\alpha \, \gamma _{{2}}+z\gamma _{{1}}+z\gamma _{{2}}} } \right) .$

Also, the 2-wave solution for Case III will be considered as

$\begin{equation} \Psi _{2} = \left. 2\, \rho _{{2}}{\mathrm{e}^{- \ \ {\frac{t\gamma _{{2}}\left( \alpha \, \gamma _{{\ 2}}\omega _{{2}}-\alpha _{{2}}\omega _{{1}}-\gamma _{{2} }\omega _{{3}}\right) }{\alpha \, \gamma _{{2}}\lambda _{{2}}-\alpha _{{2} }\lambda _{{1}}-\gamma _{{2}}\lambda _{{3}}} \ \ }+x\alpha _{{2}}-y\alpha \, \gamma _{{2}}+z\gamma _{{2}}} \ \ }\right/ \left( 1+\sigma _{{1}}{\mathrm{e}^{-{ \frac{t\gamma _{{1}}\left( \alpha \, \gamma _{{1}}\omega _{{2}}-\alpha _{{1} }\omega _{{1}}-\omega _{{3}}\gamma _{{1}}\right) }{\alpha \, \gamma _{{1} }\lambda _{{2}}-\alpha _{{1}}\lambda _{{1}}-\gamma _{{1}}\lambda _{{3}}} \ \ } +x\alpha _{{1}}-y\alpha \, \gamma _{{1}}+z\gamma _{{1}}} \ \ }+\right. \end{equation}$

(3.18)

$\left. \sigma _{{2}}{\mathrm{e}^{- \ \ {\frac{t\gamma _{{2}}\left( \alpha \gamma _{{2}}\omega _{{2}}-\alpha _{{2}}\omega _{{1}}-\gamma _{{2}}\omega _{{3} }\right) }{\alpha \gamma _{{2}}\lambda _{{2}}-\alpha _{{2}}\lambda _{{1} }-\gamma _{{2}}\lambda _{{3}}} \ \ }+x\alpha _{{2}}-y\alpha \gamma _{{2}}+z\gamma _{{2}}} \ \ }+\sigma _{{1}}\sigma _{{2}}{\mathrm{e}^{- \ \ {\frac{t\gamma _{{1}}\left( \alpha \gamma _{{1}}\omega _{{2}}-\alpha _{{1}}\omega _{{1}}-\omega _{{3} }\gamma _{{1}}\right) }{\alpha \gamma _{{1}}\lambda _{{2}}-\alpha _{{1} }\lambda _{{1}}-\gamma _{{1}}\lambda _{{3}}} \ \ }- \ \ {\frac{t\gamma _{{2}}\left( \alpha \gamma _{{2}}\omega _{{2}}-\alpha _{{2}}\omega _{{1}}-\gamma _{{2} }\omega _{{3}}\right) }{\alpha \gamma _{{2}}\lambda _{{2}}-\alpha _{{2} }\lambda _{{1}}-\gamma _{{2}}\lambda _{{3}}} \ \ }+x\alpha _{{1}}+x\alpha _{{2} }-y\alpha \gamma _{{1}}-y\alpha \gamma _{{2}}+z\gamma _{{1}}+z\gamma _{{2}}} } \right) .$

And finally, the resulting two-wave solution for Case VIII will be read as

$\begin{equation} \Psi _{3}(x, y, z, t) = 2\left( \rho _{{1}}{\mathrm{e}^{-\frac{1}{2} \ \ {\frac{ t\gamma _{{2}}\left( \alpha \, \gamma _{{2}}\omega _{{2}}-\alpha _{{2}}\omega _{{1}}-\gamma _{{2}}\omega _{{3}}\right) }{\alpha \, \gamma _{{2}}\lambda _{{2 }}-\alpha _{{2}}\lambda _{{1}}-\gamma _{{2}}\lambda _{{3}}} \ \ }+\frac{1}{2} x\alpha _{{2}}-\frac{1}{2}y\alpha \, \gamma _{{2}}+\frac{1}{2}z\gamma _{{2}}} \ \ } +\rho _{{2}}{\mathrm{e}^{- \ \ {\frac{t\gamma _{{2}}\left( \alpha \, \gamma _{{2} }\omega _{{2}}-\alpha _{{2}}\omega _{{1}}-\gamma _{{2}}\omega _{{3}}\right) }{\alpha \, \gamma _{{2}}\lambda _{{2}}-\alpha _{{2}}\lambda _{{1}}-\gamma _{{ 2}}\lambda _{{3}}} \ \ }+x\alpha _{{2}}-y\alpha \, \gamma _{{2}}+z\gamma _{{2}}} \ \ } +\right. \end{equation}$

(3.19)

$\left. \left. \rho _{{1}}\rho _{{2}}\rho _{{12}}{\ \mathrm{e}^{-\frac{3}{2}{ \frac{t\gamma _{{2}}\left( \alpha \, \gamma _{{2}}\omega _{{2}}-\alpha _{{2} }\omega _{{1}}-\gamma _{{2}}\omega _{{3}}\right) }{\alpha \, \gamma _{{2} }\lambda _{{2}}-\alpha _{{2}}\lambda _{{1}}-\gamma _{{2}}\lambda _{{3}}} \ \ }+ \frac{3}{2}x\alpha _{{2}}-\frac{3}{2}y\alpha \, \gamma _{{2}}+\frac{3}{2} z\gamma _{{2}}} \ \ }\right) \right/ \left( 1+\sigma _{{1}}{\mathrm{e}^{-\frac{1}{ 2} \ \ {\frac{t\gamma _{{2}}\left( \alpha \, \gamma _{{2}}\omega _{{2}}-\alpha _{{2 }}\omega _{{1}}-\gamma _{{2}}\omega _{{3}}\right) }{\alpha \, \gamma _{{2} }\lambda _{{2}}-\alpha _{{2}}\lambda _{{1}}-\gamma _{{2}}\lambda _{{3}}} \ \ }+ \frac{1}{2}x\alpha _{{2}}-\frac{1}{2}y\alpha \gamma _{{2}}+\frac{1}{2} z\gamma _{{2}}} \ \ }\right.$

$\left. +\sigma _{{2}}{\mathrm{e}^{- \ \ {\frac{t\gamma _{{2}}\left( \alpha \, \gamma _{{2}}\omega _{{2}}-\alpha _{{2}}\omega _{{1}}-\gamma _{{2}}\omega _{{3}}\right) }{\alpha \, \gamma _{{2}}\lambda _{{2}}-\alpha _{{2}}\lambda _{{ 1}}-\gamma _{{2}}\lambda _{{3}}} \ \ }+x\alpha _{{2}}-y\alpha \, \gamma _{{2} }+z\gamma _{{2}}} \ \ }\right) .$

3.3. Option III: Triple-wave solutions

The triple-wave function of the solution will be reduced as below form

$\begin{equation} \Psi = \frac{\Delta _{3}}{\Omega _{3}}, \end{equation}$

(3.20)

$\begin{equation} \Omega _{3} = 1+\rho _{1}e^{\Lambda _{1}}+\rho _{2}e^{\Lambda _{2}}+\rho _{3}e^{\Lambda _{3}}+\rho _{1}\rho _{2}\rho _{12}e^{\Lambda _{1}+\Lambda _{2}}+\rho _{1}\rho _{3}\rho _{13}e^{\Lambda _{1}+\Lambda _{3}}+\rho _{2}\rho _{3}\rho _{23}e^{\Lambda _{2}+\Lambda _{3}}+\rho _{1}\rho _{2}\rho _{3}\rho _{12}\rho _{13}\rho _{23}e^{\Lambda _{1}+\Lambda _{2}+\Lambda _{3}}, \ \ \end{equation}$

(3.21)

$\Delta _{3} = 2\sigma _{1}e^{\Lambda _{1}}+2\sigma _{2}e^{\Lambda _{2}}+2\sigma _{1}\sigma _{2}\sigma _{12}e^{\Lambda _{1}+\Lambda _{2}}+2\sigma _{1}\sigma _{3}\sigma _{13}e^{\Lambda _{1}+\Lambda _{3}}+2\sigma _{2}\sigma _{3}\sigma _{23}e^{\Lambda _{2}+\Lambda _{3}}+$

$2\sigma _{1}\sigma _{2}\sigma _{3}\sigma _{12}\sigma _{13}\sigma _{23}e^{\Lambda _{1}+\Lambda _{2}+\Lambda _{3}},$

in which $\Lambda _{i} = \alpha _{i}x+\beta _{i}y+\gamma _{i}x-\delta _{i}t, i = 1, 2, 3$ . Inserting (3.20) in terms of (3.21) into Eq (1.8), the below case will be reached:

$\begin{eqnarray} \alpha _{i} & = &\alpha _{i}, \ \ \gamma _{i} = \gamma _{i}, \ \ \sigma _{i} = \sigma _{i}, \ \ \rho _{1} = 0, \ \ \rho _{2} = \rho _{2}, \ \ \rho _{3} = \rho _{3}, \ \ \beta _{{i}} = -\alpha \, \gamma _{{i}}, \ \ \\ \delta _{{i}} & = & \ \ {\frac{\gamma _{{i}}\left( \alpha \, \gamma _{{i}}\omega _{{ \ 2}}-\alpha _{{i}}\omega _{{1}}-\omega _{{3}}\gamma _{{i}}\right) }{\alpha \, \gamma _{{i}}\lambda _{{2}}-\alpha _{{i}}\lambda _{{1}}-\gamma _{{i} }\lambda _{{3}}} }, \ \ i = 1, 2, 3, \ \ \rho _{ij} = \rho _{ij}, \ \ \sigma _{ij} = 1, \ \ i, j = 1, 2, 3, \ i\neq j. \end{eqnarray}$

(3.22)

Then, the solution is

$\begin{equation} \Psi _{1} = \frac{2\sigma _{1}e^{\Lambda _{1}}+2\sigma _{2}e^{\Lambda _{2}}+2\sigma _{1}\sigma _{2}e^{\Lambda _{1}+\Lambda _{2}}+2\sigma _{1}\sigma _{3}e^{\Lambda _{1}+\Lambda _{3}}+2\sigma _{2}\sigma _{3}e^{\Lambda _{2}+\Lambda _{3}}+2\sigma _{1}\sigma _{2}\sigma _{3}e^{\Lambda _{1}+\Lambda _{2}+\Lambda _{3}}} \ \ {1+\rho _{2}e^{\Lambda _{2}}+\rho _{3}e^{\Lambda _{3}}+\rho _{2}\rho _{3}\rho _{23}e^{\Lambda _{2}+\Lambda _{3}}} \ \ , \end{equation}$

(3.23)

in which $\Lambda _{i} = \alpha _{i}x-\alpha \, \gamma _{{i}}y+\gamma _{i}x-{ \frac{\gamma _{{i}}\left(\alpha \, \gamma _{{i}}\omega _{{\ 2}}-\alpha _{{i} }\omega _{{1}}-\omega _{{3}}\gamma _{{i}}\right) }{\alpha \, \gamma _{{i} }\lambda _{{2}}-\alpha _{{i}}\lambda _{{1}}-\gamma _{{i}}\lambda _{{3}}} \ \ } t, i = 1, 2, 3$ and $\Psi = \Psi (x, y, z, t)$ .

4. Novel periodic wave solutions

The triangular periodic waves for Eq (1.8) can be assumed as below:

$\begin{equation} \mathbf{f} = \exp (\tau _{1})+a_{16}\exp (-\tau _{1})+\cosh (\tau _{2})+\cos (\tau _{3})+a_{17}, \ \ \ \tau _{1} = \sum\limits_{i = 1}^{4}a_{i}x_{i}+a_{5}, \ \ \ \tau _{2} = \sum\limits_{i = 6}^{9}a_{i}x_{i-5}+a_{10}, \ \ \ \end{equation}$

(4.1)

$\begin{equation} \tau _{3} = \sum\limits_{i = 11}^{14}a_{i}x_{i-10}+a_{15}, \ \ (x_{1}, x_{2}, x_{3}, x_{4}) = (\mathbf{x}, t) = (x, y, z, t), \ \ \ \Psi (\mathbf{x} , t) = v_{0}+2\ln (\mathbf{f})_{x}, \end{equation}$

(4.2)

in which $a_{i}, i = 1, ..., 17$ are unfound values. Substituting (4.1) and (4.2) into Eq (1.8) the below consequences will be gained:

Case I:

$\begin{eqnarray} \mathbf{f} & = &{\mathrm{e}^{a_{{4}}t+a_{{1}}x- \ \ {\frac{y\left( a_{{3}}a_{{14} }\omega _{{3}}-a_{{4}}a_{{12}}\omega _{{2}}-a_{{4}}a_{{13}}\omega _{{3} }\right) }{a_{{14}}\omega _{{2}}} \ \ }+a_{{3}}z+a_{{5}}} \ \ }+\cosh \left( a_{{9}}t-{ \frac{y\left( a_{{8}}a_{{14}}\omega _{{3}}-a_{{9}}a_{{12}}\omega _{{2}}-a_{{ \ 9}}a_{{13}}\omega _{{3}}\right) }{a_{{14}}\omega _{{2}}} \ \ }+a_{{8}}z+a_{{\ 10 }}\right) \\ &&+\cos \left( ta_{{14}}+ya_{{12}}+za_{{13}}+a_{{15}}\right) . \end{eqnarray}$

(4.3)

Appending (4.3) into (4.1) and (4.2), the soliton-periodic wave solution of Eq (1.8) as below will be achieved:

$\begin{equation} \Psi _{1} = v_{{0}}+\frac{2a_{{1}}{\mathrm{e}^{a_{{4}}t+a_{{1}}x- \ \ {\frac{ y\left( a_{{3}}a_{{14}}\omega _{{3}}-a_{{4}}a_{{12}}\omega _{{2}}-a_{{4}}a_{{ 13}}\omega _{{3}}\right) }{a_{{14}}\omega _{{2}}} \ \ }+a_{{3}}z+a_{{5}}} \ \ }}{ \mathbf{f}}. \end{equation}$

(4.4)

By selecting the suitable values of parameters including

$\begin{eqnarray*} a_{1} & = &1, a_{3} = 1.5, a_{4} = 2, a_{5} = 1.5, a_{8} = 2, a_{9} = 1.5, a_{10} = 1, \\ a_{12} & = &2, a_{13} = 2.5, a_{14} = 1, a_{15} = 3.2, \omega _{2} = 1.5, \omega _{3} = 1.2, \end{eqnarray*}$

the graphical display of soliton-periodic wave solution is offered in Figure 1 such as 3D plot and density plot.

Figure 1. The soliton-periodic solution (4.4) at (f1, f2)

$x = -2, y = -2$ , (f3, f4)

$x = 0, y = 0$ , and (f5, f6)

$x = 2, y = 2$ .

DownLoad: Full-Size Img PowerPoint

Case II:

$\begin{equation} \mathbf{f} = {\mathrm{e}^{a_{{4}}t+a_{{1}}x+ya_{{2}}- \ \ {\frac { \left( {a_{{1}}} \ \ ^{3}a_{{\ 14}}-a_{{4}}a_{{13}}\omega_{{2}} \right) z}{a_{{14}}\omega_{{2}}} \ \ } +a_{ {5}}} \ \ }+\cosh \left( a_{{9}}t+ya_{{7}}+ \ \ {\frac {a_{{9}}a_{{13}}z}{a_{{14 } }}} \ \ +a_{{10}} \right) +\cos \left( ta_{{14}}+ya_{{12}}+za_{{13}}+a_{{\ 15}} \right). \end{equation}$

(4.5)

Plugging (4.5) into (4.1) and (4.2), obtain a soliton-periodic wave solution of Eq (1.8) as below case:

$\begin{equation} \Psi_2 = v_{{0}}+\frac{2a_{{1}}{\mathrm{e}^{a_{{4}}t+a_{{1}}x+ya_{{2}}- \ \ {\frac { \left( {a_{{1}}} ^ {3}a_{{14}}-a_{{4}}a_{{13}}\omega_{{2}} \right) z}{a_{{14} }\omega_{{2} }}} \ \ +a_{{5}}} \ \ } }{\mathbf{f}} . \end{equation}$

(4.6)

Case III:

$\begin{equation} \mathbf{f} = {\mathrm{e}^{a_{{4}}t+a_{{1}}x- \ \ {\frac {y \left( a_{{3}}\omega_{{3} }+a_{{4 }}\lambda_{{3}} \right) }{\omega_{{2}}} \ \ }+a_{{3}}z+a_{{5}}} \ \ }+\cosh \left( a_{{9}}t- \ \ {\frac {y \left( a_{{8}}\omega_{{3}}+a_{{9}}\lambda_{ {3}} \right) }{\omega_{{2}}} \ \ }+a_{{8}}z+a_{{10}} \right) +\cos \left( - \ \ {\frac { a_{ {13}}\omega_{{3}}y}{\omega_{{2}}} \ \ }+za_{{13}}+a_{{15}} \right). \end{equation}$

(4.7)

Incorporating (4.7) into (4.1) and (4.2), the soliton-periodic wave solution of Eq (1.8) will be gained as below:

$\begin{equation} \Psi_3 = v_{{0}}+\frac{2a_{{1}}{\mathrm{e}^{a_{{4}}t+a_{{1}}x- \ \ {\frac {y \left( a_{{3}}\omega_{{3} }+a_{{4}}\lambda_{{3}} \right) }{\omega_{{2}}} \ \ }+a_{{3} }z+a_{{5}}} \ \ } }{\mathbf{f}} . \end{equation}$

(4.8)

Case IV:

$\begin{equation} \mathbf{f} = {\mathrm{e}^{a_{{4}}t- \ \ {\frac {y \left( a_{{3}}a_{{9}}\omega_{{3} }-a_{{4}} a_{{7}}\omega_{{2}}-a_{{4}}a_{{8}}\omega_{{3}} \right) }{a_{{9} }\omega _{{2}}} \ \ }+a_{{3}}z+a_{{5}}} \ \ }+\cosh \left( a_{{9}}t+ya_{{7}}+a_{{8} }z+a_ {{10}} \right) +\cos \left( xa_{{11}}+a_{{15}} \right) . \end{equation}$

(4.9)

Plugging (4.9) into (4.1) and (4.2), the soliton-periodic wave solution of Eq (1.8) will be achieved as below:

$\begin{equation} \Psi_4 = v_{{0}}-\frac{2\sin \left( xa_{{11}}+a_{{15}} \right) a_{{11}} }{ \mathbf{f}}. \end{equation}$

(4.10)

Case V:

$\begin{equation} \mathbf{f} = {\mathrm{e}^{a_{{4}}t+ya_{{2}}+ \ \ {\frac {a_{{4}}a_{{8}}z}{a_{{9}}} \ \ } +a_{{5}} }}+\cosh \left( a_{{9}}t+ya_{{7}}+a_{{8}}z+a_{{10}} \right) +\cos \left( xa_{{11}}- \ \ {\frac { \left( {a_{{11}}} ^{2}\omega_{{3}}+\omega_{{\ 1} }\omega_{{2}} \right) a_{{11}}y}{{\omega_{{2}}} ^{2}}} \ \ + \ \ {\frac {{a_{{\ 11}}} \ \ ^{3}z}{\omega_{{2}}} \ \ }+a_{{15}} \right). \end{equation}$

(4.11)

Incorporating (4.11) into (4.1) and (4.2), we capture a soliton-periodic wave solution of Eq (1.8) as below:

$\begin{equation} \Psi_5 = v_{{0}}-\frac{2\sin \left( xa_{{11}}- \ \ {\frac { \left( {a_{{11}}} \ \ ^{2}\omega_{{3}}+ \omega_{{1}}\omega_{{2}} \right) a_{{11}}y}{{\omega_{{2}}} \ \ ^{2}}} \ \ +{\ \frac {{a_{{11}}} ^{3}z}{\omega_{{2}}} \ \ }+a_{{15}} \right) a_{{11}} }{ \mathbf{f}}. \end{equation}$

(4.12)

Case VI:

$\begin{eqnarray} \mathbf{f} & = &{\mathrm{e}^{a_{{4}}t+ \ \ {\frac{\omega _{{2}}\left( a_{{3}}a_{{9} }-a_{{4}}a_{{8}}\right) x}{a_{{9}}{a_{{11}}} ^{2}}} \ \ -\frac{1}{3}\, \ \ {\frac{ y\left( -2\, \alpha \, a_{{9}}{a_{{11}}} ^{6}\omega _{{2}}+2\, a_{{9}}{a_{{11}}} \ \ ^{6}\omega _{{3}}+3\, \alpha \, {a_{{3}}} ^{2}a_{{9}}{\omega _{{2}}} \ \ ^{3}-3\, \alpha \, a_{{3}}a_{{4}}a_{{8}}{\omega _{{2}}} ^{3}+2\, a_{{9}}{a_{{11}} }^{4}\omega _{{1}}\omega _{{2}}\right) }{{\omega _{{2}}} ^{3}\left( a_{{3}}a_{ {9}}-a_{{4}}a_{{8}}\right) }} \ \ +a_{{3}}z+a_{{5}}} }+ \\ &&\cosh \left( a_{{9}}t-\frac{1}{3} \ \ {\frac{y\Omega }{a_{{4}}{\omega _{{2}}} \ \ ^{3}\left( a_{{3}}a_{{9}}-a_{{4}}a_{{8}}\right) \left( {a_{{9}}} ^{2}{a_{{11}} }^{6}+{\omega _{{2}}} ^{2}\left( a_{{3}}a_{{9}}-a_{{4}}a_{{8}}\right) ^{2}\right) }}+a_{{8}}z+a_{{10}}\right) \\ &&+\cos \left( xa_{{11}}- \ \ {\frac{\alpha {a_{{11}}} ^{3}y}{\omega _{{2}}} \ \ }+{ \frac{{a_{{11}}} ^{3}z}{\omega _{{2}}} \ \ }+a_{{15}}\right) , \end{eqnarray}$

(4.13)

$\begin{eqnarray*} \Omega & = &3\, \alpha \, a_{{4}}a_{{8}}{\omega _{{2}}} ^{5}\left( a_{{3}}a_{{9} }-a_{{4}}a_{{8}}\right) ^{3}+\alpha \, {a_{{9}}} ^{2}{a_{{11}}} ^{6}{\omega _{{2 }}} ^{3}\left( a_{{3}}a_{{9}}+2\, a_{{4}}a_{{8}}\right) \left( a_{{3}}a_{{9} }-a_{{4}}a_{{8}}\right) \\ &&-{a_{{9}}} ^{2}{a_{{11}}} ^{4}{\omega _{{2}}} ^{2}\left( a_{{3}}a_{{9}}-a_{{4} }a_{{8}}\right) ^{2}\left( {a_{{11}}} ^{2}\omega _{{3}}+\omega _{{1}}\omega _{ {2}}\right) -{a_{{9}}} ^{2}{a_{{11}}} ^{12}\left( 3\, {a_{{4}}} ^{2}-{a_{{9}}} \ \ ^{2}\right) \left( \alpha \, \omega _{{2}}-\omega _{{3}}\right) . \end{eqnarray*}$

Appending (4.13) into (4.1) and (4.2), the soliton-periodic wave solution of Eq (1.8) will be obtained as below:

$\begin{eqnarray} \Psi _{5} & = &v_{{0}}+\frac{2}{\mathbf{f}}\left[ \ \ {\frac{\omega _{{2}}\left( a_{{3}}a_{{9}}-a_{{4}}a_{{8}}\right) }{a_{{9}}{a_{{11}}} ^{2}}{\mathrm{e}^{a_{ {4}}t+ \ \ {\frac{\omega _{{2}}\left( a_{{3}}a_{{9}}-a_{{4}}a_{{8}}\right) x}{a_{{ 9}}{a_{{11}}} ^{2}}} \ \ -\frac{1}{3}\, \ \ {\frac{y\left( -2\, \alpha \, a_{{9}}{a_{{11}} }^{6}\omega _{{2}}+2\, a_{{9}}{a_{{11}}} ^{6}\omega _{{3}}+3\, \alpha \, {a_{{3}} }^{2}a_{{9}}{\omega _{{2}}} ^{3}-3\, \alpha \, a_{{3}}a_{{4}}a_{{8}}{\omega _{{2 }}} ^{3}+2\, a_{{9}}{a_{{11}}} ^{4}\omega _{{1}}\omega _{{2}}\right) }{{\omega _{{2}}} ^{3}\left( a_{{3}}a_{{9}}-a_{{4}}a_{{8}}\right) }}+a_{{3}}z+a_{{5}}} \ \ }} \right. \\ &&\left. +\sin \left( -xa_{{11}}+ \ \ {\frac{\alpha \, {a_{{11}}} ^{3}y}{\omega _{{2 }}} \ \ }- \ \ {\frac{{a_{{11}}} ^{3}z}{\omega _{{2}}} \ \ }-a_{{15}}\right) a_{{11}}\right] . \end{eqnarray}$

(4.14)

By selecting suitable values of parameters including

$\begin{eqnarray*} \alpha & = &0.5, a_{3} = 1, a_{4} = 1.5, a_{5} = 2, a_{8} = 2, a_{9} = 1.5, a_{10} = 1, a_{11} = 2, a_{13} = 2.5, a_{14} = 1, \\ a_{15} & = &3.2, \omega _{1} = 1.5, \omega _{2} = 1.2, \omega _{3} = 1.5, \end{eqnarray*}$

the graphical display of soliton-periodic wave solution is offered in Figure 2 such as 3D chart and density chart.

Figure 2. The soliton-periodic solution (4.14) at (f1, f2)

$x = -2, y = -2$ , (f3, f4)

$x = 0, y = 0$ , and (f5, f6)

$x = 2, y = 2$ .

DownLoad: Full-Size Img PowerPoint

Case VII:

$\begin{equation} \mathbf{f} = {\mathrm{e}^{ \ \ {\frac{\Omega \, a_{{9}}t}{{a_{{11}}} ^{3}}} \ \ +a_{{1}}x- \frac{1}{6}\, \ \ {\frac{y\left( -6\, {a_{{1}}} ^{4}{a_{{11}}} ^{5}\omega _{{3}}-3\, { a_{{1}}} ^{4}{a_{{11}}} ^{3}\omega _{{1}}\omega _{{2}}+2\, {a_{{11}}} ^{7}\omega _{{1}}\omega _{{2}}+6\, \Omega \, a_{{1}}a_{{8}}{a_{{11}}} ^{2}\omega _{{2} }\omega _{{3}}+3\, \Omega \, a_{{1}}a_{{8}}\omega _{{1}}{\omega _{{2}}} \ \ ^{2}\right) }{{a_{{11}}} ^{5}{\omega _{{2}}} ^{2}a_{{1}}} \ \ }+ \ \ {\frac{\left( -{a_{{ 1}}} ^{3}{a_{{11}}} ^{3}+\Omega \, a_{{8}}\omega _{{2}}\right) z}{{a_{{11}}} \ \ ^{3}\omega _{{2}}} \ \ }+a_{{5}}} \ \ }+ \end{equation}$

(4.15)

$\cosh \left( a_{{9}}t+ya_{{7}}+a_{{8}}z+a_{{10}}\right) +\cos \left( xa_{{11} }-\frac{1}{2}\, \ \ {\frac{\left( 2\, {a_{{11}}} ^{2}\omega _{{3}}+\omega _{{1} }\omega _{{2}}\right) a_{{11}}y}{{\omega _{{2}}} ^{2}}} \ \ + \ \ {\frac{{a_{{11}}} ^{3}z }{\omega _{{2}}} \ \ }+a_{{15}}\right) ,$

$\Omega = \sqrt{-{a_{{1}}} ^{6}-2\, {a_{{1}}} ^{4}{a_{{11}}} ^{2}+{a_{{11}}} ^{6}}.$

Incorporating (4.15) into (4.1) and (4.2), the soliton-periodic wave solution of Eq (1.8) will be received as below:

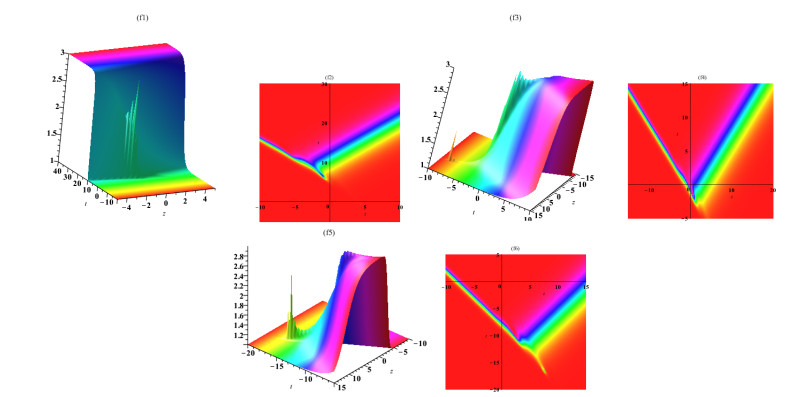
$\begin{eqnarray} \Psi _{5} & = &v_{{0}}+\frac{2}{\mathbf{f}}\left[ a_{{1}}{\mathrm{e}^{ \ \ {\frac{ \Omega \, a_{{9}}t}{{a_{{11}}} ^{3}}} \ \ +a_{{1}}x-\frac{1}{6}\, \ \ {\frac{y\left( -6\, {a_{{1}}} ^{4}{a_{{11}}} ^{5}\omega _{{3}}-3\, {a_{{1}}} ^{4}{a_{{11}}} \ \ ^{3}\omega _{{1}}\omega _{{2}}+2\, {a_{{11}}} ^{7}\omega _{{1}}\omega _{{2} }+6\, \Omega \, a_{{1}}a_{{8}}{a_{{11}}} ^{2}\omega _{{2}}\omega _{{3} }+3\, \Omega \, a_{{1}}a_{{8}}\omega _{{1}}{\omega _{{2}}} ^{2}\right) }{{a_{{11 }}} ^{5}{\omega _{{2}}} ^{2}a_{{1}}} \ \ }+ \ \ {\frac{\left( -{a_{{1}}} ^{3}{a_{{11}}} \ \ ^{3}+\Omega \, a_{{8}}\omega _{{2}}\right) z}{{a_{{11}}} ^{3}\omega _{{2}}} \ \ } +a_{{5}}} \ \ }\right. \\ &&\left. -\sin \left( xa_{{11}}-\frac{1}{2}\, \ \ {\frac{\left( 2\, {a_{{11}}} \ \ ^{2}\omega _{{3}}+\omega _{{1}}\omega _{{2}}\right) a_{{11}}y}{{\omega _{{2}} }^{2}}} \ \ + \ \ {\frac{{a_{{11}}} ^{3}z}{\omega _{{2}}} \ \ }+a_{{15}}\right) a_{{11}} \right] . \end{eqnarray}$

(4.16)

By selecting the specific amounts of parameters including

$\begin{eqnarray*} \alpha & = &0.5, a_{1} = 1, a_{4} = a_{9} = \omega _{1} = \omega _{3} = 1.5, , a_{5} = 2, a_{7} = 1.5, a_{8} = 2, \\ a_{10} & = &1, a_{11} = 2, a_{13} = 2.5, a_{14} = 1, a_{15} = 3.2, \omega _{2} = 1.2, \end{eqnarray*}$

the graphical display of soliton-periodic wave solution is offered in Figure 3 such as 3D chart, density chart, and 2D chart and below cases:

$(f3)\ y = 1, 2, 3, \ \ (f6)\ y = 1, 2, 3, \ and\ \ (f9)\ y = -1, -2, -3.$

Figure 3. The soliton-periodic solution (4.16) at (f1, f2)

$z = -2, t = -2$ , (f4, f5)

$z = 0, t = 0$ , and (f7, f8)

$z = 2, t = 2$ .

DownLoad: Full-Size Img PowerPoint

5. Novel cross-kink wave solutions

Three function containing exponential, hyperbolic, and triangular periodic waves for Eq (1.8) can be assumed as the following:

$\begin{equation} \mathbf{f} = \exp(\tau_1)+a_{16}\exp(-\tau_1)+\sinh(\tau_2)+\sin( \tau_3)+a_{17}, \ \ \ \tau_1 = \sum\limits_{i = 1}^{4}a_ix_i+a_5, \ \ \ \tau_2 = \sum\limits_{i = 6}^{9}a_ix_{i-5}+a_{10}, \end{equation}$

(5.1)

$\begin{equation} \tau_3 = \sum\limits_{i = 11}^{14}a_ix_{i-10}+a_{15}, \ \ (x_1, x_2, x_3, x_4) = (\mathbf{x} , t) = (x, y, z, t), \ \ \ \Psi(\mathbf{x}, t) = v_0+2\ln(\mathbf{f})_{x}, \end{equation}$

(5.2)

in which $a_{i}, i = 1, ..., 17$ are unfound values. Substituting (5.2) into Eq (1.8) the below consequences will be gained:

Case I:

$\begin{equation} \mathbf{f} = {\mathrm{e}^{a_{{4}}t+a_{{1}}x- \ \ {\frac{\left( a_{{3}}a_{{14} }\omega _{{3}}-a_{{4}}a_{{12}}\omega _{{2}}-a_{{4}}a_{{13}}\omega _{{3} }\right) y}{a_{{14}}\omega _{{2}}} \ \ }+a_{{3}}z+a_{{5}}} \ \ }+\sinh \left( a_{{9}}t- \ \ {\frac{\left( a_{{8}}a_{{14}}\omega _{{3}}-a_{{9}}a_{{12}}\omega _{{2}}-a_{{9 }}a_{{13}}\omega _{{3}}\right) y}{a_{{14}}\omega _{{2}}} \ \ }+a_{{8}}z+a_{{10} }\right) \end{equation}$

(5.3)

$+\sin \left( ta_{{14}}+ya_{{12}}+za_{{13}}+a_{{15}}\right) .$

Substituting (5.3) into (5.1) and (5.2), the cross-kink solution of Eq (1.8) will be gained as the following:

$\begin{equation} \Psi _{1} = v_{{0}}+\frac{2a_{{1}}{\mathrm{e}^{a_{{4}}t+a_{{1}}x- \ \ {\frac{\left( a_{{3}}a_{{14}}\omega _{{3}}-a_{{4}}a_{{12}}\omega _{{2}}-a_{{4}}a_{{13} }\omega _{{3}}\right) y}{a_{{14}}\omega _{{2}}} \ \ }+a_{{3}}z+a_{{5}}} \ \ }}{\mathbf{ f}}. \end{equation}$

(5.4)

By selecting the suitable values of parameters including

$\begin{eqnarray*} a_{1} & = &1, a_{3} = 3, a_{4} = 2, a_{5} = 1.5, a_{8} = 1.7, a_{9} = 1.5, a_{10} = 1.5, \\ a_{12} & = &2.5, a_{13} = 1.1, a_{14} = 2.1, a_{15} = 3.2, \omega _{2} = 1, \omega _{3} = 1.5, \end{eqnarray*}$

the graphical representation of cross-kink wave solution is offered in Figure 4 such 3D plot and density plot.

Figure 4. The cross-kink wave solution (5.4) at (f1, f2)

$x = 3, y = 2$ , (f3, f4)

$x = 0, y = 2$ , and (f5, f6)

$x = -3, y = 2$ .

DownLoad: Full-Size Img PowerPoint

Case II:

$\begin{equation} \mathbf{f} = {\mathrm{e}^{a_{{4}}t+a_{{1}}x+a_{{2}}y- \ \ {\frac{\left( {a_{{1}}} \ \ ^{3}a_{{14}}-a_{{4}}a_{{13}}\omega _{{2}}\right) z}{a_{{14}}\omega _{{2}}} \ \ } +a_{{5}}} \ \ }+\sinh \left( a_{{9}}t+a_{{7}}y+ \ \ {\frac{a_{{9}}a_{{13}}z}{a_{{14}}} \ \ } +a_{{10}}\right) +\sin \left( ta_{{14}}+ya_{{12}}+za_{{13}}+a_{{15}}\right) . \end{equation}$

(5.5)

Putting (5.5) into (5.1) and (5.2), the cross-kink solution of Eq (1.8) will be received as the following:

$\begin{equation} \Psi _{2} = v_{{0}}+\frac{2a_{{1}}{\mathrm{e}^{a_{{4}}t+a_{{1}}x+a_{{2}}y-{ \frac{\left( {a_{{1}}} ^{3}a_{{14}}-a_{{4}}a_{{13}}\omega _{{2}}\right) z}{a_{ {14}}\omega _{{2}}} \ \ }+a_{{5}}} \ \ }}{\mathbf{f}}. \end{equation}$

(5.6)

By selecting the suitable values of parameters including

the graphical exhibition of cross-kink solution is offered in Figure 5 such as 3D chart and density chart.

Figure 5. The cross-kink wave solution (5.6) at (f1, f2)

$x = 3, y = 2$ , (f3, f4)

$x = 0, y = 2$ , and (f5, f6)

$x = -3, y = 2$ .

DownLoad: Full-Size Img PowerPoint

Case III:

$\begin{equation} \mathbf{f} = {\mathrm{e}^{a_{{4}}t+a_{{1}}x- \ \ {\frac { \left( a_{{3}}\omega_{{3} }+a_{{4} }\lambda_{{3}} \right) y}{\omega_{{2}}} \ \ }+a_{{3}}z+a_{{5}}} \ \ }+\sinh \left( a_{{9}}t- \ \ {\frac { \left( a_{{8}}\omega_{{3}}+a_{{9}}\lambda_{{\ 3}} \right) y}{\omega_{{2}}} \ \ }+a_{{8}}z+a_{{10}} \right) +\sin \left( - \ \ {\frac { a_{{13}}\omega_{{3}}y}{\omega_{{2}}} \ \ }+a_{{13}}z+a_{{15}} \right). \end{equation}$

(5.7)

Plugging (5.7) into (5.1) and (5.2), the cross-kink solution of Eq (1.8) will be obtained as the following:

$\begin{equation} \Psi_3 = v_{{0}}+\frac{2a_{{1}}{\mathrm{e}^{a_{{4}}t+a_{{1}}x- \ \ {\frac { \left( a_{{3}}\omega_{{3}} +a_{{4}}\lambda_{{3}} \right) y}{\omega_{{2}}} \ \ }+a_{{3} }z+a_{{5}}} \ \ } }{\mathbf{f}} . \end{equation}$

(5.8)

Case IV:

$\begin{eqnarray} \mathbf{f} & = &{\mathrm{e}^{- \ \ {\frac{a_{{9}}\left( {a_{{11}}} ^{2}\left( \omega _{{3}}{a_{{1}}} ^{2}-\omega _{{1}}\omega _{{2}}\right) \left( {a_{{1}}} \ \ ^{3}+a_{{3}}\omega _{{2}}\right) -{a_{{1}}} ^{5}\omega _{{1}}\omega _{{2} }\right) t}{{a_{{1}}} ^{2}a_{{7}}{a_{{11}}} ^{2}{\omega _{{2}}} ^{2}}} \ \ +a_{{1}}x- \ \ {\frac{a_{{3}}\left( \omega _{{3}}{a_{{1}}} ^{2}-\omega _{{1}}\omega _{{2} }\right) y}{{a_{{1}}} ^{2}\omega _{{2}}} \ \ }+a_{{3}}z+a_{{5}}} \ \ }+\sin \left( xa_{{ 11}}+a_{{15}}\right) \\ &&+\sinh \left( a_{{9}}t+a_{{7}}y- \ \ {\frac{\left( {a_{{1}}} ^{3}+a_{{3}}\omega _{{2}}\right) {a_{{1}}} ^{2}a_{{7}}{a_{{11}}} ^{2}\omega _{{2}}z}{{a_{{11}}} \ \ ^{2}\left( \omega _{{3}}{a_{{1}}} ^{2}-\omega _{{1}}\omega _{{2}}\right) \left( {a_{{1}}} ^{3}+a_{{3}}\omega _{{2}}\right) -{a_{{1}}} ^{5}\omega _{{1} }\omega _{{2}}} \ \ }+a_{{10}}\right) . \end{eqnarray}$

(5.9)

Inserting (5.9) into (5.1) and (5.2), the cross-kink solution of Eq (1.8) will be gained as the following:

$\begin{equation} \Psi _{4} = v_{{0}}+2\frac{a_{{1}}{\mathrm{e}^{- \ \ {\frac{a_{{9}}\left( {a_{{11}}} \ \ ^{2}\left( \omega _{{3}}{a_{{1}}} ^{2}-\omega _{{1}}\omega _{{2}}\right) \left( {a_{{1}}} ^{3}+a_{{3}}\omega _{{2}}\right) -{a_{{1}}} ^{5}\omega _{{1} }\omega _{{2}}\right) t}{{a_{{1}}} ^{2}a_{{7}}{a_{{11}}} ^{2}{\omega _{{2}}} \ \ ^{2}}} \ \ +a_{{1}}x- \ \ {\frac{a_{{3}}\left( \omega _{{3}}{a_{{1}}} ^{2}-\omega _{{1} }\omega _{{2}}\right) y}{{a_{{1}}} ^{2}\omega _{{2}}} \ \ }+a_{{3}}z+a_{{5}}} \ \ } +\cos \left( xa_{{11}}+a_{{15}}\right) a_{{11}}} \ \ {\mathbf{f}}. \end{equation}$

(5.10)

Case V:

$\begin{equation} \mathbf{f} = {\mathrm{e}^{a_{{4}}t+a_{{2}}y+ \ \ {\frac{a_{{4}}a_{{8}}z}{a_{{9}}} \ \ } +a_{{5}}} \ \ }+\sinh \left( a_{{9}}t+a_{{7}}y+a_{{8}}z+a_{{10}}\right) +\sin \left( xa_{{11}}- \ \ {\frac{a_{{11}}\left( {a_{{11}}} ^{2}\omega _{{3}}+\omega _{{ 1}}\omega _{{2}}\right) y}{{\omega _{{2}}} ^{2}}} \ \ + \ \ {\frac{{a_{{11}}} ^{3}z}{ \omega _{{2}}} \ \ }+a_{{15}}\right) . \end{equation}$

(5.11)

Substituting (5.11) into (5.1) and (5.2), the cross-kink solution of Eq (1.8) will be received as the following:

$\begin{equation} \Psi _{5} = v_{{0}}+\frac{2\cos \left( xa_{{11}}- \ \ {\frac{a_{{11}}\left( {a_{{11} }}^{2}\omega _{{3}}+\omega _{{1}}\omega _{{2}}\right) y}{{\omega _{{2}}} ^{2}} }+ \ \ {\frac{{a_{{11}}} ^{3}z}{\omega _{{2}}} \ \ }+a_{{15}}\right) a_{{11}}} \ \ {\mathbf{f }}. \end{equation}$

(5.12)

By selecting the suitable values of parameters including

the graphical exhibition of cross-kink solution is offered in Figure 6 such as 3D chart and density chart.

Figure 6. The cross-kink wave solution (5.6) at (f1, f2)

$x = 3, y = 2$ , (f3, f4)

$x = 0, y = 2$ , and (f5, f6)

$x = -3, y = 2$ .

DownLoad: Full-Size Img PowerPoint

Case VI:

$\begin{eqnarray} \mathbf{f} & = &{\mathrm{e}^{ \ \ {\frac{\Omega \, a_{{9}}t}{{a_{{11}}} ^{3}}} \ \ +a_{{1} }x-\frac{1}{12} \ \ {\frac{\left( 3\, \Omega \, a_{{1}}a_{{8}}\omega _{{2}}\left( 4\, {a_{{11}}} ^{2}\omega _{{3}}+3\, \omega _{{1}}\omega _{{2}}\right) -12\, {a_{ {1}}} ^{4}{a_{{11}}} ^{5}\omega _{{3}}-{a_{{11}}} ^{3}\omega _{{1}}\omega _{{2} }\left( 9\, {a_{{1}}} ^{4}-2\, {a_{{11}}} ^{2}{a_{{1}}} ^{2}-2\, {a_{{11}}} \ \ ^{4}\right) \right) y}{{a_{{11}}} ^{5}{\omega _{{2}}} ^{2}a_{{1}}} \ \ } + \ \ {\frac{ \left( -{a_{{1}}} ^{3}{a_{{11}}} ^{3}+\Omega \, a_{{8}}\omega _{{2}}\right) z}{ \omega _{{2}}{a_{{11}}} ^{3}}} \ \ +a_{{5}}} \ \ } \\ &&\sinh \left( a_{{9}}t+a_7y+a_{{8}}z+a_{{10}}\right) +\sin \left( xa_{{ 11}} \! - \! \frac{1}{12} \ \ {\frac{a_{11}\left( 12{a_{{11}}} ^{2}\omega _{{3} } \! + \! 7\, \omega _1\omega _2\right) y}{{\omega _{{2}}} ^{2}}} \ \ + \ \ {\frac{a_{11}^3z}\omega _2} \! + \! a_{15}\right), \end{eqnarray}$

(5.13)

$\Omega = \sqrt{-3\, {a_{{1}}} ^{6}-4\, {a_{{1}}} ^{4}{a_{{11}}} ^{2}+{a_{{11}}} ^{6} }.$

Putting (5.13) into (5.1) and (5.2), the cross-kink solution of Eq (1.8) will be concluded as the following:

$\begin{eqnarray} \Psi _{5} & = &v_{{0}}+\frac{2}{\mathbf{f}}\left[ a_{{1}}{\mathrm{e}^{ \ \ {\frac{ \Omega \, a_{{9}}t}{{a_{{11}}} ^{3}}} \ \ +a_{{1}}x-\frac{1}{12} \ \ {\frac{\left( 3\, \Omega \, a_{{1}}a_{{8}}\omega _{{2}}\left( 4\, {a_{{11}}} ^{2}\omega _{{3} }+3\, \omega _{{1}}\omega _{{2}}\right) -12\, {a_{{1}}} ^{4}{a_{{11}}} \ \ ^{5}\omega _{{3}}-{a_{{11}}} ^{3}\omega _{{1}}\omega _{{2}}\left( 9\, {a_{{1}}} \ \ ^{4}-2\, {a_{{11}}} ^{2}{a_{{1}}} ^{2}-2\, {a_{{11}}} ^{4}\right) \right) y}{{a_{{ 11}}} ^{5}{\omega _{{2}}} ^{2}a_{{1}}} \ \ }+ \ \ {\frac{\left( -{a_{{1}}} ^{3}{a_{{11}}} \ \ ^{3}+\Omega \, a_{{8}}\omega _{{2}}\right) z}{\omega _{{2}}{a_{{11}}} ^{3}}} \ \ +a_{{5}}} \ \ }\right. \\ &&+\left. \cos \left( xa_{{11}}-\frac{1}{12} \ \ {\frac{a_{{11}}\left( 12\, {a_{{11} }}^{2}\omega _{{3}}+7\, \omega _{{1}}\omega _{{2}}\right) y}{{\omega _{{2}}} \ \ ^{2}}} \ \ + \ \ {\frac{{a_{{11}}} ^{3}z}{\omega _{{2}}} \ \ }+a_{{15}}\right) a_{{11}} \right] . \end{eqnarray}$

(5.14)

By selecting suitable values of parameters including

$\begin{eqnarray*} \alpha & = &0.5, a_{3} = 1, a_{4} = a_{9} = \omega _{1} = \omega _{3} = 1.5, a_{5} = 2, a_{8} = 2, \\ a_{10} & = &1, a_{11} = 2, a_{13} = 2.5, a_{14} = 1, a_{15} = 3.2, \omega _{2} = 1.2, \end{eqnarray*}$

the graphical representation of cross-kink solution is offered in Figure 7 such as 3D chart and density chart.

Figure 7. The cross-kink wave solution (5.14) at (f1, f2)

$x = -2, y = -2$ , (f3, f4)

$x = 0, y = 0$ , and (f5, f6)

$x = 2, y = 2$ .

DownLoad: Full-Size Img PowerPoint

Case VII:

$\begin{eqnarray} \mathbf{f} & = &{\mathrm{e}^{ \ \ {\frac{\Omega a_{{9}}t}{{a_{{11}}} ^{3}}} \ \ +a_{{1} }x-\frac{1}{6}{\ \frac{y\left( -6{a_{{1}}} ^{4}{a_{{11}}} ^{5}\omega _{{3} }-3\, {a_{{1}}} ^{4}{a_{{11}}} ^{3}\omega _{{1}}\omega _{{2}}+2\, {a_{{11}}} \ \ ^{7}\omega _{{1}}\omega _{{2}}+6\, \Omega a_{{1}}a_{{8}}{a_{{11}}} \ \ ^{2}\omega _{{2}}\omega _{{3}}+3\, \Omega \, a_{{1}}a_{{8}}\omega _{{1}}{\ \omega _{{2}}} ^{2}\right) }{{a_{{11}}} ^{5}{\omega _{{2}}} ^{2}a_{{1}}} \ \ }+{\ \frac{\left( -{a_{{1}}} ^{3}{a_{{11}}} ^{3}+\Omega a_{{8}}\omega _{{2} }\right) z}{{a_{{11}}} ^{3}\omega _{{2}}} \ \ }+a_{{5}}} \ \ }+ \\ &&\cosh \left( a_{{9}}t+ya_{{7}}+a_{{8}}z+a_{{10}}\right) + \cos \left( xa_{{\ 11}}-\frac{1}{2} \ \ {\frac{\left( 2{a_{{11}}} ^{2}\omega _{{3}}+\omega _{{1} }\omega _{{2}}\right) a_{{11}}y}{{\omega _{{2}}} ^{2}}} \ \ + \ \ {\frac{{a_{{11}}} ^{3}z }{\omega _{{2}}} \ \ }+a_{{15}}\right), \end{eqnarray}$

(5.15)

$\Omega = \sqrt{-{a_{{1}}} ^{6}-2\, {a_{{1}}} ^{4}{a_{{11}}} ^{2}+{a_{{11}}} ^{6}}.$

Inserting (5.15) into (5.1) and (5.2), the cross-kink solution of Eq (1.8) will be gained as the following:

(5.16)

By selecting suitable values of parameters including

$\alpha = 0.5, a_1 = 1, a_5 = 1.5, a_8 = 2, a_9 = 0.5, a_{10} = 1.5, a_{15} = 3.2, \omega_1 = 1.5, \omega_2 = 1.2, \omega_3 = 1.5,$

the graphical representation of cross-kink solution is offered in Figure 8 such as 3D chart and density chart.

Figure 8. The lump-periodic solution (5.16) at (f1, f2)

$z = -2$ , (f4, f5)

$z = 0$ , and (f7, f8)

$z = 2$ .

DownLoad: Full-Size Img PowerPoint

6. Novel solitary wave solutions

Three function containing exponential, hyperbolic, and triangular periodic waves for Eq (1.8) can be assumed as the following:

$\begin{equation} \textbf{f} = \exp (\tau _{1})+a_{16}\exp (-\tau _{1})+\tanh (\tau _{2})+\tan (\tau _{3})+a_{17}, \ \ \ \tau _{1} = \sum\limits_{i = 1}^{4}a_{i}x_{i}+a_{5}, \ \ \ \tau _{2} = \sum\limits_{i = 6}^{9}a_{i}x_{i-5}+a_{10}, \ \ \ \end{equation}$

(6.1)

(6.2)

in which $a_{i}, i = 1, ..., 17$ are unfound values. Substituting (6.2) into Eq (1.8) the below consequences will be gained:

Case I:

$\begin{equation} \textbf{f} = {\mathrm{e}^{a_{{4}}t+a_{{1}}x+a_{{2}}y- \ \ {\frac{\left( {a_{{1}}} \ \ ^{3}+a_{{4}}\lambda _{{2}}\right) z}{\omega _{{2}}} \ \ }+a_{{5}}} \ \ }+\tanh \left( ya_{{7}}+a_{{10}}\right) +\tan \left( ya_{{12}}+a_{{15}}\right) +a_{{17}}. \end{equation}$

(6.3)

Substituting (6.3) into (6.1) and (6.2), we can capture a solitary wave solution of Eq (1.8) as the following:

$\begin{equation} \Psi _{1} = v_{{0}}+\frac{2\, a_{{1}}{\mathrm{e}^{a_{{4}}t+a_{{1}}x+a_{{2}}y-{ \frac{\left( {a_{{1}}} ^{3}+a_{{4}}\lambda _{{2}}\right) z}{\omega _{{2}}} \ \ } +a_{{5}}} \ \ }}{\mathbf{f}}. \end{equation}$

(6.4)

Case II:

$\begin{equation} \textbf{f} = {\mathrm{e}^{a_{{1}}x+a_{{2}}y- \ \ {\frac{{a_{{1}}} ^{3}z}{\omega _{{2} }}} \ \ +a_{{5}}} \ \ }+\tanh \left( ya_{{7}}+a_{{10}}\right) +\tan \left( ya_{{12} }+a_{{15}}\right) +a_{{17}}. \end{equation}$

(6.5)

Inserting (6.5) into (6.1) and (6.2), we can capture a solitary wave solution of Eq (1.8) as below:

$\begin{equation} \Psi _{2} = v_{{0}}+\frac{2\, a_{{1}}{\mathrm{e}^{a_{{1}}x+a_{{2}}y- \ \ {\frac{{a_{{ 1}}} ^{3}z}{\omega _{{2}}} \ \ }+a_{{5}}} \ \ }}{\mathbf{f}}. \end{equation}$

(6.6)

Case III:

$\begin{equation} \textbf{f} = {\mathrm{e}^{a_{{4}}t+a_{{1}}x+a_{{2}}y- \ \ {\frac{\left( {a_{{1}}} \ \ ^{3}\omega _{{3}}+a_{{4}}\lambda _{{3}}\omega _{{2}}\right) z}{\omega _{{2} }\omega _{{3}}} \ \ }+a_{{5}}} \ \ }+\tanh \left( ta_{{9}}+ya_{{7}}- \ \ {\frac{za_{{9} }\lambda _{{3}}} \ \ {\omega _{{3}}} \ \ }+a_{{10}}\right) +\tan \left( ya_{{12}}+a_{{ 15}}\right) +a_{{17}}. \end{equation}$

(6.7)

Putting (6.7) into (6.1) and (6.2), the solitary wave solution of Eq (1.8) can be indicated as below:

$\begin{equation} \Psi _{3} = v_{{0}}+\frac{2\, a_{{1}}{\mathrm{e}^{a_{{4}}t+a_{{1}}x+a_{{2}}y-{ \frac{\left( {a_{{1}}} ^{3}\omega _{{3}}+a_{{4}}\lambda _{{3}}\omega _{{2} }\right) z}{\omega _{{2}}\omega _{{3}}} \ \ }+a_{{5}}} \ \ }}{\mathbf{f}}. \end{equation}$

(6.8)

Case IV:

$\begin{equation} \textbf{f} = {\mathrm{e}^{a_{{4}}t+a_{{1}}x+a_{{2}}y+za_{{3}}+a_{{5}}} \ \ }-\tanh \left( \ \ {\frac{ya_{{8}}\omega _{{3}}} \ \ {\omega _{{2}}} \ \ }-za_{{8}}-a_{{10} }\right) -\tan \left( \ \ {\frac{ya_{{13}}\omega _{{3}}} \ \ {\omega _{{2}}} \ \ }-a_{{13} }z-a_{{15}}\right) +a_{{17}}. \end{equation}$

(6.9)

Putting (6.9) into (6.1) and (6.2), the solitary wave of Eq (1.8) can be stated as the following:

$\begin{equation} \Psi _{4} = v_{{0}}+\frac{2\, a_{{1}}{\mathrm{e}^{a_{{4}}t+a_{{1}}x+a_{{2} }y+za_{{3}}+a_{{5}}} \ \ }}{\mathbf{f}}. \end{equation}$

(6.10)

By selecting the suitable values of parameters including

$\begin{eqnarray*} a_{1} & = &1, a_{2} = 1.5, a_{3} = 2, a_{4} = 2, a_{5} = 1.5, a_{8} = 2, a_{9} = 1.5, a_{10} = 1.5, \\ a_{13} & = &2, a_{13} = 1.1, a_{15} = 3.2, a_{17} = 2, \omega _{2} = -1.5, \end{eqnarray*}$

$\omega _{3} = 1.2, \lambda _{2} = 1, \lambda _{3} = 1.5, x = -2, t = 2,$

with the following components

$\begin{eqnarray*} \alpha & = & \ \ {\frac{{a_{{1}}} ^{3}\omega _{{3}}-a_{{1}}\omega _{{1}}\omega _{{2} }-a_{{2}}{\omega _{{2}}} ^{2}-a_{{3}}\omega _{{2}}\omega _{{3}}+a_{{4} }\lambda _{{2}}\omega _{{3}}-a_{{4}}\lambda _{{3}}\omega _{{2}}} \ \ {\omega _{{2} }{a_{{1}}} ^{3}}} \ \ , \ \ \\ \lambda _{{1}} & = &- \ \ {\frac{{a_{{1}}} ^{3}a_{{2}}\omega _{{2}}+{a_{{1}}} ^{3}a_{{ 3}}\omega _{{3}}+a_{{2}}a_{{4}}\lambda _{{2}}\omega _{{2}}+a_{{3}}a_{{4} }\lambda _{{2}}\omega _{{3}}} \ \ {a_{{1}}a_{{4}}\omega _{{2}}} }, \end{eqnarray*}$

the graphical representation of rational solitary solution is offered in Figure 9 such as 3D chart and density chart.

Figure 9. The rational solitary wave solution (6.10) at (f1)

$z = 0$ , (f2)

$z = 1$ , and (f3)

$z = 3$ .

DownLoad: Full-Size Img PowerPoint

Case V:

$\begin{equation} \ f = {\mathrm{e}^{a_{{1}}x- \ \ {\frac{a_{{3}}\omega _{{3}}y}{\omega _{{2}}} \ \ }+za_{{3}}+a_{{5}}} \ \ }-\tanh \left( \ \ {\frac{ya_{{8}}\omega _{{3}}} \ \ {\omega _{{2}} }}-za_{{8}}-a_{{10}}\right) -\tan \left( \ \ {\frac{ya_{{13}}\omega _{{3}}} \ \ { \omega _{{2}}} \ \ }-a_{{13}}z-a_{{15}}\right) +a_{{17}}. \end{equation}$

(6.11)

Incorporating (6.11) into (6.1) and (6.2), the solitary solution of Eq (1.8) will be gained as below:

$\begin{equation} \Psi _{5} = v_{{0}}+\frac{2\, a_{{1}}{\mathrm{e}^{a_{{1}}x- \ \ {\frac{a_{{3}}\omega _{{3}}y}{\omega _{{2}}} \ \ }+za_{{3}}+a_{{5}}} \ \ }}{\mathbf{f}}, \ \ \alpha = \ \ {\frac{{ a_{{1}}} ^{2}\omega _{{3}}-\omega _{{1}}\omega _{{2}}} \ \ {{a_{{1}}} ^{2}\omega _{{ 2}}} \ \ }. \end{equation}$

(6.12)

Case VI:

$\begin{equation} \mathbf{f} = {\mathrm{e}^{a_{{4}}t+a_{{1}}x- \ \ {\frac{\left( a_{{3}}\omega _{{3} }+a_{{4}}\lambda _{{3}}\right) y}{\omega _{{2}}} \ \ }+za_{{3}}+a_{{5}}} \ \ }+\tanh \left( ta_{{9}}- \ \ {\frac{y\left( a_{{8}}\omega _{{3}}+a_{{9}}\lambda _{{3} }\right) }{\omega _{{2}}} \ \ }+za_{{8}}+a_{{10}}\right) -\tan \left( {\ \frac{ ya_{{13}}\omega _{{3}}} \ \ {\omega _{{2}}} \ \ }-a_{{13}}z-a_{{15}}\right) +a_{{17}}. \end{equation}$

(6.13)

Appending (6.13) into (6.1) and (6.2), the solitary solution of Eq (1.8) can be written as the following:

$\begin{equation} \Psi _{6} = v_{{0}}+\frac{2\, a_{{1}}{\mathrm{e}^{a_{{4}}t+a_{{1}}x- \ \ {\frac{ \left( a_{{3}}\omega _{{\ 3}}+a_{{4}}\lambda _{{3}}\right) y}{\omega _{{2}}} \ \ } +za_{{3}}+a_{{5}}} \ \ }}{\mathbf{f}}, \ \ \alpha = \ \ {\frac{{a_{{1}}} ^{2}\omega _{{3} }-\omega _{{1}}\omega _{{2}}} \ \ {{a_{{1}}} ^{2}\omega _{{2}}} \ \ }. \end{equation}$

(6.14)

Case VII:

$\begin{equation} \mathbf{f} = {\mathrm{e}^{a_{{4}}t+a_{{1}}x- \ \ {\frac{a_{{3}}\omega _{{3}}y}{ \omega _{{2}}} \ \ }+za_{{3}}+a_{{5}}} \ \ }+\tanh \left( ta_{{9}}- \ \ {\frac{ya_{{8} }\omega _{{3}}} \ \ {\omega _{{2}}} \ \ }+za_{{8}}+a_{{10}}\right) -\tan \left( \ \ {\frac{ ya_{{13}}\omega _{{3}}} \ \ {\omega _{{2}}} \ \ }-a_{{13}}z-a_{{15}}\right) +a_{{17}}. \end{equation}$

(6.15)

Appending (6.15) into (6.1) and (6.2), the solitary solution of Eq (1.8) will be obtained as below:

$\begin{equation} \Psi _{7} = v_{{0}}+\frac{2\, a_{{1}}{\mathrm{e}^{a_{{4}}t+a_{{1}}x- \ \ {\frac{a_{{3 }}\omega _{{3}}y}{\omega _{{2}}} \ \ }+za_{{3}}+a_{{5}}} \ \ }}{\mathbf{f}}, \ \ \alpha = \ \ {\frac{{a_{{1}}} ^{2}\omega _{{3}}-\omega _{{1}}\omega _{{2}}} \ \ {{a_{{1}}} \ \ ^{2}\omega _{{2}}} }, \ \ \lambda _{{2}} = \ \ {\frac{\lambda _{{3}}\omega _{{2}}} \ \ { \omega _{{3}}} \ \ }. \end{equation}$

(6.16)

By choosing the specific amounts of parameters including

$\begin{eqnarray*} a_{1} & = &1, a_{2} = 1.5, a_{3} = 2, a_{4} = 2, a_{5} = 1.5, a_{8} = 2, a_{9} = 1.3, a_{10} = 1.5, \\ a_{13} & = &2, a_{13} = 2, a_{15} = 3.2, a_{17} = 2, \omega _{2} = -1.5, \\ \omega _{3} & = &1.2, \lambda _{2} = 1, \lambda _{3} = 1.5, x = -2, t = 2, \end{eqnarray*}$

the graphical representation of rational solitary solution is offered in Figure 10 such as 3D chart and density chart.

Figure 10. The rational solitary wave solution (6.16) at (f1)

$z = 0$ , (f2)

$z = 1$ , and (f3)

$z = 3$ .

DownLoad: Full-Size Img PowerPoint

Case VIII:

$\begin{equation} \mathbf{f} = {\mathrm{e}^{a_{{4}}t+a_{{1}}x+a_{{2}}y- \ \ {\frac { \left( {a_{{1}}} \ \ ^{3}a_{{\ 14}}-a_{{4}}a_{{13}}\omega_{{2}} \right) z}{a_{{14}}\omega_{{2}}} \ \ } +a_{ {5}}} \ \ }+\tanh \left( ta_{{9}}+ya_{{7}}+ \ \ {\frac {za_{{9}}a_{{13}}} \ \ {a_{{14 } }}} \ \ +a_{{10}} \right) +\tan \left( ta_{{14}}+ya_{{12}}+a_{{13}}z+a_{{\ 15}} \right) +a_{{17}} . \end{equation}$

(6.17)

Incorporating (6.17) into (6.1) and (6.2), the solitary solution of Eq (1.8) will be received as below:

$\begin{equation} \Psi_8 = v_{{0}}+\frac{2\, a_{{1}}{\mathrm{e}^{a_{{4}}t+a_{{1}}x+a_{{2}}y-{ \frac { \left( {a_{{1} }}^{3}a_{{14}}-a_{{4}}a_{{13}}\omega_{{2}} \right) z}{ a_{{14}}\omega_{ {2}}} \ \ }+a_{{5}}} \ \ } }{\mathbf{f}}, \ \ \alpha = \ \ {\frac {{a_{{1}}} \ \ ^{2}\omega_{{3}}-\omega_{{1}}\omega_{{2}}} \ \ {{a_ {{1}}} ^{2}\omega_{{2}}} }, \ \ \lambda_{{2}} = - \ \ {\frac {a_{{13}}\omega_{{2}}} \ \ {a_{{14}}} }, \ \ \lambda_{{3}} = -{ \frac {a_{{13}}\omega_{{3}}} \ \ {a_{{14}}} \ \ }. \end{equation}$

(6.18)

Case IX:

$\begin{eqnarray} \mathbf{f} & = &{\mathrm{e}^{a_{{4}}t+a_{{1}}x- \ \ {\frac{\left( a_{{3}}a_{{14} }\omega _{{3}}-a_{{4}}a_{{12}}\omega _{{2}}-a_{{4}}a_{{13}}\omega _{{3} }\right) y}{a_{{14}}\omega _{{2}}} \ \ }+a_{{3}}z+a_{{5}}} \ \ } \end{eqnarray}$

(6.19)

$\begin{eqnarray} &&+\tanh \left( ta_{{9}}- \ \ {\frac{y\left( a_{{8}}a_{{14}}\omega _{{3}}-a_{{9}}a_{{12}}\omega _{{2}}-a_{{ \ 9}}a_{{13}}\omega _{{3}}\right) }{a_{{14}}\omega _{{2}}} \ \ }+za_{{8}}+a_{{\ 10 }}\right) \end{eqnarray}$

(6.20)

$\begin{eqnarray} &&+\tan \left( ta_{{14}}+ya_{{12}}+za_{{13}}+a_{{15}}\right) +a_{{17}}. \end{eqnarray}$

(6.21)

Appending (6.21) into (6.1) and (6.2), the solitary solution of Eq (1.8) can be reached as below:

$\begin{equation} \Psi _{9} = v_{{0}}+\frac{2a_{{1}}{\mathrm{e}^{a_{{4}}t+a_{{1}}x- \ \ {\frac{\left( a_{{3}}a_{{14}}\omega _{{3}}-a_{{4}}a_{{12}}\omega _{{2}}-a_{{4}}a_{{13} }\omega _{{3}}\right) y}{a_{{14}}\omega _{{2}}} \ \ }+a_{{3}}z+a_{{5}}} \ \ }}{\mathbf{ f}}, \ \alpha = \ \ {\frac{{a_{{1}}} ^{2}\omega _{{3}}-\omega _{{1}}\omega _{{2}}} \ \ {{ a_{{1}}} ^{2}\omega _{{2}}} }, \ \end{equation}$

(6.22)

$\lambda _{{1}} = - \ \ {\frac{{a_{{1}}} ^{2}\left( a_{{12}}\omega _{{2}}+a_{{\ 13} }\omega _{{3}}\right) }{a_{{14}}\omega _{{2}}} }, \ \lambda _{{3}} = - \ \ {\frac{a_{{ 12}}\omega _{{2}}+a_{{13}}\omega _{{3}}} \ \ {a_{{\ 14}}} \ \ }.$

Case X:

$\begin{equation} \mathbf{f} = {\mathrm{e}^{a_{{4}}t+a_{{1}}x- \ \ {\frac {a_{{3}}\omega_{{3}}y}{ \omega_{{2}} }}+a_{{3}}z+a_{{5}}} \ \ }+\tanh \left( - \ \ {\frac {ya_{{8}}\omega_{{3}} }{ \omega_{{2}}} \ \ }+za_{{8}}+a_{{10}} \right) +\tan \left( ta_{{14}}-{\ \frac { ya_{{13}}\omega_{{3}}} \ \ {\omega_{{2}}} \ \ }+za_{{13}}+a_{{15}} \right) +a_{{17}}. \end{equation}$

(6.23)

Inserting (6.21) into (6.1) and (6.2), the solitary solution of Eq (1.8) will be gained as below form:

$\begin{equation} \Psi_{10} = v_{{0}}+\frac{2\, a_{{1}}{\mathrm{e}^{a_{{4}}t+a_{{1}}x- \ \ {\frac {a_{{ 3}}\omega_{{3}}y}{ \omega_{{2}}} \ \ }+a_{{3}}z+a_{{5}}} \ \ } }{\mathbf{f}}, \ \alpha = { \frac {{a_{{1}}} ^{2}\omega_{{3}}-\omega_{{1}}\omega_{{2}}} \ \ {{a_ {{1}}} \ \ ^{2}\omega_{{2}}} \ \ } , \ \lambda_{{2}} = \ \ {\frac {\lambda_{{3}}\omega_{{2}}} \ \ { \omega_{{3}}} \ \ }. \end{equation}$

(6.24)

By choosing the specific amounts of parameters including

$\begin{eqnarray*} a_{1} & = &1, a_{3} = 1.5, a_{4} = 2, a_{5} = 1.5, a_{7} = 2, a_{8} = 2, a_{9} = 2.1, \\ a_{10} & = &1.5, a_{13} = 2, a_{13} = 2, a_{14} = 2.5, a_{15} = 3.2, \\ a_{17} & = &2, \omega _{2} = -1.5, \omega _{3} = 1.2, \lambda _{3} = 1.5, x = -2, t = 2, \end{eqnarray*}$

the graphical representation of rational solitary solution is offered in Figure 11 such as 3D chart and density chart.

Figure 11. The rational solitary wave solution (6.24) at (f1)

$z = -2$ , (f2)

$z = 0$ , and (f3)

$z = 2$ .

DownLoad: Full-Size Img PowerPoint

Case XI:

$\begin{equation} \mathbf{f} = {\mathrm{e}^{a_{{4}}t+a_{{1}}x+a_{{2}}y- \ \ {\frac{\left( {a_{{1}}} \ \ ^{3}a_{{\ 14}}-a_{{4}}a_{{13}}\omega _{{2}}\right) z}{a_{{14}}\omega _{{2}}} \ \ } +a_{{5}}} \ \ }+\tanh \left( \ \ {\frac{ta_{{14}}a_{{8}}} \ \ {a_{{13}}} \ \ }+ya_{{7}}+za_{{8} }+a_{{10}}\right) +\tan \left( ta_{{14}}+za_{{13}}+a_{{15}}\right) +a_{{17}}. \end{equation}$

(6.25)

Inserting (6.25) into (6.1) and (6.2), the solitary solution of Eq (1.8) can be stated as below case:

$\begin{equation} \Psi _{11} = v_{{0}}+\frac{2a_{{1}}{\mathrm{e}^{a_{{4}}t+a_{{1}}x+a_{{2}}y-{ \frac{\left( {a_{{1}}} ^{3}a_{{14}}-a_{{4}}a_{{13}}\omega _{{2}}\right) z}{a_{ {14}}\omega _{{2}}} \ \ }+a_{{5}}} \ \ }}{\mathbf{f}}, \ \alpha = - \ \ {\frac{{a_{{1}}} \ \ ^{3}a_{{2}}+a_{{1}}a_{{3}}\omega _{{1}}+a_{{2}}a_{{3}}\omega _{{2}}+{a_{{3}}} \ \ ^{2}\omega _{{3}}} \ \ {{a_{{1}}} ^{3}a_{{3}}} }, \end{equation}$

(6.26)

$\begin{eqnarray*} \lambda _{{1}} & = & \ \ {\frac{{a_{{1}}} ^{3}a_{{2}}a_{{12}}a_{{13}}-{a_{{1}}} \ \ ^{3}a_{{3}}{a_{{12}}} ^{2}+a_{{2}}a_{{3}}a_{{12}}a_{{13}}\omega _{{2}}+a_{{2} }a_{{3}}{a_{{13}}} ^{2}\omega _{{3}}+a_{{2}}a_{{3}}a_{{13}}a_{{14}}\lambda _{{ 3}}} \ \ {a_{{3}}a_{{12}}a_{{1}}a_{{14}}} \ \ } \\ &&+\frac{-{a_{{3}}} ^{2}{a_{{12}}} ^{2}\omega _{{2}}-{a_{{3}}} ^{2}a_{{12}}a_{{ 13}}\omega _{{3}}-{a_{{3}}} ^{2}a_{{12}}a_{{14}}\lambda _{{3}}} \ \ {a_{{3}}a_{{12} }a_{{1}}a_{{14}}} \ \ , \end{eqnarray*}$

$\lambda _{{2}} = - \ \ {\frac{a_{{13}}\left( a_{{12}}\omega _{{2}}+a_{{13}}\omega _{ {3}}+a_{{14}}\lambda _{{3}}\right) }{a_{{12}}a_{{14}}} \ \ }.$

Case XII:

$\begin{equation} \mathbf{f} = {\mathrm{e}^{a_{{4}}t+a_{{1}}x- \ \ {\frac{a_{{3}}\omega _{{3}}y}{ \omega _{{2}}} \ \ }+a_{{3}}z+a_{{5}}} \ \ }+\tanh \left( ta_{{9}}+ya_{{7}}- \ \ {\frac{za_{ {7}}\omega _{{2}}} \ \ {\omega _{{3}}} \ \ }+a_{{10}}\right) +\tan \left( ta_{{14}}-{\ \frac{ya_{{13}}\omega _{{3}}} \ \ {\omega _{{2}}} \ \ }+za_{{13}}+a_{{15}}\right) +a_{{ 17}}. \end{equation}$

(6.27)

Inserting (6.27) into (6.1) and (6.2), the solitary solution of Eq (1.8) will be gained as below form:

$\begin{equation} \Psi _{12} = v_{{0}}+\frac{2a_{{1}}{\mathrm{e}^{a_{{4}}t+a_{{1}}x- \ \ {\frac{a_{{3} }\omega _{{3}}y}{\omega _{{2}}} \ \ }+a_{{3}}z+a_{{5}}} \ \ }}{\mathbf{f}}, \ \alpha = -{ \frac{{a_{{1}}} ^{3}a_{{2}}+a_{{1}}a_{{3}}\omega _{{1}}+a_{{2}}a_{{3}}\omega _{{2}}+{a_{{3}}} ^{2}\omega _{{3}}} \ \ {{a_{{1}}} ^{3}a_{{3}}} }, \end{equation}$

(6.28)

$\lambda _{{2}} = - \ \ {\frac{a_{{13}}\left( a_{{12}}\omega _{{2}}+a_{{13}}\omega _{ {3}}+a_{{14}}\lambda _{{3}}\right) }{a_{{12}}a_{{14}}} \ \ }.$

7. Stability analysis of Eq (1.8)

In the current section, we will analyze the continuous modulational instability of the nonlinear generalized KP equation. In addition, the feasibility of the localized waves in the present system is certified by linear stability analysis. First, we search the perturbed solution for the giving Eq (1.8) of the form

$\begin{equation} \Psi(x, y, z, t) = \zeta+\delta\ \Theta, \end{equation}$

(7.1)

where $\Theta = \Theta(x, y, z, t)$ and $\zeta$ is a steady state solution. Inserting (7.1) into Eq (1.8), become

$\begin{equation} \delta\, \ \ {\frac {\partial ^{4}}{\partial {x}^{3}\partial y}}\Theta +\alpha\, \delta\, \ \ {\frac {\partial ^{4}}{\partial {x}^{3} \partial z}}\Theta +3\, {\delta}^{2} \left( \ \ {\frac { \partial ^{2}}{\partial {x}^{2}}} \ \ \Theta \right) {\ \frac {\partial }{\partial y}}\Theta + 3\, {\delta}^{2 } \left( { \frac {\partial }{\partial x}}\Theta \right) \ \ {\frac {\partial ^{2}}{\partial x\partial y}}\Theta +3\, \alpha\, {\delta}^{2} \left( \ \ {\frac {\partial ^{2}}{ \partial {x}^{2}}} \ \ \Theta \right) \ \ {\frac {\partial } {\partial z}}\Theta + \end{equation}$

(7.2)

$3\, \alpha\, {\delta}^{2} \left( \ \ {\frac {\partial }{\partial x}}\Theta \right) {\ \frac {\partial ^{2}}{\partial x\partial z}}\Theta + \lambda_{{1}}\delta\, \ \ {\frac {\partial ^{2}}{\partial t\partial x}}\Theta +\lambda_{{2}}\delta\, { \frac {\partial ^{2}}{ \partial t\partial y}}\Theta + \lambda_{{3}}\delta\, { \ \frac {\partial ^{2}}{\partial t\partial z}}\Theta + \omega_{{1}}\delta\, { \frac {\partial ^{2}}{\partial x\partial z}}\Theta +\omega_{{2}}\delta\, { \frac {\partial ^{2}}{ \partial y\partial z}}\Theta +\omega_{{3}}\delta\, {\ \frac {\partial ^{2}}{\partial {z}^{2}}} \ \ \Theta = 0,$

by linerization Eq (7.2), one gets

$\begin{equation} \delta \ \ {\frac {\partial ^{4}}{\partial {x}^{3}\partial y}}\Theta +\alpha\delta \ \ {\frac {\partial ^{4}}{\partial {x}^{3} \partial z}}\Theta +\lambda_{{1} }\delta \ \ {\frac { \partial ^{2}}{\partial t\partial x}}\Theta + \lambda _{{2} }\delta \ \ {\frac {\partial ^{2}}{\partial t\partial y}}\Theta +\lambda_{{3} }\delta \ \ {\frac {\partial ^{2}}{\partial t \partial z}}\Theta +\omega_{{1} }\delta \ \ {\frac { \partial ^{2}}{\partial x\partial z}}\Theta +\omega_ {{2} }\delta \ \ {\frac {\partial ^{2}}{\partial y\partial z}}\Theta +\omega_{{3} }\delta \ \ {\frac {\partial ^{2}}{\partial {z}^ {2}}} \ \ \Theta = 0. \end{equation}$

(7.3)

Theorem 7.1. Assume that the solution of Eq (7.3) has the following case as

$\begin{equation} \Theta(x, y, z, t) = \rho_1\ e^{i(Mx+Ny+Pz+Bt)}, \end{equation}$

(7.4)

in which $M, N, P$ are the normalized wave numbers, by plugging (7.4) into Eq (7.3), separation the coefficients of $e^{i(Mx+Ny+Pz+Wt)}$ one gets

$\begin{equation} B(M, N, P) = \ \ {\frac {{M}^{3}P\alpha+{M}^{3}N-MP\omega_{{1}}-NP\omega_{{2}}-{P} ^{2} \omega_{{3}}} \ \ {M\lambda_{{1}}+N\lambda_{{2}}+P\lambda_{{3}}} \ \ }. \end{equation}$

(7.5)

Proof. By putting (7.4) into (7.3), becomes

$\begin{equation} \delta\, \ \ {\frac {\partial ^{4}}{\partial {x}^{3}\partial y}}\overline{\Theta} +\alpha\, \delta\, \ \ {\frac {\partial ^{4}}{\partial {x}^{3} \partial z}} \overline{\Theta} +\lambda_{{1}}\delta\, \ \ {\frac { \partial ^{2}}{\partial t\partial x}}\overline{\Theta} + \lambda _{{2}}\delta\, \ \ {\frac {\partial ^{2} }{\partial t\partial y}}\overline{\Phi} +\lambda_{{3}}\delta\, \ \ {\frac { \partial ^{2}}{\partial t \partial z}}\overline{\Theta} +\omega_{{1}}\delta\, \ \ {\frac { \partial ^{2}}{\partial x\partial z}}\overline{\Theta} +\omega_ {{2} }\delta\, \ \ {\frac {\partial ^{2}}{\partial y\partial z}}\overline{\Theta} +\omega_{{3}}\delta\, \ \ {\frac {\partial ^{2}}{\partial {z}^ {2}}} \ \ \overline{ \Theta} \end{equation}$

(7.6)

$= {\mathrm{e}^{i \left( Mx+Ny+Pz+Bt \right) }}\delta\, \rho_{{1}} \left( {M} ^{3}P\alpha+{M}^{3}N-MP\omega_{{1}}-MB\lambda_{{1}}-NP\omega_{{2}}-NB \lambda_{{2}}-{P}^{2}\omega_{{3}}-PB\lambda_{{3}} \right),$

in which $\overline{\Theta} = \Theta(x, y, z, t)$ . By solving and simplifying we can determine the function of $B(M, N, P)$ as the following

$\begin{equation} B(M, N, P) = \ \ {\frac {{M}^{3}P\alpha+{M}^{3}N-MP\omega_{{1}}-NP\omega_{{2}}-{P} ^{2} \omega_{{3}}} \ \ {M\lambda_{{1}}+N\lambda_{{2}}+P\lambda_{{3}}} \ \ }. \end{equation}$

(7.7)

Accordingly, the considered solution was obtained. Thereupon the proof is perfect.

It is easy to notice that modulation stability occurs when $M\lambda_{{1} }+N\lambda_{{2}}+P\lambda_{{3}}\neq0$ . So, the modulation stability achieve spectrum $\Upsilon(B)$ will be as below form:

$\begin{equation} \Upsilon(B) = \ \ {\frac {{M}^{3}P\alpha+{M}^{3}N-MP\omega_{{1}}-NP\omega_{{2}}-{P} ^{2} \omega_{{3}}} \ \ {M\lambda_{{1}}+N\lambda_{{2}}+P\lambda_{{3}}} \ \ }. \end{equation}$

(7.8)

In - can be discovered that while the sign of $B(M, N, P)$ is positive for all quantity of $M$ . Furthermore, in can be observed if the $B(M, N, P)$ is positive or negative for some quantities of $M$ . Finally, in and can be perceived that while the sign of $B(M, N, P)$ is positive for all quantity of $M$ .

Figure 12. The graphic representation

$\Upsilon(B)$ for the wave number

$M$ via considering the diverse quantities

$\lambda_1 = 1, \lambda_2 = 1.5, \lambda_3 = 2, \omega_1 = 2.2, \omega_2 = -2, \omega_3 = 3, \alpha = 0.5$ .

DownLoad: Full-Size Img PowerPoint

Figure 13. The graphic representation

$\Upsilon(B)$ for the wave number

$M$ via considering the diverse quantities

$\lambda_1 = 1, \lambda_2 = 1.5, \lambda_3 = 2, \omega_1 = 2.2, \omega_2 = -2, \omega_3 = 3, \alpha = 0.5$ .

DownLoad: Full-Size Img PowerPoint

Figure 14. The graphic representation

$\Upsilon(B)$ for the wave number

$M$ via considering the diverse quantities

$\lambda_1 = 1, \lambda_2 = 1.5, \lambda_3 = 2, \omega_1 = 2.2, \omega_2 = -2, \omega_3 = 3, \alpha = 0.5$ .

DownLoad: Full-Size Img PowerPoint

8. Usage of SIVP for Eq (1.8)

Via employing the wave alteration $\xi = k(x+ay+bz-ct)$ in Eq (1.8) once can gain to the below ODE as

$\begin{equation} k^2(\alpha b+a)\Psi^{\prime \prime \prime \prime }+6k(\alpha b+a)\Psi^{\prime }\Psi^{\prime \prime }+(ab\omega_{{2}}-ac\lambda_{{2}}+{b} ^{2}\omega_{{3}}-bc\lambda_{{3}}+b \omega_{{1}}-c\lambda_{{1}} )\Psi^{\prime \prime } = 0, \end{equation}$

(8.1)

in which $\Psi = \Psi(\xi)$ and $\Psi^{\prime } = \frac{d\Psi}{d \xi}$ . According to the SIVP ^[16,17] and by multiplying Eq (8.1) with $\Psi^{\prime }$ and integrating once respect to $\xi$ , the following stationary integral will be arises

$\begin{equation} J = \int_{0}^{\infty}\left[A_1\left(\Psi^{\prime }\Psi^{\prime \prime \prime }- \frac{1}{2}(\Psi^{\prime \prime 2}\right)+\frac{1}{3}A_2(\Psi^{\prime 3}+ \frac{1}{2}A_3(\Psi^{\prime 2}\right]d\xi, \end{equation}$

(8.2)

in which

$A_1 = k^2(\alpha b+a), \ \ \ A_2 = 6k(\alpha b+a), \ \ \ A_3 = ab\omega_{{2} }-ac\lambda_{{2}}+{b}^{2}\omega_{{3}}-bc\lambda_{{3}}+b \omega_{{1} }-c\lambda_{{1}}.$

8.1. Case I

We utilize the solitary wave function as the following

$\begin{equation} u(\xi ) = \delta \ sech(\mu \xi ). \end{equation}$

(8.3)

Hence, the stationary integral transforms to

$\begin{eqnarray} J & = &\frac{1}{30}{k}^{2}{\delta }^{2}\mu \, -21\, \alpha \, b{k}^{2}{\mu } ^{2}-21\, a{k}^{2}{\mu }^{2}-8\, \alpha \, b\delta \, k\mu -8\, a\delta \, k\mu +5\, ab\omega _{{2}} \\ &&-5\, ac\lambda _{{2}}+5\, {b}^{2}\omega _{{3}}-5\, bc\lambda _{{\ 3} }+5\, b\omega _{{1}}-5\, c\lambda _{{1}}). \end{eqnarray}$

(8.4)

Based on the SIVP and using derivative $J$ respect to $A$ and $B$ , one get

$\begin{eqnarray} \frac{\partial J}{\partial A} & = &\frac{1}{15}{k}^{2}\delta \, \mu \, -21\, \alpha \, b{k}^{2}{\mu }^{2}-21\, a{k}^{2}{\mu }^{2}-8\, \alpha \, b\delta \, k\mu -8\, a\delta \, k\mu +5\, ab\omega _{{2}}-5\, ac\lambda _{{2}} \\ &&+5\, {b}^{2}\omega _{{3}}-5\, bc\lambda _{{3}}+5\, b\omega _{{1}}-5\, c\lambda _{{1}})+\frac{1}{30}{k}^{2}{\delta }^{2}\mu \, \left( -8\, \alpha \, bk\mu -8\, ak\mu \right) = 0, \end{eqnarray}$

(8.5)

and

$\begin{eqnarray} \frac{\partial J}{\partial B} & = &\frac{1}{30}{k}^{2}{\delta }^{2}-21\, \alpha \, b{k}^{2}{\mu }^{2}-21\, a{\ k}^{2}{\mu }^{2}-8\, \alpha \, b\delta \, k\mu -8\, a\delta \, k\mu +5\, ab\omega _{{2}}-5\, ac\lambda _{{2}}+5\, {b}^{2}\omega _{{3}} \\ &&-5\, bc\lambda _{{3}}+5\, b\omega _{{1}}-5\, c\lambda _{{1}})+\frac{1}{30}{k} ^{2}{\delta }^{2}\mu \, \left( -42\, \alpha \, b{k}^{2}\mu -42\, a{k}^{2}\mu -8\, \alpha \, b\delta \, k-8\, a\delta \, k\right) = 0. \end{eqnarray}$

(8.6)

Solve the Eqs (8.5) and (8.6), become

$\begin{equation} \delta = \pm \frac{21}{2} \ \ {\frac{ab\omega _{{2}}-ac\lambda _{{2}}+{b} ^{2}\omega _{{3}}-bc\lambda _{{3}}+b\omega _{{1}}-c\lambda _{{1}}} \ \ {\sqrt{ -\left( 21\, \alpha \, b+21\, a\right) \left( ab\omega _{{2}}-ac\lambda _{{2}}+{ b}^{2}\omega _{{3}}-bc\lambda _{{3}}+b\omega _{{1}}-c\lambda _{{1}}\right) }} }, \ \ \ \end{equation}$

(8.7)

$\mu = \pm \frac{1}{21} \ \ {\frac{\sqrt{-\left( 21\, \alpha \, b+21\, a\right) \left( ab\omega _{{2}}-ac\lambda _{{2}}+{b}^{2}\omega _{{3}}-bc\lambda _{{3} }+b\omega _{{1}}-c\lambda _{{1}}\right) }}{\left( \alpha \, b+a\right) k}}.$

The condition can be obtained as below

$\begin{equation} \left( \alpha \, b+a\right) \left( ab\omega _{{2}}-ac\lambda _{{2}}+{b} ^{2}\omega _{{3}}-bc\lambda _{{3}}+b\omega _{{1}}-c\lambda _{{1}}\right) < 0. \end{equation}$

(8.8)

Finally, the solitary solution received by utilizing of SIVP can be reached as

$\begin{equation} \Psi (x, y, z, t) = \delta \ \mathrm{sech}\left[ k\mu (x+ay+bz-ct)\right] . \end{equation}$

(8.9)

8.2. Case II

We utilize the bright wave function as the following

$\begin{equation} u(\xi ) = \delta \ \mathrm{sech}^{2}(\mu \xi ). \end{equation}$

(8.10)

Hence, the stationary integral transforms to

$\begin{eqnarray} J & = &- \ \ {\frac{2\, {\delta }^{2}\mu (120\, \alpha \, b{k}^{2}{\mu }^{2}+120\, a{k} ^{2}{\mu }^{2}+35\, \alpha \, b\delta \, k\mu +35\, a\delta \, k\mu -14\, ab\omega _{{2}}} \ \ {105}} \\ &&+\frac{14\, ac\lambda _{{2}}-14\, {b}^{2}\omega _{{3}}+14\, bc\lambda _{{3} }-14\, b\omega _{{1}}+14\, c\lambda _{{1}})}{105}. \end{eqnarray}$

(8.11)

Based on the SIVP and using derivative $J$ respect to $A$ and $B$ , one get

$\begin{eqnarray} \frac{\partial J}{\partial A} & = &- \ \ {\frac{4\, \delta \, \mu (120\, \alpha \, b{k} ^{2}{\mu }^{2}+120\, a{k}^{2}{\mu }^{2}+35\, \alpha \, b\delta \, k\mu +35\, a\delta \, k\mu -14\, ab\omega _{{2}}+14\, ac\lambda _{{2}}} \ \ {105}} \\ &&+\frac{-14\, {b}^{2}\omega _{{3}}+14\, bc\lambda _{{3}}-14\, b\omega _{{1} }+14\, c\lambda _{{1}})-2\, {\delta }^{2}\mu \, \left( 35\, \alpha \, bk\mu +35\, ak\mu \right) }{105} = 0. \end{eqnarray}$

(8.12)

and

$\begin{eqnarray} \frac{\partial J}{\partial B} & = &- \ \ {\frac{2\, {\delta }^{2}(120\, \alpha \, b{k} ^{2}{\mu }^{2}+120\, a{\ k}^{2}{\mu }^{2}+35\, \alpha \, b\delta \, k\mu +35\, a\delta \, k\mu -14\, ab\omega _{{2}}+14\, ac\lambda _{{2}}-14\, {b} ^{2}\omega _{{3}}} \ \ {105}} \\ &&+\frac{+14\, bc\lambda _{{3}}-14\, b\omega _{{1}}+14\, c\lambda _{{1}})-2\, { \delta }^{2}\mu \, \left( 240\, \alpha \, b{k}^{2}\mu +240\, a{k}^{2}\mu +35\, \alpha \, b\delta \, k+35\, a\delta \, k\right) }{105} = 0. \end{eqnarray}$

(8.13)

Solve the Eqs (8.12) and (8.13), become

$\begin{equation} \delta = \pm \frac{48}{5} \ \ {\frac{ab\omega _{{2}}-ac\lambda _{{2}}+{b} ^{2}\omega _{{3}}-bc\lambda _{{3}}+b\omega _{{1}}-c\lambda _{{1}}} \ \ {\sqrt{ -\left( 21\, \alpha \, b+21\, a\right) \left( ab\omega _{{2}}-ac\lambda _{{2}}+{ b}^{2}\omega _{{3}}-bc\lambda _{{3}}+b\omega _{{1}}-c\lambda _{{1}}\right) }} }, \ \ \ \end{equation}$

(8.14)

$\mu = \pm \frac{1}{30} \ \ {\frac{\sqrt{-\left( 21\, \alpha \, b+21\, a\right) \left( ab\omega _{{2}}-ac\lambda _{{2}}+{b}^{2}\omega _{{3}}-bc\lambda _{{3} }+b\omega _{{1}}-c\lambda _{{1}}\right) }}{\left( \alpha \, b+a\right) k}}.$

The condition of definition of the above relations can be expressed as

$\begin{equation} \left( \alpha \, b+a\right) \left( ab\omega _{{2}}-ac\lambda _{{2}}+{b} ^{2}\omega _{{3}}-bc\lambda _{{3}}+b\omega _{{1}}-c\lambda _{{1}}\right) < 0. \end{equation}$

(8.15)

Finally, the solitary solution gained by utilizing of SIVP will be as

$\begin{equation} \Psi (x, y, z, t) = \delta \ \mathrm{sech}^{2}\left[ k\mu (x+ay+bz-ct)\right] . \end{equation}$

(8.16)

8.3. Case III

Assume the dark soliton wave solution be as the below case as

$\begin{equation} u(\xi ) = \delta \ \tanh ^{2}(\mu \xi ). \end{equation}$

(8.17)

Hence, the stationary integral transforms to

$\begin{eqnarray} J & = & \ \ {\frac{2\, {\delta }^{2}\mu (-120\, \alpha \, b{k}^{2}{\mu }^{2}-120\, a{k} ^{2}{\mu }^{2}+35\, \alpha \, b\delta \, k\mu +35\, a\delta \, k\mu +14\, ab\omega _{{2}}} \ \ {105}} \\ &&+\frac{-14\, ac\lambda _{{2}}+14\, {b}^{2}\omega _{{3}}-14\, bc\lambda _{{3} }+14\, b\omega _{{1}}-14\, c\lambda _{{1}})}{105}. \end{eqnarray}$

(8.18)

Based on the SIVP and using derivative $J$ respect to $A$ and $B$ , one get

$\begin{eqnarray} \frac{\partial J}{\partial \delta } & = & \ \ {\frac{4\, \delta \, \mu (-120\, \alpha \, b{k}^{2}{\mu }^{2}-120\, a{k}^{2}{\mu }^{2}+35\, \alpha \, b\delta \, k\mu +35\, a\delta \, k\mu +14\, ab\omega _{{2}}-14\, ac\lambda _{{2}}} \ \ {105}} \\ &&+\frac{+14\, {b}^{2}\omega _{{3}}-14\, bc\lambda _{{3}}+14\, b\omega _{{1} }-14\, c\lambda _{{1}})+2\, {\delta }^{2}\mu \, \left( 35\, \alpha \, bk\mu +35\, ak\mu \right) }{105} = 0, \end{eqnarray}$

(8.19)

and

$\begin{eqnarray} \frac{\partial J}{\partial \mu } & = & \ \ {\frac{2\, {\delta }^{2}(-120\, \alpha \, b{k }^{2}{\mu }^{2}-120\, a{\ k}^{2}{\mu }^{2}+35\, \alpha \, b\delta \, k\mu +35\, a\delta \, k\mu +14\, ab\omega _{{2}}-14\, ac\lambda _{{2}}+}{105}} \\ &&+\frac{14\, {b}^{2}\omega _{{3}}-14\, bc\lambda _{{3}}+14\, b\omega _{{1} }-14\, c\lambda _{{1}})+2\, {\delta }^{2}\mu \, \left( -240\, \alpha \, b{k} ^{2}\mu -240\, a{k}^{2}\mu +35\, \alpha \, b\delta \, k+35\, a\delta \, k\right) }{ 105} = 0. \end{eqnarray}$

(8.20)

Solve the Eqs (8.19) and (8.20), one get

$\begin{equation} \delta = \mp \frac{48}{5} \ \ {\frac{ab\omega _{{2}}-ac\lambda _{{2}}+{b} ^{2}\omega _{{3}}-bc\lambda _{{3}}+b\omega _{{1}}-c\lambda _{{1}}} \ \ {\sqrt{ -\left( 21\, \alpha \, b+21\, a\right) \left( ab\omega _{{2}}-ac\lambda _{{2}}+{ b}^{2}\omega _{{3}}-bc\lambda _{{3}}+b\omega _{{1}}-c\lambda _{{1}}\right) }} }, \ \ \ \end{equation}$

(8.21)

The condition of definition of the above relations can be presented the following form

$\begin{equation} \left( \alpha \, b+a\right) \left( ab\omega _{{2}}-ac\lambda _{{2}}+{b} ^{2}\omega _{{3}}-bc\lambda _{{3}}+b\omega _{{1}}-c\lambda _{{1}}\right) < 0. \end{equation}$

(8.22)

Finally, the dark solution acquired by utilizing of SIVP will be as

$\begin{equation} \Psi (x, y, z, t) = \delta \ \mathrm{tanh}^{2}\left[ k\mu (x+ay+bz-ct)\right] . \end{equation}$

(8.23)

8.4. Case IV

Let the singular soliton wave solution be as the below case as

$\begin{equation} u(\xi ) = \delta \ csch^{2}(\mu \xi ). \end{equation}$

(8.24)

Then, the stationary integral transforms to

$\begin{eqnarray} J & = &- \ \ {\frac{2\, {\delta }^{2}\mu (-120\, \alpha \, b{k}^{2}{\mu }^{2}-120\, a{k }^{2}{\mu }^{2}-70\, \alpha \, b\delta \, k\mu -70\, a\delta \, k\mu +14\, ab\omega _{{2}}} \ \ {105}} \\ &&+\frac{-14\, ac\lambda _{{2}}+14\, {b}^{2}\omega _{{3}}-14\, bc\lambda _{{3} }+14\, b\omega _{{1}}-14\, c\lambda _{{1}})}{105}. \end{eqnarray}$

(8.25)

Based on the SIVP and using derivative $J$ respect to $A$ and $B$ , become

$\begin{eqnarray} \frac{\partial J}{\partial \delta } & = &- \ \ {\frac{4\, \delta \, \mu (-120\, \alpha \, b{k}^{2}{\mu }^{2}-120\, a{k}^{2}{\mu }^{2}-70\, \alpha \, b\delta \, k\mu -70\, a\delta \, k\mu +14\, ab\omega _{{2}}-14\, ac\lambda _{{2} }}{105}} \\ &&+\frac{+14\, {b}^{2}\omega _{{3}}-14\, bc\lambda _{{3}}+14\, b\omega _{{1} }-14\, c\lambda _{{1}})-2\, {\delta }^{2}\mu \, \left( -70\, \alpha \, bk\mu -70\, ak\mu \right) }{105} = 0 \end{eqnarray}$

(8.26)

and

$\begin{eqnarray} \frac{\partial J}{\partial \mu } & = &- \ \ {\frac{2\, {\delta }^{2}(-120\, \alpha \, b{ k}^{2}{\mu }^{2}-120\, a{k}^{2}{\mu }^{2}-70\, \alpha \, b\delta \, k\mu -70\, a\delta \, k\mu +14\, ab\omega _{{2}}-14\, ac\lambda _{{2}}+14\, {b} ^{2}\omega _{{3}}} \ \ {105}} \\ &&+\frac{-14\, bc\lambda _{{3}}+14\, b\omega _{{1}}-14\, c\lambda _{{1}})-2\, { \delta }^{2}\mu \, \left( -240\, \alpha \, b{k}^{2}\mu -240\, a{k}^{2}\mu -70\, \alpha \, b\delta \, k-70\, a\delta \, k\right) }{105} = 0. \end{eqnarray}$

(8.27)

Solve the Eqs (8.26) and (8.27), one get

$\begin{equation} \delta = \pm \frac{24}{5} \ \ {\frac{ab\omega _{{2}}-ac\lambda _{{2}}+{b} ^{2}\omega _{{3}}-bc\lambda _{{3}}+b\omega _{{1}}-c\lambda _{{1}}} \ \ {\sqrt{ -\left( 21\, \alpha \, b+21\, a\right) \left( ab\omega _{{2}}-ac\lambda _{{2}}+{ b}^{2}\omega _{{3}}-bc\lambda _{{3}}+b\omega _{{1}}-c\lambda _{{1}}\right) }} }, \ \ \ \end{equation}$

(8.28)

The condition of definition of the above relations can be presented as the below form as

$\begin{equation} \left( \alpha \, b+a\right) \left( ab\omega _{{2}}-ac\lambda _{{2}}+{b} ^{2}\omega _{{3}}-bc\lambda _{{3}}+b\omega _{{1}}-c\lambda _{{1}}\right) < 0. \end{equation}$

(8.29)

Finally, the singular solution acquired by utilizing of SIVP will be as

$\begin{equation} \Psi (x, y, z, t) = \delta \ \mathrm{csch}^{2}\left[ k\mu (x+ay+bz-ct)\right] . \end{equation}$

(8.30)

9. Conclusion

In this article, the MEFM employed for searching the MSSs for the gKP equation, which contains 1-wave, 2-wave, and 3-wave solutions. The periodic wave, cross-kink, and solitary wave solutions have been obtained. In continuing, the modulation instability applied to discuss the stability of earned solutions. It is quite visible that these novel schemes have plenty of family solutions containing rational exponential, hyperbolic, and periodic functions with selecting particular parameters. Also, the semi-inverse variational principle will be used for the gKP equation. Four major cases containing the solitary, bright, dark and singular wave solutions were studied from four different ansatzes.

By means of symbolic computation, these analytical solutions and corresponding rogue waves are obtained. Via various curve plots, density plot and three-dimensional plots, dynamical characteristics of these rouge waves are exhibited. Because of the strong nonlinear characteristic of Hirota bilinear method, the test function constructed by the Hirota operator, which can be regarded as the test function constructed by considered model. The results are beneficial to the study of the plasma, optics, acoustics, fluid dynamics and fluid mechanics. All computations in this paper have been employed quickly with the help of the Maple 18. Moreover, the method applied in this paper provides an effective tool to obtain exact solutions of nonlinear system and can in common use for other NLEEs.

Acknowledgments

This work is supported by the Education and scientific research project for young and middle-aged teachers of Fujian Province (No. JAT.190666-No.JAT200469)

Conflict of interest

The authors declare that there is no conflict of interests regarding the publication of this paper.

References

[1]	S. T. R. Rizvi, K. Ali, M. Ahmad, Optical solitons for Biswas-Milovic equation by new extended auxiliary equation method, Optik, 204 (2020), 164181. doi: 10.1016/j.ijleo.2020.164181
[2]	B. Nawaz, K. Ali, S. O. Abbas, S. T. R. Rizvi, Q. Zhou, Optical solitons for non-Kerr law nonlinear Schrödinger equation with third and fourth order dispersions, Chinese J. Phys., 60 (2019), 133-140. doi: 10.1016/j.cjph.2019.05.014
[3]	M. Dehghan, J. M. Heris, A. Saadatmandi, Application of the Exp-function method for solving a partial differential equation arising in biology and population genetics, Int. J. Num. Meth. Heat, 21 (2011), 736-753. doi: 10.1108/09615531111148482
[4]	M. Dehghan, J. Manafian, A. Saadatmandi, Solving nonlinear fractional partial differential equations using the homotopy analysis method, Num. Meth. Part. D. E., 26 (2010), 448-479. doi: 10.1002/num.20460
[5]	M. Dehghan, J. Manafian, The solution of the variable coefficients fourth-order parabolic partial differential equations by homotopy perturbation method, Z. Naturforsch. A, 64 (2009), 420-430. doi: 10.1515/zna-2009-7-803
[6]	J. Manafian, S. Heidari, Periodic and singular kink solutions of the Hamiltonian amplitude equation, Adv. Math. Mod. Appl., 4 (2019), 134-149.
[7]	A. R. Seadawy, J. Manafian, New soliton solution to the longitudinal wave equation in a magneto-electro-elastic circular rod, Results Phys., 8 (2018), 1158-1167. doi: 10.1016/j.rinp.2018.01.062
[8]	J. Manafian, Novel solitary wave solutions for the (3+1)-dimensional extended Jimbo-Miwa equations, Comput. Math. Appl., 76 (2018), 1246-1260. doi: 10.1016/j.camwa.2018.06.018
[9]	W. X. Ma, Y. Zhou, Lump solutions to nonlinear partial differential equations via Hirota bilinear forms, J. Diff. Eq., 264 (2018), 2633-2659. doi: 10.1016/j.jde.2017.10.033
[10]	W. X. Ma, A search for lump solutions to a combined fourthorder nonlinear PDE in (2+1)-dimensions, J. Appl. Anal. Comput., 9 (2019), 1319-1332.
[11]	W. X. Ma, Interaction solutions to Hirota-Satsuma-Ito equation in (2+1)-dimensions, Front. Math. China, 14 (2019), 619-629. doi: 10.1007/s11464-019-0771-y
[12]	J. Manafian, B. Mohammadi-Ivatlo, M. Abapour, Lump-type solutions and interaction phenomenon to the (2+1)-dimensional Breaking Soliton equation, Appl. Math. Comput., 356 (2019), 13-41.
[13]	O. A. Ilhan, J. Manafian, Periodic type and periodic cross-kink wave solutions to the (2+1)-dimensional breaking soliton equation arising in fluid dynamics, Mod. Phys. Lett. B, 33 (2019), 1950277.
[14]	W. X. Ma, Y. Zhou, R. Dougherty, Lump-type solutions to nonlinear differential equations derived from generalized bilinear equations, Int. J. Mod. Phys. B, 30 (2016), 1640018. doi: 10.1142/S021797921640018X
[15]	J. Q. Lü, S. Bilige, X. Q. Gao, Y. X. Bai, R. F. Zhang, Abundant lump solution and interaction phenomenon to Kadomtsev-Petviashvili-Benjamin-Bona-Mahony equation, J. Appl. Math. Phys., 6 (2018), 1733-1747. doi: 10.4236/jamp.2018.68148
[16]	J. H. He, Some asymptotic methods for strongly nonlinear equations, Int. J. Mod. Phys. B, 20 (2006), 1141-1199. doi: 10.1142/S0217979206033796
[17]	J. H. He, A modified Li-He's variational principle for plasma, Int. J. Numer. Meth. Heat, 31 (2021), 1369-1372.
[18]	S. S. Chen, B. Tian, L. Liu, Y. Q. Yuan, C. R. Zhang, Conservation laws, binary Darboux transformations and solitons for a higher-order nonlinear Schrödinger system, Chaos Solitons Frac., 118 (2019), 337-346. doi: 10.1016/j.chaos.2018.11.010
[19]	X. X. Du, B. Tian, X. Y. Wu, H. M. Yin, C. R. Zhang, Lie group analysis, analytic solutions and conservation laws of the (3+1)-dimensional Zakharov-Kuznetsov-Burgers equation in a collisionless magnetized electronpositron- ion plasma, Eur. Phys. J. Plus, 133 (2018), 378. doi: 10.1140/epjp/i2018-12239-y
[20]	S. S. Ray, On conservation laws by Lie symmetry analysis for (2+1)-dimensional Bogoyavlensky-Konopelchenko equation in wave propagation, Comput. Math. Appl., 74 (2017), 1158-1165. doi: 10.1016/j.camwa.2017.06.007
[21]	X. H. Zhao, B. Tian, X. Y. Xie, X. Y. Wu, Y. Sun, Y. J. Guo, Solitons, Bäcklund transformation and Lax pair for a (2+1)-dimensional Davey-Stewartson system on surface waves of finite depth, Wave Random Complex, 28 (2018), 356-366. doi: 10.1080/17455030.2017.1348645
[22]	J. Manafian, An optimal galerkin-homotopy asymptotic method applied to the nonlinear second-order bvps, Proc. Inst. Math. Mech., 47 (2021), 156-182.
[23]	Q. L. Zha, A symbolic computation approach to constructing rogue waves with a controllable center in the nonlinear systems, Comput. Math. Appl., 75 (2018), 3331-3342. doi: 10.1016/j.camwa.2018.02.001
[24]	W. H. Liu, Y. F. Zhang, Multiple rogue wave solutions for a (3+1)-dimensional Hirota bilinear equation, Appl. Math. Lett., 98 (2019), 184-190. doi: 10.1016/j.aml.2019.05.047
[25]	H. M. Baskonus, H. Bulut, Exponential prototype structures for (2+1)-dimensional Boiti-Leon-Pempinelli systems in mathematical physics, Wave Random Complex, 26 (2016), 189-196. doi: 10.1080/17455030.2015.1132860
[26]	M. Inc, A. I. Aliyu, A. Yusuf, D. Baleanu, Optical solitary waves, conservation laws and modulation instabilty analysis to nonlinear Schrödinger's equations in compressional dispersive Alfvan waves, Optik, 155 (2018), 257-266. doi: 10.1016/j.ijleo.2017.10.109
[27]	B. B. Kadomtsev, V. I. Petviashvili, On the stability of solitary waves in weakly dispersive media, Dokl. Akad. Nauk SSSR, 192 (1970), 753-756.
[28]	A. M. Wazwaz, Multi-front waves for extended form of modified Kadomtsev-Petviashvili equations, Appl. Mech. Engl. Ed. 32 (2011), 875-880.
[29]	T. Xiao, Y. B. Zeng, A new constrained mKP hierarchy and the generalized Darboux transformation for the mKP equation with self-consistent sources, Phys. A, 353 (2005), 38-60. doi: 10.1016/j.physa.2005.02.043
[30]	W. X. Ma, T. C. Xia, Pfaffianized systems for a generalized Kadomtsev-Petviashvili equation, Phys. Scr., 87 (2013), 055003. doi: 10.1088/0031-8949/87/05/055003
[31]	W. X. Ma, Z. N. Zhu, Solving the (3+1)-dimensional generalized KP and BKP equations by the multi expfunction algorithm, Appl. Math. Comput., 218 (2012), 11871-11879.
[32]	R. Hirota, The Direct Method in Soliton Theory, Cambridge: Cambridge University Press, 2004,198.
[33]	A. M. Wazwaz, S. A. El-Tantawy, A new (3+1)-dimensional generalized Kadomtsev-Petviashvili equation, Nonlinear Dyn., 84 (2016), 1107-1112. doi: 10.1007/s11071-015-2555-6
[34]	X. F. Cao, Lump Solutions to the (3+1)-Dimensional Generalized B-Type Kadomtsev-Petviashvili Equation, Adv. Math. Phys., 2018 (2018), 7843498.
[35]	X. Guan, W. J. Liu, Q. Zhou, A. Biswas, Some lump solutions for a generalized (3+1)-dimensional Kadomtsev-Petviashvili equation, Appl. Math. Comput., 366 (2020), 124757.
[36]	J. G. Liu, Y. He, Abundant lump and lump-kink solutions for the new (3+1)-dimensional generalized Kadomtsev-Petviashvili equation, Nonlinear Dyn., 92 (2018), 1103-1108. doi: 10.1007/s11071-018-4111-7
[37]	J. Manafian, M. Lakestani, Interaction among a lump, periodic waves, and kink solutions to the fractional generalized CBS-BK equation, Math. Meth. Appl. Sci., 44 (2021), 1052-1070. doi: 10.1002/mma.6811
[38]	J. Manafian, B. M. Ivatloo, M. Abapour, Breather wave, periodic, and cross-kink solutions to the generalized Bogoyavlensky-Konopelchenko equation, Math. Meth. Appl. Sci., 43 (2020), 1753-1774. doi: 10.1002/mma.6000
[39]	J. Manafian, O. A. Ilhan, L. Avazpour, A. Alizadeh, N-lump and interaction solutions of localized waves to the (2+1)-dimensional asymmetrical Nizhnik-Novikov-Veselov equation arise from a model for an incompressible fluid, Math. Meth. Appl. Sci., 43 (2020), 9904-9927. doi: 10.1002/mma.6665
[40]	X. Y. Gao, Mathematical view with observational/experimental consideration on certain (2+1)-dimensional waves in the cosmic/laboratory dusty plasmas, Appl. Math. Lett., 91 (2019), 165-172. doi: 10.1016/j.aml.2018.11.020
[41]	K. S. Nisar, O. A. Ilhan, S. T. Abdulazeez, J. Manafian, S. A. Mohammed, M.S. Osman, Novel multiple soliton solutions for some nonlinear PDEs via multiple Exp-function method, Results Phys., 21 (2021), 103769. doi: 10.1016/j.rinp.2020.103769
[42]	J. Zhao, J. Manafian, N. E. Zaya, S. A. Mohammed, Multiple rogue wave, lump-periodic, lump-soliton, and interaction between k-lump and k-stripe soliton solutions for the generalized KP equation, Math. Meth. Appl. Sci., 44 (2021), 5079-5098. doi: 10.1002/mma.7093
[43]	C. Q. Dai, Y. Fan, N. Zhang, Re-observation on localized waves constructed by variable separation solutions of (1+1)-dimensional coupled integrable dispersionless equations via the projective Riccati equation method, Appl. Math. Lett., 96 (2019), 20-26. doi: 10.1016/j.aml.2019.04.009
[44]	H. Q. Sun, A. H. Chen, Lump and lump-kink solutions of the (3+1)-dimensional Jimbo-Miwa and two extended Jimbo-Miwa equations, Appl. Math. Lett., 68 (2017), 55-61. doi: 10.1016/j.aml.2016.12.008
[45]	B. Q. Li, Y. L. Ma, Multiple-lump waves for a (3+1)-dimensional Boiti-Leon-Manna-Pempinelli equation arising from incompressible fluid, Comput. Math. Appl., 76 (2018), 204-214. doi: 10.1016/j.camwa.2018.04.015
[46]	Y. Zhang, H. H. Dong, X. E. Zhang, H. W. Yang, Rational solutions and lump solutions to the generalized (3+1)-dimensional shallow water-like equation, Comput. Math. Appl., 73 (2017), 246-252. doi: 10.1016/j.camwa.2016.11.009
[47]	M. Hamid, M. Usman, T. Zubair, R. U. Haq, A. Shafee, An efficient analysis for N-soliton, Lump and lump-kink solutions of time-fractional (2+1)-Kadomtsev-Petviashvili equation, Phys. A, 528 (2019), 121320. doi: 10.1016/j.physa.2019.121320
[48]	A. R. Adem, The generalized (1+1)-dimensional and (2+1)-dimensional Ito equations: Multiple exp-function algorithm and multiple wave solutions, Comput. Math. Appl., 71 (2016), 1248-1258. doi: 10.1016/j.camwa.2016.02.005
[49]	Y. Long, Y. H. He, S. L. Li, Multiple soliton solutions for a new generalization of the associated camassa-holm equation by exp-function method, Math. Prob. Eng., 2014 (2014), 418793.
[50]	J. G. Liu, L. Zhou, Y. He, Multiple soliton solutions for the new (2+1)-dimensional Korteweg-de Vries equation by multiple exp-function method, Appl. Math. Lett., 80 (2018), 71-78. doi: 10.1016/j.aml.2018.01.010
[51]	J. P. Yu, J. Jing, Y. L. Sun, S. P. Wu, (n+1)-Dimensional reduced differential transform method for solving partial differential equations, Appl. Math. Comput., 273 (2016), 697-705.
[52]	J. P. Yu, Y. L. Sun, Study of lump solutions to dimensionally reduced generalized KP equations, Nonlinear Dyn., 87 (2017), 2755-2763. doi: 10.1007/s11071-016-3225-z

This article has been cited by:

1.	Chaudry Masood Khalique, Oke Davies Adeyemo, Kentse Maefo, Symmetry solutions and conservation laws of a new generalized 2D Bogoyavlensky-Konopelchenko equation of plasma physics, 2022, 7, 2473-6988, 9767, 10.3934/math.2022544
2.	K. Hosseini, D. Baleanu, S. Rezapour, S. Salahshour, M. Mirzazadeh, M. Samavat, Multi-complexiton and positive multi-complexiton structures to a generalized B-type Kadomtsev−Petviashvili equation, 2022, 24680133, 10.1016/j.joes.2022.06.020
3.	Abdul-Majid Wazwaz, Naisa S. Alatawi, Wedad Albalawi, S. A. El-Tantawy, Painlevé analysis for a new (3 +1 )-dimensional KP equation: Multiple-soliton and lump solutions, 2022, 140, 0295-5075, 52002, 10.1209/0295-5075/aca49f
4.	Yan Zhu, Chuyu Huang, Junjie Li, Runfa Zhang, Lump solitions, fractal soliton solutions, superposed periodic wave solutions and bright-dark soliton solutions of the generalized (3+1)-dimensional KP equation via BNNM, 2024, 112, 0924-090X, 17345, 10.1007/s11071-024-09911-2
5.	Adisie Fenta Agmas, Fasika Wondimu Gelu, Meselech Chima Fino, A robust, exponentially fitted higher-order numerical method for a two-parameter singularly perturbed boundary value problem, 2025, 10, 2297-4687, 10.3389/fams.2024.1501271

Reader Comments

Your name:*

Email:*
© 2021 the Author(s), licensee AIMS Press. This is an open access article distributed under the terms of the Creative Commons Attribution License (http://creativecommons.org/licenses/by/4.0)

通讯作者: 陈斌, bchen63@163.com

1.
沈阳化工大学材料科学与工程学院沈阳 110142

AIMS Mathematics

1.8 3.4

Metrics

Article views(3341) PDF downloads(127) Cited by(5)

Preview PDF

Download XML

Export Citation

Article outline

Show full outline

Figures and Tables

Figures(14)

AIMS Mathematics

Modulational instability, multiple Exp-function method, SIVP, solitary and cross-kink solutions for the generalized KP equation

Related Papers:

Abstract

1. Introduction

2. Multiple Exp-function method

3. MSSs for the (3+1) gKP equation

3.1. Option I: 1-wave solution

3.2. Option II: 2-wave solutions

3.3. Option III: Triple-wave solutions

4. Novel periodic wave solutions

5. Novel cross-kink wave solutions

6. Novel solitary wave solutions

7. Stability analysis of Eq (1.8)

8. Usage of SIVP for Eq (1.8)

8.1. Case I

8.2. Case II

8.3. Case III

8.4. Case IV

9. Conclusion

Acknowledgments

Conflict of interest

References

This article has been cited by:

Reader Comments

通讯作者: 陈斌, bchen63@163.com

Metrics

Figures and Tables

Other Articles By Authors

Catalog

AIMS Mathematics

Modulational instability, multiple Exp-function method, SIVP, solitary and cross-kink solutions for the generalized KP equation

Related Papers:

Abstract

1. Introduction

2. Multiple Exp-function method

3. MSSs for the (3+1) gKP equation

3.1. Option I: 1-wave solution

3.2. Option II: 2-wave solutions

3.3. Option III: Triple-wave solutions

4. Novel periodic wave solutions

5. Novel cross-kink wave solutions

6. Novel solitary wave solutions

7. Stability analysis of Eq (1.8)

8. Usage of SIVP for Eq (1.8)

8.1. Case I

8.2. Case II

8.3. Case III

8.4. Case IV

9. Conclusion

Acknowledgments

Conflict of interest

References

This article has been cited by:

Reader Comments

通讯作者: 陈斌, bchen63@163.com

Metrics

Figures and Tables

Other Articles By Authors

Related pages

Tools

Export File

Citation

Format

Content

Catalog