Derivation of some solitary wave solutions for the (3+1)- dimensional pKP-BKP equation via the IME tanh function method

1.   Introduction

With the rapid development of science and technology, it is becoming more and more important to solve the optimal control problem by using appropriate numerical methods for satisfying various different actual requirements. Many numerical methods, such as finite volume element method, finite element method, mixed finite element method, and spectral method have been applied to approximate the solutions of optimal control problems (see, e.g., ^{[4,7,8,9,12,16,18,20,21,22,23,25]}). The optimal control problem of bilinear type considered in this paper includes a useful model of parameter estimation problems. It plays a very important role in many fields of science and engineering, where prior errors can improve accuracy and promote the development of related practical applications, such as air and water pollution control, oil exploration, and other fields. Although numerical analysis for bilinear optimal control problem was considered in a number of ^[11,23,28], there were few papers that consider the error estimates of finite volume element method for bilinear parabolic optimal control problem.

The finite volume element methods lie somewhere between finite difference and finite element methods, they have a flexibility similar to that of finite element methods for handling complicated solution domain geometries and boundary conditions, and they have a simplicity for implementation comparable to finite difference methods with triangulations of a simple structure. The finite volume methods are effective discretization technique for partial differential equations. Bank and Rose obtained some results for elliptic boundary value problems that the finite volume element approximation was comparable with the finite element approximation in $H^1$-norm which can be found in ^[3]. In ^[15], the authors presented the optimal $L^2$-error estimates for second-order elliptic boundary value problems under the assumption that $f\in H^1$, they also obtained the $H^1$-norm and $L^\infty$-norm error estimates for those problems. In ^[27], Luo and Chen used the finite volume element method to obtain the approximation solution for optimal control problem associate with a parabolic equation by using optimize-then-discretize approach and the variational discretization technique. The authors also derived some error estimates for the semi-discrete approximation. Recently, the first author of this paper investigated $L^\infty$-error estimates of the bilinear elliptic optimal control problem by rectangular Raviart-Thomas mixed finite element methods in ^[23]. In this paper, we will study a priori error estimates for the finite volume element approximation of bilinear parabolic optimal control problem. By using finite volume element method to discretize the state and adjoint equations. Under some reasonable assumptions, we obtained some optimal order error estimates. Moreover, by employing the backward Euler method for the discretization of time, and using finite volume element method to discretize the state and adjoint equations, we will construct the fully discrete finite volume element approximation scheme for the bilinear optimal control problem. Then we obtain a priori error estimates for the fully discrete finite volume element approximation of bilinear parabolic optimal control problem.

In this paper, we use the standard notations $W^{m, p}(\Omega)$ for Sobolev spaces and their associated norms $||v||_{m, p}$ (see, e.g., ^[1]) in these paper. To simplify the notations, we denote $W^{m, 2}(\Omega)$ by $H^m(\Omega)$ and drop the index $p = 2$ and $\Omega$ whenever possible, i.e., $||u||_{m, 2, \Omega} = ||u||_{m, 2} = ||u||_m, \ ||u||_0 = ||u||$. Set $H^1_0(\Omega) = \{v\in H^1: v|_{\partial\Omega} = 0\}$. As usual, we use $(\cdot, \cdot)$ to denote the $L^2(\Omega)$-inner product.

We consider the following bilinear parabolic optimal control problem

where $\alpha$ is a positive constant,

$\Omega\subset {\mathbb{R}}^2$ is a bounded convex polygonal domain and $\Gamma$ is the boundary of $\Omega$, $f(\cdot, t)\in L^{2}(\Omega)$ or $H^1(\Omega)$, $J = (0, T]$, $A = (a_{i, j})_{2\times 2}$ is a symmetric, smooth enough and uniformly positive definite matrix in $\Omega$, $y_0(x) = 0, x\in \Gamma$. It is assumed that the functions $y$ have enough regularity and they satisfy appropriate compatibility conditions so that the boundary value problems (1.1)–(1.3) has a unique solution. $U_{ad}$ is a set defined by

The remainder of this paper is organized as follows. In Section 2, we present some notations and the finite volume element approximation for the bilinear parabolic optimal control problem. In Section 3, we analyze the error estimates between the exact solution and the finite volume element solution. In Section 4, a priori error estimates for the fully discrete finite volume element approximation of the bilinear optimal control problem are presented. a numerical example is presented to test the theoretical results in Section 5. Finally, we briefly give conclusions and some possible future works in Section 6.

2.   Finite volume element approximation for bilinear parabolic optimal control

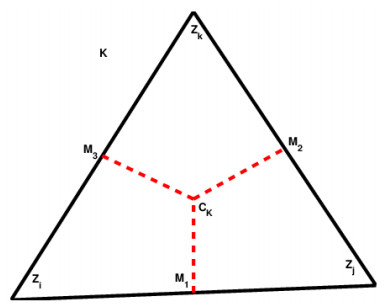
For the convex polygonal domain $\Omega$, we consider a quasi-uniform triangulation $\mathcal {T}_h$ consisting of closed triangle elements $K$ such that $\bar{\Omega} = \bigcup\limits_{K\in\mathcal {T}_h}K$. We use $N_h$ to denote the set of all nodes or vertices of $\mathcal {T}_h$. To define the dual partition $\mathcal {T}_h^*$ of $\mathcal {T}_h$, we divide each $K\in\mathcal {T}_h$ into three quadrilaterals by connecting the barycenter $C_K$ of $K$ with line segments to the midpoints of edges of $K$ as is shown in Figure 1.

The control volume $V_i$ consists of the quadrilaterals sharing the same vertex $z_i$ as is shown in Figure 2.

The dual partition $\mathcal {T}_h^*$ consists of the union of the control volume $V_i$. Let $h = \max\{h_K \}$, where $h_K$ is the diameter of the triangle $K$. As is shown in ^[15], the dual partition $\mathcal {T}_h^*$ is also quasi-uniform. Throughout this paper, the constant $C$ denotes different positive constant, which is independent of the mesh size $h$ and the time step $k$.

We define the finite dimensional space $V_h$ associated with $\mathcal {T}_h$ for the trial functions by

and define the finite dimensional space $Q_h$ associated with the dual partition $\mathcal {T}_h^*$ for the test functions by

where $P_l(K)$ or $P_l(V)$ consists of all the polynomials with degree less than or equal to $l$ defined on $K$ or $V$.

To connect the trial space and test space, we define a transfer operator $I_h: V_h\rightarrow Q_h$ as follows:

where $\chi_i$ is the characteristic function of $V_i$. For the operator $I_h$, it is well known that there exists a positive constant $C$ such that for all $v\in V_h$, we can get

Let $a(w, v) = \int_\Omega A\nabla w\cdot \nabla vdx$. We assume $a(v, v)$ satisfies the coercive conditions, then coercive property of $a(\cdot, \cdot)$ is that there exists a positive constant $c$ such that for all $v\in V_h$, we can obtain (see, e.g., ^[5])

As is defined in ^[6], we define the standard Ritz projection $R_h: H^1_0\rightarrow V_h$ by

For the projection $R_h$, it has the property that (see, e.g., ^[6])

Now, we will use the optimize-then-discretize approach to obtain the semi-discrete finite volume element scheme for the bilinear parabolic optimal control problem.

As is seen in ^[25], the necessary and sufficient optimal condition of (1.1)–(1.3) consists of the state equation, a co-state equation and a variational inequality, i.e., find $y(\cdot, t)$, $p(\cdot, t) \; \in H^1_0(\Omega)$ and $u(\cdot, t)\in U_{ad}$ such that

If $y(\cdot, t)\in H^1_0(\Omega)\cap C^2(\Omega)$ and $p(\cdot, t)\in H^1_0(\Omega)\cap C^2(\Omega)$, then the optimal control problems (2.5)–(2.7) can be written by

Then, we use the finite volume element method to discretize the state and co-state equations directly. Then the continuous optimal control problems (2.8)–(2.12) can be approximated by: find $(y_h(\cdot, t), p_h(\cdot, t), u_h(\cdot, t))\in V_h\times V_h\times U_{ad}$ such that

where $a_h(\phi, I_h\psi) = -\sum\limits_{z_i\in N_h}\psi(z_i)\int_{\partial V_i}A \nabla\phi\cdot \textbf{n}ds$.

Similar to ^[17], we can find that the variational inequality (2.12) is equivalent to

And then the variational inequality (2.17) is equivalent to

3.   A priori error estimates

In this section, we will analyze the error between the exact solution and the finite volume element solution. Let $\varepsilon_a(x, y) = a(x, y)-a_h(x, I_hy)$, it is well known (see, e.g., ^[6,13]) that for all $y\in V_h$:

To deduce the error estimates, let ($y_h(u)$, $p_h(u)$) be the solution of

where for all $w_h, q_h\in V_h$, note that $y_h = y_h(u_h)$, $p_h = p_h(u_h)$, we have the following lemma for $y_h(u)$ and $p_h(u)$.

Let $(p(u), y(u))$ and $(p_h(u), y_h(u))$ be the solutions of (2.13)–(2.15) and (3.2)–(3.4), respectively. Let $J(\cdot):U_{ad}\rightarrow \mathbb{R}$ be a $G$-differential convex functional near the solution $u$ which satisfies the following form:

Then we have a sequence of convex functional $J_h:U_{ad}\rightarrow \mathbb{R}$:

It can be shown that

In the following we estimate $\| u-u_h\|_{L^2(J; L^2)}$. We assume that the cost function $J$ is strictly convex near the solution $u$, i.e., for the solution $u$ there exists a neighborhood of $u$ in $L^2$ such that $J$ is convex in the sense that there is a constant $c > 0$ satisfying:

For all $v$ in this neighborhood of $u$. The convexity of $J(\cdot)$ is closely related to the second order sufficient optimality conditions of optimal control problems, which are assumed in many studies on numerical methods of the problem. For instance, in many references, the authors assume the following second order sufficiently optimality condition (see ^[14,26]): there is $c > 0$ such that $J^{\prime\prime}(u)v^2\geq c \| v\|_{0}^2$.

From the assumption (3.6), by the proof contained in ^[2,10], there exists a constant $c > 0$ satisfying

Now, we estimate the error of the approximate control in $L^2$-norm.

Theorem 3.1. Let $(y, p, u)$ and $(y_h, p_h, u_h)$ are the solutions of (2.5)–(2.7) and (2.13)–(2.17), respectively. Then there exists a constant $h_0 > 0$ such that for all $0 < h\leq h_0$, we have

Proof. Let $v = u_h$ in (2.7) and $v = u$ in (2.17), then we have

From (3.9) and (3.10), it is easy to see that

By using (3.7) and (3.11), we obtain

Now, we estimate all terms at the right side of (3.12). By using the Theorem 4.2 in ^[24] and the $\delta$-Cauchy inequality, we have

In the same way, we also have

Putting (3.13) and (3.14) into (3.12) and choosing appropriate value for $\delta$, we can obtain the result (3.8). □

Theorem 3.2. Let $(y, p, u)$ and $(y_h, p_h, u_h)$ are the solutions of (2.5)–(2.7) and (2.13)–(2.17), respectively. Then there exists a constant $h_0 > 0$ such that for all $0 < h\leq h_0$, we have

Proof. By employing the triangle inequality, we have

Similar to Lemma 3.1 in ^[16], it implies that

By using Theorem 3.1, (3.17)–(3.18) and Theorem 4.2 of ^[24], we can easily obtain

In the same way, we also have

Combining (3.19) with (3.20), we can prove (3.15). Similarly, we can obtain (3.18). □

4.   Fully discrete finite volume element approximation

In this section, we will present a fully discrete scheme and error estimates of the finite volume element approximation.

Now, we shall construct the fully discrete approximation scheme for semi-discrete scheme (2.13)–(2.17). Let $0 = t_0 < t_1 < \cdots < t_{M-1} < t_m = T$, $t_i = i\triangle t$, $\triangle t = \frac{T}{M}$, for $i = 1, 2, \cdots, M$. And let $\psi^i = \psi(x, t_i)$, $\partial \psi^i = (\psi^i-\psi^{i-1})/\triangle t$. We define a discrete time-dependent norm for $1\leq s < \infty$ by $|||\psi|||_{L^s(J; H^m(\Omega))} = \Big(\sum\limits_{i = 1}^{M}\triangle t||\psi^i||_m^s\Big)^{1/s}$ (e.g., $|||\psi|||_{L^2(J; L^2)} = \Big(\sum\limits_{i = 1}^{M}\triangle t||\psi^i||^2\Big)^{1/2}$, $|||\psi|||_{L^\infty(J; L^2)} = \max\limits_{1\leq i\leq M}||\psi^i||$). By using the backward Euler method for the discretization of time in (2.13) and (2.15), we can obtain the fully discrete scheme of (2.13)–(2.17) is to find $(y_h^i, p_h^{i-1}, u_h^i)\in V_h\times V_h\times U_{ad}$ such that

To derive the fully discrete error analysis, let $(y_h^i(u), p_h^{i-1}(u))$ be the solution of

Let $u_h = (u_h^0, u_h^1, \cdots, u_h^M)$, $y_h = (y_h^0, y_h^1, \cdots, y_h^M)$ and $p_h = (p_h^0, p_h^1, \cdots, p_h^M)$. For $(y_h^i(u), p_h^{i-1}(u))$, we have the following lemma.

Lemma 4.1. Assume that $(y_h^i, p_h^{i-1}, u_h^i)$ and $(y_h^i(u), p_h^{i-1}(u))$ are the solutions of (4.1)–(4.5) and (4.6)–(4.9), respectively. Then we have the following results:

Proof. Let $\eta^k = y_h^k(u)-y_h^k\ (1\leq k\leq i)$. Subtracting (4.1) from (4.6), we have

Let $w_h = \partial \eta^k$, we have

Due to $\varepsilon_a(x, y) = a(x, y)-a_h(x, I_hy)$, we can get

Note that

Thanks to $a(\eta^k-\eta^{k-1}, \eta^k-\eta^{k-1})\geq 0$, we obtain

The inverse estimate and (3.1) imply that

Note that

and

Using the equivalent properties of $(\cdot, \cdot)$, $(\cdot, I_h(\cdot))$ and $(I_h(\cdot), I_h(\cdot))$, we derive

Choosing appropriate value for $\delta$, we have

Applying the coercive property of $a(\cdot, \cdot)$, and summing $k$ from 1 to $i$ and noticing $\eta^0 = 0$, we can get

By using the discrete Gronwall's lemma (see, e.g., Lemma 3.3 in ^[9]), Then we have

Then we prove (4.10). In the same way as (4.10), we can obtain (4.11). □

We can get the error estimate for $u_h$ in the discrete $L^2(J; L^2)$-norm by using Lemma 4.1.

Theorem 4.1. Let $(y, p, u)$ and $(y_h^i, p_h^{i-1}, u_h^i)$ be the solutions of problems (2.5)–(2.7) and (4.1)–(4.5), respectively. Then there exists a constant $h_0 > 0$ such that for all $0 < h\leq h_0$, we have

Proof. Note that $y_h^i = y_h^i(u_h^i)$ and $p_h^i = p_h^i(u_h^i)$, it can be shown that

From (2.7), (3.7), and (4.5), we have

For the first term $T_1$, using the Cauchy inequality and the Theorem 4.1 in ^[29], we can obtain

For the second term $T_2$, we can derive

Connecting $T_1$ and $T_2$, we can obtain (4.12) easily for sufficiently small $h$. □

Then we can obtain the following result from Lemma 4.1 and Theorem 4.1.

Theorem 4.2. Let $(y, p, u)$ and $(y_h^i, p_h^{i-1}, u_h^i)$ be the solutions of problems (2.5)–(2.7) and (4.1)–(4.5), respectively. Then there exists a constant $h_0 > 0$ such that for all $0 < h\leq h_0$, we have

5.   Numerical example

In this section, we give a numerical example to validate the error estimates for the control, state and adjoint state. We consider the bilinear parabolic optimal control problem:

where $\Omega = [0, 1]\times [0, 1]$, $J = (0, 1]$, and $U_{ad} = \{u: u\geq 0\}.$ The dual equation of the state equation is

Then we assume that

Firstly, we adopt the same mesh partition for the state and the control such that $\triangle t = h^{\frac{3}{2}}$ in our test. In this case, we investigate the convergence order for the solutions which compute on a series of uniformly triangular meshes. We present the $L^2(J; L^2)$, $L^\infty(J; L^2)$ and $L^\infty(J; L^2)$ errors for $u$, $y$ and $p$ in Table 1, which means that the convergent rates are $O(h^{\frac{3}{2}}+\triangle t)$. We show the convergence orders in Figure 3, where dofs denotes degree of freedoms. It is easy to see that this is consistent with the results proved in the previous.

In order to explore more on the rates of convergence separately in time and space, we try to validate the estimates by separating the discretization errors. We consider the behavior of the errors under refinement of the spatial triangulation for fixed $\triangle t = \frac{1}{80}$. Then, we show the errors for $u$, $y$, and $p$ in Table 2. Figure 4 depicts the convergence orders under refinement of the spatial triangulation for fixed $\triangle t = \frac{1}{80}$. We can observe the order is $O(h^{\frac{3}{2}})$.

Finally, we examine the behavior of the errors for a sequence of discretizations with decreasing size of the time steps and a fixed spatial triangulation with $h = \frac{1}{256}$. The $L^2(J; L^2)$, $L^\infty(J; L^2)$ and $L^\infty(J; L^2)$ error norms for control variable state variable and adjoin variable are shown in Table 3. In Figure 5, the convergence orders under refinement of the time steps for $h = \frac{1}{256}$ are shown. Up to the discretization errors it exhibits the proven convergence order $O(\triangle t)$. From the numerical results, we observe that convergence of order $O(\triangle t)$ which demonstrates our theoretical results.

Seen from the numerical results listed in Tables 1–3 and Figures 3–5, it is easy to find that the convergent orders match the theories derived in the previous sections.

6.   Conclusions

In this paper, we established semi-discrete and fully discrete finite volume element approximation scheme of bilinear parabolic optimal control problem. Then we used the finite volume element method to discretize the state and adjoint equations of the system. Under some reasonable assumptions, we obtained some error estimates. To our best knowledge in the context of optimal control problems, the priori error estimates of finite volume element method for bilinear parabolic optimal control problems are new.

In the future, we shall consider the finite volume element method for bilinear hyperbolic optimal control problems. Furthermore, we shall consider a priori error estimates and superconvergence of the finite volume element solutions for hyperbolic optimal control problems.

Use of AI tools declaration

The authors declare they have not used Artificial Intelligence (AI) tools in the creation of this article.

Acknowledgments

This work is supported by National Science Foundation of China (11201510), National Social Science Fund of China (19BGL190), Natural Science Foundation of Chongqing (CSTB2022NSCQ-MSX0286), Scientific and Technological Research Program of Chongqing Municipal Education Commission (KJZD-K202001201), Chongqing Key Laboratory of Water Environment Evolution and Pollution Control in Three Gorges Reservoir Area (WEPKL2018YB04), Research Center for Sustainable Development of Three Gorges Reservoir Area (2022sxxyjd01), Guangdong Basic and Applied Basic Research Foundation of Joint Fund Project (2021A1515111048), and Guangdong Province Characteristic Innovation Project (2021WTSCX120).

Conflict of interest

The authors declare that they have no competing interests.