New delay-range-dependent exponential stability criterion and $H_\infty$ performance for neutral-type nonlinear system with mixed time-varying delays

1.   Introduction

Neutral time-delay systems contain delays both in the state and in the derivatives of the state which can be found in various dynamic systems, such as chemical reactors, nuclear reactors, biological systems, economical systems, water pipes, population ecology, power systems, etc. ^{[1,2,3,4,5,6,7,8,9,10,11,12,13,14]}. On the other hand, nonlinear uncertainties are commonly encountered because it is very problematic to derive a certain mathematical model due to slowly varying parameters, environmental noise and so on. Stability criteria for time-delay systems are classified into two categories: delay-independent and delay-dependent. In general, the delay-dependent criteria are less conservative than the delay-independent ones, especially when the size of the delay is small. Therefore, many researchers have dedicated much effort to studying the delay-dependent stability criteria for neutral time-delay systems with nonlinear uncertainties in recent years; see, for instance, ^{[1,5,6,10,15,16,17,18]}.

The stability analysis of neutral-type systems is considered with various inequality techniques and Lyapunov approaches, which are significant to reduce conservatism. Therefore, many inequality techniques have been applied in the published literature to estimate the upper bound of the time derivative of the introduced Lyapunov-Krasovskii functional (LKF). In ^[1], the new stability conditions for the neutral delay differential system are derived by applying Jensen's integral inequality. In order to reduce the conservatism, Wirtinger's integral inequality was introduced in ^[19]. The free weighting matrices were utilized with a new integral inequality lemma in ^[6] to achieve less conservative results.

As pointed out in ^{[1,9,10,11,16,17,20]}, the exponential stability problem is also significant since it can determine the convergence rate of system states to equilibrium points. The problem of delay-dependent exponential stability criteria for neutral systems with nonlinear uncertainties have been investigated in ^[10,11,20]. Recently, many researchers have paid a lot of attention to the $H_{\infty}$ control problem in time-delay systems ^{[21,22,23,24]}. Li and Hu ^[25] studied the problem of $H_{\infty}$ control for neutral systems without nonlinear uncertainties. The $H_{\infty}$ control for uncertain neutral systems have been reported in ^[26]. The problem of $H_{\infty}$ performance for a neutral system with discrete, neutral and distributed time-varying delays and nonlinear uncertainties have been investigated in ^[19]. Their results are restricted on delay-independent criteria for neutral systems ^[25] or uncertain neutral systems without the condition of lower bounds of time-varying delays ^[19,26].

Motivated by the above statement, in this paper, the problem of $H_{\infty}$ performance and exponential stability analysis for a neutral system with interval discrete, neutral and distributed time-varying delays and nonlinear uncertainties are considered based on Jensen's integral inequality, the Wirtinger-based integral inequality, an extended Wirtinger's integral inequality, Peng-Park's integral inequality, the Leibniz-Newton formula, utilization of a zero equation, a decomposition matrix technique and the appropriate LKF. In the numerical part, we give some examples to present the effectiveness of the theorem. The main contributions and highlights of this paper are summarized in the following key points.

● We consider the problem of exponential stability for a neutral system with interval discrete, neutral and distributed time-varying delays and nonlinear uncertainties based on an $H_\infty$ performance condition. It is noted that this work is the first study of the exponential stability and $H_\infty$ performance for an uncertain neutral system with three (discrete, neutral, and distributed) interval time-varying delays.

● We construct the LKFs including single, double, and triple integral terms involving lower and upper bounds of time delays and use them to formulate a new delay-range-dependent stability criterion for a neutral system. In addition, the LKF consists of five new triple integral terms, i.e., $\frac{\lambda_{2}^{2}}{2}\int_{-\lambda_{2}}^{0}\int^{0}_{s}\int_{t+\theta}^{t}e^{2\kappa (u+\theta-t)}\dot{\varphi}^{T}(u)Z_{21}\dot{\varphi}(u)du d\theta ds$, $\lambda_{2}^{2}\int_{-\lambda_{2}}^{0}\int^{0}_{s}\int_{t+\theta}^{t}e^{2\kappa (u+\theta-t)}\dot{\varphi}^{T}(u)Z_{22}\dot{\varphi}(u) dud \theta ds$, $\int_{-\lambda_2}^0\int_{s}^{0}\int_{t+\theta}^{t}e^{\kappa(u+\theta -t)}\dot{\varphi}^T(u)Z_{23}\dot{\varphi}(u)dud\theta ds$, $\lambda_{1}^2\int_{-\lambda_{1}}^{0}\int^{0}_{s}\int_{t+\theta}^{t}e^{2\kappa (u+\theta-t)}\dot{\varphi}^{T}(u)Z_{24}\dot{\varphi}(u)du d\theta ds$ and $\frac{(\lambda_2^2-\lambda_1^2)}{2}\int_{-\lambda_{2}}^{-\lambda_1}\int^{0}_{s}\int_{t+\theta}^{t}e^{2\kappa (u+\theta-t)}\dot{\varphi}^{T}(u)Z_{25}\dot{\varphi}(u)du d\theta ds$, that were not used in ^[25,26].

● We apply tighter inequalities to improve the stability criterion, such as Jensen's integral inequality (Lemma 1) and extended single and double Wirtinger's integral inequalities (Lemmas 9 and 10). Using the above new LKFs and the lemmas leads to less conservatism of the obtained results than in published literature, as presented via numerical examples.

● We derive new delay-range-dependent sufficient conditions for the exponential stability with $H_{\infty}$ performance (Theorem 1). Moreover, we obtain the improved delay-range-dependent exponential stability criterion of a neutral system with discrete, neutral and distributed time-varying delays, and nonlinear uncertainties. The proposed conditions are less conservative than the other references as shown in Theorem 1.

● We present numerical examples to demonstrate the feasibility and effectiveness of the theorem.

The outline of this work is structured as follows. In Section 2, we give the problem statement, definitions and lemmas. We discuss some results for a neutral system and their proofs in Section 3. In Section 4, we give two numerical examples to present the effectiveness of the obtained criterion. Section 5 shows the conclusion of our results.

Notations: $\mathbb{R}^n$ denotes the $n-$dimensional Euclidean space, and $\mathbb{R}^{m\times n}$ is the set of all $m\times n$ real matrices. For a matrix $A$, $A > 0$ means that $A$ is a symmetric positive definite matrix and $\lambda_{\min}(P)$ and $\lambda_{\max}(P)$ denote the minimum and maximum eigenvalues of $A$, respectively. The superscript "$T$" denotes matrix transposition. $\operatorname{diag}\{\ldots\}$ denotes the block diagonal matrix. $\operatorname{Sym}\{A\} = A+A^T$.

2.   Problem formulation and preliminaries

We introduce the following neutral system with interval time-varying delays and nonlinear uncertainties of the form

where $\varphi(t)\in {\mathbb{R}}^{n}$ is the state of the system, $w(t)\in {\mathbb{R}}^{p}$ is the disturbance input which belongs to $L_2[0, \infty]$, $\chi(t)\in {\mathbb{R}}^{q}$ is the controlled output, $\phi(t)$ is the initial condition function that is continuously differentiable on $[- \max\{\lambda_2, \sigma_2, \rho_2\}, 0]$ with $\|\phi\| = \sup_{s\in[-\max\{\lambda_2, \sigma_2, \rho_2\}, 0]}\|\phi(s)\|$, $A_1$, $A_2$, $A_3$, $A_4$, $B$, $C_1$, $C_2$ and $D$ are real constant matrices with appropriate dimensions and $\lambda(t)$, $\sigma(t)$ and $\rho(t)$ are time-varying discrete, neutral and distributed delays, respectively. The delays satisfy the following conditions:

where $\sigma_1, \ \sigma_2, \ \sigma_d, \ \lambda_{1}, \ \lambda_{2}, \ \lambda_{d}, \ \rho_1, \ \rho_2$ and $\rho_d$ are positive real constants and $\zeta_1(t, \varphi(t))$, $\zeta_2(t, \varphi(t-\lambda(t)))$ and $\zeta_3(t, \dot{\varphi}(t-\sigma(t)))$ are nonlinear uncertainties that are assumed to satisfy the following inequalities

where $\eta_{1}, \eta_{2}$ and $\eta_{3}$ are known positive real constants. We consider the Leibniz-Newton formula of the form

In order to improve the discrete delay $\lambda(t)$ in (2.2), let us decompose the constant matrix $A_2$ as

where $H_1$, $H_2$ $\in \mathbb{R}^{n\times n}$ are real constant matrices. By (2.8) and (2.9), System (2.1) can be represented in the form

Remark 1. In System $(2.1)$, we assume that the delays in the discrete delay term and the distributed delay term are different but these two delay terms in ^[19] are the same.

Definition 1. ^[20] If there exist real positive scalars $\beta$ and $\kappa$ that satisfy

then System $(2.1)$ is exponentially stable.

Definition 2. ^[3] For a given real positive scalar $\delta$, we say that System $(2.1)$ is exponentially stable with the $H_{\infty}$ performance level $\delta$ if the system is exponentially stable and also satisfies ${\lVert \chi(t) \rVert}_{2} \leq \delta {\lVert w(t) \rVert}_{2}$, for all nonzero $w(t) \in L_2 [0, \infty)$ under the zero initial condition.

Lemma 1. (Jensen's inequality ^[19]). For any positive definite symmetric matrix $W\in R^{n\times n}$, $k_2$ is a positive scalar and the vector function ${\omega}:[-k_2, 0]\rightarrow \mathbb{R}^{n}$ such that the integrals concerned are well defined; the following inequality holds:

Lemma 2. ^[2] For any positive definite symmetric matrix $W\in R^{n\times n}$, $k_2$ is a positive scalar and the vector function $\dot{\omega}:[-k_2, 0]\rightarrow {R}^{n}$ such that the integrals concerned are well defined; then,

where

Lemma 3. ^[27] For any positive definite symmetric matrix $W\in \mathbb{R}^{n\times n}$, $k_1 < k_2$ are positive scalars and the vector function $\dot{\omega}:[-k_2, -k_1]\rightarrow \mathbb{R}^{n}$ such that the integrals concerned are well defined; then,

where

Lemma 4. ^[19] For any positive definite symmetric matrix $W\in \mathbb{R}^{n\times n}$, $k(t)$ is a time-varying delay with $0 < k_1 < k(t) < k_2$, $k_2\in\mathbb{R}$ and the vector function $\omega:[-k_2, -k_1]\rightarrow \mathbb{R}^{n}$ such that the integrals concerned are well defined; then,

where

Lemma 5. ^[19] For any constant matrices $Y_{1}, \ Y_{2}, \ Y_{3}\in \mathbb{R}^{n\times n}$, $Y_{1}\geq 0, \ Y_{3} > 0$, $\begin{bmatrix} Y_{1} & Y_{2} \\ * & Y_{3} \\ \end{bmatrix}\geq 0$, $k(t)$ is a time-varying delay with $0\leq k_1\leq k(t)\leq k_2$, $k_1, \ k_2\in \mathbb{R}$ and the vector function $\dot{\omega}:[-k_{2}, -k_1]\rightarrow \mathbb{R}^{n}$ such that the integrals concerned are well defined; then,

where

Lemma 6. ^[19] For any constant matrices $W, \ Y_{i}\in \mathbb{R}^{n\times n}$, $i = 4, 5, ..., 8$, $k(t)$ is a time-varying delay with $0\leq k_1\leq k(t)\leq k_2$, $k_2\in\mathbb{R}$ and the vector function $\dot{\omega}:[-k_2, -k_1]\rightarrow \mathbb{R}^n$ such that the integrals concerned are well defined; then,

where

Lemma 7. (Wirtinger-based integral inequality ^[28]). For any positive definite symmetric matrix $W\in \mathbb{R}^{n\times n}$, $k_1 < k_2$ are positive scalars and the vector function $\dot{\omega}:[-k_2, -k_1]\rightarrow \mathbb{R}^{n}$ such that the integrals concerned are well defined; then,

where

Lemma 8. (Peng-Park's integral inequality ^[29]). If $W$ and $S$ are real constant matrices such that $\begin{bmatrix} W & S \\ * & W \\ \end{bmatrix}\geq 0$, $k(t)$ is a time-varying delay with $0 < k(t) < k_2, \ k_2\in \mathbb{R}$ and the vector function $\dot{\omega}:[-k_2, 0]\rightarrow \mathbb{R}^{n}$ is well defined; then, the following inequality holds:

where

Lemma 9. (An extended Wirtinger's integral inequality ^[30]). For any positive definite symmetric matrix $W\in \mathbb{R}^{n\times n}$, $k_1$ and $k_2$ are positive scalars and the vector function $\omega:[k_1, k_2]\rightarrow \mathbb{R}^{n}$ such that the integrals concerned are well defined; then,

where

Lemma 10. ^[31] For any positive definite symmetric matrix $W\in \mathbb{R}^{n\times n}$, $k_1$ and $k_2$ are positive scalars and the vector function $\dot{\omega}:[k_1, k_2]\rightarrow \mathbb{R}^{n}$ such that the integrals concerned are well defined; then,

where

3.   Main results

We introduce the following notations for later use:

where

and the other terms are 0;

where $\widehat{\sum}_{i, j} = {\widehat{\sum}}^T_{i, j} = {\sum}_{i, j}, \ i, j = 1, 2, 3, ..., 27,$ except

and the other terms are 0.

Theorem 1. For a prescribed scalar $\delta > 0$, given positive scalars $\lambda_2$, $\sigma_2$, $\rho_2$, $\lambda_d$, $\sigma_d$ and $\rho_d$, the System (2.1) is exponentially stable for a decay rate $\kappa > 0$ with the $H_\infty$ performance $\delta$; if $\Vert A_3\Vert+\eta_3 < 1,$ there exist positive definite symmetric matrices $Z_i$, $i = 1, 2, ..., 25$, any appropriate dimensional matrices $S, Q_j, j = 1, 2, ..., 12$, $G_l, l = 1, 2, ...6$, and $F_k, k = 1, 2, ..., 10$, and positive real constants $\varepsilon_n,$ $n = 1, 2, 3,$ such that the following symmetric linear matrix inequalities hold

Proof. Under the condition of the theorem, we first show the exponential stability of System (2.10). Consider System (2.10) with $w(t) = 0$, that is,

Construct an LKF candidate for the System (2.10) of the form

where

wherein

The time derivative of $V(t)$ along the solution of (2.10) is given by

We compute $\dot{V}_1(t)$, $\dot{V}_2(t)$, $\dot{V}_3(t)$ and $\dot{V}_4(t)$ as

where

By Lemmas 3 and 4, we obtain $\dot{V}_{5}(t)$ and $\dot{V}_6(t)$ as follows

Applying Lemmas 6–9, we obtain

where

From Lemma 5, we compute $\dot{V}_8(t)$ as

where

Using Lemma 2, Lemma 3 and Lemma 10, $\dot{V}_9(t)$ can be estimated as follows

where

From (2.5)–(2.7), for any scalars ${\varepsilon_1}$, ${\varepsilon_2}$ and ${\varepsilon_3}$ that are positive real constants, it can be determinded that the following inequalities hold:

According to (3.10)–(3.13), it is straightforward to see that

where

If Conditions (3.3)–(3.8) and $\Sigma < 0$ hold, then

So, we have

where $M, \kappa\in R^+.$ This means that System (2.1) with $w(t) = 0$ is exponentially stable. Next, we shall establish the $H_{\infty}$ performance of System (2.1) under the zero initial condition. We now introduce

Under the zero initial condition, (3.15) becomes

where $V(t)$ is defined in (3.9). After some algebraic manipulations, we obtain

where

We can verify that the condition (3.8) guarantees $\chi^{T}(s)\chi(s)-\delta^{2}w^{T}(s)w(s)+\dot{V}(t)\leq0$. Therefore, $J(t) < 0$, which implies that ${\lVert \chi(t) \rVert}_{2} \leq \delta {\lVert w(t) \rVert}_{2}$ for any nonzero $w(t) \in L_{2}[0, \infty).$ The proof of the theorem is complete.

4.   Numerical examples

Example 1. Consider the uncertain neutral system (2.1) with the following parameters:

Decompose a matrix $A_2 = H_1+H_2$, where

Let the interval discrete time-varying delay be $\lambda(t) = |cos(t)|$ and the interval neutral and distributed time-varying delays be $\sigma(t) = \rho(t) = \sin^2(0.6t)$ for $t\in[-1, 0]$. By solving the linear matrix inequality (3.8) in Theorem 1, the maximum allowable upper bounds of $\rho_2$ for Example 1 are listed in Table 1 for various values of $\lambda_2$ and $\sigma_2$. We can see in Table 1 that the upper bound of the distributed delay $\rho_2$ has an effect on $\lambda_2$. For any given $\lambda_2$, $\sigma_2$ decreases as $\rho_2$ increases. Table 2 presents the maximum allowable upper bounds of $\lambda_2$ for Example 1 with different values of $\kappa$ and $\sigma_2$. It shows that all of the conditions stated in Theorem 1 have been satisfied; hence, System (2.1) with the above given parameters has exponential stability with $H_\infty$ performance.

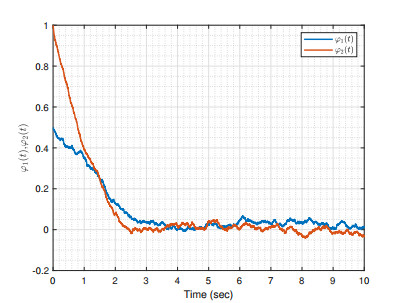
For the initial condition $\phi(t) = [0.5\quad 1]^T$, $\zeta_1 (t, \varphi(t)) = \eta_1\varphi(t)sin(\varphi(t))$, $\zeta_2 (t, \varphi(t - \lambda(t))) = \eta_2sin(\varphi(t - \lambda(t)))e^{-2.3\varphi(t - \lambda(t))}cos(\varphi(t - \lambda(t)))$ and $\zeta_3 (t, \dot{\varphi}(t - \rho(t))) = \eta_3\dot{\varphi}(t - \rho(t))cos(t)$. Figure 1 shows the trajectories of the solution $\varphi^{T}(t) = [\varphi_1(t), \varphi_2(t)]$ of the neutral system (2.1) with mixed time-varying delays and nonlinear uncertainties.

Example 2. Consider the following neutral system with $w(t) = 0,$ $C_1 = C_2 = \begin{bmatrix} 0 & 0 \\ 0 & 0 \\ \end{bmatrix}:$

when $\eta_1 = 0.1$, $\eta_2 = \eta_3 = 0.05,$ $\lambda_d = 0.7,$ $\rho_1 = 0.3,$ $\rho_2 = \rho_d = 0.4$ $\sigma_1 = 0.3,$ $\sigma_2 = 0.5$ and $\sigma_d = 0.1.$ We separate a matrix $A_2$ as $A_2 = H_1+H_2$, where

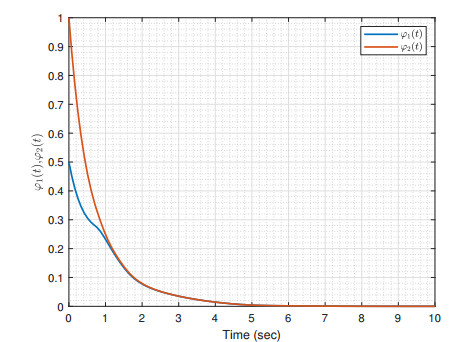
Table 3 shows a comparison of the upper bounds for the exponential stability of System (2.1) by different methods. It can be concluded that our results are less conservative than those in ^[11]. Figure 2 demonstrates the trajectories of the solution $\varphi_1(t)$ and $\varphi_2(t)$ of the uncertain neutral system (2.1) with mixed time-varying delays when $w(t) = 0$.

Example 3. Consider the System (2.1) with the following parameters:

Decompose a matrix $A_2 = H_1+H_2$, where

Using the Matlab LMI toolbox, we obtain the maximum allowable upper bounds of $\lambda_2$, which are listed in Table 4. Table 4 describes the maximum allowable upper bounds of delays that guarantee the asymptotic stability of System (2.1) when $\kappa = 0$. It is clear that the obtained results in this study are better than those in ^{[7,10,32,33,34]}.

Remark 2. The less conservatism of Theorem 1 benefits from the construction of new LKFs with the application of Jensen's integral inequality (Lemma 1), Peng-Park's integral inequality (Lemma 8) and extended Wirtinger's integral inequalities (Lemmas 9 and 10). These allowed our maximum delay to be greater than those in ^{[7,10,11,32,33,34]} as shown in Tables 3 and 4.

5.   Conclusions

In this article, the problem of exponential stability and $H_\infty$ performance with mixed discrete, neutral and distributed interval time-varying delays and nonlinear uncertainties has been studied. To obtain delay-range-dependent sufficient conditions that can be achieved in the form of linear matrix inequalities for the $H_\infty$ performance with exponential stability of the system, we have introduced an appropriate LKF and applied a decomposition matrix technique, the Leibniz-Newton formula, a zero equation, Peng-Park's integral inequality, Jensen's integral inequality and the Wirtinger-based integral inequality. Numerical examples have been provided to verify the effectiveness of the presented results, showing that our results are better than the existing results.

Acknowledgments

This work was supported by the Research and Graduate Studies Program, Khon Kaen University (Research Program, grant number RP65-8-004).

Conflict of interest

The authors declare that there are no conflicts of interest regarding the publication of this manuscript.