Finite-time stability of singular switched systems with a time-varying delay based on an event-triggered mechanism

1.   Introduction

Switched systems consist of switched signals and subsystems. Over the past decades, much research has been carried out on switched systems because of the widespread existence of such systems, such as power systems and control systems, in real life ^[1,2]. This form also allows these systems to represent more realistic situations when modelling systems. In some publications, singular systems are sometimes known as systems with generalized variables, systems of general state spaces ^[3], etc. A system with multiple subsystems, where at least one of the subsystems is singular, is called a switched singular system ^[4]. When studying singular switched systems, the distinction between dealing with standard switched systems and dealing with singular switched systems is the importance of paying attention to the compatibility of the system states before and after the switching moment. If the conditions that a switched system has only one solution are to be satisfied, they must be regular and impulse-free and should be met for each subsystem ^[5,6].

The study of stability is always an important topic. Current research has more often studied the Lyapunov asymptotic stability of systems, that is, the dynamic behavior of systems in an infinite time interval ^[7]. For the study of stability, scholars have used the Lyapunov function to solve the exponential stability problem of some continuous systems ^[8,9], the state decomposition method to solve the stability problem of some discrete systems ^[10,11,12] and multiple Lyapunov functions to give sufficient criteria for the exponential stability of some stochastic systems ^[6,13,14]. In nonlinear systems, finite-time stability (FTS) is possible with an arbitrary switched law, and conditions for availability of dynamic characteristics like stability, attraction, invariance, and boundedness were formulated in terms of the common Lyapunov functions or multiple homomorphisms ^[15].

In practice, however, it is often important to investigate a system's transient performance across short time intervals. In general, if the overshoot is too large, then this control system is not applicable in many practical engineering applications, so it becomes necessary to study the FTS of the system. When the starting value of a system deviates by a certain margin from the equilibrium point, the FTS may be understood as the state of systems during a specified time interval. In recent years, FTS has received increasing attention from scholars at home and abroad, and many research results have been achieved ^[16]. To ensure the FTS of a system, different control methods have been proposed by domestic and foreign scholars for different systems, among which the method of average residence time is widely utilized ^[17,18,19]. Furthermore, Ma et al. studied the finite-time feedback control issue of linear systems controlled by the switched law that has a time-varying delay and parametrically limited external perturbations using an event-triggered mechanism ^[20], but they did not address singular systems. However, the method of averaging the residence time also has some limitations, so there is a need to find other methods in the study of FTS. For example, using finite-time escape functions ^[21], the average impulse interval (AII) method ^[22], state space partitioning to construct switched laws ^[23,24] and an event-triggered mechanism ^[25], the system studied in this paper does not contain a time-delay term.

Recently, the advantages of event-triggered mechanisms have been gradually recognized by scholars ^[26,27,28]. Furthermore, the idea of constructing switched laws using a division of the state space was applied for the first time to singular switched systems with time-varying delays, which makes the determination of state switched easy ^[23,24]. Real-time feedback adds considerable computational burden to a system. In an event-triggered control structure, a new control mission can only be completed when an external event is satisfied. Given an external event, a defined event-triggered mechanism is generated, and data collection is not performed at a fixed time, as in traditional periodic collection methods. At the moment of sampling, the control input to the system changes instantaneously. As a consequence, the time required for control mission runs and sensor-controller communication frequency may be greatly lowered while still providing good closed-loop performance.

In the literature, event-triggered mechanisms have not been applied to time-varying delay singular switched systems. In this process, the construction of the V-function directly affects the ease of judging the system's admissibility conditions and is a necessary consideration when considering singular systems, and therefore the construction of the V-function has some degree of limitation. In summary, the previous studies ^[20,23,25] had issues that have not been considered, including variable time lag terms and singular systems. In the literature ^[29], singular systems with time-delay terms are considered, but there is no switched and no perturbation in the system. This motivates us to carry out the present work, and this paper considers both of these issues. Therefore, our contributions are given as follows: (1) The control method for the FTS of singular switched systems with time-varying delay and perturbations is investigated. (2) Sufficient requirements are also presented for meeting the finite-time stable $H_\infty$-performance index $\gamma$.

In this paper, finite-time stabilization of continuously singular switched systems that have a time-varying delay and an issue of state feedback control are explored. First, the conditions for the existence of unique solutions of continuously singular switched systems are discussed, and the definitions of compatible switched and finite-time stabilization of normal delay singular switched systems are given. Second, sufficient conditions for FTS with perturbation cases are given using the L-K function and state-space decomposition methods. Finally, sufficient conditions for satisfying the finite-time stable $H_\infty$ performance index $\gamma$ are given. Ultimately, the applicability of the theory is verified by numerical arithmetic examples.

2.   Preliminary

$\mathbb{N}$ and $\mathbb{R}^+$ stand for the set of all natural numbers and the set of positive real numbers, $\mathbb{R}^n$ represents the set of all column vectors with $n$ real components, where $\left\| x\right\| = \sqrt{x^{\top}x}$. $\mathbb{R}^{n \times n}$ is the set of all real $n \times n$ matrices, $I_r$ is a unit matrix of order $r$, $A{^\top}$ is the transpose of $A$, $\left\| A\right\| = \sqrt{\lambda_{max}(A^{\top}A)}$ and $\lambda_{max}(A^{\top}A)$ denotes the maximum eigenvalue of the matrix $A^{\top}A$. $C([a, b], \mathbb{R}^n)$ denotes all of the continuous functions satisfying $[a, b]\to \mathbb{R}^n$, $A\ge 0$ means $A$ is a semipositive definite matrix, and $> 0$ means a matrix is a positive definite matrix. In the same way, $A\ge B$ means $A-B\ge 0$. $r\left(E \right)$ stands for $rank\left(E \right)$.

On the basis of the literature ^{[20,23,25,29]}, we have added time-delay terms and perturbation terms and have written the system as a more general switched singular system; then, the following class of delay continuously singular switched systems is considered:

where system state $x(t) \in \mathbb{R}^n$, control input $u(t) \in \mathbb{R}^q$ is a piecewise constant vector, and control output $z(t) \in \mathbb{R}^p$. The disturbance $w(t) \in \mathbb{R}^m, t\in [0, +\infty)$, is a continuous function caused by external circumstances. $\tau(t)$ is the time-varying delay. The complexity of studying singular systems is largely a consequence of the fact that not every initial state $\phi(t)$ has a solution ^[3]. Here, $\phi (t) \in C([a, b], \mathbb{R}^n)$ is a compatible initial condition. The switched signal $\sigma(x(t)): \mathbb{R}^{+} \to M = \left\lbrace 1, 2, \dots, p\right\rbrace$ is a segmented constant value function, which is dependent on the system's state and takes values in the finite set $M = \left\lbrace 1, ..., p\right\rbrace$. Define $\sigma(x(t)) = l$.

$A_l\in \mathbb{R}^{n\times n}, B_l\in \mathbb{R}^{n\times m}, D_l\in \mathbb{R}^{n\times n}, F_l\in \mathbb{R}^{n\times q}, V_l\in \mathbb{R}^{p\times n}, N_l\in \mathbb{R}^{p\times p} (l \in M)$ are constant matrices.

Some assumptions are given.

(A1) $E\in \mathbb{R}^{n\times n}$ is a singular matrix $E$ satisfying $r(E) < n$.

(A2) For all $t\in [0, T]$ satisfying

where $d \ge 0, T > 0$.

(A3) $\tau(t)$ satisfies $0 < \tau_1\le \tau(t)\le \tau_2$ and $\dot{\tau}(t)\le \rho < 1$, where $\tau_1$, $\tau_2$ and $\rho$ are constants, and $\dot{\tau}(t)$ represents the derivative of $\tau(t)$.

To design a proper controller, some necessary definitions, lemmas and assumptions are given as follows.

Definition 1. ^[30] For any two matrices $E, A\in \mathbb{R}^{n\times n}$, if we can find a constant scalar $\alpha \in C$ such that $det(\alpha E+A)\neq 0$ or for $s\in C$ polynomials $det(sE-A)\not \equiv 0$, we say that the matrix pair $(E, A)$ is regular.

Definition 2. ^[29,31] For any two matrices $E, A\in \mathbb{R}^{n\times n}$, the matrix pair $(E, A)$ is impulse-free if $deg(det(sE-A)) = r(E)$.

Remark 1. Sometimes the term "quite regular" is used instead of "impulse-free". The regularity is satisfied for any general system described by differential equations. However, for singular systems, regularity is not necessarily satisfied. If a singular system is irregular, it means that the system may not be unique; and if a singular system is regular, as shown in reference ^[32], it means that the solution of the system is either unique or infinite, which is caused by impulses. In this case, a nonimpulsive singular system does not have an infinite solution. Therefore, a regular and impulse-free system is the basic requirement to ensure the existence and uniqueness of the solution.

Definition 3. ^[29] If the requirement of matrix pair $(E, A_l)$ is regular, we say the singular system (2.1) that has a time delay is regular.

Definition 4. ^[33] The system is impulse-free if the requirement that there is no discontinuous solution of the system when the external input $u(t) = 0$ for any initial value is satisfied.

Lemma 1. ^[4] For the matrices $E, A$ in System (2.1), there always exist reversible matrices $M, G$ such that $MEG = \left({\begin{array}{*{20}{c}} I_r & 0\\ 0 & 0 \end{array}} \right)$, $MAG = \left({\begin{array}{*{20}{c}} A_{11} & A_{12}\\ A_{21} & A_{22} \end{array}} \right)$, where $A_{11}\in \mathbb{R}^{r \times r}$, $r = rank(E)$. At this point, the matrix pair $(E, A)$ can be regarded as regular and impulse-free when and only when $A_{22}$ is a nonsingular matrix.

Definition 5. ^[18] In a switched singular system, a switch is said to be compatible if the subsystem's state at the moment of a new subsystem to be engaged is compatible.

Definition 6. ^[18] For singular switched systems, a system is regarded as regular and impulse-free if all subsystems are regular and impulse-free under compatible switching.

Here, an event-triggered mechanism is introduced.

where $e(t) = {x}({t}_i)-x(t)$ and $\eta > 0$ is a constant. If event (2.2) is triggered, data collection occurs immediately, and the state information is then fed back to the controller. Denote the sequence of event triggering moments as $\left\lbrace{t}_i\right\rbrace ^{\infty}_0$, where ${t}_i < {t}_{i+1}$. Let the moment of the first collection of information be $t = 0$. From this, the moment of the next collection of information after moment $t_i$ can be defined as $t_{i+1} = \rm{int}\left\lbrace t > t_i:\left\| e(t)\right\| ^2\ge \eta \left\| x(t)\right\|^2\right\rbrace$. Suppose that the event is triggered $m$ times within $\left[ t_k, t_{k+1} \right)$. Based on such a collection mechanism, it is possible to design a state feedback controller shaped like

where $K_l$ is the controller gain matrix. At acquisition time ${t}_i$, the controller receives state information ${x}({t}_i)$ and holds it until the next acquisition time ${t}_{i+1}$ so that the controller input is updated at the sampling moment. The control input $u(t)$ exists in the interval $[0, T]$.

Assumption 1. It is assumed that no Zeno behavior occurs during event-triggered data collection.

Assumption 2. It is assumed that all switches in the system are compatible switches.

System (2.1) combined with feedback controller (2.3) forms a closed loop system as follows.

For switched singular system (2.4), some definitions of the system are shown.

Definition 7. ^[23,34] For the given positive numbers $T$, $c_1$ and $c_2$, symmetric matrix $Q\in \mathbb{R}^{n \times n}$ is positive definite, and System (2.4) is finite-time stable under $\sigma(x(t))$ for the corresponding $(c_1, c_2, T, Q)$ if System (2.4) is regular and impulse-free and for every $x_ {\sigma}(t, \phi)$,

is satisfied.

Definition 8. ^[19] A singular switched system based on an event-triggered mechanism is said to satisfy a finite-time $H_{\infty}$ performance index $\gamma$ about a given $(0, c_2, d, l, Q, T, \sigma)$, where $c_2 > 0, T > 0, \gamma > 0$, $Q$ is positive definite, and $\sigma(t)$ is a switched signal, if

(1) System (2.4) is finite-time stable, and

(2) under the initial condition $\Phi(t) = 0, \forall t\in \left[ -\tau_2, 0\right)$, control output $z(t)$ satisfies

The goal of this research is to select a set of switched laws $\sigma(x(t))$ that enable a system to remain finite-time stable under the actions of $\sigma(x(t))$. The switched law for System (2.4) is built by partitioning the state space on a convex cone in such a way that each system mode is engaged in a specific cone area with a certain negative quadratic function in each subregion.

Definition 9. ^[23] We can say that the matrix $\left\lbrace L_i\right\rbrace _{i = 1}^{p}$ is strictly complete if there exists $i$ such that $x^TL_ix < 0$, $\forall x \in \mathbb{R}^n/\left\lbrace 0\right\rbrace$.

It is easy to see that the matrix $\left\lbrace L_i\right\rbrace _{i = 1}^{p}$ is strictly complete if and only if $\Omega_i = \left\lbrace x\in \mathbb{R}^n:x^TL_ix < 0\right\rbrace, i = 1, \dots, p$, satisfies $\bigcup_{i = 1}^{p}\Omega_i = \mathbb{R}^n/\left\lbrace 0\right\rbrace$.

Lemma 2. ^[23] The matrix $\left\lbrace L_i\right\rbrace _{i = 1}^{p}$ is strictly complete if we can find a scalar $\xi_i\ge 0, i = 1, \dots, p, \sum_{i = 1}^{p}\xi_i > 0$ satisfying $\sum_{i = 1}^{p}\xi_iL_i > 0$.

Lemma 3. (Schur complementary lemma) For a given constant matrix $A, B, C, B = B^{\top}$, we have $A+C^{\top}B^{\top}C < 0\Leftrightarrow \left({\begin{array}{*{20}{c}} A & C^{\top} \\ C & -B \end{array}} \right) < 0$.

Lemma 4. (Cauchy matrix inequality) For any positive define matrix $N \in \mathbb{R}^{n\times n}$, we have $2y^{\top}x\le x^{\top}Nx+y^{\top}N^{-1}y$.

Lemma 5. ^[30] The matrix pair $(E, A)$ is regular iff there exist reversible matrices $M_1$ and $G_1$ satisfying

where $n_1+n_2 = n$, $J\in \mathbb{R}^{n_1\times n_1}$, $N\in \mathbb{R}^{n_2\times n_2}$, and $N$ is the nilpotent matrix.

3.   Main results

This section first gives conditions for determining that a system is regular and impulse-free using the coefficient matrix of the system. Next, we explore sufficient criteria for the FTS of a singular switched system with time-varying delays based on the state space decomposition approach.

Theorem 1 is obtained by a similar method to that used in ^[35,36].

Theorem 1. Under Assumption 2, for any switched law $\sigma(x(t))$, System $(2.1)$ is regular and impulse-free, and the solution exists uniquely if matrix pair $(E, A_l)$ is regular and impulse-free for any $l \in M$ under compatible initial conditions.

Proof. All we have to do now is show that any subsystem is impulse-free.

Without loss of generality, we next consider the system

where $A\in \mathbb{R}^{n \times n}$, $B\in \mathbb{R}^{n \times m}$ and $D\in \mathbb{R}^{n \times n}$ are constant matrices.

Here, $x = G_1\tilde{x}$, $x$ is replaced with $G_1\tilde{x}$, and $M_1$ is multiplied on the left-hand side of the first equation to obtain

For simplicity, $\tilde{x}$ is still written as $x$, $M_1DG_1 = \left({\begin{array}{*{20}{c}} {{D}_{11}} & D{}_{12} \\ {{D}_{21}} & {{D}_{22}} \\ \end{array}} \right)$, $M_1B = \left({\begin{array}{*{20}{c}} {{B}_{1}} \\ {{B}_{2}} \\ \end{array}} \right)$, and the above equation can be written as two equations:

Let $x_1\in \mathbb{R}^{n_1}$, $x_2\in \mathbb{R}^{n_2}$. By Lemma 5, $N$ is a nilpotent matrix. Since $M_1(sE-A)G_1 = diag(sI-J, sN-I)$, $deg(det(sE-A)) = deg(det(sI-J)) = n_1$.

If $deg(det(sE-A)) = r(E)$ is satisfied, then the value of $deg(det(sI-J))$ is equal to $r(E)$ and equal to $r(I)+r(N)$. Therefore, $r(N) = 0$ is obtained, i.e., $N = 0$.

Since $0 = x_2(t)+D_{21}x_1(t-\tau(t))+D_{22}x_2(t-\tau(t))+{B_2w(t)}$, $x_2(t)$ is a continuous function on $[-\tau_2, \infty)$. By substituting $x_2(t)$ into the first equation and Definition 4, it is easy to see that a solution of Eq (3.1) is impulse-free and unique, considering that the first equation is a generalized differential equation with respect to $t, x_1$.

In the following proof process, the constructed L-K function $V(x(t))$ is shortened to $V(t)$ for brevity.

By Lemma 1, let $A_{l}+F_{ l}K_{l} = \bar{A_l}$ and $F_{l}K_{l} = \bar{F_{l}}$; then, we have

Under the state transformations $y(t) = G^{-1} x(t)$, new state $y(t)^{\top}$ can be written as $\left(y_{1}(t)^{\top}, y_{2}(t)^{\top}\right)$, where $y_{1}(t)$ has $r$ dimensions, $y_{2}(t)$ has $n-r$ dimensions, and $\sigma(x(t)) = l$; hence, System (2.4) can be written as

Remark 2. From the above equations, it can be seen that the initial conditions for such systems need to satisfy the following requirements: Let the initial condition be $x(t) = \phi(t)$; then, we have $y(t) = G^{-1} \phi(t),$ for $t \in[-\tau_2, 0]$. $y(0)$ needs to satisfy $0 = A_{21}^{l} y_{1}(0)+A_{22}^{l} y_{2}(0)+D_{21}^{l} y_{1}(-\tau(0))+D_{22}^{l} y_{2}(-\tau(0))+B_{2}^{l} w(0)$.

Due to incompatible initial conditions, switched singular time-delay system (2.4) may exhibit discontinuities at switched points. To ensure the continuity of states, the requirements of $0 = A_{21}^{l}{{y}_{1}}(0)+A_{22}^{l}{{y}_{2}}(0)+D_{21}^{l}{{y}_{1}}(-\tau (0))+D_{22}^{l}{{y}_{2}}(-\tau (0))+B_{2}^{l}w(0)$ and Assumption 2 must be met at switched points. Under Assumption 2, switched points in these systems happen when the trajectories cross the compatibility space of the new subsystems and then the switched case system's trajectories are continuous everywhere ^[37].

Some notations are given for the sake of simplicity.

Theorem 2. Assume that ($A1$–$A3$) hold. For $(c_1, c_2, T, Q)$, $c_1, c_2, T$ are given constants, and symmetric matrix $Q\in \mathbb{R}^{n\times n}$ is positive definite. For any $l, l = 1, ..., p$, if a nonsingular matrix $P$, positive definite matrices $Q_1 > 0$, $Q_2 > 0$, $Q_4 > 0$, and $Q_3\ge 0$; scalars $\xi_l \ge 0, l = 1, ..., p, \sum_{l = 1}^{p} = \xi_l > 0$; and the constant $\beta > 0$ can be found such that the conditions

are satisfied then switched singular system $(4)$ has FTS with respect to $(c_1, c_2, T, Q)$ under the action of the controller ${{u}_{l}}(t), l = 1, ..., p$.

Proof. First, we prove the regular and impulse-free nature of the System (2.4).

Write ${{G}^{\bot }}P{{M}^{-1}} = \left({\begin{array}{*{20}{c}} {{P}_{11}} & {{P}_{12}} \\ {{P}_{21}} & {{P}_{22}} \\ \end{array}} \right)$, where $P_{11}$ is an $r(E)$-order matrix. Note that

Because of Eq (3.3), $P_{21} = 0$, and $P_{11} = P_{11}^{\top} > 0$. It follows from Eq (3.5) that $0.5\bar{A_l}^{\top}P^{\top}+0.5P\bar{A_l}-\beta PE < -(1-\rho)^{-1}(Q_1+Q_3) < 0$. Therefore, we have

Considering the block matrix in the lower right-hand corner, we can see that $P_{22}A_{22}+P_{22}^{\top}A_{22}^{\top} < 0$, so $det(A_{22})\neq0$, and matrix pair $(E, A_l)$ is regular and impulse-free by ^[4]. By Theorem 1, System (2.4) is regular and impulse-free.

Next, System $(4)$ is proven to be finite-time stable.

Finally, it can be seen from references ^[25,38,39] that the L-K function can be a piecewise function or a continuous function. The construction method in this paper is the same as that in the literature ^[34,38], which also introduces an event-triggered mechanism to the switched system. We adopt a continuous L-K function to reduce the complexity of the calculation of the method because $P$ in the following function does not need to change, as the subsystem changes, and the control input $u(t)$ changes. The L-K function is constructed as

where $V_1(x_t) = x^T(t)PEx(t)$, $V_2(x_t) = \frac{1}{1-\rho}\int_{t-\tau(t)}^{t}x^T(s)Q_1x(s)ds$, and $V_3(x_t) = \frac{1}{1-\rho}\int_{t-\tau(t)}^{t}x^T(s)Q_3x(s)ds$. The derivative of V in terms of time t is

Because of Lemma 4, we have

From the condition $\left\| e(t)\right\| ^2\le \eta \left\| x(t)\right\|^2$, we have that

where $L_l = 0.5P\bar{A_l}+0.5\bar{A_l}^{\top}P^{\top}+\eta Q_4$ and $C_l = 0.5P\bar{A_l}+0.5\bar{A_l}^{\top}P^{\top}-\beta PE+P\bar{F_l}Q_4^{-1}$$\bar{F_l}^{\top}P^{\top}+PD_lQ_1^{-1}D_l^{\top}P^{\top} +PB_lQ_2^{-1}B_l^{\top}P^{\top})x(t)+(1-\rho)^{-1}x^{\top}(t)(Q_1+Q_3)x(t)$.

It follows from Schur complements that $C_l < 0$ iff Eq (3.5) holds.

Due to condition Eq (3.6), the matrix $\left\lbrace L_i\right\rbrace _{i = 1}^{p}$ is strictly complete. Thus, we have $\bigcup_{i = 1}^{p}\Omega_i = \mathbb{R}^n/\left\lbrace 0\right\rbrace$ and $\bigcup_{l = 1}^{p} \bar{\Omega}_{l} = \mathbb{R}^{n}$; for any $l_{1} \neq l_{2}$, we have $\bar{\Omega}_{l_{1}} \cap \bar{\Omega}_{l_{2}} = \emptyset$. Thus, for any $x(t)$, there is only one $l\in \left\lbrace 1, 2, ..., p\right\rbrace$, and there is

Therefore, it follows from Eq (3.5) and Eq (3.6) that

By multiplying both sides by $e^{-\beta t}$ and integrating both sides simultaneously from 0 to t, we obtain

Thus, $V(x_t)\le e^{\beta t}[V(x_0)+\lambda_{max}(Q_2)dt], \; \; \forall t\in [0, T]$.

It follows from Eq (9), Eq (10) and Eq (15) of reference ^[34] that

provided that $\sup\limits_{t \in [-\tau_2, 0]}\phi^{\top}(t)Q\phi(t) < c_1$.

Note that

which implies that

Next, we estimate $\left\| y_2(t)\right\|$,

From inequality (3.10),

When $t \in [0, \tau_1]$, it follows from $(A3)$ that $t-\tau(t)\in [\tau_2, 0]$. This indicates that

i.e., $(1-\sqrt{\eta}\left\| (A_{22}^{l})^{-1}\bar{F^l_2}\right\| \left\| G\right\|)\left\| y_2(t)\right\|\le \gamma \sqrt{\frac{e^{\beta T}\alpha_{3}}{\lambda_{min}(Q_{11})}}+$$\left\| (A_{22}^{l})^{-1}D_{22}^{l}\right\|\left\| G^{-1}\right\|\sqrt{\frac{c_1}{\lambda_{min}(Q)}}+\left\| (A_{22}^{l})^{-1}B_{2}^{l}\right\|\sqrt{d}$. Therefore, $\left\| y_2(t)\right\|\le \gamma_{1}\sqrt{\frac{e^{\beta T}\alpha_{3}}{\lambda_{min}(Q_{11})}}+\gamma_{2}\left\| G^{-1}\right\|\sqrt{\frac{c_1}{\lambda_{min}(Q)}}+\gamma_{3}\sqrt{d}$.

When $t\in [\tau_1, 2\tau_1]$, it follows that $t-\tau(t)\in [\tau_1-\tau_2, \tau_1]$. There are two cases. First, when $t-\tau(t)\in [\tau_1-\tau_2, 0]$, we have

when $t-\tau(t)\in [0, \tau_1]$, we have

i.e.,

Thus, we have

By induction, for all $t\in [(k-1)\tau_1, k\tau_1], k\in \mathbb{Z_+}$,

holds, which implies that

Finally, by (3.7) and (3.12), for any $0 \le t\le T$, we have

The proof has been completed.

Theorem 3. Assume that $(A1-A3)$ hold. For $(c_1, c_2, T, Q)$, $c_1, c_2, T$ are given constants, and symmetric matrix $Q\in \mathbb{R}^{n\times n}$ is positive definite. For any $l, l = 1, ..., p$, if a nonsingular matrix $P$; positive definite matrices $Q_1 > 0$, $Q_2 > 0$, $Q_4 > 0$, and $Q_3\ge0$; scalars $\xi_l \ge 0, l = 1, ..., p, \sum_{l = 1}^{p} = \xi_l > 0$; and the constant $\beta > 0$ can be found such that the conditions

are satisfied then switched singular system $(4)$ has FTS with respect to $(c_1, c_2, T, Q)$ under the action of the controller ${{u}_{l}}(t), l = 1, ..., p$, where $K_l = F_l^{-1}P^{-1}$.

Proof. Here, $\bar{A_l}$ in Theorem 2 is replaced by $A_{l}+F_{l}K_{l}$, and $\bar{F_l}$ in Theorem 2 is replaced by $F_{l}K_{l}$. Considering $K_l = F_l^{-1}P^{-1}$, it follows from Theorem 2 that System (2.4) has FTS.

Corollary 1. For System (2.4), provided the conditions of Theorem 3 are satisfied, if $V_l, N_l$ is required to satisfy

System (2.4) satisfies the finite-time $H_\infty$ performance metric $\gamma$, where $\gamma = \sqrt{\lambda_{max}(Q_1+Q_3)}$.

Proof. The following shows that System (2.4) has a finite-time $H_\infty$ performance index $\gamma$. The L-K function $V(x_t) = V_1(x_t)+V_2(x_t)+V_3(x_t)$ is chosen.

The proof of Theorem 3.2 and the conditions give $\dot{V}(x_t)\le \beta V(t)+w^{\top}(x)(Q_1+Q_3)w(t)-z^{\top}(t)z(t)+ \lambda_{max}(Q_2)d$.

Integrating both sides simultaneously from 0 to $\tau_2$ gives

so we have $V(\tau_2)\le \lambda_{max}(Q_2) d\tau_2e^{\beta\tau_2}+\int_{0}^{\tau_2}e^{\beta (\tau_2-s)}\Gamma(s)ds$. By induction, we can obtain that

where $\Gamma(s) = w^{\top}(s)(Q_1+Q_3)w(s)-z^{\top}(t)z(t)$.

By letting $\int_{[\frac{t}{\tau_2}]\tau_2}^{t}\dot{V}(s)ds = \int_{j\tau_2}^{t}\dot{V}(s)ds$, we have

By calculation, we obtain

Substituting (3.19) into (3.20) yields

Under the condition that the initial state is 0, since $V(t)\ge 0$, when $t = 0$ we have

The theorem is proved.

4.   Numerical simulation

To test the applicability and effectiveness of the proposed strategy, two examples are presented in this section.

Example 1. Consider system $(2.4)$ with the switched signal $\sigma(t) = 1, 2$, where

Then,

which satisfy $MEG = \left({\begin{array}{*{20}{c}} I_r & 0\\ 0 & 0 \end{array}} \right)$ are chosen.

The initial value is $\phi(t) = (0\; 0)^{\top}$ for $t\in[-0.15, 0]$. The continuous external perturbation is $w(t) = (0.01{\rm sin}(0.1t)\; 0)^{\top}$. For given $c_1 = 0.0001, \; c_2 = 2.1, \; T = 1, \; Q = \left({\begin{array}{*{20}{c}}6 & 0 \\ 0 & 0.4\end{array}} \right)$, $\eta = 0.02, \; \tau(t) = 0.02{\rm sin}(t)+0.13, \; d = 0.000005, \; \rho = 0.5, \; \tau_1 = 0.11, \; \tau_2 = 0.15$, we have the following results.

We can obtain $\beta = 1.1$ and $\bar{P} = \left({\begin{array}{*{20}{c}}1.3285 & 0.5500\\0.3321 & 0.8875\end{array}} \right)$.

In this case, we can obtain by calculation that

The matrix system ${L_1, L_2}$ is therefore strictly complete.

The division of the state space is performed as follows:

Then, the switched law is

In addition, it is possible to verify that $\bar{P}E = E^{\top}P^{\top} = \left({\begin{array}{*{20}{c}} 5.3140 & 1.3285\\ 1.3285 & 0.3321 \end{array}} \right)\ge 0$.

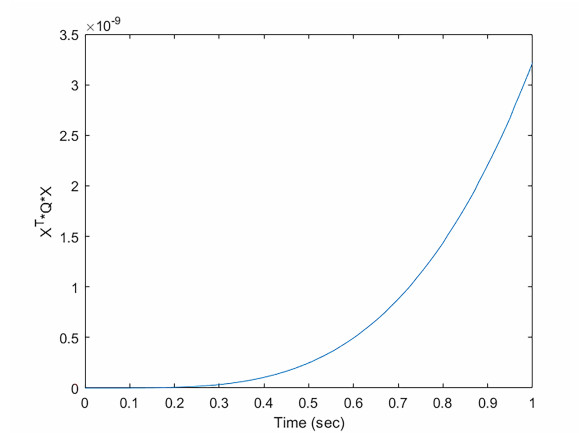
Figure 1 gives information about state response $x^{\top}Qx$ for the initial conditions, and Figure 2 shows the variation in the switched signal with time. The system state of the two-dimensional system can be seen in Figure 3. From Definition 7, the system has FTS for the corresponding $(0.001, 2.1, 5, Q)$ under the switched law $\sigma(x(t)) = l$. Figure 4 shows the division of the state space corresponding to the different subsystems.

Example 2. Consider system $(2.4)$ with the switched signal $\sigma(t) = 1, 2$, where

Then,

are chosen and satisfy $MEG = \left({\begin{array}{*{20}{c}} I_r & 0\\ 0 & 0 \end{array}} \right)$. The initial value is $\phi(t) = (0.001\; 0.01)^{\top}{\rm sin}(\pi t)$ for $t\in[-0.21, 0]$. The continuous external perturbation is $w(t) = (0.01{\rm sin}(0.1t)\; 0)^{\top}$. For a given $c_1 = 0.001, \; c_2 = 3.7, \; T = 3, \; Q = \left({\begin{array}{*{20}{c}}6 & 0 \\ 0 & 0.6\end{array}} \right)$, $\eta = 0.4, \; \tau(t) = 0.08{\rm sin}(t)+0.13, \; d = 0.0001, \; \rho = 0.08, \; \tau_1 = 0.05, \; \tau_2 = 0.21$, we have the following results.

We can obtain $\beta = 0.1$ and $\bar{P} = \left({\begin{array}{*{20}{c}}247.1713 & -54.5035\\123.5856 & -26.7517\end{array}} \right)$. In this case, we can obtain by calculation that

The matrix system ${L_1, L_2}$ is therefore strictly complete.

The division of the state space is performed as follows:

Then, the switched law is

In addition, it is possible to verify that $\bar{P}E = E^{\top}P^{\top} = \left({\begin{array}{*{20}{c}} 494.3426 & 247.1713\\ 247.1713 & 123.5857 \end{array}} \right)\ge 0$.

Figure 5 gives information about the state response $x^{\top}Qx$ for the initial conditions, and Figure 6 shows the variation in the switched signal with time. The system state of the two-dimensional system can be seen in Figure 7. From Definition 7, the system has FTS for the corresponding $(0.001, 3.7, 3, Q)$ under the switched law $\sigma(x(t)) = l$. Figure 8 shows the division of the state space corresponding to the different subsystems.

From the two examples above, we can see that the numerical example verifies that the system satisfies that $x^{\top}Qx$ is in the given interval for a given finite time period under the control scheme proposed in this paper.

Remark 3. In terms of Eq (3.3) or Eq (3.13), let symmetric matrix $P$ be positive definite, $S\in R^{n\times n-r}$ be a column full rank matrix that satisfies $E^{\top}S = 0$, and $V$ be a matrix of any suitable dimension. Let $\bar{P} = (PE+SV)^{\top }$, and we have $\bar{P}E = E^{\top}\bar{P}^{\top} = E^{\top}PE\ge 0$. Thus, Eq (3.13) is a linear matrix inequality that replaces $P$ with the notation $\bar{P} = (PE+SV)^{\top }$.

Remark 4. If we let $P_l = I$, then this paper addresses the question of what kind of singular switched systems can be finite-time stable with a deterministic control input $u = K_lx(t_q)$.

This paper focuses on the problem of how a singular switched system with a time-varying delay can be calibrated in finite time. There are various possible ways to construct L-K functions, but in view of the ease of computation, this paper does not use double integration to construct the L-K function, and a more conservative construction method will be considered and discussed in subsequent work.

A robust stability analysis of switched singular positive systems based on event triggering mechanisms will be a topic of future research.

5.   Conclusions

In this paper, unlike some existing research that focused on the Lyapunov stability property of a switched system with time-varying delays, we focus on finite-time stability. For singular switched systems with time-varying delays and perturbations, the results are given in relation to the derivatives of the time-varying delay. The control law is constructed with the help of an event-triggered mechanism and with the division of state space. After constructing a suitable Lyapunov-like function, in combination with a Lyapunov-like function approach, an FTS criterion is developed and proved. Finally, sufficient requirements are also presented to satisfy the finite-time stable $H_\infty$ performance index $\gamma$. An important and challenging future investigation is how to extend the results in this paper to switched nonlinear systems and how to reduce the conservatism of the method.

Acknowledgments

The paper was supported by the National Natural Science Foundation of China Nos. 11861013, 11771444; the Fundamental Research Funds for the Central Universities, China University of Geosciences (Wuhan) No. 2018061; the Fundamental Research Funds for National University, China University of Geosciences (Wuhan) No.CUGDCJJ202216.

Conflict of interest

The authors have no relevant financial or non-financial interests to disclose.