1.
Introduction
Today's cutting-edge technologies, such as machine learning and natural language processing, significantly minimize the amount of work required to address multiclass classification-related issues [1,2]. Typically, the classification task in machine learning occurs in a number of disciplines, including engineering [3], medicine [4], economics and finance [5,6]. In these disciplines, multiclass classification is an important problem. Every instance in the training set belongs to a unique label set that was formerly created for multiclass classification. Building a learning model from a training set of labeled data is the aim of supervised classification techniques, with the goal that it may categorize fresh data with unseen labels [7]. By expanding the binary classification problem, the multiclass classification problem can be resolved using a variety of machine learning techniques, such as k-Nearest Neighbors, Support Vector Machines, NNs, Naive Bayes and decision trees [8].
Primarily utilized for classification and regression issues, NNs have been successfully applied in a number of disciplines, including engineering, medicine, economics and finance. They are commonly utilized in the field of engineering for linear time varying systems and feedback control system stabilization [9,10], solar system measurements [11] and alloy behavior analysis [12]. Additionally, NNs are frequently used in the field of medicine to diagnose conditions including flat feet [13], breast cancer [14], lung cancer [15], and diabetic retinopathy [16], while they are commonly utilized in the fields of finance and economics for portfolio management [17], exchange rate analysis [18], time series forecasting [19] and macroeconomic factors prediction [20]. In this paper, a novel bio-inspired NN has been developed to address multiclass classification-related issues, and its effectiveness is then evaluated through applications in occupational classification systems.
Systems of occupational classification serve as frameworks for classifying jobs and related information. They are frequently used by government agencies to standardize the manner in which job descriptions and data on employment are gathered [21]. These systems therefore function as a fairly basic indication of occupational exposure, which is why they are highly significant in social science research [22]. In general, multiclass classification tasks are extensively used in social science research, including analyzing the connection between cancer and changes in occupational characteristics [23], describing occupational mobility [24], carrying out case-control studies in the healthcare industry [25], and assessing the viability of teleworking in certain occupations [26]. By translating a person's job description and the nature of their business into a standardized numerical code, public health professionals and academics can analyze patterns and trends in work-related illnesses, accidents, and exposures [27]. The systematic monitoring of any population, from the general population of the world to the people who compose a medium or small-sized business or town, is based on a variety of classification systems [28]. In the case of occupational classification, there exist several frequently updated national and international classification schemes [29], such as the Occupational Information Network Standard Occupational Classification (O*NET-SOC) and the International Standard Classification of Occupations (ISCO). Due of their widespread use, O*NET-SOC and ISCO are used in this study.
The U.S. Department of Labor developed the Occupational Information Network (O*NET), a comprehensive system for gathering, organizing, characterizing, and disseminating information on occupational characteristics and worker traits [30]. The O*NET makes information available online in a searchable database, making it simpler to acquire data on occupations at various levels of detail and enhancing its usefulness for a range of users. O*NET tools and products can be used by businesses and human resources professionals for a variety of tasks, such as creating job descriptions, increasing the number of qualified applicants for open positions, coordinating organizational development with workplace requirements, and adjusting recruitment and training objectives [31]. To find jobs that match their interests, values, skills, and experience, explore career growth profiles using the most recent labor market data, make wise career decisions to maximize earning potential and job satisfaction, and develop an understanding of what it takes to succeed in their fields and in related occupations, job seekers can use the information provided by O*NET [32]. Researchers who investigate topics relating to the U.S. workplace and labor market can benefit greatly from O*NET. To stay current with the jobs currently available on the labor market, the O*NET-SOC taxonomy is continually updated. Crosswalks between the original professions that were evaluated based on the work values and the new occupations have been created as part of these adjustments. On the other hand, ISCO was developed to make comparing occupational statistics between nations easier and to serve as guidelines for countries developing or adjusting their own national occupational classification schemes. It was created to fulfill a variety of administrative and research needs, and it is fully endorsed by the international community as an accepted norm for global labor statistics [33].
Crosswalks between different systems of occupation classification were formerly only made occasionally because they demand significant effort in terms of time and resources. For example, a crosswalk to go from the O*NET-SOC 2000 classification to ISCO-88 was used to explore employment consequences of automation and offshoring [34]. A crosswalk to go from the Brazilian Occupation Classification (BOC) 2002 to O*NET-SOC 2010 was used to analyze occupational profiles in the Brazilian workforce [35]. A crosswalk to go from the ISCO-88 to O*NET-SOC 2010 was used to analyze the Covid-19 pandemic impact of the global process of automation on employment in a developing economy [36]. A crosswalk to go from the National Classification of Occupations (CNO) 2011 to ISCO-88 was used to investigate job polarization [37]. It is important to note that the most recent versions, ISCO-08 and O*NET-SOC 2019, were adopted in 2008 and 2019, respectively, while the previous versions, ISCO-88 and O*NET-SOC 2010, were adopted in 1988 and 2010, respectively.
The main objective of this study is to provide a crosswalk between O*NET-SOC 2010 and ISCO-08 by using NNs. We will use a feedforward NN (FNN) that can handle multiclass classification tasks to accomplish this. In particular, a weights and structure determination (WASD) training algorithm will be employed as an alternative to the popular back-propagation technique, which is utilized to train FNNs. The WASD approach, as opposed to the back-propagation strategy, which iteratively adjusts the network's topology, computes the optimal set of weights directly utilizing the weights direct determination (WDD) method. This helps to reduce computational complexity and prevents the occurrence of local minima [38].
{Nowadays, one of the rapidly expanding fields of study in evolutionary computation is memetic algorithms [10].} To enhance the performance of the WASD based NNs for multiclass classification-related issues, the metaheuristic beetle antennae search (BAS) algorithm [39] is combined with the WASD algorithm in this research. This is done in consideration of the strong performance of the bio-inspired WASD algorithms for regression-related issues proposed in [20,40]. As a result, we introduce a new bio-inspired WASD for multiclass classification tasks (BWASDC) algorithm to train a 3-layer FNN. The advantages of the BWASDC algorithm over conventional WASD algorithms are listed below:
● it can more accurately determine the minimum optimal hidden layer structure of the NN with the use of BAS;
● the use of BAS aids the BWASDC in preventing the development of local minima more effectively.
It is noteworthy to highlight that BAS, which is capable of effective global optimization, has recently gained substantial traction in a number of scientific domains, including machine learning [10], robotics [41], engineering [42,43,44] and finance [20], and that it has undergone a number of modifications, including binary [45] and semi-integer [46] versions, to better address various tasks. Furthermore, taking into account two official ISCO and O*NET-SOC datasets, we employ the official crosswalk to build concordance between the two codes, O*NET-SOC 2010 and ISCO-08. This concordance is then utilized for assessing the performance of the BWASDC NN. According to the findings of four occupational classification experiments, the BWASDC model outperformed some of the most modern classification models obtainable through MATLAB's classification learner app on all fronts.
The following is a list of the paper's contributions.
● A new 3-layer bio-inspired WASD FNN for multiclass classification tasks, named BWASDC, is introduced.
● The BWASDC algorithm combines the WASD and BAS algorithms to further enhance the structure and performance of the WASD based NNs when dealing with multiclass classification-related issues.
● The O*NET-SOC 2010 and ISCO-08 codes' official concordance datasets are utilized to assess the performance of the BWASDC NN.
● In four experiments, the BWASDC model is compared with some of the most modern classification models available through MATLAB's classification learner app. The fine tree (FTR), narrow NN (NNN), ensemble bagged trees (EBT) and fine k-nearest neighbors (FKN) are these classification models [47]. Furthermore, a comparison is also made between the BWASDC model and the deep learning transformer MATLAB model (TRA) from [48].
The sections that follow provide a description of the paper's structure. A breakdown of the WDD process for multiclass classification tasks and the 3-layer BWASDC FNN structure is given in Section 3.2. Additionally, Section 3.2 provides an extensive description of the BWASDC algorithm, along with the entire procedure for training and testing the BWASDC NN model. The findings of four crosswalk experiments between occupational classifications using the BWASDC model and some of the most modern classification models obtainable through MATLAB's classification learner app are displayed and discussed in Section 3. It is important to mention that the datasets utilized in our research and the steps necessary to prepare them for usage with the BWASDC NN are also described in Section 3. Finally, Section 4 includes closing remarks and reflections.
2.
The bio-inspired WASD neural network model
This section describes the WDD process for multiclass classification-related issues, the structure of the 3-layer bio-inspired FNN and the BWASDC algorithm.
2.1. The WDD process for multiclass classification tasks
Because it avoids the need for tedious, time-consuming, and usually inaccurate repetitive computations to obtain the necessary weights matching the present hidden layer structure, the WDD process is a crucial part of any WASD technique. In comparison to conventional weight determination methods, the WDD procedure is said to provide for both lower computational complexity and speed while solving some of the associated problems [38]. It is vital to mention that the WDD only accepts input data in the form of real numbers. The data must also be normalized to a range of [0,1] before being fed into the NN model. In this way, the NN can handle over-fitting. If required, we can accomplish that by using the linear transformation shown in [5].
Here, thorough justifications of significant theoretical foundations and research are offered for the development of the BWASDC NN. However, it is crucial to first note a few of the major symbols employed in this paper: ()T signifies transposition; ζ! signifies the factorial of ζ; ()† signifies pseudoinversion; ()⊙ signifies the elementwise exponential; round(⋅) signifies a round function.
Below is a restatement of the Taylor polynomial approximation (TA) theorem from [49].
Theorem 2.1. When a target function, U(⋅), has the (Θ+1)-order continuous derivative on the range [γ1,γ2], and Θ is a nonnegative integer, the following is true:
where CK(ζ) and BK(ζ) signify the error term and Θ-order TA of U(ζ), respectively.
Consider U(δ)(β) to be the value of the δ-order derivative of U(x) at the point β. Below is shown the approximate representation of U(ζ):
Proposition 2.1. Theorem 2.1 may be utilized for multivariable function approximation. Consider U(ζ1,ζ2,…,ζv) to be the target function with v variables and (Θ+1)-order continuous partial derivatives in an origin's neighborhood (0,…,0). Below is shown the Θ-order TA BΘ(ζ1,ζ2,…,ζv) about the origin:
where δ1,δ2,…,δv are nonnegative integers.
Consider the input A=[A1,A2,…,Am]∈R1×m and the target vector D∈R. Based on the power activated multi-input NNs presented in [38], the link between the input variables A1,A2,…,Am and the NN's output target D can be expressed using the nonlinear function presented below:
Further, in line with Proposition 2.1, the Θ-order TA BΘ(A1,A2,…,Am) may map (2.4) as presented below:
where kh=Gh(A1,A2,…,Am)∈R1×mn refers to a power activation function, wh∈Rmn is the weight that corresponds to kh, and h implies both the power value and the hidden layer neurons' number.
For a given number of samples r∈N, the input becomes a matrix A=[A1,A2,…,Am]∈Rr×m, where Ai∈Rr for i=1,…,m, and the target vector D∈Rr. Thereafter, setting kr,h=Gh(A1,A2,…,Am)∈Rr×mn, the input-activation matrix K and the weight vector W are presented below:
Then, using the WDD technique described below, the weights of the Θ-order TA NN are directly generated, as opposed to utilizing the iterative weight training methods used in conventional NNs [49]:
It is crucial to note that the next power maxout elementwise activation function is recommended when dealing with multiclass classification tasks:
where Ai is the i-th row, and Aij is the ij-th element of the input matrix A.
2.2. The neural network structure
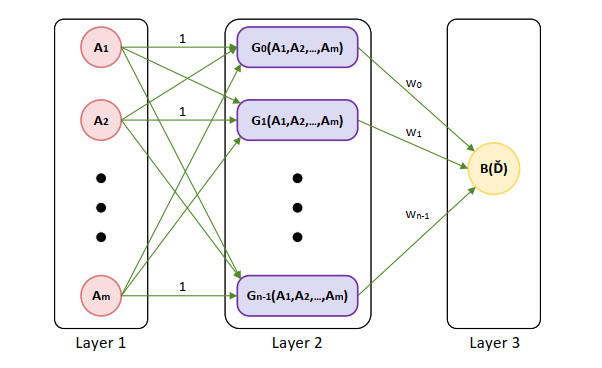
Figure 1 depicts the 3-layer FNN architecture. In particular, the NN gets the input values A1,A2,…,Am from Layer 1 (also known as the input layer) and distributes them equally to the appropriate neuron of Layer 2. Observe that there is a maximum number n of active neurons in Layer 2. Additionally, the WDD is utilized to acquire the neurons that connect Layers 2 and 3, and they have weights Wc,c=0,1,…,n−1. The formula described below is utilized to calculate the predictions ˇD:
Finally, one activated neuron is present in Layer 3 (also known as the output layer), and it uses the elementwise function described below:
2.3. The BWASDC algorithm
The NN model is trained using the BWASDC algorithm, which incorporates the BAS algorithm [39]. It is important to mention that the strength of the odor that beetles perceive on the antennas determines how they use their two antennas to find food (see Figure 2). The BAS algorithm's optimal solution finder mimics this tendency, and this strategy enables the application of cutting-edge optimization techniques (see [50,51,52]). By imitating the beetle's behavior, BWASDC finds the optimal number of the NN's hidden layer neurons along with their power values. The next steps, S1 to S3, outline the BWASDC algorithmic procedure.
S1: First, an objective function must be defined. Consider the training set Xtr∈Rr×m, where r is the number of samples, and their target Dtr∈Rr. The K matrix is created in line with (2.8) and (2.6), and the weights of the NN are calculated by (2.7) using Dtr. Then, the NN predictions ˇDtr are obtained by (2.9), and the mean absolute error (MAE) between ˇDtr and the target value Dtr is measured through the following formula:
Note that the MAE measures errors between paired observations that describe the same phenomenon, and it is frequently applied as a loss function in machine learning for classification-related issues. Considering the vector x, which contains the power values of the NN's hidden layer neurons, the aforementioned process is formulated as an objective function in Algorithm 1.
S2: Second, the objective function in Algorithm 1 is minimized by mimicking the beetle's behavior. Assume the vector x, where its elements take the integer values 0, 1, …,nmax−1 or nmax with nmax denoting the user-specified maximum number of hidden layer neurons. These nmax+1 numbers correspond to the activation functions' powers for each hidden layer neuron. For example, if x=[5,7]T, it denotes the existence of two hidden layer neurons, the first of which uses the power of 5 in (2.8), and the second of which uses the power of 7.
In our approach, the aforementioned vector x represents the beetle's position, and the objective function f(x) in Algorithm 1 represents the odor concentration at the position x, with the minimum value of f(x) serving as a link to the odor's source. To determine the hidden layer neurons' number in the NN at the position x, the following functions will be used:
Additionally, to represent the beetle's position at the t-th moment, we use the notation xt with t=1,2,3,…,tmax with tmax denoting the maximum number of iterations specified by the user. The beetle's erratic search path thus defines the paradigm of searching behavior as follows:
where λ∈Rnmax denotes a vector of nmax random elements, and ϵ=2−52. The right (xR) and left (xL) antennae are formulated as below to replicate the searching behaviors of the beetle's antennae:
where ζt denotes the antennae's sensing width that correlates to exploitation capacity at the t-th moment. Additionally, consider the candidate optimal solution (xC),
where ξt denotes a size step that takes into consideration the convergence's pace following an increase in t during the search. Then, the behavior of detecting is expressed as below:
Last, the update rules for ζ and ξ are described next:
It is significant to note that the following are the initial conditions for the aforementioned strategy:
where k=round(nmax/2).
S3: Last, the BWASD algorithm determines and returns the optimal W on the entire training data set along with the optimal power value N of each hidden layer neuron. That is, it sets in N only the nonnegative elements of xt+1, it calculates the matrix K according to (2.8) and (2.6) under the power values contained in N, and it finds the W via the WDD method using K and D.
In this manner, the NN's MAE can be decreased while the BWASDC algorithm maintains the fewest hidden layer neurons possible. The flowchart in Figure 3 shows the BWASDC algorithm's entire process.
After optimizing the structure of the BWASDC NN model of Figure 1 and determining its optimal weights, we consider the testing set Xte to obtain the NN predictions ˇDte through (2.9). The flowchart of Figure 4 shows the step-by-step process for predicting and modeling using the BWASDC NN model.
3.
Experiments between occupational classifications
Four crosswalk experiments are performed between the occupational classifications of the ISCO-ONET and ONET-ISCO datasets in this section. Notice that the creation of these datasets is described in the following Section 3.1. In these experiments, the BWASDC NN's performance is examined and contrasted with some of the most modern classification models available in the MATLAB classification learner app. The FTR, NNN, EBT and FKN are these classification models [47]. Notice that the TRA model from [48], which utilizes a pretrained bidirectional encoder with representations from a transformer model, is also compared to the BWASDC model. Additionally, in all experiments, we have set the parameters ζ0=20, ξ0=10, tmax=21 and nmax=10 for the BWASDC model, while the default settings have been used in MATLAB classification models. It is important to note that you can access GitHub's full development and application of the computational techniques and concepts discussed in Sections 3.2 and 3.1 by visiting the following link:
The MATLAB repository includes thorough installation instructions along with the complete implementation.
3.1. Datasets and data preparation
The three datasets utilized in our research and the steps necessary to prepare them for usage with the BWASDC NN are described in this section.
ISCO is a tool for categorizing occupations into groups that are precisely specified based on the duties and responsibilities carried out in the work, and it is intended to be utilized in a variety of client-oriented tasks and statistics implementations. This split is advantageous from a social, economic, and medical standpoints [21]. The ISCO's 4-level hierarchy-based categorization system also allows for the categorization of jobs into 436 groupings of 4 digits, 130 minor groupings of 3 digits, 43 sub-major groupings of 2 digits and 10 major groupings of 1 digit. The first dataset, which comes from the International Labor Organization and includes a sample of job titles with associated ISCO-08 codes, may be accessed at the following link: https://www.ilo.org/ilostat-files/ISCO/newdocs-08-2021/ISCO-08/ISCO-08%20EN%20Structure%20and%20definitions.xlsx. For ease of use, we will call this dataset ISCOD. It is important to mention that the classification tasks in our approach only include the 4-digit unit groups. As a result, there are 436 distinct classes of professions in the ISCOD, and each class contains a wide range of job titles.
The work carried out in the U.S. is divided into about 1000 occupational groups using the O*NET-SOC system. Data on the significance and level of a number of occupational variables, such as Knowledge, Skills, Abilities, Tasks, and General Work Activities, are related with these occupations. The 2018 Standard Occupational Classification (SOC) system serves as the foundation for the O*NET-SOC system. All federal agencies that gather and disseminate occupational data are required to adopt this classification system, according to the U.S. Office of Management and Budget. Anybody can get extensive information on a job inside the O*NET-SOC system and links to other sources of national, state, and local SOC-based occupational information by using a code and a job title [30]. The second dataset, which comes from the O*NET resource center and includes a sample of job titles with associated O*NET-SOC codes, can be accessed at the following link: https://www.onetcenter.org/dl_files/database/db_20_1_excel/Sample%20of%20Reported%20Titles.xlsx For ease of use, we will call this dataset ONETD. It is important to mention that there are 873 distinct classes of professions in the ONETD, and each class contains a wide range of job titles.
The third dataset, which contains the crosswalk between the O*NET-SOC 2010 and ISCO-08, is taken from the U.S. Bureau of Labor Statistics and can be accessed at the following link: https://www.bls.gov/soc/ISCO_SOC_Crosswalk.xls. For ease of use, we will refer to the crosswalk from ISCO-08 to O*NET-SOC 2010 of this dataset as ISCO-ONET, and the crosswalk from O*NET-SOC 2010 to ISCO-08 as ONET-ISCO. Particularly, ISCO-ONET consists of 1123 samples, each of which comprises an ISCO-08 code and an O*NET-SOC 2010 code, and the job titles that go with these two codes can be taken from either ISCOD or ONETD. Additionally, ONET-ISCO consists of 1123 samples, each of which comprises an O*NET-SOC 2010 code and an ISCO-08 code, and the job titles that go with these two codes can be taken from either ISCOD or ONETD.
Only actual numerical data can be utilized as input to train and test the BWASDC NN model owing to the restrictions imposed by the WDD method. Because strings are present in both the ISCO-ONET and ONET-ISCO datasets used in our investigation, the data must be appropriately prepared and processed before being fed into the model. The next phases, P1 to P3, outline the procedure that each dataset should follow.
P1: For each sample i,i=1,…,m of the dataset (i.e. ISCO-ONET or ONET-ISCO), a foundational vocabulary must be created. To do this, one of the two classification codes (i.e. O*NET-SOC 2010 or ISCO-08) and the corresponding job titles must be tokenized in accordance with guidelines of Unicode Standard Annex #29 [53], with all letters being changed to lowercase and punctuation being eliminated. We therefore construct the structure vector Q=[q1,q2,…,qm], where qi,i=1,…,m, is the vocabulary of sample i, provided that there are m classes.
P2: Suppose that r samples, each containing the type of classification code we selected in P1 and one job title, will be used to validate or test the NN model. For each sample i,i=1,…,r, a vocabulary needs to be created. Each job title must be tokenized, all letters must be changed to lowercase, and punctuation must be eliminated in a manner similar to P1 in order to accomplish this. We therefore construct the structure vector Z=[z1,z2,…,zr], where zi is the vocabulary of a certain class for i=1,…,r.
P3: Taking into account that some terms of a vocabulary in Z may belong to many separate vocabularies of samples in Q, we generate an input matrix A that comprises the rates of similarity among the vocabularies in Q to train or test the model. The input matrix A∈Rr×m is specifically created by taking into account the following procedure. Suppose that the aforementioned structure vectors Q and Z have vocabularies qj,j=1,…,m, and zi,i=1,…,r, that have kj and hi words, respectively. The ratio of the vocabulary qj to the vocabulary zi is then:
where the function strcmp(⋅) outputs 1 (true) when the input strings are the same and 0 (false) otherwise [54]. Be aware of the fact that qj(h) is the h-th entry (word) of the vocabulary qj in (3.1). Additionally, the ratio of the vocabulary zi to the vocabulary qj is:
Notice that J(j,i) and R(j,i) take values in the range [0,1].
It should be stressed that steps P1 through P3 are a heuristic technique that achieves the problem's objectives, namely, text to number conversion as well as matrix standardization to avoid overfitting. For the creation of the training and testing sets, the following phases P4 and P5 are considered.
P4: For training the NN model, the input set Xtr and the target Dtr are created. Particularly, we set Z=Q in phase P3 to create the input matrices Jtr,Rtr∈Rm×m. Following that, we set Xtr=pJtr+(1−p)Rtr with a proportion of p=1/2, where the ji-th entry of Xtr reflects the average of (3.1) and (3.2) and takes values in the range [0,1]. Furthermore, the target vector of Xtr has been set to Dtr=[1,2,…,m]T∈Rm, where each entry refers to a unique class.
P5: For testing the NN model, the input set Xte and the target Dte are created. To do this, a new dataset Vi is created using the chosen dataset (i.e., ISCO-ONET or ONET-ISCO). Particularly, Vi is identical to the chosen dataset with the exception that includes only the i-th occupation. Next, we set the dataset [V1;Vlast] in phase P2, and create the input matrices Jte,Rte∈R2m×m in phase P3.
Following that, we set Xte=pJte+(1−p)Rte with a proportion of p=1/2, where the ji-th entry of Xte reflects the average of (3.1) and (3.2) and takes values in the range [0,1]. Furthermore, the target vector of Xte has been set to Dte=[Dtr;Dtr]∈R2m.
After implementing the phases P4 and P5 in both datasets (i.e., ISCO-ONET and ONET-ISCO), we will take Xtr∈R1123×1123, Dtr∈R1123, Xte∈R2246×1123 and Dte∈R2246.
3.2. Experiment 1
In this experiment, the job titles are taken from ONETD and used with the ISCO-ONET dataset. By using the ISCO-08 code and the job titles as input, the crosswalk from ISCO-08 to O*NET-SOC 2010 is predicted through the NN models. The BWASDC training error is shown in Figure 5a, while the hidden layer neurons' number of its iteration of the algorithm is shown in Figure 5b. These figures show that the BWASDC algorithm needed 4 iterations to reach the smallest error with the minimum neurons' number. The minimum number of neurons is 6, and their optimal powers are [3,2,4,6,3,4]T. Furthermore, Figure 5c and 5d, respectively, show the predictions made based on the training and testing sets. Comparing Figure 5c and 5d, we see that the BWASDC, FKN, and EBT perform similarly on the training set, while BWASDC performs significantly better on the testing set. Particularly, Figure 5c shows that FKN has the greatest results on the training set, EBT has the 2nd greatest, BWASDC has the 3rd greatest, and FTR has the worst. On the other hand, Figure 5d shows that BWASDC has the greatest results on the testing set, FKN has the 2nd greatest, and FTR has the worst. In other words, BWASDC outperforms the other models in its ability to classify unknown data.
In Table 1, a number of performance metrics are applied to the outputs of the models on the training and testing sets in order to statistically evaluate their performances. The accuracy, MAE, specificity, sensitivity, precision, Matthews correlation coefficient (MCC), F-score, Cohen's κ and false positive rate (FPR) are the performance measures considered in our analysis. See [55,56] for more information and a thorough study of these measures. On the training set, the MAE and accuracy of the models are measured. We observe that FKN has the best MAE and accuracy, the EBT has the 2nd best, BWASDC has the 3rd best, and FTR has the worst. On the testing set, we note that BWASDC outperforms the other models in the majority of the measures. Particularly, BWASDC has the best MAE, accuracy, specificity, FPR, F-score, Cohen's k and precision. FKN has the 2nd best accuracy, specificity, FPR, Cohen's k and precision, and EBT has the 2nd best MAE and F-score, while FTR has the best sensitivity and MCC. Overall, the BWASDC performs better than the other models, while FTR and TRA put on the worst and second-worst performances, respectively.
3.3. Experiment 2
In this experiment, the job titles are taken from ONETD and used with the ONET-ISCO dataset. By using the O*NET-SOC 2010 code and the job titles as input, the crosswalk from O*NET-SOC 2010 to ISCO-08 is predicted through the NN models. The BWASDC training error is shown in Figure 6a, while the hidden layer neurons' number of its iteration of the algorithm is shown in Figure 6b. These figures show that the BWASDC algorithm needed 3 iterations to reach the smallest error with the minimum neurons' number. The minimum number of neurons is 3, and their optimal powers are [4,4,2]T. Furthermore, Figure 6c and 6d, respectively, show the predictions made based on the training and testing sets. Comparing Figure 6c and 6d, we see that the BWASDC, FKN, and EBT perform similarly on the training set, while BWASDC performs significantly better on the testing set. Particularly, Figure 6c shows that FKN has the greatest results on the training set, BWASDC has the 2nd greatest, and FTR has the worst. On the other hand, Figure 6d shows that BWASDC has the greatest results on the testing set, FKN has the 2nd greatest, and FTR has the worst. In other words, BWASDC outperforms the other models in its ability to classify unknown data.
In Table 2, a number of performance metrics are applied to the outputs of the models on the training and testing sets in order to statistically evaluate their performances. On the training set, the MAE and accuracy of the models are measured. We observe that FKN has the best accuracy and the 2nd best MAE, while BWASDC has the best MAE and the 2nd best accuracy. Additionally, EBT has the 3rd best MAE and accuracy, while FTR has the worst. On the testing set, we note that BWASDC outperforms the other models in the majority of the measures. Particularly, BWASDC has the best MAE, accuracy, specificity, sensitivity, FPR, F-score, Cohen's k and precision. FKN has the 2nd best accuracy, specificity, FPR, Cohen's k and precision, and EBT has the 2nd best MAE and F-score, while FTR has the best MCC and the 2nd best sensitivity. Overall, the BWASDC performs better than the other models, while FTR and NNN put on the worst and second-worst performances, respectively.
3.4. Experiment 3
In this experiment, the job titles are taken from ISCOD and used with the ISCO-ONET dataset. By using the ISCO-08 code and the job titles as input, the crosswalk from ISCO-08 to O*NET-SOC 2010 is predicted through the NN models. The BWASDC training error is shown in Figure 7a, while the hidden layer neurons' number of its iteration of the algorithm is shown in Figure 7b. These figures show that the BWASDC algorithm needed 12 iterations to reach the smallest error with the minimum neurons' number. The minimum number of neurons is 5, and their optimal powers are [5,3,7,8,6]T. Furthermore, Figure 7c and 7d, respectively, show the predictions made based on the training and testing sets. Comparing Figure 7c and 7d, we see that the BWASDC, FKN, and EBT perform similarly on the training set, while BWASDC performs significantly better on the testing set. Particularly, Figure 7c shows that FKN has the greatest results on the training set, EBT has the 2nd greatest, BWASDC has the 3rd greatest, and FTR has the worst. On the other hand, Figure 7d shows that BWASDC has the greatest results on the testing set, FKN has the 2nd greatest, and FTR has the worst. In other words, BWASDC outperforms the other models in its ability to classify unknown data.
In Table 3, a number of performance metrics are applied to the outputs of the models on the training and testing sets in order to statistically evaluate their performances. On the training set, the MAE and accuracy of the models are measured. We observe that FKN has the best accuracy and the 3rd best MAE, while BWASDC has the best MAE and the 3rd best accuracy. Additionally, EBT has 2nd best MAE and accuracy, while FTR has the worst. On the testing set, we note that BWASDC outperforms the other models across all measures. Further, FKN has the 2nd best MAE, accuracy, specificity, FPR, F-score, Cohen's k and precision, while FTR has the 2nd best sensitivity and MCC. Overall, the BWASDC performs better than the other models, while FTR and TRA put on the worst and second-worst performances, respectively.
3.5. Experiment 4
In this experiment, the job titles are taken from ISCOD and used with the ONET-ISCO dataset. By using the O*NET-SOC 2010 code and the job titles as input, the crosswalk from O*NET-SOC 2010 to ISCO-08 is predicted through the NN models. The BWASDC training error is shown in Figure 8a, while the hidden layer neurons' number of its iteration of the algorithm is shown in Figure 8b. These figures show that the BWASDC algorithm needed 5 iterations to reach the smallest error with the minimum neurons' number. The minimum number of neurons is 7, and their optimal powers are [3,2,4,2,7,5,9]T. Furthermore, Figure 8c and 8d, respectively, show the predictions made based on the training and testing sets. Comparing Figure 8c and 8d, we see that the BWASDC, FKN, and EBT perform similarly on the training set, while BWASDC performs significantly better on the testing set. Particularly, Figure 8c shows that FKN has the greatest results on the training set, BWASDC has the 2nd greatest, EBT has the 3rd greatest, and FTR has the worst. On the other hand, Figure 8d shows that BWASDC has the greatest results on the testing set, FKN has the 2nd greatest, and FTR has the worst. In other words, BWASDC outperforms the other models in its ability to classify unknown data.
In Table 4, a number of performance metrics are applied to the outputs of the models on the training and testing sets in order to statistically evaluate their performances. On the training set, the MAE and accuracy of the models are measured. We observe that FKN has the best accuracy and the 2nd best MAE, while BWASDC has the best MAE and the 2nd best accuracy. Additionally, EBT has 3rd best MAE and accuracy, while FTR has the worst. On the testing set, we note that BWASDC outperforms the other models across all measures. Further, FKN has the 2nd best MAE, accuracy, specificity, FPR, F-score, Cohen's k and precision, while FTR has the 2nd best sensitivity and MCC. Overall, the BWASDC performs better than the other models, while FTR and NNN put on the worst and second-worst performances, respectively.
4.
Conclusions
This paper presented a new 3-layer bio-inspired WASD FNN for multiclass classification tasks, termed BWASDC. The efficacy of the BWASDC was then assessed by applications in occupational classification systems, specifically through four crosswalk experiments between the O*NET-SOC 2010 and ISCO-08 codes. The findings of these experiments show that the BWASDC model outperforms some of the most modern classification models obtainable through MATLAB's classification learner app. The BWASDC model has thus proven to be a great substitute for dealing with multiclass classification-related issues. It is significant to note that only actual numerical data may be utilized as input to train and test the BWASDC NN model owing to restrictions imposed by the WDD process, which is utilized by the BWASDC algorithm. The BWASDC model's only testing involved applications in occupational classification systems, which is another drawback. Future study will consequently focus on its suitable adaptation and application to various multiclass classification problems across numerous scientific fields.
Use of AI tools declaration
The authors declare they have not used artificial intelligence (AI) tools in the creation of this article.
Acknowledgments
This work was supported by the Key Science and Technology Research of Henan Province, China (Grant No. 222102210279, 232102210129, 232102210076, 232102210074, 232102211038 and 222102210232), and by the Postgraduate Joint Training Base Project of Henan Province, China (Grant No. YJS2022JD45).
Conflict of interest
The authors declare no conflict of interest. Vasilios N. Katsikis is a Guest Editor for AIMS Mathematics and was not involved in the editorial review or the decision to publish this article. All authors declare that there are no competing interests.









 DownLoad:
DownLoad: