Abundant solitary wave solutions of Gardner's equation using three effective integration techniques

Ghazala Akram; Saima Arshed; Maasoomah Sadaf; Hajra Mariyam; Muhammad Nauman Aslam; Riaz Ahmad; Ilyas Khan; Jawaher Alzahrani; Ghazala Akram; Saima Arshed; Maasoomah Sadaf; Hajra Mariyam; Muhammad Nauman Aslam; Riaz Ahmad; Ilyas Khan; Jawaher Alzahrani

doi:10.3934/math.2023413

AIMS Mathematics

2023, Volume 8, Issue 4: 8171-8184. doi: 10.3934/math.2023413

Previous Article Next Article

Research article

Abundant solitary wave solutions of Gardner's equation using three effective integration techniques

1.
Department of Mathematics, University of the Punjab, Lahore, Pakistan
2.
Department of Mathematics and Statistics, The University of Lahore, Lahore, Pakistan
3.
Department of Mathematics and Statistics, Nanjing University of Information Science and Technology, Nanjing, China
4.
Department of Mathematics, College of Science Al-Zulfi, Majmaah University, Al-Majmaah 11952, Saudi Arabia

Received: 14 October 2022 Revised: 04 December 2022 Accepted: 26 December 2022 Published: 01 February 2023

Gardner's equation has been discussed in the article for finding new solitary wave solutions. Three efficient integration techniques, namely, the Kudryashov's R function method, the generalized projective Ricatti method and $\frac{G'}{G^2}$ -expansion method are implemented to obtain new dark soliton, bright soliton, singular soliton, and combo soliton solutions. Moreover, some of the obtained solutions are graphically depicted by using $3$ D-surface plots and the corresponding $2$ D-contour graphs.

Keywords:

Citation: Ghazala Akram, Saima Arshed, Maasoomah Sadaf, Hajra Mariyam, Muhammad Nauman Aslam, Riaz Ahmad, Ilyas Khan, Jawaher Alzahrani. Abundant solitary wave solutions of Gardner's equation using three effective integration techniques[J]. AIMS Mathematics, 2023, 8(4): 8171-8184. doi: 10.3934/math.2023413

Related Papers:

[1]	Ghazala Akram, Maasoomah Sadaf, Mirfa Dawood, Muhammad Abbas, Dumitru Baleanu . Solitary wave solutions to Gardner equation using improved tan $\left(\frac{\Omega(\Upsilon)}{2}\right)$ -expansion method. AIMS Mathematics, 2023, 8(2): 4390-4406. doi: 10.3934/math.2023219
[2]	Yunmei Zhao, Yinghui He, Huizhang Yang . The two variable (φ/φ, 1/φ)-expansion method for solving the time-fractional partial differential equations. AIMS Mathematics, 2020, 5(5): 4121-4135. doi: 10.3934/math.2020264
[3]	Jalil Manafian, Onur Alp Ilhan, Sizar Abid Mohammed . Forming localized waves of the nonlinearity of the DNA dynamics arising in oscillator-chain of Peyrard-Bishop model. AIMS Mathematics, 2020, 5(3): 2461-2483. doi: 10.3934/math.2020163
[4]	Hammad Alotaibi . Solitary waves of the generalized Zakharov equations via integration algorithms. AIMS Mathematics, 2024, 9(5): 12650-12677. doi: 10.3934/math.2024619
[5]	Weiping Gao, Yanxia Hu . The exact traveling wave solutions of a class of generalized Black-Scholes equation. AIMS Mathematics, 2017, 2(3): 385-399. doi: 10.3934/Math.2017.3.385
[6]	M. Hafiz Uddin, M. Ali Akbar, Md. Ashrafuzzaman Khan, Md. Abdul Haque . New exact solitary wave solutions to the space-time fractional differential equations with conformable derivative. AIMS Mathematics, 2019, 4(2): 199-214. doi: 10.3934/math.2019.2.199
[7]	Huiqing Wang, Md Nur Alam, Onur Alp İlhan, Gurpreet Singh, Jalil Manafian . New complex wave structures to the complex Ginzburg-Landau model. AIMS Mathematics, 2021, 6(8): 8883-8894. doi: 10.3934/math.2021515
[8]	Mostafa M. A. Khater, S. H. Alfalqi, J. F. Alzaidi, Samir A. Salama, Fuzhang Wang . Plenty of wave solutions to the ill-posed Boussinesq dynamic wave equation under shallow water beneath gravity. AIMS Mathematics, 2022, 7(1): 54-81. doi: 10.3934/math.2022004
[9]	Chun Huang, Zhao Li . Soliton solutions of conformable time-fractional perturbed Radhakrishnan-Kundu-Lakshmanan equation. AIMS Mathematics, 2022, 7(8): 14460-14473. doi: 10.3934/math.2022797
[10]	Huaji Cheng, Yanxia Hu . Exact solutions of the generalized (2+1)-dimensional BKP equation by the G'/G-expansion method and the first integral method. AIMS Mathematics, 2017, 2(3): 562-579. doi: 10.3934/Math.2017.2.562

Abstract

1. Introduction and preliminary results

Soliton theory has become a topic of great interest for mathematicians since its emergence with the discovery of solitary waves by Russel in $1834$ . Afterward, Kruskal and Zabusky introduced the concept of solitons triggering the worldwide study of solitons along with the development in mathematics and other fields of science and engineering. Solitons have many applications in different mathematical-oriented disciplines of science due to their shape-preserving properties and other useful aspects. Solitary waves are more stable over long distances because they preserve their shape and velocity after the interaction. This special characteristic of solitons stimulates mathematicians and physicists to work on them, which is why soliton theory is still progressing.

Various nonlinear PDEs have been investigated during recent years for getting their solitary wave solutions of various types. For instance, soliton solutions of mKdV equation ^[1] and ^[39], nonlinear Schrödinger equation ^[2], KE equation ^[40], Date-Jimbo-Kashiwara-Miwa equation ^[3], Boussinesq equation ^[4], Lakshmanan-Porsezian-Daniel equation ^[5], Kwahara equation^[6], ZK-Burgers equation ^[7], Lakshmanan-Porsezian-Daniel equation ^[8] and the Błaszak-Marciniak lattice equations ^[41] have been extracted by different researchers. Discrete nonlinear Schrödinger equations have also been extensively studied in various physical contexts, for instance, in studying the diode-like transmission properties of waves ^{[9,10,11,12,13]}. Soliton theory has become the popular topic of research and has attracted the attention of researchers in recent years. Soliton resolution and the asymptotic stability of N-soliton solution for the Wadati-Konno-Ichikawa equation are discussed in ^[26]. Soliton resolution for the Wadati-Konno-Ichikawa equation with weighted Sobolev initial data is discussed in ^[27]. Soliton resolution for the complex short pulse equation with weighted Sobolev initial data in space-time solitonic regions is presented in ^[28]. Riemann-Hilbert problem and dynamics of soliton solutions of the fifth-order nonlinear Schrödinger equation are discussed in ^[29]. Integrable discretizations and soliton solutions of an Eckhaus-Kundu equation are presented in ^[30]. Traveling wave, lump Wave, rogue wave, multi-kink solitary wave and interaction solutions in a (3+1)-dimensional Kadomtsev-Petviashvili equation using Bäcklund transformation are presented in ^[31]. The interpretation and understanding of natural processes require the extraction of analytical and numerical solutions of nonlinear evolution equations (NLEEs). In the quest of finding solutions of NLEEs, a number of novel and effective techniques have been reported including Riemann-Hilbert method ^[32,33], Hirota method ^[34], Darboux transformation method ^[35] and Lie symmetry analysis approach ^[36], etc.

This paper addresses Gardner's equation ^[14,15,16] in the form

$\begin{equation} \eta_t+2 \alpha \eta\eta_x+3\beta\eta^2\eta_x+\gamma \eta_{xxx} = 0. \end{equation}$

(1.1)

In the above model, $\alpha, \; \beta$ and $(\gamma > 0)$ are nonzero constants. The proposed model was first introduced by Gardner in $1968$ during his research on KdV equation ^[17]. This equation has great importance in various branches of science, especially in the quantum field and plasma physics ^[18]. The derivation of higher-order Gardner's equation is considered very imperative for analyzing nonlinear properties of solitary waves ^[19]. In recent years, Gardner's equation has been studied by many researchers ^[42,43,44] for extracting soliton solutions. Moreover, there are also Jacobi elliptic solutions in the Gardner's equation ^[37].

The purpose of writing this article is to construct new soliton solutions of the proposed model Eq (1.1) via three effective schemes, namely, Kudryashov's R function method, generalized projective Riccati equations method and $\frac{G'}{G^{2}}-$ expansion approach.

In $2020$ , Kudryashov was the first who developed Kudryashov's R function approach ^[20]. This method is effective and applicable to a variety of nonlinear problems. The generalized Riccati equations method (GPRE) originated from the research work of Conte and Musette ^[21] in which they proposed the idea of writing an NLPDE as a polynomial of two functions. The third method utilized in this paper is $\frac{G'}{G^{2}}-$ expansion method that was introduced in $2009$ ^[22]. Application of these methods to the considered Gardner's equation has resulted in novel soliton solutions.

The paper contains seven sections. In Section $2$ , the governing equation is converted into an ODE via traveling wave transformation. Sections $3$ – $5$ deal with solution of Gardner's equation using the suggested methods. Few of the obtained solutions are graphically illustrated in Section $6$ , whereas the concluding points are given in Section $7$ .

2. Governing equation

The proposed model is considered, as

$\begin{eqnarray} \eta_t+2 \alpha \eta\eta_x+3\beta\eta^2\eta_x+\gamma \eta_{xxx} = 0. \end{eqnarray}$

(2.1)

If the $\beta > 0$ , Eq (2.1) admits two families of solitons and oscillating wave packets (called breathers), whereas if $\beta < 0$ , only one category of solitons exists. Eq (2.1) is also called the combined KdV-mKdV equation.

The following wave transformation is used for extracting solitary wave solutions of (2.1), as

$\begin{eqnarray} \eta(x, t) = P(a), \quad a = \mu x-\theta t, \end{eqnarray}$

(2.2)

where $P$ is real-valued function and $\mu, \; \theta$ are nonzero constants.

Applying transformation (2.2) on Eq (2.1), an ordinary differential equation (ODE) is yielded, as

$\begin{eqnarray} -\theta P^{'}+2\alpha \mu P P^{'}+3\beta \mu P^{2}P^{'}+\gamma \mu^{3} P^{'''} = 0. \end{eqnarray}$

(2.3)

Now upon integrating Eq (2.3), we have

$\begin{eqnarray} -\theta P+\alpha \mu P^2+\beta \mu P^3+\gamma \mu^3 P^{''} = 0. \end{eqnarray}$

(2.4)

Further, Eq (2.4) is solved using three proposed efficient analytical methods in the following subsections to derive solitary wave solutions of the proposed model Eq (2.1).

3. Solitary wave solutions using Method 1: Kudryashov's R function method

In this section, Method 1 and its implementation of the proposed model have been illustrated.

3.1. Description of Method 1

According to Method 1 ^[24], the predicted solution of Eq (2.4) takes the following form

$\begin{eqnarray} P(a) = \sum\limits_{i = 0}^{N} A_{i}\left(R(a)\right)^{i}. \end{eqnarray}$

(3.1)

Where $N$ is a balancing number, determined by applying homogenous balancing principle (balancing the highest order derivative and nonlinear term) on Eq (2.4). $R(a)$ has the the following form

$\begin{eqnarray} R(a) = \frac{1}{\epsilon e^{\delta a}+ \varepsilon e^{-\delta a}}, \end{eqnarray}$

(3.2)

where $\epsilon, \; \varepsilon$ and $\delta$ are parameters. $R(a)$ obeys the following ODE

$\begin{eqnarray} R_{a}^{2} = \delta R^{2}\left( 1-4 \epsilon \varepsilon R^{2}\right). \end{eqnarray}$

Application of homogeneous balance rule on Eq (2.4) gives $N = 1$ . The solution takes the form

$\begin{equation} P(a) = A_{0}+A_{1}\left(\frac{1}{\epsilon e^{\delta a}+ \varepsilon e^{-\delta a}}\right). \end{equation}$

(3.3)

3.2. Mathematical analysis

Inserting Eq (3.3) into the ODE (2.4). A set of equations in $A_0$ and $A_1$ is yielded by plugging coefficients of different powers of $R(a)$ to zero. The values of $A_{0}$ and $A_{1}$ are extracted upon solving the obtained system. The extracted values have been summarized as below

$\begin{eqnarray} &&A_{0} = -\frac{\alpha}{3\beta}, \quad\quad A_{1} = \pm\frac{2\sqrt{2\epsilon\varepsilon}}{3\beta}, \\&& \; \gamma = \frac{\alpha^2}{9\beta \mu^2}, \quad\quad\quad \theta = -\frac{2\alpha^2\mu}{9\beta}. \end{eqnarray}$

Bright solitons have been obtained using the values of $A_{0}$ and $A_{1}$ in Eq (3.3) as

$\begin{eqnarray} \eta(x, t) = -\frac{\alpha}{3\beta}\pm\frac{2\sqrt{2\epsilon\varepsilon}}{3\beta}\left[\frac{1}{\epsilon e^{\delta (\mu x+\frac{2\alpha^2\mu}{9\beta}t)}+ \varepsilon e^{-\delta( \mu x+\frac{2\alpha^2\mu}{9\beta}t)}}\right]. \end{eqnarray}$

(3.4)

Upon choosing the values of constants, as $\beta = \epsilon = 1, \; \varepsilon = 1, \; \alpha = 1, \; \mu = \delta = 1$ , the above solution becomes

$\begin{eqnarray} \eta(x, t) = \frac{1}{3}\left[-1\pm \sqrt{2} {\rm{sech}}\left( x+\frac{2}{9} t\right)\right]. \end{eqnarray}$

(3.5)

4. Solitary wave solutions using Method 2: Generalized projective Ricatti equations (GPRE) method

This section deals with the general algorithm of the GPRE method and its application to the proposed equation.

4.1. Description of Method 2

According to GPRE method ^[25], the predicted solution of Eq (2.4) has the following form

$\begin{equation} P(a) = A_{0}+ A_{1}f(a)+B_{1}g(a), \end{equation}$

(4.1)

where $A_{0}, \; A_{1}$ and $B_{1}$ are unknowns to be evaluated. The ODEs satisfied by $f(a)$ and $g(a)$ have solutions of the following types.

Type 1.

When $e = -1$ , $r = -1$ , $R > 0$ ,

$\begin{equation} \nonumber f_{1}(a) = \frac{R {\rm{sech}}(\sqrt{R}a)}{m\; {\rm{sech}}(\sqrt{R}a)+1}, \quad\quad g_{1}(a) = \frac{\sqrt{R}\tanh(\sqrt{R}a)}{m\; {\rm{sech}}(\sqrt{R}a)+1}. \end{equation}$

Type 2.

When $e = -1$ , $r = 1$ , $R > 0$ ,

$\begin{equation} \nonumber f_{2}(a) = \frac{R {\rm{csch}}(\sqrt{R}a)}{m\; {\rm{csch}}(\sqrt{R}a)+1}, \quad\quad g_{2}(a) = \frac{\sqrt{R}\coth(\sqrt{R}a)}{m\; {\rm{csch}}(\sqrt{R}a)+1}. \end{equation}$

4.2. Mathematical analysis

Inserting Eq (4.1) into Eq (2.4), then plugging the coefficients of $g^{p}(a)$ , $p = 0, 1, \ldots$ to zero, a system of equations is extracted.

Upon solving the extracted system of equations, the constants $A_0$ , $A_1$ , $B_1$ , $m$ and $R$ , are determined. The obtained results are given as SET1 below:

$\begin{eqnarray} &&A_{0} = -\frac{\sqrt{\theta}}{\sqrt{2\mu}\sqrt{-\beta}}, \quad\quad A_{1} = \frac{\sqrt{(m^2+r)\mu^5}\gamma}{\sqrt{2\theta}\sqrt{-\beta}}, \quad B_1 = \mp\frac{\sqrt{\gamma}\mu}{\sqrt{2}\sqrt{-\beta}}, \\&& \alpha = -\frac{3\sqrt{-\beta}\sqrt{\theta}}{\sqrt{2\mu}}, \quad\quad R = \frac{\theta}{\gamma\mu^3}, \end{eqnarray}$

provided $\beta < 0$ , $\theta > 0$ and $\mu > 0$ .

The obtained results are given as SET2 below:

$\begin{eqnarray} &&A_{0} = 0, \quad\quad A_{1} = -\frac{\sqrt{2\theta(m^2+r)}}{R\sqrt{-\beta}\sqrt{\mu}}, \quad B_1 = 0, \\&& \alpha = -\frac{3m\sqrt{-\beta}\sqrt{\theta}}{\sqrt{2\mu(m^2+r)}}, \quad\quad R = \frac{\theta}{\gamma\mu^3}, \end{eqnarray}$

provided $\theta > 0, \; \mu > 0$ and $\beta < 0$ .

According to Type 1, the following solutions have been extracted for SET 1, as

$\begin{eqnarray} \eta(x, t) = -\frac{\sqrt{\theta}}{\sqrt{2\mu}\sqrt{-\beta}}+\frac{\sqrt{(m^2-1)\mu^5}\gamma}{\sqrt{2\theta}\sqrt{-\beta}} \left[\frac{R {\rm{sech}}(\sqrt{R}a)}{m\; {\rm{sech}}(\sqrt{R}a)+1}\right] \mp\frac{\sqrt{\gamma}\mu}{\sqrt{2}\sqrt{-\beta}}\left[\frac{\sqrt{R}\tanh(\sqrt{R}a)}{m\; {\rm{sech}}(\sqrt{R}a)+1}\right], \\ \end{eqnarray}$

(4.2)

where $a = \mu x-\theta t$ .

According to Type 2, solutions relating to SET 1 is given, as

$\begin{eqnarray} \eta(x, t) = -\frac{\sqrt{\theta}}{\sqrt{2\mu}\sqrt{-\beta}}+\frac{\sqrt{(m^2+1)\mu^5}\gamma}{\sqrt{2\theta}\sqrt{-\beta}} \left[\frac{R {\rm{csch}}(\sqrt{R}a)}{m\; {\rm{csch}}(\sqrt{R}a)+1}\right] \mp\frac{\sqrt{\gamma}\mu}{\sqrt{2}\sqrt{-\beta}}\left[\frac{\sqrt{R}\coth(\sqrt{R}a)}{m\; {\rm{csch}}(\sqrt{R}a)+1}\right], \\ \end{eqnarray}$

(4.3)

provided that $\left(m\; {\rm{csch}}(\sqrt{R}a)+1\right)\neq 0$ and $a = \mu x-\theta t$ .

According to Type 1, the following solutions have been extracted for SET 2 as

$\begin{eqnarray} \eta(x, t) = -\frac{\sqrt{2\theta(m^2+r)}}{R\sqrt{-\beta}\sqrt{\mu}} \left[\frac{R {\rm{sech}}(\sqrt{R}a)}{m\; {\rm{sech}}(\sqrt{R}a)+1}\right], \end{eqnarray}$

(4.4)

where $a = \mu x-\theta t$ .

According to Type 2, solutions relating to SET 2 is given, as

$\begin{eqnarray} \eta(x, t) = -\frac{\sqrt{2\theta(m^2+r)}}{R\sqrt{-\beta}\sqrt{\mu}} \left[\frac{R {\rm{csch}}(\sqrt{R}a)}{m\; {\rm{csch}}(\sqrt{R}a)+1}\right], \end{eqnarray}$

(4.5)

provided that $\left(m\; {\rm{csch}}(\sqrt{R}a)+1\right)\neq 0$ and $a = \mu x-\theta t$ .

5. Soliton solutions using Method 3: $\frac{G'}{G^2}$ -expansion method

In this section, solitons for the proposed model have been retrieved with the help of Method 3.

5.1. Description of $\frac{G'}{G^2}$ -expansion method

According to $\frac{G'}{G^2}$ -expansion method ^[23], the solution of (2.4) has the form

$\begin{eqnarray} P(a)& = & A_{0}+A_{1}\left(\frac{G^{'}}{G^{2}}\right)+B_{1}\left(\frac{G^{'}}{G^{2}}\right)^{-1}, \end{eqnarray}$

(5.1)

where $A_0$ , $A_1$ and $B_1$ are constants to be determined. The differential equation satisfied by $\frac{G'}{G^2}$ has three types of solutions ^[25].

Type 1.

If $\varpi \pi > 0$ ,

$\begin{eqnarray} \frac{G'}{G^2}& = &\sqrt{\frac{\varpi}{\pi}}\left[\frac{C\cos\sqrt{\varpi \pi}a +D\sin\sqrt{\varpi \pi}a}{C\cos\sqrt{\varpi \pi}a-D\sin\sqrt{\varpi \pi}a}\right], \end{eqnarray}$

(5.2)

provided that $\left(C\cos\sqrt{\varpi \pi}a-D\sin\sqrt{\varpi \pi}a\right)\neq 0$ .

Type 2.

If $\varpi \pi < 0$ ,

$\begin{eqnarray} \frac{G'}{G^2}& = &-\frac{\sqrt{|\varpi \pi|}}{\pi}\left[\frac{C\sinh(2\sqrt{|\varpi \pi|}a) +C\cosh(2\sqrt{|\varpi \pi|}a)+D}{C\sinh(2\sqrt{|\varpi \pi|}a)+C\cosh(2\sqrt{|\varpi \pi|}a)-D}\right]. \end{eqnarray}$

(5.3)

Type 3.

If $\varpi = 0, \pi\neq 0$ ,

$\begin{eqnarray} \frac{G'}{G^2}& = &-\frac{C}{\pi\left[C a+D\right]}, \end{eqnarray}$

(5.4)

where $C$ and $D$ are nonzero constants.

5.2. Mathematical analysis

Inserting Eq (5.1) into Eq (2.4), and equating the coefficients of each power of $\frac{G'}{G^2}$ to zero. A system of equations is obtained. Upon solving the system following solution sets are obtained as

Set 1.

$\begin{eqnarray} &&A_{0} = \frac{3\theta}{2\mu\alpha}, \quad\quad A_{1} = -\frac{3\sqrt{\mu \gamma \theta}\pi}{\alpha}, \quad B_1 = - \frac{3\sqrt{\theta^3}}{16\sqrt{\mu^5}\alpha\sqrt{\gamma} \pi}, \\&& \beta = -\frac{2\mu\alpha^2}{9\theta}, \quad \varpi \pi = -\frac{\theta}{16 \mu^3 \gamma}, \end{eqnarray}$

provided $\theta > 0$ and $\mu > 0$ .

Set 2.

$\begin{eqnarray} &&A_{0} = \frac{3\theta}{2\mu\alpha}, \quad\quad A_{1} = 0, \quad B_1 = \pm \frac{3\sqrt{\mu\gamma\theta}\varpi}{\alpha}, \\&& \beta = -\frac{2\mu\alpha^2}{9\theta}, \quad \varpi \pi = -\frac{\theta}{4 \mu^3\gamma}, \end{eqnarray}$

provided $\theta > 0$ and $\mu > 0$ .

Set 3.

$\begin{eqnarray} &&A_{0} = \frac{3\theta}{2\mu\alpha}, \quad\quad A_{1} = \pm \frac{3\sqrt{\mu\gamma\theta}\pi}{\alpha}, \quad B_1 = 0, \\&& \beta = -\frac{2\mu\alpha^2}{9\theta}, \quad \varpi \pi = -\frac{\theta}{4 \mu^3\gamma}, \end{eqnarray}$

provided $\theta > 0$ and $\mu > 0$ . According to Type 2, the solution for SET 1 is given, as

$\begin{eqnarray} \eta(x, t)& = &\frac{3\theta}{2\mu\alpha}- \frac{3\sqrt{\mu \gamma \theta}\pi}{\alpha}\left[-\frac{\sqrt{|\varpi \pi|}}{\pi}\left[\frac{C\sinh(2\sqrt{|\varpi \pi|}a) +C\cosh(2\sqrt{|\varpi \pi|}a)+D}{C\sinh(2\sqrt{|\varpi \pi|}a)+C\cosh(2\sqrt{|\varpi \pi|}a)-D}\right]\right]\\&&- \frac{3\sqrt{\theta^3}}{16\sqrt{\mu^5}\alpha\sqrt{\gamma} \pi}\left[-\frac{\sqrt{|\varpi \pi|}}{\pi}\left[\frac{C\sinh(2\sqrt{|\varpi \pi|}a) +C\cosh(2\sqrt{|\varpi \pi|}a)+D}{C\sinh(2\sqrt{|\varpi \pi|}a)+C\cosh(2\sqrt{|\varpi \pi|}a)-D}\right]\right]^{-1}, \end{eqnarray}$

where $a = \mu x-\theta t$ .

Choosing $C = D$ , the above solution becomes

$\begin{eqnarray} \eta(x, t)& = &\frac{3\theta\left[2+\coth\left(\frac{\sqrt{\theta}a}{4\sqrt{\mu^3\gamma}}\right)+\tanh\left(\frac{\sqrt{\theta}a}{4\sqrt{\mu^3\gamma}}\right)\right]}{4\mu \alpha}, \end{eqnarray}$

(5.5)

where $a = \mu x-\theta t$ .

According to Type 2, the solution for SET 2 is obtained by choosing $C = D$ , as

$\begin{eqnarray} \eta(x, t)& = &\frac{3\theta\left[1\mp\tanh\left(\frac{\sqrt{\theta}a}{2\sqrt{\mu^3\gamma}}\right)\right]}{2\mu \alpha}, \end{eqnarray}$

(5.6)

where $a = \mu x-\theta t$ .

According to Type 2, the solution for SET 3 is obtained by choosing $C = D$ , as

$\begin{eqnarray} \eta(x, t)& = &\frac{3\theta\left[1\mp\coth\left(\frac{\sqrt{\theta}a}{2\sqrt{\mu^3\gamma}}\right)\right]}{2\mu \alpha}. \end{eqnarray}$

(5.7)

6. Graphical illustration

In this section, the graphical descriptions of a few extracted solutions have been discussed. The graphical description is the fundamental tool used for visualizing the properties of solutions physically. The numerical simulations of the extracted solutions have been carried out using $3$ D-surface graphs and $2$ D-contour graphs.

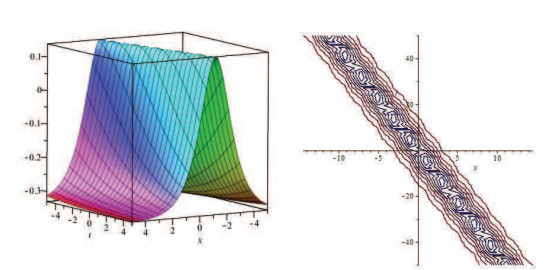
shows the graphical illustration for the solution expressed by Eq (3.5) which is determined by Kudryashov's $R$ function method. The graphs show that the solution represents a bright soliton. The corresponding contour plot is added to show the structure of the bright soliton.

Figure 1. The 3D graph for Set 1 in Eq (3.5).

DownLoad: Full-Size Img PowerPoint

The graphical simulations for three solutions retrieved by GPRE method are shown in Figures 2–4. The integral surface of the solution expressed by Eq (4.2) is presented in Figure 2 which shows that the wave profile sharply changes from one asymptotic state to another. This kind of traveling wave is termed as a kink soliton. Figure 3 presents the surface graph and contour plot for the solution given by Eq (4.4). The graphs represent a dark soliton as a localized decrease in the wave amplitude can be observed. Figure 4 shows that the solution expressed by Eq (4.5) represents a dark-bright soliton.

Figure 2. The 3D plot graph of Eq (4.2).

DownLoad: Full-Size Img PowerPoint

Figure 3. The 3D graph of Eq (4.4).

DownLoad: Full-Size Img PowerPoint

Figure 4. The 3D graph of Eq (4.5).

DownLoad: Full-Size Img PowerPoint

– provide the graphical representations for three solutions retrieved by $\frac{G'}{G^{2}}$ -expansion method. The graphs depict that a variety of solitonic behavior is evident corresponding to the obtained results.

Figure 5. The 3D graph of Eq (5.5).

DownLoad: Full-Size Img PowerPoint

Figure 6. The 3D graph of Eq (5.6).

DownLoad: Full-Size Img PowerPoint

Figure 7. The 3D graph of Eq (5.7).

DownLoad: Full-Size Img PowerPoint

7. Conclusions

In this research, we have investigated the most important model termed as "Gardner's equation" of quantum field theory and plasma physics. This research aims to extract different forms of soliton solutions, which are classified as singular soliton, bright soliton, dark soliton, and dark singular combo soliton solutions. Three powerful and reliable analytical techniques have made this retrieval of soliton solutions possible. The existence criteria have been discussed for all the obtained solutions. The derived results may help describe the related physical phenomena. On comparing our derived results with the results reported in ^{[18,42,43,44]}, it has been observed that our obtained soliton solutions are novel and have been firstly reported in this article. In future, we can apply the same technique as explained in ^[38] on "Gardner's equation" for retrieving intersecting results.

Acknowledgements

The authors extend their appreciation to the deputyship for Research and Innovation, Ministry of Education in Saudi Arabia for funding this research work through the project number (IFP-2020-XX).

Conflict of interest

The authors declare no conflict of interest.

References

[1]	W. X. Ma, Riemann-Hilbert problems and N-soliton solutions for a coupled mKdV system, J Geom. Phys., 132 (2018), 45–54. http://doi.org/10.1016/j.geomphys.2018.05.024 doi: 10.1016/j.geomphys.2018.05.024
[2]	G. Z. Wu, C. Q. Dai, Nonautonomous soliton solutions of variable-coefficient fractional nonlinear Schrödinger equation, J. Math. Lett., 106 (2020), 106365. http://doi.org/10.1016/j.aml.2020.106365 doi: 10.1016/j.aml.2020.106365
[3]	F. H. Ismael, H. Bulut, C. Park, M. S. Osman, M-lump, N-soliton solutions, and the collision phenomena for the (2 + 1) dimensional Date-Jimbo-Kashiwara-Miwa equation, Results Phys., 19 (2020), 103329. http://doi.org/10.1016/j.rinp.2020.103329 doi: 10.1016/j.rinp.2020.103329
[4]	M. B. Almatrafi, A. R. Alharbi, C. Tun, Constructions of the soliton solutions to the good Boussinesq equation, Adv. Differ. Equ., 2020 (2020), 629. http://doi.org/10.1186/s13662-020-03089-8 doi: 10.1186/s13662-020-03089-8
[5]	H. Rezazadeh, D. Kumar, A. Neirameh, M. Eslam, M. Mirzazadeh, Applications of three methods for obtaining optical soliton solutions for the Lakshmanan–Porsezian–Daniel model with Kerr law nonlinearity, Pramana, 94 (2020), 39. http://doi.org/10.1007/s12043-019-1881-5 doi: 10.1007/s12043-019-1881-5
[6]	N. Nasreen, A. R. Seadawy, D. C. Lu, Construction of soliton solutions for modified Kawahara equation arising in shallow water waves using novel techniques, Int. J. Mod. Phys. B, 34 (2020), 2050045. http://doi.org/10.1142/S0217979220500459 doi: 10.1142/S0217979220500459
[7]	N. Faraz, M. Sadaf, G. Akram, I. Zainab, Y. Khan, Effects of fractional order time derivative on the solitary wave dynamics of the generalized ZK-Burgers equation, Results Phys., 25 (2021), 104217. http://doi.org/10.1016/j.rinp.2021.104217 doi: 10.1016/j.rinp.2021.104217
[8]	G. Akram, M. Sadaf, S. Arshed, F. Sameen, Bright, dark, kink, singular and periodic soliton solutions of Lakshmanan-Porsezian-Daniel model by generalized projective Riccati equations method, Optik, 241 (2021), 167051. http://doi.org/10.1016/j.ijleo.2021.167051 doi: 10.1016/j.ijleo.2021.167051
[9]	M. A. Wasay, Nonreciprocal wave transmission through an extended discrete nonlinear Schrödinger dimer. Phys. Rev. E, 96 (2017), 052218. http://doi.org/10.1103/PhysRevE.96.052218 doi: 10.1103/PhysRevE.96.052218
[10]	M. A. Wasay, Asymmetric wave transmission through one dimensional lattices with cubic-quintic nonlinearity, Sci. Rep., 8 (2018), 5987. http://doi.org/10.1038/s41598-018-24396-x doi: 10.1038/s41598-018-24396-x
[11]	M. A. Wasay, M. L. Lyra, B. S. Ham, Enhanced nonreciprocal transmission through a saturable cubic-quintic nonlinear dimer defect, Sci. Rep., 9 (2019), 1871. http://doi.org/10.1038/s41598-019-38872-5 doi: 10.1038/s41598-019-38872-5
[12]	M. A. Wasay, M. Johansson, Multichannel asymmetric transmission through a dimer defect with saturable inter-site nonlinearity, J. Phys. A: Math. Theor., 53 (2020), 395702. http://doi.org/10.1088/1751-8121/aba145 doi: 10.1088/1751-8121/aba145
[13]	M. A. Wasay, F. X. Li, Q. H. Liu, Stationary transmission through lattices with asymmetric nonlinear quadratic-cubic defect, Phys. Lett. A, 447 (2022), 128301. http://doi.org/10.1016/j.physleta.2022.128301 doi: 10.1016/j.physleta.2022.128301
[14]	C. S. Gardner, J. S. Greene, M. D. Kruskal, R. M. Miura, Method for solving the Korteweg-deVries equation, Phys. Rev. Lett., 19 (1967), 1095. http://doi.org/10.1103/PhysRevLett.19.1095 doi: 10.1103/PhysRevLett.19.1095
[15]	R. Grimshaw, D. Pelinovsky, E. Pelinovsky, T. Talipova, Wave group dynamics in weakly nonlinear long-wave models, Phys. D: Nonlinear Phenom., 159 (2001), 35–57. http://doi.org/10.1016/S0167-2789(01)00333-5 doi: 10.1016/S0167-2789(01)00333-5
[16]	H. Gunerhan, Exact traveling wave solutions of the Gardner's equation by the improved-expansion method and the wave ansatz method, Math. Probl. Eng., 2020 2020, 5926836. http://doi.org/10.1155/2020/5926836 doi: 10.1155/2020/5926836
[17]	R. M. Miura, C. S. Gardner, M. D. Kruskal, Korteweg‐devries equation and generalizations, Ⅱ. Existence of conservation laws and constants of motion, J. Math. Phys., 9 (1968), 1204–1209. http://doi.org/10.1063/1.1664701 doi: 10.1063/1.1664701
[18]	Z. T. Fu, S. D. Liu, S. K. Liu, New kinds of solutions to Gardner's equation, Chaos Solitons. $&$ Fractal., 20 (2004), 301–309. http://doi.org/10.1016/S0960-0779(03)00383-7 doi: 10.1016/S0960-0779(03)00383-7
[19]	D. Daghan, O. Donmez, Exact solutions of the Gardner's equation and their applications to the different physical plasmas, Braz. J. Phys., 46 (2016), 321–333. http://doi.org/10.1007/s13538-016-0420-9 doi: 10.1007/s13538-016-0420-9
[20]	N. A. Kudryashov, Method for finding highly dispersive optical solitons of nonlinear differential equations, Optik, 206 (2020), 163550. http://doi.org/10.1016/j.ijleo.2019.163550 doi: 10.1016/j.ijleo.2019.163550
[21]	R. Conte, M. Musette, Link between solitary waves and projective Riccati equations, J. Phys. A: Math. Gen., 25 (1992), 5609.
[22]	W. A. Li, H. Chen, G. C. Zhang, The (w/g)-expansion method and its application to Vakhnenko equation, Chinese Phys. B, 18 (2009), 400.
[23]	S. Arshed, A. Biswas, F. Mallawi, M. R. Belic, Optical solitons with complex Ginzburg-Landau equation having three nonlinear forms, Phys. Lett. A, 383 (2019), 126026. http://doi.org/10.1016/j.physleta.2019.126026 doi: 10.1016/j.physleta.2019.126026
[24]	J. Dan, S. Sain, S. Ghose-Choudhury, S. Garai, Solitary wave solutions of nonlinear PDEs using Kudryashov's R function method, J. Mod. Optic., 67 (2020), 1499–1507. http://doi.org/10.1080/09500340.2020.1869850 doi: 10.1080/09500340.2020.1869850
[25]	M. Z. Elsayed, A. A. Khaled, The generalized projective Riccati equations method and its applications for solving two nonlinear PDEs describing microtubules, Int. J. Phys. Sci., 10 (2015), 391–402. http://doi.org/10.5897/IJPS2015.4289 doi: 10.5897/IJPS2015.4289
[26]	Z. Q. Li, S. F. Tian, J. J. Yang, On the soliton resolution and the asymptotic stability of N-soliton solution for the Wadati-Konno-Ichikawa equation with finite density initial data in space-time solitonic regions, Adv. Math., 409 (2022), 108639. http://doi.org/10.1016/j.aim.2022.108639 doi: 10.1016/j.aim.2022.108639
[27]	Z. Q. Li, S. F. Tian, J. J. Yang, Soliton resolution for the Wadati-Konno-Ichikawa equation with weighted Sobolev initial data, Ann. Henri Poincare, 23 (2022), 2611–2655. http://doi.org/10.1007/s00023-021-01143-z doi: 10.1007/s00023-021-01143-z
[28]	Z. Q. Li, S. F. Tian, J. J. Yang, E. G. Fan, Soliton resolution for the complex short pulse equation with weighted Sobolev initial data in space-time solitonic regions, J. Differ. Equations, 329 (2022), 31–88. http://doi.org/10.1016/j.jde.2022.05.003 doi: 10.1016/j.jde.2022.05.003
[29]	J. J. Yang, S. F. Tian, Riemann-Hilbert problem and dynamics of soliton solutions of the fifth-order nonlinear Schrödinger equation, Appl. Math. Lett., 128 (2022), 107904. http://doi.org/10.1016/j.aml.2022.107904 doi: 10.1016/j.aml.2022.107904
[30]	S. F. Tian, J. M. Tu, T. T. Zhang, Y. R. Chen, Integrable discretizations and soliton solutions of an Eckhaus-Kundu equation, Appl. Math. Lett., 122 (2021), 107507. http://doi.org/10.1016/j.aml.2021.107507 doi: 10.1016/j.aml.2021.107507
[31]	S. F. Tian, D. Guo, X. B. Wang, T. T. Zhang, Traveling wave, lump Wave, rogue wave, multi-kink solitary wave and interaction solutions in a (3+1)-dimensional Kadomtsev-Petviashvili equation with Bäcklund transformation, J. Appl. Anal. Comput., 11 (2021), 45–58. http://doi.org/10.11948/20190086 doi: 10.11948/20190086
[32]	J. J. Yang, S. F. Tian, Z. Q. Li, Riemann-Hilbert problem for the focusing nonlinear Schrödinger equation with multiple high-order poles under nonzero boundary conditions, Phys. D: Nonlinear Phenom., 432 (2022), 133162. http://doi.org/10.1016/j.physd.2022.133162 doi: 10.1016/j.physd.2022.133162
[33]	X. F. Zhang, S. F. Tian, J. J. Yang, Inverse scattering transform and soliton solutions for the Hirota equation with N distinct arbitrary order poles, Adv. Appl. Math. Mech., 14 (2022), 893–913.
[34]	Z. Y. Yin, S. F. Tian, Nonlinear wave transitions and their mechanisms of (2+1)-dimensional Sawada-Kotera equation, Phys. D: Nonlinear Phenom., 427 (2021), 133002. http://doi.org/10.1016/j.physd.2021.133002 doi: 10.1016/j.physd.2021.133002
[35]	X. B. Wang, S. F. Tian, Exotic vector freak waves in the nonlocal nonlinear Schrödinger equation, Phys. D: Nonlinear Phenom., 442 (2022), 133528. http://doi.org/10.1016/j.physd.2022.133528 doi: 10.1016/j.physd.2022.133528
[36]	S. F. Tian, M. J. Xu, T. T. Zhang, A symmetry-preserving difference scheme and analytical solutions of a generalized higher-order beam equation, P. Roy. Soc. A, 477 (2021), 20210455. http://doi.org/10.1098/rspa.2021.0455 doi: 10.1098/rspa.2021.0455
[37]	R. Z. Gong, D. S. Wang, Formation of the undular bores in shallow water generalized Kaup-Boussinesq model, Phys. D: Nonlinear Phenom., 439 (2022), 133398. http://doi.org/10.1016/j.physd.2022.133398 doi: 10.1016/j.physd.2022.133398
[38]	D. S. Wang, S. Y. Lou, Prolongation structures and exact solutions of equations, J. Math. Phys., 50 (2009), 123513. http://doi.org/10.1063/1.3267865 doi: 10.1063/1.3267865
[39]	D. S. Wang, L. Xu, Z. X. Xuan, The complete classification of solutions to the Riemann problem of the defocusing complex modified KdV equation, J. Nonlinear Sci., 32 (2022), 3. http://doi.org/10.1007/s00332-021-09766-6 doi: 10.1007/s00332-021-09766-6
[40]	D. S. Wang, B. L. Guo, X. L. Wang, Long-time asymptotics of the focusing Kundu-Eckhaus equation with nonzero boundary conditions, J. Differ. Equations, 266 (2019), 5209–5253. http://doi.org/10.1016/j.jde.2018.10.053 doi: 10.1016/j.jde.2018.10.053
[41]	D. S. Wang, Q. Li, X. Y. Wen, L. Liu, Matrix spectral problems and integrability aspects of the Błaszak-marciniak lattice equations, Rep. Math. Phys., 86 (2020), 325–353. http://doi.org/10.1016/S0034-4877(20)30087-2 doi: 10.1016/S0034-4877(20)30087-2
[42]	B. Ghanbari, D. Baleanu, New solutions of Gardner's equation using two analytical methods, Front. Phys., 7 (2019), 202. http://doi.org/10.3389/fphy.2019.00202 doi: 10.3389/fphy.2019.00202
[43]	K. J. Wang, Traveling wave solutions of the Gardner equation in dusty plasmas, Results Phys., 33 (2022), 105207. http://doi.org/10.1016/j.rinp.2022.105207 doi: 10.1016/j.rinp.2022.105207
[44]	A. Wazwaz, New solitons and kink solutions for the Gardner equation, Commun. Nonlinear Sci., 12 (2007), 1395–1404. http://doi.org/10.1016/j.cnsns.2005.11.007 doi: 10.1016/j.cnsns.2005.11.007

This article has been cited by:

1.	Guobin Lin, Hu Huang, The Dynamical and Kinetic Equations of Four-Five-Six-Wave Resonance for Ocean Surface Gravity Waves in Water with a Finite Depth, 2024, 16, 2073-8994, 618, 10.3390/sym16050618
2.	Aamir Farooq, Wen Xiu Ma, Muhammad Ishfaq Khan, Exploring exact solitary wave solutions of Kuralay-II equation based on the truncated M-fractional derivative using the Jacobi Elliptic function expansion method, 2024, 56, 1572-817X, 10.1007/s11082-024-06841-6
3.	Nisa Çelik, Duygu Tetik, New dynamical analysis of the exact traveling wave solutions to a (3+1)-dimensional Gardner-KP equation by three efficient architecture, 2024, 179, 09600779, 114444, 10.1016/j.chaos.2023.114444
4.	Jing Liu, Zhao Li, Bifurcation Analysis and Soliton Solutions to the Kuralay Equation Via Dynamic System Analysis Method and Complete Discrimination System Method, 2024, 23, 1575-5460, 10.1007/s12346-024-00990-5
5.	Hajar F. Ismael, Haci Mehmet Baskonus, Azad Piro Shakir, Hirota–Maccari system arises in single-mode fibers: abundant optical solutions via the modified auxiliary equation method, 2024, 56, 1572-817X, 10.1007/s11082-024-06698-9
6.	Guiming Jin, Xueping Cheng, Jianan Wang, Hailiang Zhang, (3+1)-Dimensional Gardner Equation Deformed from (1+1)-Dimensional Gardner Equation and its Conservation Laws, 2024, 19, 0973-5348, 9, 10.1051/mmnp/2024004

Reader Comments

Your name:*

Email:*
© 2023 the Author(s), licensee AIMS Press. This is an open access article distributed under the terms of the Creative Commons Attribution License (http://creativecommons.org/licenses/by/4.0)

通讯作者: 陈斌, bchen63@163.com

1.
沈阳化工大学材料科学与工程学院沈阳 110142

AIMS Mathematics

1.8 3.4

Metrics

Article views(1789) PDF downloads(97) Cited by(6)

Preview PDF

Download XML

Export Citation

Article outline

Show full outline

Figures and Tables

Figures(7)

AIMS Mathematics

Abundant solitary wave solutions of Gardner's equation using three effective integration techniques

Related Papers:

Abstract

1. Introduction and preliminary results

2. Governing equation

3. Solitary wave solutions using Method 1: Kudryashov's R function method

3.1. Description of Method 1

3.2. Mathematical analysis

4. Solitary wave solutions using Method 2: Generalized projective Ricatti equations (GPRE) method

4.1. Description of Method 2

4.2. Mathematical analysis

5. Soliton solutions using Method 3: $\frac{G'}{G^2}$ -expansion method

5.1. Description of $\frac{G'}{G^2}$ -expansion method

5.2. Mathematical analysis

6. Graphical illustration

7. Conclusions

Acknowledgements

Conflict of interest

References

This article has been cited by:

Reader Comments

通讯作者: 陈斌, bchen63@163.com

Metrics

Figures and Tables

Other Articles By Authors

Catalog

AIMS Mathematics

Abundant solitary wave solutions of Gardner's equation using three effective integration techniques

Related Papers:

Abstract

1. Introduction and preliminary results

2. Governing equation

3. Solitary wave solutions using Method 1: Kudryashov's R function method

3.1. Description of Method 1

3.2. Mathematical analysis

4. Solitary wave solutions using Method 2: Generalized projective Ricatti equations (GPRE) method

4.1. Description of Method 2

4.2. Mathematical analysis

5. Soliton solutions using Method 3: G′G2 \frac{G'}{G^2} -expansion method

5.1. Description of G′G2 \frac{G'}{G^2} -expansion method

5.2. Mathematical analysis

6. Graphical illustration

7. Conclusions

Acknowledgements

Conflict of interest

References

This article has been cited by:

Reader Comments

通讯作者: 陈斌, bchen63@163.com

Metrics

Figures and Tables

Other Articles By Authors

Related pages

Tools

Export File

Citation

Format

Content

Catalog

5. Soliton solutions using Method 3: $\frac{G'}{G^2}$ -expansion method

5.1. Description of $\frac{G'}{G^2}$ -expansion method