W-shaped soliton solutions to the modified Zakharov-Kuznetsov equation of ion-acoustic waves in (3+1)-dimensions arise in a magnetized plasma

1.   Introduction and preliminaries

Consider a simple and connected graph. Let $V(G)$ represent the vertex set of $G$ and $E(G)$ represent the edge set of $G$. The distance between two vertices $a$ and $b$ in a graph $G$, denoted by $d(a, b)$, represents the number of edges connecting them. Let $Q$ be an ordered set vertices $a_1, a_2, \dots, a_k$ of the given graph. A vertex $a\in V(G)$ and the representation $r(a|Q)$ of a vertex $a$ with respect to $Q$ is the vector $(d(a|a_1), d(a|a_2), \dots, d(a|a_k))$. A set $Q$ is called the resolving set if all vertices have unique representations with respect to $Q$. The metric dimension of a graph, denoted as $dim(G)$, is the minimum cardinality of the resolving set. This concept is interested by the challenge of exactly defining the location of a vertex in relation to a particular group of vertices. Slater presented the idea of metric dimension and mentioned to it as a locating set in ^[1,2]. Later, Harary and Melter showed separate investigations into the same idea, that they called metric dimension ^[3,4]. The literature gives a detailed information of resolving set, metric basis, and metric dimension ^[5,6]. Further deliberations on different subjects are presented in the references ^[7,8,9].

Consider a $k$-ordered partition of vertices of $G$ represented as ${R}{p} = \{{R}{p_1}, {R}{p_2}, \dots, {R}{p_k}\}$ and the representation $r(a|{R}{p})$ of a vertex $a$ with respect to ${R}{p}$ is the vector $(d(a|{R}{p_1}), d(a|{R}{p_2}), \dots, d(a|{R}{p_k}))$. The partition is called a resolving partition of $G$ if $r(a|{R}{p}) \ne r(b|{R}{p})$ for all distinct $a, b\in V(G)$. The partition dimension of a graph, denoted by $pd(G)$, is the cardinality of a minimum resolving partition of $G$. Chartrand et al. defined this definition in their work and determining the classification of the partition dimension of any connected, simple graph as an NP-hard problem has been decisively recognized.

The literature extensively discusses the topic of partition resolving set and partition dimension. The partition dimension of the $(4, 6)$ fullerene was computed in ^[10], revealing that its partition dimension is limited. The study in ^[11] examines the bounded partition dimension of the Cartesian product of graphs. The authors of the paper ^[12] defined limits for dividing different graphs into smaller parts. The authors of ^[13] outline the constraints of trees. The study of the boundaries of uni-cyclic graphs is conducted by examining subgraphs, as described in ^[14]. The honeycomb network is examined in ^[15], whereas ^[16] specifically explores architectures with a partition dimension of three. In ^[17], authors investigated a few structures linked to wheels and cycles.

The partition dimension is considered to be a logical extension of the metric dimension, and a correlation between the metric dimension and partition dimension of a graph G is established in Chartrand's work ^[18] as follows:

Resolving partitions are utilized in diverse domains, including network verification and discovery ^[19], robot navigation ^[20], the Djokovic-Winkler relation with the metric dimension, discussed by Hernando ^[21], and resolving sets applied to mastermind game strategies as described in ^[22]. Furthermore, the applications of resolving sets are thoroughly explained in the references cited as ^[23,24]. To gain additional understanding of the practical uses of this concept in networks, specifically in the fields of electronics and the polyphenyl industry, please consult the references ^{[25,26,27,28,29]}.

Theorem 1.1. ^[30] Let ${R_p}$ be a resolving partition of $V(G)$ and $a, b\in V(G)$. If $d(a, x) = d(b, x)$ for all vertices $x \in V(G)\backslash (a, b)$, then $a, b$ belong to different classes of ${R_p}$.

Theorem 1.2. ^[30] Let $G$ be a simple and connected graph, then

● $pd(G)$ is $2$ iff $G$ is a path graph;

● $pd(G)$ is $n$ iff $G$ is a complete graph.

Obtaining consistent and accurate values for the partition dimension is a complex job, which makes the calculation of bounds for the partition dimension of graphs a difficult effort. This study has determined precise and constant partition dimension of different convex structures of polytopes, specifically referred to as $\mathbb{T}_n, \ \mathbb{U}_n, \ \mathbb{V}_n, \ \mathbb{A}_n$.

Convex polytopes and their importance:

Convex polytopes are fundamental geometric objects with wide range applications in various academic disciplines. Due to diverse properties and extensive range of operations, the practicality of these geometrical entities become more useful in different application areas. Geometric modelling refers to the process of creating mathematical representations and their visualization in precise and detailed manner. These objects become more beneficial for representing and analyzing complex geometric arrangements in two and three-dimensional spaces due to their inherent simplicity and well-defined geometric properties. Linear programming: Convex polytopes play vital role in the field of linear programming, which is a mathematical approach used for optimizing the solutions. The feasible zone in a linear programming is typically illustrated as a convex polytope. Linear constraints can be effectively addressed within this geometric framework for optimization problems. Computational geometry is a field of study that focuses on the development and analysis of algorithms for solving geometric problems. Computational geometry utilizes the methods that specifically deal with convex polytopes to tackle issues related to geometric objects. Convex hull algorithms are used for optimization in several computer applications particularly in computer graphics.

Combinatorial structure refers to the arrangement and organization of elements of a discrete structure, to define the families of convex polytopes combinatorial properties defines lot of theorems to find the equivalence among the structures. Analytical studies on the facets, vertices, and edges of convex polytopes provides useful insights into the combinatorial properties of the corresponding polytope. This analysis makes important contributions to the fields of combinatorial geometry and combinatorics. Polyhedral combinatorics: Polyhedral combinatorics is the investigation of the combinatorial properties of polyhedra and polytopes, with specific focus on convex polytopes, which are highly interrelated in this area of research. Statistical modelling and data analysis: Convex polytopes are extensively utilized in statistics for data modelling and analysis. In robust statistics, convex hulls are used to define central regions that are less affected by outliers, hence enabling more robust estimation of central tendencies.

Operations research: Convex polytopes have practical uses in operations research as they are used to specify feasible regions and constraints in optimization problems. Linear programming, integer programming, and other optimization methods make use of the geometric properties of convex polytopes to efficiently solve problems. Quantum information theory: Convex polytopes are employed in quantum information theory for representing the set of quantum states. This application is essential for understanding and describing the behavior of quantum systems, which can be employ in the fields of quantum computing and communication. Topological data analysis: Convex structures are used in the discipline of topological data analysis to investigate the geometric attributes and topological features of data. This phenomenon has important implications for understanding the fundamental structure of complex datasets in various fields, including biology and neuroscience. Polytope theory is a branch of algebraic geometry. Toric varieties establish a direct connection between the study of convex polytopes and algebraic geometry. Polytope theory provides a geometric framework for characterizing algebraic varieties, making them more useful in the fields of algebraic geometry and mathematical physics.

The fundamental geometric simplicity of these objects, together with their vast combinatorial and computational properties, make them particularly effective tools for modelling, analysis, and problem-solving in several mathematical fields and beyond. More detailed information about convex polytopes can be found in references ^[30,31,32].

2.   The $\mathbb{T}_n$ convex polytope-like graph

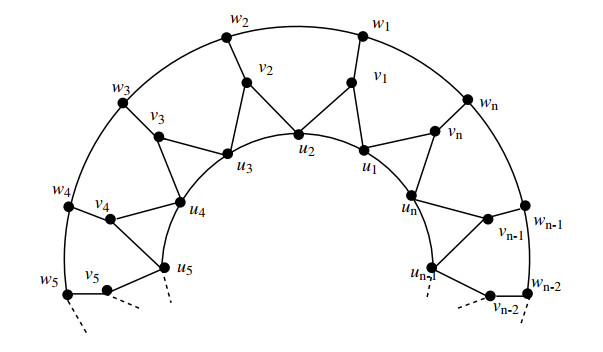
The graph illustrated in Figure 1 portrays a distinct variant of a convex polytope graph, denoted as $\mathbb{T}_n$. All convex polytopes in this family are planar graphs. The creation of the convex polytope graph $\mathbb{T}_n$ can be obtained by modifying the structure of the convex polytope graph $R_n$ described in ^[33]. This modification involves eliminating the edges $v_{\phi} v_{{\phi}+1}$. The graph $\mathbb{T}_n$ is a convex polytope that consists of ${n}$, ${5}$, and ${3}$-dimensional faces. In accordance with the fundamental definition, the vertex and edge sets are defined as $V({\mathbb{T}_n}) = {V(R_n)}$ and $E(\mathbb{T}_n) = \{E(R_n)\} \backslash \{v_{\phi} v_{{\phi}+1}: 1\leq {\phi} \leq n\}$, respectively. It is crucial to emphasise that in every construction of convex polytope graphs, we take into account that ${v}_{n+1} = {v}_{1}$. For a more comprehensive analysis of convex polytopes, additional sources can be referred ^[34,35,36].

We split the vertices $\{u_{\phi}, v_{\phi}, w_{\phi} : 1\leq {\phi} \leq n\}$ as inner, central, and outer cycles respectively.

Theorem 2.1. If $\mathbb{T}_n$ is a convex polytope-like graph, given $n\geq6$, then $pd(\mathbb{T}_n) = 4$.

Proof. We split the proof into two steps as:

Step 1: For $n = {2{\psi} }, {{\psi}} \geq 3, {{\psi}} \in N$, let ${R_p} = \{{R_p}_1, {R_p}_2, {R_p}_3, {R_p}_4\}$ be a resolving set, where ${R_p}_1 = \{u_1\}$, ${R_p}_2 = \{u_3\}$, ${R_p}_3 = \{u_{{{\psi}}+1}\}$, and ${R_p}_4 = V(\mathbb{T}_n) \setminus \{{R_p}_1, {R_p}_2, {R_p}_3\}$. Here, we showed the distinct representation of all vertices $V(\mathbb{T}_n)\backslash{{R_p}_1\cup{R_p}_2\cup{R_p}_3}$ with respect to the partition resolving set ${R_p}$.

The representation of vertices of inner, central, and outer cycles are given below:

We can see that every vertex $v\in V(\mathbb{T}_n)$ has distinct representation in correspondence with the partition resolving set ${R_p}$ for $n$ even.

Step 2: For $n = {2{\psi}} + 1, \; {{\psi}} \geq 3, \; {{\psi}} \in N$, let ${R_p} = \{{R_p}_1, {R_p}_2, {R_p}_3, {R_p}_4\}$ be a resolving set, where ${R_p}_1 = \{u_1\}$, ${R_p}_2 = \{u_2\}$, ${R_p}_3 = \{u_{{{\psi}}+1}\}$, and ${R_p}_4 = V(\mathbb{T}_n) \setminus \{{R_p}_1, {R_p}_2, {R_p}_3\}$. The representation of all inner, central, and outer cycles vertices with respect to the partition resolving set ${R_p}$ are given below:

We can see that every vertex $v\in V(\mathbb{T}_n)$ has distinct representation in correspondence with the partition resolving set ${R_p}$ for $n$ odd. From Steps 1 and 2, we can conclude that all the vertices that have distinct representation hence, $pd(\mathbb{T}_n) \leq 4$.

For the purpose of contradiction, lets assume that there exists a resolving set $R_{p}$ of cardinality equal to 3, to distinguish the path for $\mathbb{T}_n$. This means any two different vertices $u$ and $v$ belonging to $V(\mathbb{T}_n)$, there exists a vertex $r \in R_{p}$ such that the distance between $u$ and $r$ is not equal to the distance between $v$ and $r$. Nevertheless, given the distinct structure and characteristics of the convex polytope graph $\mathbb{T}_n$, it is feasible to ascertain that the vertices may be unequivocally distinguished based on their respective distances from the vertices in the resolving set $R_{p}$.

Specifically, the positions of the vertices $u_{\phi}, v_{\phi}$, and $w_{\phi}$ in the inner, central, and outer cycles, respectively, can be uniquely determined by the distances to the resolving set $R_{p}$. Now, let us take a pair of vertices denoted as $u_{\phi}$ and $w_{\phi}$, where $\phi$ is a variable. These vertices are located in the inner and outer cycles, respectively, and they possess identical distance values to the resolving set $R_{p}$.

Hence, the existence of any resolving set $R_{p}$ that can differentiate between $u_{\phi}$ and $w_{\phi}$ contradicts the assumption that the persistence diagram of $\mathbb{T}_n$ has a persistence dimension of 3. This contradiction suggests that the partition dimension of $\mathbb{T}_n$ is not equals to three. Hence, $pd(\mathbb{T}_n) \neq 3$, supports the converse proof. Therefore, we can conclude that $pd(\mathbb{T}_n) = 4$.

3.   The $\mathbb{U}_n$ convex polytope-like graph

Figure 2 illustrates the graph of convex polytope $\mathbb{U}_n$. The construction of this specific type, $\mathbb{U}_n$-convex polytope graph, can be derived from $R_n$-convex polytope graph as presented in ^[33] by incorporating new $x_{\phi} x_{{\phi}+1}$-edges. The graph $\mathbb{U}_n$ consists of ${n}$, $6$, $5$, and $3$-sided faces.

The vertex and edge sets are defined as follows:

There are $5n$, $8n$, and $3n+2$ number of vertices, edges, and faces of $\mathbb{U}_n$, respectively. For our simplicity the vertices are categorized as: inner cycle vertices ($\{u_{{\phi}}: 1\leq {\phi}\leq n\}$); interior vertices ($\{v_{{\phi}}: 1\leq {\phi}\leq n\}$); middle vertices ($\{w_{{\phi}}: 1\leq {\phi}\leq n\}$); exterior vertices ($\{x_{{\phi}}: 1\leq {\phi}\leq n\}$) and outer vertices ($\{y_{{\phi}}: 1\leq {\phi}\leq n\}$). Furthermore, Figure 2 introduces a generalized approach for labeling vertices.

In the next theorem, we showed that the partition dimension of the convex polytope graph $\mathbb{U}_n$ is four.

Theorem 3.1. For $n\geq6$, if $\mathbb{U}_n$ is a convex polytope-like graph, then $pd(\mathbb{U}_n) = 4$.

Proof. We split the proof into two steps as:

Step 1: When $n = {2{\psi}}, \; {{\psi}} \geq 3, \; {{\psi}} \in N$. let ${R_p} = \{{R_p}_1, {R_p}_2, {R_p}_3, {R_p}_4\}$ be a resolving set where ${R_p}_1 = \{u_1\}$, ${R_p}_2 = \{a_2\}$, ${R_p}_3 = \{u_{{{\psi}}+1}\}$, and ${R_p}_4 = V(\mathbb{U}_n) \setminus \{{R_p}_1, {R_p}_2, {R_p}_3\}$. Here, we showed the distinct representation of all vertices $V(\mathbb{U}_n)\backslash{{R_p}_1\cup{R_p}_2\cup{R_p}_3}$ with respect to the partition resolving set ${R_p}$. The representation of the vertices of convex polytope graph with respect to ${R_p}$ is given below:

We can see that every vertex $v\in V(\mathbb{U}_n)$ has distinct representation in correspondence with the partition resolving set ${R_p}$ for $n$ even.

Step 2: When $n = {2{\psi}} + 1, \; {{\psi}} \geq 3, \; {{\psi}} \in N$. Let ${R_p} = \{{R_p}_1, {R_p}_2, {R_p}_3, {R_p}_4\}$ be a resolving set where ${R_p}_1 = \{u_1\}$, ${R_p}_2 = \{u_2\}$, ${R_p}_3 = \{u_{{{\psi}}+1}\}$, and ${R_p}_4 = V(\mathbb{U}_n) \setminus \{{R_p}_1, {R_p}_2, {R_p}_3\}$. Here, we showed the distinct representation of all vertices $V(\mathbb{U}_n)\backslash{{R_p}_1\cup{R_p}_2\cup{R_p}_3}$ with respect to the partition resolving set ${R_p}$.

We can see that every vertex $v\in V(\mathbb{U}_n)$ has distinct representation in correspondence with the partition resolving set ${R_p}$ for $n$ even. From Steps 1 and 2, we can conclude that all the vertices that have distinct representation hence, $pd(\mathbb{U}_n) \leq 4$.

Every category of a vertex (namely, inner, interior, middle, exterior and outer) possesses a distinct positional relationship in relation to the resolving set. The unique location of the vertices can determine by evaluating their distances from the resolving set. The distinguishability of cycles in a graph is determined by the distinct structural functions played by different types of vertices within these cycles. The aforementioned structural differentiation facilitates the process of partitioning.

The $x_{\phi} x_{{\phi}+1}$-edges can significantly improve the connectivity and structural properties of the graph. The presence of these supplementary edges enhances the distinct identification of vertices and provides additional evidence in favor of an upper limit of 4 in the partition dimension. The given configuration, consisting of $5n$ vertices, $8n$ edges, and $3n+2$ faces, corresponds to the anticipated complexity that requires a higher partition dimension. The necessity for a resolving set of minimum four subgroups is underscored by the presence of structural variety observed in these counts.

The generalized labeling strategy, illustrated in Figure 2, gives a logical approach for assigning labels to vertices. The act of labeling aids in creating a resolving set that can differentiate vertices by their respective positions. In conclusion, the evidence provided substantiates the assertion that the upper limit of the partition dimension for the convex polytope graph $\mathbb{U}_n$ is 4. The explanation for this upper bound is supported by several factors, including the distinct positioning of vertices, the presence of unique cycles, the inclusion of additional edges, the calculation of structural counts, and the use of a generalized labeling approach. Understanding this concept is crucial for creating resolving sets that are successful and for completely analyzing the partitioning properties of the graph.

4.   The $\mathbb{V}_n$ convex polytope-like graph

The structure of the convex polytope graph $S_n$ is explained in ^[37]. This convex polytope graph $\mathbb{V}_n$ is derived from $S_n$ by introducing additional $v_{\phi} v_{{\phi}+1}$-edges. The vertex and edge set of $\mathbb{V}_n$ are defined as $V(\mathbb{V}_n) = V(\mathbb{U}_n)$ and $E(\mathbb{V}_n) = E(\mathbb{U}_n)\cup\{v_{\phi} v_{{\phi}+1}:1\leq {\phi}\leq n\}\setminus \{x_{\phi} x_{{\phi}+1}:1\leq {\phi}\leq n\}$, respectively.

For our convenient, the arrangements of cycles and vertices that are shown in Figure 3 are considered as: inner cycle ($\{u_{\phi}: 1\leq {\phi}\leq n\}$); middle cycle ($\{v_{\phi}: 1\leq {\phi}\leq n\}$); interior vertices ($\{w_{\phi}: 1\leq {\phi}\leq n\}\cup\{x_{\phi}: 1\leq {\phi}\leq n\}$) and outer cycle ($\{y_{\phi}: 1\leq {\phi}\leq n\}$).

In the next theorem, we determined partition dimension for the convex polytope graph $\mathbb{V}_n$. For the upper bound of the partition dimension of $\mathbb{V}_n$, it sufficient to prove that the $4$ subsets are enough for different representation of vertices.

Theorem 4.1. If $\mathbb{V}_n$ is a convex polytope-like graph, where $n\geq6$, then $pd(\mathbb{V}_n) = 4$.

Proof. We split the proof into two steps as:

Step 1: When $n = {2{\psi}}, \; {{\psi}} \geq 3, \; {{\psi}} \in N$. Let ${R_p} = \{{R_p}_1, {R_p}_2, {R_p}_3, {R_p}_4\}$ be a resolving set where ${R_p}_1 = \{u_1\}$, ${R_p}_2 = \{a_2\}$, ${R_p}_3 = \{u_{{{\psi}}+1}\}$, and ${R_p}_4 = V(\mathbb{V}_n) \setminus \{{R_p}_1, {R_p}_2, {R_p}_3\}$. Here, we showed the distinct representation of all vertices $V(\mathbb{V}_n)\backslash{{R_p}_1\cup{R_p}_2\cup{R_p}_3}$ with respect to the partition resolving set ${R_p}$.

As we can see that every vertex $v\in V(\mathbb{V}_n)$ are having distinct representations in correspondence with the partition resolving set ${R_p}$ for given $n$ is even.

Step 2: When $n = {2{\psi}} + 1, \; {{\psi}} \geq 3, \; {{\psi}} \in N$. Let ${R_p} = \{{R_p}_1, {R_p}_2, {R_p}_3, {R_p}_4\}$ be a resolving set where ${R_p}_1 = \{u_1\}$, ${R_p}_2 = \{u_2\}$, ${R_p}_3 = \{u_{{{\psi}}+1}\}$, and ${R_p}_4 = V(\mathbb{V}_n) \setminus \{{R_p}_1, {R_p}_2, {R_p}_3\}$. Here, we showed the distinct representation of all vertices $V(\mathbb{V}_n)\backslash{{R_p}_1\cup{R_p}_2\cup{R_p}_3}$ with respect to the partition resolving set ${R_p}$.

Again, we can see that every vertex $v\in V(\mathbb{V}_n)$ are having distinct representations in correspondence with the partition resolving set ${R_p}$ for given $n$ is odd. From Steps 1 and 2, we can conclude that no two vertices that have same representation hence, $pd(\mathbb{V}_n) \leq 4$.

This statement suggests that for any distinct vertices $u$ and $v$ in the vertex set $V(\mathbb{V}_n)$, for a given graph, there must exist a vertex $r$ in the set $R_{p}$ such that the distance between $u$ and $r$ is not equal to the distance between $v$ and $r$.

Now, let us examine the structure and cycles within the vertices $\mathbb{V}_n$ of the graph. The set of vertices $\{u_{\phi}: 1\leq {\phi}\leq n\}$ represents the inner cycle. The set of vertices $\{v_{\phi}: 1\leq {\phi}\leq n\}$ represents the central cycle. The set of vertices $\{w_{\phi}: 1\leq {\phi}\leq n\}\cup\{x_{\phi}: 1\leq {\phi}\leq n\}$ represents the middle cycle. The cycle consists of vertices $\{y_{\phi}: 1\leq {\phi}\leq n\}$ is referred to the outer cycle.

Now, let us consider the vertices denoted by $u_{1}$ and $y_{1}$, from the inner and outer cycles, respectively. Given that they are in distinct cycles, it follows that the distances to any partition resolving set $R_{p}$ must be unique. Nevertheless, the incorporation of the variable $v_{\phi} v_{{\phi}+1}$. The presence of such edges in the set of vertices $\mathbb{V}_n$ establishes a structural linkage connecting the inner and outer cycles by means of the middle cycle. This relationship serves to undermine the distinctiveness of distances and ultimately gives rise to a paradox.

Let $r \in R_{p}$ be a vertex in the partition resolving set that distinguishes distances. The presence of $v_{\phi} v_{{\phi}+1}$-edges introduces connectivity, which in turn affects the distance between $u_{1}$ and $r$ due to the influence of the middle cycle. The distance between $y_{1}$ and $r$ is affected by the intermediate cycle in a similar manner. The existence of this shared impact challenges the premise that the distance between $u_{1}$ and $r$ is not equal to the distance between $y_{1}$ and $r$.

The presence of a contradiction suggests that the premise of $pd(\mathbb{V}_n) = 3$ is invalid. Hence, the partition dimension of $\mathbb{V}_n$ is not equal to three. Therefore, the contrary demonstration demonstrates that the partition dimension of the graph of the convex polytope $\mathbb{V}_n$ is not three, thereby providing support for the earlier assertion that dividing sets into four subsets is adequate.

5.   The $\mathbb{A}_n$ convex polytope-like graph

The structure of convex polytope graph $R_n$ was defined in ^[38] and its further detail can be find in ^[39,40]. Also, the graph of convex $\mathbb{A}_n$ can be obtained by $R_n$ by adding the edge $\{w_{\phi} v_{{\phi}+1}:1\leq {\phi}\leq n\}$. Accordingly to Figure 4, the vertex and edge set defined as: $V(\mathbb{A}_n) = V(R_n) = \{u_{\phi}, v_{\phi}, w_{\phi}: 1\le \phi \le n\}$ and $E(\mathbb{A}_n) = E(R_n)\cup\{w_{\phi} v_{{\phi}+1}:1\leq {\phi}\leq n\} = \{u_{\phi}u_{\phi+1}, v_{\phi}v_{\phi+1}, w_{\phi}w_{\phi+1}, u_{\phi}v_{\phi}, v_{\phi}w_{\phi}, v_{\phi}u_{\phi+1}, w_{\phi}v_{\phi+1}: 1\leq {\phi}\leq n \}$.

Theorem 5.1. If $\mathbb{A}_n$ is a convex polytope-like graph, where $n\geq6$, then $pd(\mathbb{A}_n) = 4$.

Proof. We split the proof into two steps as:

Step 1: When $n = {2{\psi}}, \; {{\psi}} \geq 3, \; {{\psi}} \in N$. Let ${R_p} = \{{R_p}_1, {R_p}_2, {R_p}_3, {R_p}_4\}$ be a partition resolving set, where ${R_p}_1 = \{u_1\}$, ${R_p}_2 = \{u_2\}$, ${R_p}_3 = \{u_{{{\psi}}+1}\}$, and ${R_p}_4 = V(\mathbb{A}_n) \setminus \{{R_p}_1, {R_p}_2, {R_p}_3\}$. Here, we showed the distinct representation of all vertices $V(\mathbb{A}_n)\backslash{{R_p}_1\cup{R_p}_2\cup{R_p}_3}$ with respect to the partition resolving set ${R_p}$.

As we can see that every vertex $v\in V(\mathbb{A}_n)$ are having distinct representations in correspondence with the partition resolving set ${R_p}$ for $n$ even.

Step 2: When $n = {2{\psi}}+1, \; {{\psi}} \geq3, \; {{\psi}}\in N$. Let ${R_p} = \{{R_p}_1, {R_p}_2, {R_p}_3, {R_p}_4\}$ be a partition resolving set where ${R_p}_1 = \{u_1\}$, ${R_p}_2 = \{v_1\}$, ${R_p}_3 = \{w_{{{\psi}}+1}\}$, and ${R_p}_4 = V(\mathbb{A}_n) \setminus \{{R_p}_1, {R_p}_2, {R_p}_3\}$. Here, we showed the distinct representation of all vertices $V(\mathbb{A}_n)\backslash{{R_p}_1\cup{R_p}_2\cup{R_p}_3}$ with respect to the partition resolving set ${R_p}$.

Again, we can see that every vertex $v\in V(\mathbb{A}_n)$ are having distinct representations in correspondence with the partition resolving set ${R_p}$ for $n$ odd. From Steps 1 and 2, we can conclude that no two vertices that have same representation hence, $pd(\mathbb{A}_n) \leq 4$.

The convex polytope graph $\mathbb{A}_n$ is obtained by augmenting the structure $R_n$ with extra edges of the type $w_{\phi} v_{{\phi}+1}$. This configuration yields a graph characterized by a certain distribution of vertices and edges, which subsequently gives rise to faces of order ${n}$. The sets of edges and vertices are explicitly defined as follows: The vertex set of the graph $\mathbb{A}n$ is equal to the vertex set of the graph $R_n$, denoted by $V(\mathbb{A}_n) = V(R_n)$, and the edge set of $\mathbb{A}_n$ is equal to the union of edge set of $R_n$ and set of edges of the form $w_{\phi} v_{{\phi}+1}$, where $1\leq {\phi}\leq n$. The cycles depicted in the graph are classified into distinct categories.

The cyclic structure that comprises of vertices ${u_{\phi}: 1\leq {\phi}\leq n}$ is recognized as the inner cycle. The middle cycle is composed of vertices ${v_{\phi}: 1\leq {\phi}\leq n}$. The outer cycle is denoted by ${w_{\phi}: 1\leq {\phi}\leq n}$. The approach depicted in Figure 4 illustrates a generalized labeling technique that gives a logical strategy for assigning labels to the vertices.

The graph exhibits the distinct structural role for each cycle, like inner, middle and outer cycles. The unique location of vertices plays a significant role in facilitating the identification of vertices by their respective cycle memberships. The incorporation of $w_{\phi} v_{{\phi}+1}$-edges significantly improves the connectedness and structural intricacy of the graph. Addition of these supplementary edges enhanced the distinction between vertices and provides evidence in favor of an upper limit of 4 for the partition dimension.

The necessity for a resolving set of minimum four subgroups is underscored by the presence of structural variety observed in these counts.

The numerical values of vertices, edges, and ${n}$-order faces provided insight into the intricacy of the graph, thereby highlighting the necessity for a resolving set of minimum four subgroups to accurately differentiate between vertices. The application of labels to the vertices in a generalized approach ensures a logical and uniform means of identifying vertices. The utilization of this labeling methodology is vital in the formation of partition resolving sets.

Given evidence are substantiate to assert that the upper limit of the partition dimension for the convex polytope graph $\mathbb{A}_n$ is 4. The explanation for this upper bound is supported by several factors, including the distinctive arrangement of cycles, the inclusion of extra edges, the calculation of structural counts, and the implementation of a generalized labeling method. The comprehension of this concept holds significant importance in the development of efficient partition resolving sets and comprehensive analysis of the partitioning properties of the graph.

6.   Conclusions and discussion

In this paper, we gave the upper bounds of the partition dimension of convex polytope graph $\mathbb{A}_n$, $\mathbb{V}_n$, $\mathbb{U}_n$ and $\mathbb{T}_n$ and their partition dimension is bounded by four, as shown. We also put forward the given below hypothesis:

Conjecture 6.1. The following equities are true:

Difference between metric and partition dimension

Metric dimension: The metric dimension of a graph refers to the minimum number of vertices required in a subset such that each vertex in the graph can be uniquely identified based on its distances to the vertices in the subset. A collection of vertices is considered a resolving set if, for any given pair of vertices inside the graph, there exists at least one vertex in the resolving set such that the distances from that vertex to the pair of vertices are unique. The metric dimension refers to the smallest possible size of resolving sets. One of the practical applications of resolving sets is in network architecture, where they can be utilized to represent the positions of sensors or landmarks for the purpose of achieving effective navigation. For more detail on metric dimension, see ^[41,42].

Partition dimension: The partition dimension of a graph refers to the minimum number of sets required to partition the vertex set so that each partition generates an injective function on the vertex set. A partition refers to a grouping of non-empty sets that are pairwise disjoint, with the property that their union forms the vertex set of the graph. The vertex set is subjected to an injective function induced by each partition. Stated otherwise, it may be observed that there is no occurrence of several vertices being mapped to a single element within the partition. The partition dimension refers to the smallest possible cardinality of partitions. The practical applications encompass coding theory, wherein partitions can serve as representations of encoding schemes or blocks inside error-correcting codes. For more detail on the partition dimension one can see ^[43,44].

Key differences: Characteristics of the sets: Resolving sets, in the metric dimension context, are subsets of vertices that allow for the exclusive determination of distances between vertices. Within the framework of partition dimension, the sets, referred to as partitions, consist of vertices that induce injective functions on the vertex set. The objective of dimension: The goal of metric dimension is to find a set of vertices that uniquely determines the distances between all pairs of vertices. The purpose of the partition dimension is to determine partitions of the vertex set that yield injective functions. Interpretation from the perspective of graph theory: Metric dimension refers to the identification of sets that precisely determine the placements of vertices by considering the distances inside the graph. Partition dimension refers to the act of separating the vertices in a way that preserves unique interactions inside each partition. Real-world uses: Metric dimension is commonly employed in the domains of network design and location-based issues. The concept of partition dimension is employed in diverse domains, such as coding theory, where the importance of injective functions is highlighted. To gain a more comprehensive understanding of the distinction between the metric and partition dimension, go to the sources cited as ^[45,46].

Simply said, the metric dimension and partition dimension are two separate indicators of a graph's structural intricacy. The metric dimension largely deals with determining distances inside the graph, whereas the partition dimension focuses on the existence of partition-induced injective functions.

Use of AI tools declaration

The authors declare they have not used Artificial Intelligence (AI) tools in the creation of this article.

Acknowledgments

The authors extend their appreciation to the Deputyship for Research and Innovation, Ministry of Education in Saudi Arabia for funding this research work through the project number ISP-2024.

Conflict of interest

The authors declare that there are no conflicts of interest regarding the publication of this article.