Dynamical behavior of traveling waves in a generalized VP-mVP equation with non-homogeneous power law nonlinearity

1.   Introduction

Interpretation of piezocone penetration test (CPTU) results in silty soils is complex due to the partially drained conditions during penetration and the natural variability of many deposits. Silty soils often consist of a mixture of both finer and coarser materials with little cohesion. The nature of these soils complicates the sampling and handling in the laboratory as well as the interpretation of the laboratory and CPTU results. Furthermore, little guidance exists for practicing engineers on how to determine the appropriate soil parameters for a specific geotechnical design problem. Up to now only a few field research projects on undisturbed silty soil have been published in the literature analyzing the behaviour of these soils even though they are present in many parts of the world. In Norway, several research sites exist which have been studied in detail over the last years. Sandven ^[1] studied a test site, underlain by a thick silt deposit, close to the present research site near Trondheim in Norway. Various field and laboratory tests were carried out and furthermore a case record of building settlement was reported and analyzed. Another glaciomarine silt test site in the western part of Norway close to Bergen (Os) was described by Long et al. ^[2]. The Os site was thoroughly investigated by using CPTU, in situ vane tests, total soundings and soil sampling using different soil samplers. A more recent comprehensive study was reported by Blaker et al. ^[3] which comprised work at the Norwegian Geotechnical Test Site (NGTS) silt test site at Halden approximately 120 km south of Oslo. The low plasticity clayey silt deposit was characterized in detail over several years including geophysical and geotechnical in situ investigation methods as well as intensive soil sampling using different sizes and samplers. All of these investigations highlight the lack of a practicable framework for establishing sample quality and determining design parameters for silty soils. Up to now engineers apply clay or sand based methods which often reveal questionable results for silty materials ^[4,5].

The present study carried out by the Norwegian University of Science and Technology (NTNU) incorporates a detailed field and laboratory study of a coarse sandy silt at a site in Stjørdal some 35 km north-east of Trondheim. One of the main objectives of the work was to gain a better understanding and insight into the behaviour of silty soils and to give guidance to practicing engineers on how to determine geotechnical parameters for design purposes. In order to obtain more knowledge, series of CPTUs were performed to study the behaviour of the silt deposit in situ. Shear wave velocity measurements close to the test area were used to support the CPTU measurements. A series of samples using thin walled 54 mm steel sample tubes have been taken in the field and analysed in the laboratory. Oedometer and triaxial tests were used to study the compressional behaviour of the silt and to obtain effective stress soil parameters and the undrained shear strength of the material.

The present paper initially gives some details of the background geology and then focuses on the outcome of the laboratory investigation program. Sample quality has been examined using the well known clay based volume change criteria ^[4] and more recently developed models employing strain energy and compression ratios ^[6]. The oedometer tests have been interpreted using the methods proposed by Janbu ^[7] and Becker et al. ^[8]. Undrained shear strength values have been determined by applying different criteria as suggested by Brandon et al. ^[9]. A series of standard speed CPTUs are presented and applied to several existing soil behaviour charts ^[1,10,11].

The present study provides information to geotechnical engineers working with silts as well as for researchers so as to broaden the database for establishing new correlations for these materials.

2.   Halsen-Stjørdal site

The research site Halsen-Stjørdal is situated in the Stjørdal valley about 35 km north-east of Trondheim in Norway. The silt deposit is about 9 m thick (4-13 m). It is overlain by a very coarse and stiff 4 m sand layer. In order to carry out CPTU tests the top layer needed predrilling. A more clayey silt—sand layer is present underneath the silt deposit. No evidence of the depth to bedrock was found. The ground water table was at about 2.8 m depth.

2.1.   Geology background

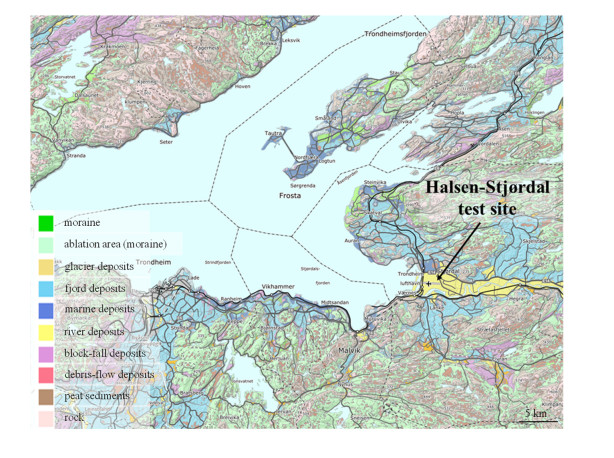
The geological history of the location has been studied in detail by Sveian ^[12]. The youngest glacial period, also named the Quaternary period, lead to an enormous icecap that covered the entire Scandinavia. Numerous phases of warmer and colder climate caused the icecap to retreat or advance respectively. This period of time had the most significant influence on the Norwegian geology especially with respect to the type and structure of the deposited materials. During the last de-glaciation, the Stjørdal valley was transformed into a deep and long fjord. Figure 1 shows a Quaternary map of the Stjørdal region indicating that the test area consists mainly of river deposits (the yellow parts on the map).

Fine-grained particles as silt and clay were transported by rivers or melt-water into the sea forming thick deposits of these sediments reaching 200-300 m of thickness over bedrock. Clayey materials govern these deposits but in some parts due to increased or irregular water velocities, silts and fine sands are more dominant. This is typical for the Halsen-Stjørdal test site where the fine sediments are dominated by silt, with layers and pockets of clay and coarse sand forming a very irregular picture. However, there is no evidence that the Halsen-Stjørdal silt deposit has been overridden by any massive ice glacier since indications of glacial advances have only been found further up the Stjørdal valley. Furthermore, researchers found out that the location of the Halsen-Stjørdal site is not directly influenced by previous river erosion. Hence one can expect no distinct pre-consolidation due to erosion of the soils in the area ^[14].

2.2.   Testing program

The field-testing program at the Halsen-Stjørdal site included 25 CPTUs with various penetration rates down to a depth of 18 m. Only the standard rate penetration tests are used for the present study. The test site covers an area of about 11 m × 8 m and the different CPTU tests have been distributed evenly in order to characterize the rather irregular soil deposit. A standard 35.7 mm friction cone was used and the pore pressures were measured at the u₂ position directly behind the cone head ^[15]. Furthermore, various samples were taken at 1 m intervals down to a depth of 13 m using thin walled 54 mm steel sample tubes in three different boreholes ^[5].

The boreholes surround the CPTU tests and are spread over the test area to detect spacial variations of the soil deposit. All samples used for this project have been handled carefully during the sampling process and when taken back to the laboratory. To ensure the highest possible sample quality, all samples taken in the field have been extruded in the laboratory within 24 hours of sampling time ^[16]. The laboratory investigation program included basic index testing as well as oedometer and triaxial testing. Figure 2 shows an overview map of the test site.

3.   Field test results

3.1.   Cone penetration tests

Several CPTUs have been carried out at the test field. Generally, the test was carried out with a standard rate of 20 mm/s ± 5 mm/s according to the ISO 22476-1 ^[18]. This approach assumes undrained penetration conditions for clays and fully drained conditions for sands. However, this is often not the case when dealing with silty soils. Partial drained conditions are most likely to occur during a penetration test in a silty material leading to over- or underestimation of the geotechnical soil parameters.

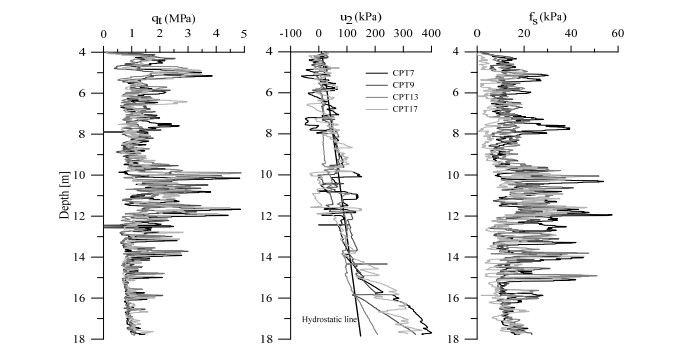
Figure 3 shows some of the results from the standard speed CPTUs from the site in terms of cone resistance (q_t) corrected for out of balance pore water pressure effects, pore pressure u₂ and sleeve friction (f_s). The top layer of the deposit consists of a very coarse and stiff sand which was difficult to penetrate without losing saturation of the pore pressure filters. Therefore, it was decided to predrill down to about 4 m. The hydrostatic pore pressure is drawn into the pore pressure plot with a ground water table at about 2.8 m depth. Measurements of the cone resistance are rather irregular detecting thin layers of various materials over short lengths. The average cone resistance is about 1.5 MPa with some peaks between 10 m and 12 m, which represents a typical value for a loose to medium stiff silt. The pore pressure measurements are somewhat more scattered reflecting the natural variability of the soil deposit. Changes in thin layers can easily be identified confirming the good quality of the tests. Especially above 12 m the values dilate rather frequently showing even negative pore pressures for some readings. The four CPTUs show very scattered sleeve friction results which increase with depth in the silt layer. Lunne et al. ^[15] stated that measurements of the sleeve friction are less reliable and have to be used with care. Nevertheless, the results confirm the coarse silty nature of the deposit. Below 11 m, the soil becomes more clayey which results in a reduced cone resistance to about 1 MPa and an increase in pore pressure to values which exceed hydrostatic levels. Even though measurements of the sleeve friction are scattered, one can notice a reduction in friction in the more clayey part of the deposit. Overall, the CPTU readings show a very layered and rather turbulent silt deposit which confirm the previous laboratory results from this study.

From the measured parameters during a CPTU test, several derived values can be used to deduce soil parameters and describe the soil behaviour using various published soil behaviour charts. The application of these charts is used as a guide to define soil behaviour rather than a definition of soil type or grain size distribution. It is recommended to combine all three measured parameters to achieve a more reliable soil classification. Senneset and Janbu ^[19] introduced the pore pressure parameter B_q ( = (u₂ - u₀)/(q_t - σ_v0)). Using B_q together with the cone resistance, Senneset et al. ^[1] established a soil behaviour chart without using sleeve friction measurements. One of the most widely used charts in the world has been suggested by Robertson et al. ^[20] and later revised ^[11]. These are based on a combination of either normalized cone resistance Q_t ( = (q_t - σ_v0)/σ’_v0) and B_q or friction ratio F_r ( = f_t/(q_t - σ_v0)). Recent developments in this field focus more in detail on detecting the behaviour of intermediate soils as for example the diagram by Schneider et al. ^[10] which is based on Q_t and Δu/σ’_v0 and accounts for partial consolidation stress effects. Figure 4 shows results of the application of the test data to the charts described above. Friction based diagrams have been neglected due to the rather less reliable results. The data has been divided into the sandy silt layer (4-8 m) and the clayey silt layer (8-14 m). Senneset et al. ^[1] defines the soil mainly as stiff clay to silt without clearly differing between the two layers although showing some tendency towards the loose silt. The Robertson’s approach ^[11] plots the data mostly in zone 4 and 5 for the upper layer (silt and sand mixtures) and in zone 3 for the lower layer (clay to silty clay). The Schneider et al. ^[10] diagram plots the data for both layers into zone 3 corresponding to a transitional soil behaviour. All three soil behaviour charts work well in identifying the soil as a silty material, nevertheless the Schneider et al. ^[10] diagram shows the most consistent results classifying the soil as intermediate soil behaviour throughout the whole layer.

3.2.   Shear wave velocity

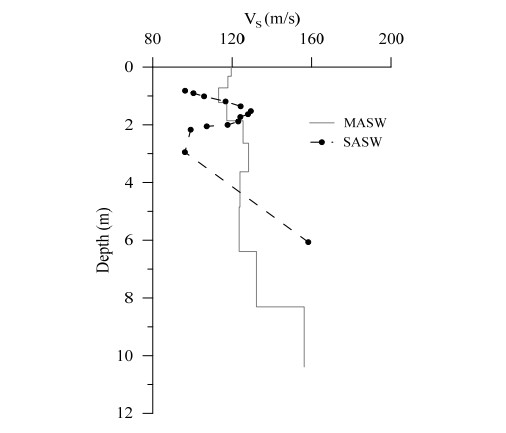
Profiles of shear wave velocity (V_s) which were generated at the Halsen-Stjørdal site used both the Spectral Analysis of Surface Waves (SASW) method and the Multichannel Analysis of Surface Waves Method (MASW) ^[21]. The SASW method uses a single pair of receivers that are placed collinear with an impulsive source (e.g. a sledgehammer). The test is repeated a number of times for different geometrical configurations. The MASW technique was introduced in the late 1990’s by the Kansas Geological Survey ^[22] in order to address some problems associated with SASW. The MASW method exploits multichannel recording and processing techniques that are similar to those used in conventional seismic reflection surveys. At Halsen 24, 10 Hz geophones spaced at 1 m intervals were used for MASW and a 5 kg sledgehammer was used as the impulsive source. Source receiver offsets were 0 m and 2 m. The MASW data was inverted using the software Surfseis, assuming a 10 layer soil model. A simple hand calculation approach was used to invert the SASW data assuming that the depth of penetration, z, of a particular wave is one third its wavelength (λ), i.e. z = λ/3 ^[23].

The SASW and MASW profiles obtained at Halsen are shown on Figure 5. The irregularity of the SASW profile is due to the simple method of inversion used. Nevertheless, the SASW values are of similar magnitude as the MASW data and serve to give confidence in the MASW results. The values of V_s from the MASW profile in the silt layer are on average about 125 m/s which is a quite low value and corresponds to a rather loose material (class E) according to NIBS ^[24]. Furthermore, V_s values are similar to the well-known loose Holmen sand deposit from Drammen, where average values of about 140 m/s were reported ^[25].

4.   Laboratory results

4.1.   Sample quality

The evaluation of sample quality is of high importance for practicing engineers and in research since the results of the laboratory test will be used directly for design and to establish new approaches and correlations. Using low quality soil data will eventually lead to an over- or underestimation of the geotechnical problem which may have crucial outcome. The two concepts that are mostly used worldwide are either based on evaluating the strain upon reconsolidation to in situ stress, named volumetric strain ε_vol ^[5] or on the change of the void ratio (e) normalized by the in situ void ratio Δe/e₀ ^[4]. The latter method has been widely used in Norway and is recommended for use in the assessment of sample quality ^[26]. The background for all these methods is that high quality samples experience less strain induced by sampling than low quality samples. Therefore, comparing the recompression strain that evolves during reconsolidation of the sample to the in situ stress state has become a useful tool. It should be noted that the criteria for the evaluation of sample disturbances originates from studies on marine clays (sampling depth 0-25 m) with an overconsolidation ratio (OCR) varying between 1-4 and plasticity index (IP) between 6% and 43%. Table 1 summarizes the criteria used to assess sample quality for clay materials depending on the OCR.

Care has to be taken when applying these methods to soils whose parameters fall outside the above mentioned range ^[28]. In particular, silts may suffer from densification during shearing and sampling, showing an unrealistic low void ratio or change of volumetric strain upon recompression to in situ stresses. Therefore, the samples appear to be of high quality even though they are highly disturbed ^[3,29]. Applying these methods to silty materials is challenging and will often result in misleading conclusions and may not reflect the true quality of the specimen ^[30]. Other methods exist to identify sample quality which make use of measuring shear wave velocity or suction but are not part of the present study ^[31,32]. No well-founded framework currently exists to assess the sample quality in silts. Recent studies have shown that strain energy and compression ratios can be useful tools to assess sample quality in intermediate, low plastic soils ^[6]. This method is based on the concept developed by Becker et al. ^[8] and defines the work as the energy necessary to compress the soil to a given stress state. Equation 1 defines the work per unit volume for a given load increment, where σ_i’ and σ_i+1’ are effective stresses and ε_i and ε_i+1 strains at the beginning (i) and at the end (i+1) of the current load increment:

The cumulative work increments (ΔW_oed) for loading to a certain stress level (σ_v') can be plotted against σ_v' and be used to interpret the preconsolidation stress (p_c') from an oedometer test. The in situ vertical effective stresses ranged from 70 to 140 kPa for the present study. Based on this concept, strain energy compression indices were established for both recompression (C_rw) and virgin compression (C_cw). C_rw represents the initial recompression strain energy index, which is defined from seating stress to in situ condition. Since the oedometer tests in the present study have no unloading reloading loop, C_cw has been evaluated from the stress interval between 2.5-5 p_c'^[6].

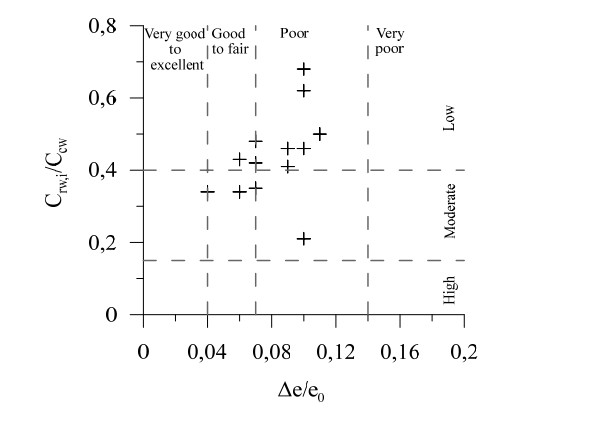
The ratio of strain energy compression indices (C_rw/C_cw) is a useful indication of sample disturbance for a wide range of soil types and in situ conditions since it normalizes the influence of plasticity and is independent of the in situ stress and OCR. The database used to establish the quality criteria consists of soil mixtures with IP varying between 0 to 31% and maximum effective in situ stress of 1000 kPa. Table 2 shows the recommended ranges to assess the sample quality in terms of void ratio or strain energy ^[6]. Since C_rw/C_cw is the same as C_r/C_c for a linear stress-strain behaviour, the ranges in the table have identical numbers.

In the present study, the data has been applied to the clay based volume change criteria as well as to the strain energy and compression ratio criteria using results from the oedometer tests. Figure 6 shows the sample quality assessment for the clay based volume change criteria. The volumetric strain defines all samples as poor quality whereas the void ratio defines half of the samples as good to fair and the other half as poor. None of them are characterized as very poor. It seems that the sample quality is decreasing with increasing depth which supports findings from other researchers who report that the approach using void ratio is effective stress dependent. This means that deeper samples are expected to have lager Δe independent of sample quality ^[33].

The results for strain energy and compression ratios can be seen on the right hand side of Figure 6 and on Figure 7. None of the samples have been classified as high quality whereas four samples are of moderate and the remaining ones are of low quality. Since the method is independent of the present effective stress level it also reveals reasonable results for deeper samples (see Figure 6). The sample quality could be increased by extruding the samples directly in the field. Thereby any influence on the sample quality due to transport, temperature changes, vibrations or shock loads can be eliminated ^[34]. Figure 7 shows the combination of the void ratio and the compression ratio approach. Even though most of the samples are defined as poor quality for the present material, none of them is defined as very poor. Given the fact that high quality samples in silt are difficult to obtain, the achieved sample quality is acceptable for this type of material, hence using the results from the oedometer and triaxial tests is reasonable.

4.2.   Index test results

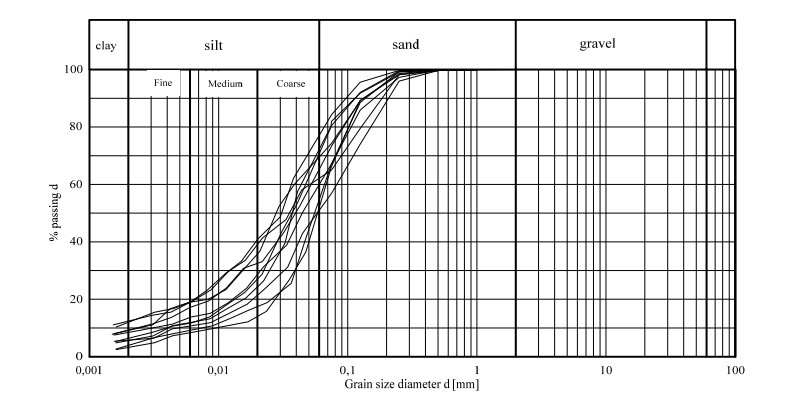
Grain size distributions have been determined and Figure 8 shows a summary plot of some of the tests. In Norway, the recommendations made by the Norwegian Geotechnical Society (NGF) are used in practice to classify soil types ^[35]. A soil is hereby defined as a SILT if more than 45% of the grains are between 0.002 mm and 0.06 mm and if less than 15% of the particles are clay (<0.002 mm).

Figure 8 shows that the soil deposit consists of either sandy or clayey SILT with an average silt content of 55% and the majority of the particles falling into the coarse silt spectrum (0.02-0.06 mm).The average coefficient of uniformity (C_u) is 17 which reflects a well graded silt deposit. While the clay content varies with depth, reflecting the irregularity of the soil deposit, it generally increases with depth. Measurements of the soil grain density yielded an average value of 2.66 g/cm³. Sandven ^[14] carried out a microscope study nearby the present research field which showed mainly angular grain shapes with some slightly rounded grains, which is typical for short-transported glaciofluvial sediments.

Figure 9 gives an overview of the index test results that were obtained from the laboratory tests. Due to the very low plasticity and very coarse structure of the sediment, it was not possible to achieve results for the Atterberg limits. Sensitivity (S_t) values from the falling cone test increase with depth being about 10 at the top of the silt layer and 28 at 14 m, which is characteristic for a medium sensitive silt. In Norway a material is defined as medium sensitive if the sensitivity is between 8 and 30 ^[35]. The measured natural water content of the deposit varies between 20% and 35% with an average content of 25%. Measurements of the bulk density are more scattered with an average of about 2.1 g/cm³. The in situ void ratio, which was calculated using the water content and grain density measurements from the index test results, decreases with depth from about 0.7 to a minimum of 0.5.

Figure 10 shows a cross section of a soil specimen at 7.5 m depth from the test site showing small lenses of sand and clay as well as cracks and holes. Due to the non-uniform soil deposit, it was moredifficult to obtain representative soil parameters for the site. The findings from the laboratory investigations reflect the natural variation of the silty material of this deposit and emphasize the challenges in handling the soil in the laboratory and in the field. Overall, the results from the basic laboratory tests are in accordance to findings from previous work on a research site close to Halsen-Stjørdal ^[14]. There exist up to now two different research silt sites in Norway ^[2,3] in addition to the present test site, which report a different material behavior and composition, making this test site a useful extension to the existing database.

4.3.   Oedometer tests

Interpretation of 1D compression curves for silty soils is difficult and, currently no international accredited framework exists to deduce deformation parameters. A series of oedometer tests were conducted on 2 cm thick specimens to study the vertical one dimensional compression behaviour of the silty material using the standard procedure proposed by Sandbæ kken et al. ^[37]. Due to the more confined procedure using a steel ring to support the soil and smaller size of specimen, handling the material during preparation of the sample prior to testing was not as challenging as for the triaxial tests. Constant rate of strain (CRS) tests with axial strain rates varying between 2%/h and 5%/h were carried out on samples taken from depths between 4-13 m. The pore pressure was measured at the bottom of the specimen allowing for a single drainage. The measured pore pressure decreased for all tests continuously and stabilized towards the end of the test procedure. Figure 11 shows the results in terms of σ_v' versus void ratio (e), constrained modulus (M) and strain (ε) respectively. The log σ_v' versus e plots are of rounded nature and no distinct preconsolidation stress can be identified. This might be partly due to the silty nature of the material itself and partly due to the influence of sample disturbance ^[2,38]. Overall, it can be assumed that especially the upper silty layer has experienced some densification as shown by the relative flat σ_v' versus e plots indicating little change in void ratio during further compression. Furthermore, the results do not lead to a unique Normal Compression Line (NCL), which is typical for many silts, but instead continue parallel to one another with increasing stress level. Several researchers studying the behaviour of silt, have reported this phenomenon and have suggested that complex factors govern the non-convergent behaviour of silty soils ^[31,39,40].

All test results show a steady increase of the constrained modulus with increasing σ_v' and no constant behaviour in the lower stress ranges or reduction around the preconsolidation stress as would be seen for clay soils. The behaviour observed is characteristic for silty soils ^[41]. In order to deduce modulus numbers (m) and M values for the silt, the tangent modulus concept has been applied to the data which defines M as the ratio of Δσ_v' over Δε. M varies for different types of soils and hence can be expressed with the following general equation by means of m, the reference stress (σ_a) and an exponent number (a) which varies with soil type ^[7]:

Using an exponent number a of 0.25, the measured data can be reproduced fairly well. Figure 11 B and E show the results for two different depths applying the method described above by Janbu ^[7]. The results obtained coincide well with findings from other silt sites in Norway using the proposed method and confirm the gradual increase of M with increasing σ_v' of silty soils under compression ^[2,3,14].

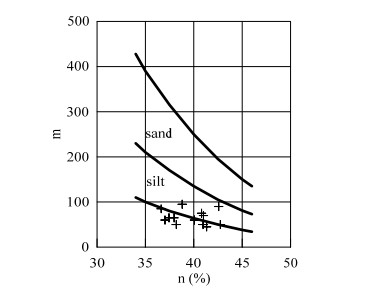
Figure 12 shows how the modulus numbers deduced by the above mentioned method fit into the ranges suggested by ^[7]. This defines the lower and upper bounds for silt and sand respectively and suggests a rapid drop of m for increasing porosity (n). Modulus numbers for the present silt vary in the range of 45 to 110 with relatively low porosities between 36% and 43% which is characteristic for Norwegian silts and represent the lower bound of the silt range suggested by ^[7].

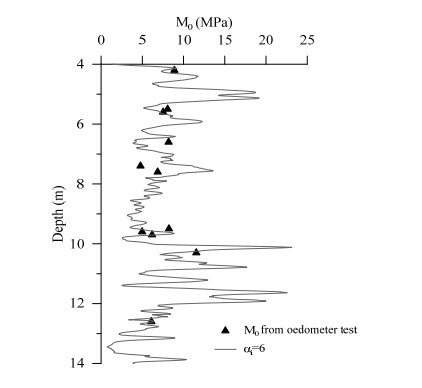
Furthermore the deformation Modulus (M), which corresponds to the constrained deformation modulus from an oedometer test, can be predicted by CPTU test data. Senneset et al. ^[42] stated that a linear correlation between the cone resistance and M₀ (Modulus at σ_v0’) gave the best fit for the data by using M₀ = 2q_t (for q_t < 2.5 MPa). Sandven ^[43] proposed later the following relationship for loose to medium dense silts using a dimensionless modulus number α_i = 6 ± 2:

Figure 13 shows the M₀ values from the oedometer tests together with the predictions given by the CPTU results. The results from the oedometer test vary between 5 MPa and 11 MPa. Using α_i = 6 reveal constrained modulus numbers from the CPTU measurements which are very similar to the oedometer test results.

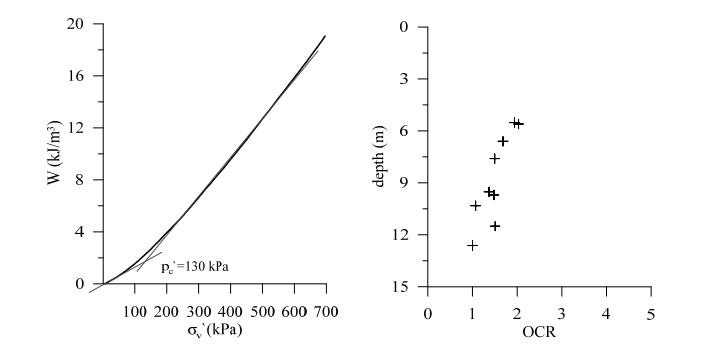
Due to the particular compression behaviour of the silty material, existing methods to deduce p_c' often do not work. Geometric approaches based on axial strain plots are not useful due to the characteristic shapes of the curves ^[44]. The constrained modulus curves cannot be used due to the lack of a significant change of behaviour in the preconsolidation stress region as for clayey materials ^[7]. Several studies have been carried out to review existing methods used for clay and to investigate their application to silty soils ^[38,45]. In the absence of a reliable method for determining the preconsolidation stress in silts, the technique outlined by Becker et al. ^[8] based on the work criteria has been used in the present study. It is important to note that this method was developed for clay soils which exhibited classical “clay” like behaviour when plotted in log σ’_v versus e format, often showing a change of behavior around the preconsolidation stress level. As can be seen from Figures 11A, B this type of behaviour may not necessarily be observed in the case of silts. Although the technique shows promising results, further work on its use in silts is warranted. Figure 14 shows results for an oedometer test from 7.4 m depth, yielding a preconsolidation stress of about 130 kPa. Furthermore, the values for OCR over depth can be seen for the whole range of samples between 4 m and 13 m. Overall, it can be noted that the silt is normally to lightly overconsolidated with values between 1-2 and decreasing with depth. These findings support the geological history of the location and reflect previous results carried out in the area ^[14] Furthermore it supports the successful application of the technique by Becker et al. ^[8] for the material in the present study.

4.4.   Triaxial tests

Silty materials can show different behaviour patterns during undrained triaxial compression. Usually, positive pore pressures are generated at relatively low strains for both dense and loose silts. For loose silts, the peak pore pressure occurs at low strain levels and remains constant. In contrast a dense silt develops negative pore pressures with increasing strains. Examining the stress path, both dense and loose silts reach failure at relatively low strain levels. As shearing proceeds, the stress paths move parallel to the failure line. Due to the dilative nature of these materials, the undrained strength of silts increases during shear without a clear maximum value. This means there is no unique shear strength value. This might be one of the significant differences between clay and silt when it comes to triaxial compression ^[46].

Anisotropically consolidated undrained compression tests (CAUC) have been executed on all of the samples, applying the standard test procedure as described by Berre ^[47]. Challenges experienced during handling the low plastic material in the laboratory included the process of preparing the sample prior to be building it into the triaxial apparatus. It was difficult to keep the sample in place and lateral displacements occurred at times due to self weight of the specimen. For future tests it is recommended to try taking 75 mm samples instead of 54 mm and use an approach where the sample is extruded directly into the triaxial membrane ^[48]. By using 75 mm samples, a larger cross sectional area can be tested which is especially welcome for non-homogenous soil deposits. The larger the sample the more representative is the test result. Furthermore, it was difficult to keep the sample vertical during trimming due to the rather coarse structure. Due to the above discussed challenges, it cannot be precluded that densification of the samples might have occured during preparation. This issue will be addressed later when discussing shear strength of the material. All samples have been consolidated to in situ effective stress levels using an assumed coefficient of earth pressure at rest (K₀) of 0.5 for the site which is in accordance with work carried out by other researchers ^[14]. B-values were checked prior to consolidation and reached the appropriate value of > 0.95. For most of the tests, a backpressure of 200 kPa is used and the specimen are sheared at a strain rate of 4%/hour. During consolidation back to in situ stress level, volumetric strains were recorded varying between 1.1% and 4.5%. Figure 15 shows the results of the tests in terms of shear stress (τ) or pore pressure (Δu) vs. axial strain (ε) or effective radial stress (σ₃'). Part A-C represents results from the upper silt layer (4-8 m) whereas part D-F of Figure 15 shows the results from 9 m to 13 m.

The results for the samples taken from 4 m to 10 m show a strong dilative behaviour with increasing τ and increasing ε without reaching a defined maximum shear. The stress paths reveal some contraction upon shearing followed by dilation with increased effective stress level continuing along a clear failure line. Up to a strain level of about 1%, the pore pressure increases for all samples. Subsequently it decreases and dilates into negative pore pressures at higher strains. The triaxial tests taken at deeper depths from 11 m and 12 m behave differently and show a contractive behaviour with a more distinct maximum shear strength and positive pore pressures at lager strains due to less coarser material and higher clay content at deeper depths.

Effective stress strength parameters in terms of friction angle (φ') and effective cohesion (c’) have been determined from the triaxial tests and are especially required for long-term stability analysis. Due to the varied nature of the silt at Halsen-Stjørdal, a range of friction angles have been found, varying between 34.2° and 38.7° with an average of 36.9° and cohesion value of 7 kPa for the silt layer. These results are consistent with findings from other silt sites in Norway. Blaker et al. ^[8] found a friction angle of 36° for the Halden silt in the Oslo region whereas Long et al. ^[2] reported a friction angle of 35° for the Os silt south of Bergen.

Despite the fact that many researchers doubt the use of undrained shear strength (s_u) for partially drained materials, this parameter is still frequently applied by many engineers ^[2]. Figure 16 shows shear strength plots for the test site. It is common practice in Norway to apply the SHANSEP method to establish a site specific s_u-profile by using s_u/σ_v' = S(OCR)^m. As recommended by Ladd et al. ^[49] a m-value of 0.8 and S_silt = 0.2-0.3 were used. By including upper and lower bound values for OCR as indicated by the oedometer tests this resulted in s_u/σ_v' limits of 0.3-0.52 for this present silt. The results from index testing using the Swedish fall cone are varied as expected with values between 20 kPa and 80 kPa for the deeper samples, but most of the results plot close to the s_u/σ_v' limits. The results from the CAUC tests have been analysed using different strength criteria presented by Brandon et al. ^[9] for the purposes of deriving s_u:

(1) at peak deviatoric stress

(2) at ε = 1%

(3) at reaching the Mohr-Coulomb line

(4) at Δu = 0

(5) at maximum pore pressure (u_max)

The results for the different strength criteria can be found in Figure 16. There is currently little guidance in literature which criteria to use for silty materials. Also, the dilative tendency of the low plastic silt makes it difficult to define general failure criteria. For the upper silt layer (4-10 m) the criteria (1) and (3) did not work since the shear strength values are unrealistically high due to the dilatoric behaviour of the material. The pore pressure criterion equal zero (4) could not be applied either since it occurs at high strains, which leads to unrealisticly high values of shear strength. Criterion 4 does however show less scattered results than criteria (1) and (3). Criteria (2) and (5) revealed the most consistent and realistic values for this site, since the limit strain has been chosen at 1% where no dilation occurs. This coincides for almost all tests with the strain at maximum pore pressure. Shear strength values for these two criteria follow the 0.3-0.52 s_u/σ_v' limits and are less scattered. Several researchers found the u_max criterion as the most promising one since it is on the conservative side being well below the fully mobilized failure and ensuring no dilative pore pressures ^[2,9]. It should be noted that the shear strength criteria work in a more consistent way for the lower layer, which is more clayey. Other than criterion (4), which cannot be applied due to contractive pore pressure development, all shear strength values plot well between the su/σ_v' limits. This demonstrates the application for these models in more contractive soils.

5.   Summary and conclusions

Dealing with low plastic silts and silty material is difficult and challenging both in the field and in the laboratory. It is often assumed that silts behave either as clay or as sand but in reality neither of these reflect the real behaviour of these soils. The ongoing research project at NTNU incorporates an extensive field and laboratory study at a very low plasticity silt test site Halsen-Stjørdal in Norway. The present paper discusses results from various laboratory tests and results from CPTU tests. Sample quality predictions in silt have been discussed.No well-founded framework currently exist to assess the sample quality in these soils. A recent developed method using strain energy and compression ratios seems promising and shows quality results in consistence with the void ratio criteria, revealing a reasonable sample quality without being dependent on plasticity, effective stress level or OCR ^[6].

The CRS curves show mostly rounded shape curves with no distinct preconsolidation stress which is mostly due to the silty nature of the material itself and partly due to the influence of sample disturbance ^[2]. The Janbu 1D model ^[7] for compression has been successfully applied and revealed realistic modulus values for the site. Using the linear approach proposed by Senneset et al. ^[42] gave constrained modulus numbers from the CPTU which are very similar to the oedometer rest results and confirm the usefulness of this tool for silty soils. The technique developed by Becker et al. ^[8] to deduce preconsolidation stress revealed reliable results and showed a lightly overconsolidated material for the silt deposit which is in agreement with the geological history of the area and earlier reported values by Sandven ^[14]. For future work, it is worth looking deeper into the method proposed by Becker et al. ^[8] and apply strain calculations as suggested by Janbu ^[7].

Triaxial test results revealed a strong dilative behaviour and significant negative pore pressures were developed with increasing axial strain. The lower more clayey samples showed a more contractive behaviour in triaxial compression. Due to the dilative nature of the materials, the undrained shear strength of silts increases during shear without reaching a clear maximum value, e.g. no unique shear strength value exist. This might be one of the most significant differences between clay and silt when it comes to triaxial compression and identifies the dilemma many practicing engineers face when dealing with silty soils. The present study shows that picking s_u at a defined maximum strain (here 1%) or at maximum pore pressure ensuring no dilation gives values close to the 0.3 to 0.52 σ_v' lines as proposed by Ladd ^[49]. Given the fact that possible densification took place during sampling, one has to be careful when choosing parameters for geotechnical design purposes and especially the CAUC tests need to be treated with caution. Therefore, choosing strength values on the conservative side is appropriate.

For future tests it is recommended to use bigger samples, e.g. 75 mm instead of 54 mm in order to be able to overcome problems related to building and preparing the samples for testing. Furthermore, a larger cross sectional area can be tested which is especially welcome for non-homogenous soil deposits. Using an approach where the sample is extruded directly into the triaxial membrane is highly recommended ^[48]. Due to the complex soil structure of the silt deposit, taking block samples would be impossible. The soil would fall apart and it would be difficult to get the block out of the borehole. Furthermore, trimming the sample in the laboratory would be difficult due to the coarse and partly loose structure.

The piezocone penetration test is a reliable tool in silt where shear induced pore pressures due to dilation have significant effects. The Schneider et al. ^[10] chart suggests the soils investigated are intermediate soils which is consistent with the general behaviour of the deposit. Shear wave velocity measurements have been used to confirm the loose structure of the soil deposit. Due to the issues mentioned above concerning densification and disturbance during sampling, more emphasis should be placed on in situ testing methods in future investigations of deposits such as these.

The present study carried out at NTNU contributes to broaden the database of the engineering behaviour of silts for Norway and internationally and increases the understanding of these rather difficult materials. More research is needed on natural silty soils to be able to establish a reliable framework and to form recommendations for practicing engineers.

Conflict of interest

All authors declare no conflict of interest.