1.
Introduction
The gun-launched UAV is a combination of conventional artillery, guided missiles and UAV technology. It has the advantages of flexibility, rapid deployment, diversified tasks and reusable use, it is one of the key equipments for the modernization transformation of the traditional army combat equipment. At present, each military power has carried out the research and development of gun-launched UAV, and started mass equipment, such as American switchblade cruise missile, Russian R-90 UAV, British "Fireshadow" gun-launched UAV and so on.
At present, gun-launched UAVs mostly perform reconnaissance, damage effect evaluation, precision strike and other tasks. However, with the wide use of UAVs, a large number of low-cost UAVs have been widely used in various missions. For cheap "Low-slow-small" UAVs, conventional interception methods face problems such as high cost, untimely emergency response and low interception efficiency, how to counter these "Low-slow-small" targets have become an urgent problem to be solved in the battlefield environment.
The UAV system's plan to counter the "Low-slow-small" target is being tried by various countries and has entered the actual deployment stage: June 7th, 2021, the gun-launched coaxial twin-rotor UAV developed by the Mobile Force Protection (MFP) project of DARPA shot down the UAV. March 26th, 2020, The American XQ-58A "Valkyria" unmanned aerial vehicle successfully launched an ALTIUS-600 small unmanned aerial vehicle from its built-in weapon cabin to destroy air targets. The actual application of UAV system to counter "Low-slow-small" target Russia is in the forefront. Since 2019, The "Cube" and the "Lancet" suicide attack drones have been deployed in actual combat in the Syrian battlefield. Gun-launched drones are cheap and can fly for long periods of time, offering a new way of thinking about counteracting "Low-slow-small" targets.
In the practical engineering interception application, it is faced with the problems of detection error and system delay. The use of high-precision detection and guidance equipment will certainly solve this problem, but it will increase the use cost of the system. In order to solve these problems, cooperative detection target trajectory prediction has become an effective means to improve the detection and guidance accuracy.
For interception operations, the common guidance method is Proportional Navigation (PN) method [1,2]. The PN methods can be divided into two kinds of processing methods which take the velocity of interceptor as the reference [3,4] and the line-of-sight as the reference [5]. Among them, the velocity reference PN methods acceleration is perpendicular to the direction of interceptor velocity, which is mainly applicable to target interceptor guidance in atmosphere [6,7,8]. The line-of-sight reference PN method has an acceleration perpendicular to a direction associated with the line-of-sight [9,10], and is commonly used in extrasolar interceptions and rendezvous guidance interceptions [11,12,13,14].
True proportion guidance (TPN) is a line-of-sight guidance method, and real proportion guidance law (RTPN) is an engineering form of TPN. The real-time missile-target approach velocity is used as a reference to guide the missile-target collision, which is easier to deal with and analyze mathematically. Therefore, many researchers have conducted in-depth research and analysis of this method [15,16,17]. Based on the Lyapunov-like method, the literature [9] analyzed the capture area of RTPN intercepting any maneuvering target under the assumption that the acceleration direction of the maneuvering target is perpendicular to the line of sight of the projectile and the acceleration magnitude is arbitrary and bounded. Reference [15] prove that RTPN has the same interception and capture capability in 3D space as in 2D space. Reference [17] derived the upper bound of 3D RTPN command acceleration by using a new Lyapunov-like method. Reference [18] analyzes the characteristics of RTPN capture area, movable distance, movable time and so on. Reference [19] derived the complete solutions of 3D RTPN for nonmaneuvering and maneuvering targets. Reference [20] proposed the variable structure RTPN guidance law. However, there are many limitations in engineering applications because the RTPN acquisition region does not consider the upper bound of the command acceleration and the overload saturation limit of the kinetic kill vehicle.
The interception of "Low-slow-small" targets by gun-launched UAV is a rendezvous guidance interception problem in the atmosphere. Aiming at the actual situation of gun-launched UAV intercepting "Low-slow-small" target and combining with the specific maneuverability of gun-launched UAV, this paper makes further research on the method of [9]. The main contribution points include:
1) Based on the analysis results of command acceleration upper limit of RTPN intercepting arbitrary maneuvering target, the saturation overload limit of interceptor is considered in this paper.
2) According to the effective interception condition that the relative distance between the gun- launched UAV and the intercepting target is less than the allowable miss distance and the absolute value of the missile target approach speed is greater than the allowable collision speed, this paper analyzes the capture area that RTPN can effectively intercept any maneuvering target when the interceptor is limited by saturation overload.
3) Aiming at the measurement error and the dynamic response delay of engineering system gun- launched UAV, a target trajectory prediction algorithm for cooperative detection is proposed in this paper, which solves the problems of low-cost and high-precision measurement and system delay.
2.
Design of guidance law for cooperative intercepting maneuvering target of two gun-launched UAV
2.1. RTPN guidance law
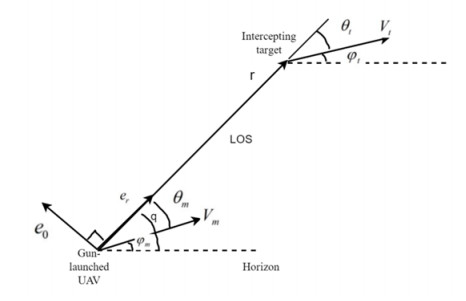
Before introducing the guidance law, the relative motion formulas between the gun-launched UAV and the intercepting target are established. The interception process is smaller in space and shorter in time, so the influence of earth curvature and rotation is not considered in the interception process. At the same time, both the gun-launched UAV and the intercepted target are considered as particles, and a coordinate system (er, eθ) is established in the direction and perpendicular to the line of sight (LOS) as shown in Figure 1:
Here, r is the relative distance between the gun-launched UAV and the intercepting target, vr=˙r is the approaching velocity of the gun-launched UAV and the intercepting target, ar=¨r is the relative acceleration of the missile and the target along the line of sight, q is the line-of-sight angle, and ˙q is the line-of-sight rate. For line-of-sight angular acceleration, at is for gun-launched UAV acceleration, am is for intercepting target acceleration, vθ=r˙q is for gun-launched UAV and intercepting target vertical relative velocity.
In this coordinate system, the relative motion formula between the gun-launched UAV and the intercepted target can be expressed as:
According to the actual situation, the relative velocity of gun-launched UAV and intercepting target is larger, and the intercepting target's maneuver along line of sight plays less role in penetration. Therefore, in the design of guidance law, only the maneuvering acceleration perpendicular to the line-of-sight is considered. If the following conditions are met for intercepting the target's mobility:
In the formula, α is a constant of the upper limit of the target maneuvering acceleration. In the actual interception task, this value can be determined according to the maximum value that the gun-launched UAV is allowed to intercept the target object.
The RTPN guidance law is that the acceleration perpendicular to the line of sight (LOS) and proportional to the LOS rate, namely
2.2. Upper guidance instruction acceleration limit
Before analyzing the upper limit of guidance instruction acceleration, capture region of the criterion of effective interception is introduced. The capture region is a state space related to the relative motion state of the target and the gun-launched UAV. When the relative motion state of the gun-launched UAV and the intercepting target enters the space, the relative motion of the UAV must finally satisfy the effective constraint condition of intercepting as Eq (2.5), this means that the target will be intercepted and captured at some final moment. The effective constraints for interception are:
In formula: rmiss to allow miss distance, vrImp to allow collision velocity.
For the capture region, Reference [9] gives the following conclusions: guidance systems as shown in Eq (2.1), for kinetic energy interceptors using RTP as shown in Eqs (2.3) and (2.4), for maneuvering targets as shown in Eq (2.2), If the approaching speed of gun-launched UAV and intercepting target meets the following conditions during interception:
in the formula, t0∈[0,+∞] is a constant, the proportional guidance coefficient satisfies the
then the following is true:
and the RTPN guidance instruction acceleration satisfies:
Further from this conclusion, when N > 2, for any maneuvering target, no matter the target can be intercepted or not, the instruction acceleration of RTPN is less than N/(N−2) times the maximum maneuvering overload of the target. Therefore, the maximum maneuver overload of the interceptor can be reasonably selected according to the conclusion and target maximum maneuver capability level (the value of α) predicted in advance.
2.3. Analysis of capture region of guidance law
It is a conclusion under the condition that the intercepting target has limited maneuverability, that is, the restraint condition described in Eq (2.2). However, the definition of target acquisition in Eq (2.5) is too stringent in terms of the relative distance between the gun-launched UAV and the intercepted target, and the requirements for the approaching speed of the gun-launched UAV and the intercepted target are too broad, lack of concrete guiding significance in engineering application.
In order to solve this problem, this paper analyzes the capture region of arbitrary maneuvering target which satisfies maneuver acceleration Eq (2.2) by RTPN in the definition of target acquisition of Eq (2.3). At the same time, the acquisition region of arbitrary maneuvering target by RTPN is analyzed when the saturation overload limit of interceptor is considered.
Firstly, the acquisition region of arbitrary maneuvering target by RTPN without considering the saturation and overload of the interceptor is analyzed. Under these conditions, we have the following theorem.
Theorem 1. A guidance system as shown in Eq (2.1) for a kinetic energy interceptor using RTPN as shown in Eqs (2.3) and (2.4) for a maneuvering target as shown in Eq (2.2) if the initial relative state of the missile and target is satisfied:
and the proportional guidance coefficient of RTPN satisfies:
the following conditions are valid for interception:
then there must be a terminal moment tf∈[0,+∞) to make Eq (3) true and realize effective interception of the target.
Proof. Assuming that Eq (2.12) is not true, since r and ˙r is continuous, there must be a constant to make the following formula true:
Since the ˙r is continuous, according to Eqs (2.10) and (2.13), can get Eq (2.14):
From Eqs (2.11) and (2.14), according to Theorem 1, we can get:
The Eq (2.15) is replaced by the Eq (2.1), and when atr=0, amr=0, there are:
Since ¨r=d˙r/dt=˙rd˙r/dr, Eq (2.16) can be expressed as:
Integral of the above formula can be obtained:
Since, r, ˙r, f(r) and are continuous, the Eqs (2.14) and (2.18) have constants t2∈[0,t1) that make them true, namely
Since r(t2) meets the effective interception conditions, there is r2(t2)>r2Miss. Further available:
The next will to prove it in two steps.:
1) if the following formula holds:
at this point, δ=αN−2, the Eq (2.20) can be:
The Eqs (2.22) and (2.11) show that there is an obvious contradiction between them. Therefore, the hypothesis is not valid, then Eq (2.12) is valid, and from Eqs (2.21) and (2.22) can be derived Eq (2.10).
2) If Eq (2.21) does not hold, that is
at this point, δ=|˙r(0)˙q(0)|, the Eq (2.20) can be expressed as:
The Eqs (2.24) and (2.10) show that there is an obvious contradiction between them. Therefore, the hypothesis is not valid, then Eq (2.12) is valid, and from Eqs (2.23) and (2.10) can be derived Eq (2.11).
Theorem 1 gives the capture area of RTPN for maneuvering target without considering the saturated maneuvering overload of interceptor, and selects the corresponding proportional guidance coefficient N. When considering the maneuver overload limit of kinetic energy interceptor, the acquisition region of arbitrary maneuvering target by RTPN should be calculated according to the Theorem 2.
Theorem 2. For guidance systems shown in Eq (2.1), for kinetic energy interceptors using RTPN as shown in Eq (2.5), for maneuvering targets shown in Eq (2.2), if the gun-launched UAV and intercepts the target initial relative motion condition to satisfy the following conditions:
and the proportional guidance coefficient N of the RTPN is determined by Eq (2.27),
then the Eq (2.12) holds, and the following formula is satisfied in the interception process:
Proof.
Lemma 1. Guidance system as shown in Eq (2.1) for kinetic energy interceptors using RTPN as shown in Eqs (2.3) and (2.4), for maneuvering targets as shown in Eq (2.2), if Eq (2.6) is established and the initial relative state of gun-launched UAV and intercepting target meets:
and the proportional guidance coefficient satisfies:
then the following formula holds:
According to Lemma 1, if Eq (2.29) holds, then Eq (2.32) holds, and the proportional guidance coefficient can be determined by Eq (2.30).
From the Eqs (2.30) and (2.31), the Eqs (2.10) and (2.11) holds, then Theorem 2 holds. That is, Eq (2.12) holds. If t0=+∞, since Eqs (2.30), (2.31) and (2.12) holds, shows that Lemma 1 holds. That is t0=+∞, Eq (2.31) holds, so Eq (2.28) is proved.
According to Theorem 2, when proportional guidance coefficient N is selected according to Eq (2.30) in two-dimensional plane, the region of acquisition of maneuvering target by RTPN is determined by Eqs (2.27) and (2.29). If Eq (2.30) is used to select the proportional guidance coefficient N of RTPN when the relative motion state of gun-launched UAV and intercepting target enters the acquisition region at the initial time or at any time during the interception, the interceptor can intercept and capture any maneuvering target with acceleration satisfying Eq (2.2).
3.
Trajectory prediction
The above conclusion is an effective capture and intercept situation under the condition of ignoring the measurement error of gun-launched UAV and its ground guidance system detection system as well as the dynamic response delay of gun-launched UAV itself. In fact, the error of guidance measurement and the delay of dynamics exist objectively.
At the same time, the gun-launched UAV system is a low-cost, mobile and flexible tactical weapon without the support of large, heavy and high-precision ground guidance radar, the entire operational use unit has only a few lift-off launchers and a simple 3-D radar. In order to solve the problem of guidance measurement error and dynamic delay, the scheme of multi-computer cooperative measurement and intercepting target trajectory prediction is adopted in this paper.
The altitude information of intercepting target can be provided directly by three-coordinate radar, and the spatial orientation information can be provided by the guidance device of gun-launched UAV. Suppose the intercepted target is a controlled system, the position information of the intercepted target is xy(k)=[s(k)θ(k)]T, and the velocity and spatial orientation of the intercepted target is s(k), θ(k). At the same time, the intercepting target position state information can be expressed in the form of plane position coordinates x(k)=[x(k)y(k)]T. The relationship between the two formulas of state is as follows,
The transformation of Eq (3.1) into a state-space form is obtained:
The extended Kalman filter fusion (EKF) algorithm is used to fuse the data and predict the trajectory of the interception target. The EKF requires that the form Eq (3.2) be linearized at each sampling time. Therefore, the first order Taylor approximation is carried out at the work point ˆxy(k)=[ˆs(k)ˆθ(k)]T, and the substitution (3.2) is obtained, can get:
In the formula: B(k)=[ts^cosθ(k)−tsˆs(k)^sinθ(k)ts^sinθ(k)−tsˆs(k)^cosθ(k)], w(k)=[ωx(k)ωy(k)]T error caused by linearization.
The measurement formula for the airborne guidance device of a gun-launched UAV with serial number i is as follows:
In the formula: yi(k), Hi, vi(k) are respectively the measurement vector, the observation matrix, and the sensor noise, vi(k)=[vxi(k)[vyi(k)]T the covariance matrix is V.
If there are n artillery gun-launched UAVs to measure the same interception target, the measurement equation can be extended to:
For convenience of expression, the Eq (3.5) is expressed in the following form:
If the system dynamics delay is constant τ, then the target's position after the intercept time should be predicted based on the current measurements. Then the dynamic delay problem of the system is transformed into the track prediction problem of the intercepting target.
For each prediction problem with a prediction step size of τ, the optimal solution with the least error covariance is obtained, namely:
In the formula, x(k+τ) and x(k+τ|k) are the measured position and predicted value of the intercepted target, respectively. x(k+τ) can be obtained by the following formula.
The selection of x(k+τ|k) enable Eq (3.3) yields an optimal trajectory prediction value, that is
In the formula, ˆx(k+τ|k) an estimate for an intercepting target.
4.
Simulation validation
4.1. EKF track prediction
In order to verify the trajectory prediction algorithm of gun-launched UAV cooperative detection for intercepting targets, this paper adopts three gun-launched UAV cooperative detection to verify the algorithm. The simulation condition is that the system delay time constant is 0.2 seconds and the sampling period of airborne detector is 10 Hz. The simulation results are shown in Figures 2–4.
As can be seen from Figures 2 and 3, intersecting with reference trajectory, there are certain errors in the measurement results of the three UAVs alone. After using EKF fusion algorithm to fuse the data detected by three UAVs in this paper. The predicted trajectory and the reference trajectory have high consistency. The simulation results show that the EKF algorithm described in this paper can effectively improve the measurement accuracy and eliminate noise interference.
As can be seen from Figure 4, the method described in this paper can accurately predict the flight trajectory of obstacles when the system delay is 0.2 s, so that the predicted flight trajectory basically coincides with the reference trajectory. The method described in this paper can predict the flight path of intercepting targets and eliminate the influence of system delay.
4.2. RTPN guidance interception law verification
The intercepting guidance law in this paper is based on the assumption that the gun-launched UAV and the intercepting target are at the same altitude. As far as the mobility of gun-launched UAV is concerned, its mobility is as follows: the mobility is not more than 3, the radial mobility is not more than 1 g, intercept target limit overload is 2.5 g. From the previous conclusion, we can see that proportional coefficient should satisfy N≤2.5. When the scaling factor is 0.5, 1.0, 2.0, 2.5, the simulation results are shown in Figures 5–7.
From the simulation effect Figure 5, it can be seen that the gun-launched UAV successfully intercepted the intercepted target and destroyed the target. At the same time, from Figure 6, during the interception process, gun-launched UAV normal overload smooth, without violent fluctuations. As can be seen from Figure 7, during the interception, the radial overload of gun-launched UAV gradually converges to zero, and the target of steady interception with constant velocity is achieved.
In reality, the intercept Target's motion is three-dimensional motion in space. In order to verify the effectiveness of intercepting a target with 3D maneuverability, the interception simulation in 3D environment was carried out. The simulation results are shown in Figures 8–10.
From the simulation result Figure 8, it can be seen that the gun-launched UAV successfully intercepts the interception target with three-dimensional maneuverability and destroys the target. At the same time, it can be seen from Figure 9 that during the interception, the normal overload of the gun-launched UAV is stable without violent fluctuation. As can be seen from Figure 10, during the interception, the radial overload of gun-launched UAV gradually converges to zero and the target of steady interception with constant velocity is achieved.
From the above simulation results, it can be seen that the method proposed in this paper is effective, the problems of saturation overload limitation, effective acquisition, measurement error and dynamic response delay of gun-launched UAV are solved effectively.
4.3. Contrast verification
In order to verify the effect of this method, the RTPN method described in [9] is compared and verified here. Similarly, the interception guidance law here is based on the interception simulation verification of the gun-launched UAV and the interception target at the same altitude. Simulation conditions are as follows:the normal maneuverability of gun-launched UAV is not more than 3 g. The radial mobility is not more than 1 g. The interception target limit overload is 2.5 g. The simulation results are as follows.
From the simulation results, it can be seen that the method proposed in this paper intersects with the method in [9]. The process of maneuvering overload is smaller, the trajectory is smoother, and the target is intercepted earlier.
5.
Conclusions
Aiming at the actual scene of gun launched UAV intercepting "Low-slow-small" targets, combined with the specific maneuverability of gun launched UAV, this paper designs a guidance interception method based on RTPN, and analyzes the engineering problems faced by RTPN method, such as saturation overload limit and capture area of arbitrary maneuvering targets. At the same time, aiming at the measurement error and the delay of dynamic response of the gun-launched UAV, the EKF data fusion track prediction algorithm is proposed.
The simulation results show that the EKF data fusion trajectory prediction method can effectively improve the detection accuracy of intercepting targets and overcome the influence of system delay. At the same time, Using the scale coefficient range and effective capture region proved in this paper, gun launched UAV can effectively intercept maneuvering targets. The process of proof in this paper can effectively solves the problems of RTPN guidance, such as saturation overload limitation, effective acquisition, measurement error and delay of dynamics response of gun-launched UAV.
In this paper, within the author's current knowledge and ability, all the problems of RTPN method in intercepting "Low-slow-small" targets by gun-launched UAV have been solved as much as possible, but there are still the following problems to be further studied by scholars.
1) In order to improve the detection accuracy of "Low-slow-small" targets and reduce the system cost, this paper uses EKF data fusion track prediction algorithm to predict the target trajectory. However, the fusion data of the predicted target trajectory in this paper is only the target motion trajectory detected by several UAVs in cooperation, and the target attitude data is not fused for prediction. The deep fusion of target attitude data and position data to improve the detection accuracy is a problem that has not been done in this paper.
2) In order to intercept the "Low-slow-small" targets, this paper adopts the cooperative detection and interception method of multiple UAVs, but the cooperation of multiple UAVs in this paper is only cooperative detection. How to intercept the "Low-slow-small" target more effectively through more in-depth cooperative algorithm of multiple UAVs is a problem that has not been done in this paper.
Acknowledgments
Thanks to the editors and reviewers for their hard work; thank you to all students for their efforts in flight validation of this study; thanks to Xi'an Aeronautical University, Nanjing University of Aeronautics and Astronautics for providing the research environment and equipment.
Thanks to the following funding projects for funding this research:
1) Shanxi Natural Science Youth Fund Project(2021JQ-858);
2) National Defense Science and Technology Key Laboratory Fund (6142101200108);
3) Shaanxi Provincial Natural Science Foundation Key Project (2022JZ-37);
4) Shaanxi Higher Education Teaching Reform Research Project (21BY163).
Conflict of interest
The author confirms that all the authors of this paper declare that there is no conflict of interest in their contributions in this paper.









 DownLoad:
DownLoad:













