Complex solitons in the conformable (2+1)-dimensional Ablowitz-Kaup-Newell-Segur equation

Wei Gao; Gulnur Yel; Haci Mehmet Baskonus; Carlo Cattani; Wei Gao; Gulnur Yel; Haci Mehmet Baskonus; Carlo Cattani

doi:10.3934/math.2020034

AIMS Mathematics

2020, Volume 5, Issue 1: 507-521. doi: 10.3934/math.2020034

Previous Article Next Article

Research article Special Issues

Complex solitons in the conformable (2+1)-dimensional Ablowitz-Kaup-Newell-Segur equation

1.
School of Information Science and Technology, Yunnan Normal University, Yunnan, China
2.
Final International University, Kyrenia Mersin 10, Turkey
3.
Harran University, Faculty of Education, Sanliurfa, Turkey
4.
Tuscia University, Engineering School (DEIM), Viterbo, Italy

Received: 09 October 2019 Accepted: 29 November 2019 Published: 06 December 2019
MSC : 35Qxx, 35C08, 35L05

In this paper, we study on the conformable (2+1)-dimensional Ablowitz-KaupNewell-Segur equation in order to show the existence of complex combined dark-bright soliton solutions. To this purpose an effective method which is the sine-Gordon expansion method is used. The 2D and 3D surfaces under some suitable values of parameters are also plotted.
Citation: Wei Gao, Gulnur Yel, Haci Mehmet Baskonus, Carlo Cattani. Complex solitons in the conformable (2+1)-dimensional Ablowitz-Kaup-Newell-Segur equation[J]. AIMS Mathematics, 2020, 5(1): 507-521. doi: 10.3934/math.2020034

Related Papers:

Abstract

In this paper, we study on the conformable (2+1)-dimensional Ablowitz-KaupNewell-Segur equation in order to show the existence of complex combined dark-bright soliton solutions. To this purpose an effective method which is the sine-Gordon expansion method is used. The 2D and 3D surfaces under some suitable values of parameters are also plotted.

References

[1]	J. H. He, Application of Homotopy Perturbation Method to Nonlinear Wave Equations, Chaos Soliton. Fract., 26 (2005), 695-700. doi: 10.1016/j.chaos.2005.03.006
[2]	J. H. He, Homotopy perturbation method for bifurcation of nonlinear problems, Int. J. Nonlinear Sci., 6 (2005), 207-208.
[3]	S. J. Liao, Beyond Perturbation: Introduction to the Homotopy Analysis Method, Chapman and Hall/CRC Press, 2003.
[4]	Z. F. Kocak, H. Bulut, G. Yel, The solution of fractional wave equation by using modified trial equation method and homotopy analysis method, AIP Conference Proceedings, 1637 (2014), 504-512. doi: 10.1063/1.4904617
[5]	J. H. He, Exp-function Method for Fractional Differential Equations, Int. J. Nonlinear Sci., 14 (2013), 363-366.
[6]	S. Zhang, H. Q. Zhang, An Exp-function method for new N-soliton solutions with arbitrary functions of a (2+1)-dimensional vcBK system, Comput. Math. Appl., 61 (2011), 1923-1930. doi: 10.1016/j.camwa.2010.07.042
[7]	A. Ali, M. A. Iqbal, Q. M. UL Hassan, et al. An efficient technique for higher order fractional differential equation, Springer Plus, 5 (2016), 281.
[8]	C. Cattani, T. A. Sulaiman, H. M. Baskonus, On the soliton solutions to the Nizhnik-NovikovVeselov and the Drinfeld-Sokolov systems, Opt. Quant. Electron., 50 (2018), 138.
[9]	H. Bulut, T. A. Sulaiman, H. M. Baskonus,et al. Optical solitons and other solutions to the conformable space-time fractional Fokas-Lenells equation, Optik, 172 (2018), 20-27. doi: 10.1016/j.ijleo.2018.06.108
[10]	W. Xian-Lin and T. Jia-Shi, Travelling Wave Solutions for Konopelchenko-Dubrovsky Equation Using an Extended sinh-Gordon Equation Expansion Method, Commun. Theor. Phys., 50 (2008), 1047.
[11]	T. A. Sulaiman, G. Yel, H. Bulut, M-fractional solitons and periodic wave solutions to the Hirota Maccari system, Mod. Phys. Lett. B, 33 (2019), 1950052.
[12]	R. Hirota, Exact solution of the Korteweg-de Vries equation for multiple collisions of solitons, Phys. Rev. Lett., 27 (1971), 1192-1194. doi: 10.1103/PhysRevLett.27.1192
[13]	M. R. Miura, Bäcklund Transformation, Springer-Verlag, Berlin, 1978.
[14]	M. J. Ablowitz, D. J. Kaup, A. C. Newell, et al. The inverse scattering transform-fourier analysis for nonlinear problems, Stud. Appl. Math., 53 (1974), 249-315. doi: 10.1002/sapm1974534249
[15]	M. A. Helal, A. R.Seadawy, M. H. Zekry, Stability analysis solutions for the fourth-order nonlinear Ablowitz-Kaup-Newell-Segurwater wave equation, Appl. Math. Sci., 7 (2013), 3355-3365.
[16]	V. B. Matveev, A. O. Smirnov, Solutions of the Ablowitz-Kaup- Newell-Segur hierarchy equations of the "rogue wave" type: an unified approach, Theor. Math. Phys., 186 (2016), 3355-3365.
[17]	A. M. Wazwaz, The (2+1) and (3+1)-dimensional CBS equations: multiple soliton solutions and multiple singular soliton solutions, Z. Naturforsch Pt A., 65 (2010), 173-181.
[18]	T. Özer, New traveling wave solutions to AKNS and SKdV equations, Chaos Soliton. Fract., 42 (2009), 577-583. doi: 10.1016/j.chaos.2009.01.023
[19]	Z. Cheng, X. Hao, The periodic wave solutions for a (2+1)-dimensional AKNS equation, Appl. Math. Comput., 234 (2014), 118-126.
[20]	A. Ali, A. R. Seadawy, D. Lu, Computational methods and traveling wave solutions for the fourthorder nonlinear Ablowitz-Kaup-Newell-Segur water wave dynamical equation via two methods and its applications, Open Phys., 16 (2018), 219-226. doi: 10.1515/phys-2018-0032
[21]	S. Zhang, Z. Wang, Bilinearization and new soliton solutions of Whitham-Broer-Kaup equations with time-dependent coefficients, J. Nonlinear Sci. Appl., 10 (2017), 2324-2339. doi: 10.22436/jnsa.010.05.05
[22]	D. Y. Chen, X. Y. Zhu, J. B. Zhang, et al. New soliton solutions to isospectral AKNS equations, Chinese Journal of Contemporary Mathematics, 33 (2012), 167-167.
[23]	H. C. Yaslan, A. Girgin, New exact solutions for the conformable space-time fractional KdV, CDG, (2+1)-dimensional CBS and (2+1)-dimensional AKNS equations, Journal of Taibah University for Science, 13 (2018), 1-8. doi: 10.1016/j.jtumed.2017.06.003
[24]	F. Ferdous, M. G. Hafez, Oblique closed form solutions of some important fractional evolution equations via the modified Kudryashov method arising in physical problems, Journal of Ocean Engineering and Science, 3 (2018), 244-252. doi: 10.1016/j.joes.2018.08.005
[25]	R. Khalil, M. Al Horani, A. Yousef, et al. A new definition of fractional derivative, J. Comput. Appl. Math., 264 (2014), 65-70. doi: 10.1016/j.cam.2014.01.002
[26]	A. Atangana, D. Baleanu, A. Alsaedi, New properties of conformable derivative, Open Math., 13 (2015), 889-898.
[27]	C. Cattani, T. A. Sulaiman, H. M. Baskonus, et al. Solitons in an inhomogeneous Murnaghan's rod, European Physical Journal Plus, 133 (2018), 1-12. doi: 10.1140/epjp/i2018-11804-8
[28]	H. M. Baskonus, New acoustic wave behaviors to the Davey-Stewartson equation with power-law nonlinearity arising in fluid dynamics, Nonlinear Dynam., 86 (2016), 177-183. doi: 10.1007/s11071-016-2880-4
[29]	C. Yan, A simple transformation for nonlinear waves, Phys. Lett. A, 224 (1996), 77-84. doi: 10.1016/S0375-9601(96)00770-0
[30]	H. Bulut, T. A. Sulaiman, H. M. Baskonus, New solitary and optical wave structures to the Korteweg-de Vries equation with dual-power law nonlinearity, Opt. Quant. Electron., 48 (2016), 1-14. doi: 10.1007/s11082-015-0274-3
[31]	Z. Yan, H. Zhang, New explicit and exact travelling wave solutions for a system of variant Boussinesq equations in mathematical physics, Phys. Lett. A, 252 (1999), 291-296. doi: 10.1016/S0375-9601(98)00956-6
[32]	H. M. Baskonus, T. A. Sulaiman, H. Bulut, New Solitary Wave Solutions to the (2+1)-Dimensional Calogero-Bogoyavlenskii-Schi and the Kadomtsev-Petviashvili Hierarchy Equations, Indian J. Phys., 91 (2017), 1237-1243. doi: 10.1007/s12648-017-1033-z
[33]	Y. Zhen-Ya, Z. Hong-Oing, F. En-Gui, New explicit and travelling wave solutions for a class of nonlinear evolution equations, Acta. Phys. Sin, 48 (1999), 1-5.
[34]	C. Cattani, T. A. Sulaiman, H. M. Baskonus, et al. On the soliton solutions to the Nizhnik-NovikovVeselov and the Drinfel'd-Sokolov systems, Opt. Quant. Electron., 50 (2018), 138.
[35]	Z. Hammouch, T. Mekkaoui, Travelling-wave solutions for some fractional partial differential equation by means of generalized trigonometry functions, International Journal of Applied Mathematical Research, 1 (2012), 206-212.
[36]	A. Houwe, M. Justin, S. Y. Doka, et. al, New traveling wave solutions of the perturbed nonlinear Schrodinger equation in the left-handed metamaterials, Asian-European Journal of Mathematics, (2018), 2050022.
[37]	M. A. Khan, O. Kolebaje, A. Yildirim, et al, Fractional investigations of zoonotic visceral leishmaniasis disease with singular and non-singular kernel, The European Physical Journal Plus, 134 (2019), 481.
[38]	R. Jan, M. A. Khan, P. Kumam, et. al, Modeling the transmission of dengue infection through fractional derivatives, Chaos Soliton. Fract., 127 (2019), 189-216. doi: 10.1016/j.chaos.2019.07.002
[39]	W. Wang, M. A. Khan, P. Kumam, et al. A comparison study of bank data in fractional calculus, Chaos Soliton. Fract., 126 (2019), 369-384. doi: 10.1016/j.chaos.2019.07.025
[40]	A. Atangana, M. A. Khan, Validity of fractal derivative to capturing chaotic attractors, Chaos Soliton. Fract., 126 (2019), 50-59. doi: 10.1016/j.chaos.2019.06.002
[41]	M. A. Khan, F. Gómez-Aguilar, Tuberculosis model with relapse via fractional conformable derivative with power law, Math. Method. Appl. Sci., 42 (2019), 7113-7125. doi: 10.1002/mma.5816
[42]	M. A. Khan, A. Khan, A. Elsonbaty, et al, Modeling and simulation results of a fractional dengue model, The European Physical Journal Plus, 134 (2019), 379.
[43]	A. Yokus, S. Gulbahar, Numerical Solutions with Linearization Techniques of the Fractional Harry Dym Equation, Applied Mathematics and Nonlinear Sciences, 4 (2019), 35-42. doi: 10.2478/AMNS.2019.1.00004
[44]	X. J. Yang, New general fractional-order rheological models with kernels of Mittag-Leffler functions, Rom. Rep. Phys, 69 (2017), 118.
[45]	K. M. Owolabi, Z. Hammouch, Mathematical modeling and analysis of two-variable system with noninteger-order derivative, Chaos: An Interdisciplinary Journal of Nonlinear Science, 29 (2019), 013145.
[46]	D. W. Brzeziński, Review of numerical methods for NumILPT with computational accuracy assessment for fractional calculus, Applied Mathematics and Nonlinear Sciences, 3 (2018), 487-502. doi: 10.2478/AMNS.2018.2.00038
[47]	M. A. Khan, Z. Hammouch, D. Baleanu, Modeling the dynamics of hepatitis E via the Caputo-Fabrizio derivative, Mathematical Modelling of Natural Phenomena, 14 (2019), 311.
[48]	X. J. Yang, New rheological problems involving general fractional derivatives with nonsingular power-law kernels, Proceedings of the Romanian Academy Series A-Mathematics Physics Technical Sciences Information Science, 19 (2018),45-52.
[49]	D. W. Brzeziński, Comparison of Fractional Order Derivatives Computational Accuracy - Right Hand vs Left Hand Definition, Applied Mathematics and Nonlinear Sciences, 2 (2017), 237-248. doi: 10.21042/AMNS.2017.1.00020
[50]	C. Cattani, Haar wavelet-based technique for sharp jumps classification, Math. Comput. Model., 39 (2004), 255-278. doi: 10.1016/S0895-7177(04)90010-6
[51]	M. Eslami, H. Rezazadeh, The first integral method for Wu-Zhang system with conformable timefractional derivative, Calcolo, 53 (2016), 475-485. doi: 10.1007/s10092-015-0158-8
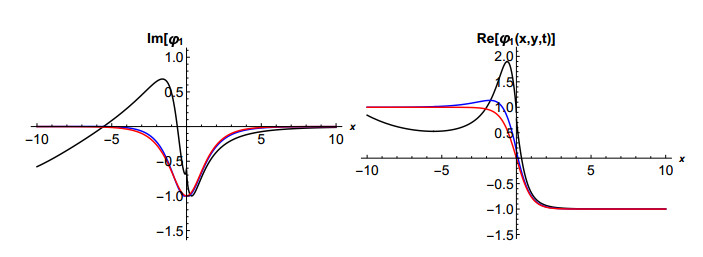
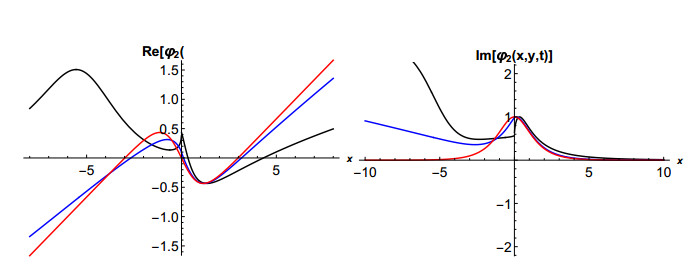
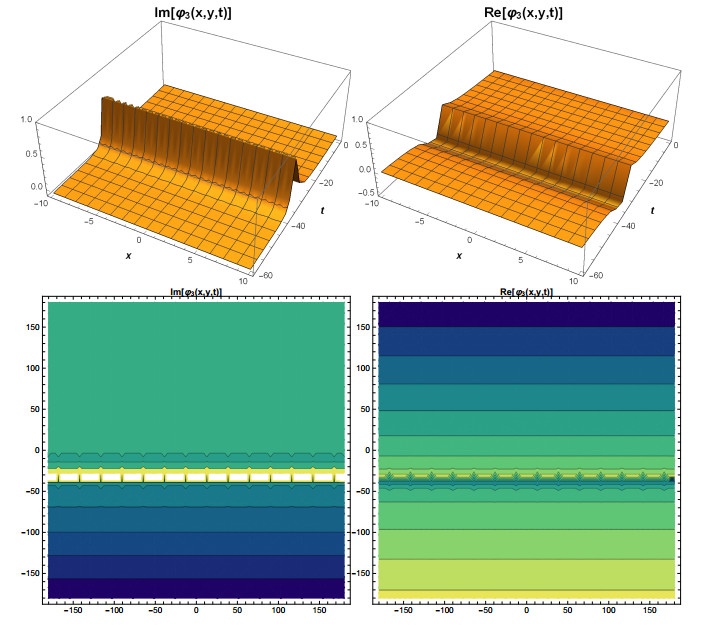
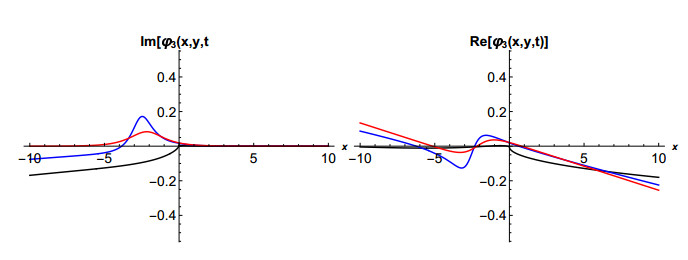
[52]	P. Veeresha, D. G. Prakasha, H. M. Baskonus, Novel Simulations to the time-fractional Fisher's equation, Mathematical Sciences, 13 (2019), 33-42. doi: 10.1007/s40096-019-0276-6
[53]	I. K. Youssef, M. H. El Dewaik, Solving Poisson's Equations with fractional order using Haarwavelet, Applied Mathematics and Nonlinear Sciences, 2 (2079), 271-284.
[54]	X. J. Yang, F. Gao, Y. Ju, H. W. Zhou, Fundamental solutions of the general fractional-order diffusion equations, Mathematical Methods in the Applied Sciences, 41 (2018), 9312-9320. doi: 10.1002/mma.5341
[55]	J. Singh, D. Kumar, Z. Hammouch, et al. A fractional epidemiological model for computer viruses pertaining to a new fractional derivative, Applied Mathematics and Computation, 316 (2018), 504-515. doi: 10.1016/j.amc.2017.08.048
[56]	A. Atangana, Fractional discretization: The African's tortoise walk, Chaos Soliton. Fract., 130 (2020), 109399.
[57]	C. Ravichandran, K. Jothimani, H. M. Baskonus, et al. New results on nondensely characterized integrodifferential equations with fractional order, European Physical Journal Plus, 133 (2018), 1-10. doi: 10.1140/epjp/i2018-11804-8
[58]	K. S. Al-Ghafri, H. Rezazadeh, Solitons and other solutions of (3+1)-dimensional space-time fractional modified KdV-Zakharov-Kuznetsov equation, Applied Mathematics and Nonlinear Sciences, 4 (2019), 289-304. doi: 10.2478/AMNS.2019.2.00026
[59]	W. Gao, B. Ghanbari, H. M. Baskonus, New numerical simulations for some real world problems with Atangana-Baleanu fractional derivative, Chaos Soliton. Fract., 128 (2019), 34-43. doi: 10.1016/j.chaos.2019.07.037
[60]	A. Atangana, D. Baleanu, New fractional derivatives with non-local and non-singular kernel theory and application to heat transfer model, Therm. Sci., 20 (2016), 763-769. doi: 10.2298/TSCI160111018A
[61]	A. Atangana, B. T. Alkahtani, Analysis of the Keller-Segel model with a fractional derivative without singular kernel, Entropy, 17 (2015), 4439-4453. doi: 10.3390/e17064439

Reader Comments

Your name:*

Email:*
© 2020 the Author(s), licensee AIMS Press. This is an open access article distributed under the terms of the Creative Commons Attribution License (http://creativecommons.org/licenses/by/4.0)