Periodic traveling wave, bright and dark soliton solutions of the (2+1)-dimensional complex modified Korteweg-de Vries system of equations by using three different methods

1.   Introduction

Nonlinear partial differential equations (NPDEs) are generally applied to model nonlinear processes in many domains of physics, mathematical biology, and chemistry ^[1,2,3,4,5]. For example, the (1+1)-dimensional Korteweg–de Vries (KdV) equation ^[6,7] and the (1+1)-dimensional modified Korteweg–de Vries (mKdV) equation ^[8,9,10] define the evolution of small amplitude dispersive waves that occur in the shallow water. The (1+1)-dimensional complex modified Korteweg-de Vries (cmKdV) equation is completely integrable and has been suggested as a model for the nonlinear evolution of plasma waves ^{[11,12,13,14,15,16]}. The nonlinear Schrodinger (NLS) equation is a universal model, that describes the evolution of quasi-monochromatic and weakly nonlinear wave trains in media with cubic nonlinearities. In optics, the NLS equation is the main model that characterises the propagation of optical waves in Kerr media ^[17].

Due to the interest in these problems, various analytical solution methods as the Exp-function method ^[18,19], the Darboux transformation ^[20,21], the Hirota method ^{[22,23,24,25]}, the variational approach ^[26,27,28], the sine-cosine method ^[29,30,31], the tanh method ^[32,33,34], and so on were developed.

The great interest in physics and mathematics is the study of the nonlinear excitations of the spin models ^{[35,36,37,38,39]}. In this motivation, Myrzakulov et.al had been presented various integrable spin systems in (2 + 1) dimensions by proposing the interaction of the spin field with vector potential or scalar potential in Ref. ^[39]. Researchers obtained Lax pairs and various interesting reductions in (1+1) and (2+1) dimensions.

In the current work, we mainly study the (2+1)-dimensional cmKdV system of equations that is given by ^[39]

where $q(x, y, t)$ is a complex function, $q^*(x, y, t)$ is a complex conjugate function, $v(x, y, t)$, $w(x, y, t)$ are real functions, $\delta = \pm1$, and subscripts denote the partial derivatives with respect to the variables $x, y, t$. This model is a generalization of the cmKdV equation in the (2+1)-dimension and has great importance for applied ferromagnetism and nanomagnetism ^[39]. In several articles Eqs (1.1) are studied by Darboux transformation (DT). The one-soliton and two-soliton solutions are obtained from DT starting from the zero seed in Ref. ^[40]. The deformed solitons are obtained by $n$-fold DT in ^[41]. Periodic line wave solutions and breather solutions are obtained by starting with a plane wave seed in ^[42]. The order-$n$ breather solutions are derived in Ref. ^[43]. Nonlocal (2+1)-dimensional cmKdV equations are presented in ^[44]. However, traveling wave solutions for Eqs (1.1) have not been found in other studies, which will be our main focus of this paper.

We investigate the (2+1)-dimensional cmKdV equations (1.1) using the sine-cosine method, the tanh-coth method and the Kudryashov method. These methods have been widely applied to nonlinear dispersive and dissipative equations to obtain different types of solutions. For example, the sine-cosine method was used for the Camassa–Holm–KP equation ^[45], the fifth-order KdV equation ^[46], the two-dimensional nonlinear Schrodinger equation ^[47], the coupled Maccari's system ^[48], and other. The authors found solutions in the form solitary waves, periodic solutions. As for the tanh-coth method, it leads to a broader class traveling wave solutions such as bright and dark solitons, kink and anti-kink type solitons, traveling wave, periodic solitary wave, trigonometric functions solutions. The researchers were applied the tanh-coth method to the fifth-order KdV equation ^[49], the coupled Konno-Oono equation ^[50], the system of ion sound and Langmuir waves ^[51], the generalized nonlinear Schrodinger equations ^[52] and so on. The Kudryashov method was applied for the generalized Kuramoto–Sivashinsky equation, the Burgers-Korteweg-de Vries equation, the Bretherton equation, the Kawahara equation, and others in Refs. ^{[53,54,55,56]}.

The paper is organized as follows. Lax pair for the (2+1)-dimensional cmKdV equations are given in Sect. 2. Then the three methods are used to construct the exact solutions in Sect. 3–Sect. 5. The physical interpretation is presented in Sect. 6. Finally, we present concluding remarks in Sect. 7.

2.   Lax pair

The corresponding Lax pair for Eqs (1.1) is

where

with

The compatibility condition

infers the following (2+1)-dimensional coupled cmKdV equations:

where $q, r$ are complex functions, $v, w$ are real functions. By setting $r = \delta q^{*}$ Eqs (2.4) reduce to the (2+1)-dimensional cmKdV equations (1.1).

3.   The sine-cosine method

We use the sine-cosine method to obtain sine and cosine solutions for the (2+1)-dimensional cmKdV system of equations (1.1). The description of the method used in the following subsection is given in ^[4].

3.1.   Description of method

According to method the partial differential equation (PDE)

can be transformed to ordinary differential equation (ODE)

by applying a wave variable

As long as all terms contain derivatives Eq (3.2) is integrated. The solutions of ODE (3.2) can be presented in the form

or

where $\xi = x+y+ct$ and the parameters $\beta, \mu$ and $\alpha$ will be defined, $c$, $\mu$ are constants. The derivatives of Eq (3.3) are

and the derivatives of Eq (3.4) become

and so on for the other derivatives.

Applying (3.3)–(3.8) into the reduced ODE (3.2) yields a trigonometric equation of $\cos^K(\mu\xi)$ or $\sin^K(\mu\xi)$ terms. Thereafter, we define the parameters by first balancing exponents of each pair of sine or cosine to determine $K$. Further, all coefficients of the identical power in $\cos^k(\mu\xi)$ or $\sin^k(\mu\xi)$ are collected, where these coefficients have to vanish. Then, a system of algebraic equations with the unknown $\alpha, \mu, \beta$ will be obtained and from that coefficients can be determined.

3.2.   Implementation

For applying the sine-cosine method, we have to reduce Eqs. (1.1) to ODE. By taking transformation

where $a, b, d$ are real constants and $Q(x, y, t)$ is the real valued function, Eqs (1.1) are reduced to the following system

Substituting the wave transformation

into system of Eqs (3.10)–(3.12), we obtain that

Integrating Eqs (3.17)–(3.18) once, with respect to $\xi$ and taking constants of integration is zero, we obtain

Substituting Eq (3.19) into Eq (3.16), we derive the following ODE

where prime denotes the derivation with respect to $\xi$. By separating real and imaginary parts in Eq (3.20), we get the ordinary differential equations:

Integrating Eq (3.21) once, with respect to $\xi$, gives

where $L$ is a constant of integration. As the same function $Q(\xi)$ satisfies both Eqs (3.22) and (3.23), we have the next constraint condition:

By using condition (3.24), we have

We rewrite Eq (3.22) as

In the next subsection, we solve Eq (3.26) by the sine-cosine method.

3.2.1.   The sine solutions

According to method the solution of Eq (3.26) can be found by transformation

To find the sine solution we use Eq (3.27) and its second order derivative

Substitute (3.27) and (3.28) into (3.26) we get

Applying the balance method, by equating the exponents of $sin(\mu\xi)$, from (3.29) we determine $\beta$:

Substituting Eq (3.30) into Eq (3.29) to get

We equate exponents and coefficients of each pair of the $\sin(\mu \xi)$ functions and obtain a system of algebraic equations

By solving the system (3.32)–(3.33), we obtain:

By substituting Eq (3.34) into Eq (3.27) and then obtained result in Eq (3.19) and Eq (3.9) we derive the exact solutions for the (2+1)-dimensional cmKdV equations (1.1)

where $c = 2ab+a^2+\frac{d-a^2b}{2a+b}$.

3.2.2.   The cosine solutions

The cosine solution of (3.26) can be found by transformation

To find the cosine solution we use Eq (3.41) and its second order derivative

Substitute (3.41) and (3.42) into (3.26) we get

Applying the balance method, by equating the exponents of $cos(\mu\xi)$, from Eq (3.43) we determine $\beta$:

Substituting Eq (3.44) into Eq (3.43) to get

We equate exponents and coefficients of each pair of the $\cos(\mu \xi)$ functions and obtain a system of algebraic equations

Next, by solving the system (3.46)–(3.47), we get:

By substituting Eq (3.48) into Eq (3.41) and then obtained expression in Eq (3.19) and Eq (3.9) we derive the exact solutions for the (2+1)-dimensional cmKdV equations (1.1)

where $c = 2ab+a^2+\frac{d-a^2b}{2a+b}$.

The solutions (3.35)–(3.41) and (3.51)–(3.57) are depicted in Figures 1–5.

4.   The tanh-coth method

We apply the tanh-coth method to derive traveling wave solutions for the (2+1)-dimensional cmKdV system of equations. The first, the tanh method was presented by Malfliet ^[26,27,28] and then was expanded by Wazwaz ^[4,29] In the next subsection, the description of the method is given by ^[4].

4.1.   Description of method

Partial differential equation (PDE)

can be transformed to an ordinary differential equation (ODE)

by applying a wave variable

where $c$ is a constant. As long as all terms contain derivatives Eq (4.2) is integrated. Applying a new independent variable

where $\mu$ is the wave number, we have the next change of derivatives:

The tanh-coth method allows the application of the finite expansion in the next form:

where $a_0, a_1, a_2, ..., a_N$, $b_1, b_2, ..., b_N$ are unknown coefficients. Parameter $M$ is defined by balancing nonlinear terms and the highest order derivative term in Eq (4.2). By substituting the value of $Q(\xi)$ from (4.4) in Eq (4.2), and comparing the coefficient of $Y^n$ we can derive the coefficients $a_0, a_1, a_2, ..., a_N$, $b_1, b_2, ..., b_N$.

4.2.   Implementation

Let's study ODE (3.26)

where prime denotes the derivation with respect to $\xi$. To find the value of $M$ we balance the highest order derivative $Q^{''}$, which has the exponent $M+2$, with the nonlinear term $Q^3$, which has the exponent $3M$ in Eq (4.5). It gives $3M = M+2$ that yields $M = 1$. Then, the tanh-coth method let to apply the substitution

We substitute Eq (4.6) into Eq (4.5) and collect the coefficients of $Y^{n}$, then we have a system of algebraic equations for $\mu, a_0, a_1, b_1$. By solving the obtained system with the aid of Maple, we get the next results:

Result 1:

Result 2:

Result 3:

Result 4:

By substituting Eq (4.6) into Eq (3.19) and Eq (3.9) we have solutions as

where $\xi = x+y+ct$ with $c = 2ab+a^2+\frac{d-a^2b}{2a+b}$.

Finally, applying the coefficients (4.7)–(4.12) into Eqs (4.13)–(4.15), we derive exact solutions for the (2+1)-dimensional cmKdV equations (1.1) in the next forms

Result 1:

Result 2:

Result 3:

Result 4:

where $\xi = x+y+ct$, with $c = 2ab+a^2+\frac{d-a^2b}{2a+b}$.

The solutions (4.16)–(4.27) are presented in Figures 6–14.

5.   Kudryashov method

5.1.   Description of method

Partial differential equation (PDE)

can be transformed to an ordinary differential equation (ODE)

by applying a wave variable

where $c$ is a constant. As long as all terms contain derivatives Eq (5.2) is integrated. To find dominant terms we substitute

into all terms of equation (5.2). Then we ought to compare degrees of all terms of equations and choose two or more with the highest degree. The maximum value of $p$ is called the pole of the equation (5.2) and we denote it as $N$. The method can be applied when $N$ is integer. The exact solution of equation (5.2) is looked in the form

where $R(\xi)$ is the following function

We can calculate number of derivatives by

5.2.   Implementation

Let's study ODE (3.26)

where prime denotes the derivation with respect to $\xi$. From Eq (3.26) we find $N = 1$ then we look for the solution of Eq (3.26) in the form

The second derivative of Eq (5.9) is

Substituting (5.9)–(5.10) into (3.26) we obtain the system of algebraic equations. By solving it we find coefficients as

Substituting (5.11) in (5.9) and then obtained expressions in Eq (3.19) and Eq (3.9) we have solutions for Eqs (1.1) by the following form

where $\xi = x+y+ct$, with $c = 2ab+a^2+\frac{d-a^2b}{2a+b}$.

6.   Physical interpretation

In this section, we will give the physical explanation of the obtained exact solutions in Sect. 3–Sect. 4. In general, Eqs (1.1) have the parameter $\delta = \pm1$, in our research we take case when $\delta = -1$. In order to analyze solutions (3.35)–(3.40) and (3.49)–(3.54), we consider two cases that can yield various solutions.

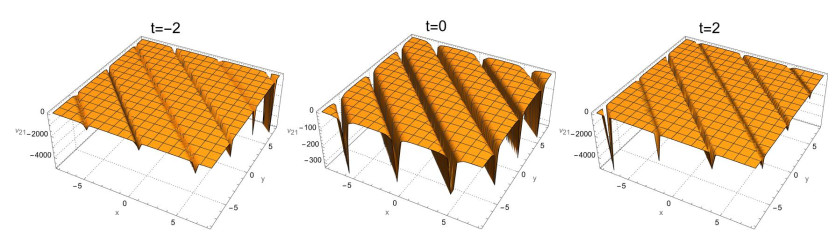
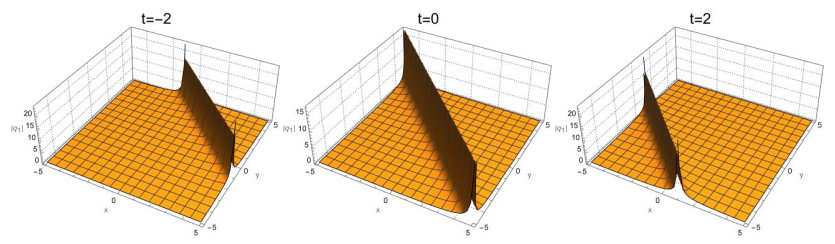
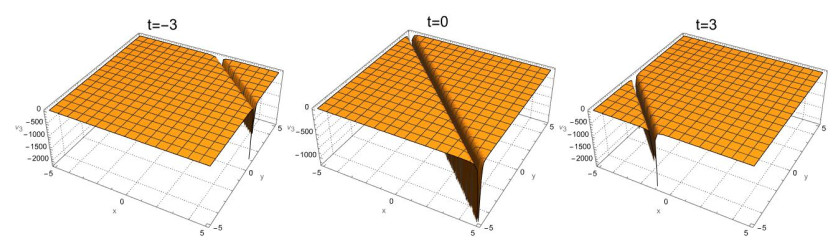
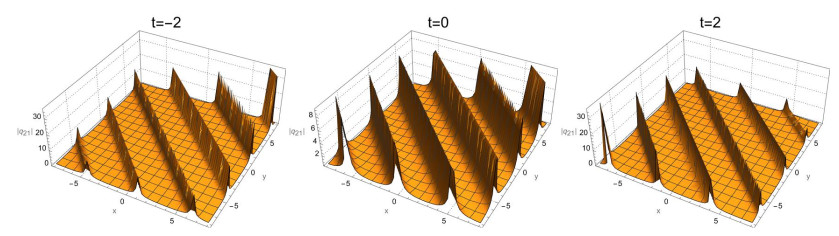
In case $\frac{(d-a^2b)}{(2a+b)} > 0$, $\delta = -1$ by taking the values as $a = 1, b = 1, d = 2, \delta = -1$ in Eqs (3.34) and (3.50) we obtain that $c = \frac{10}{3}, \alpha = \frac{1}{\sqrt{3}}, \mu = \frac{1}{\sqrt{3}}, \frac{(d-a^2b)}{(2a+b)} = \frac{1}{3}$. With the above parameters in Figures 1–2 we present 3D plots of solutions $q_{21}, v_{21}$ (3.49)–(3.50) on the $x-y$ plane at $t = -2, t = 0, t = 2$. We notice that solutions are obtained with the sine-cosine method gives the periodic solutions. To consider the case $\frac{(d-a^2b)}{(2a+b)} < 0, \delta = -1$ we take the parameters as $a = 1, b = 1, d = -2, \delta = -1$ in Eqs (3.34) and (3.50) and then obtain $c = 2, \alpha = i, \mu = i, \frac{(d-a^2b)}{(2a+b)} = -1$.The graphical representations of the solutions with complex $\alpha$ and $\mu$ are given in Figures 3–4. As we see, the solutions $q_{12}, v_{12}$ (3.38)-(3.39) can be soliton solutions. It can be seen that the bright one-soliton $q_{12}$ and dark one-soliton $v_{12}$ keep their directions, widths, and amplitudes invariant during the propagation on the $x-y$ plane. Figure 5 displays propagation of the bright soliton solutions $q_{22}, v_{22}, w_{22}$ in 2D plot at $y = 0, t = -2, t = 0, t = 2$. It is well known that bright soliton is a pulse on a zero-intensity background. However, the dark soliton is featured as a localized intensity dip below a continuous-wave background.

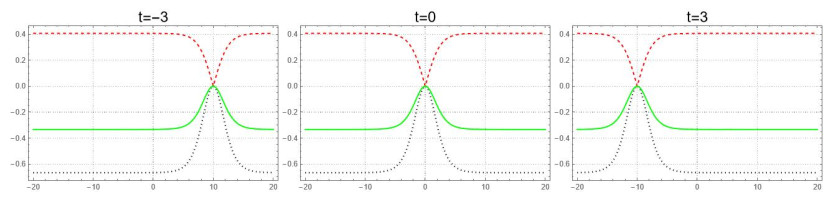
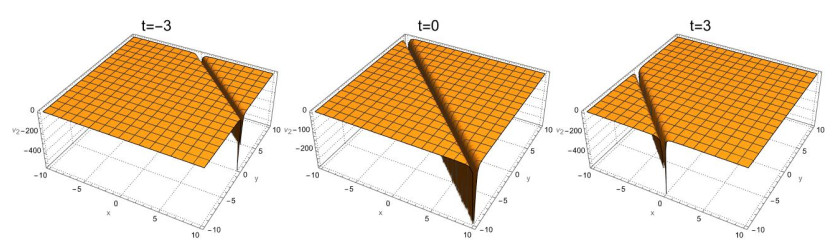
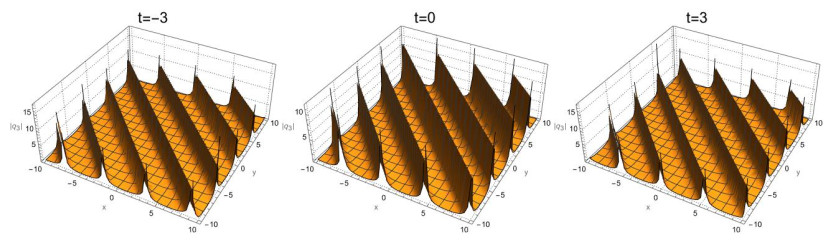
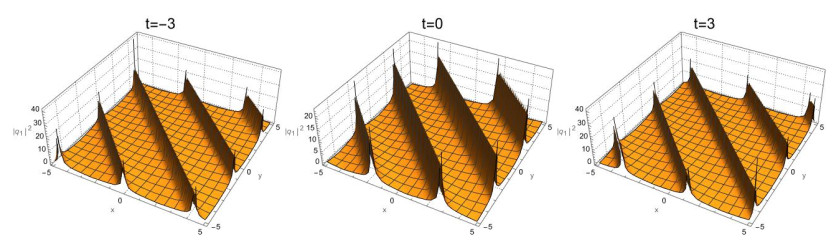
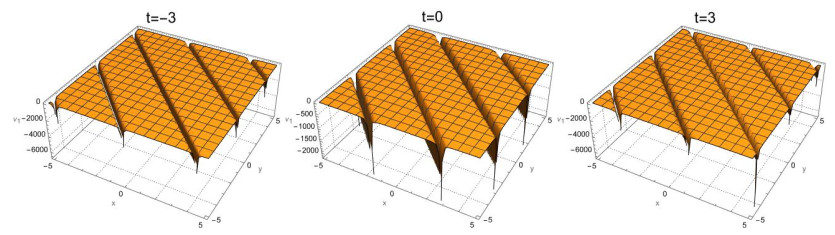
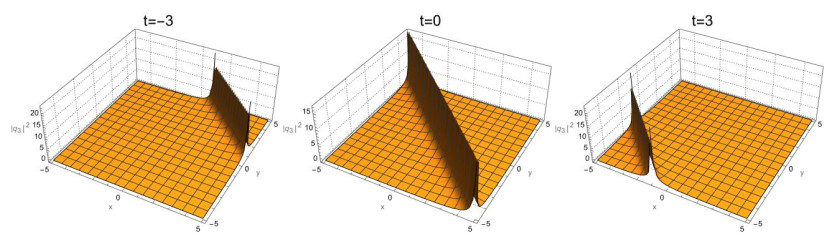
Figure 6 displays the time evolutions of the solutions (4.16)–(4.18) with the values $a = 1, b = 1, d = 2, \delta = -1, c = \frac{10}{3}, a_0 = 0, a_1 = \frac{1}{\sqrt{6}}, b_1 = 0, \mu = \frac{1}{\sqrt{6}}, \frac{(d-a^2b)}{(2a+b)} = \frac{1}{3}, y = 0$. As we notice from 2D plots the solution $q_{31}$ is dark soliton, $v_{31}, w_{31}$ are bright solitons. The evolution of the bright soliton $q_{32}$ and dark solitons $v_{32}$ in 3D at $t = -3, t = 0, t = 3$ are displayed in Figures 7–8. It can be seen that the bright solitons and the dark solitons keep their directions invariant during the propagation on the x–y plane. Moreover, periodic type solutions $q_{33}, v_{33}$ at $t = -3, t = 0$, and $t = 3$ are presented in Figures 9–10. The shape of solutions $q_{34}, v_{34}, w_{34}$ are almost same as $q_{32}, v_{32}, w_{32}$. It gives the soliton solutions. In case $\frac{(d-a^2b)}{(2a+b)} < 0, \delta = -1$ we take the parameters as $a = 1, b = 1, d = -2, \delta = -1$ in Eqs (4.16)–(4.18), then the solutions $q_{31}, v_{31}$ give the periodic solutions that are displayed in Figures 11–12. But for $q_{33}, v_{33}$ the bright and dark soliton solutions can be derived that is Figures 13–14. In case $q_{33}, v_{33}$ the values are taken as $a = 1, b = 1, d = -2, \delta = -1, c = 2, a_0 = 0, a_1 = -\frac{1}{2}, b_1 = \frac{1}{2}, \mu = -\frac{1}{2}, \frac{(d-a^2b)}{(2a+b)} = -1$ within the interval $-5\leq x, y\leq 5$ for $t = -3, t = 0, t = 3$. The periodic solutions are obtained also for $q_{32}, v_{32}, w_{32}, q_{34}, v_{34}, w_{34}$.

Thus, the considered above cases show that the different choices of the parameters $a, b, d, c$ yield a number of waveforms such as periodic solutions, bright soliton, and dark soliton. Moreover, the tanh-coth method yields more solutions compared to solutions by the sine-cosine method, the Kudryashov method.

7.   Conclusions

In the paper, the (2+1)-dimensional cmKdV system of equations is studied using the sine-cosine method, the tanh-coth method, and the Kudryashov method. As a result, various types of exact solutions such as bright solitons, dark solitons, and periodic wave solutions are obtained. In addition, we have shown the graphical structures of some derived results in Figures 1–14 and then interpreted the nature of the profiles shown. The main advantage of the sine-cosine, tanh-coth and Kudryashov methods is that, unlike existing methods such as Hirota's bilinear method or the inverse scattering method, tedious algebra and guesswork can be avoided. By varying the choice of parameters, different waveforms can be generated, such as the bell shape, the anti-bell shape, and other forms of the solutions. The results obtained are new, as solutions for traveling waves have not been found before. Moreover, this work extends the work on the (2+1)-dimensional cmKdV equations [39–44] by deriving a variety of exact solutions. It is expected that the methods used in this work will open new horizons for the study of NPDEs arising in physics. Moreover, it will also be interesting to study the integrability properties such as the infinite number of conservation laws and geometry properties for Eqs (1.1). Related work is underway and results will be reported separately.

Acknowledgments

The research work was prepared with the financial support of the Committee of Science of the Ministry of Education and Science of the Republic of Kazakhstan, IRN project AP09057947.

Conflict of interest

The authors declare no conflict of interest.