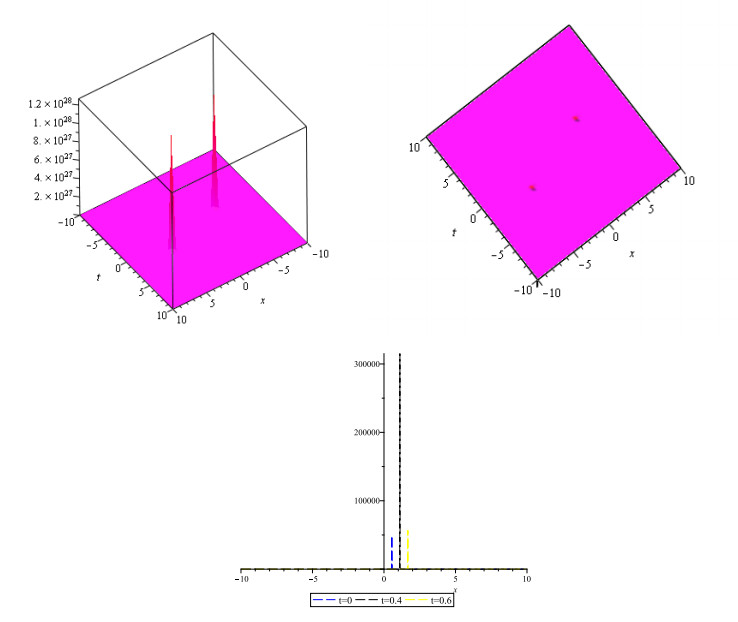
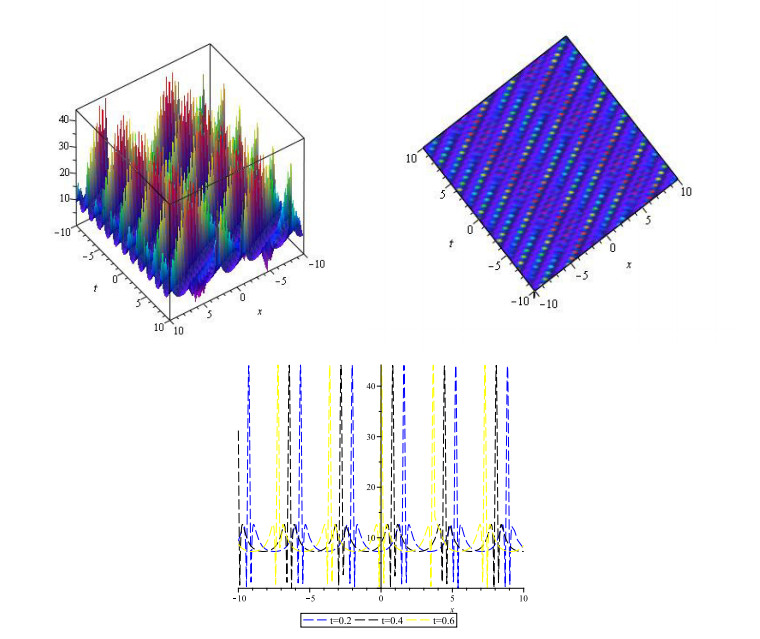
We present new exact solutions for the (1+1)-dimensional Kaup-Kupershmidt (KK) equation by employing method of double (G′/G,1/G)-expansion. We express solutions by hyperbolic, trigonometric and rational functions explicitly. Computational results indicate the efficiency and applicability potential of the method.
1.
Introduction
Nonlinear evolution equations (NLEEs) model many complex phenomena in physics including plasma, solid state, chemical and optical fibers, nonlinear optics, fluid mechanics, etc. Exploring exact traveling wave solutions plays a significant role in nonlinear physics. For this purpose, a number of techniques were developed including method of modified Khater [1,2], first integral [3,4], functional variable [5], expansions [6,7] of new generalized (G′/G) [8,9,10], new Φ6-model [11], Jacobi elliptic function [12,13], sine-Gordon [14], bifurcation [15,16], exp-function [17,18], new auxiliary equation [19], exp(-ϕ(ξ))-expansion [20,21], fan sub-equation [22,23], inverse scattering [24], generalized Kudryshov [25,26,27], Hirota's bilinear [28,29], extended direct algebraic [30], Lie group [31].
Consider the (2+1)-dimensional KK equations [32]
where σ2=1,∂−1x=∫dx. This equation has been widely applied in many branches of physics like plasma physics, fluid dynamics, nonlinear optics, and so forth. If we take u(x,y,t)=u(x,t), Eq (1.1) becomes the (1+1)-dimensional KK equation [32]
In [33], method of exp-function was applied to Eq (1.2). In [32], symmetric method was applied to the nonlinear (2+1)-KK equation.
The method of the present paper, a candid, succinct and efficient technique, considered as a generalization of (G′/G)-expansion technique [34,35,36,37] was developed in [38,39,40,41,42,43,44,45]. Main purpose of this paper is to investigate the applicability of the method to (1+1)-dimensional KK equation which was not considered in the history of research so far.
2.
Double (G′/G,1/G)-expansion technique
We shortly overview the method in such a fashion that maintains four remarks and five basic postulates:
Remark I. If we set up
in
then we must have the relations
wherein λ and β are parameters.
Remark II. If λ is negative, general solution of (2.2) is:
and we receive the following relation
wherein D1 and D2 are arbitrary constants and α1=D21−D22.
Remark III. If λ is positive, general solution of (2.2) is:
consequently, we obtain
wherein α2=D21+D22.
Remark IV. If λ=0, the general solution of (2.2),
and therefore we get,
Now let us consider:
wherein R is a polynomial function in u and ut=∂u∂t, ux=∂u∂x, uy=∂u∂y, uxx=∂2u∂x2, uyy=∂2u∂y2, uxy=∂2u∂x∂y and so on.
Postulate 1. Consider:
wherein η, ω and c are parameters. By traveling wave transformations (2.11), the Eq.(2.10) can be reduced to:
wherein T is a polynomial.
Postulate 2. Let us assume that the following relation is the general solution expressed by a polynomial:
wherein a0, ai and bi(i=1,2,3,...,N) are the constant coefficients such that a2N+b2N≠0.
Postulate 3. By homogeneous balance, we determine N in Eq (2.13).
Postulate 4. To convert the left-hand-side of Eq (2.12) into a polynomial function in ψ and ϕ, we write Eq (2.13) into Eq (2.12) with Eq (2.3) and Eq (2.5). By solving polynomial, we obtain the system: in a0, ai, bi(i=1,2,3,...,N), λ(<0), β, η, ω, c, D1 and D2. We solve this system with Mathematica. Setting values of above algebraic constants in Eq (2.13), solutions by hyperbolic functions in Eq (2.12) are obtained.
Postulate 5. Similar to Postulate 4, substituting Eq (2.13) into Eq (2.12), using Eq (2.3) and Eq (2.5) (or Eq (2.3) and Eq (2.7)), we obtain the exact traveling wave solutions of Eq (2.12) demonstrated by trigonometric functions.
3.
Applications
Let us consider transformation:
wherein c is a parameter, which reduces Eq (1.2) to:
According to postulate 2, the positive number N=2 is obtained by balancing between u(5) and u2u′, thus general solutions of Eq (3.2) is:
whereina0, ai and bi(i=1,2) are constant coefficients such thata2N+b2N≠0(N=1,2), ϕ(ξ) and ψ(ξ) are satisfied by the Eq (2.3). Now, there are three categories of solutions of Eq (3.2):
Category 1: When λ<0 (solutions by hyperbolic functions):
Writing Eq (3.3) with Eq (2.3) and Eq (2.5) into Eq (3.2), Eq (3.2) forms a polynomial in ψ(ξ) and ϕ(ξ). Solving this polynomial, we obtain a system: a0, a1, a2, b1, b2, λ(<0), β, c and α1. Solving this system with Mathematica, we obtain the values of a0 a1, a2, b1, b2, β and c as:
Result 1:
Writing these constants from Eq (3.4) into (3.3) and by Eq (2.1) and Eq (2.4), we obtain explicit solutions of Eq (1.2):
wherein ξ=x−11λ2t9 and α1=D21−D22.
In particular, if we choose D1≠0, D2=0 and β=0 in Eq (3.5), we get:
Similarly, if we choose D2≠0, D1=0 and β=0 in Eq (3.5), we get:
wherein i=√−1.
Result 2:
Explicit solutions of Eq (1.2) are given by:
wherein ξ=x−λ2t144 and α1=D21−D22.
In particular, if we choose D1≠0, D2=0 and β=0 in Eq (3.9), we get:
Similarly, if we choose D2≠0, D1=0 and β=0 in Eq (3.9), we get:
wherein i=√−1.
Result3:
wherein β2+λ2α1≠0.
We get explicit solutions of Eq (1.2) as:
wherein ξ=x−λ2t(β4−28λ2β2α1+16λ4α21)144(β2+λ2α1)2 and α1=D21−D22.
In particular, if we choose D1≠0, D2=0 and β=0 in Eq (3.13), we get:
Similarly, if we choose D2≠0, D1=0 and β=0 in Eq (3.14), we get:
Category 2: For λ>0, (i.e. trigonometric functions),
According to Postulate 5, if we execute as the category 1, we attain the values of a0, a1, a2, b1, b2, β and c as the following results:
Result 1:
Writing constants in Eq (3.16) into Eq (3.3) and by Eq (2.1) and Eq (2.6), we get explicit solutions of Eq (1.2):
wherein ξ=x−11λ2t9 and α2=D21+D22.
Result 2:
We get explicit solutions of Eq (1.2) as:
wherein ξ=x−λ2t144 and α2=D21+D22.
Result 3:
wherein −β2+λ2α2≠0.
We get explicit solutions of Eq (1.2) as:
wherein ξ=x−λ2t(β4+28λ2β2α2+16λ4α22)144(−β2+λ2α2)2 and α2=D21+D22.
Category 3: For λ=0, (i.e.rational functions),
According to Postulate 5, if we execute as the category 1, we attain the values of a0, a1, a2, b1, b2, β and c as the following results:
We get explicit solutions of Eq (1.2) as:
wherein ξ=x−5β4t16(−D21+2βD)2 and −D21+2βD≠0.
If we set up the particular values of the arbitrary constants if we choose D1, D2 and βin the above Eq (3.17), Eq (3.19), Eq (3.21) and Eq (3.23), we attain abundant new explicit wave solutions of KK equation which are unexposed for minimalism of length of the paper.
4.
Conclusions and outlook
We obtained new explicit solutions for the (1+1)-dimensional KK equation. We achieved solitary wave solutions for analogous traveling wave solutions of Eq (1.2). These affluent solutions including bell and anti-bell solitons, kink and anti-kink solitons, periodic and rational functions of KK equation indicate that double (G′/G,1/G)-expansion technique is more powerful than the method of (G′/G,1/G)-expansion. Comparing the solutions with the ones in [33], we presume that all the solutions are renewed which are un-indicted elsewhere. Our mentioned method is more powerful and also an offering method to demonstrate many higher order nonlinear PDEs. We will investigate the applicability of the method to (2+1)-dimensional KK equation in a future extension of the present work.
Acknowledgments
The work was supported by the Natural Science Foundation of China (Grant Nos. 61673169, 11301127, 11701176, 11626101, 11601485).
Conflict of interest
The authors declare that they have no known competing financial interests or personal relationships that could have appeared to influence the work reported in this paper.
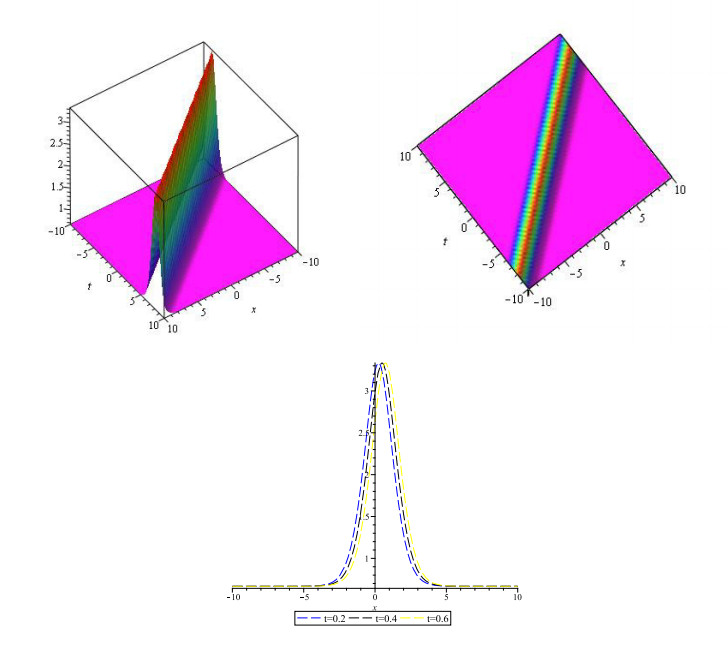









 DownLoad:
DownLoad: