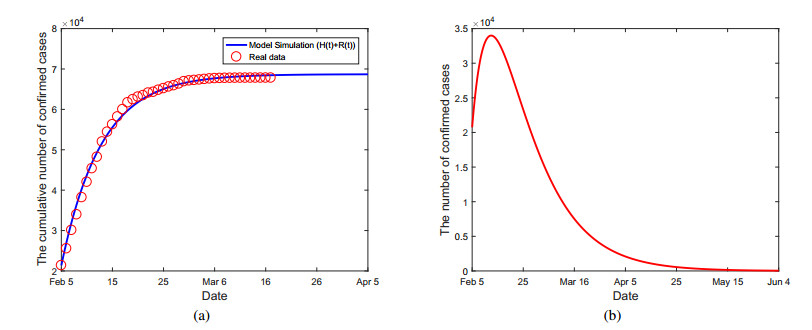
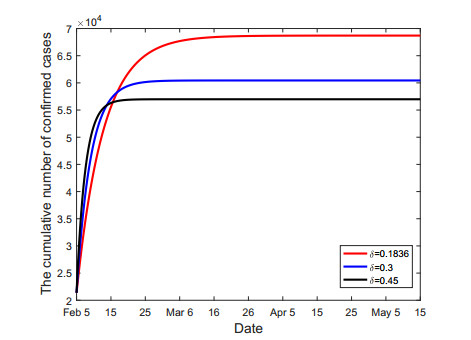
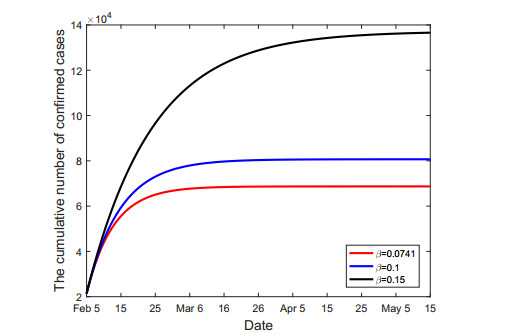
Corona Virus Disease 2019 (COVID-19) which was firstly reported in Wuhan city last December, and then spread throughout the country rapidly. In this paper, we propose an SIHR model that predicts the course of the epidemic to help plan an effective control strategy. The values of parameters in the model are estimated on the basis of fitting to the reported data of COVID-19 from February 5 to March 17, 2020, in Hubei province. The results showed that (i) the peak of total confirmed cases will arrive around late February of 2020, (ii) the cumulative number of confirmed cases to be around 68,000 cases, (iii) the disease will end in mid-May of 2020. All these findings are consistent with the actual situation of Hubei province. Based on the empirical results, it is recommended to strengthen community closures and increase medical resources, which is the key to controlling the spread of COVID-19 in Hubei province.
1.
Introduction
Suppose the quasi-periodic linear system
in which t∈R, x∈Cr, A(ω1t,ω2t,…,ωrt) is quasi-periodic(q-p) time dependent r×r matrix and the basic frequencies ω1,…,ωr are rational independent.
The system (1.1) is said to be reducible, if there exists a so called quasi-periodic Lyapunov-Perron (L-P) transformation x=P(ω1t,…,ωrt)y, so that the transformed system is a linear system with constant coefficients. We call the transformation x=P(ω1t,…,ωrt)y is quasi-periodic L-P transformation, if P(t) is non singular and P, P−1 and ˙P are quasi-periodic and are bounded in t∈R.
Many researchers have discussed the reducibility problems for quasi-periodic linear systems. For r=1, i.e., the periodic case, the well known Floquet theorem states that there always exists a periodic change of variables x=P(ω1t)y so that the system ˙x=A(ω1t)x is reducible to a constant coefficient system ˙y=By,˙φ=ω, where B is a constant matrix. For r>1, i.e., quasi-periodic case, there is an example in [1] which shows that the system (1.1) is not always reducible. Earlier for q-p case, Coppel [2] proved that a linear differential equation with bounded coefficient matrix is pseudo-autonomous if and only if it is almost reducible and Johnson and Sell [3] showed that if (1.1) satisfies the full spectrum assumption, then there is a quasi-periodic linear change of variables x=P(ω1t,…,ωrt)y that transforms (1.1) to a constant coefficient system ˙y=By, where B is a constant matrix. Their results failed for the pure imaginary spectrum [4].
The first reducibility result by KAM method was given by Dinaburg and Sinai [5] who proved that the linear Schrödinger equation d2xdt2+q(ω1t,ω2t,…,ωrt)x=λx is reducible for 'most' large enough λ in measure sense, where ω is fixed satisfying the Diophantine condition: |⟨k,ω⟩|>α−1|k|τ, 0≠k∈Zr, where α,τ are positive constants. See also Rüssmann [6] for a refined result.
In 1992 Jorba and Simó [7] considered the following linear differential system
in which A,ˉQ are constant diagonal matrices, and Q is an analytic q-p matrix having r basic frequencies, and with a small parameter λ. Using the KAM method, They proved that there exists a positive measure Cantor subset E⊂(0,λ0), λ0≪1 such that for any λ∈E, the system (1.2) is reducible, provided that the following non-degeneracy conditions
where αi(λ), 1≤i≤m, are the eigenvalues of ˉA=A+λˉQ. In 1999, Xu [8] improved the result for the weaker non-degeneracy conditions.
Eliasson [9] considered the following linear Shrödinger equation
For almost all λ∈(a,b), the full measure reducibility result is proved in a Lebesgue measure sense provided that Q is small. On the other hand, Krikorian [10] generalized the work for linear systems with coefficients in so(3). Then, Eliasson [11] discussed the full measure reducibility result for the following parameter dependent systems
in which t∈R, x∈Cd, a constant matrix A of dimension d×d, the parameter λ∈(a,b), and an analytic mapping Q:Tr×(a,b)→gl(m,C), a Diophantine vector (ω1,…,ωr) and for sufficiently small |Q|.
He and You [12] proved the positive measure reducibility result for the following quasi-periodic skew-product systems: dxdt=(A(λ)+Q(φ,λ))x,˙φ=ω, close to constant. The result is proved by using KAM method, under weaker non-resonant conditions and non-degeneracy conditions.
All the above mentioned results only discuss the reducibility of linear systems with the Diophantine condition
where α>1 and τ>d−1.
In our problem, we are going to focussed on the Brjuno-Rüssmann condition (see [13,14]) which is slightly weaker than the Diophantine condition (1.5), if the frequencies ω=(ω1,…,ωd) satisfy
where α>1 and some Rüssmann approximation function Δ. These are continuous, increasing and unbounded functions Δ:[0,+∞)→[1,+∞) such that Δ(0)=1 and
Remark: If we have Δ(t)=tτ, then the Brjuno-Rüssmann conditions (1.6) becomes the Diophantine conditions (1.5).
Furthermore, in this article we will generalize the result of He and You [12] for quasi-periodic linear systems using Brjuno-Rüssmann non-resonant condition which is slightly weaker than the Diophantine condition.
This article is organized as: at the end of Section 1, the statement of the main result is given and in Section 2 proof of the main result is given.
To state our main result, we now give some definitions and results.
Definition 1.1. ([15,16])
A vector ω∈Rd is Brjuno if the following condition is satisfied
The set of Brjuno vectors is of full Lebesgue measure. In particular, it contains all Diophantine vectors. Conversely, there are vectors that are Brjuno and are not Diophantine.
This article aims to discuss the positive measure reducibility for q-p linear systems like (1.4) proposed by He and You [12]. The existed positive measure reducibility is discussed by using the Diophantine conditions, but we will discuss the positive measure reducibility using the Brjuno-Rüssmann condition.
Equivalently, for the system (1.4), we suppose the following skew-product system
where x∈Cr, the parameter λ∈Λ=(a,b), A is a r×r constant matrix, and Q(φ,λ) is an analytic mapping from Tr×(a,b) to gl(m,C), (ω1,ω2,⋯,ωn) is a Brjuno vector and |Q| is sufficiently small.
In our discussion, we will use the following equivalent formulation of reducibility:
Consider
be an analytic q-p linear system. For the skew-product system, it can be rewritten as:
where b,B are in the Lie algebra g=g(m,C) and their solutions have values in the Lie group G=GL(m,C). For a complex neighbourhood Wh(Tr) if B is an analytic on Wh(Tr), then we represent B∈Cωh(Tr,g). It is said that the analytic g-valued functions B1,B2∈Cωh(Tr,g) are conjugated, if ∃ a L-P transformation G-valued function P∈Cωh(Tr,G), s.t. for the solutions Z1,Z2 corresponding to B1,B2, we have the following relation
and the conjugate relation can be denoted by:
It is easy to prove that B1≡B2(modP) can equivalently be written in the form of following equality
where Dω=∂∂φ⋅˙φ denotes the derivative in the direction of frequency vector ω. B1 is known to be reducible if it conjugates to a constant B2.
In our article, we shall prove that, for any λ∈Λ=(a,b), where λ is the parameter and Λ is a positive measure set, then ∃ a L-P transformation P(φ), such that the system A+Q(φ) is transformed into a constant system A∗.
For the positive measure reducibility, we will use the non-degeneracy conditions (or the transverse conditions as in Eliasson and Krikorian terminology). Without loss of generality, let's suppose a block-diagonal matrix A(λ)=diag(A1(λ),⋯,As(λ)) with
where σ(Ai) represents the eigenvalues set for Ai. Let (see in [12] for definition)
For the skew-product system (1.7), by using Lemmas 1.1 and 1.2 in [12], we set for ∀ ⟨k,ω⟩∈R
Remark: It is easily seen that if A∈Cω(Λ,g) and the division of σ(A) is sufficiently separated, then all gij are analytic functions of λ, ∀ 1≤i,j≤s.
For the proof of this remark (see in [17]).
Thus, we assume the following:
Non-degeneracy Conditions: There exist an integer d≥0 and ς≥0 such that
uniformly hold ∀ λ∈Λ and ⟨k,ω⟩∈R.
Remark: The condition (1.11) will assure that the small denominator condition always holds for the "most" parameter λ. Here, we take only those k in which |⟨k,ω⟩|≤2δ0, because for the large enough |⟨k,ω⟩|, we always see that the matrix i⟨k,ω⟩Ililj−(Ili⊗Aj(λ)−ATi(λ)⊗Ilj) is automatically non-singular and the small denominator condition is satisfied. It can easily be seen that the condition (1.11) is weaker than the non-degeneracy condition (1.3) used by Jorba and Simó.
Moreover, the property that gij(k,λ) depends analytically on λ can be preserved under small perturbations, and at each iterative step, we will preserve the non-degeneracy conditions.
1.1. Statement of the main result
To state the main result, consider Q as an analytic g-valued function that can be defined on a complex neighbourhood of Tr×Λ:
where λ∈Λ=(a,b). Defined the norm of Q as:
similarly
where ||⋅|| denotes the matrix norm.
Theorem 1.1. Consider the skew-product system (1.7) in which ω is a fixed Brjuno vector and it satisfies the Brjuno-Rüssmann condition (1.6) and A(λ) satisfies the non-degeneracy condition (1.11), and there exists K>0 such that ||A||≤K. Then there exist ε>0,h>0, such that if ||Q(⋅,⋅)||h=ε1<ε, the measure of the set of parameter λ′s for which the system (1.7) is non-reducible is no larger than CL(10ε1)c, with some positive constants C,c, and L denotes the length of the parameter interval Λ.
2.
Proof of the Theorem 1.1
Theorem 1.1 will be proven by KAM iteration. At each iterative step, we have a L-P transformation close to identity as
where Z(φ)∈Cωh(Tr,g), P(φ)∈Cωh(Tr,G) and by using the L-P transformation (2.1), the quasi-periodic system dxdt=(A+Q)x is changed into
Since Z is very small and in the expansion form P−1 can be written as:
So, we have
In general, we have to find a small Z in which the transformed system is still of the form dxdt=(A++Q+)x, where A+ is block-diagonal as A and Q+ is much smaller than Q.
To do this, we have to calculate Z solving the following linearized equation
where [A,Z]=AZ−ZA and to prove
is more smaller.
2.1. Solution of the linearized equation
In this subsection, we will solve the linearized equation, for this we need the following:
Definition: Let u=(u1,⋯,um)∈Tm. Its norm is denoted by ||u|| and is defined as:
Definition: For a m×m matrix S=(sij), its operator norm is denoted by ||S|| and is equivalent to m×max|sij|.
Notation: Let F∈Cωh(Tr×Λ,g) and its Fourier series is F=∑k∈ZrFkei⟨k,φ⟩, then the kth Fourier coefficients of F denoted by Fk, given by Fk=∫Tre−i⟨k,φ⟩F(φ)dφ.
Remark 2.1. For F∈Cωh(Tr×Λ,g), we have
Note. For k∈Zd, we denote |k|=d∑n=1|kn|. Similarly, for a function f, its modulus is denoted by |f|.
Throughout the discussion, to simplify notations, the letters c,C denote different positive constants.
By substituting the Fourier series expansions of Z,Q into the Eq (2.3), and then by equating the corresponding Fourier coefficients on both sides, we obtain
suppose that the eigenvalues of the linear operator i⟨k,ω⟩Id+[A,⋅] in the left part are
The eigenvalues will be αi−αj for k=0. As the considered matrix A=diag(A1,⋯,As) is a block-diagonal with different blocks Ai,Aj and each block have different eigenvalues, .i.e. αu≠βv if αu∈Ai,βv∈Aj for i≠j, from conclusions as seen from other researchers [12,17,18,19,20], we see that the matrix Ili⊗Aj−ATi⊗Ilj is non-singular if i≠j.
In block-diagonal form, let Qk can be written as (Qkij), where Qkij is a matrix of order li×lj, 1≤i,j≤s and li,lj are the orders of matrices Ai,Aj respectively.
Now, for k=0, we solve the equation (2.4). Suppose
and
For k=0, the equation (2.4) can be written as
Equation (2.5) can not be solved completely because the eigenvalues involved the multiplicity. However, the following equation
has a solution Z0=(Z0ij) with Z0ii=0 and
has the unique solution Z0ij.
Moreover, we have the estimate [12]
and
as dist(σ(Ai(λ)),σ(Aj(λ)))>ϱ>0, for i≠j. Moreover, we get
Now, we solve the Eq (2.4) for k≠0. From Lemma 3.2 as seen in [12], the solution of (2.4) is equivalent to the solution of the following vector equation
By using corollaries [12], Eq (2.8) is solvable ⟺ the matrix J(k,λ) is invertible. Suppose P=I+∑Zk is a L-P transformation. Then by using the L-P transformation, the new system becomes
where
Since A and Qd0 are block-diagonal matrices, therefore A+ is also a block-diagonal. Next, we will show that in a smaller domain Q+ is much smaller and the non-degeneracy condition is satisfied by A+.
Estimation of Q+.
First of all, we estimate Zk. Actually, to control the solution of Zk, we need the following small denominator condition, .i.e. if there exist N>0 such that ∀i,j
where Δ is an approximation function as defined above.
In order to estimate Zk, we need to estimate the operator J−1ij(k,λ) for k≠0.
Lemma 2.1. For k≠0 and the small denominator conditions (2.10) are satisfied by all parameters λ, then we have
where c denotes constant.
Proof. Since Jij is a non-singular matrix, so its inverse is defined as J−1ij=adJij/detJij. By the small denominator conditions (2.10), we have
and
using the definition of the norm ||Jij|| and the small denominator condition (2.10), the estimate (2.11)can be found easily. Also as detJ=∏1≤i,j≤sdetJij, similarly we can calculate the estimations (2.12) and (2.13).
For k≠0, from Eq (2.8), we have
as Z′k,Q′k are the transpose of Zk and Qk respectively, therefore it is easy to prove ||Zk||=||Z′k||,||Qk||=||Q′k|| (see in [12] for the proof).
In our article, we represent F(λ) a λ-dependent matrix as:
Since Q∈Cωh(Tr×Λ,g), then by the Remark 2.1, we have
As a result, for k≠0 and for any 0<ˉh<h, we have
or
In particular, take an approximation function Δ(t)=etδ,δ<1, which satisfy the Brjuno-Rüssmann condition (1.6), since the function etδ2rn2⋅e−t(h−ˉh) has the maximal value at t=(2rn2δh−ˉh)−1δ−1, one has
Consider
choose h′:0<h′<ˉh s.t. if ˉh−h′=h−h′<1. So, using the Lemma 4 in [7], we obtain
Let s=δ2−δ−1δ−1+m, and s′=δδ−1+m, we get
similarly, we can find
Hence, from Eq (2.9), we get
Verification of the non-degeneracy conditions for A+.
Since
Let
The new determinant D+ij is analytic with respect to λ as well.
The above determinant can be rewritten as
where Dij(k,λ)=det[i⟨k,ω⟩Ililj+(Ili⊗Aj(λ)−ATi(λ))⊗Ij)] and Yij(k,λ) is a summary of 2lilj−1 determinants denoted by yt(k,λ)(1≤t≤2lilj−1). Furthermore, there exist at least one column in each determinant yt such that the entries in this column are either 0 or of the form c−d, where c and d are entries of Q0jj and Q0ii respectively.
As |Qd0|h≤|Q|h<ε, we get
similarly,
So, we have
The proof is obvious. Note that, here we only need to choose such k′s so that |(k,λ)| is not large enough, .i.e., |(k,λ)|≤CK, where |A|≤K, because for large enough |(k,λ)|, the matrix J(k,λ) becomes automatically non-singular. So, when |(k,λ)| has large values, then J+(k,λ) becomes naturally non-singular and no need to preserve non-degenerate property.
Alternatively, we know from the perturbation theory of matrices that the continuous change of eigenvalues depends on the entries, and by Ostrowski theorem (see [21]), the distance between eigenvalues of any two blocks can be estimated as
Now, we summarize the above discussions in the following conclusion.
Conclusion 1.
Consider Λ subset of (a, b) be some parameter segment, a one parameter family of constant elements A∈Cω(Λ,g), and Q∈Cωh(Tr×Λ,g) be the perturbation. Suppose that there exist K,ε,N>0 s.t.
● |A|≤K,|Q|h<ε,
● for all λ∈Λ, the non-degeneracy conditions (1.11) and the small denominator conditions (2.10) hold.
Then, ∃h′>0 and a map Z∈Cωh′(Tr×Λ,g), and
such that
1) A+=A+Qd0,A++Q+≡A+Q
2) We have the estimation (2.19), .i.e. |Q+|h′≤CK2n4+1N2r+1n2[1(h−h′)2s+1−1(h−h′)2s′+1]|Q|2h.
3) We have preserved the non-degeneracy conditions.i.e., max0≤l≤r|∂l∂λlg+ij(k,λ)|≥ς′.
4) ϱ+>ϱ−cε1n,K+<K+ε.
2.2. Iteration
In this subsection, we will prove that the perturbation Q goes to zero very quickly provided that the small divisor conditions hold.
First of all, consider the following two iterative sequences:
where γ≥r is a constant, and η will be considered as in the following lemma
Lemma 2.2. There exist positive constants η<1,b, s.t., if ε1 is sufficiently small, then ∀m≥1
Proof. Suppose that if we do this up to mth step, we have
and
By induction, we need to prove that
and
Indeed Eq (2.25) is satisfied as
And from Eq (2.19), we have
To prove Eq (2.24), we need
Then by using Eqs (2.22) and (2.25), we have
Let Rm(η)=N2r+1n2mηb−1, if we choose
then by Eq (2.23) we see that for smaller value of η, the value of Rm also goes smaller. Now, firstly we set η=η0<1. As the sequence
is bounded from above, let's denote its maximum by ˉβ. In order to satisfy Eq (2.26), it is enough to choose η s.t.
Thus, define
and so we obtained the Eq (2.26). If we choose η=(10ε1)1/b, then it is enough to take
Hence, the proof of lemma is finished.
From Eq (2.18), it can be seen that the sequence |Zm|hm converges to 0 with super-exponential velocity, then by the transformation Pm=I+Zm, we have Pm→I, and so the composition of transformations Pm∘Pm−1∘⋯∘P1 will also be convergent. On the other hand, from conclusion 1, we have
so
for small enough ε1. Thus, the preservation of the non-degeneracy conditions is proved. By the way, for small enough ε1, we also have the estimate
2.3. Measure of the removed set
In this subsection, we will show that the set of parameters satisfying the small denominator conditions is of the large Lebesgue measure. In the end, we estimate the measure of the removed parameter set. At the mth step, for ∀i,j,1≤i,j≤s, we denote the removed set as:
and consider
To calculate the estimate for the measure of Rmkij, the following lemma is needed:
Lemma 2.3. Consider g(x) is a CM function on the closure ˉI, where I∈R1 is an interval of length L. Let Ih={x:|g(x)|≤h,h>0}. If for some constant r>0,|g(M)(x)|≥r for ∀x∈I, then |Ih|≤cLh1/M, where |Ih| denotes the Lebesgue measure of Ih and constant c=2(2+3+⋯+M+r−1).
For the proof, see [22].
Then, let L denotes the length of the parameter interval Λ, and using above Lemma 2.3, we obtain
where c=2(2+3+⋯+r+2/ς), as gmij(k,λ)∈Cm(Λ) and using the non-degeneracy conditions and Eq (2.30). Thus,
For Δ(|k|)=e|k|δ,δ<1, we have
By Eq (2.23), Nm>2mγηγ, we have
Therefore, for η=(10ε1)1b and γ≥r, one has
Hence, the proof of the main result is completed.
3.
Conclusions
In this article, we discussed the positive measure reducibility for quasi-periodic linear systems and proved that the system (1.7) is reduced to a constant coefficient system. The result was proved for a Brjuno vector ω and small parameter λ by using the KAM method, Brjuno-Rüssmann condition and non-degeneracy condition.
Acknowledgments
The authors extend their appreciation to the Yibin University, Yibin, China.
Conflict of interest
The authors declare no conflicts of interest in this paper.















 DownLoad:
DownLoad: