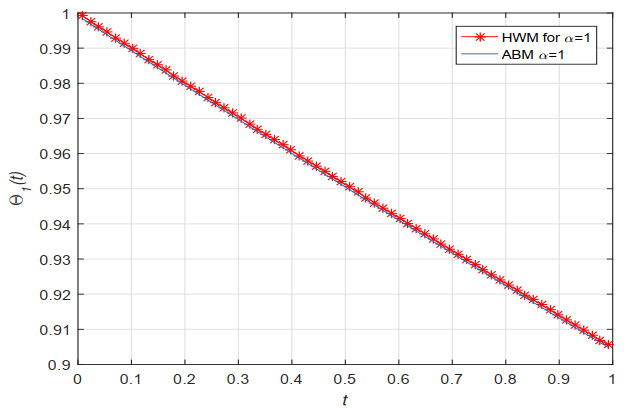
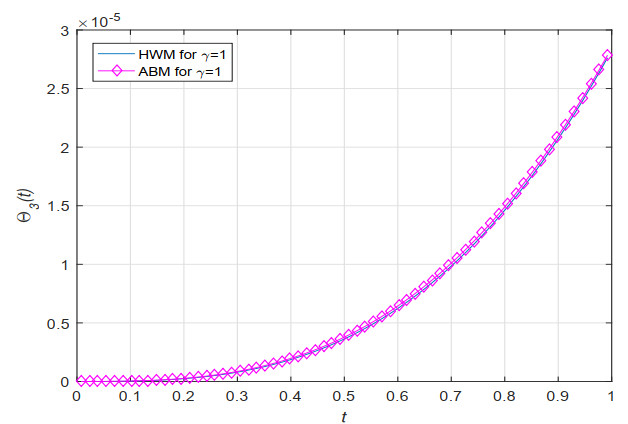
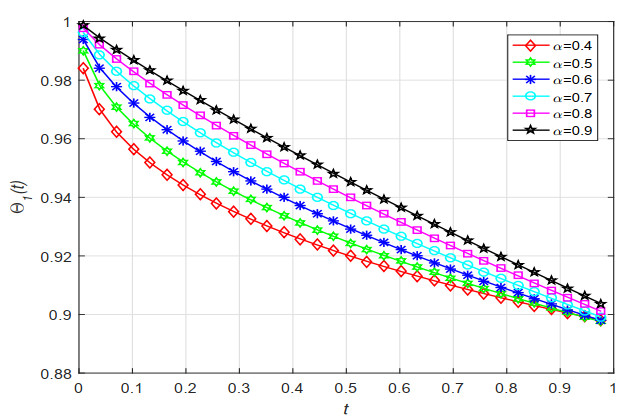
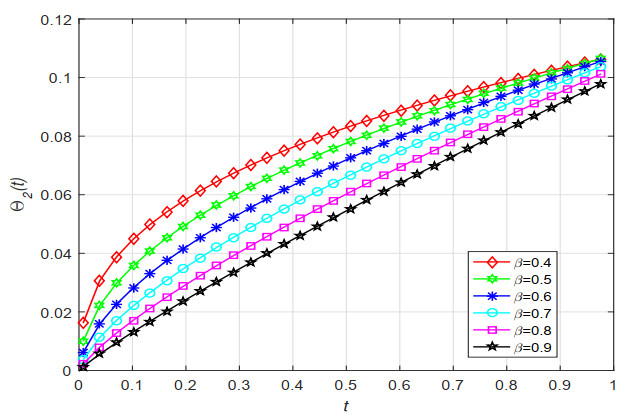
The essential objective of this work is to implement Adam Bashforth's Moulton (ABM) and Haar wavelet method (HWM) to solve fractional chemical kinetics and another problem that relates the condensations of carbon dioxide (CO2) and phenyl glycidyl ether (PGE) with two variety of Drichlet and a mixed set of Neumann boundary and Drichlet type conditions respectively. We have been solved the above system of differential equations by Adam Bashforth's Moulton and Haar wavelet operational method where this technique is to convert the system of differential equations into the system of algebraic equation which can be solved easily. This work is expects to contribute the vast advantage of Haar wavelets in chemical science. The Adam Bashforth's Moulton and Haar wavelet method is impressive and convenient for obtaining numerical solutions of chemical engineering type problems. A complete agreement is acheived between Adam Bashforth's Moulton solution and Haar wavelet solution. To manifest about the performance and applicability of the method, two test examples are deliberated.
1.
Introduction
In the theory of fixed points, there are interesting strategies to approach the related problems, in order to provide adequate answers to the questions implied by it. An almost universal example is the Banach contraction principle, which can be proved by working mainly with inequalities between distances, empowered by the triangle inequality. Remarkable progress in this field was made by encapsulating properties in relations defined by suitable mappings that preserve characteristics which are needed in the study of the existence and uniqueness of fixed points. For instance, this is the case of φ-contractions, where φ is a comparison function. An exhaustive study of them can be found in the monograph by Berinde [1]. Samreen et al. [2] developed fixed point outcomes related to extended b-comparison functions. In their paper, Karapınar et al. [3] developed new techniques to prove the existence and uniqueness of fixed points for operators defined by means of inequalities involving comparison type functions. Their work has been extended in [4], where Dumitrescu and Pitea studied almost (φ,θ)-contractions, given by an inequality that unifies the properties of comparison functions with θ-mappings. Both papers are made in the settings of Jleli-Samet generalized metric spaces. Over the last years, such types of new contractive conditions have led to the emergence of new interesting problems in literature. In 2015, Alsulami et al. [5] developed the notion of (α,ψ)-rational type contraction, which is a mixture of mappings with different properties, connected by an inequality condition. Their study was made in the context of generalized metric spaces, where the third axiom is an extension of the classical one by the addition of a new term, and therefore the triangle-type inequality plays a crucial role in proving some existence results for fixed points. Such extensions of the usual metric are widely used in literature. For instance, b-metric spaces by Bakhtin [6] and Czerwik [7], extended b-metric spaces by Kamran et al. [8], or modular spaces having Fatou's property by Hitzler and Seda [9], are examples of generalized metrics defined by changing the triangle inequality in some different ways. Various contractive conditions have been developed in these spaces, such as that used to solve Volterra integral inclusion by Ali et al. [10], to point out some nonlinear contractions related to common fixed point properties in Shatanawi [11], or some rational type inequalities in modular metric spaces in Okeke et al. [12]. It can be mentioned that a recent approach on Hill's equation was made in the context of convex modular spaces by Nowakowski and Plebaniak [13]. Aslantas et al. [14] introduced the concept of strong Mb-metrics and proved some Caristi type fixed point theorems. The context of M-metrics was used by Aslantas et al. [15] in order to prove results regarding mixed multivalued mappings. Another idea of extending classical metrics can be found in Arutyunov and Greshnov [16], and in Greshnov and Potapov [17], where the authors study the (q1,q2)-quasimetric spaces (which also include b-metrics). The interesting fact about these spaces is the absence of the symmetry axiom. The same idea of modifying the triangle inequality can be observed in the case of Jleli-Samet generalized metric spaces [18] where the third axiom is a limit-type inequality. In this setting, Altun and Samet [19] proved existence results for pseudo Picard operators, and Karapınar et al. [20] developed Meir-Keeler type theorems. Senapati et al. [21] explored implicit type contractive conditions in the same background. Another step in the study of new suitable weak contractions was achieved by Wu and Zhao in [22], where they introduced the (α,ψ)-rational type contractions in the setting of b-metrics. The importance of the rational contractions led to extensions of the theory in spaces which possess different structures compared with the classical ones. For instance, in [23], Thounaojam et al. developed a concise theory of such rational operators in the setting of general parametric metrics, which are spaces equipped with metric-type mappings depending on positive parameters.
The aim of this paper is to extend ideas in the context of Jleli and Samet generalized metrics, discovered and studied by the authors in their work [18]. Through a combination of technical ideas and an adequate methodology, fixed point results will be proved by taking into account the restrictions imposed by working without the benefits of any kind of triangle inequality. Our approach is different than that in [5], where the authors use a generalized metric in which a quadrilateral inequality holds.
The structure of this paper will be as follows: first, some basic definitions related to JS-spaces will be recalled in Section 2. In Section 3, (α,ψ)-rational contractions are introduced on our working space, and several fixed point theorems are proved, referring to the existence and uniqueness of such points, related to these classes of rational contractive type operators. Examples and comments unify our approach.
2.
Preliminaries
The framework chosen here is one that generalizes in a great manner the triangle axiom of the classic metric spaces. In 2015, Jleli and Samet [18] came up with a version of the Banach principle in the context of a new type of metrics, which strictly include those of b-metric spaces, dislocated metric spaces and modular metric spaces with suitable properties. The consistent change that comes with this definition is the third condition, which is more general than the triangle inequality.
In the following paragraphs, we recall some important tools from study [18].
Definition 2.1 ([18]). Let us consider the arbitrary set X≠∅ and let D:X×X→[0,∞] be a mapping.
We say that D is a JS−metric on X if the following axioms are satisfied:
(D1) For all x, y∈X, the next implication holds true
(D2) For every x, y∈X, the symmetry condition D(x,y)=D(y,x) is satisfied;
(D3) There is a constant C>0 such that for every x, y∈X, and for each sequence {xn} which converges to x (that is limn→∞D(xn,x)=0), the following inequality is valid
In our study, the pair (X,D) will denote a Jleli-Samet metric space (or a JS-space).
Note that, if we consider a classic metric space (X,d), the third axiom of JS-metric spaces is accomplished because of the continuity of d, so any metric space is a JS space.
As in the classical case, we say that a sequence {xn} in X is convergent to x∈X if
Moreover, it is immediate that the limit of a convergent sequence is unique.
It must be said that if (X,D) does not possess convergent sequences, then the third axiom is automatically fulfilled.
Example 2.1. Let us take X={0,1,2} equipped with the function D:X×X→[0,∞] defined as follows:
This is a proper example of a Jleli-Samet which is not contained in any of the classes of spaces mentioned above. To justify this, the first two requirements of the definition are apparent. In one take x∈X and a sequence {xn} which converges to x, it follows that there is an index n0∈N such that D(xn,x)<1, for all n≥n0. Looking at the presentation of D, we conclude that convergent sequences in this case are stationary at 0 from a given rank. By this observation, the third axiom is accomplished with C=1.
Other topological characteristics can be enumerated, as follows.
Definition 2.2. ([18]). Let (X,D) be a JS-metric space and {xn} be a sequence in X. It will be said that {xn} is a D-Cauchy sequence if
This means that for every ε>0, there is N∈N such that D(xn,xm)<ε wherever n,m≥N.
A JS-space (X,D) having all D-Cauchy sequences being D-convergent to an element in X is called D-complete. For a concise presentation of more topological tools, we invite the reader to consult [24]. To be able to work properly with JS metrics, the next notations will be useful. Define
where n0∈N is an index from which we study the values of the metric, and
Denote the orbit of an element ˜x by an operator T:X→X as
Classes of (α,ψ)-rational contractions will be provided for the setting of Jleli and Samet.
First of all, let us present the properties which feature the functions α and ψ.
Definition 2.3 ([5]). Let us consider X≠∅, and the map α:X×X→[0,∞). An operator T:X→X is α-admissible if α(x,y)≥1 imply that α(Tx,Ty)≥1, for all x, y∈X.
Definition 2.4 ([22]). Let us consider X≠∅ and the map α:X×X→[0,∞). We say that T:X→X is triangular α-admissible if α(x,y)≥1 implies that α(Tx,Ty)≥1, and α(x,y)≥1,α(y,z)≥1 implies that α(x,z)≥1 for all x, y, z∈X.
Definition 2.5 ([5]). Let (X,D) be a Jleli-Samet space and α:X×X→[0,∞). X is called JS α-regular if for {xn} convergent to x and α(xn,xn+1)≥1, there is a subsequence of the initial sequence such that α(xnk,x)≥1, for all k∈N.
Let us denote by Ψ the family of functions ψ:[0,∞)→[0,∞) such that the following properties are accomplished:
i) ψ is upper semi-continuous and strictly increasing;
ii) {ψn(t)} converges to 0, for all t>0.
Remark 2.1. As known [1], functions which fulfill the strictly monotone property and condition ii) form the class of comparison functions, that are widely used in the literature for defining different types of contractive operators. Among their properties, we emphasize that ψ(t)<t, for all t>0, and ψ(0)=0.
Some well known examples of functions from the set Ψ are given by:
ⅰ) ψ(t)=at, for all t∈[0,∞), where a∈[0,1);
ⅱ) ψ(t)=t1+t, for every t∈[0,∞).
3.
Main results
We can now study classes of rational contractions involving the above properties. The following theorems provide the existence and uniqueness of fixed points for specific operators defined by means of rational-type contractions.
As the term M(x,y) changes its form in the right hand side of the contractive inequality, new interesting problems arise. In some cases, it suffices to suppose that the space X is α-regular, while, in other cases, the continuity of the main operator is needed. It can be observed that the form of the maximum quantity plays a crucial role in our study.
An important observation should be made. In the next theorems, we assume that the fixed points of the studied operator also satisfy the contractive inequality imposed in the hypotheses of the underlying theorems.
Theorem 1. Let (X,D) be a complete Jleli-Samet metric space, T:X→X be an operator and α:X×X→[0,∞) be a given mapping. Suppose that the following conditions are fulfilled:
i) T is a triangular α-admissible mapping;
ii) there is x0∈X with δ(D,T,x0)<∞ such that α(x0,Tx0)≥1;
iii) there is a function ψ∈Ψ such that:
where
for all x, y∈O′T(x0)=OT(x0)∪{ω∈X:limn→∞D(Tnx0,ω)=0};
iv) X is α-regular;
v) D(Tnx0,Tnx0)=0, for all n∈N.
Then the sequence {Txn} is convergent to a point x∗∈X. If D(x∗,Tx∗)<∞, then x∗ is a fixed point of T. In addition, if there is another fixed point of T, denoted by y∗, with D(y∗,y∗)<∞, α(x∗,y∗)≥1 and D(x∗,y∗)<∞, then x∗=y∗.
Proof. Let us take x0∈X fulfilling the second item from the above theorem, and denote {xn=Tnx0} the Picard sequence, where n∈N.
Considering the situation in which xp=xp+1 for some p∈N, it can be observed that xp is the fixed point of T.
Let us take the case when xn≠xn+1, for all n∈N.
We are going to show that limn→∞D(xn,xn+1)=0, by taking advantage of the properties of the mappings α and ψ.
Knowing the fact that T is α-admissible, α(x0,Tx0)≥1 implies that α(Tx0,Tx1)=α(x1,x2)≥1. Using the mathematical induction, one can prove that α(xn,xn+1)≥1, for all n∈N.
If we look at the contractive relation, we get:
given that
If there exists t0∈N such that M(xt0,xt0+1)=D(xt0,Txt0)D(xt0+1,Txt0+1)1+D(xt0,xt0+1), using the contractive inequality and the fact that D(xt0,Txt0)D(xt0+1,Txt0+1)1+D(xt0,xt0+1)<D(xt0+1,xt0+2), one can say that:
which is a contradiction, as we have assumed that xn≠xn+1, for all n.
As a consequence, for all n∈N, we have M(xn,xn+1)=D(xn,xn+1), which gives us:
Taking into account the properties of ψ, we obtain that D(xn+1,xn+2)≤ψn+1(D(x0, x1)), for all n∈N. Therefore, it is true that limn→∞D(xn, xn+1)=0.
From the triangular α-admissibility, it follows that α(xm, xn)≥1, for all m, n∈N with n>m.
Denote by k0 the smallest rank n for which D(xn,xn+1)<1. As the sequence {D(xn,xn+1)} is non-increasing, we get that D(xn,xn+1)<1, for all n≥k0.
The contractive inequality implies, for n>m≥k>k0, that:
for n>m≥k>k0, where
The contractive inequality shows that:
Passing through the supremum in the above relation, and having in mind the symmetry of D and hypothesis v), it follows that:
Using the fact that {δk(D,T,x0)} is a non-increasing sequence of positive numbers, there exists L∈[0,∞) satisfying limk→∞δk(D,T,x0)=L. Taking into account that ψ is upper semi-continuous and passing through the limit over k, we have L≤ψ(L). Therefore, L=0 and, consequently, limm,n→∞D(xm,xn)=0.
Now, since (X,D) is supposed to be JS complete, one can find x∗∈X such that {xn} is convergent to x∗.
Since X is α-regular, there will be a subsequence {xnk} of {xn} for which α(xnk,x∗)≥1, for all k∈N. Writing again the contractive relation, we find out that:
where
Combining the properties of the above sequences, there is N∈N such that D(xnk,x∗)<ε, D(xnk,xnk+1)<ε, and D(xnk,xnk+1)D(x∗,Tx∗)<ε, for all k≥N, where ε>0 is arbitrary chosen. It implies that for all ε>0, there is an index nε=N, with M(xnk,x∗)<ε, for all k≥N, which leads to D(xnk+1,Tx∗)<ε, for all k≥N. It follows that we have limk→∞D(xnk+1,Tx∗)=0. Therefore, using the property (D3), we get:
so Tx∗=x∗. Note that D(x∗,x∗)=0.
Finally, let us take y∗ a different fixed point of T endowed with the properties mentioned in the hypotheses. By the fact that α(x∗,y∗)≥1, we have to look at the contractive property one more time:
where
By the properties of ψ, we can conclude that D(x∗,y∗)=0 and x∗=y∗. □
In the next theorem, we weaken the contractive condition by adding an additional term in the right hand side, and for this reason we need another condition instead of that in ⅳ).
Theorem 2. Let (X,D) be a complete Jleli-Samet metric space, T:X→X be an operator and α:X×X→[0,∞) be a given mapping. Suppose that the following items are accomplished:
i) T is a triangular α-admissible mapping;
ii) there is x0∈X with δ(D,T,x0)<∞ such that α(x0,Tx0)≥1;
iii) there is a mapping ψ∈Ψ such that:
where
for all x, y∈OT(x0);
iv) T is continuous;
v) D(Tnx0,Tnx0)=0, for all n∈N.
Then T has a fixed point x∗∈X and the Picard sequence {Tnx0} goes to x∗. In addition, if there is another fixed point of T, denoted by y∗, with D(y∗,y∗)=0, α(x∗,y∗)≥1 and D(x∗,y∗)<∞, then x∗=y∗.
Proof. Let x0∈X satisfying the second condition from the theorem, and {xn=Tnx0}, where n∈N.
The case when xd=xd+1 for some d∈N, is clear and xd will be fixed point of T.
Let us suppose that xn≠xn+1, for all n∈N.
We will prove that limn→∞D(xn,xn+1)=0, by means of the properties of the mappings α and ψ. Taking into account that T is α-admissible, α(x0,Tx0)≥1 implies α(Tx0,Tx1)=α(x1,x2)≥1. By an inductive argument, we get α(xn,xn+1)≥1, for all n∈N.
Now, if one uses the contractive inequality, we obtain:
given that
If there exists p0∈N, M(xp0,xp0+1)=D(xp0+1,xp0+2), using the contractive inequality, one can say that:
which is a contradiction. Therefore, for all n∈N, we have M(xn,xn+1)=D(xn,xn+1), which leads us to:
Using the properties of ψ, we conclude that D(xn+1,xn+2)≤ψn+1(D(x0, x1)), for all n∈N. It means that limn→∞D(xn,xn+1)=0.
By the triangular α-admissibility, it follows that α(xm,xn)≥1, for all m, n∈N with n>m.
Denote by k0 the smallest index n for which D(xn,xn+1)<1. Since {D(xn,xn+1)} is a non-increasing sequence, it follows that D(xn,xn+1)<1, for all n≥k0.
The contractive inequality becomes:
for n>m≥k>k0, where
The contractive inequality provides:
We have, keeping in mind the symmetry of D and condition ⅴ), after passing through the supremum in the above relation, that:
Taking into account that {δk(D,T,x0)} is a non-increasing sequence of positive numbers, there is L∈[0,∞) such that limk→∞δk(D,T,x0)=L. Using the fact that ψ is upper semi-continuous and passing through the limit over k, we have L≤ψ(L). As a consequence, L=0 and limm,n→∞D(xm,xn)=0.
Now, since (X,D) is supposed to be JS complete, there will be x∗∈X such that {xn} is convergent to x∗.
Knowing that T is continuous, it can be observed that
By the property (D3), there is C>0 such that:
From D(x∗,Tx∗)=0, it follows Tx∗=x∗ and x∗ is indeed a fixed point of T.
Last but not least, let us suppose that y∗ is another fixed points of T, which fulfills the conditions of the theorem. By the fact that α(x∗,y∗)≥1, we can use the contractive property one more time:
where
It follows that D(x∗,y∗)<ψ(D(x∗,y∗)), and, by the properties of ψ, we can conclude that D(x∗,y∗)=0 and x∗=y∗. □
We present in the next paragraphs two concrete examples to illustrate the applicability of our results.
Example 3.1. Let us consider the set A={0,1,2,…,N}, where N≥2, endowed with the Jleli-Samet metric D:A×A→[0.∞] defined in the following way:
The first and the second axiom from the definition of the Jleli Samet spaces are fulfilled. In addition, it can be observed that a sequence {xn} is convergent in D if and only if {xn} is stationary from some rank; more precisely, it means that xn=k, for all n≥n0 where n0∈N and k∈A∖{N}. Therefore, the inequality from the third axiom becomes equality with C=1. As a consequence, (A,D) is a Jleli-Samet space.
If one takes {yn} to be a D-Cauchy sequence, there will be some index n1∈N such that D(yn,ym)<13, for all n≥m≥n1. It results that D(yn,ym)=0, for all n≥m≥n1. Thus we obtain yn=yM, for all n≥n1, where yM∈A∖{N}. Therefore, a sequence is convergent if and only if it is a D-Cauchy sequence, so (A,D) is a complete Jleli-Samet space.
Let us take T:A→A given by T(0)=T(1)=⋯=T(N−1)=1 and T(N)=N−1. Consider then ψ:[0,∞)→[0,∞), ψ(t)=23t and α:A×A→[0,∞) with α(x,y)=1, for all x, y∈A.
If x, y∈{0,1,…,N−1}, the contractive inequality is obviously fulfilled.
If x∈{0,1,…,N−1} and y=N we have:
which holds true, since N≥2.
If x=y=N the contractive relation is automatically accomplished. It is clear that all conditions of Theorem 1 are satisfied, and the fixed point is x∗=1.
The following example shows the case when the Jleli-Samet space is a real interval and the operator T is not contractive.
Example 3.2. Take X=[0,∞) equipped with the Euclidean distance D(x,y)=|x−y|. In this case, (X,D) is a complete Jleli-Samet space, as a particular case of a complete metric space. One can define
and
Taking α(x,y)=1, for all x, y∈X, it can be shown that T is an (α,ψ)-rational contraction. Indeed, the following inequality is true, for all x, y∈X:
It is immediate that all requirements from the above theorem are established, so there is a fixed point of T, x∗=0, which comes as a consequence of our result.
By changing the form of the term M(x,y), we obtain other interesting fixed point results in this setting.
For such a class of operators, there is another theorem of existence and uniqueness that can be proved.
Theorem 3. Let (X,D) be a complete JS space, T:X→X be an operator and α:X×X→[0,∞) be a given function. Suppose that the following constraints are satisfied:
i) T is a triangular α-admissible mapping;
ii) there is x0∈X with δ(D,T,x0)<∞ such that α(x0,Tx0)≥1;
iii) there is a mapping ψ∈Ψ such that:
where
for all x, y∈O′T(x0);
iv) X is α-regular;
v) D(Tnx0,Tnx0)=0 for all n∈N.
Then {Tnx0} is convergent to x∗. If D(x∗,Tx∗)<∞, then x∗ is a fixed point of T. Moreover, if there is another fixed point of T, denoted by y∗ with D(y∗,y∗)<∞, α(x∗,y∗)≥1 and D(x∗,y∗)<∞, then x∗=y∗.
Proof. In order to keep all details clear, we use the same notations as in the previous proofs. Also, without loss of generality, assume that D(xn,xn+1)≠0, for all n.
Firstly, it can be shown by induction that α(xn,xn+1)≥1 for all n∈N. If we use the contractive relation, precious information about the sequence {D(xn,xn+1)} is found. For instance:
knowing that
It follows that
Taking advantage of the properties of ψ, we state that D(xn+1,xn+2)≤ψn+1(D(x0, x1)), for all n∈N. It means that limn→∞D(xn,xn+1)=0.
Let us show that the Picard sequence is D-Cauchy. The property that α(xm,xn)≥1 for all m,n∈N with n>m follows naturally.
Denote by k0 the smallest positive integer n such that D(xn,xn+1)<1. The monotone of {D(xn,xn+1)} implies that D(xn,xn+1)<1, for all n≥k0.
We use the contractive relation for n>m≥k>k0, where k∈N, to obtain the following relation:
taking into account that
It is useful to see that M(xm−1,xn−1)≤δk−1(D,T,x0) for all n>m≥k>k0. We can say that:
Having in mind the hypotheses, if we take the supremum, it follows that
Recall the fact that {δk(D,T,x0)} is a non-increasing sequence of positive numbers, so there is L∈[0,∞) such that limk→∞δk(D,T,x0)=L. Passing through the limit over k in the above relation and using the properties of ψ, we conclude that L=0. The Picard sequence is D-Cauchy. From the fact that (X,D) is supposed to be JS complete, there will be x∗∈X such that {xn} is convergent to x∗.
Since X is α-regular, there exists a subsequence {xnk} of {xn} for which α(xnk,x∗)≥1, for all k∈N. Writing carefully the contractive relation, we find out:
where
From the properties of {D(xn,xn+1)} and hypothesis ii), it is clear that limk→∞M(xnk,x∗) =0 and, using the property (D3) from the axioms of JS spaces, we get D(x∗,Tx∗)=0. In conclusion, we can say that Tx∗=x∗.
For the last part of the proof, let us suppose that x∗ and y∗ are two different fixed points of T. By the fact that α(x∗,y∗)≥1, we can use the contractive property to obtain:
where the quantity
By the properties of ψ, we can conclude that D(x∗,y∗)=0 and x∗=y∗. □
Another result can be established if one changes the structure of the rational functions.
Theorem 4. Let (X,D) be a complete JS space, T:X→X be a self mapping and α:X×X→[0,∞) be a given function. Suppose that the following items are satisfied:
i) T is a triangular α-admissible mapping;
ii) there is x0∈X with δ(D,T,x0)<∞ such that α(x0,Tx0)≥1;
iii) there is a mapping ψ∈Ψ such that:
where
for all x, y∈OT(x0);
iv) T is continuous;
v) D(Tnx0,Tnx0)=0 for all n∈N.
Then T has a fixed point x∗∈X and the Picard sequence {Tnx0} has the limit x∗. Moreover, if there is another fixed point of T, denoted by y∗ with D(y∗,y∗)=0, α(x∗,y∗)≥1 and D(x∗,y∗)<∞, then x∗=y∗.
Proof. The first part of the proof can be done following the proof of Theorem 2. The presence of the third term in the right-hand side part of the contractive inequality does not affect the fact that limn→∞D(xn,xn+1)=0.
As in the previous proofs, let us take k0 as the smallest rank n such that D(xn,xn+1)<1. From the monotone of {D(xn,xn+1)}, it follows that D(xn,xn+1)<1, for all n≥k0.
Working with the new form of the contractive relation, for n>m≥k>k0, where k∈N, we get:
knowing the fact that
We have M(xm−1,xn−1)≤δk−1(D,T,x0), for all n>m≥k>k0. It follows that:
Passing through the supremum and considering the hypotheses, it can be said that
Taking into account that {δk(D,T,x0)} is a non-increasing sequence of positive numbers, there will be L∈[0,∞) such that limk→∞δk(D,T,x0)=L.
Taking the limit over k in the above inequality and working with the properties of ψ, we can say that L=0. The Picard sequence is proved to be D-Cauchy. From the statement of the theorem (X,D) is supposed to be JS complete, so there is x∗∈X such that {xn} converges to x∗.
From the fourth condition of the theorem, T is continuous, so it follows that
By the axiom (D3), there is C>0 such that:
Since D(x∗,Tx∗)=0, we get Tx∗=x∗ and x∗ is a fixed point of T.
Ultimately, let us consider x∗ and y∗ two different fixed points of T. Being aware that α(x∗,y∗)≥1, the contractive relation turns into:
where
Eventually, looking again of the properties of ψ, we proved that D(x∗,y∗)=0 and x∗=y∗. □
Example 3.3. Let us consider X={0,1,2} endowed with the metric D:X×X→[0,∞) defined by:
One can prove that (X,D) is a complete Jleli-Samet space.
Next, let us define T:X→X in the following way:
If we take ψ(t)=2t3, it can be easily checked that all conditions from the above theorem are fulfilled, and T has a fixed point.
There is another interesting combination of rational terms in the maximum quantity. For this class of operators, it can be proved one more theorem of existence and uniqueness which completes our study. Additionally, if the space X has a particular value of C in the third axiom, then the statement of the theorem remains true.
Theorem 5. Let (X,D) be a complete JS space, T:X→X be an operator and α:X×X→[0,∞) be a given function. Suppose that the following constraints are satisfied:
i) T is a triangular α-admissible operator;
ii) there is x0∈X with δ(D,T,x0)<∞ such that α(x0,Tx0)≥1;
iii) there is a mapping ψ∈Ψ such that:
where
for all x, y∈O′T(x0);
iv) either T is continuous, or X is α-regular with C=1 and D(u,Tu)<∞, where u is any accumulation point of X;
v) D(Tnx0,Tnx0)=0, for all n∈N.
Then T has a fixed point x∗∈X and the Picard sequence {Tnx0} has the limit x∗. Moreover, if there is another fixed point of T, denoted by y∗ with D(y∗,y∗)=0, α(x∗,y∗)≥1 and D(x∗,y∗)<∞, then x∗=y∗.
Proof. In order to be concise, we are going to present the main ideas from the proof.
First of all, in order to prove that limn→∞D(xn,xn+1)=0 we need to look at the new contractive relation:
having in mind that
As we have done before, it will follow that M(xn,xn+1)=D(xn,xn+1), so, by the properties of ψ, we conclude limn→∞D(xn,xn+1)=0.
The Picard sequence is D-Cauchy for the following reasons.
First, let us consider k0 the smallest rank n such that D(xn,xn+1)<1, so D(xn,xn+1)<1, for all n≥k0.
Looking at the contractive inequality, for n>m≥k>k0, where k∈N, we see that:
having in mind that
Second, one must note that M(xm−1,xn−1)≤δk−1(D,T,x0) for all n>m≥k>k0. Combining all the information about the sequence {δk(D,T,x0)} and the mappings involved, we can say that {xn} is D-Cauchy.
If T is continuous, it is immediate that x∗∈X (such that {xn} converges to x∗) is a fixed point of the operator.
In the case when X is α-regular one can find a subsequence {xnk} of {xn} such that α(xnk,x∗)≥1, for all k∈N. Writing down the contractive inequality, we note that:
where
Assume that D(x∗,Tx∗)≠0. There is an ε∈(0,1) and ˜k∈N such that D(xnk,x∗)<ε and D(xnk,xnk+1)<ε for all k≥˜k, with D(x∗,Tx∗)>ε. It is clear that M(xnk,x∗)=D(x∗,Tx∗) for all k≥˜k. By consequence, it follows that limk→∞M(xnk,x∗)=D(x∗,Tx∗). From the statement, we know that C=1. If we write again the axiom (D3) it is immediate that:
Therefore, Tx∗=x∗. The case when D(x∗,Tx∗)=0 is trivial and the conclusion is obvious.
The last part of the proof, regarding the uniqueness, follows directly and the theorem is completely proved. □
4.
Comments and further studies
In this work, we provide new results in fixed point theory by means of new contractive operators in the setting of Jleli-Samet generalized metric spaces. We have used rational type contractive conditions defined also by the use of comparison functions endowed with an adequate type of continuity. Existence and uniqueness results regarding these (α,ψ)-rational contractions have been proved. Some examples sustained the usability of our results.
As a direction for further studies, our results may be extended in the case of ordered Jleli-Samet metric spaces. Additionally, inspired by [25], we intend to start a research of weakly K-nonexpansive mappings in this setting. Also, we have in view the development of some applications regarding periodic boundary value problems in JS metric spaces, having [26] as a starting point for this purpose, and other possible applications in engineering, following [27].
Conflict of interest
The authors declare that they have no conflicts of interest.















 DownLoad:
DownLoad: