New solutions for the unstable nonlinear Schrödinger equation arising in natural science

1.   Introduction

In recent years, nonlinear partial differential equations (NPDEs) are widely used to describe complex phenomena in various fields of sciences, such as fluid mechanics, plasma, chemical reactions, optical fibers, solid state physics, relativity, ecology, gas dynamics physics and optical fiber, ^{[1,2,3,4,5,6,7,8,9,10,11]}. Therefore, exploring exact solutions for NPDEs plays an important role in nonlinear science. These solutions might be essential and important for the exploring some physical phenomena. Therefore investigating new technique to solve so many problems is so interesting topic. Thus, many new methods have been introduced, such as the F-expansion method ^[12,13], $(\frac{G^{'}}{G})-$ expansion method ^[14,15], tanh-sech method ^[16,17,18], exp-expansion method ^[19,20], the homogeneous balance method ^[21,22], Jacobi elliptic function method ^[23,24], sine-cosine method ^[25,26,27], extended tanh-method ^[28,29] and the Riccati-Bernoulli sub-ODE method ^{[30,31,32,33]} proposed for solving more complicated problems. Indeed, there are recent development in analytical methods for investigation solutions for NPDEs, see ^{[34,35,36,37,38,39,40]}.

The nonlinear Schrödinger equations (NLSEs) are so important models in nonlinear evolution equations, which come in many areas of applied sciences such as nonlinear optics, quantum mechanics, fluid dynamics, molecular biology, elastic media, hydrodynamics, biology and plasma physics.

This paper is concerned with the unstable nonlinear Schrödinger equation (UNS) ^[41,42] given by

where, $\eta, \gamma$ is a free parameter and $q = q(x, t)$ is a complex-valued function. Equation (1.1) is a type of nonlinear Schrödinger equation with space and time exchanged. This equation prescribes a time evolution of disturbances in unstable media. The behavior of type occurs for the two-layer baroclinic instability and the lossless symmetric two-stream plasma instability ^[43]. To the best of our knowledge, no previous research work has been done using the proposed methods for solving the unstable nonlinear Schrödinger equation. Actually, many numerical and analytical methods have been also implemented to get solutions for Eq (1.1) such as modified Kudraysov method, the sine-Gordon expansion approach ^[41], $exp_{a}$ method and hyperbolic function method ^[42], the new Jacobi elliptic function rational expansion method and the exponential rational function method ^[44], the extended simple equation method^[45].

The main aim of this paper is to explore the UNS equation using exp$(-\varphi(\xi))$-expansion method, sine-cosine method and Riccati-Bernoulli sub-ODE method. We also show that the Riccati-Bernoulli sub-ODE technique gives infinite solutions. Actually, we introduce new types of exact analytical solutions. Comparing our results with other results, one can see that our results are new and most extensive. Indeed the new solutions presented in this article are so important in the theory of soliton. Moreover these solutions turn out to be very useful for Physicists to explain many interesting physical phenomena.

The rest of the paper is arranged as follows: In Section 2, the exp-function method, sine-cosine method and Riccati-Bernoulli sub-ODE method are briefly reviewed. In Section 3, some new exact solutions of the unstable Schrödinger equation are presented. Discussion of our results and comparing with the results of other authors is in Section 4. Conclusion and future works will appear in Section 5.

2.   Description of methodologies

We present a brief description about the exp$(-\varphi(\xi))$-expansion method, sine-cosine method and Riccati-Bernoulli sub-ODE method to obtain new exact solutions for a given NPDE. For this goal, consider a NPDE in two independent variables $x$ and $t$ as

where $G$ is a polynomial in $\vartheta(x, t)$ and its partial derivatives. The main steps are as follows ^[30]:

Step 1. Introducing the transformation

varies Eq (2.1) to the following ordinary differential equation (ODE):

where $D$ is a polynomial in $\vartheta(\xi)$ and its derivatives such that the superscripts denote the ordinary derivatives with respect to $\xi$.

2.1.   The exp$(-\varphi(\xi))$-expansion method

According to the exp$(-\varphi(\xi))$-expansion technique ^[19,20,31], we assume that the solution of Eq (2.3) can be written in a polynomial form of $exp(-\varphi(\xi))$ as follows

where $\varphi(\xi)$ obeys the following ODE

Eq (2.5) has the following solutions:

1. At $\lambda^{2}-4\nu > 0, \nu\neq0,$

2. At $\lambda^{2}-4\nu < 0, \nu\neq0,$

3. At $\lambda^{2}-4\nu > 0, \nu = 0, \lambda \neq0$

4. At $\lambda^{2}-4\nu = 0, \nu\neq0, \lambda\neq0,$

5. At $\lambda^{2}-4\nu = 0, \nu = 0, \lambda = 0,$

Here $C$ is an arbitrary constant.

Finally, superseding Eq (2.4) with Eq (2.5) into Eq (2.3) and agammaegating all terms of the same power $exp\left(-m\varphi(\xi)\right)$, $m = 0, 1, 2, 3, ...$. After that equating them to zero, we get algebraic equations solved by Mathematica or Maple to obtain the values of $a_i$. Hence, we get the solutions (2.4), which give the exact solutions of Eq (2.3).

2.2.   The sine-cosine technique

The solutions of Eq (2.3) can be expressed in the form ^[46,47]

or in the form

where $\alpha, \; \beta$ and $r\neq0$, are parameters determined in sequel. From (2.11) we have

and from (2.12) we have

Finally, superseding Eq (2.13) or Eq (2.14) into Eq (2.3), then balance the terms of the cosine functions (2.14) or the sine functions (2.13). Then, we sum all terms with the same power in $cos^{r}(\beta\xi)$ or $sin^{r}(\beta\xi)$ and equating their coefficients to zero in order to obtain an algebraic equations in the unknowns $\beta, \; \alpha$ and $r$. Solving this system yields these unknown constants.

2.3.   Riccati-Bernoulli sub-ODE method

According to description of this method ^{[30,31,32,33,48,49]}, we assume that Eq (2.3) has the following solution:

where $a, b, c$ and $n$ are constants calculated later. From Eq (2.15), we get

The exact solutions of Eq (2.15), for an arbitrary constant $\mu$ are given as follow:

1. For $n = 1$, the solution is

2. For $n \neq 1$, $b = 0$ and $c = 0$, the solution is

3. For $n \neq 1$, $b\neq0$ and $c = 0$, the solution is

4. For $n \neq 1$, $a\neq 0$ and $b^2-4ac < 0$, the solution is

and

5. For $n \neq 1$, $a\neq 0$ and $b^2-4ac > 0$, the solution is

and

6. For $n \neq 1$, $a\neq0$ and $b^2-4ac = 0$, the solution is

Bäcklund transformation

When $\vartheta_{m-1}(\xi)$ and $\vartheta_{m}(\xi)(\vartheta_{m}(\xi) = \vartheta_{m}(\vartheta_{m-1}(\xi)))$ are the solutions of Eq (2.15), we obtain

namely

Integrating Eq (2.26) once with respect to $\xi$, we obtain the following Bäcklund transformation of Eq (2.15):

where $K_1$ and $K_2$ are arbitrary constants. If we get a solution for this equation, we use Eq (2.27) to obtain infinite sequence of solutions of Eq (2.15), as well of Eq (2.1).

3.   Application

In order to solve the Eq (1.1), using exp$(-\varphi(\xi))$-expansion method and the Riccati-Bernoulli sub-ODE method, the following solution structure is selected

where $p$, $\nu$, $k$ and $\omega$ are constants. Substituting (3.1) into (1.1), we have the ODE

Now we apply exp$(-\varphi(\xi))$-expansion and the Riccati-Bernoulli sub-ODE methods for Eq (3.2).

3.1.   Solving Eq (1.1) using the exp$(-\varphi(\xi))$-expansion method

According to the exp$(-\varphi(\xi))$-expansion technique, Eq (3.2) has the following solution

where $A_0$ and $A_1$ are constants and $A_1 \neq 0$. It is easy to see that

Superseding $u$, $u''$, $u^3$ into Eq (3.2) and hence equating the coefficients of $exp{(-\varphi)}$ to zero, we obtain

Solving Eqs (3.6)–(3.9), we get

We consider only one case, whenever the other cases follow similarly. In this case, the solution of Eq (3.3) reads as:

Superseding Eqs (2.6)–(2.7) into Eq (3.10), we obtain:

Case 1. At $\lambda^{2}-4\mu > 0, \mu\neq0,$

Using Eqs (3.1) and (3.11) the solutions of equation (1.1) are

Case 2. At $\lambda^{2}-4\mu < 0, \mu\neq0,$

Using Eqs (3.1) and (3.13) the solutions of Eq (1.1) are

Case 3. At $\lambda^{2}-4\mu > 0, \mu = 0, \lambda \neq0$

Using Eqs (3.1) and (3.15) the solutions of Eq (1.1) are

Case 4. At $\lambda^{2}-4\mu = 0, \mu\neq0, \lambda\neq0,$

Using Eq (3.1) and (3.17) the solutions of Eq (1.1) are

Case 5. At $\lambda^{2}-4\mu = 0, \mu = 0, \lambda = 0,$

Using Eqs (3.1) and (3.19) the solutions of Eq (1.1) are

Here $k, \lambda, \mu, C$ are constants, $\xi = k (x -2pt)$ and $\chi = p x -\frac{1}{2}(4\gamma+k^2(\lambda^2-4\mu)+2p^2)\; t$.

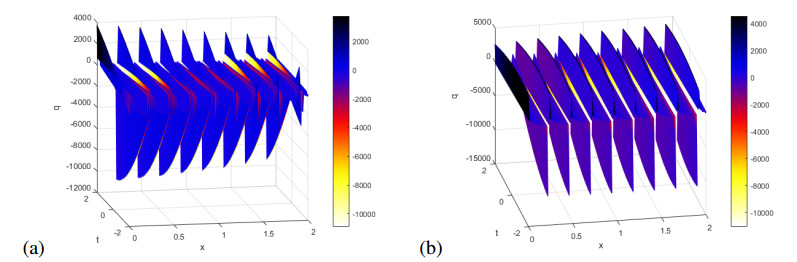
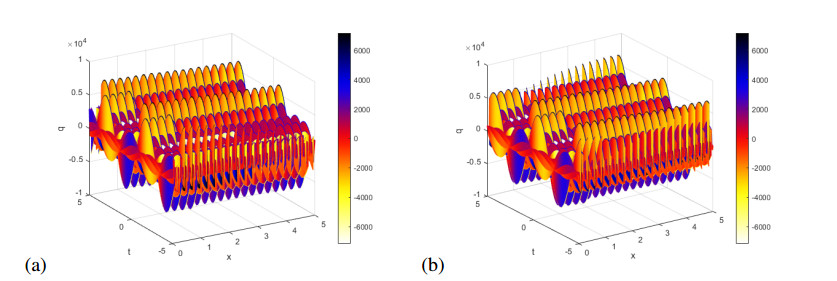
We have plotted these solutions in Figures 1–5. Figure 1(a) shows the real part of $q = q_1(x, t)$ in (3.12), while Figure 1(b) shows imaginary part of this solution for $k$ = 1.5, $p$ = 1.5, $\gamma$ = 1.3, $\lambda$ = 2.3, $\mu = 1$, $\omega$ = -4.5, $\nu$ = -6.3012 and $C$ = 1.4.

Figure 2(a) shows the real part of $q = q_3(x, t)$ in (3.14), while Figure 2(b) shows imaginary part of this solution for $k$ = 1.2, $p$ = 1.2, $\gamma$ = 1.8, $\lambda$ = 1.2, $\mu = 2$, $\omega$ = -2.88, $\nu$ = -0.3168 and $C$ = 0.4.

Figure 3(a) shows the real part of $q = q_5(x, t)$ in (3.16), while Figure 3(b) shows imaginary part of this solution for $k$ = 0.4, $p$ = 0.6, $\gamma$ = 0.3, $\lambda$ = 1.2, $\mu = 0$, $\omega$ = -0.48, $\nu$ = -1.0752 and $C$ = 1.

Figure 4(a) shows the real part of $q = q_7(x, t)$ in (3.18), while Figure 4(b) shows imaginary part of this solution for $k$ = 0.5, $p$ = 0.5, $\gamma$ = 2.3, $\lambda$ = 2, $\mu = 1$, $\omega$ = -0.5, $\nu$ = -4.85 and $C$ = 4.

Figure 5(a) shows the real part of $q = q_9(x, t)$ in (3.20), while Figure 5(b) shows imaginary part of this solution $k$ = -0.7, $p$ = -0.5, $\gamma$ = 0.8, $\lambda = \mu$ = 0, $\omega$ = -0.7, $\nu$ = -1.85 and $C$ = 4.

3.2.   Solving Eq (1.1) using the sine-cosine method

According to sine-cosine technique, subtitling Eq (2.13) into Eq (3.2), gives

Thus by comparing the coefficients of the sine functions, we get

Solving this system gives

for $p^{2}+\nu+2\gamma < 0$ and $k \neq0$. We get the same result if we also use the cosine method (2.14). Thus, the periodic solutions are

and

Using Eqs (3.1) and (3.19) the solutions of Eq (1.1) are

and

However, for $p^{2}+\nu+2\gamma > 0$ and $k \neq0$. we obtain the soliton and complex solutions

and

Using Eqs (3.1) and (3.19) the solutions of equation

and

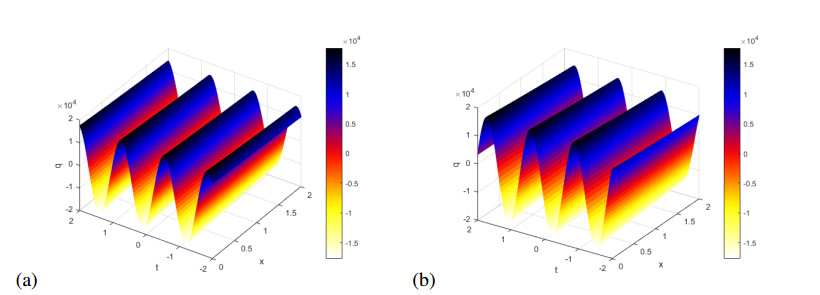
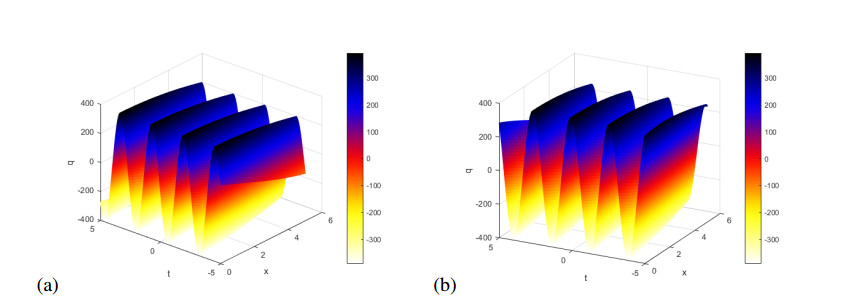
Figure 6(a) shows the real part of $q = \tilde{q}_1(x, t)$ in (3.26), while Figure 6(b) shows imaginary part of this solution for $p$ = 2, $\nu$ = -2, $\gamma$ = -3, $k$ = 2 and $\omega$ = 1.

Figure 7(a) shows the real part of $q = \tilde{q}_5(x, t)$ in (3.30), while Figure 7(b) shows imaginary part of this solution for $p$ = 2.6, $\nu$ = 2.1, $\gamma$ = 3.1, $k$ = 1.2 and $\omega$ = 2.

3.3.   Solving Eq (1.1) using the Riccati-Bernoulli Sub-ODE method

According to Riccati-Bernoulli Sub-ODE technique, substituting Eq (2.16) into Eq (3.2), we get

Putting $n = 0$, Eq (3.32) becomes

Putting each coefficient of $u^i (i = 0, 1, 2, 3)$ to zero, we get

Solving Eqs (3.34)–(3.37), we have

Hence, we give the cases of solutions for Eq (3.2) as follows

Rational function solutions: (When $b = 0$ and $c = 0$, i.e., $p^{2}+\nu+2\gamma = 0$)

The solution of Eq (3.2) is

Therefore, using Eqs (3.1) and (3.42), the following new explicit exact solution of the unstable nonlinear Schrödinger equation can be acquired

where $p, \nu, \gamma, k, \omega, \mu$ are arbitrary constants.

Trigonometric function solution: (When $p^{2}+\nu+2\gamma > 0$)

Superseding Eq (3.1) and Eqs (3.38)–(3.41) into Eqs (2.21) and (2.22), then the exact solutions of Eq (1.1) are

and

Consequently, using Eqs (3.1) and (3.42), the following new explicit exact solution for the unstable nonlinear Schrödinger equation can be obtained

and

where $p, \nu, \gamma, k, \omega, \mu$ are arbitrary constants.

Hyperbolic function solution : (When $p^{2}+\nu+2\gamma < 0$)

Substituting Eq (3.1) and Eqs (3.38)–(3.41) into Eqs (2.23) and (2.24), then the exact solutions of Eq (1.1) are

and

Subsequently, the following new explicit exact solution to the unstable nonlinear Schrödinger equation can be gained

and

where $p, \nu, \gamma, k, \omega, \mu$ are arbitrary constants.

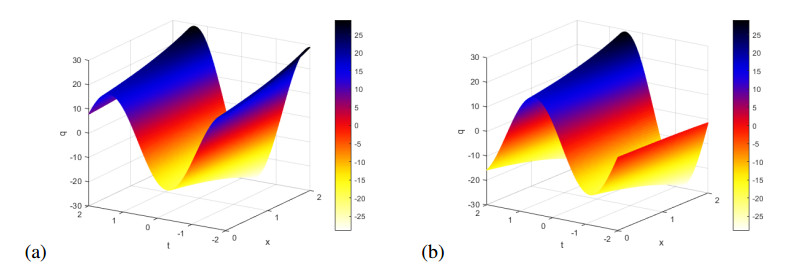
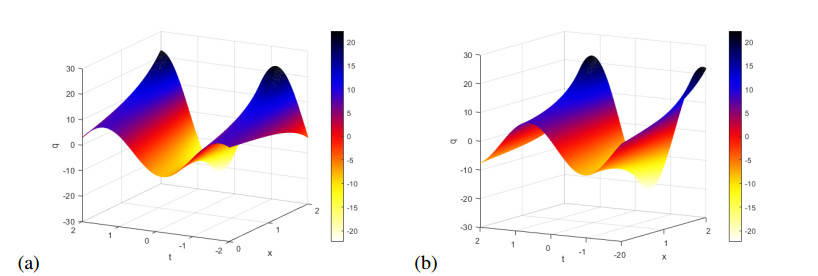
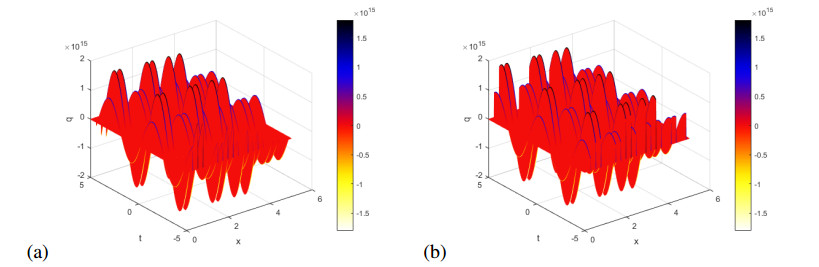
We have plotted these solutions in Figures 8–10. Figure 8(a) shows the real part of $q = \hat{q}_2(x, t)$ in (3.46), while Figure 8(b) shows imaginary part of this solution for $k$ = 0.5, $p$ = -1.3, $\omega$ = 1.3, $\nu$ = 1.4, $\gamma$ = 1.5 and $\mu$ = 1.

Figure 9(a) shows the real part of $q = \hat{q}_6(x, t)$ in (3.50), while Figure 9(b) shows imaginary part of this solution for $k$ = 1.5, $p$ = 1.3, $\omega$ = -3.9, $\nu$ = -2.4, $\gamma$ = -1.3 and $\mu$ = 1.

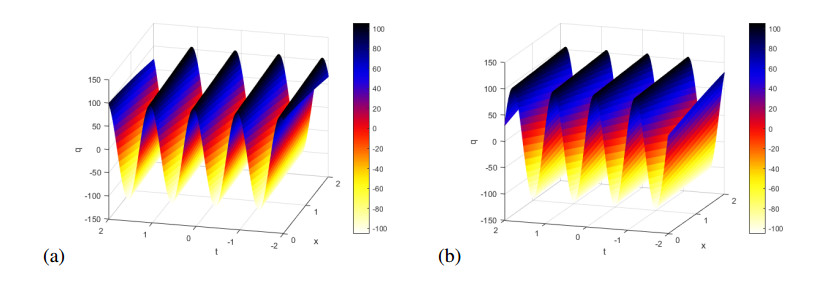
Figure 10(a) shows the real part of $q = \hat{q}_1(x, t)$ in (3.43), while Figure 10(b) shows imaginary part of this solution for $k$ = 0.2, $a$ = 5, $p$ = 1.2, $\omega$ = -0.48, $\nu$ = 1.4 and $\mu$ = 1.

Remark 1. Using Eq (2.27) for $u_i(x, y)$, $i$ = 1, ..., 9, once, then Eq (3.2) as well as for Eq (1.1) has an infinite solutions. In sequence, by applying this process again, we get new families of solutions.

where $B_{3}, p, \nu, \gamma, k, \omega$ and $\mu$ are arbitrary constants.

4.   Results and discussions

In this article, the exp$(-\varphi(\xi))$-expansion, the sine-cosine and Riccati-Bernoulli sub-ODE techniques have been efficiently applied to construct many new solutions. As an outcome, a number of new exact solutions for the UNS equation were formally derived. Namely, the exp$(-\varphi(\xi))$-expansion method gives a first family of ten solutions. Whereas, sine-cosine method give another different second family of eight solutions. Indeed, Riccati-Bernoulli sub-ODE method gives a wide range of new explicit exact solutions including rational functions, trigonometric functions, hyperbolic functions and exponential functions in a straightforward manner. The effectiveness and helpfulness of the exp$(-\varphi(\xi))$-expansion, the sine-cosine and Riccati-Bernoulli sub-ODE methods to deal with UNS equation was proved. As a success, a wide range of new explicit exact solutions were obtained in a straightforward manner. Our study shows that the proposed three methods are reliable in handling NPDEs to establish a variety of exact solutions. Finally, we have plotted some 3D graphs of these solutions and we have shown that these graphs can be controlled by adjusting the parameters.

Remark 2.

1. Comparing our results concerning the UNS equation with the results in ^{[41,42,44,45]}, one can see that our results are new and most extensive. Indeed, choosing suitable values for the parameters similar solutions can be verified.

2. The Riccati-Bernoulli sub-ODE method has an interesting feature, that admits infinite solutions, which has never given for any other method.

3. The three proposed methods in this article are efficient, powerful and adequate for solving other types of NPDEs and can be easily extended to solve nonlinear fractional differential equations, see ^{[32,33,49,50,51,52,53,54,55,56]}.

5.   Conclusions and future works

The exp$(-\varphi(\xi))$-expansion, sine-cosine and Riccati-Bernoulli sub-ODE techniques have successfully been applied for the UNS equation. Many new exact solutions are obtained during the analytical treatment. The availability of computer systems like Matlab or Mathematica facilitates avoids us the tedious algebraic calculations. Indeed, the obtained solutions are of significant importance in the studies of applied science as they help in explaining some interesting physical mechanism for the complex phenomena. The 3D graphs of some exact solutions are plotted for suitable parameters. Finally, the proposed methods can be applied for a wide range of nonlinear partial differential equations arising in natural sciences. Currently, work is in progress on the applications of the proposed methods in this paper in order to solve the other nonlinear partial differential equations. Indeed these methods can be extended to solve fractional partial differential equations.

Acknowledgments

The authors thank the editor and anonymous reviewers for their valuable comments and suggestions.

Conflict of interest

The authors declare no conflict of interest.