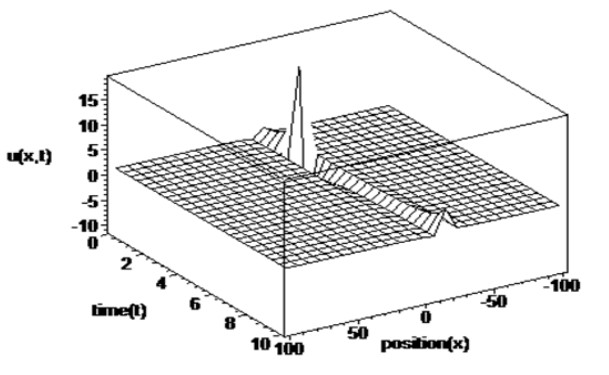
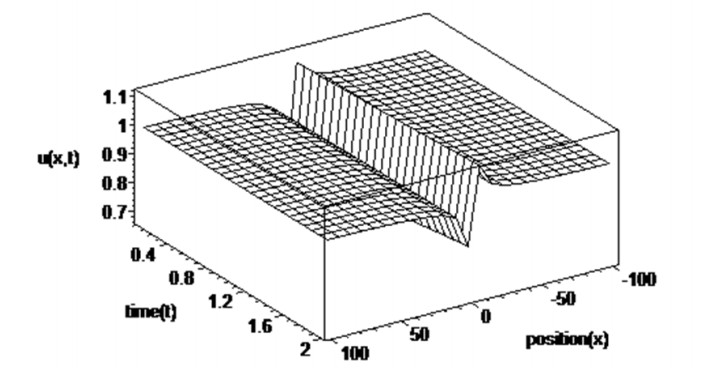
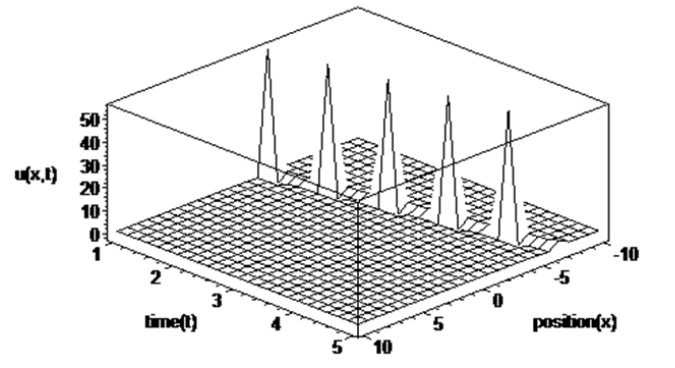
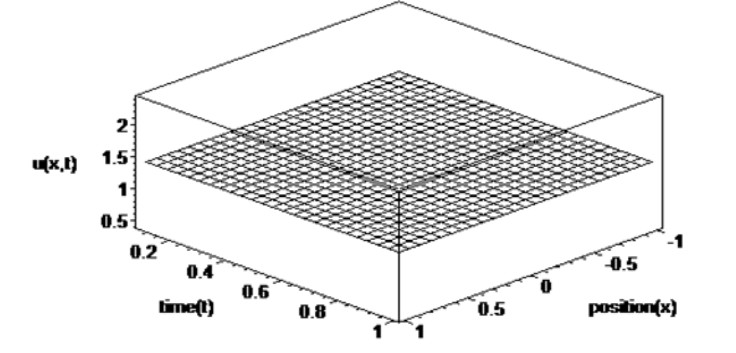
In this work, two algorithms namely, the generalized exp(-w(ξ)) and rational (G'/G2)-expansion methods are suggested for constructing new optical solitons solutions for the perturbed fractional nonlinear Schrodinger equation. The solutions include hyperbolic, trigonometric or rational function. Our results indicate that, group of new solutions are obtained with much reliability, accuracy and efficiency of the proposed methods. Eventually, our pending may become of wide relevance in addition to realize the main features and even propagation of the nonlinear waves in fractal medium.
1.
Introduction
The moderately thick plate is an important material that can adapt to high pressure, high temperature and strong radiation environments, often used in construction engineering, machinery manufacturing, container manufacturing and so on [1,2]. In recent years, many achievements have been made in numerical methods of moderately thick plate problems. There are some typical numerical methods, such as the meshless local radial point interpolation method [3], discrete singular convolution methods [4], spectral element method [5], meshless local Petrov-Galerkin method [6], differential quadrature element method [7], finite integral transform method [8] and chaotic radial basis function method [9]. Compared with numerical methods, the analytical method seems more accurate, but it is difficult to construct. Through the efforts of many scholars, the analytical method has made great progress. The common analytical methods are integral transformation [10,11], semi-inverse method [12], infinite series method [13] and Green's functions [14]. Traditionally, some analytical solutions always need to eliminate the unknown functions by various methods in the range of a class of variables so as to obtain a partial differential equation with higher order, which will make separating variables and other mathematical methods impossible. In order to avoid this phenomenon, academician Zhong put forward the SEA in the 1990s. The essence of SEA is the method of separation of variables based on Hamiltonian system, which does not need to assume the form of the solution in advance. The main feature of the approach is that the solution procedure is conducted in the symplectic space with dual variables rather than in the Euclidean space with one kind of variables, which is rational to solve the equations of elasticity [15,16]. Within the framework of the Hamiltonian system, the analytical solutions of the considered problem can be obtained by using the complete expansion of symplectic eigenvectors without any prior assumptions of the solution forms, which shows the distinctive advantage of the SEA.
SEA needs to transform the equations of elasticity into a proper Hamiltonian system and can uniformly solve the state vector, including as many unknown functions, such as the physical displacements and forces, as possible. Nowadays, the SEA has been extensively studied by some scholars. Xu et al. [17] presented the fracture analysis of fractional two-dimensional viscoelastic media by SEA. Zhao et al. [18] utilized SEA on the wave propagation problem for periodic structures with uncertainty. Qiao et al. [19] considered the plane elasticity problems of two-dimensional octagonal quasicrystals by SEA. Zhou et al. [20] studied new buckling solutions of moderately thick rectangular plates by the symplectic superposition method within the Hamiltonian system framework. Li et al. [21] explored new analytic free vibration solutions of doubly curved shallow shells. Su et al. [22] obtained an analytical free vibration solution of fully free orthotropic rectangular thin plates on two-parameter elastic foundations by the symplectic superposition method. In the last thirty years, the application of SEA has been extended continuously in the electromagnetic elastic solid problem [23], non-Lévy-type functionally graded rectangular plates [24], special mechanical problems in mine engineering [25], buckling of regular and auxetic honeycombs [26], free vibration of non-Lévy-type porous FGM rectangular plates [27], the free vibration of edge-cracked thick rectangular plates [28], the buckling problem of CNT reinforced composite rectangular plates [29] and so on.
In this paper, based on the idea of [30], the mechanical problems of rectangular moderately thick plates are analyzed and a unified framework for a class of rectangular moderately thick plates under the simply supported boundary conditions at opposite sides is given. The model considered in this paper contains free parameters, including the bending, buckling and free vibration problems of the plates. We start from the basic equations of the rectangular moderately thick plates, guide the equations to the Hamiltonian system by introducing appropriate state vector and solve the eigenvalue problem of the Hamiltonian operators. More importantly, we prove the completeness of the eigenfunction system, which lays a theoretical foundation for our general solution. For the applications of the model, based on the obtained general solutions, rectangular moderately thick plates under Winkler type foundation and Pasternak type two-parameter foundation are numerically simulated, which have not been solved by SEA so far. The free vibration problem of moderately thick plates with fully simply supported is also discussed. The satisfactory agreement further confirms the stability of the present method.
2.
The model of the moderately thick plates and SEA
2.1. Basic equations and Hamiltonian system
For the problems of rectangular moderately thick plates, we propose the following general model:
where q1, q2, q3 are loads, α1, β1, γ are free parameters, Mx, My, Mxy, Qx, Qy, w are the bending moments, torsional moment, shear forces and the transverse modal displacement, respectively.
The internal forces of the plates can be presented as
where C=5Eh12(1+ν), D=Eh312(1−ν2) are the elastic constants, in which E denotes the modulus of elasticity, h represents the thickness of the plate and ν is Poisson's ratio. From (2.1a)–(2.2e), the model can be transformed into the following form:
where α=α1+C, β=β1+C.
Setting Vx=α∂w∂x−Cψx and combing (2.2a)–(2.3c), we can obtain the following matrix equation:
It is easy to verify that H=(0TS0) satisfies H∗=JHJ in which J=(0I3−I30), I3 is a 3×3 unit matrix. So H is a Hamiltonian operator matrix, in which
f(x,y)=(0,−q2(x,y),q3(x,y),q1(x,y),0,0)T, U(x,y)=(ψx,−Mxy,Vx,Mx,ψy,w)T.
Next, we continue to use the SEA to solve the Hamiltonian system (2.4).
2.2. Eigenvalues and eigenvectors of H
To solve the Hamiltonian system, the homogeneous system is first considered:
Using the separation of variables method, assuming U(x,y)=X(x)Y(y), we have
with eigenvalue μ and eigenvector Y(y).
The considered model is solved in the rectangular region {(x,y)∣0≤x≤a,0≤y≤b} and the boundary conditions are simply supported at y=0 and y=b as
The eigenvalue problems (2.6) and (2.7) are actually quite complicated to compute. For the sake of brevity below, let ζn=nπb and
n∈N, where N stands for the set of positive integers.
With the help of symbolic software, the corresponding results may be divided into the following three cases.
Case 1. μ1=√2√−C√D(ν−1),μ2=−μ1 are eigenvalues of H and the corresponding eigenvectors are
where the superscript T signifies the transpose of a vector.
Case 2. When Pn≠0, H has six simple eigenvalues, which can be expressed as
and
The corresponding eigenvectors with respect to μni can be rewritten as
(i=1,2,⋯,6; n∈N).
Case 3. When Pn=0, H contains two single eigenvalues:
and two double eigenvalues:
In this case, the basic eigenvectors corresponding to μn1(i=1,2,3,4;n∈N) are
As for the situation of double eigenvalues, there exist first-Jordan form eigenvectors. Solving γ from Pn=0 yields that
and
for further simplification. According to HY1n,i(y)=μnY1n,i(y)+Y0n,i(y), (i=3,4,n∈N). The first-order Jordan eigenvectors are simplified as follows.
Case 3.1. If γ=γn1=−2√αC32−C2−αC−αDζ2n+βDζ2nD, then
Case 3.2. If γ=γn2=2√αC32−C2−αC−αDζ2n+βDζ2nD, we have
2.3. Properties of eigenvectors
After calculating the eigenvalues and eigenvectors, we continue to study the properties of the eigenvectors. And for the analytical solution of this kind of moderately thick rectangular plates, the choice of space is very important, and here we choose the Hilbert space Y:=L2(0,b)⊕L2(0,b)⊕L2(0,b)⊕L2(0,b)⊕L2(0,b)⊕L2(0,b), where L2(0,b) is the space of all square integrable functions. For L(y),R(y)∈Y, the symplectic inner product is defined as
where J is the 6×6 symplectic unit matrix.
2.3.1. When the eigenvalues are simple
Symplectic orthogonality of eigenvectors lays a foundation for the expansion of eigenvectors. The following lemma can be obtained via straightforward calculation.
Lemma 1. The system of eigenvectors {Y1(y),Y2(y)}∪{Yn,i(y)∣n∈N,i=1,2,⋯,6} of H satisfies the following symplectic orthogonality relations:
where the constants A34,n and A56,n are presented in Appendix A. Other eigenvectors satisfy the following symplectic orthogonal relation:
Based on the conjugate symplectic orthogonality of eigenvectors, we can obtain the following completeness theorem of eigenvectors, which provides the theoretical basis for the expression of general solutions.
Theorem 1. The system of eigenvectors {Y1(y),Y2(y)}∪{Yn,i(y)}+∞n=1 (i=1,2,⋯,6) of H is complete in Y.
Proof. For any F(y)=(f1(y),f2(y),f3(y),f4(y),f5(y),f6(y))T∈Y, it is sufficient to prove that there exist constant sequences {Cn,i}+∞n=1, (i=1,2,⋯,6), C1 and C2 such that
Applying Lemma 1, we can take
and then
Clearly, \, +∞∑n=12b(∫b0fi(y)sinnπybdy)sinnπyb (i=1,3,4,6) and
are the corresponding Fourier series expansions of fi(y) based on the orthogonal systems {sinnπyb}+∞n=1 and {cosnπyb}+∞n=0 in L2(0,b), respectively. Thus, F(y) can be expressed by (2.9) and the proof is completed. □
2.3.2. When the eigenvalues are double
Similar to the case where the eigenvalues are single, we first discuss the orthogonal conjugations of eigenvectors. These relations play a crucial role in proving the symplectic eigenvectors expansion theorem.
Lemma 2. The system of eigenvectors {Y1(y),Y2(y)}∪{Y0n,i(y),Y1n,j(y)∣n∈N;i=1,2,3,4;j=3,4} of H have the following symplectic orthogonality relations:
When γ=γn1=−2√αC32−C2−αC−αDζ2n+βDζ2nD, we have
If γ=γn2=2√αC32−C2−αC−αDζ2n+βDζ2nD, then
Based on Lemma 2, the following completeness theorem is obtained.
Theorem 2. The system of eigenvectors {Y1(y),Y2(y)}∪{Y0n,i(y)}∪{Y1n,j(y)}, (i=1,2,3,4;j=3,4;n∈N) of H is complete in Y.
Proof. It is similar to the proof of Theorem 1 that we just have to take the sequences:
It is easy to calculate that
As a result,
□
2.4. General solution
This section will give the general solutions of the Hamiltonian system under simply supported boundary conditions at y=0 and y=b based on the proven completeness theorems.
2.4.1. When eigenvalues are simple
The general solution of (2.5) can be represented in the form:
The nonhomogeneous term f(x,y) expands to
From the above formulas and (2.6), we obtain
where Ψ1,Ψ2,Ψni are undetermined constants, θ1,θ2,θn,i can be expressed in a form similar to (2.10) via the symplectic inner product of f(x,y) and Y. In particular, if qk(x,y) (k=1,2,3) is the uniformly distributed load acting on the plate, which means that it is a constant independent of x and of y. If qk(x,y) (k=1,2,3) represents a concentrated load of strength B acting on the plate, then it can be expressed as Bδ(x−x0,y−y0) in which δ(x−x0,y−y0) denotes the δ function.
The general solutions of ψy and w can be obtained by taking the fifth and sixth components of (2.13), respectively.
2.4.2. When eigenvalues are double
Compared to the case where the eigenvalues are simple, we combine (2.6) with (2.12) to obtain:
where Q1,Q2,Q0n,i,Q1n,j (i=1,2,3,4; j=3,4) are undetermined constants, Θ1,2,Θ0n,i,Θ1n,j can be computed similarly as (2.11) by symplectic inner product between f(x,y) and the eigenvectors.
Then, U(x,y) can be given by
As for the case of double eigenvalues, in order to obtain a simple form of ψy and w, a classification discussion is carried out.
(i) When γ=γn1=−2√αC32−C2−αC−αDζ2n+βDζ2nD, we have
(ii) When γ=γn2=2√αC32−C2−αC−αDζ2n+βDζ2nD, we can see that
3.
Applications
The general solution of a kind of rectangular moderately thick plates has been obtained, which can be applied to some specific mechanical problems, such as bending, buckling, free vibration, etc. For example, when α=β=C,γ=q1(x,y)=q2(x,y)=0,q3(x,y)=−q, the model reduces to the bending problem of moderately thick plates with two opposite edges simply supported [31]; when α=C+Nx,β=C+Ny,γ=q1(x,y)=q2(x,y)=q3(x,y)=0, it represents the document [20] in discussing the buckling problem of the moderately thick rectangular plates; the reference [32] discusses a free vibration problem of rectangular moderately thick plates, corresponding to α=β=C,γ=ρhϖ2 (ϖ represents circular frequency) and q1(x,y)=q2(x,y)=q3(x,y)=0. For these specific problems, although they may correspond to different boundary conditions, most of them can be obtained by the general solution in this paper.
So far, SEA has not been applied to the problems of moderately thick rectangular plates with elastic foundations. In this section, the solutions to two elastic foundation problems are discussed first based on the general solutions of the considered model and the numerical results are compared with those in the literature.
Example 1. Consider a uniformly loaded and simply supported square plate resting on a Winkler-type elastic foundation with side length a. In this case, q1(x,y)=q2(x,y)=0, q3(x,y)=q, α1=β1=0 and γ=k, where q denotes the uniform load and k is the subgrade reaction operator for Winkler-type foundation [33,34,35], the corresponding equilibrium has the following form,
According to (2.17), the form of w is given in detail:
where μn3=√−−2Cζ2n+k+P12C,μn5=√2Cζ2n−k+P12C,P1=√k(4C2D+k),
Herein, Ψn,i are determined by the simply supported conditions at x=0 and x=a. The constants E=2.06×1011pa, ν=0.3, q=6.89×107pa, a=1.016m are taken from [33,34,35]. The corresponding results are shown in Table 1.
In Table 1, we only take the first 20 items of the series to achieve a better fitting effect with the data in the reference [33,34,35], which indicates that it is satisfactory to use the SEA to solve this moderately thick rectangular plate with single parameter foundation. Table 2 presents the data convergence analysis for the central deflection w when ha=0.3 with the different parameters k. It can be seen that convergence of the series is satisfactory, and it confirms the completeness theorem of the eigenvector system discussed above in this paper.
Example 2. The equilibrium equations of the bending problem of rectangular moderately thick plates with Pasternak type two-parameter elastic foundation are[36,37]:
For comparison with the data in [36,37], a square plate with the side length of a is considered under fully simply supported conditions. This is the special case of our model when q1(x,y)=q2(x,y)=0, q3(x,y)=−q, α=β=C+Gf, γ=−kf. Furthermore, we take the general solution of the fifth and sixth components of U(x,y), and the specific forms of the deflection w and the bending moment Mx are as follows:
in which
θn,3 and θn,5 are displayed in Appendix B.
Taking the first 20 terms of the above formula, we calculate the center deflection w and bending moment Mx corresponding to different values of h/a and Gf. The relevant results are listed in Table 3 and compared with the data in the references. The reference [36] uses the grid method of 19×19, and the reference [37] uses the analytic trigonometric series method. Compared with them, it is shown that the SEA in the presented paper is effective.
Example 3. For the free vibration of fully simply supported moderately thick rectangular plates, its governing equations are as follows[38,39]:
where ρ is the mass per unit area of the plate and ω is the natural frequency of the plates.
By analyzing the boundary conditions and letting the determinant of the coefficients be equal to zero, the frequency equation of the free vibration problem is given by
To justify the frequency equation, we give the numerical results for the lowest six natural frequency parameters ˉk=ρω2b4Dπ4 in Table 4. Compared with [38] and [39], the satisfactory agreement further confirms the stability of the present model and method.
4.
Conclusions
In this paper, the model for a class of moderately thick rectangular plates is considered, which includes the bending, free vibration and buckling problems of plates and is expected to be widely used in functionally graded material problems. Based on the equilibrium equations of the model, the relationship between force and displacement, the Hamiltonian system and the corresponding Hamiltonian operator are derived, which provides the possibility for the applications of SEA and the symplectic superposition method. Based on the calculation of eigenvalues and the analysis of the properties of symplectic eigenvectors, the general solution under the simply supported boundary conditions of opposite edges is given by using the proved completeness theorem. In addition, the general solution is applied to two kinds of elastic foundation problems of moderately thick rectangular plates, which have not been solved by the SEA before. At the same time, the higher order and more accurate frequency parameters are solved for the free vibration problem of moderately thick rectangular plates with fully simply supported. The satisfactory numerical results confirm the effectiveness and universality of our model. It should be noted that the buckling problem of the plates is solved similarly to the free vibration problem except that the buckling factor is included in the values of α1 and β1 in the model.
Use of AI tools declaration
The authors declare they have not used Artificial Intelligence (AI) tools in the creation of this article.
Acknowledgments
This work was supported by the Natural Science Foundation of Inner Mongolia (No.2021MS01004)
Conflict of interest
All authors declare no conflicts of interest in this paper.
Appendix
Appendix A
Appendix B









 DownLoad:
DownLoad: