1.
Introduction
The purpose of this article is to study the asymptotic behavior of the high frequency modes of thin plates when the thickness of the plates goes to zero. The asymptotic methods were used to study a large variety of problems in thin elastic structures. Let us refer to Ciarlet and Destunder [1] for the justification of the two-dimensional linear plate model, Ciarlet et al. [2] for the junctions between three-dimensional and two-dimensional linear elastic structures, Le Dret [3,4] for modeling of a folded plate and [5] for modeling of the junction between two rods, and Trabucho and Viaño [6] for asymptotic analysis of linearized elastic beams.
The problem of modeling the vibrations of thin elastic structures using a rigourous asymptotic technique was first done by Ciarlet and Kessavan [7] in the case of a clamped plate. The techniques introduced in this article were adapted and used to study different spectral problems: Le Dret [8] for the vibrations of a folded plate, Bourquin and Ciarlet [9] and Lods [10] for a plate inserted in a three-dimensional body, Kerdid [11,12] for a single rod and junction between two rods. All these works are concerned with the convergence of low frequency modes of the three-dimensional linear elasticity, as the thickness of the body tends to zero. The limit problems obtained are the classical spectral problems associated with the flexural displacement of the structure. However, these techniques fail to obtain the limit problem for higher frequency modes.
We also refer to some interesting works related to this article, which deal with the asymptotic analysis of the eigenvalue problem in different thin linear elastic structures: Jumbo and Rodriguez Mulet [13] and Jumbo et al. [14] for thin elastic rod with non-uniform cross-section, Serpilli and Lenci [15] for laminated beams, Tamba˜ca [16] for curved rods and Qaudiello et al. [17] for a thin T-like shaped structure.
A nonstandard technique has been proposed in Irago et al. [18,19] to study the behavior of the high frequency modes and their associated eigenfunctions in thin elastic rods. The limit problem obtained is the coupled one-dimensional spectral problem giving the classical equations for torsion and stretching vibrations.
In this work, we combine the techniques of [7,19] to study the asymptotic behavior of high frequency modes in a thin clamped plate when the thickness of the plate goes to zero. Indeed, we will construct a suitable families of index {ℓmε}ε>0, which varies with ℓ and ε, and for which the high frequency eigenvalues ηℓmε converge when the thickness of the plate approaches zero towards the eigenvalues ηm of a two-dimensional spectral problem. The limit problem is identified to be the standard eigenvalue problem associated with the classical equations for stretching vibrations of the plate. The limit eigenfunctions are determined by the couples (ζm1,ζm2) of functions of the longitudinal variables of the plate that are the unique solution of the limit problem and correspond to the stretching displacements of the plate.
2.
The three-dimensional problem
Let ω be an open bounded set of R2 and γ=∂ω its boundary which is assumed to be sufficiently smooth. Given ε≥0 we define
Ωε is assumed to be the reference configuration of the plate under consideration. The plate is clamped on its boundary Γε. The material that constitute the plate is assumed to be homogeneous and isotropic with Young's modulus E and Poisson's ratio ν, all independent of ε.
We will also use the Lame's coefficients λ and μ related to E and ν by the formulas:
In the sequel, we shall use the repeated index convention, the Greek indices take their values in the set {1,2} and the Latin indices take their values in the set {1,2,3}.
The classical eigenvalue problem for the plate under consideration consists in finding pairs (ηε,uε) satisfying:
where σε(uε) is the stress tensor, nε is the outer unit normal vector to ∂Ωε, and eε(uε) is the linearized strain tensor corresponding to the displacement uε:
In order to put the above problem in variational form, we introduce the space
So, problem (2.3) is equivalent to finding (ηε,uε)∈R×Vε satisfying
Thanks to Korn inequality, the bilinear form
is Vε-elliptic. From spectral theory, it is known that problem (2.6) has a sequence of eigenvalues (ηεm)m≥1 satisfying
with
associated with a family of eigenfunctions (uε,m)m≥1, that is
which can be orthonormalized as
and which make a basis in both Hilbert spaces Vε and [L2(Ωε)]3.
3.
The scaled problem
In order to define a problem equivalent to problem (2.6) but posed over a domain which does not depend on ε, we let
and
We introduce the following mapping:
and the scaling functions v(ε)∈V defined as:
Due to scaling, we have
Substituting (3.4) in (2.6), we obtain the following scaled variational formulation:
Find (ηm(ε),um(ε))∈R×V such that for, all v∈V
where
and
with the normalization condition
We define the space of Kirchhoff-Love on Ω as
Elements of this space are characterized by
where ζα∈H1(ω) and ζ3∈H2(ω).
4.
Convergence of low frequency modes
A first convergence analysis of the low frequencies of the three-dimensional linearized elasticity system in a thin plate, when the thickness of the plate approaches zero, was done in [7]. It has been shown that the standard biharmonic two-dimensional eigenvalue problem associated with the flexural displacements of the plate can be derived mathematically from the standard three-dimensional eigenvalue problem of linear elasticity through a rigourous convergence analysis as the thickness of the plate tends to zero.
More precisely, it has been proven that for each integer m≥1,
and
with
where (λm(0),um3)∈R×H20(ω) are eigensolutions of the limit spectral problem:
Find (λ,u)∈R×H20(ω) such that, for all v∈H20(ω)
The pairs (λm(0),um3) are solutions of the eigenvalue problem for the biharmonic operator Δ2
corresponding to the classical equations for the flexural vibrations.
5.
Convergence of high frequency modes
Our objective in this work is to characterize the limit problem associated to high frequency modes when the thickness of the plate goes to zero. Unfortunately, the techniques used in [7] are not adapted for the asymptotic analysis of higher frequency modes. So, we will be inspired by the idea proposed in [18,19] for the convergence analysis of high frequencies in a thin rod in order to characterize the limit problem for high frequency modes in a thin plate.
Let us start with the following lemma:
Lemma 5.1. There exists an increasing sequence of constants Km>0,m≥1, independent of ε such that
Proof. From [7] Lemma 1 we have, for each integer m≥1,ε−2ηm(ε)≤Km where Km is a constant independent of ε, which gives (5.1). □
So, if we fix the index m and we make ε tend to zero, all the sequence ηm(ε) goes to zero. indeed, the high frequency modes are concentrated at infinity when ε approaches zero and cannot be obtained using such a passage to the limit. So, the idea in order to characterize this kind of frequencies, consists in associating to each integer m≥1, a family of index {ℓmε}ε>0 that depend on ε and such that
and
This family of index can be defined by
It is clear that (5.4) satisfies (5.2) and (5.3).
The family of index {ℓmε} varies with m and ε, and for each ε>0, {ℓmε}m≥1 is an increasing subsequence of positive integers satisfying ℓmε≥m,∀m≥1. It contains the indices of the stretching modes among all the modes {ηm(ε)}m≥1 of the plate.
To illustrate this idea and show the layout of the stretching modes {ηℓmε(ε)} when m and ε vary, let us represent the family {ηℓmε(ε)} in a double-entry table. Consider a decreasing sequence {εn}n≥1 converging to 0, the elements of the sequences {ηm(εn)}m≥1 are arranged in rows while the elements of the sequences {ηm(εn)}n≥1 are arranged in columns.
Since for each m≥1 the family {ℓmεn}n≥1 is increasing, that is ℓmεn≥ℓmεn′ for n>n′, the elements of the sequence {ηℓmεn(εn)}n≥1 corresponding to the modes associated to the stretching vibrations of the plate are arranged diagonally. As the stretching vibrations are high frequency modes and are concentrated at infinity when ε approaches zero, they can only be reached through such a family of indices.
The following theorem summarize the results obtained when passing to the limit on these families of sequences and constitute the main result of this paper.
Theorem 5.2. For each integer m≥1, there exists a sequence {ℓmε} such that
where ηm(0) is an eigenvalue of the limit spectral problem:
Find (η,ζ)∈R×[H10(ω)]2 such that, for all ξ∈[H10(ω)]2,
In addition, there exists a subsequence (still denoted ε) and um(0)∈V such that
where
and
with
If (ζm1,ζm2)≠(0,0) then it is an eigenfunction associated to ηm(0).
To prove this theorem we combine the techniques in [7,19]. First, we start by establishing an appropriate bound for the eigenfunctions.
Lemma 5.3. For each m≥1, there exists a constant Cm>0 independent of ε, such that
Proof. Let us define the scaled strain tensors
Taking v=uℓmε(ε) in (3.5) and using (3.7) and (3.8), we obtain
So, we have
and consequently, since 0 < {{\varepsilon}} \leq 1 ,
Therefor, (5.10) is obtained using Korn inequality in H_\Gamma^1(\Omega; {{\mathbb R}}^3) . □
Lemma 5.4. For each m\geq 1 , there exists a subsequence (still denoted {{\varepsilon}} ) such that
and
where
with \zeta_\alpha \in H^1_0(\omega) and \zeta_3 \in H^2_0(\omega).
Proof. Convergences (5.14) and (5.15) come from (5.3) and (5.10). Now using (5.13) we have
and since
then
Thus,
Therefore, u^{m}(0) \in V_{KL} and consequently, we deduce (5.16) and (5.17) from (3.10). □
Lemma 5.5. For each m\geq 1 , if \eta_m(0)\neq0 then
Proof. Let v = (0, 0, v_3), \; v_3 \in H_0^1(\omega) we have
Substituting in (3.5) and multiplying the equation by {{\varepsilon}}^2 we have
Passing to the limit as {{\varepsilon}} \rightarrow 0 , we obtain
Therefore,
□
Lemma 5.6. For each m\geq 1 , there exists a subsequence (still denoted {{\varepsilon}} ) such that
with
and
Proof. Convergence (5.19) comes from (5.12) and from (5.15) we have
Using (5.16) and (5.18) we obtain
and then
Taking now v = \left(v_1, v_2, 0 \right) \hbox{in} (3.5), \hbox{with} \; v_\alpha\in H_\Gamma^1(\Omega) , and multiplying the equation by {{\varepsilon}} , we obtain
Passing to the limit as {{\varepsilon}} \rightarrow 0 , we obtain
which has as unique solution (see [8])
□
Lemma 5.7. For each m\geq 1 , there exists a subsequence, still denoted {{\varepsilon}} , such that
where
Proof. Let v = (0, 0, v_3), \; v_3 \in H_\Gamma^1(\Omega) , we have
Substituting in (3.5) and multiplying the equation by {{\varepsilon}}^2 , we obtain
Which gives by passing to the limit as {{\varepsilon}} \rightarrow 0
and consequently,
□
Lemma 5.8. The stretching displacements (\zeta_1^m, \zeta_2^m) \in [H_0^1(\omega)]^2 satisfy for all (\xi_1, \xi_2) \in [H_0^1(\omega)]^2 ,
Proof. Taking v = (\xi_1, \xi_2, 0) in (3.5) with (\xi_1, \xi_2) \in [H_0^1(\omega)]^2 , we have
Passing to the limit when {{\varepsilon}} \rightarrow 0 , we obtain
which can be written, using (5.25)
Replacing \kappa^m_{\alpha\beta}(0) by their expressions (5.20), we obtain
(5.26) comes using relations (2.2). □
Lemma 5.9. For each m\geq 1 , the whole family (\eta_{{\ell_\varepsilon^m}}({{\varepsilon}}))_{{{\varepsilon}} > 0} converges as {{\varepsilon}} \rightarrow 0 . In addition, if \eta_m(0) is a simple eigenvalue of (5.26), then \eta_{{\ell_\varepsilon^m}}({{\varepsilon}}) is also a simple eigenvalue of (3.5) for {{\varepsilon}} < {{\varepsilon}}_0 small enough.
Proof. See [7]. □
Proposition 5.10. For each m\geq 1 , the limit eigensolutions (\eta_m(0), \zeta_1^m, \zeta_2^m) verify the classical equations of stretching vibrations:
with
Proof. Performing an integrating by part in the left-hand side of Eq (5.26) we obtain, for all (\xi_1, \xi_2) \in [H_0^1(\omega)]^2
which gives, for all (\xi_1, \xi_2) \in [H_0^1(\omega)]^2
(5.27) is obtained by taking respectively \xi_2 = 0 and \xi_1 = 0 . □
6.
Conclusions
In this work, we have proved that the stretching frequencies of an elastic thin plate is the limit of a family of high frequencies of the three-dimensional elastic model of the plate, as the thickness approaches zero. We have also shown, that the standard spectral problem associated to stretching modes in linear elastic plates can be derived mathematically from the standard three-dimensional eigenvalue problem of linear elasticity through a non-standard asymptotic analysis technique. This technique can be used to study a wide variety of problems of modeling vibrations for thin structure like folded plate and junction between different thin structures.
Use of AI tools declaration
The authors declare they have not used Artificial Intelligence (AI) tools in the creation of this article.
Acknowledgments
The author gratefully acknowledges the financial support of the Deanship of research of Imam Mohammad Ibn Saud Islamic University (IMSIU).
Conflict of interest
The author declares no conflict of interest.









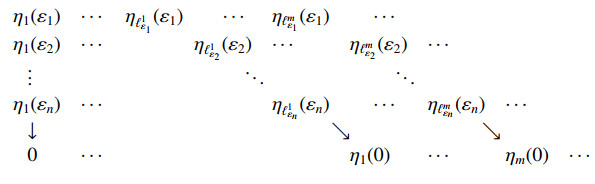




 DownLoad:
DownLoad: