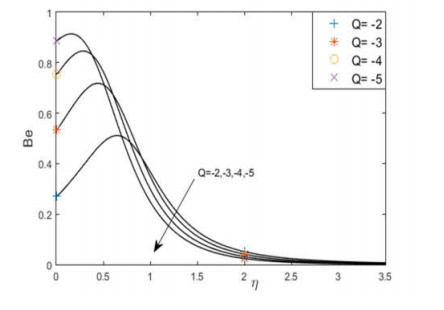
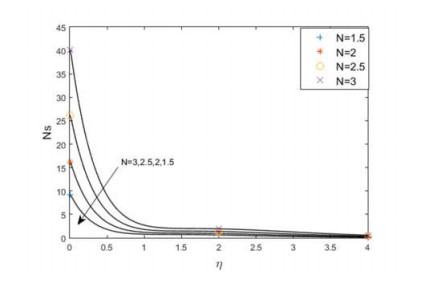
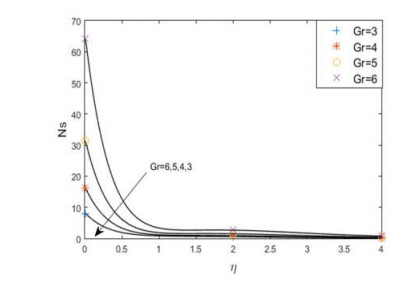
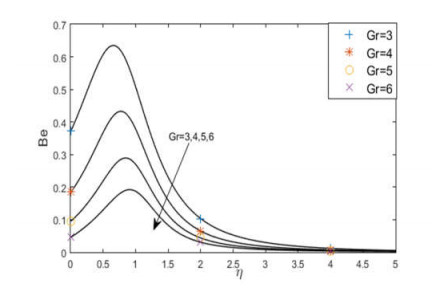
This paper reports entropy generation analysis of radiative micropolar fluid flow in porous medium. The mathematical model depicting convective boundary layer flow due to a vertically moving infinite plate bounding the porous medium on one side is solved numerically. An implicit finite difference method together with Gauss elimination method is used. The numerically computed velocity and temperature fields are employed to analyze entropy. The plots for entropy generation number for various sets of parameters are drawn. It is found that entropy generation number Ns decreases with increasing values of heat sink parameter Q and radiation parameter N whereas it increases with increasing values of Grashoff number Gr, Brinkman number Br. The Bejan number shows pronounced variations for the parameters entering into the problem.
1.
Introduction
This paper considers a fractional coupled system on an infinite interval involving the Erdélyi-Kober derivative:
where δ1,δ2∈(1,2], γ∈(−2,−1), and β>0. Dγ,δ1β, Dγ,δ2β are Erdélyi-Kober fractional derivatives (EKFDs for short), and Iδ1+γ,2−δ1,Iδ2+γ,2−δ2 are the Erdélyi-Kober fractional integrals. F,G are continuous functions. We discuss the existence of positive solutions for (1.1).
During the past several decades, fractional equations have been studied widely; see [1,2,3,4,5,6,7,8,9,10,11,12,13,14,15,16,17,18,19,20,21,22,23,24,25,26,27,28,29,30,31,32,33,34,35,36] for instance. From the literature, we can see that there are many fractional derivatives used in differential equations. Among these various definitions, the widely used ones are the Riemann-Liouville and Caputo fractional derivatives, in many works. To generalize the Riemann-Liouville fractional derivative, Erdélyi-Kober defined a new fractional derivative, and we call it the Erdélyi-Kober fractional derivative. Moreover, the Erdélyi-Kober operator is very useful; we can refer to [6,9,14,15,16,17] and the references therein. The Erdélyi-Kober operator is a fractional integration operation which was given by Arthur Erdélyi and Hermann Kober in 1940 [23]. Some of these definitions and results were given in Samko et al. [3], Kiryakova [19], and McBride [20].
Nowadays, the theory of fractional operators in the Erdélyi-Kober frame has attracted much interest from researchers. The study of fractional systems is also very important, as these systems appear in various applications, especially in biological sciences. Recently, some problems of Erdélyi-Kober type fractional differential equations on infinite intervals received widespread attention from many scholars; see [8,21,22] for example.
Recently, in [8], the authors investigated the following equation:
where σ∈(1,2), ϑ∈(1,2), θ>0, and F is a given continuous function, Dϑ,σθ denotes the EKFD, and Iσ+ϑ,2−σ denotes the Erdélyi-Kober fractional integral. The authors studied the existence and nonexistence of positive solutions for this problem by utilizing a fixed point result which uses the strongly positive-like operators and eigenvalue criteria.
In [9], the authors studied a fractional coupled system:
where cDϱ,cDς1,cDς,cDϱ1 are the Liouville-Caputo fractional derivatives of order 2<ϱ,ς≤3, 1<ς1,ϱ1<2. Iξ,Iζ are the Riemann-Liouville fractional integrals of order 1<ξ,ζ<2. Jυ,ϖρ,Jω,θv are the Erdélyi-Kober fractional integrals of order ϖ,θ>0, with v,ω>0, ρ, ϑ∈(−∞,+∞). F,G:K×(−∞,+∞)4→(−∞,+∞) and ϕ1,ϕ2:C(K,(−∞,+∞))→(−∞,+∞) are continuous functions. γ,δ,ε1,ε2 are positive real constants. The existence result was given by the Leray-Schauder alternative, and the uniqueness result was obtained due to Banach's fixed-point theorem. By the same methods, Arioua and Titraoui [18] studied system (1.1). Moreover, In [10], Arioua and Titraoui also investigated a new fractional problem involving the Erdélyi-Kober derivative. Inspired by the above articles, we use different methods to consider the fractional coupled system involving Erdélyi-Kober derivative (1.1). We employ the Guo-Krasnosel'skii fixed point theorem to discuss (1.1) in a special Banach space, and we also use the monotone iterative technique to study this system. Some existence results of positive solutions for system (1.1) are obtained, including the existence results of at least two positive solutions.
2.
Preliminaries
Definition 2.1. (see [2]) Let α∈(−∞,+∞). Cnα, n∈N, denotes a set of all functions f(t),t>0, with f(t)=tpf1(t) with p>α and f1∈Cn[0,∞).
Definition 2.2. (see [1,2]) For a function u∈Cα, the σ-order right-hand Erdélyi-Kober fractional integral is
in which, Γ is the Euler gamma function.
Definition 2.3. (see [2]) Let n−1<δ≤n,n∈N, and for u∈Cα, the σ-order right-hand Erdélyi-Kober fractional derivative is
where
Lemma 2.1. (see [10]) Let 1<σ≤2, −2<γ<−1, β>0, and h∈C2α, with ∫∞0sβ(γ+m)−1h(τ)dτ<∞, m=1,2. The fractional problem
has a unique solution given by u(x)=∫∞0Gσ(x,s)sβ(γ+1)−1h(s)ds, where
Lemma 2.2. (see [10]) For 1<σ≤2, −2<γ<−1, and β>0, the function Gσ, defined in (2.1), has the following properties:
(i) Gσ(x,s)1+x−β(1+γ)>0, for x,s>0;
(ii) Gσ(x,s)1+x−β(1+γ)≤βΓ(σ), for x,s>0;
(iii) for 0<τλ≤x≤τ and s>τλ2, where λ>1,τ>0, we have
where p(τ)=(σ−1)τ−β(1+γ)λβ(1+γ)(1+τ−β(1+γ)).
Lemma 2.3. (see [18]) Let 0<σ1,σ2≤1 and F,G∈C2α with
Then, (1.1) has a unique solution given by
where
The following result is our main tool.
Lemma 2.4. (Guo-Krasnosel'skii fixed point theorem; see [37]) P is a cone in a Banach space E, and D1 and D2 are bounded open sets in E with θ∈D1, ¯D1⊂D2. A:P∩(¯D2∖D1)→P is a completely continuous operator. Consider the following conditions (ⅰ), (ⅱ):
(i) ‖Aw‖≤‖w‖ for w∈P∩∂D1, ‖Aw‖≥‖w‖ for w∈P∩∂D2;
(ii) ‖Aw‖≥‖w‖ for w∈P∩∂D1, ‖Aw‖≤‖w‖ for w∈P∩∂D2.
If one of the preceding conditions (ⅰ), (ⅱ) holds, then A has at least one fixed point in P∩(¯D2∖D1).
Next, we present some hypotheses that will play an important role in the subsequent discussion:
(H1) F,G:(0,+∞)×(−∞,+∞)×(−∞,+∞)→(0,+∞) are continuous and nondecreasing with respect to the second, third variables on (0,+∞).
(H2) For (x,u,v)∈(0,+∞)×(−∞,+∞)×(−∞,+∞),
such that
with ωi,~ωi∈C((0,+∞),(0,+∞)) nondecreasing and φi,ψi∈L1(0,+∞), i=1,2.
(H3) There are positive functions qi,˜qi,i=1,2, with
such that
for any u,v,˜u,˜v∈(−∞,+∞) and x∈(0,+∞).
(H4) F,G:(0,+∞)×(0,+∞)×(0,+∞)→(0,+∞) are continuous, such that
where a1,a2∈L1((0,+∞),(0,+∞)), F1,G1∈C((0,+∞)×(0,+∞)×(0,+∞),(0,+∞)), 0<∫ττλa1(x)dx<∞, 0<∫ττλa2(x)dx<∞, with τ>0, λ>1. Moreover, xβ(1+γ)−1F(x,u,v), xβ(1+γ)−1G(x,u,v):[0,+∞)×(0,+∞)×(0,+∞)→[0,+∞) also are continuous.
Remark 2.1. These conditions ensure the continuity and integrability of nonlinear terms in an infinite interval, which play a very important role in the proof of completely continuity for the relevant integral operators.
3.
Main results
In this section, we use two Banach spaces defined by
with the norm
and
with the norm
So, (X×Y,‖(u,v)‖X×Y) is a Banach space, with the norm ‖(u,v)‖X×Y=‖u‖X+‖v‖Y.
Lemma 3.1. If F,G are continuous, then (u,v)∈X×Y is a solution of system (1.1)⇔(u,v)∈X×Y is a solution of the following equations:
For (u,v)∈X×Y, we define an operator A:X×Y→X×Y as follows:
where
with Gσi(x,s),i=1,2, given by (2.2) and (2.3).
Remark 3.1. Let σ1,σ2,β,γ,λ,τ∈R, such that 1<σ1,σ2≤2,β>0,−2<γ<−1,λ>1,τ>0. If (H2) and (H4) hold, then for (u,v)∈X×Y with u(x),v(x)>0,
where η=max{η1,η2} with η1=1+ιϱ1(λ2−1),η2=1+ι∗ϱ2(λ2−1)>1, ϱ1,ϱ2,ι,ι∗>0.
Proof. By (H4), for x∈[τλ2,τ], we know that there exist two constants ϱ1,ϱ2>0, such that
So, for (u,v)∈X×Y with u(x),v(x)>0,
and hence,
By (H4), we know that there exist two constants ι,ι∗>0, such that
Thus,
Therefore, we can obtain
Similarly,
Take η=max{η1,η2}, and thus
hold. □
4.
A positive solution
Define two cones
Obviously, K1×K2={(u,v)∈X×Y∣u(x)>0,v(x)>0,∀x>0; minx∈[τλ,τ]u(x)1+x−β(1+γ)≥p(τ)η‖u‖X,minx∈[τλ,τ]v(x)1+x−β(1+γ)≥p(τ)η‖v‖Y} is also a cone. For convenience, we first list the following definitions:
Lemma 4.1. If assumptions (H1) and (H2) hold, then A:K1×K2→K1×K2 is completely continuous.
Proof. First, we show A:K1×K2→K1×K2. By (H1) and (H2), for (u,v)∈K1×K2,
Similarly,
By (H1) and Lemma 2.2, for (u,v)∈K1×K2, we have A1(u,v)(x)>0,A2(u,v)(x)>0,x>0. From Lemma 2.2 and Remark 3.1, for x∈[τλ,τ],τ>0, and λ>1,
So, A1(u,v)(x)1+x−β(1+γ)≥p(τ)η‖A1(u,v)‖X. Similarly, A2(u,v)(x)1+x−β(1+γ)≥p(τ)η‖A2(u,v)‖Y. Therefore,
That is, A:K1×K2→K1×K2 is true.
Second, it will give a simply prove that A is continuous. Let D={(u,v)|(u,v)∈K1×K2,‖(u,v)‖X×Y≤K,K>0}, a bounded subset in K1×K2. Let (un,vn)∈D be a sequence that converges to (u,v) in ∈K1×K2. Then ‖(un,vn)‖X×Y≤K. From Lemma 2.2,
By (H2),
By the continuity of sβ(γ+1)−1F(s,u(s),v(s)) and the Lebesgue dominated convergence theorem,
Therefore, ‖A1(un,vn)−A1(u,v)‖X→0,n→∞. Similarly, ‖A2(un,vn)−A2(u,v)‖Y→0,n→∞.
So, ‖A(un,vn)−A(u,v)‖X×Y→0,n→∞. That is, A is continuous in D. In the end, we know that A(D) is relatively compact on (0,∞) and is equi-convergent at ∞ by [18]. Therefore, A:K1×K2→K1×K2 is completely continuous. □
Theorem 4.1. Assume that (H2) and (H4) hold. If F0=0,G∗0=0,f∞=∞,g∗∞=∞, then the system (1.1) has at least one positive solution.
Proof. We divide the proof into several steps.
Step 1. A:K1×K2→K1×K2 is completely continuous. This result easily follows from Lemma 4.1.
Step 2. We show that there exist R1>0 and D1={(u,v)∈X×Y,‖(u,v)‖X×Y<R1} such that ‖A(u,v)‖X×Y≤‖(u,v)‖X×Y, (u,v)∈(K1×K2)∩∂D1.
Because F0=0,G∗0=0, we choose R1>0, such that
for 0<u+v≤R1,x>0, where ϵ1,ϵ2>0 satisfy
So, for (u,v)∈K1×K2 and ‖(u,v)‖X×Y=R1, by Lemma 2.2,
By (H4),
Similarly,
Therefore,
Let D1={(u,v)∈X×Y,‖(u,v)‖X×Y<R1}. Then,
Step 3. We show that there exist R2>0 and D2={(u,v)∈X×Y,‖(u,v)‖X×Y<R2} such that
Because f∞=∞,g∗∞=∞, there exists R>0, such that
for u+v≥R,x>0, where m1,m2>0 satisfy
Let R2≥max{R1,ηRp(τ)}, and D2={(u,v)∈X×Y,‖(u,v)‖X×Y<R2}. Then, D1⊂D2.
Thus, for (u,v)∈K1×K2, ‖(u,v)‖X×Y=R2, we have
So,
By (H4), for x∈[τλ,τ], we can obtain
Similarly, A2(u,v)(x)1+x−β(1+γ)≥12‖(u,v)‖X×Y. Therefore,
Finally, by Lemma 2.4, A has a fixed point in (K1×K1)∩∂(¯D2∖D1). So, (1.1) has at least one positive solution. □
Theorem 4.2. Assume that (H2) and (H4) hold. If f0=∞,g∗0=∞,F∞=0,G∗∞=0, then (1.1) has at least one positive solution.
Proof. We divide the proof into several steps.
Step 1. A:K1×K2→K1×K2 is completely continuous. This result easily follows from Lemma 4.1.
Step 2. We show that there exist r1>0 and D1={(u,v)∈X×Y,‖(u,v)‖X×Y<r1} such that
Because f0=∞,g∗0=∞, there exists r1>0 such that
for 0<u+v≤r1,x>0, where M1,M2>0, satisfy
Let D1={(u,v)∈X×Y,‖(u,v)‖X×Y<r1}. So, for (u,v)∈K1×K2 with ‖(u,v)‖X×Y=r1, and x∈[τλ,τ], then by (H4),
Similarly, A2(u,v)(x)1+x−β(1+γ)≥12‖(u,v)‖X×Y. Thus,
Step 3. We show that there exist r2>0 and D2={(u,v)∈X×Y,‖(u,v)‖X×Y<r2} such that
Because F∞=0,G∗∞=0, there exists r>0, such that
for u+v>r,x>0, where ϵ1,ϵ2>0 satisfy
Let D2={(u,v)∈X×Y,‖(u,v)‖X×Y<r2}, where r2>max{r1,r}. Then D1⊂D1. We define two functions U1,U2 as follows:
For (u,v)∈K1×K2 and ‖(u,v)‖X×Y=r2,
By Lemma 2.2 and (H4),
Similarly, A2(u,v)(x)1+x−β(1+γ)≤12‖(u,v)‖X×Y. Therefore, ‖A(u,v)‖X×Y≤‖(u,v)‖X×Y, for (u,v)∈(K1×K2)∩∂D2. Finally, by Lemma 2.4, A has a fixed point in (K1×K1)∩∂(¯D2∖D1). So, the system (1.1) has at least one positive solution. □
5.
Multiple positive solutions
In the section, we obtain the multiplicity of positive solution of (1.1) by using the monotone iterative technique.
Theorem 5.1. If (H1) and (H2) hold, then (1.1) has two positive solutions (u∗,v∗) and (w∗,z∗) satisfying 0≤‖(u∗,v∗)‖X×Y≤Υ and 0≤‖(w∗,z∗)‖X×Y≤Υ, where Υ is a positive preset constant. Moreover, limn→∞(un,vn)=(u∗,v∗) and limn→∞(wn,zn)=(w∗,z∗), where (un,vn) and (wn,zn) are given by
with
and
with (w0(x),z0(x))=(0,0). In addition,
Proof. First, from Lemma 4.1, A(K1×K2)⊂K1×K2 for (u,v)∈K1×K2. Let
and Υ≥Υ1+Υ2 with DΥ={(u,v)∈K1×K2:‖(u,v)‖X×Y≤Υ}. For any (u,v)∈DΥ, from (H2) and Lemma 2.2,
Similarly, ‖A2(u,v)‖Y≤Υ2 for (u,v)∈DΥ. Thus,
That is, A(DΥ)⊂DΥ. We construct two sequences as follows:
Obviously, (u0(x),v0(x)),(w0(x),z0(x))∈DΥ. Because A(DΥ)⊂DΥ, (un,vn),(wn,zn)∈DΥ,n=1,2,…. We need to show that there exist (u∗,v∗) and (w∗,z∗) satisfying limn→∞(un,vn)=(u∗,v∗) and limn→∞(wn,zn)=(w∗,z∗) which are two monotone sequences for approximating positive solutions of the system (1.1).
For x∈(0,+∞),(un,vn)∈DΥ, from Lemma 2.2 and (5.1),
and
that is,
So, by the condition (H1),
For x∈(0,+∞), the sequences {(un,vn)}∞n=0 satisfy (un+1(x),vn+1(x))≤(un(x),vn(x)). By the iterative sequences (un+1,vn+1)=A(un,vn) and the complete continuity of the operator A, (un,vn)→(u∗,v∗), and A(u∗,v∗)=(u∗,v∗).
Similarly, for the sequences {(wn,zn)}∞n=0, we have
Then, by the condition (H1),
Analogously, for x∈(0,+∞), we have (wn+1(x),zn+1(x))≥(wn(x),zn(x)). By the iterative sequences (wn+1,zn+1)=A(wn,zn) and the complete continuity of the operator A , (w_{n}, z_{n})\rightarrow (w^{\ast}, z^{\ast}) , and A(w^{\ast}, z^{\ast}) = (w^{\ast}, z^{\ast}) .
Finally, we prove that (u^{\ast}, v^{\ast}) and (w^{\ast}, z^{\ast}) are the minimal and maximal positive solutions of (1.1). Assume that (\varsigma(x), \mu(x)) is any positive solution of (1.1). Then, A(\varsigma(x), \mu(x)) = (\varsigma(x), \mu(x)) , and
Therefore,
That is, (w_{1}(x), z_{1}(x))\leq(\varsigma(x), \mu(x))\leq(u_{n}(x), v_{n}(x)) . So, (5.3) holds. By (H_{1}) , (0, 0) is not a solution of (1.1). From (5.1), (w^{\ast}, z^{\ast}) and (u^{\ast}, v^{\ast}) are two extreme positive solutions of (1.1), which can be constructed via limitS of two monotone iterative sequences in (5.1) and (5.2). □
6.
Examples
Example 6.1. We consider the following system:
where \sigma_{1} = \frac{5}{3}, \sigma_{2} = \frac{3}{2}, \gamma = -\frac{3}{2}, \beta = 1 ,
First, for F_{1}(x, u, v) = x^{\beta(1+\gamma)-1}F(x, (1+x^{-\beta(1+\gamma)})u, (1+x^{-\beta(1+\gamma)})v) = e^{-x}(u^{2}, v^{2}) , we choose \omega_{1}(u) = u^{2}\in C((0, +\infty), (0, +\infty)) , \omega_{2}(v) = v^{2}\in C((0, +\infty), (0, +\infty)) , and \varphi_{1}(x) = \psi_{1}(x) = e^{-x}\in L^{1}(0, +\infty) . Then,
Similarly, for F_{2}(x, u, v) = x^{\beta(1+\gamma)-1}G(x, (1+x^{-\beta(1+\gamma)})u, (1+x^{-\beta(1+\gamma)})v) = xe^{-2x^{2}}[u^{2}\ln(u^{2}+1)+ v^{2}\ln(v^{2}+1)] , we choose \widetilde{\omega_{1}}(u) = u^{2}\ln(u^{2}+1)\in C((0, +\infty), (0, +\infty)) , \widetilde{\omega_{2}}(v) = v^{2}\ln(v^{2}+1)\in C((0, +\infty), (0, +\infty)) , and \varphi_{2}(x) = \psi_{2}(x) = xe^{-2x^{2}}\in L^{1}(0, +\infty) . Then,
So, the condition (H_{2}) holds. Obviously, F, G:(0, +\infty)\times(0, +\infty)\times(0, +\infty)\rightarrow (0, +\infty) are continuous.
where a_{1}(x) = e^{-x}, a_{2}(x) = xe^{-2x^{2}} , F_{1}(x, u, v) = (\frac{u}{1+x^{\frac{1}{2}}})^{2}+(\frac{v}{1+x^{\frac{1}{2}}})^{2} , G_{1}(t, u, v) = (\frac{u}{1+x^{\frac{1}{2}}})^{2}\ln(1+(\frac{u}{1+x^{\frac{1}{2}}})^{2}) +(\frac{u}{1+x^{\frac{1}{2}}})^{2}\ln(1+(\frac{u}{1+x^{\frac{1}{2}}})^{2}) . So, x^{-\frac{3}{2}}f(x, u, v), x^{-\frac{3}{2}}G(x, u, v):[0, +\infty)\times(0, +\infty)\times(0, +\infty)\rightarrow [0, +\infty) are continuous. Hence, the condition (H_{4}) holds. Finally,
Therefore, from Theorem 4.1, (6.1) has at least one positive solution (u(x), v(x)) . Further,
Example 6.2. We consider the following system:
where \sigma_{1} = \frac{3}{2}, \sigma_{2} = \frac{7}{6}, \gamma = -\frac{3}{2}, \beta = 1 ,
First, for
we choose \omega_{1}(u) = \arctan u^{2}+\frac{1}{\pi}\in C((0, +\infty), (0, +\infty)), \omega_{2}(v) = \arctan v^{2}+\pi\in C((0, +\infty), (0, +\infty)) , and \varphi_{1}(x) = \psi_{1}(x) = xe^{-2x^{2}+1}\in L^{1}(0, +\infty) . Then,
Similarly, for
we choose \widetilde{\omega_{1}}(u) = \arctan(\ln(u^{2}+1))+\frac{3}{2}\pi\in C((0, +\infty), (0, +\infty)) , \widetilde{\omega_{2}}(v) = \arctan(\ln(v^{2}+1))+1\in C((0, +\infty), (0, +\infty)) , and \varphi_{2}(x) = \psi_{2}(x) = e^{-x}\in L^{1}(0, +\infty) . Then,
That is, (H_{2}) holds. Second, F, G:(0, +\infty)\times(0, +\infty)\times(0, +\infty)\rightarrow (0, +\infty) are continuous. And
where a_{1}(x) = xe^{-2x^{2}+1}, a_{2}(x) = e^{-x} , F_{1}(x, u, v) = \arctan(\frac{u}{1+x^{\frac{1}{2}}})^{2}+\frac{1}{\pi}+\arctan(\frac{v}{1+x^{\frac{1}{2}}})^{2}+\pi , G_{1}(x, u, v) = \arctan(\ln((\frac{u}{1+x^{\frac{1}{2}}})^{2}+1))+\frac{3}{2}\pi+\arctan(\ln((\frac{v}{1+x^{\frac{1}{2}}})^{2}+1))+1 . So, x^{-\frac{3}{2}}F(x, u, v), x^{-\frac{3}{2}}G(x, u, v):[0, +\infty)\times(0, +\infty)\times(0, +\infty)\rightarrow [0, +\infty) are continuous. That is, (H_{4}) holds. In addition,
Therefore, from Theorem 4.2, (6.2) has at least one positive solution (u(x), v(x)) . Further,
Example 6.3. We consider the following system:
where \sigma_{1} = \frac{5}{3}, \sigma_{2} = \frac{3}{2}, \gamma = -\frac{3}{2}, \beta = 1 ,
Obviously, F, G:(0, +\infty)\times(-\infty, +\infty)\times(-\infty, +\infty)\rightarrow (0, +\infty) are continuous and nondecreasing with respect to the second and the third variables on (0, +\infty) . That is, (H_{1}) holds. Next,
We choose \omega_{1}(u) = \mid u\mid\in C((0, +\infty), (0, +\infty)) , \omega_{2}(v) = \ln(\mid v\mid+1)\in C((0, +\infty), (0, +\infty)) , and \varphi_{1}(x) = \frac{e^{-x}}{3}, \psi_{1}(x) = \frac{xe^{-2x^{2}+1}}{10}\in L^{1}(0, +\infty) . Then,
Similarly, for
we choose \widetilde{\omega_{1}}(u) = \arctan(\mid u\mid+\frac{1}{\sqrt{\pi}})\in C((0, +\infty), (0, +\infty)) , \widetilde{\omega_{2}}(v) = \mid v\mid\in C((0, +\infty), (0, +\infty)) , and \varphi_{2}(x) = xe^{-2x^{2}+1}, \psi_{2}(x) = x\frac{e^{-2x^{2}+1}}{5}\in L^{1}(0, +\infty) . Then,
That is, (H_{2}) holds. Therefore, from Theorem 5.1, (6.3) has two positive solutions (u^{\ast}, v^{\ast}) and (w^{\ast}, z^{\ast}) with (0, 0)\leq (u^{\ast}(x), v^{\ast}(x)), (w^{\ast}(x), z^{\ast}(x))\leq ((1+x^{\frac 12})\Upsilon_{1}, (1+x^{\frac 12})\Upsilon_{2}) , where \Upsilon_{1}+\Upsilon_{2}\leq \Upsilon , and \Upsilon satisfies
7.
Conclusions
This paper studies the Erdélyi-Kober fractional coupled system (1.1), where the variable is in an infinite interval. We give some proper conditions and set a special Banach space. We obtain the existence of at least one positive solution for (1.1) by using the Guo-Krasnosel'skii fixed point theorem, and we get the existence of at least two positive solutions for (1.1) by using the monotone iterative technique. Our methods and results are different from ones in [18]. Moreover, we give three examples to show the plausibility of our main results. For future work, we intend to use other fixed point theorems to solve some Erdélyi-Kober fractional differential equations.
Use of AI tools declaration
The authors declare they have not used artificial intelligence (AI) tools in the creation of this article.
Acknowledgments
This paper is supported by the Fundamental Research Program of Shanxi Province (202303021221068).
Conflict of interest
The authors declare that they have no competing interests.
















 DownLoad:
DownLoad: