1.
Introduction
In the last decades, many researchers have formulated various mathematical models to characterize the human immune system reaction on invading viruses [1,2,3,4,5,6]. The two mean immune system reactions are the cell-mediated immunity and the humoral immunity. The cell-mediated immunity is based on Cytotoxic T Lymphocytes (CTLs) which kill the infected cells, while the humoral immunity is based on antibodies which are produced by B cells and neutralize the free viruses from the plasma. Some existing models describe the virus dynamics under the effect of cell-mediated immune response (see e.g., [7,8,9,10], see also [11] and the references therein) or humoral immune response [12,13,14,15,16,17]. Wodarz [18] has formulated a virus dynamics model with five compartments; susceptible cells (S), infected cells (I), virus particles (V), B cells (A) and CTL cells (B) as:
The model has been extended in [19,20,21,22,23], but with virus-to-cell transmission. Cell-to-cell infection plays an important role in increasing the number of infected cells. Mathematical models of virus dynamics with both virus-to-cell and cell-to-cell transmissions have been studied in several works (see e.g., [24,25,26,27,28,29,30,31,32,33,34]). In very recent works [35], both CTL cells and B cells have been incorporated into the viral infection models with both cell-to-cell and virus-to-cell transmissions. However, in [35], only one class of infected cells (actively infected cells) is considered. It has been reported in [36] and [37] that the time from the contact of viruses and susceptible cells to the death of the cells can be modeled by dividing the process into n short stages I1→I2→....→In. In [38], virus dynamics models with multi-staged infected cells, humoral immunity and with only virus-to-cell infection have been studied.
The aim of the present paper is to formulate a virus dynamics model by incorporating (ⅰ) multi-staged infected cells, (ⅱ) both cell-mediated and humoral immune responses (ⅱ) both cell-to-cell and virus-to-cell infections as:
where, Ik, k=1,2,...,n represents the concentration of the i-th stage of infected cells. The model assumes that the susceptible cells are infected by virus particles at rate η1S(t)V(t) and by infected cells at rate η2S(t)In(t).
2.
Well-Posedness of solutions
Let Ωj>0, j=1,2,...,n+3 and define
Proposition 1. The compact set Θ is positively invariant for system (1.2).
Proof. We have
This insures that, S(t)>0, Ik(t)≥0, k=1,...,n, V(t)≥0, A(t)≥0, and C(t)≥0 for all t≥0.
To show the boundedness of S(t) and I1(t) we let Ψ1(t)=S(t)+I1(t), then
where ϕ1=min{α,b1}. It follows that,
Hence, 0≤Ψ1(t)≤Ω1 if Ψ1(0)≤Ω1 for t≥0, where Ω1=ρϕ1. Since S(t)>0 and I1(t)≥0, then 0≤S(t),I1(t)≤Ω1 if S(0)+I1(0)≤Ω1. From the fourth equation of system (1.2) in case of k=2, we have
It follows that, 0≤I2(t)≤Ω2 if I2(0)≤Ω2, where Ω2=d1Ω1b2. Similarly, we can show0≤Ik(t)≤Ωk if Ik(0)≤Ωk, where Ωk=dk−1Ωk−1bk, k=3,...,n−1. Further, we let Ψ2(t)=In(t)+μσC(t), then
where ϕ2=min{bn,π}. It follows that, 0≤Ψ2(t)≤Ωn if Ψ2(0)≤Ωn, where Ωn=dn−1Ωn−1ϕ2. Since In(t)≥0 and C(t)≥0, then 0≤In(t)≤Ωn and 0≤C(t)≤Ωn+1 if In(0)+μσC(0)≤Ωn, where Ωn+1=σμΩn. Finally, let Ψ3(t)=V(t)+γτA(t), then
where ϕ3=min{ε,ζ}. It follows that, 0≤Ψ3(t)≤Ωn+2 if Ψ3(0)≤Ωn+2, where Ωn+2=dnΩnϕ3. It follows that, 0≤V(t)≤Ωn+2 and 0≤A(t)≤Ωn+3 if V(0)+γτA(0)≤Ωn+2, where Ωn+3=τγΩn+2.
3.
Threshold parameters and equilibria
In this section, we derive five threshold parameters which guarantee the existence of the equilibria of the model.
Lemma 1. System (1.2) has five threshold parameters ℜ0>0, ℜA1>0, ℜC1>0, ℜC2>0 and ℜA2>0 with ℜC1<ℜ0 such that
(ⅰ) if ℜ0≤1, then there exists only one steady state Ɖ0,
(ⅱ) if ℜA1≤1 and ℜC1≤1<ℜ0, then there exist only two equilibria Ɖ0 and ˉƉ,
(ⅲ) if ℜA1>1 and ℜC2≤1, then there exist only three equilibria Ɖ0, ˉƉ and ˆƉ,
(ⅳ) if ℜC1>1 and ℜA2≤1, then there exist only three equilibria Ɖ0, ˉƉ and ∨Ɖ, and
(ⅴ) if ℜA2>1 and ℜC2>1, then there exist five equilibria Ɖ0, ˉƉ, ˆƉ, ∨Ɖ and ˜Ɖ.
Proof. Let (S,I1,...,In,V,A,C) be any equilibrium of system (1.2) satisfying the following equations:
We find that system (1.2) admits five equilibria.
(ⅰ) Infection-free equilibrium Ɖ0=(S0,n+3⏞0,...,0,0), where S0=ρ/α.
(ⅱ) Chronic-infection equilibrium with inactive immune response ˉƉ=(ˉS,ˉI1,...,ˉIn,ˉV,0,0), where
Therefore, ˉƉ exists when
At the equilibrium ˉƉ the disease persists while the immune response is inhibited. The basic infection reproductive ratio for system (1.2) is defined as:
The parameter ℜ0 determines whether the disease will progress or not. In terms of ℜ0, we can write
(ⅲ) Chronic-infection equilibrium with only active humoral immune response ˆƉ=(ˆS,ˆI1,...,ˆIn,ˆV,ˆA,0), where
where
is the positive solution of
with
We note that ˆƉ exists when dnτεζˆIn>1. Let us define the active humoral immunity reproductive ratio
which determines when the humoral immune response is activated. Thus, ˆA=εγ(ℜA1−1).
(ⅳ) Chronic-infection equilibrium with only active cell-mediated immune response ∨Ɖ=(ˇS,ˇI1,...,ˇIn,ˇV,0,ˇC), where
We note that ∨Ɖ exists when σρ(η1dn+η2ε)dn[π(η1dn+η2ε)+αεσ](n∏i=1dibi)>1. The active cell-mediated immunity reproductive ratio is stated as:
The parameter ℜC1 determines when the cell-mediated immune response is activated. Thus, ˇC=bnμ(ℜC1−1) and ℜC1<ℜ0.
(ⅴ) Chronic-infection equilibrium with both active humoral and cell-mediated immune responses ˜Ɖ=(˜S,˜I1,...,˜In,˜V,˜A,˜C), where
It is obvious that ˜Ɖ exists when σρ(η1ζσ+η2τπ)dnπ[ατσ+η1ζσ+η2τπ](n∏i=1dibi)>1 and dnπτεσζ>1. Now we define
where ℜC2 refers to the competed cell-mediated immunity reproductive ratio and appears as the average number of T cells activated due to infectious cells in the scene that the humoral immune response has been constructed, while, ℜA2 refers to the competed humoral immunity reproductive ratio and appears as the average number of B cells activated due to mature viruses in the scene that the cell-mediated immune response has been constructed. Clearly, ˜Ɖ exists when ℜC2>1 and ℜA2>1 and we can write ˜C=bnμ(ℜC2−1) and ˜A=εγ(ℜA2−1).
The five threshold parameters are given as follows:
We define the active humoral immunity reproductive ratio ℜAhumoral which comes from the limiting (linearized) A-dynamics near A=0 as:
Lemma 2. (ⅰ) if ℜA1<1, then ℜAhumoral<1,
(ⅱ) if ℜA1>1, then ℜAhumoral>1,
(ⅲ) if ℜA1=1, then ℜAhumoral=1,
Proof. (ⅰ) Let ℜA1<1, then from Eq. 3.10 we have ˆIn<εˆVdn. Then, using Eq. 3.8 we get
which leads to
Using Eq. 3.9 we derive
Thus, ℜAhumoral<1. Using the same argument one can easily confirm part (ⅱ) and (ⅲ).
4.
Global stability analysis
The global stability of the each equilibria will be investigated by constructing Lyapunov functions using the method presented [39,40,41,42,43,44,45]. Let us define the function ϝ:(0,∞)→[0,∞) as ϝ(υ)=υ−1−lnυ. Denote (S,I1,...,In,V,A,C)=(S(t),I1(t),...,In(t),V(t),A(t),C(t)). The following equalities will be used:
where I∗∈{ˉI,ˆI,ˇI,˜I}.
Theorem 1. If ℜ0≤1, then the infection-free equilibrium Ɖ0 is globally asymptotically stable.
Theorem 2. Suppose that ℜA1≤1 and ℜC1≤1<ℜ0, then the chronic-infection equilibrium with inactive immune response ˉƉ is globally asymptotically stable.
Theorem 3. If ℜA1>1 and ℜC2≤1, then the chronic-infection equilibrium with only active humoral immune response ˆƉ is globally asymptotically stable.
Theorem 4. Suppose that ℜC1>1 and ℜA2≤1, then the chronic-infection equilibrium with only active cell-mediated immune response ∨Ɖ is globally asymptotically stable.
Theorem 5. If ℜA2>1 and ℜC2>1, then the chronic-infection equilibrium with both active humoral and cell-mediated immune responses ˜Ɖ is globally asymptotically stable.
The proofs of Theorems 1–5 are given in a Supplementary.
5.
Numerical simulations
In this section, we perform some numerical simulations in case of three stages of infected cells i.e. n=3.
The threshold parameters ℜ0, ℜA1, ℜC1, ℜC2, and ℜA2 for system (5.1) are given by:
where
Table 1 contains the values of the parameters of model (5.1).
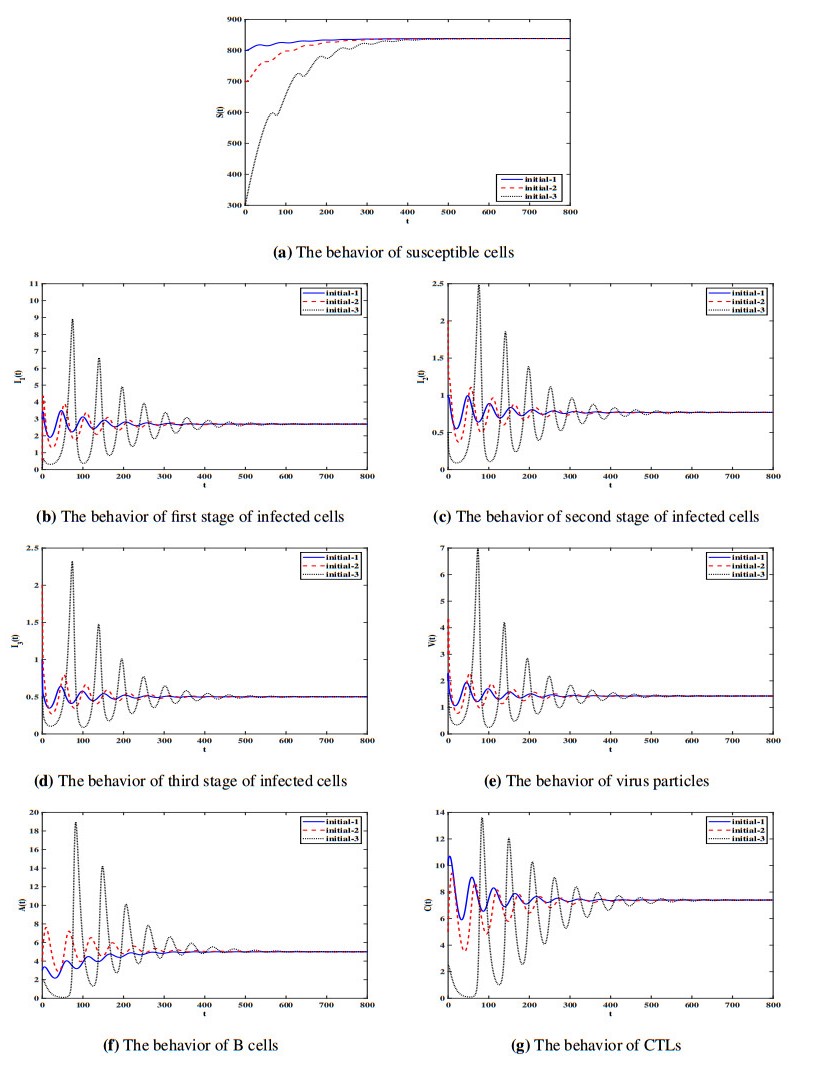
The results of Theorems 1–5 will be investigated by choosing the values of η1, η2, τ and σ under three different initial conditions for model (5.1) as follows:
Initial–1: (S(0),I1(0),I2(0),I3(0),V(0),A(0),C(0))=(800,3,1,1,2,3,10), (Solid lines in the figures)
Initial–2: (S(0),I1(0),I2(0),I3(0),V(0),A(0),C(0))=(700,0.5,2,2,3,4,5), (Dashed lines in the figures)
Initial–3: (S(0),I1(0),I2(0),I3(0),V(0),A(0),C(0))=(300,0.1,0.5,0.5,1.5,2,2.5). (Dotted lines in the figures)
Stability of Ɖ0: η1=η2=0.0001,
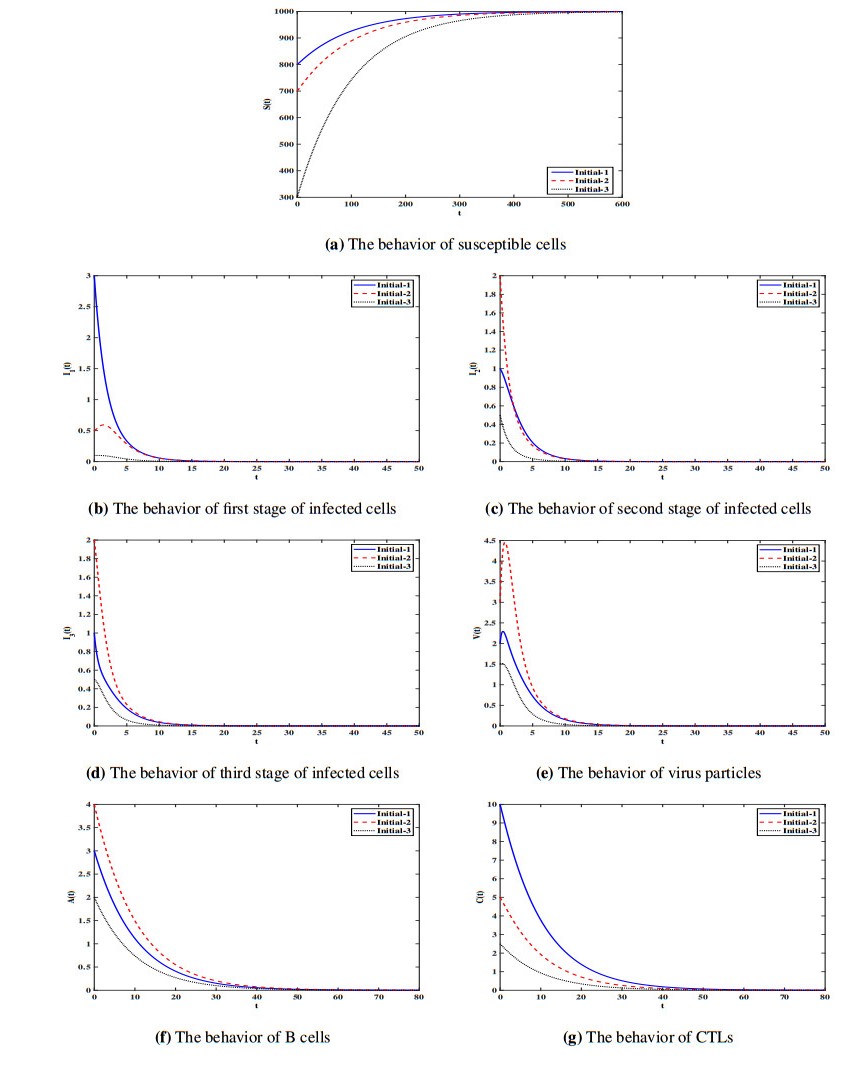
τ=0.001 and σ=0.01. For this set of parameters, we have ℜ0=0.26<1, ℜA1=0.10<1, ℜC1=0.18<1 and ℜC2=0.31<1. Figure 1 illustrates that the solution trajectories starting from different initial conditions reach the equilibrium Ɖ0=(1000,0,0,0,0,0,0). This ensures that Ɖ0 is globally asymptotically stable according to the result of Theorem 1. In this situation the viruses will be died out.
Stability of ˉƉ: η1=η2=0.001,
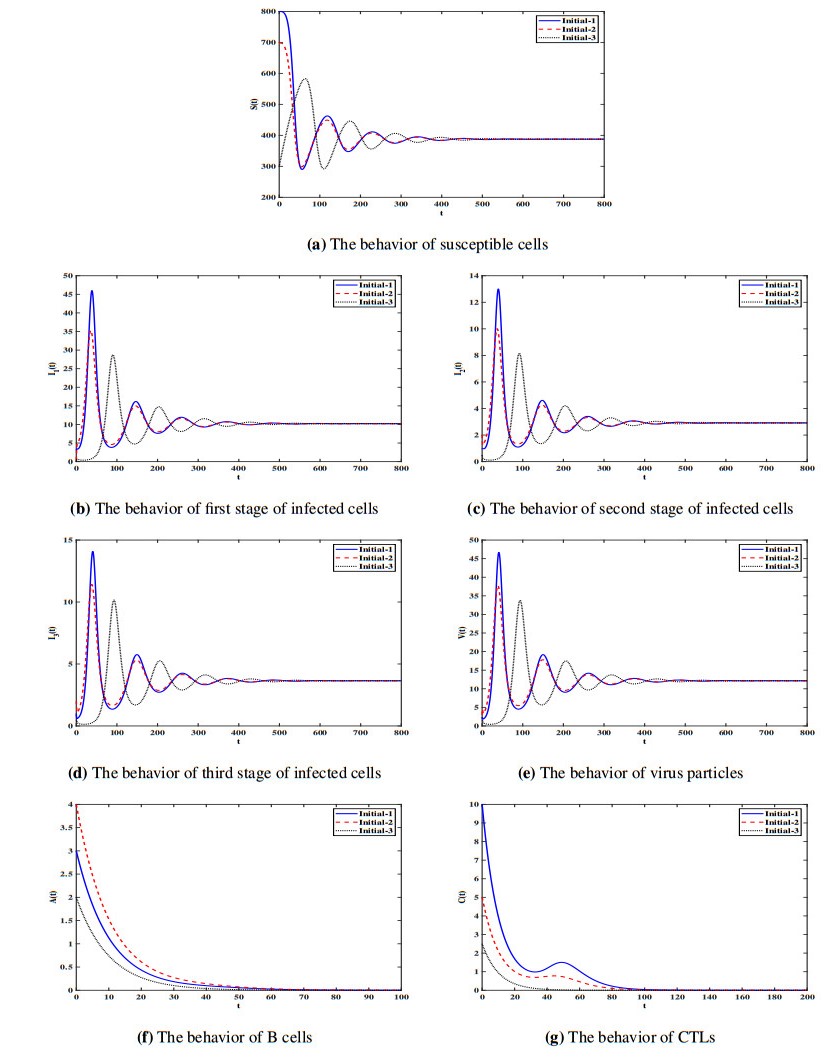
τ=0.001 and σ=0.01. With such choice we get, ℜA1=0.18<1 and ℜC1=0.48<1<ℜ0=2.58 and ˉƉ exists with ˉƉ=(387.68,10.21,2.92,3.65,12.15,0,0). Thus, Lemma 1 is verified. Figure 2 shows that the solution trajectories starting from different initial conditions tend to ˉƉ and this support Theorem 2. This case represents the persistence of the viruses but with inhibited humoral and cell-mediated immune responses.
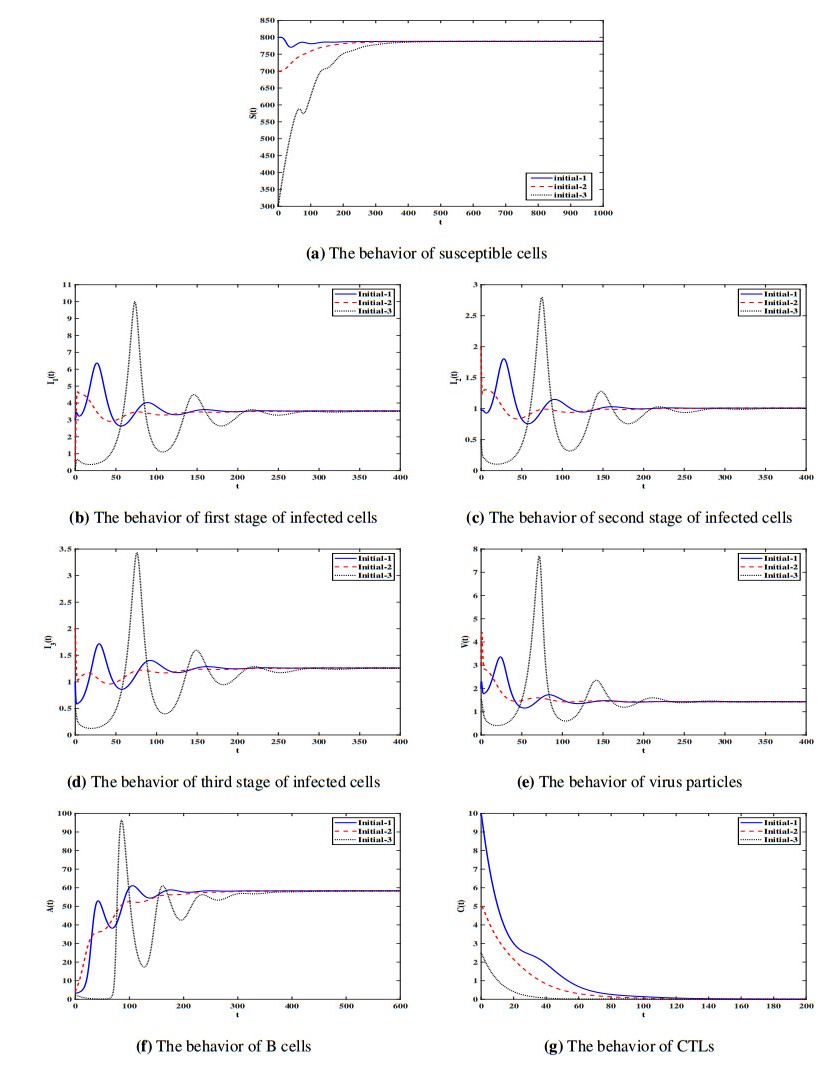
Stability of ˆƉ: η1=η2=0.001, τ=0.07 and σ=0.05. Then, we calculate ℜ0=2.58>1, ℜA1=2.94>1 and ℜC2=0.76<1. The numerical results show that ˆƉ=(787.99,3.53,1.01,1.26,1.43,58.34,0) which confirm Lemma 1. The global stability result given in Theorem 3 is illustrated by Figure 3. This situation represents the case when the infection is chronic and the humoral immune response is active, while the cell-mediated immune response is inhibited.
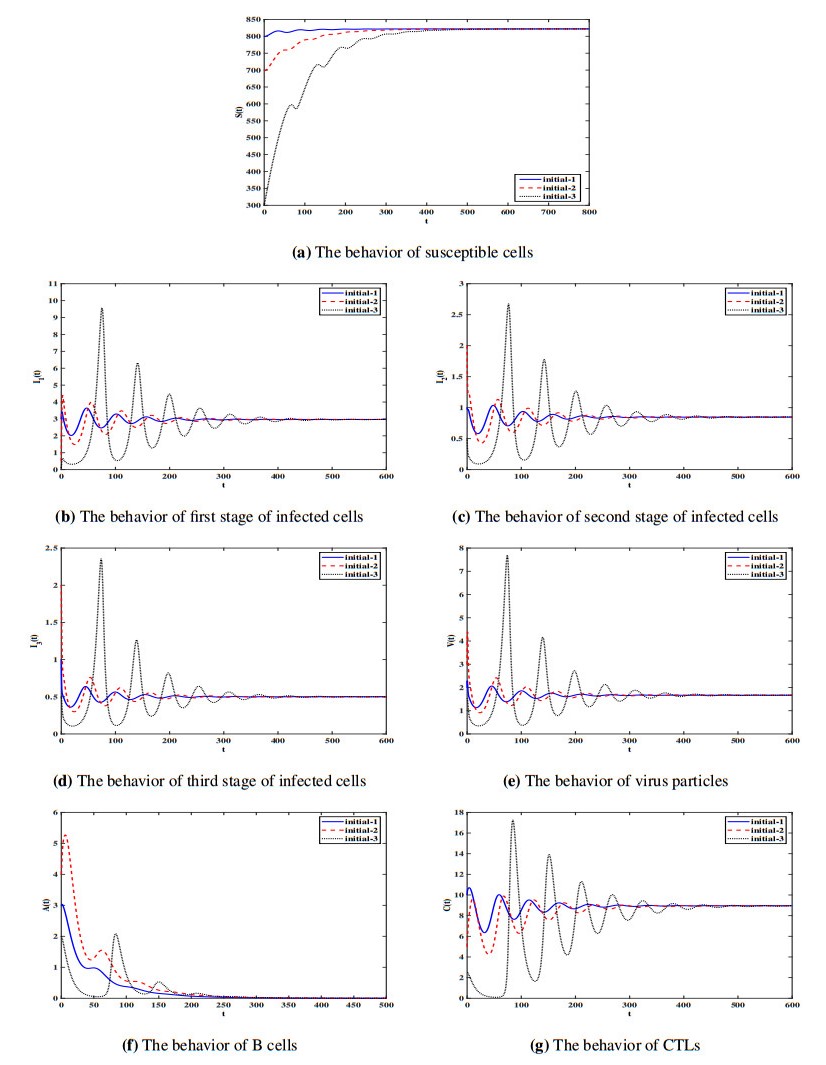
Stability of ∨Ɖ: η1=η2=0.001,
τ=0.05 and σ=0.2. Then, we calculate ℜ0=2.58>1, ℜC1=2.12>1 and ℜA2=0.83<1. The results presented in Lemma 1 and Theorem 4 show that the equilibrium ∨Ɖ exists and it is globally asymptotically stable. Figure 4 supports the results of Theorem 4, where the solution trajectories of the system starting from different initial conditions reach the equilibrium point ∨Ɖ
=(821.91,2.97,0.85,0.50,1.67,0,8.96). This situation represents the case when the infection is chronic and the cell-mediated immune response is active, while the humoral immune response is inhibited.
Stability of ˜Ɖ: η1=η2=0.001, τ=0.07 and σ=0.2. Then, we calculate ℜ0=2.58>1 and ℜA2=1.17>1, ℜC2=1.92>1. The numerical results show that ˜Ɖ=(838.32,2.69,0.77,0.50,1.43,5.00,7.40) which ensure Lemma 1. Moreover, the global stability result given in Theorem 5 is demonstrated in Figure 5. It can be seen that the solution trajectories of the system starting from different initial conditions converge to the equilibrium ˜Ɖ. This situation represents the case when the infection is chronic and both immune responses are active.
5.1. Comparison results
We consider system (5.1) under the effect of two types of treatment as:
where, the parameter ϵ1∈[0,1] is the efficacy of antiretroviral therapy in blocking infection by virus-to-cell mechanism, and ϵ2∈[0,1] is the efficacy of therapy in blocking infection by cell-to-cell mechanism [47].
The basic reproduction number of system (5.2) is given by
where
When the cell-to-cell transmission is neglected, system (5.2) leads to the following system:
The basic reproduction number of system (5.3) is given by
Without loss of generality we let ϵ1=ϵ2=ϵ. Now we calculate the minimum drug efficacy ϵ which stabilize the infection-free equilibrium for systems (5.2) and (5.3). For system (5.2) one can determine the minimum drug efficacy ϵmin(5.2) such that ℜ0,(5.2)(ϵ)≤1 for all ϵmin(5.2)≤ϵ≤1 as:
For system (5.3) the minimum drug efficacy ϵmin(5.3) such that ℜ0,(5.3)(ϵ)≤1, ϵmin(5.3)≤ϵ≤1 is given by:
Comparing Eqs. (5.5) and (5.4) we get that ϵmin(5.3)≤ϵmin(5.2). Therefore, if we apply drugs with ϵ such that ϵmin(5.3)≤ϵ<ϵmin(5.2), this guarantee that ℜ0,(5.3)(ϵ)≤1 and then Ɖ0 of system (5.3) is globally asymptotically stable, however, ℜ0,(5.2)>1 and then Ɖ0 of system (5.2) is unstable. Therefore, more accurate drug efficacy ϵ is determined when using the model with both virus-to-cell and cell-to-cell transmissions. This shows the importance of considering the effect of the cell-to-cell transmission in the virus dynamics.
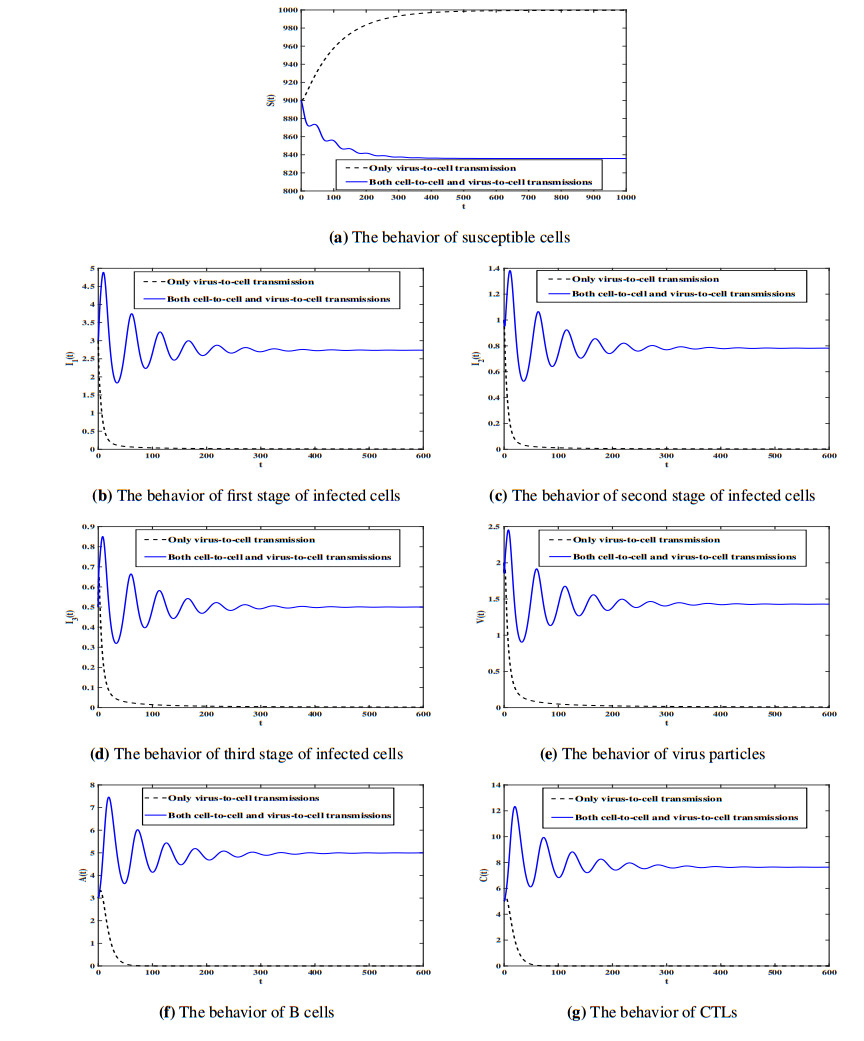
Now we perform numerical simulation for both systems (5.2) and (5.3). Using the values given in Table 1 and choosing η1=0.001, η2=0.005, τ=0.07 and σ=0.2. Then we get
Now we select ϵ=0.5 and choose the initial condition as follows:
Initial–4: (S(0),I1(0),I2(0),I3(0),V(0),A(0),C(0))=(900,3,1,0.5,2,3,5).
From Figure 6 we can see that the trajectory of model (5.3) tends to Ɖ0, while the trajectory of model (5.2) tends to ˜Ɖ. It means that if one design treatment using model (5.3) where the cell-to-cell transmission is neglected, then this treatment will not suffice to clear the viruses from the body.
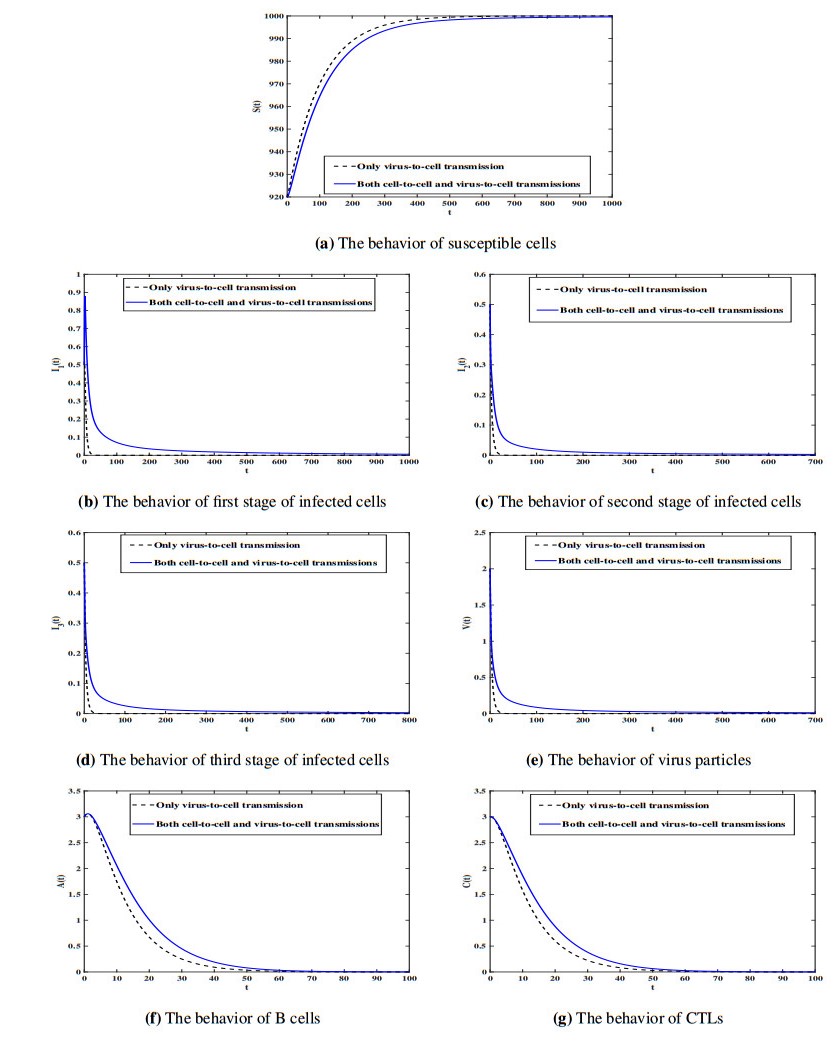
On the other hand, we choose ϵ=0.8 and consider the following initial condition:
Initial–5: (S(0),I1(0),I2(0),I3(0),V(0),A(0),C(0))=(920,0.5,0.5,0.5,2,3,3).
From Figure 7 we can see that the trajectories of both systems (5.2) and (5.3) tend to Ɖ0. Therefore, this treatment will suffice to clear the viruses from the body.
6.
Conclusion and discussion
In this paper, we formulated and analyzed a virus dynamics model with both CTL and humoral immune responses. We incorporated both virus-to-cell and cell-to-cell transmissions. We assumed that the infected cells pass through n stages to produce mature viruses. We showed that the solutions of the system are nonnegative and bounded, which ensures the well-posedness of the proposed model. Further, we obtained five threshold parameters, ℜ0 (the basic infection reproductive ratio), ℜA1 (the active humoral immunity reproductive ratio), ℜC1 (the active cell-mediated immunity reproductive ratio), ℜC2 (the competed cell-mediated immunity reproductive ratio), and ℜA2 (the competed humoral immunity reproductive ratio). The global asymptotic stability of the five equilibria Ɖ0, ˉƉ, ˆƉ, ∨Ɖ, ˜Ɖ was investigated by constructing Lyapunov functions and applying LaSalle's invariance principle. To support our theoretical results, we conducted some numerical simulations. We note that the incorporation of cell-to-cell transmission mechanism into the viral infection model increases the basic reproduction number ℜ0, since ℜ0=ℜ01+ℜ02>ℜ01. Therefore, neglecting the cell-to-cell transmission will lead to under-evaluated basic reproduction number. Model with two types of treatment was presented. We showed that more accurate drug efficacy which is required to clear the virus from the body is calculated by using our proposed model.
There are some factors that can extend our model (1.2):
a. The infected cells may begin to present the viral antigen earlier than when they reach the terminal stage n (i.e. at stage m where m≤n). Therefore, infected cells Im, Im+1,...,In are subject to be targeted by the CTL immune response.
b. Model (1.2) is formulated by assuming that the virus is purely lytic, that is, only the bursting cells are capable of releasing the free virions. However, many viruses are somewhat mixed, in the sense that they are partially lytic and partially budding, where the release of free virions can be from the infected cells Im, Im+1,...,In.
c. The cell-to-cell infection mechanism can also be expanded to the contact between susceptible cells with infected cells Im, Im+1,...,In.
d. The loss of virions upon the infection could also be added to the model. In fact, there is some speculation that the virions may be indiscriminately entering not only the susceptible cells, but also the cells that are already infected [26,53].
Then, taking into account the above factors will leads to the following model:
where, n∑k=mηkSIk represent the incidence rates due to the contact of the infected cells Im,Im+1,...,In with susceptible cells. The term ˉη1SV is the loss of virus upon entry of a susceptible cell. The term Vn∑k=1ϰkIk represents the absorption of free virions into already infected cells I1,I2,...,In. The production rate of the viruses and the activation rate of the CTL cells are modeled by n∑k=mδkIk and n∑k=mσkCIk, respectively. The k-stage infected cells Ik, are attacked by CTL cells at rate μkCIk, k=m,m+1,...,n. Analysis of system (6.1) is not straightforward, therefore we leave it for future works.
It is commonly observed that in viral infection processes, time delay is inevitable. Herz et al. [59] formulated an HIV infection model with intracellular delay and they obtained the analytic expression of the viral load decline under treatment and used it to analyze the viral load decline data in patients. Several viral infection models presented in the literature incorporated discrete delays (see, e.g., [36] and [44]) or distributed delays (see, e.g., [7,23] and [48,49,50]). In these papers, the global stability of equilibria was proven by utilizing global Lyapunov functional that was motivated by the work in [51] and [52]. Model (6.1) can be extended to incorporate distributed time delays. Moreover, considering age structure of the infected class or diffusion in the virus dynamics model will lead to PDE model [54,55,56,57,58]. These extensions require more investigations, therefore we leave it for future works.
Acknowledgements
This project was funded by the Deanship of Scientific Research (DSR), King Abdulaziz University, Jeddah, under grant No. (DG–14–247–1441). The authors, therefore, gratefully acknowledge the DSR technical and financial support.
Conflict of interest
There is no conflicts of interest.
Supplementary
Proof of Theorem 1. Constructing a Lyapunov function:
It is seen that, Φ0(S,I1,...,In,V,A,C)>0 for all S,I1,...,In,V,A,C>0, and Φ0 has a global minimum at Ɖ0. We calculate dΦ0dt along the solutions of model (1.2) as:
Using (4.3), we have
Then,
Using S0=ρ/α, we obtain
Therefore, dΦ0dt≤0 for all S,In,A,C>0 with equality holding when S(t)=S0 and In(t)=A(t)=C(t)=0 for all t. Let Υ0={(S(t),I1(t),...,In(t),V(t),A(t),C(t)):dΦ0dt=0} and Υ′0 is the largest invariant subset of Υ0. We note that, the solutions of system (1.2) are confined to Υ0 [46]. The set Υ0 is invariant and contains elements which satisfy In(t)=0. Then, ˙In(t)=0 and from Eq. 3.4 we have
It follows that, In−1(t)=0 for all t. Since we have In−1(t)=0, then ˙In−1(t)=0 and from Eq. 3.3, we have ˙In−1(t)=dn−2In−2=0 which yields In−2(t)=0. Consequently, we obtain Ik(t)=0, where k=1,...,n. Moreover, since S(t)=S0 we have ˙S(t)=0 and Eq. 3.1 implies that
which insures that V(t)=0. Noting that ℜ0≤1, then Ɖ0 is globally asymptotically stable using LaSalle's invariance principle.
Proof of Theorem 2. Let us define a function Φ1(S,I1,...,In,V,A,C) as:
Calculating dΦ1dt as:
Collecting terms of Eq. 6.5 and using Eqs. 4.2 and 4.3, we derive
Using the equilibrium conditions for ˉƉ:
We obtain
Since we have
then
Also we have when k=n,
Therefor Eq. 6.7 becomes
Since the arithmetical mean is greater than or equal to the geometrical mean, then
From Lemma 2 we have ˉV<ˆV and since ℜC1≤1<ℜ0 then dΦ1dt≤0 for all S,Ik,V,A,C>0 with equality holding when S(t)=ˉS, Ik(t)=ˉIk, k=1,2,...,n, V(t)=ˉV, and A(t)=C(t)=0 for all t. It can be easily verified that Υ′1={ˉƉ} is the largest invariant subset of Υ1={(S(t),I1(t),...,In(t),V(t),A(t),C(t)):dΦ1dt=0}[46]. Then, ˉƉ is globally asymptotically stable using LaSalle's invariance principle.
Proof of Theorem 3. The candidate Lyapunov function is
We calculate dΦ2dt as:
Collecting terms of Eq. 6.10 and using Eqs. 4.2 and 4.3, we derive
Using the equilibrium conditions for ˆƉ:
We obtain
Using Eq. 6.12 in case of k=n we get
Thus, Eq. 6.13 will become
Eq. 6.14 can be written as
Thus, if ℜC2≤1, then ˜Ɖ dose not exist since ˜C=bnμ(ℜC2−1)≤0. This guarantee that ˙C(t)=σ(In(t)−πσ)C(t)=σ(In(t)−˜In)C(t)≤0 for all C>0, which implies that ˆIn<˜In. Hence dΦ2dt≤0 for all S,Ik,V,A,C>0 with equality holding when S(t)=ˆS, Ik(t)=ˆIk, k=1,2,...,n, V(t)=ˆV, and C(t)=0 for all t. We note that, the solutions of system (1.2) are tend to Υ′2 the largest invariant subset of Υ2={(S(t),I1(t),...,In(t),V(t),A(t),C(t)):dΦ2dt=0} [46]. For each element of Υ′2 we have In(t)=ˆIn, V(t)=ˆV, then ˙V(t)=0 and from Eq. 3.5 we have
which gives A(t)=ˆA. Therefore, Υ′2={ˆƉ}. Applying LaSalle's invariance principle we get ˆƉ is globally asymptotically stable.
Proof of Theorem 4. Define a function Φ3(S,I1,...,In,V,A,C) as:
We calculate dΦ3dt as:
Collecting terms of Eq. 6.16 and using Eqs. 4.2 and 4.3, we derive
Using the equilibrium conditions for ∨Ɖ:
we obtain
Since we have in case of k=n:
Then,
Hence, if ℜA2=τˇVζ≤1, then dΦ3dt≤0 for all S,Ik,V,A,C>0 with equality holding when S(t)=ˇS, Ik(t)=ˇIk, k=1,2,...,n, V(t)=ˇV and A(t)=0 for all t. It can be easily verified that the largest invariant subset of Υ3={(S(t),I1(t),...,In(t),V(t),A(t),C(t)):dΦ3dt=0} is Υ′3={∨Ɖ} [46]. Applying LaSalle's invariance principle we get that ∨Ɖ is globally asymptotically stable.
Proof of Theorem 5. Define Φ4(S,I1,...,In,V,A,C) as:
Calculating dΦ4dt as:
Collecting terms of Eq. 6.20 and using Eqs. 4.2 and 4.3, we obtain
Using the equilibrium conditions for ˜Ɖ:
We obtain
Since we have
and
Then, Eq. 6.22 will be reduced to the form
Hence, dΦ4dt≤0 for all S,Ik,V,A,C>0 with equality holding when S(t)=˜S, Ik(t)=˜Ik, k=1,2,...,n, and V(t)=˜V for all t. It can be easily verified that the largest invariant subset of Υ4={(S(t),I1(t),...,In(t),V(t),A(t),C(t)):dΦ3dt=0} is Υ′4={˜Ɖ} [46]. LaSalle's invariance principle implies that ˜Ɖ is globally asymptotically stable.









 DownLoad:
DownLoad: