1.
Introduction
We initiate our study by the conception of concavity of functions which is stated as follows: Any function E:[σ1,δ1]→R is referred to as a convex function if,
where κ∈[0,1]. Due to the several important geometrical and analytical aspects, convex functions are discussed from multiple approaches and directions in the literature. They have an immense amount of applications and generalizations to investigate non-convex problems. Their role in the emergence of inequalities is unprecedented because this concept itself is closely linked with inequalities. One can easily relate the that almost fundamental results in the theory of inequalities having a governing role can be achieved directly or indirectly by considering the convex functions. Now we provide a significant consequence of convex functions:
Let E:[σ1,δ1]→R be a convex function. Then,
From this inequality, one can conclude that this result computes the bounds for the average mean value integral of convex functions. Moreover, this result serves as a criteria to discuss the concavity of functions. Also, this result has several applications in the theory of means, special functions, error analysis probability theory, information theory, etc. Due to the effective range of implications, several variants of trapezium-type inequalities have been developed and assessed through various classes of convex functions along with applications. For recent developments, consult [1,2,3,4,5,6].
Furthermore, we recover the essential prelude relative to interval analysis to attain the required result. Suppose that the space of all non-empty compact intervals and positive non-degenerate compact intervals of the real line R are specified by RI and R+I. For any A,A2∈RI such that A1=[σ1⋆,σ1⋆] and A2=[δ1⋆,δ1⋆] and λ∈R, then
and
The concept of generalized Hukuhara(gh) difference was developed in [7].
Let A1,A2∈RI. The gh difference is illustrated as:
Also, for A1∈RI, the length of interval is computed by w(A1)=σ1⋆−σ1⋆. Then, for all A1,A2∈RI, we have
Some properties linked with the gH-difference are described as follows:
(1) A1⊖gA1={0},A1⊖g{0}=A1,{0}⊖gA1=−A1,
(2) A1⊖gA2=(−A2)⊖g(−A1)=−(A2⊖gA1),
(3) A1⊖g(−A2)=A2⊖g(−A1)=−(A2⊖gA1),
(4) (A1+A2)⊖gA2=A1,
(5) λA1⊖gλA2=λ(A1⊖gA2).
For any A1,A2,C,D∈RI, consider κ1=w(A1)−w(C),κ2=w(A2)−w(D),κ3=w(A1)−w(A2), and κ4=w(C)−w(D). Then:
(1) (A1+A2)⊖g(C+D)={(A1⊖gC)+(A2⊖gD),κ1κ2≥0,(A1⊖gC)⊖g(−A2⊖gD),κ1κ2<0.
(2) (A1⊖gA2)+(C⊖gD)={(A1⊖g(−C))⊖g(A2⊖g(−D)),κ1κ2≥0,κ3κ4<0,(A1⊖g(−C))+(−A2⊖gD),κ1κ2<0,κ3κ4<0,(A1+C)⊖g(A2+D),κ3κ4≥0.
(3) (A1⊖gA2)⊖g(C⊖gD)={(A1⊖gC)⊖g(A2⊖gD),κ1κ2≥0,κ3κ4≥0,(A1⊖gC)+(−(A2⊖gD)),κ1κ2<0,κ3κ4≥0,(A1+(−C))⊖g(A2+(−D)),κ3κ4<0.
Any function E:[σ1,δ1]→RI is regarded as an I.V function if E(ϱ)=[E⋆(ϱ),E⋆(ϱ)] such that E⋆(ϱ)≤E⋆(ϱ),∀ϱ∈[σ1,δ1]. It is crucial to understand that limϱ→ϱ0E(ϱ) exist ⇔ limϱ→ϱ0E⋆(ϱ) and limϱ→ϱ0E⋆(ϱ).
Additionally, an I.V function E is assumed to be continuous ⇔ both E⋆ and E⋆ are continuous. Presume that E,g:[σ1,δ1]→RI. Then, (E⊖gg)(ϱ)=E(ϱ)⊖gg(ϱ):[σ1,δ1]→RI, and
exists, if limϱ→ϱ0E(ϱ)=A and limϱ→ϱ0g(ϱ)=δ1.
One can notice that if E,g:[σ1,δ1]→RI obey the continuous property of functions, then E⊖gg is referred to as a continuous function.
Consequently, we come up with differentiability concepts based on the ⊖g difference.
Definition 1.1. Presume that E:[σ1,δ1]→R is an I.V function. Then,
is termed as ⊖g derivative at ϱ∈[σ1,δ1]. E is differentiable on (σ1,δ1) if it is differentiable almost everywhere in the domain.
Now, we discuss the concept of I.V convex functions.
Definition 1.2. Let E:[σ1,δ1]→R be an I.V function satisfying E(ϱ)=[E⋆(ϱ),E⋆(ϱ)]. Then, it is considered as an I.V convex, if
The next result ensures the convexity of functions in the interval domain.
Theorem 1.1. Let E:[σ1,δ1]→R be an I.V function such that E(ϱ)=[E⋆(ϱ),E⋆(ϱ)]. Then, E is an I.V convex function ⇔ E⋆ is a convex function and E⋆ is a concave function.
The I.V analogues of the trapezium inequality through containments ordering relation is given as follows:
Let E:[σ1,δ1]→R+I be an I.V convex function. Then,
For, comprehensive review, see [8].
q-Symmetric Calculus. Throughout the investigation, let I=[σ1,δ1] be any arbitrary subset of R such that 0∈I and q∈(0,1). Then, the q-geometric set is expressed as Iq={qϱ|ϱ∈I}. Taking the benefit of this set, the classical symmetric quantum difference operator and left and right quantum symmetric derivatives are defined as follows:
Definition 1.3 ([9]). Let E:I→R. Then the symmetric quantum variant derivative operator is
And, DsqE(0)=E′(0), where κ=0 provided that E is differentiable at κ=0. If E is a differentiable function at κ∈Iq, then limq→1DsqE(κ)=E′(κ).
The quantum symmetric number is described as
Recently, Bilal et al. [10] proposed the conception of quantum symmetric operators over finite intervals. Presume that I=[σ1,δ1]⊂R, 0∈I and 0<q<1. Then the left sided quantum symmetric derivative is given as
Definition 1.4 ([10]). Presume that E:J→R is a continuous function. Then
And, σ1DsqE(σ1)=limq→1σ1DsqE(κ), if the limit exists. If σ1=0, then σ1DsqE=DsqE.
Consequently, the corresponding integral operator is stated as
Definition 1.5. Presume that E:J→R is a continuous function. Then
If σ1=0, then it coincides with the symmetric quantum integrals operator in [9]. For more detail concerning quantum symmetric differences, see [11].
Definition 1.6 ([12]). Let E:I→R be a continuous function. Then,
And, δ1DsqE(δ1)=limq→1δ1DsqE(κ), if the limit exists. If δ1=0, then δ1DsqE=DsqE.
Definition 1.7 ([12]). Presume that E:I→R is a continuous function. Then,
Clearly, a function is said to be right quantum symmetric integrable if ∑∞n=0q2n+1E(q2n+1κ+(1−q2n+1)δ1) converges. Remember that the above-discussed operators do not coincide with classical Jackson q operators.
Recently, Cortez et al. [13] investigated the interval-valued quantum symmetric operators in interval space based on Hukuhara differences, which are described as
Definition 1.8. Suppose E:I→RI is continuous I.V function. Then, the left interval-valued quantum symmetric difference operator is defined as
And the corresponding integral operator is expressed as
Definition 1.9. Let E∈C([σ1,δ1],RI). Then the σ1Iiq,s-integral operator is described as
One can easily observe that a function is considered to be I.V left q-symmetric integrable if ∑∞n=0q2nE(q2n+1δ1+(1−q2n+1)σ1) converges.
In 2013, Tariboon and Ntouyas [14] noticed some limitations of q-Jackson operators over finite intervals in impulsive difference equations and developed the q operators over finite interval connected with left point and explored its several implications in both impulsive difference equations and inequalities as well. This development paved another way to investigate integral inequalities. Alp and his coauthors [15] noticed that the trapezoidal inequality established in [16] is not correct and provided the new proof by using the concept of the support line of differentiable convex functions, and also established several midpoint quantum estimates. The authors of [17] analyzed the error estimates of the Milne rule by utilizing the Jensen-Mercer inequality and quantum calculus along with applications. Nosheen et al. [18] concluded some new quantum symmetric counterparts of basic inequality results, such as Hölder's inequality and error inequalities of one point rule associated with s-convex functions. To prove the Hermite-Hadamard inequality analytically, it was necessary to introduce the right quantum operators. In 2022, Kunt et al. [19] introduced the right sided quantum operators and provided their applications in integral inequalities. For a complete investigation, see [20,21,22,23,24,25,26,27].
In [28,29] the authors computed the estimates of one one-point integration rule incorporated with I.V functions and delivered applications as well. Following the idea of the previously discussed paper, Costa and Roman Flores [30] computed several fuzzy integral inequalities. In 2018, Zhao et al. [31,32] delivered the Jensen's and trapezium type inequalities associated with I.V-h-convex functions and I.V Chebyshev kinds of inequalities, respectively. Budak et al. [33] initiated the development of fractional versions of the trapezium inequalities for I.V convex functions defined by the means of containment ordering. In [34] the authors presented the class of I.V two-dimensional harmonic convex functions and derived several fractional integral inequalities by taking into account Raina's integral operators. Lou et al. [35] devoted their efforts to develop the notion of I.V quantum calculus based on generalized Hukuhara differences and provided the applications to inequalities. Motivated by the technique of [35], Kalsoom et al.[36] worked on I.V general quantum calculus by the means of Hukuhara differences and explored some applications to the Hermite-Hadamard inequality. Ali et al. [37] extended the idea presented in [36] for the right I.V-(p,q) operators and presented their key properties. Bin-Mohsin et al. [38] stated another class of generalized convexity based on a left-right ordering relation named as LR-fuzzy bi-convex function, and delivered Hermite-Hadamard and its weighted forms type results. In 2022, Duo and Zhou [39] examined the coordinated integral inequalities by considering the two-dimensional convex functions generalized integral operator having a non-singular kernel. In [40] the authors reported the parametric fractional versions of inequalities through convex functions and presented some visuals to support their outcomes.
The principal intent of this article is to examine the right sided quantum symmetric operators in the frame work of I.V functions along with their implications. We structured our study into two portions: In the initial portion of the study, we recover some vital details, background, and inspiration of the research. In the next part, we introduce the I.V right symmetric quantum derivative operators based on generalized Hukuhara difference and discuss their key properties. Based on the newly developed quantum operators, a new antiderivative operator is developed. Also, some crucial results, including the fundamental theorem of calculus and several other properties will be provided. Later on, several Hermite-Hadamard-type inequalities will be proved by both graphical and analytical methods essentially utilizing the convexity of the function. We will also present a visual breakdown in the support of principal findings.
2.
I.V right q-symmetric operators
In the current part of the study, interval-valued quantum symmetric operators are based on ⊖g differences. First, we introduce the notion of interval-valued right symmetric quantum difference and integral operators and their properties.
2.1. δ1diq,s-operator and its properties
Now, we investigate the right I.V q-symmetric difference and integral operators and their properties. The space of left q-symmetric differentiable operators is denoted by δ1diq,s.
Definition 2.1. Suppose E:I→RI is a continuous I.V function. Then, the right I.V q-symmetric difference operator is defined as
Example 2.1. Assume that E:[0,1]→RI is an I.V function such that E(ϱ)=[−4ϱ,5ϱ]. Then, we compute δ1Diq,sE(ϱ) by implementing Definition 2.1, and we have
Theorem 2.1. A function E:[σ1,δ1]→RI is I.V right q-symmetric differentiable at ϱ∈[σ1,δ1] ⇔ E⋆ and E⋆ are right quantum symmetric differentiable at ϱ∈[σ1,δ1], and
Proof. Assume that E is an I.V right q-symmetric differentiable function. Then, there exists g⋆ and g⋆ such that δ1Diq,sE(ϱ)=[g⋆,g⋆], and by considering Definition 1.8, we that
and
exist. Then, δ1Dq,sE⋆(ϱ) and δ1Dq,sE⋆(ϱ) exist, and (2.1) is straight forward.
Conversely, suppose E⋆ and E⋆ are right q-symmetric differentiable at ϱ. If δ1Dq,sE⋆(ϱ)≤δ1Dq,sE⋆(ϱ), then
Similarly, if δ1Dq,sE⋆(ϱ)≤δ1Dq,sE⋆(ϱ), then δ1Diq,sE(ϱ)=[δ1Dq,sE⋆(ϱ),δ1Dq,sE⋆(ϱ)]. □
Now we provide another characterization of the right q-symmetric derivative based on the monotonic property of functions.
Theorem 2.2. Let E:[σ1,δ1]→RI and if it is I.V right q-symmetric differentiable on [σ1,δ1], then
(1) δ1Diq,sE(ϱ)=[δ1Dq,sE⋆(ϱ),δ1Dq,sE⋆(ϱ)], if E is l-increasing.
(2) δ1Diq,sE(ϱ)=[δ1Dq,sE⋆(ϱ),δ1Dq,sE⋆(ϱ)], if E is l-decreasing.
Proof. Suppose that E is an l-increasing and right q-symmetric differentiable function on [σ1,δ1], and we observe that q−1ϱ+(1−q−1)δ1>qϱ+(1−q)δ1 for q∈(0,1). Since l(E)=E⋆−E⋆ is increasing,
Thus,
Hence, the required result is achieved. By a similar process, we can prove the 2nd result. □
Remark 2.1. If υ∈(σ1,δ1) and E is l-increasing on [σ1,υ) and l-decreasing on (υ,δ1], then δ1Diq,sE(ϱ)=[δ1Dq,sE⋆(ϱ),δ1Dq,sE⋆(ϱ)] on [σ1,υ) and δ1Diq,sE(ϱ)=[δ1Dq,sE⋆(ϱ),δ1Dq,sE⋆(ϱ)] on (υ,δ1].
Theorem 2.3. Let E:[σ1,δ1]→RI be a left symmetric q-differentiable function. Then, for any υ=[υ⋆,υ⋆]∈RI and α∈R, the mappings E+υ and αE are also right q-symmetric differentiable on [σ1,δ1]. Then:
(1) δ1Diq,s(E(ϱ)+υ)=δ1Diq,sE(ϱ).
(2) δ1Diq,s(αE)(ϱ)=αδ1Diq,sE(ϱ).
Proof. For ϱ∈[σ1,δ1] and from Definition 1.8, we have
We leave the second proof for interested readers. □
Theorem 2.4. Let E:[σ1,δ1]→RI be a right q-symmetric differentiable function. For any υ=[υ⋆,υ⋆]∈RI, if l(E)−l(υ) has a constant sign over [σ1,δ1], then E⊖gυ is right q-symmetric differentiable.
Proof. Take ϱ∈[σ1,δ1]. Then,
□
Theorem 2.5. Let E,g:[σ1,δ1]→R be right q-symmetric differentiable mappings. Then, the sum E+g is a right q-symmetric differentiable if one of the following cases hold:
(1) If E,g are equally l-monotonic on [σ1,δ1], then
(2) If E,g are differently l-monotonic on [σ1,δ1], then
Moreover, in both cases, δ1Diq,s(E(ϱ)+g(ϱ))⊆δ1Diq,sE(ϱ)+δ1Diq,sg(ϱ).
Proof. Suppose E,g are I.V right q-symmetric differentiable and l-increasing on [σ1,δ1]. Then, E⋆,E⋆,g⋆ and g⋆ are left q-symmetric differentiable, and δ1Dq,sE⋆(ϱ)≤δ1Dq,sE⋆(ϱ), δ1Dq,sg⋆(ϱ)≤δ1Dq,sg⋆(ϱ). Then, E⋆+g⋆ and E⋆+g⋆ are q-symmetric and
By similar proceedings, we can obtain that the result for both E and g are l-decreasing.
Now, suppose that E is l increasing and g is l-decreasing. Then δ1Dq,sE⋆(ϱ)≤δ1Dq,sE⋆(ϱ), δ1Dq,sg⋆(ϱ)≤δ1Dq,sg⋆(ϱ). Also,
And,
By comparing (2.2) and (2.3), we conclude that δ1Diq,s(E(ϱ)+g(ϱ))=δ1Diq,sE(ϱ)⊖g(−1)δ1Diq,sg(ϱ).
Since E+g is l-increasing and decreasing, we get δ1Diq,s(E(ϱ)+g(ϱ))⊆δ1Diq,sE(ϱ)+δ1Diq,sg(ϱ), and the opposite case can be obtained by a similar procedure.
Hence, the required results are achieved. □
Theorem 2.6. Let E,g:[σ1,δ1]→R be right q-symmetric differentiable mappings and l(E)−l(g) have constant sign over domain. Then, E⊖gg is a right q-symmetric differentiable if one of the following cases hold
(1) If E,g are equally l-monotonic on [σ1,δ1], then
(2) If E,g are differently l-monotonic on [σ1,δ1], then
Proof. Assume that l(E)≥l(g) and E⊖gg=[E⋆−g⋆,E⋆−g⋆].
Suppose E,g are I.V right q-symmetric differentiable and l-increasing on [σ1,δ1]. Then, E⋆,E⋆,g⋆ and g⋆ are left q-symmetric differentiable, and δ1Dq,sE⋆(ϱ)≤δ1Dq,sE⋆(ϱ), δ1Dq,sg⋆(ϱ)≤δ1Dq,sg⋆(ϱ). Then, E⋆−g⋆ and E⋆−g⋆ are q-symmetric differentiable, and thus E⊖gg is a right q-symmetric differentiable function such that
By similar proceedings, we can obtain the result that both E and g are l-decreasing.
Now, suppose that E is l increasing and g is l-decreasing. Then, δ1Dq,sE⋆(ϱ)≤δ1Dq,sE⋆(ϱ), δ1Dq,sg⋆(ϱ)≤δ1Dq,sg⋆(ϱ). Also,
And,
Comparing (2.4) and (2.5) yields the required result. □
2.2. δ1Iiq,s-integral operator and its properties
In the current part of the study, we introduce the concept of I.V right q-symmetric integral operator and its essential characterization. For our convenience, we specify the space of I.V right q-symmetric integrable mappings and the space of all continuous I.V mappings by δ1Iiq,s and υ([σ1,δ1],RI) respectively.
Definition 2.2. Let E∈υ([σ1,δ1],RI). Then, the δ1Iiq,s-integral operator is described as:
One can easily observe that a function is considered to be I.V right q-symmetric integrable if ∑∞n=0q2nE(q2n+1σ1+(1−q2n+1)δ1) converges.
Theorem 2.7. Let E∈υ([σ1,δ1],RI). Then, E∈δ1Iiq,s ⇔ both E⋆ and E⋆ are right q-symmetric integrable mappings. Also,
Proof. Consider E∈δ1Iiq,s. Then,
This implies that both E⋆ and E⋆ are right q-symmetric integrable mappings.
Conversely, suppose that E⋆ and E⋆ are q-symmetric integrable mappings and E⋆≤E⋆. Then, the result is obvious.
So, the result is proven. □
Example 2.2. Let E:[0,2]→RI such that E(κ)=[2κ,3κ2]. Then,
Theorem 2.8. Let E,g∈υ([σ1,δ1],RI) and (σ1,ϱ)⊆[σ1,δ1]. Then, for α∈RI :
(1) ∫δ1σ1[E(κ)+g(κ)]δ1dsqκ=∫δ1σ1E(κ)δ1dsqκ+∫δ1σ1g(κ)δ1dsqκ.
(2) ∫δ1σ1(αE)(κ)δ1dsqκ=α∫δ1σ1E(κ)δ1dsqκ.
Proof. From Definition 2.2,
Hence, the first proof is obtained. The proof of the second result is obvious. □
Theorem 2.9. Let E,g∈υ([σ1,δ1],RI) and (σ1,ϱ)⊆[σ1,δ1]. Then,
Furthermore, l(E)−l(g) has constant sign on [σ1,δ1], thus
Proof. We observe that
Also, we have
Comparison of (2.6) and (2.7) results in the desired inequality.
By the notion of generalized Hukuhara difference, if l(E)≥l(g), then E⊖gg=[E⋆−g⋆,E⋆−g⋆], and if l(E)≤l(g), then [E⋆−g⋆,E⋆−g⋆]. We suppose that l(E)≥l(g) on [σ1,δ1] such that E⊖gg=[E⋆−g⋆,E⋆−g⋆]. This implies that ∫δ1σ1(E⋆−g⋆)δ1dsqκ≤∫δ1σ1(E⋆−g⋆)δ1dsqκ. Now,
Hence, the desired result is obtained. □
Theorem 2.10. Let E:[σ1,δ1]→RI be right a q-symmetric differentiable function on (σ1,δ1) and δ1Diq,s(E(ϱ)∈υ([σ1,δ1],RI). If E is l-monotone on [s,κ], then
Proof. If E is a I.V right q-symmetric differentiable function, then E⋆ and E⋆ are right q-symmetric differentiable mappings, so σ1Dq,sE⋆ and σ1Dq,sE⋆ are right q-symmetric integrable mappings. Therefore, E is also a I.V right q-symmetric integrable function. If E is l-increasing on [σ1,δ1], then δ1Diq,s(E(κ)=[δ1Diq,sE⋆(κ),δ1Diq,sE⋆(κ)], and then
Since E⋆≤E⋆ and from (2.9) and (2.10), we acquire
Now, from the notion of gh-difference, then
If E is l-decreasing on [σ1,δ1], then δ1Diq,s(E(κ))=[δ1Diq,sE⋆(κ),δ1Diq,sE⋆(κ)], and
Hence, the required result is acquired. □
Remark 2.2. If E is l-increasing, then (2.8) can be interpreted as
If E is l-decreasing, then (2.8) can be interpreted as
It is interesting to observe that the above result does not hold, if E is not l-monotone.
2.3. Applications to Hermite-Hadamard's inequality
Now we develop the trapezium type inequalities by utilizing the I.V right q-symmetric integral operator.
Theorem 2.11. Suppose E:[σ1,δ1]→RI is an I.V right q-symmetric differentiable function. Then
Proof. Since E is an I.V right q-symmetric differentiable function on (σ1,δ1), there exist two tangents at σ1q2+δ11+q2 given as
and
Since E is an I.V convex function, H1(ϱ)⊆E(ϱ), and then applying the I.V right q-symmetric integration, we have
Now we establish the proof of second containments.
Moreover, the secant line through (σ1,E(σ1)) and (δ1,E(δ1)) can be expressed as:
and
Since E(ϱ)=[E⋆(ϱ),E⋆(ϱ)] is an I.V convex mapping and ω1(ϱ)⊆E(ϱ), then
□
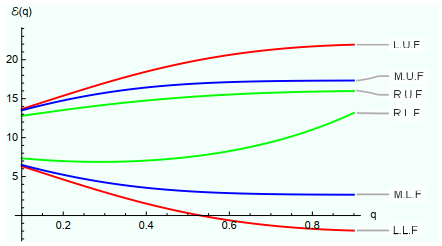
Example 2.3. Let E:[0,2]→RI be an I.V right q-symmetric differentiable function such that E(ϱ)=[2ϱ2,−2ϱ2+20]. Then, from Theorem 2.11, we obtain
Next, the I.V right q-symmetric derivative of E is given as δ1Dq,sE(ϱ)=[−4(2−q),4(2−q)].
Furthermore, the I.V right q-symmetric integral of E is given as
From the above computations, we have the following containment:
For q=13 in (2.14), we have
For graphical validation (Figure 1), we take q∈(0,1) in (2.14).
Theorem 2.12. Suppose E:[σ1,δ1]→RI is an I.V right q-symmetric differentiable function. Then,
Proof. Since E is an I.V right q-symmetric differentiable function on (σ1,δ1), there exist two tangents at σ1+δ1q21+q2 given as
and
Since E is an I.V convex function, H2(ϱ)⊆E(ϱ), and then applying the I.V right q-symmetric integration, we have
□
Example 2.4. Let E:[0,2]→RI be an I.V right q-symmetric differentiable function such that E(ϱ)=[2ϱ2,−2ϱ2+20]. Then, from Theorem 2.12, we obtain:
From (2.17) and (2.13), we obtain the following expression for Theorem 2.12:
For q=13 in (2.18), we have
For graphical validation (Figure 2), we take q∈(0,1) in (2.18).
Theorem 2.13. Let E:[σ1,δ1]→R+I be an I.V convex function. Then,
Proof. Since E is an I.V convex function, then
Applying quantum symmetric integration on (2.19) with respect to ′ϱ′ over [0,1], we have
and
Similarly,
The combination of (2.20)–(2.22), results in the first containment. To prove our second containment, we employ the convexity of E. Hence, the result is completed. □
Example 2.5. Let E:[0,2]→RI be an I.V right q-symmetric differentiable function such that E(ϱ)=[2ϱ2,−2ϱ2+20]. Then, from Theorem 2.13, we obtain:
For q=13 in (2.23), we have
For graphical validation (Figure 3), we take q∈(0,1) in (2.23).
3.
Conclusions
In the realm of mathematical analysis, one of the key research aims is how to find the derivative of absolute functions, which are not differentiable at certain points. To handle such kinds of problems, symmetric calculus plays a vital role. In this paper, we have developed the concept of right interval-valued quantum symmetric operators and examined numerous properties of operators in the setting of interval analysis. We have discussed the utility of these operators in inequalities. In favor of our findings, some visuals have been provided. It is important to observe that the derivative of I.V functions involving absolute functions can be computed from our proposed operators. We hope these operators and techniques will create new avenues of research. Based on these operators, several kinds of inequalities can be obtained. Also, by utilizing these operators, several results of optimization theory can be updated. In the future, we will try to establish some error bounds of numerical formulas in the interval-domain associated with the developed theory.
Author contributions
Yuanheng Wang: conceptualization, investigation, writing-review and editing, visualization, validation; Muhammad Zakria Javed: conceptualization, software, formal analysis, investigation, writing-original draft preparation, writing-review and editing, visualization; Muhammad Uzair Awan: conceptualization, software, validation, formal analysis, investigation, writing-review and editing, visualization, supervision; Bandar Bin-Mohsin: conceptualization, validation, investigation, writing-review and editing, visualization; Badreddine Meftah: conceptualization, software, validation, formal analysis, investigation, writing-review and editing, visualization; Savin Treanta: conceptualization, software, investigation, writing-review and editing. All authors have read and agreed to the published version of the manuscript.
Acknowledgments
This research is supported by "The National Natural Science Funds of China (No. 12171435)". Prof Bandar is thankful to King Saud University, Riyadh, Saudi Arabia for the project "Researchers Supporting Project number (RSP2024R158), King Saud University, Riyadh, Saudi Arabia". The authors are thankful to the editor and the anonymous reviewers for their valuable comments and suggestions.
Conflict of interest
The authors declare no conflict of interest.









 DownLoad:
DownLoad:





