1.
Introduction
With the rapid development of multimedia technologies, the collection and transmission of multimedia data have become greatly convenient and easy. Meanwhile, multimedia data quality of imaging equipment limits the wider application of machine vision inspection system. The method to improve the image resolution through algorithms—namely, image interpolation, has become an active research field in machine vision. To a certain extent, image interpolation can effectively increase the image resolution to meet various applications, such as motion tracking and pose estimation. In addition, image interpolation has a wide range of practical applications and commercial prospects in remote sensing, cloud-of-thing system [1], surveillance [2,3], medical imaging [4], multimedia processing [5,6], and consumer electronic [7].
In the registration and fusion of non-homologous images, image size adjustment is needed. Especially in the visual measurement process of large machined parts, due to the angle of view, the images captured by different cameras have different dimensions [8]. This will lead to dimensional distortion. In order to reduce visual artifacts and adapt to the requirements of arbitrary magnification, a more flexible image interpolation method is needed. Although the visual quality of simple linear interpolation methods is not sufficient, these methods still are widely used due to their low computational complexity and interpolation flexibility. Linear interpolation methods include nearest-neighbor interpolation [9,10], bilinear interpolation [11,12], bicubic interpolation [11,12,13,14], cubic spine interpolation [15,16] and iterative linear interpolation [17,18], which predict the gray intensity of an unknown pixel according to the distance between the unknown pixel and the reference pixels. The pixels at the boundary, edge or texture of a low-resolution (LR) image have a greater impact on image interpolation than those perpendicular to the edge. Therefore, an isotropic low-pass filter is employed to weaken the high-frequency components of the edges, and the edges are consequently smoothed, which will lead to artifacts such as blurring, ringing, checkerboard effects, edge discontinuities and jagging.
2.
Related works
In related works, contributions have been made in reducing the visual artifacts. However, those methods are mainly suitable for integer magnification of images. Some edge-guided interpolation algorithms have been designed to correct the artifacts stemming from isotropic filters. The explicit methods [19,20] were developed to consider local structural information such as edges or isophotes. However, for images containing many complex structures, an edge map estimated with these methods tends to be unpredictable, which increases the intensity variation of the interpolation. Implicit adaptive methods have been proposed, which embed the local structures into an objective function that can be solved using linear or nonlinear optimization methods. These methods can model the image’s local patterns and estimate the unknown pixels around the edges in texture-rich areas. Li and Orchard [21] proposed an edge adaptive interpolation method according to the geometric duality of edges, named new edge directed interpolation (NEDI). Geometric duality between the low-resolution covariance and the high-resolution covariance, couple the pair of pixels along the same orientation, enables NEDI to estimate the high-resolution covariance from its low-resolution counterpart with a qualitative model characterizing the relationship between the covariance and the resolution. Because the inaccurate estimation of covariance in texture-rich regions, jagging and color infidelity are inescapable on some RGB samples. Analogous to NEDI, other studies have been conducted with geometrical similarity measurement [22,23,24,25,26] to improve interpolation accuracy. Chang and Kevin [27] used collaborative representation and exploiting non-local self-similarity of natural images and introduced the external HR information into the interpolation process. Zhang and Wu [22] proposed soft-decision adaptive interpolation (SAI), which learnt and adapted varying pixel structures by using a piecewise autoregressive (PAR) model in the local rectangular window of the image. To implicitly use AR models for better solving nonlinear problems at the edges in image interpolation, irregular windows were employed by Guo et al. [23,24,25], which were extended adaptively from the root windows according to the geometric features, such as isophotes and curvatures. The similarities between pixels were calculated using patch-geodesic distance. Cheng et al. [28] used Fast Fourier Transformation (FFT) multichannel interpolation to reconstruct or approximate the continuous signals from a series of discrete points. A spatial interpolation can predict the values of the unknown points by processing the surrounding variables with meaningful values within the same region. In our previous work [26], a spatial general autoregressive model (SGAR), which is a uniform expression for both linear and nonlinear AR models, was employed to implement a noise-insensitive and edge-preserving interpolator. Although it still uses regular windows, its nonlinear description ability was improved by introducing nonlinear terms into the model. The similarities between pixels in the image windows were implicitly exploited by the robust parameter estimation method named generalized M-estimator.
In contrast to the above interpolation strategies, the curvature-based interpolation algorithms introduced high-order derivative information of image intensity to achieve high-accuracy interpolation from the rough results. Giachetti and Asuni [29] proposed the iterative curvature based interpolation (ICBI) method, which generates high-resolution (HR) pixels within the grid along the directions with the lowest second-order derivative and subsequently updates the values of the HR pixels by minimizing the local variations of curvature of the image intensity. However, the hole-filling strategy limits the application with non-integer upscaling factors. Kim and Cha [30] proposed the curvature interpolation method (CIM) based on a partial differential equation (PDE). Because it is known to tend to converge to a piecewise constant image [31,32]. These methods share the characteristic that the final interpolation results are modified by an iterative procedure upon the initial values provided by other methods. It was emphasized that better interpolation accuracy was achieved, which was largely due to the first-step interpolation method.
Therefore, an arbitrary scale factor image interpolation based on the SGAR model was used to implement image interpolation from three aspects: image window adaptive extension by gradient angle, model regularization by the elastic network, and refinement of the result accuracy by the curvature constraints. The rest of the paper is structured as follows. Section 3 introduces the SGAR model-based image interpolation method and its implementation. Experimental results and a comparison study with some existing popular image interpolation techniques are presented in Section 4. Section 5 contains our conclusions.
3.
SGAR model-based image interpolation method and its implementation
3.1. Image description based on SGAR model
AR models are effective tools for image modeling [22,23,24,25,26,33,34,35]. The linear AR model in vectorization form is shown as follows.
where ˆy is the prediction value, θ=[θ0,θ1,…,θn] is the model’s parameter vector, containing the intercept term θ0 and the feature weights θ1 to θn, x is the sample’s feature vector, and hθ is the hypothesis function, using the model parameters θ.
However, the data distributions of a digital image are more complex. To better fit the image model, the product of the pixels can be involved in the polynomial regression, which is adopted as a new feature, such as the SGAR model that was initially explored based on Weierstrass theory for digital image adaptive filtering in our previous work [36]. The pixels in the image follow a certain regular pattern that indicates the direction of gray scale change. In other words, every unknown interpolated pixel in a piecewise image can be estimated by its known adjacent neighbor with certain weights. The original images are broken up into small fragments. Based on the stationarity assumption in piecewise images [22], we model the fragments as a locally stationary Gaussian process. Inevitably, some fragments with abrupt and unnatural gray scale changes are brought into the modeling process and have negative effect on describing the pattern of the image [36]. An adaptive filter was implemented based on the SGAR model. The new filter removed these artifacts while effectively conserving detailed image information. This was because the SGAR model fuses both linear and nonlinear AR models into a uniform expression[26]. However, the high-order polynomial regression model will probably overfit the training data. Moreover, simple linear regression has the problem of underfitting. For an image window, it could be modeled as
where x(p) is the forecasted value of a pixel, x(sk) is the reference value of a pixel in the LR image, a(p) is the modeling residuals. s is a two-dimensional vector representing the location of the specific pixel. A is a set of vectors representing the location of adjacent reference pixels. The composition of set A is discussed in section 2.3. ϕ(s1, s2, …, si) is the model parameters. r is the order of the model, which indicates the dimension of vector ϕ. When r is 1, the SGAR model is degenerated into AR model as is shown in Eq (3). The dimension of the vector ϕ is only 8 and consists of the eight adjacent pixels around the anchor pixel.
When r is 2, the SGAR model can also be rewritten as Eq (4). The dimension of vector ϕ is 44 and consists of 8 adjacent pixels around the anchor pixel, 36 self-multiplication and product of two different pixels are taken into consideration. We will explain how to obtain the parameters of the SGAR model in section 2.4.
3.2. Interpolation accommodate arbitrary scaling factor
The relationship between the coordinates of the pixels of the HR image and those of the corresponding LR image must first be clarified.
where (u, v) represents a pixel in the LR image, (i,
j) represents a pixel in the HR image, and h is an arbitrary scaling factor.
The reference pixels in the LR image of a reconstructed pixel in the HR image is determined with the following mathematical expression.
where φ(·) is a function that tends toward negative infinity, and ρ(·) is a function that rounds to the nearest decimal or integer.
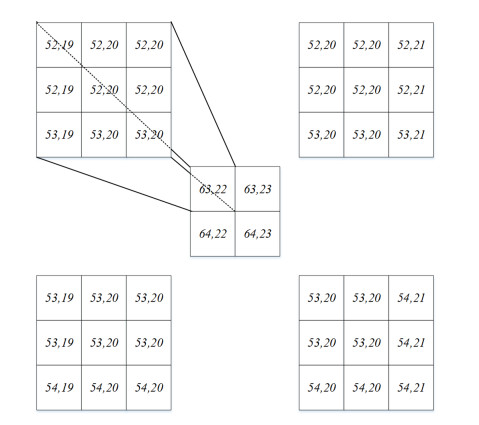
When r is 1, the product of the LR pixels and SGAR model parameter vector ϕ is regard as the value of interpolated pixel. For the convenience of understanding, taking 1.25× interpolation as an example, the coordinates of HR pixels are (63, 22), (63, 23), (64, 22), (64, 23), the LR reference pixels coordinate at (52, 19), (52, 20), (53, 19) and (53, 20) are used to interpolate the HR pixel at (63, 22), other HR pixels interpolation schemes are shown in Figure 1:
When r is 2, the parameters of SGAR model are estimated by modified samples. For each sample, it consist of an anchor pixel at the center of 3 × 3 image block, 8 LR reference pixels and 36 parameters generated by these pixels. Then the LR pixels used in the interpolation process share the same arrangement with the samples used in parameter estimation process.
3.3. Gradient guided model extension
The gradient guided method [20,37] was employed to adaptively extend the parameters estimation window to increase the training dataset and ultimately improve the interpolation accuracy. The gradient angles were calculated by the Scharr operator, which is formulated as follows.
where Gx and Gy are the gradients of the x and y directions, respectively, according to the Scharr operator.
Therefore, the gradient angle is
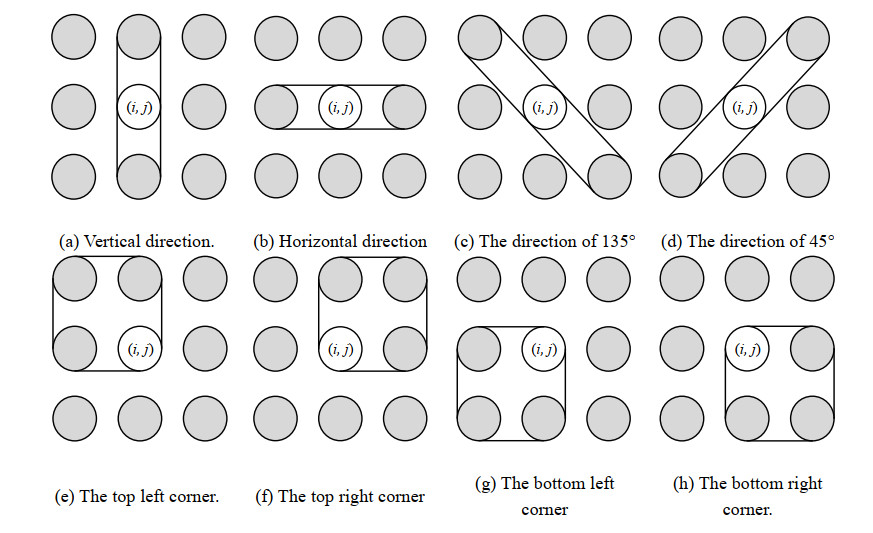
The gradient angle space was divided into eight regions according to an interval of 45°, and the eight regions were placed into four groups.
According to the gradient direction of each pixel in the LR image, the image windows involved in modeling the image with the SGAR model would be extended in four directions as shown in Figure 2.
The gradient directions of the n pixels were grouped according to Eq (10), and the frequency of each group was counted. The extension direction was subsequently determined according to the maximum of the four frequencies. The number of fitting samples is determined by the gradient based extension method. In fact, 15 samples were used in the horizontal and vertical directions, and 19 samples are used in the other two directions.
3.4. Regularized SGAR model-based on elastic network
The earlier works suggested that the patterns of the AR models containing only a linear relationship between pixels are sufficient. The least-square (LS) method [18], which is highly sensitive to outliers, was used to solve the parameters of the interpolation model with cross-direction constraints. To address the noise-sensitive problem of the ordinary LS, some methods were proposed [38]. Among them, regularization is a common method. The l1-norm and l2-norm regularization terms were added to the objective function by Liu et al. to enhance the stability of the LS solution. The l2-norm penalty term [27] (i.e., ridge regression) was used to regularize the objective function. Weighted ridge regression (WRR) [19] was adopted to restrain the expansion of variance and thus achieve more reliable estimations by modulating weights into the regression to evaluate the reliability of each sample. In contrast to the above methods, the products of the pixels were employed to increase the description capacity of the SGAR model to suit various digital images, and the overfitting problem was noted.
The SGAR model adopted the products of pixels as the new features, so the descriptive power of the model was improved compared with some interpolation methods that consider only simple linear relations [15]. Therefore, the model was especially effective for rebuilding the local nonlinear relationship of image windows [22,23,26,35]. However, it may cause overfitting problems because of the higher degree of freedom [26]. To address this problem, the regularization method could be used to reduce the freedom of models and keep the complexity of the object function in a reasonable state. Ridge regression [23,39], Lasso regression [40] and Elastic Network [41] are the three most commonly used methods.
Therefore, the mean square error (MSE) was calculated to represent the intensity differences between the reference pixels and the predicted pixels. The parameters of the SGAR model will obtained by minimizing the loss function. The loss function of Elastic Network is shown as:
where m is the number of the samples in the dataset, n is the number of fit parameters, X(i) is the vector combined with all features of sample i, θ is the vector of the model parameter, y(i) is the anchor pixel of the region, and is the original value of LR pixel at the center of 3 × 3 image block, α is the hyper-parameter that dominates the regularization level of the model, and t is the hyper-parameter that dominates the mixing ratio. In the experiment, the value of t was manually set to 0.5.
3.5. Regularized SGAR model-based on elastic network
The SGAR model learns the structural relationship between pixels and their neighbors in the LR image to recover as much missing information as possible during down-sampling [42]. However, we know that it is very difficult to recover HR images in high-frequency areas where the gray level of the image changes rapidly, such as textures and gradients. Only a relatively small part of the missing information can be restored. Compared with low-frequency areas, modeling of image windows with the SGAR model in these areas is more likely to encounter the problem of insufficient generalization ability, which makes interpolation stability difficult to control. Therefore, in these regions, a strategy that updates the interpolated pixels by minimizing the local variation of the second derivative of the image was adopted. By transforming the two-dimensional image plane into three-dimensional space, the surface optimization methods [43] were employed to solve the problem of artifacts.
An analogous filtering-based method proposed by Gong et al. [43] was explored to reduce the regularization part of the variational energy while guaranteeing non-increasing total energy. That is, the iterative curvature method was modulated into SGAR mode. The second-order derivatives of p (i, j) on the HR image were calculated in eight different directions, as shown in Figure 3, and were used as an approximation of the curvatures. The eight second-order derivatives were thresholded, and a set consisting of the thresholded second-order derivatives was obtained. P (i, j) was updated according to the absolute minimum of the elements of the set. The second-order derivatives of the digital image window were calculated repeatedly until the curvature of p (i, j) in all directions was below the threshold or the maximum iterations were reached. The curvatures around p (i,
j) gradually adapted to the directions that shared a similar curvature change tendency. Thus, the accuracy of the interpolation results obtained in the first step was improved with the following algorithm process in List 1.
4.
Results and discussions
Experiments were carried out on a dataset provided by ICBI [29] with different magnification factors to systematically verify the performance of the proposed method in terms of objective measurements, subjective visual effect and computational cost. For thoroughness and fairness, the chosen dataset contains images with different resolutions and various objects—animals, flowers and buildings—with a wide range of color and natural textures, which include typical and unique digital images for interpolation research.
Comparisons of non-integer factors are studied with bicubic interpolation, bilinear interpolation, nearest neighbor interpolation and AREA interpolation. These methods are well-established in Open Source Computer Vision Library (OpenCV). The mean square error (MSE), peak signal to noise ratio (PSNR), structural similarity index (SSIM), feather similarity index (FSIM) and Correlation coefficient (CC) were used to evaluate the result of the interpolation algorithm. The experiments are conducted in non-integer interpolation factors to substantiate the performance of proposed method in arbitrary scaling. In 1.7× and 2.2× enlargement, images with 512 × 512 pixels are resized into 435 × 435 pixels and 563 × 563 pixels with bicubic method, and used as the reference for computing the MSE, PSNR and SSIM index. The images with 256 × 256 pixels are enlarged to the same size by proposed method and other four conventional non-integer interpolation methods. The experimental results are tabulated in Tables 1 and 2.
In 1.7× interpolation experiment of 25 images, our method obtains best MES index in 17 of 25 images, best PSNR index among 18 of 25 interpolated HR images, best SSIM index in 4 of 25 images, best FSIM index in 19 of 25 images and best CC index in 17 of 25 images. In 2.2× interpolation experiment, proposed method achieved best performance over MSE, PSNR and SSIM index in all of 25 images, best FSIM index in 9 of 25 images and best CC index in 15 of 25 images.
In comparison with other edge-directed methods NEDI [21], improved NEDI (i-NEDI) [34] and ICBI [29] are invited to evaluate the result of the interpolation algorithm. All of 25 images with 512 × 512 pixels are the references for computing the MSE, PSNR and SSIM, the 25 images with 256 × 256 pixels are enlarged by propose method and other interpolation methods. In order to prevent the pixel-shift in ICBI and our proposed method, the left-top 511 × 511 part of original images (and the results of other methods) is used to compute the MSE, PSNR and SSIM. The experimental results are tabulated in Table 3.
In 2× interpolation experiment, proposed method together with our previous SGAR method achieved best MSE in 18 of 25 images, best PSNR in 18 of 25 images, best SSIM index in 21 of 25 images, best FSIM index in 8 of 25 images and best CC index in 21 of 25 images. According to the PNSR index, 7 of the 25 interpolated HR images obtained the best effect with the ICBI method, and the other 18 interpolated HR images obtained the best effect with the method proposed in this paper. The ratio was as high as 72%. For these 18 images, the PSNRs of the proposed method were improved to different degrees compared to those of the other four methods. The maximum, minimum and average increments were 1.672, 0.005 and 0.267 dB, respectively, and the maximum, minimum and average relative increments were 5.79, 0.02 and 0.94%, respectively. Compared with NEDI, the maximum, minimum and average increases in PSNR were 1.672, 0.234 and 0.547 dB, respectively, and the relative maximum, minimum and average increases were 5.79, 0.78 and 1.94%, respectively. Compared with bicubic, the maximum, minimum and average increases in PSNR were 0.429, 0.063 and 0.182 dB, respectively, and the relative maximum, minimum and average increases were 1.45, 0.22 and 0.63%, respectively. Compared with i-NEDI, the maximum, minimum and average increases in PSNR were 0.577, 0.051 and 0.281 dB, respectively, and the relative maximum, minimum and average increases were 1.82, 0.17 and 0.98%, respectively. Compared with ICBI, the maximum, minimum and average increases in PSNR were 0.353, 0.005 and 0.06 dB, respectively, and the relative maximum, minimum and average increases were 1.11, 0.02 and 0.21%, respectively. According to the SSIM index, 17 of 25 images obtain the best effect with the ICBI method, compare with the second best ICBI method, the maximum, minimum and average increments were 0.02099, 0.00019 and 0.00251, respectively, and the maximum, minimum and average relative increments were 2.284, 0.021 and 0.283%, respectively. Compared with bicubic, which accommodate non-integer scaling factors, the maximum, minimum and average increases in SSIM were 0.00976, 0.00124 and 0.00461, respectively, and the relative maximum, minimum and average increases were 1.35, 0.13 and 0.52%, respectively. Two points should be specified: First, both bicubic and the proposed method can achieve non-integer image magnification. Compared with the bicubic method, the proposed method improved the interpolation effect to a certain extent in all 25 experimental images. Second, both ICBI and the proposed method use the iterative curvature method. Thus, the two methods achieved the best interpolation effect among these edge-directed methods. The difference is that the method presented in this paper uses the SGAR model to predict the unknown pixels in the first step the increasing trend is obvious.
Compared with the most popular non-integer interpolate methods and other conventional edge-directed methods, the HR images obtained by the proposed method have significantly fewer blurring effects. As is shown in Figures 5 and 6, these defects are especially obvious among non-integer methods. In the visual comparison of 2× magnification, these defects also exist in Figures 7(a) and 8(a). It can be seen that the interpolation method based on the SGAR model has a better image description ability than the interpolation method based on the B-spline theory. The isotropic low-pass filter can enhance the smoothing effect, while the SGAR model is more suitable for revealing the distribution pattern of the image. Furthermore, the interpolation method based on the SGAR model has better color fidelity than NEDI, as shown in Figure 9. As shown in Figures 7 (d) and 8 (d), the HR image interpolated by ICBI has more visual defects. To analyze the reason, the proposed method used a curvature iterative method based on the discrete features of the image to describe the curvature change of anchor pixels in more directions, which helps eliminate interpolation defects such as artifacts in edge areas and texture-rich areas.
In machine vision system, especially in online visual inspection system. Due to the vibration and overheating problems, image noise will inevitably in the poor working conditions. In order to better combine with the potential application scenarios, gaussian noise with mean value of 0 and variance of 0.005 is added to gray image. The images used in this part of the experiment are shown in Figure 10.
We add the latest learning-based Meta-SR [44] to the comparison. In comparing with the traditional Bicubic interpolation method and Meta-SR [44], our previous SGAR method and the proposed method have made better progress in objective indicators on all of 5 images. Although, on some objective indicators, the edge-based method like NEDI and i-NEDI are better results than ours, these methods also magnify the impact of noise on visual quality. These artifacts are especially obvious in Figure 11.
Frankly, the naive for-loop and iterative process make the processing time relatively long, the proposed method is much slower than ICBI, let along other popular non-integer interpolation methods well-established in OpenCV. However, the processing speed can be improved with parallel threads simultaneously process on NVIDIA CUDA devices and the research will continue in future works.
5.
Conclusions
Based on our and others’ previous work, this paper introduced a new method for image interpolation via integration. The new method is based on the SGAR model and can accommodate arbitrary scaling factors. First, the paper discussed how to use the SGAR model to describe the image window, including the establishment of a linear autoregressive model, the SGAR model and the relationship between anchor pixels and their neighboring pixels. By grouping the gradient directions, the adaptive extension direction of the image window was determined, and an image window adaptive extension method based on the gradient angles for the SGAR model was formed. Because the product terms were introduced into the SGAR model, the degree of freedom of the model was increased, and the ability to describe the model was enhanced, but this may cause overfitting problems. Therefore, an elastic network was introduced into the solution of the objective function to address the overfitting problem. Finally, the curvatures were calculated in eight directions, and the interpolation results were updated accordingly to improve the interpolation accuracy. Experiments on 25 images show that the objective measures of the proposed method were improved to a certain extent. Subjective visual effect evaluations were carried out, and much better results were achieved. Therefore, the method presented in this paper improved the objective index of image interpolation and enhanced the subjective visual effect.
Acknowledgments
Mr. Hu reports grants from National Natural Science Foundation of China, grants from Postgraduate Research & Practice Innovation Program of Jiangsu Province, during the conduct of the study.
This work was supported in part by the National Natural Science Foundation of China under Grant No. 51705238 and the Postgraduate Research & Practice Innovation Program of Jiangsu Province Grant No. SJCX19_0491.
Conflict of interests
We declare that we do not have any commercial or associative interest that represents a conflict of interest in connection with the work submitted.









 DownLoad:
DownLoad: