Solutions of some typical nonlinear differential equations with Caputo-Fabrizio fractional derivative

Zhoujin Cui; Zhoujin Cui

doi:10.3934/math.2022779

AIMS Mathematics

2022, Volume 7, Issue 8: 14139-14153. doi: 10.3934/math.2022779

Previous Article Next Article

Research article Special Issues

Solutions of some typical nonlinear differential equations with Caputo-Fabrizio fractional derivative

Zhoujin Cui ^{1,2
,
,}

1.
School of Economics and Management, Jiangsu Maritime Institute, Nanjing 211170, China
2.
State Key Laboratory of Mechanics and Control of Mechanical Structures, Nanjing University of Aeronautics and Astronautics, Nanjing 210016, China

Received: 31 March 2022 Revised: 20 May 2022 Accepted: 23 May 2022 Published: 27 May 2022
MSC : 26A33, 34A08

In this paper, the solutions of some typical nonlinear fractional differential equations are discussed, and the implicit analytical solutions are obtained. The fractional derivative concerned here is the Caputo-Fabrizio form, which has a nonsingular kernel. The calculation results of different fractional orders are compared through images. In addition, by comparing the results obtained in this paper with those under Caputo fractional derivative, it is found that the solutions change relatively gently under Caputo-Fabrizio fractional derivative. It can be concluded that the selection of appropriate fractional derivatives and appropriate fractional order is very important in the modeling process.

Keywords:

Citation: Zhoujin Cui. Solutions of some typical nonlinear differential equations with Caputo-Fabrizio fractional derivative[J]. AIMS Mathematics, 2022, 7(8): 14139-14153. doi: 10.3934/math.2022779

Related Papers:

[1]	Shuo Wang, Juan Liu, Xindong Zhang . Properties of solutions for fractional-order linear system with differential equations. AIMS Mathematics, 2022, 7(8): 15704-15713. doi: 10.3934/math.2022860
[2]	Sadia Arshad, Iram Saleem, Ali Akgül, Jianfei Huang, Yifa Tang, Sayed M Eldin . A novel numerical method for solving the Caputo-Fabrizio fractional differential equation. AIMS Mathematics, 2023, 8(4): 9535-9556. doi: 10.3934/math.2023481
[3]	Abdon Atangana, Seda İğret Araz . A successive midpoint method for nonlinear differential equations with classical and Caputo-Fabrizio derivatives. AIMS Mathematics, 2023, 8(11): 27309-27327. doi: 10.3934/math.20231397
[4]	Muath Awadalla, Abdul Hamid Ganie, Dowlath Fathima, Adnan Khan, Jihan Alahmadi . A mathematical fractional model of waves on Shallow water surfaces: The Korteweg-de Vries equation. AIMS Mathematics, 2024, 9(5): 10561-10579. doi: 10.3934/math.2024516
[5]	Khalid K. Ali, K. R. Raslan, Amira Abd-Elall Ibrahim, Mohamed S. Mohamed . On study the fractional Caputo-Fabrizio integro differential equation including the fractional q-integral of the Riemann-Liouville type. AIMS Mathematics, 2023, 8(8): 18206-18222. doi: 10.3934/math.2023925
[6]	Gulalai, Shabir Ahmad, Fathalla Ali Rihan, Aman Ullah, Qasem M. Al-Mdallal, Ali Akgül . Nonlinear analysis of a nonlinear modified KdV equation under Atangana Baleanu Caputo derivative. AIMS Mathematics, 2022, 7(5): 7847-7865. doi: 10.3934/math.2022439
[7]	Eman A. A. Ziada, Salwa El-Morsy, Osama Moaaz, Sameh S. Askar, Ahmad M. Alshamrani, Monica Botros . Solution of the SIR epidemic model of arbitrary orders containing Caputo-Fabrizio, Atangana-Baleanu and Caputo derivatives. AIMS Mathematics, 2024, 9(7): 18324-18355. doi: 10.3934/math.2024894
[8]	Yudhveer Singh, Devendra Kumar, Kanak Modi, Vinod Gill . A new approach to solve Cattaneo-Hristov diffusion model and fractional diffusion equations with Hilfer-Prabhakar derivative. AIMS Mathematics, 2020, 5(2): 843-855. doi: 10.3934/math.2020057
[9]	Ritu Agarwal, Mahaveer Prasad Yadav, Dumitru Baleanu, S. D. Purohit . Existence and uniqueness of miscible flow equation through porous media with a non singular fractional derivative. AIMS Mathematics, 2020, 5(2): 1062-1073. doi: 10.3934/math.2020074
[10]	Thanin Sitthiwirattham, Rozi Gul, Kamal Shah, Ibrahim Mahariq, Jarunee Soontharanon, Khursheed J. Ansari . Study of implicit-impulsive differential equations involving Caputo-Fabrizio fractional derivative. AIMS Mathematics, 2022, 7(3): 4017-4037. doi: 10.3934/math.2022222

Abstract

1. Introduction

Fractional calculus is a generalization of classical integer calculus. Just like the powerful application of classical calculus, the effectiveness of fractional calculus does not depend on its mathematical properties, but on its ability to describe related phenomena. Because it has genetic characteristics and can well describe memory, the modeling of many phenomena mostly depends on fractional calculus. It has become a useful tool to describe complex dynamics in engineering, industrial scientific application, biomedical science and technological development (see ^[1,2] and the references therein). As we all know, there is no universal definition of fractional calculus, and fractional differential operators have many forms, such as Riemann-Liouville, Caputo, Riesz, Riesz-Caputo, Weyl, Hadamard, Chen, etc. Many researchers are still committed to introducing new fractional derivative operators and applying these new operators to some practical problems ^[3,4]. Of course, each constructed fractional operator has its specific characteristics and properties, which are suitable and easy to represent specific problems. The list of reasonable properties of fractional operators has been given in ^[5]. In addition, some researchers have proposed that if the differential operator does not satisfy the exponential law, it cannot be called derivative or fractional derivative. Labora and Nieto and Rodríguez-López proved that there is no a fractional derivative that verifies index law ^[6].

Fractional calculus has become an active research field for some reasons or weaknesses: the case of the local singular kernel and the case of the nonlocal nonsingular kernel. The fractional derivatives such as Riemann-Liouville type and Caputo type, which are widely used in many models, have singular kernels in their definitions. In recent years, the development of new nonlocal fractional derivatives with nonsingular kernels have attracted great interest ^[7,8]. The idea is to have more types of nonlocal fractional derivatives, and which fractional order model is appropriate will be measured in the simulation. In order to avoid the singular kernel problem, Caputo and Fabrizio proposed a new fractional derivative, which uses the exponential function as the kernel function ^[9]. The emergence of the new definition of fractional derivative with exponential kernel is an answer to the requirement that many classical constitutive equations can not fully simulate the dissipation process of energy (heat), mass (diffusion) and stress behavior of many new materials in modern technology. In many investigations, it has been found that the new operator can effectively describe various processes in the best way (for example, see ^[10,11]). Caputo-Fabrizio derivative has also been used to represent many problems in various fields, such as, biology, infectious diseases and even to study the evolution of COVID-19 epidemic, which can be found in ^{[12,13,14,15,16,17,18]}. The concept of Caputo-Fabrizio fractional derivative and its applications have been modified in literatures ^[19,20,21]. In ^[21], the logistic differential equation of fractional order and nonsingular kernel was solved and the analytical solution was obtained. For more information on the meaning of nonsingular kernel, please refer to ^[22,23]. For the solutions of fractional differential equations with singular or nonsingular kernels, see ^{[24,25,26,27,28,29,30,31,32,33]}.

Inspired by ^[21], this paper studies the solutions of three kinds of classical differential equations with the Caputo-Fabrizio fractional derivative: the Riccati equation, the anti logistic equation and the simple nonlinear model for the ENSO phenomenon. Some of the examples considered are classical ones in order to obtain the comparison of results under different fractional order operators. The purpose is to give some new results of classical differential equations. The structure of this paper is as follows: in Section 2, the related properties of Caputo-Fabrizio fractional order operators are reviewed. In Section 3, the implicit solutions are studied for the specific equations, and the image of the implicit solutions are drawn. Section 4 summarizes the work of this paper.

2. Basic properties of Caputo-Fabrizio fractional derivative

Definition 2.1. Fractional derivative in Caputo sense defined as

$\begin{equation} ^CD_t^\alpha f(t) = \frac{1}{\Gamma(1-\alpha)}\int_{0}^t(t-\tau)^{-\alpha}f'(\tau){\rm d}\tau, \end{equation}$

(2.1)

in which $0 < \alpha < 1$ is the fractional order, $\Gamma(s) = \int_0^{+\infty}{\rm e}^{-t}t^{s-1}{\rm d}t$ is the Gamma function.

Definition 2.2. The answer of Caputo and Fabrizio to the new call in modeling of relaxation process in dissipative phenomena was the following constitutive definition ^[9,18,19,26]

$\begin{equation} ^{CF}D_t^\alpha f(t) = \frac{M(\alpha)}{1-\alpha}\int_{0}^t{\rm e}^{-\frac{\alpha}{1-\alpha}(t-\tau)}f'(\tau){\rm d}\tau, \end{equation}$

(2.2)

in which $f$ is a real smooth function, $M(\alpha)$ is a normalization function such that $M(0) = M(1) = 1$ .

This is the definition of the Caputo-type because there is a derivative under the integral sign. It is analogous to the Caputo fractional derivative in the fact that one replaces the constant $\frac{1}{\Gamma(1-\alpha)}$ by $\frac{M(\alpha)}{1-\alpha}$ and the singular kernel

$K(t, \tau) = (t-\tau)^{-\alpha}$

is replaced by the exponential function kernel

$\mathcal{K}(t, \tau) = {\rm e}^{-\frac{\alpha}{1-\alpha}(t-\tau)},$

but now the kernel $\mathcal{K}(t, \tau)$ is no singularity for $t = \tau$ .

By the Definition (2.2), it follows directly that if $f(t) = C$ ( $C$ is a constant), then $^{CF}D_t^\alpha C = 0$ as in the Caputo sense (2.1). Moreover, it follows that

$\begin{equation} \lim\limits_{\alpha\rightarrow1} [^{CF}D_t^\alpha f(t)] = \frac{\rm d}{\rm dt}f(t), \end{equation}$

(2.3)

and

$\begin{equation} \lim\limits_{\alpha\rightarrow 0} [^{CF}D_t^\alpha f(t)] = f(t)-f(a). \end{equation}$

(2.4)

Then, the Caputo-Fabrizio fractional integral (anti-derivative) of order $\alpha$ was proposed as

$\begin{equation} ^{CF}I^\alpha g(t) = \frac{2(1-\alpha)}{(2-\alpha)M(\alpha)}g(t)+\frac{2\alpha}{(2-\alpha)M(\alpha)}\int_{0}^tg(\tau){\rm d}\tau, \; t\geq0, \end{equation}$

(2.5)

in which $g\in H^1$ , $0 < \alpha\leq1$ .

Losada and Nieto improved the original definition of the fractional derivative of Caputo-Fabrizio and suggested a new definition ^[19], namely

$\begin{equation} ^{CF}D_t^\alpha f(t) = \frac{1}{1-\alpha}\int_{0}^t{\rm e}^{-\frac{\alpha}{1-\alpha}(t-\tau)}f'(\tau){\rm d}\tau, \end{equation}$

(2.6)

here $M(\alpha)$ is accepted to equal 1. The Caputo-Fabrizio derivatives (2.6) of some elementary functions have been derived in ^[23].

The corresponding fractional integral of a function $g$ is

$\begin{equation} ^{CF}I^\alpha g(t) = (1-\alpha)[g(t)-g(0)]+\alpha\int_{0}^tg(\tau){\rm d}\tau. \end{equation}$

(2.7)

In fact, the integral of the function given by Caputo and Fabrizio is the average of the function itself and its Riemann-Liouville integral.

Thus,

$\begin{equation} ^{CF}I^\alpha [^{CF}D_t^\alpha f(t)] = f(t)+c, \end{equation}$

(2.8)

where $c$ an arbitrary constant. However, as remarked in ^[18,21]

$\begin{equation} ^{CF}D_t^\alpha [^{CF}I^\alpha f(t)] = f(t)-f(0){\rm e}^{-\frac{\alpha}{1-\alpha}t}. \end{equation}$

(2.9)

3. Solutions of some typical nonlinear differential equations

In this section, some nonlinear ordinary differential equations with Caputo-Fabrizio fractional derivative will be solved, and the calculated results of different fractional orders are compared.

Example 1. Consider the $\alpha$ -th order fractional Riccati equation,

$\begin{equation} ^{CF}D_t^\alpha y(t) = -y^2(t)+1, \;\;\;\;t > 0, \;\;0 < \alpha\leq1, \end{equation}$

(3.1)

subject to the initial condition $y(0) = 0$ .

In the case of $\alpha = 1$ , the fractional equation is simplified to the classical integer-order Riccati differential equation. The importance of these equations usually appears in the optimal control problem. The feedback gain of linear quadratic optimal control depends on the solution of Riccati differential equation, which must be found in the whole time range of the control process ^[34,35].

When $\alpha = 1$ , the exact solution is given in ^[35]

$\begin{equation} y(t) = \frac{{\rm e}^{2t}-1}{{\rm e}^{2t}+1}, \end{equation}$

(3.2)

and we can observe that, as $t\rightarrow \infty$ , $y(t)\rightarrow 1$ .

Let $y(t)$ is a solution of (3.1), integrating of (3.1) can be obtained

$\begin{equation} ^{CF}I^\alpha[^{CF}D_t^\alpha y(t)] = ^{CF}I^\alpha[-y^2(t)+1]. \end{equation}$

(3.3)

By (2.8), the following expression can be obtained,

$\begin{equation} y(t)-y(0) = (1-\alpha)[-y^2(t)+1-(-y^2(0)+1)]+\alpha\int_{0}^t[-y^2(\tau)+1]{\rm d}\tau. \end{equation}$

(3.4)

Sort out (3.4) and we can get

$\begin{equation} y(t) = -(1-\alpha)y^2(t)+\alpha\int_{0}^t[-y^2(\tau)+1]{\rm d}\tau. \end{equation}$

(3.5)

Taking the first derivative,

$\begin{equation} y'(t) = -2(1-\alpha)y(t)y'(t)+\alpha[-y^2(t)+1], \end{equation}$

(3.6)

$\begin{equation} y'(t)+2(1-\alpha)y(t)y'(t) = \alpha[-y^2(t)+1]. \end{equation}$

(3.7)

In the case of $\alpha = 1$ , (3.7) is restored to the integer-order ordinary differential equation, the constants $-1$ and $1$ are solutions. For $0 < \alpha < 1$ , when $y(t)\neq-1, 1$ , we rewrite (3.7) as following

$\begin{equation} \frac{y'(t)}{-y^2(t)+1}+(1-\alpha)\frac{2y(t)y'(t)}{-y^2(t)+1} = \alpha. \end{equation}$

(3.8)

Integrating of (3.8) can be obtained

$\begin{equation} (2\alpha-1)\ln|1-y^2|-\ln(1-y)^2 = 2(\alpha t+c). \end{equation}$

(3.9)

Therefore

$\begin{equation} |1-y|^{2\alpha-3}\cdot|1+y|^{2\alpha-1} = {\rm e}^{2\alpha t}\cdot {\rm e}^{2c}. \end{equation}$

(3.10)

By the initial condition $y(0) = 0$ , we can get

$\begin{equation} {\rm e}^{2c} = 1, \end{equation}$

(3.11)

then $c = 0$ .

Therefore, we obtain the implicit solution of (3.1) is

$\begin{equation} |1-y|^{2\alpha-3}\cdot|1+y|^{2\alpha-1} = {\rm e}^{2\alpha t}. \end{equation}$

(3.12)

It can be verified that the implicit solution (3.12) is consistent with (3.2) when $\alpha = 1$ .

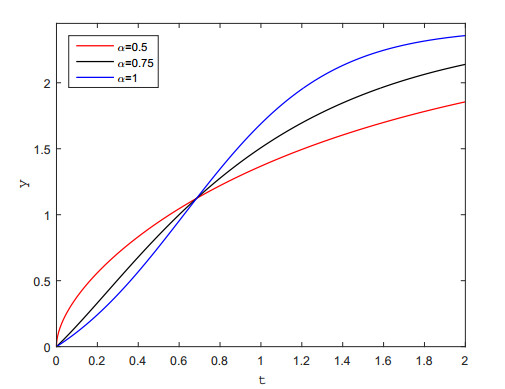
The implicit solutions (3.12) for different fractional order $\alpha$ is plotted in . As can be seen from , the higher the $\alpha$ is, the more the corresponding image is above.

Figure 1. Solutions of the fractional Riccati equation for the initial condition

$y(0) = 0$ .

DownLoad: Full-Size Img PowerPoint

Table 1 shows a comparison of the results between the Riccati equation under Caputo fractional derivative solved by the modified homotopy perturbation method in ^[35] and the values of (3.12) under Caputo-Fabrizio fractional derivative. From the comparison in , it is clear that when $\alpha$ = $0.5, 0.75$ the values of (3.12) is smaller than the approximate solutions in ^[35]. It can also be found that the value of the solution under the Caputo-Fabrizio fractional derivative changes more gently from $\alpha = 0.75$ to $\alpha = 1$ . It can also be seen from that when $\alpha = 1$ , the degree of solution coincidence in the two cases is high, indicating that the results of the two forms of fractional operators are consistent when the order tends to integer order.

Table 1. Comparison between implicit solutions (3.12) and approximate solutions under Caputo fractional derivative.

$t$	Approximate solutions in ^[35]			Implicit solutions (3.12)
$t$	$\alpha=0.5$	$\alpha=0.75$	$\alpha=1$	$\alpha=0.5$	$\alpha=0.75$	$\alpha=1$
$t=0.0$	0.0	0.0	0.0	0.0	0.0	0.0
$t=0.1$	0.273875	0.184795	0.099668	0.048771	0.073513	0.099668
$t=0.2$	0.454125	0.313795	0.197375	0.095163	0.143775	0.197375
$t=0.3$	0.573932	0.414562	0.291312	0.139292	0.210478	0.291313
$t=0.4$	0.644422	0.492889	0.379944	0.181269	0.273435	0.379949
$t=0.5$	0.674137	0.462117	0.462078	0.221199	0.332556	0.462117
$t=0.6$	0.671987	0.597393	0.535867	0.259182	0.387834	0.537050
$t=0.7$	0.648003	0.631772	0.603631	0.295312	0.439322	0.604368
$t=0.8$	0.613306	0.660412	0.661706	0.329680	0.487123	0.664037
$t=0.9$	0.579641	0.687960	0.709919	0.362372	0.531372	0.716298
$t=1.0$	0.558557	0.718260	0.746032	0.393469	0.572229	0.761594
¹ The Riccati equation under Caputo fractional derivative is solved by the modified homotopy perturbation method in ^[35].

| Show Table

DownLoad: CSV

Example 2. Consider the following fractional Riccati equation,

$\begin{equation} ^{CF}D_t^\alpha y(t) = 2y(t)-y^2(t)+1, \;\;\;\;t > 0, \;\;0 < \alpha\leq1, \end{equation}$

(3.13)

subject to the initial condition $y(0) = 0$ .

When $\alpha = 1$ , the exact solution is

$\begin{equation} y(t) = 1+\sqrt{2}\tanh(\sqrt{2}t+\frac{1}{2}\ln(\frac{\sqrt{2}-1}{2}+1)), \end{equation}$

(3.14)

and we can get that, as $t\rightarrow \infty$ , $y(t)\rightarrow 1+\sqrt{2}$ .

Let $y(t)$ is a solution of (3.13), similar to the method of Example 1, it can be obtained that

$\begin{equation} y'(t) = (1-\alpha)[2y'(t)-2y(t)y'(t)]+\alpha[2y(t)-y^2(t)+1], \end{equation}$

(3.15)

$\begin{equation} (2\alpha-1)y'(t)+2(1-\alpha)y(t)y'(t) = \alpha[2y(t)-y^2(t)+1]. \end{equation}$

(3.16)

When $\alpha = 1$ , (3.16) is restored to the classical ordinary differential equation, the constants $1+\sqrt{2}$ and $1-\sqrt{2}$ are solutions. For $0 < \alpha < 1$ , when $y(t)\neq1\pm\sqrt{2}$ , we rewrite (3.16) as following

$\begin{equation} (\alpha-1)\cdot\frac{2y'(t)-2y(t)y'(t)}{2y(t)-y^2(t)+1}+\frac{y'(t)}{2y(t)-y^2(t)+1} = \alpha. \end{equation}$

(3.17)

Integrating of (3.17) can be obtained

$\begin{equation} (\alpha-1)\ln|2y(t)-y^2(t)+1|+\frac{1}{2\sqrt{2}}\ln|\frac{y(t)-1+\sqrt{2}}{y(t)-1-\sqrt{2}}| = \alpha t+c. \end{equation}$

(3.18)

Therefore

$\begin{equation} |2y(t)-y^2(t)+1|^{\alpha-1}\cdot|\frac{y(t)-1+\sqrt{2}}{y(t)-1-\sqrt{2}}|^{\frac{1}{2\sqrt{2}}} = {\rm e}^{\alpha t}\cdot e^{c}. \end{equation}$

(3.19)

By the initial condition $y(0) = 0$ , we can get

$\begin{equation} {\rm e}^{c} = (\sqrt{2}-1)^{\frac{1}{\sqrt{2}}}. \end{equation}$

(3.20)

Therefore, we obtain the implicit solution of (3.13) is

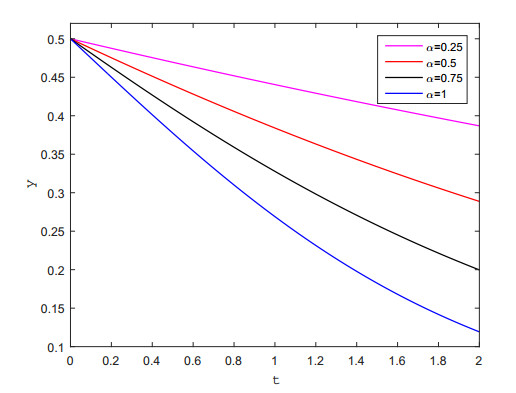
$\begin{equation} |y-1+\sqrt{2}|^{\alpha-1+\frac{\sqrt{2}}{4}}\cdot|y-1-\sqrt{2}|^{\alpha-1-\frac{\sqrt{2}}{4}} = (\sqrt{2}-1)^{\frac{1}{\sqrt{2}}}{\rm e}^{\alpha t}. \end{equation}$

(3.21)

The implicit solutions (3.21) for different fractional order $\alpha$ is plotted in Figure 2. Table 2 shows the comparison between the approximate solutions under Caputo fractional derivative solved in ^[35] and the values of (3.21) under Caputo-Fabrizio fractional derivative. From the results in Table 2, it is clear that the values of (3.21) is smaller than the approximate solutions in ^[35] in most cases and the growth of value is also relatively flat. It can also be seen from that when $\alpha = 1$ , the degree of solution coincidence in the two cases is high.

Figure 2. Solutions of the fractional Riccati equation for the initial condition

$y(0) = 0$ .

DownLoad: Full-Size Img PowerPoint

Table 2. Comparison between implicit solutions (3.21) and approximate solutions under Caputo fractional derivative in ^[35].

$t$	Approximate solutions in ^[35]			Implicit solutions (3.21)
$t$	$\alpha=0.5$	$\alpha=0.75$	$\alpha=1$	$\alpha=0.5$	$\alpha=0.75$	$\alpha=1$
$t=0.0$	0.0	0.0	0.0	0.0	0.0	0.0
$t=0.1$	0.321730	0.216866	0.110294	0.376371	0.159532	0.110295
$t=0.2$	0.629666	0.428892	0.241965	0.559950	0.331427	0.241977
$t=0.3$	0.940941	0.654614	0.395106	0.707172	0.506890	0.395105
$t=0.4$	1.250737	0.891404	0.568115	0.833517	0.679413	0.567812
$t=0.5$	1.549439	1.132763	0.757564	0.945223	0.844685	0.756014
$t=0.6$	1.825456	1.370240	0.958259	1.045639	1.000123	0.953566
$t=0.7$	2.066523	1.594278	1.163459	1.136858	1.144380	1.152949
$t=0.8$	2.260633	1.794879	1.365240	1.220318	1.276947	1.346364
$t=0.9$	2.396839	1.962239	1.554960	1.297077	1.397869	1.526911
$t=1.0$	2.466004	2.087384	1.723810	1.367947	1.507537	1.689498

| Show Table

DownLoad: CSV

Example 3. Consider the following initial value problem,

$\begin{equation} ^{CF}D_t^\alpha y(t) = -y(t)+y^2(t), \;\;\;\;t > 0, \;\;0 < \alpha\leq1, \end{equation}$

(3.22)

subject to the initial condition $y(0) = \frac{1}{2}$ .

The equation in the form of (3.22) is called anti (inverse) logistic equation (logistic equation for population decrease). The anti logistic equation is different from the logistic equation, which is mainly reflected in that the logistic equation bifurcates in a certain period of time, and then forms a dynamic nonlinear chaotic state, while the anti logistic equation does not have this property, that is, it has no chaotic state. It is well-known that both logistic equation and anti logistic equation have significant application not only in population dynamics, i.e., in the biology and demography, but also in the chemistry, economics and sociology.

When $\alpha = 1$ , the exact solution is

$\begin{equation} y(t) = \frac{1}{{\rm e}^{t}+1}. \end{equation}$

(3.23)

Let $y(t)$ is a solution of (3.22), it can be obtained that

$\begin{equation} y'(t) = (1-\alpha)[-y'(t)+2y(t)y'(t)]+\alpha[-y(t)+y^2(t)], \end{equation}$

(3.24)

$\begin{equation} (2-\alpha)y'(t)-2(1-\alpha)y(t)y'(t) = \alpha[-y(t)+y^2(t)]. \end{equation}$

(3.25)

When $\alpha = 1$ , (3.25) is restored to the classical ordinary differential equation, the constants $0$ and $1$ are solutions. For $0 < \alpha < 1$ , when $y(t)\neq0, 1$ , we rewrite (3.25) as following

$\begin{equation} (2-\alpha)\cdot\frac{y'(t)-2y(t)y'(t)}{-y(t)+y^2(t)}+2\cdot\frac{y'(t)}{-1+y(t)} = \alpha. \end{equation}$

(3.26)

Integrating of (3.26) can be obtained

$\begin{equation} -(2-\alpha)\ln|y-y^2|+2\ln|1-y| = \alpha t+c. \end{equation}$

(3.27)

Therefore

$\begin{equation} \frac{(1-y)^2}{|y-y^2|^{2-\alpha}} = {\rm e}^{\alpha t}\cdot {\rm e}^{c}. \end{equation}$

(3.28)

By the initial condition $y(0) = \frac{1}{2}$ , we can get

$\begin{equation} {\rm e}^{c} = 4^{1-\alpha}. \end{equation}$

(3.29)

Therefore, we obtain the implicit solution of (3.22) is

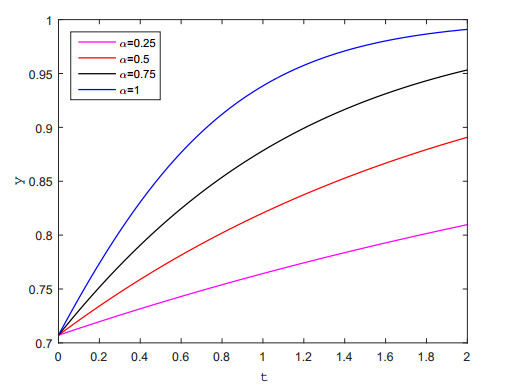
$\begin{equation} |1-y|^\alpha\cdot |y|^{\alpha-2} = 4^{1-\alpha}\cdot {\rm e}^{\alpha t}. \end{equation}$

(3.30)

The implicit solutions (3.30) for different fractional order $\alpha$ is plotted in Figure 3. It can be seen from Figure 3 that, unlike the logistic equation, the solution of the anti logistic equation decreases, and the larger the fractional order is, the lower the image.

Figure 3. Solutions of the fractional anti logistic equation for the initial condition

$y(0) = \frac{1}{2}$ .

DownLoad: Full-Size Img PowerPoint

Example 4. Consider the following initial value problem,

$\begin{equation} ^{CF}D_t^\alpha y(t)-y(t)[1-y^2(t)] = 0, \;\;\;\;t > 0, \;\;0 < \alpha\leq1, \end{equation}$

(3.31)

subject to the initial condition $y(0) = \frac{\sqrt{2}}{2}$ .

The prototype of (3.31) is the nonlinear model of El Ni $\widetilde{\rm n}$ o/Southern Oscillation (ENSO) phenomenon and Allen-Cahn equation. Initially, a simple nonlinear model for the ENSO phenomenon was proposed in ^[36], as shown below

$\begin{equation} \frac{{\rm d}T}{{\rm d} t} = T-T^3, \end{equation}$

(3.32)

in which $T$ represents the amplitude of the growing disturbance. In recent years, the new fractional derivative to the nonlinear ENSO model was discussed in ^[37,38]. On the other hand, the classical 1D Allen-Cahn equation is given as follows

$\begin{equation} \frac{\partial u}{\partial t}-\varepsilon^2 u_{xx}-u[1-u^2] = 0, \end{equation}$

(3.33)

which was used to simulate phase separation and mean curvature motion in binary mixtures ^[39,40,41]. Here, (3.31) can be considered as the special case of (3.33) in which $\varepsilon = 0$ and the derivative with respect to time is change into fractional derivative.

When $\alpha = 1$ , the exact solution of (3.31) is

$\begin{equation} y(t) = \frac{1}{\sqrt{{\rm e}^{-2t}+1}}, \end{equation}$

(3.34)

$y(t)\rightarrow 1$ when $t\rightarrow \infty$ .

Let $y(t)$ is a solution of (3.31), it can be obtained that

$\begin{equation} y'(t) = (1-\alpha)[y'(t)-3y(t)y'(t)]+\alpha[y(t)-y^3(t)], \end{equation}$

(3.35)

$\begin{equation} \alpha y'(t)+3(1-\alpha)y^2(t)y'(t) = \alpha[y(t)-y^3(t)]. \end{equation}$

(3.36)

When $\alpha = 1$ , (3.36) is restored to the classical ordinary differential equation, the constants $0$ and $1$ are solutions. For $0 < \alpha < 1$ , when $y(t)\neq0, 1$ , we rewrite (3.36) as following

$\begin{equation} \alpha\cdot\frac{y'(t)}{y(t)-y^3(t)}+(1-\alpha)\cdot\frac{3y^2(t)y'(t)}{y(t)-y^3(t)} = \alpha. \end{equation}$

(3.37)

Integrating of (3.37) can be obtained

$\begin{equation} 2\alpha\ln|y|+(2\alpha-3)\ln|y^2-1| = 2(\alpha t+c). \end{equation}$

(3.38)

Therefore

$\begin{equation} |y^2-1|^{2\alpha-3}\cdot|y|^{2\alpha} = {\rm e}^{2\alpha t}\cdot {\rm e}^{2c}. \end{equation}$

(3.39)

By the initial condition $y(0) = \frac{\sqrt{2}}{2}$ , we can get

$\begin{equation} {\rm e}^{2c} = 2^{3-3\alpha}. \end{equation}$

(3.40)

Therefore, the implicit solution of (3.31) is

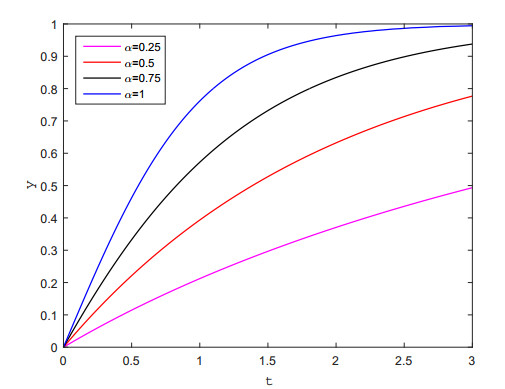
$\begin{equation} |y^2-1|^{2\alpha-3}\cdot|y|^{2\alpha} = 8^{1-\alpha}\cdot {\rm e}^{2\alpha t}. \end{equation}$

(3.41)

The implicit solutions (3.41) for different fractional order $\alpha$ is plotted in Figure 4. It can be seen from Figure 4 that, the solutions of (3.31) are increase, and the larger the fractional order is, the more the image is above.

Figure 4. Solutions of (3.31) for the initial condition

$y(0) = \frac{\sqrt{2}}{2}$ .

DownLoad: Full-Size Img PowerPoint

4. Conclusions

In this paper, the solutions of several kinds of classical differential equations with Caputo-Fabrizio derivative are studied. This derivative has a nonsingular kernel which can effectively describe various processes in the best way. The implicit solutions of the problem are obtained, and the solutions under different fractional orders are compared intuitively through images. In addition, by comparing the results obtained in this paper with those under Caputo fractional derivative, it is found that the solutions changes relatively gently under Caputo-Fabrizio fractional derivatives. It can be concluded that the selection of appropriate fractional derivative and appropriate fractional order is very important in the modeling process. Moreover, it can be found that the calculation process of the differential equation under Caputo-Fabrizio derivative is relatively simple and convenient, which is not possessed by other types of fractional derivatives.

With the continuous improvement and deepening of fractional calculus theory, there are many different types of fractional calculus definitions. The vitality of fractional calculus does not only depend on its mathematical properties, but also on its ability to describe relevant practical problems. Therefore, the direction of further research is to verify which fractional order operator can describe more accurately according to practical problems.

Acknowledgments

This work is supported the Major Projects of Natural Sciences of University in Jiangsu Province of China (No.18KJA110003), and the collaborative innovation base of Jiangsu Maritime Intelligent shipping research.

Conflict of interest

The author declares that the research was conducted in the absence of any conflict of interest.

References

[1]	Z. Cui, M. Shi, Z. Wang, Bifurcation in a new fractional model of cerebral aneurysm at the circle of Willis, Int. J. Bifurcat. Chaos, 31 (2021), 2150135. https://doi.org/10.1142/S0218127421501352 doi: 10.1142/S0218127421501352
[2]	Z. Cui, Z. Wang, Primary resonance of a nonlinear fractional model for cerebral aneurysm at the circle of Willis, Nonlinear Dyn., in press. https://doi.org/10.1007/s11071-022-07445-z
[3]	G. Sales-Teodoro, J. Tenreiro Machado, E. Capelas De Oliveira, A review of definitions of fractional derivatives and other operators, J. Comput. Phys., 388 (2019), 195–208. https://doi.org/10.1016/j.jcp.2019.03.008 doi: 10.1016/j.jcp.2019.03.008
[4]	R. Goyal, P. Agarwal, A. Parmentier, C. Cesarano, An extension of Caputo fractional derivative operator by use of Wiman's function, Symmetry, 13 (2021), 2238. https://doi.org/10.3390/sym13122238 doi: 10.3390/sym13122238
[5]	M. Ortigueira, J. Tenreiro Machado, What is a fractional derivative? J. Comput. Phys., 293 (2015), 4–13. https://doi.org/10.1016/j.jcp.2014.07.019 doi: 10.1016/j.jcp.2014.07.019
[6]	D. Labora, J. Nieto, R. Rodríguez-López, Is it possible to construct a fractional derivative such that the index law holds? Progr. Fract. Differ. Appl., 4 (2018), 1–3. https://doi.org/10.18576/pfda/040101 doi: 10.18576/pfda/040101
[7]	S. Momani, O. Abu Arqub, B. Maayah, Piecewise optimal fractional reproducing kernel solution and convergence analysis for the Atangana-Baleanu-Caputo model of the Lienard's equation, Fractals, 28 (2020), 2040007. https://doi.org/10.1142/S0218348X20400071 doi: 10.1142/S0218348X20400071
[8]	S. Momani, B. Maayah, O. Abu Arqub, The reproducing kernel algorithm for numerical solution of Van der Pol damping model in view of the Atangana-Baleanu fractional approach, Fractals, 28 (2020), 2040010. https://doi.org/10.1142/S0218348X20400101 doi: 10.1142/S0218348X20400101
[9]	M. Caputo, M. Fabrizio, A new definition of fractional derivative without singular kernel, Progr. Fract. Differ. Appl., 1 (2015), 73–85. https://doi.org/10.12785/pfda/010201 doi: 10.12785/pfda/010201
[10]	A. Atangana, B. Alkahtani, New model of groundwater flowing within a confine aquifer: application of Caputo-Fabrizio derivative, Arab. J. Geosci., 9 (2016), 8. https://doi.org/10.1007/s12517-015-2060-8 doi: 10.1007/s12517-015-2060-8
[11]	A. Atangana, J. Gomez-Aguilar, Decolonisation of fractional calculus rules: breaking commutativity and associativity to capture more natural phenomena, Eur. Phys. J. Plus, 133 (2018), 166. https://doi.org/10.1140/epjp/i2018-12021-3 doi: 10.1140/epjp/i2018-12021-3
[12]	A. Atangana, J. Nieto, Numerical solution for the model of RLC circuit via the fractional derivative without singular kernel, Adv. Mech. Eng., 7 (2015), 1–7. https://doi.org/10.1177/1687814015613758 doi: 10.1177/1687814015613758
[13]	S. Ullah, M. Khan, M. Farooq, Z. Hammouch, D. Baleanu, A fractional model for the dynamics of tuberculosis infection using Caputo-Fabrizio derivative, Discrete Cont. Dyn.-S, 13 (2020), 975–993. https://doi.org/10.3934/dcdss.2020057 doi: 10.3934/dcdss.2020057
[14]	A. Boudaoui, Y. El hadj Moussa, Z. Hammouch, S. Ullah, A fractional-order model describing the dynamics of the novel coronavirus (COVID-19) with nonsingular kernel, Chaos Soliton. Fract., 146 (2021), 110859. https://doi.org/10.1016/j.chaos.2021.110859 doi: 10.1016/j.chaos.2021.110859
[15]	M. ur Rahman, S. Ahmad, R. Matoog, N. Alshehri, T. Khan, Study on the mathematical modelling of COVID-19 with Caputo-Fabrizio operator, Chaos Soliton. Fract., 150 (2021), 111121. https://doi.org/10.1016/j.chaos.2021.111121 doi: 10.1016/j.chaos.2021.111121
[16]	F. Mansal, N. Sene, Analysis of fractional fishery model with reserve area in the context of time-fractional order derivative, Chaos Soliton. Fract., 140 (2020), 110200. https://doi.org/10.1016/j.chaos.2020.110200 doi: 10.1016/j.chaos.2020.110200
[17]	M. Caputo, M. Fabrizio, Applications of new time and spatial fractional derivatives with exponential kernels, Progr. Fract. Differ. Appl., 2 (2016), 1–11. https://doi.org/10.18576/pfda/020101 doi: 10.18576/pfda/020101
[18]	J. Losada, J. Nieto, Fractional integral associated to fractional derivatives with nonsingular kernels, Progr. Fract. Differ. Appl., 7 (2021), 137–143. https://doi.org/10.18576/pfda/070301 doi: 10.18576/pfda/070301
[19]	J. Losada, J. Nieto, Properties of a new fractional derivative without singular kernel, Progr. Fract. Differ. Appl., 1 (2015), 87–92. https://doi.org/10.12785/pfda/010202 doi: 10.12785/pfda/010202
[20]	H. Yépez-Martínez, J. Gómez-Aguilar, A new modified definition of Caputo-Fabrizio fractional order derivative and their applications to the Multi Step Homotopy Analysis Method (MHAM), J. Comp. Appl. Math., 346 (2019), 247–260. https://doi.org/10.1016/j.cam.2018.07.023 doi: 10.1016/j.cam.2018.07.023
[21]	J. Nieto, Solution of a fractional logistic ordinary differential equation, Appl. Math. Lett., 123 (2022), 107568. https://doi.org/10.1016/j.aml.2021.107568 doi: 10.1016/j.aml.2021.107568
[22]	N. Tuan, R. Ganji, H. Jafari, A numerical study of fractional rheological models and fractional Newell-Whitehead-Segel equation with non-local and non-singular kernel, Chinese J. Phys., 68 (2020), 308–320. https://doi.org/10.1016/j.cjph.2020.08.019 doi: 10.1016/j.cjph.2020.08.019
[23]	G. Nchama, Properties of Caputo-Fabrizio fractional operators, New Trends in Mathematical Sciences, 1 (2020), 1–25. https://doi.org/10.20852/ntmsci.2020.393 doi: 10.20852/ntmsci.2020.393
[24]	S. Roshan, H. Jafari, D. Baleanu, Solving FDEs with Caputo-Fabrizio derivative by operational matrix based on Genocchi polynomials, Math. Method. Appl. Sci., 41 (2018), 9134–9141. https://doi.org/10.1002/mma.5098 doi: 10.1002/mma.5098
[25]	M. Firoozjaee, H. Jafari, A. Lia, D. Baleanu, Numerical approach of Fokker-Planck equation with Caputo-Fabrizio fractional derivative using Ritz approximation, J. Comput. Appl. Math., 339 (2018), 367–373. https://doi.org/10.1016/j.cam.2017.05.022 doi: 10.1016/j.cam.2017.05.022
[26]	N. Djeddi, S. Hasan, M. Al-Smadi, S. Momani, Modified analytical approach for generalized quadratic and cubic logistic models with Caputo-Fabrizio fractional derivative, Alex. Eng. J., 59 (2020), 5111–5122. https://doi.org/10.1016/j.aej.2020.09.041 doi: 10.1016/j.aej.2020.09.041
[27]	M. Khader, K. Saad, Z. Hammouch, D. Baleanu, A spectral collocation method for solving fractional KdV and KdV-Burgers equations with non-singular kernel derivatives, Appl. Numer. Math., 161 (2021), 137–146. https://doi.org/10.1016/j.apnum.2020.10.024 doi: 10.1016/j.apnum.2020.10.024
[28]	S. Abbas, M. Benchohra, J. Nieto, Caputo-Fabrizio fractional differential equations with instantaneous impulses, AIMS Mathematics, 6 (2021), 2932–2946. https://doi.org/10.3934/math.2021177 doi: 10.3934/math.2021177
[29]	R. Adiguzel, Ü. Aksoy, E. Karapinar, İ. Erhan, On the solution of a boundary value problem associated with a fractional differential equation, Math. Method. Appl. Sci., in press. https://doi.org/10.1002/mma.6652
[30]	R. Sevinik-Adıgüzel, Ü. Aksoy, E. Karapinar, İ. Erhan, Uniqueness of solution for higher-order nonlinear fractional differential equations with multi-point and integral boundary conditions, RACSAM, 115 (2021), 155. https://doi.org/10.1007/s13398-021-01095-3 doi: 10.1007/s13398-021-01095-3
[31]	J. Lazreg, S. Abbas, M. Benchohra, E. Karapinar, Impulsive Caputo-Fabrizio fractional differential equations in b-metric spaces, Open Math., 19 (2021), 363–372. https://doi.org/10.1515/math-2021-0040 doi: 10.1515/math-2021-0040
[32]	H. Hammad, P. Agarwal, S. Momani, F. Alsharari, Solving a fractional-order differential equation using rational symmetric contraction mappings, Fractal Fract., 5 (2021), 159. https://doi.org/10.3390/fractalfract5040159 doi: 10.3390/fractalfract5040159
[33]	H. Khalil, M. Khalil, I. Hashim, P. Agarwal, Extension of operational matrix technique for the solution of nonlinear system of Caputo fractional differential equations subjected to integral type boundary constrains, Entropy, 23 (2021), 1154. https://doi.org/10.3390/e23091154 doi: 10.3390/e23091154
[34]	B. Anderson, J. Moore, Optimal control: linear quadratic methods, New Jersey: Prentice-Hall, 1990.
[35]	Z. Odibat, S. Momani, Modified homotopy perturbation method: application to quadratic Riccati differential equation of fractional order, Chaos Soliton. Fract., 36 (2008), 167–174. https://doi.org/10.1016/j.chaos.2006.06.041 doi: 10.1016/j.chaos.2006.06.041
[36]	M. Suarez, P. Schopf, A delayed action oscillator for ENSO, J. Atmos. Sci., 45 (1988), 3283–3287. https://doi.org/10.1175/1520-0469(1988)045<3283:ADAOFE>2.0.CO;2 doi: 10.1175/1520-0469(1988)045<3283:ADAOFE>2.0.CO;2
[37]	J. Singha, D. Kumar, J. Nieto, Analysis of an El Ni $\widetilde {\rm{n}}$ o-Southern Oscillation model with a new fractional derivative, Chaos Soliton. Fract., 99 (2017), 109–115. https://doi.org/10.1016/j.chaos.2017.03.058 doi: 10.1016/j.chaos.2017.03.058
[38]	A. Jhinga, V. Daftardar-Gejji, Dynamics and stability analysis of fractional model for El-Nino involving delay, Chaos Soliton. Fract., 151 (2021), 111233. https://doi.org/10.1016/j.chaos.2021.111233 doi: 10.1016/j.chaos.2021.111233
[39]	S. Allen, J. Cahn, A microscopic theory for antiphase boundary motion and its application to antiphase domain coarsening, Acta Metall., 27 (1979), 1085–1095. https://doi.org/10.1016/0001-6160(79)90196-2 doi: 10.1016/0001-6160(79)90196-2
[40]	T. Hou, D. Xiu, W. Jiang, A new second-order maximum-principle preserving finite difference scheme for Allen-Cahn equations with periodic boundary conditions, Appl. Math. Lett., 104 (2020), 106265. https://doi.org/10.1016/j.aml.2020.106265 doi: 10.1016/j.aml.2020.106265
[41]	J. Jia, H. Zhang, H. Xu, X. Jiang, An efficient second order stabilized scheme for the two dimensional time fractional Allen-Cahn equation, Appl. Numer. Math., 165 (2021), 216–231. https://doi.org/10.1016/j.apnum.2021.02.016 doi: 10.1016/j.apnum.2021.02.016

This article has been cited by:

1.	Ahmed E. Abouelregal, A comparative study of a thermoelastic problem for an infinite rigid cylinder with thermal properties using a new heat conduction model including fractional operators without non-singular kernels, 2022, 92, 0939-1533, 3141, 10.1007/s00419-022-02228-9
2.	Jinhong Jia, Hong Wang, Analysis of asymptotic behavior of the Caputo–Fabrizio time-fractional diffusion equation, 2023, 136, 08939659, 108447, 10.1016/j.aml.2022.108447
3.	Masakazu Onitsuka, Iz-iddine EL-Fassi, GENERALIZED CAPUTO-FABRIZIO FRACTIONAL DIFFERENTIAL EQUATION, 2024, 14, 2156-907X, 964, 10.11948/20230221
4.	Zhoujin Cui, Tao Lu, Bo Chen, Ammar Alsinai, Traveling Wave Solutions of the Conformable Fractional Klein–Gordon Equation With Power Law Nonlinearity, 2024, 2024, 2314-4629, 10.1155/2024/8367957
5.	Zhoujin Cui, Xiaorong Zhang, Tao Lu, Resonance analysis and time-delay feedback controllability for a fractional horizontal nonlinear roller system, 2024, 9, 2473-6988, 24832, 10.3934/math.20241209
6.	Zhoujin Cui, Guanghua Wei, Tao Lu, Simultaneous Resonance Analysis of a Fractional Cerebral Aneurysm Model with Double-Frequency Excitation, 2025, 13, 2523-3920, 10.1007/s42417-024-01566-y

Reader Comments

Your name:*

Email:*
© 2022 the Author(s), licensee AIMS Press. This is an open access article distributed under the terms of the Creative Commons Attribution License (http://creativecommons.org/licenses/by/4.0)

通讯作者: 陈斌, bchen63@163.com

1.
沈阳化工大学材料科学与工程学院沈阳 110142

AIMS Mathematics

1.8 3.4

Metrics

Article views(2624) PDF downloads(202) Cited by(6)

Preview PDF

Download XML

Export Citation

Article outline

Show full outline

Figures and Tables

Figures(4) / Tables(2)

AIMS Mathematics

Solutions of some typical nonlinear differential equations with Caputo-Fabrizio fractional derivative

Related Papers:

Abstract

1. Introduction

2. Basic properties of Caputo-Fabrizio fractional derivative

3. Solutions of some typical nonlinear differential equations

4. Conclusions

Acknowledgments

Conflict of interest

References

This article has been cited by:

Reader Comments

通讯作者: 陈斌, bchen63@163.com

Metrics

Figures and Tables

Other Articles By Authors

Catalog

AIMS Mathematics

Solutions of some typical nonlinear differential equations with Caputo-Fabrizio fractional derivative

Related Papers:

Abstract

1. Introduction

2. Basic properties of Caputo-Fabrizio fractional derivative

3. Solutions of some typical nonlinear differential equations

4. Conclusions

Acknowledgments

Conflict of interest

References

This article has been cited by:

Reader Comments

通讯作者: 陈斌, bchen63@163.com

Metrics

Figures and Tables

Other Articles By Authors

Related pages

Tools

Export File

Citation

Format

Content

Catalog