Generalized perimantanes diamondoid structure and their edge-based metric dimensions

1.   Introduction

Due to its superlative physical qualities and its beauty, the diamond is a renowned structure. Polishing, drilling, cutting, and heatsink in electronics are numerous practical and industrial applications of diamond. Its hardness, exceptional thermal conductivity determines by its rigor of composition. A single molecule with macroscopic size makes it into a diamond crystal. The model depicting fundamental atomic groupings undergoes alterations while going from molecules to materials, both in terms of idea and actual manifestation, as well as insignificant computational processing ^[1].

There are four types of higher diamondoids and each have been assigned with four different colors and name. The assigned colors are yellow, red, blue, and green, while the names are, nonbranched rodlike zigzag catamantanes associated with yellow. The regularly branched catamantanes are linked with blue diamondoids, chiral diamondoids are red-colored and the green-colored are perimantanes diamondoids. All these series have been isolated and found from petroleum ^[2].

A chemical/molecular graph is a hydrogen-depleted molecular structure in which the edges represent bonds and the vertices represent atoms in the underlying organic chemical compounds. The chemical graph theory is the study of these chemical graphs ^[3,4]. There are enough data available on this assumption and transformation from a chemical structure to a graph (vertex-edge-based structure). More detail can be found in the recent literature such as ^[5,6,7].

The notion of resolving set was proposed by the researcher in ^[8]. It is the first study to look at the notion of finding a graph's metric dimension using the definition of a resolving set. The least cardinality of a resolving set is the metric dimension. The impetus for inventing the notion of finding the set came from LORAN and sonar stations. After that, several academics took this concept and labeled it in a variety of ways. The idea of a resolving set is dubbed as a metric dimension in ^[9]'s study. While the researchers in ^[10,11] renamed the same notion with metric foundation or resolving set in a purely theoretical fashion. A more advanced definition of a resolving set was developed in the last decade. Researchers of ^[12] in which the idea of edge resolving set is explained. The notion is referred to as a fault-tolerant edge resolving set is defined in ^[13], and it is a generalized form of the edge resolving set. A Further generalized version of edge and resolving set is named as mixed-metric resolving set is defined in ^[14].

Many concepts and implementations sprang from the generalized approach of resolving set. In electronics ^[15], a recent innovation reveals the implementation of locating set (and its extensions). A method for studying diverse polyphenyl structures, especially for the polymer industry citation Nadeem2021b. In the broader view, this idea is used in combinatorial optimization ^[16], some complex games or robotic roving ^[17], image processing ^[8], pharmaceutical chemistry ^[18].

The job of determining a graph's resolving set is a non-deterministic polynomial-time hard problem (NP), with an unknown algorithmic difficulty ^[19,20,21]. Metric dimension or resolving set has a large literature because of its many variations and applications in various disciplines. Few research are discussed by ^{[22,23,24,25,26,27,28,29]}. There is extensive research on the edge metric only the most current and broad results will be discussed here. A generalized structure of convex polytopes are discussed in ^[30], barycentric is an operation of graph discussed in ^[31], study of edge and its base parameter studied in ^[32], necklace structure is discussed in ^[33], generalized version of edge resolving set is discussed in ^[34,35], computer networks and their edge metric based parameters are studied in ^[36,37,38]. For the mixed metric, ^[39] studied the generalized Peterson graph, rotational symmetric graph is discussed in ^[40], generalized path related graphs are studied in ^[41], generalized class of an aromatic compound are studied in ^[42,43], quartz based structure discussed in ^[44]. Moreover, some recent studies and literature are available at ^[45,46].

Given below are some basic concepts elaborated for further use in our main results.

Definition 1.1. ^[12,37] A vertex ${\alpha}\in {V}\left({G}\right)$ and a branch ${e} = {\alpha}_1{\alpha}_2\in{E}\left({G}\right),$ the distance between ${\alpha}$ and edge ${e}$ is defined as $d({e}, {\alpha}) = min\{d({\alpha}_1, {\alpha}), d({\alpha}_2, {\alpha})\}.$ Suppose ${R}_{e}\subset{V}\left({G}\right)$ is the subset of vertex set and defined as ${R}_{e} = \{{\alpha}_1, {\alpha}_2, \dots, {\alpha}_s\},$ and a branch ${e}\in{E}\left({\alpha}\right).$ The identification $r({e}|{R}_{e})$ of a branch ${e}$ with respect to ${R}_{e}$ is actually a $s-$tuple distances $(d({e}, {\alpha}_1), d({e}, {\alpha}_2), \dots, d({e}, {\alpha}_s)).$ If each branch from ${E}\left({G}\right)$ have unique identification according to ${R}_{e},$ then ${R}_{e}$ is called an edge metric resolving set of network ${\aleph}.$ The minimum count of the elements in ${R}_{e}$ is called the edge metric dimension of ${\aleph}$ and it is represented by $dim_{e}\left({G}\right).$

Definition 1.2. ^[13,37] An edge metric resolving set ${R}_{e}$ of a network ${G}$ is said to be fault-tolerant edge metric $({R}_{{e}, {f}})$ if for each ${\alpha}\in{R}_{e},$ ${R}_{e}\backslash{\alpha}$ is also a edge metric resolving set for ${G}.$ The minimum amount of the fault-tolerant edge metric resolving set is known as the fault-tolerant edge metric dimension and described as $dim_{{e}, {f}}\left({\alpha}\right).$

Definition 1.3. ^[36] A vertex $\alpha$ of a connected associated graph ${G}$ of a chemical structure, differentiates nodes ($\alpha_1$) and edges (${e}_1$), if $d\left({\alpha_1, \alpha}\right)\neq d\left({\alpha, {e}_1}\right).$ A subset ${R}_{m}$ is a mixed resolving set if any different pair of components of ${G}$ are separated by a node of ${R}_{m}.$ The minimum number of nodes in mixed resolving set for ${G}$ is named the mixed metric dimension and is denoted by $dim_{m}({G}).$ It is also known as blended version of both metric ^[8] and edge metric dimension ^[10].

In this draft, we have discussed edge metric dimension and their generalizations for the generalized perimantanes diamondoid structure and proved that each parameter depends on the copies of original or base perimantanes diamondoid structure and changes with the parameter ${n}$ or its number of copies. The next section will present some main results, conclusions are drawn and at the end, references are given for more and deep insight into this topic and structure.

2.   Construction and methodology of presented work

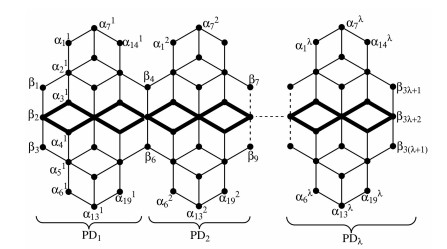
The structure shown in Figure 1, is a green-colored perimantanes diamondoid and one of a higher diamond structure. Its topological version is found in ^[1,2] and motivated by the structures applications and usage, we look into the metric-based parameters of this structure.

The perimantanes diamondoid structure has total $\left|{V}\left({PD}_{\lambda}\right)\right| = 22{\lambda}+3,$ number of vertices and total edges are $\left|{E}\left({PD}_{\lambda}\right)\right| = 38{\lambda}+2.$ The labeling of vertices and edges is described in Figure 1 and is utilized in the major results. Furthermore, vertex and edge are stated given below.

Presented below are the main results of this novel structure.

Lemma 2.1. Let ${PD}_{\lambda}$ is a structure of perimantanes diamondoid with ${\lambda}\geq1,$ and ${R}_{e}$ is the edge resolving set of ${PD}_{\lambda}$ for ${\lambda} = 1.$ Then one of the possible edge resolving set is defined as

Proof. Using the definition of edge resolving set and find the locations of each edge with regards to the chosen edge resolving set. Let ${R}_{e} = \{{\beta}_1, {\beta}_{4}, {\alpha}_{7}^1\},$ is one of a candidate subset and given below are the representations of every edge of structure of perimantanes diamondoid ${PD}_{1},$ and the structure shown in the Figure 1.

${r}\left({\beta}_{\xi}{\alpha}_{2}^{j}|{R}_{e}\right) = \left(0, 3, 2\right), {r}\left({\beta}_{\xi}{\alpha}_{3}^{j}|{R}_{e}\right) = \left(1, 4, 3\right),$ ${r}\left({\beta}_{\xi}{\alpha}_{4}^{j}|{R}_{e}\right) = \left(1, 4, 4\right), {r}\left({\beta}_{\xi}{\alpha}_{5}^{j}|{R}_{e}\right) = \left(2, 5, 6\right).$ ${r}\left({\beta}_{\xi}{\alpha}_{15}^{j}|{R}_{e}\right) = \left(3, 0, 2\right), {r}\left({\beta}_{\xi}{\alpha}_{16}^{j}|{R}_{e}\right) = \left(4, 1, 3\right),$ ${r}\left({\beta}_{\xi}{\alpha}_{17}^{j}|{R}_{e}\right) = \left(4, 1, 4\right), {r}\left({\beta}_{\xi}{\alpha}_{18}^{j}|{R}_{e}\right) = \left(5, 2, 5\right).$

It is obvious by looking at the position of every edge, that there are no two edges having the same code. In the short entire structure of perimantanes diamondoid with ${\lambda} = 1,$ has unique representations with regard to the chosen subsets. It is proved that the chosen subset is one of the possible candidates for the edge resolving set with three minimum elements in it.

Lemma 2.2. Let ${PD}_{\lambda}$ is a structure of perimantanes diamondoid with ${\lambda} = 1.$ Then

Proof. The subset chosen in the Lemma 2.1 is a base case of this lemma. In the lemma, it is proved that ${R}_{e} = \{{\beta}_1, {\beta}_{4}, {\alpha}_{7}^1\},$ is one of a candidate subset for the edge resolving set of structure of perimantanes diamondoid ${PD}_{1},$ and the structure shown in the Figure 1. Having three members in it, this proved that $dim_{e}\left({PD}_1\right)\leq3.$ For the twofold inequality, chose contrary method and we will proved that $dim_{e}\left({PD}_1\right)\neq2.$ Given below are some reasons on this assertion.

Case 2.1. Let a chosen subset ${R}_{e}^\prime$ having two distinct elements, say ${R}_{e}^{\prime} = \{{\beta}_{1}, {\beta}_{2}\}.$ The contradiction will be arise due the edges which have two distance to any of the chosen element of ${R}_{e}^\prime.$ Mathematically, it can be written as $r\left({\alpha}_{\xi}^1|{R}_{e}^{\prime}\right) = r\left({\alpha}^1_{j}|{R}_{e}^{\prime}\right) = d\left({\alpha}_{\xi}^1, {\beta}_1\right) = 2.$

Case 2.2. Let a chosen subset ${B}^\prime$ having two distinct elements, say ${R}_{e}^{\prime} = \{{\beta}_{1}, {\beta}_{3}\}.$ The contradiction will be arise due the edges which have two distance to any of the chosen element of ${R}_{e}^{\prime}.$ Mathematically, it can be written as $r\left({\alpha}_{\xi}^1|{R}_{e}^{\prime}\right) = r\left({\alpha}^1_{j}|{R}_{e}^{\prime}\right) = d\left({\alpha}_{\xi}^1, {\beta}_1\right) = 2.$

Case 2.3. Let a chosen subset ${R}_{e}^{\prime}$ having two distinct elements, say ${R}_{e}^{\prime} = \{{\beta}_{2}, {\beta}_{3}\}.$ The contradiction will be arise due the edges which have two distance to any of the chosen element of ${R}_{e}^{\prime}.$ Mathematically, it can be written as $r\left({\alpha}_{\xi}^1|{R}_{e}^{\prime}\right) = r\left({\alpha}^1_{j}|{R}_{e}^{\prime}\right) = d\left({\alpha}_{\xi}^1, {\beta}_1\right) = 2.$

Case 2.4. Let a chosen subset ${R}_{e}^{\prime}$ having two distinct elements, say ${R}_{e}^{\prime} = \{{\alpha}_{\xi}^1, {\alpha}_{1}^{j}\},$ with distinct ${\xi}, {j}.$ The contradiction will be arise due the edges which have two and three distance to any of the chosen element of ${R}_{e}^{\prime}.$ Mathematically, it can be written as $r\left({\alpha}_{\alpha}^1|{R}_{e}^{\prime}\right) = r\left({\alpha}^1_{\beta}|{R}_{e}^{\prime}\right) = d\left({\alpha}_{\alpha}^1, {\alpha}_{\xi}^1\right) = \{2, 3\}.$

All the chosen cases resulted in the same location of at least two edges of the structure and our main assertion resulted in the contradiction. So, it is proved that $dim_{e}\left({PD}_1\right)\neq2.$

Hence, $dim_{e}\left({PD}_1\right) = 3.$

Theorem 2.1. Let ${PD}_{\lambda}$ is a structure of perimantanes diamondoid with ${\lambda}\geq2.$ Then

Proof. We will proved that the entire structure of perimantanes diamondoid have $dim_{e}\left({PD}_{\lambda}\right) = {\lambda} + 2$ for every possible value of ${\lambda}\geq2.$ For this prove, we will employ the technique of mathematical induction and the base case, which is for $\lambda = 1$ and $dim_{e}\left({PD}_{1}\right) = 3,$ is already proved in the Lemmas 2.1 and 2.2. Now, suppose the assertion is true for ${\lambda} = {\chi},$ and the equation becomes

We will show that it is true for ${\lambda} = {\chi}+1.$ Suppose

Using Eqs 2.3 and 2.5 in Eq 2.6, we have

As a result, the conclusion holds for all positive integers ${\lambda}\geq1.$

Moreover, the generalized edge resolving set for the generalized structure of perimantanes diamondoid, is in the set form is given by ${R}_{e} = \{{\beta}_1, {\beta}_{3{\lambda}+1}, {\alpha}_{7}^1, {\alpha}_{7}^2, {\alpha}_{7}^3, \dots, {\alpha}_{7}^{\lambda}\}.$ This concludes.

Lemma 2.3. Let ${PD}_{\lambda}$ is a structure of perimantanes diamondoid with ${\lambda}\geq1,$ and ${R}_{{e}, {f}}$ is the fault-tolerant edge resolving set of ${PD}_{\lambda}$ for ${\lambda} = 1.$ Then one of the possible fault-tolerant edge resolving set is defined as

Proof. Using the definition of fault-tolerant edge resolving set and found the locations of each edge with regards to the chosen fault-tolerant edge resolving set. Let ${R}_{{e}, {f}} = \{{\beta}_1, {\beta}_{4}, {\alpha}_{7}^1, {\beta}_{3}, {\beta}_{6}, {\alpha}_{13}^{1}\},$ is one of a candidate subset and given below are the representations of every edge of structure of perimantanes diamondoid ${PD}_{1},$ and the structure shown in the Figure 1.

It is obvious by looking at the position of every edge, that there are no two edges having the same code. BY eliminating any element from ${R}_{{e}, {f}}$ making its cardinality five still remains the candidate for the edge resolving set and overall candidate for the fault-tolerant edge resolving set. In short entire structure of perimantanes diamondoid with ${\lambda} = 1,$ have unique representations with regard to the chosen subsets. It is proved that the chosen subset is one of a possible candidate for the fault-tolerant edge resolving set with six minimum elements in it.

Lemma 2.4. Let ${PD}_{\lambda}$ is a structure of perimantanes diamondoid with ${\lambda} = 1.$ Then

Proof. The subset chosen in the Lemma 2.3 is a base case of this lemma. In the lemma, it is proved that ${R}_{{e}, {f}} = \{{\beta}_1, {\beta}_{4}, {\alpha}_{7}^1, {\beta}_{3}, {\beta}_{6}, {\alpha}_{13}^{1}\},$ is one of a candidate subset for the fault-tolerant edge resolving set of structure of perimantanes diamondoid ${PD}_{1},$ and the structure shown in the Figure 1. Having six members in it, this proved that $dim_{e, f}\left({PD}_1\right)\leq6.$ For the twofold inequality, chose contrary method and we will proved that $dim_{e, f}\left({PD}_1\right)\neq5.$ Given below are some reasons on this assertion.

Let a chosen subset ${R}_{e, f}^\prime$ having five distinct elements, say ${R}_{e, f}^\prime = \{{\alpha}_{\xi}^1, {\alpha}_{1}^{j}\},$ with distinct ${\xi}, {j}.$ The contradiction will be arise due the edges which have two and three distance to any of the chosen element of ${R}_{e, f}^\prime.$ Mathematically, it can be written as $r\left({\alpha}_{\alpha}^1|{R}_{e, f}^\prime\right) = r\left({\alpha}^1_{\beta}|{R}_{e, f}^\prime\right) = d\left({\alpha}_{\alpha}^1, {\alpha}_{\xi}^1\right) = \{2, 3\}.$

All the chosen cases resulted in the same location of at least two edges of the structure and our main assertion resulted in the contradiction. Either the same locations of edges or by eliminating any vertex from the chosen subset not fulfilling the definition. So, it is proved that $dim_{e, f}\left({PD}_1\right)\neq5.$

Hence, $dim_{e, f}\left({PD}_1\right) = 6.$

Theorem 2.2. Let ${PD}_{\lambda}$ is a structure of perimantanes diamondoid with ${\lambda}\geq2.$ Then

Proof. We will proved that the entire structure of perimantanes diamondoid have $dim_{{e}, {f}}\left({PD}_{\lambda}\right)$ = $2\left({\lambda} + 2\right)$ for every possible value of ${\lambda}\geq2.$ For this prove, we will employ the technique of mathematical induction and the base case, which is for $\lambda = 1$ and $dim_{{e}, {f}}\left({PD}_{\lambda}\right) = 6,$ is already proved in the Lemmas 2.3 and 2.4.

Now, suppose the assertion is true for ${\lambda} = {\chi},$ and the equation becomes

We will show that it is true for ${\lambda} = {\chi}+1.$ Suppose

Using Eqs 2.10 and 2.12 in Eq 2.13, we have

As a result, the conclusion holds for all positive integers ${\lambda}\geq1.$

Moreover, the generalized fault-tolerant edge resolving set for the generalized structure of perimantanes diamondoid, is in the set form is given by ${R}_{{e}, {f}} = \{{\beta}_1, {\beta}_3, {\beta}_{3{\lambda}+1}, {\beta}_{3{\lambda}+3}, {\alpha}_{7}^1, {\alpha}_{7}^2, {\alpha}_{7}^3, \dots, {\alpha}_{7}^{\lambda}, {\alpha}_{13}^1, {\alpha}_{13}^2, {\alpha}_{13}^3, \dots, {\alpha}_{13}^{\lambda}\}.$ This concludes.

Lemma 2.5. Let ${PD}_{\lambda}$ is a structure of perimantanes diamondoid with ${\lambda}\geq1,$ and ${R}_{m}$ is the vertex-edge resolving set of ${PD}_{\lambda}$ for ${\lambda} = 1.$ Then one of the possible vertex-edge resolving set is defined as

Proof. Using the definition of mixed-metric resolving set and found the locations of each edge and vertex with regards to the chosen mixed-metric resolving set. Let ${R}_{m} = \{{\beta}_1, {\beta}_{4}, {\alpha}_{7}^1, {\alpha}_{13}^1\},$ is one of a candidate subset and given below are the representations of every edge and vertex of structure of perimantanes diamondoid ${PD}_{1},$ and the structure shown in the Figure 1.

Given below are representations of every vertex of structure of perimantanes diamondoid ${PD}_{1},$ by using the definition of shortest distances. If we found every vertex having unique positions then we will further compute the locations of edges of this structure.

Given below are representations of every edge of structure of perimantanes diamondoid ${PD}_{1},$ by using the definition of shortest distances. If we found every edge having also unique positions then we will further compare the locations of vertices with edges of this structure.

It is obvious by looking the position of every edge and every vertex, that there are no two edges having same code, there are no two vertices having the same code and there is no single edge having the same code with any vertex. In short entire structure of perimantanes diamondoid with ${\lambda} = 1,$ have unique representations. It is proved that the chosen subset is one of a possible candidate for the mixed metric resolving set with four minimum elements in it.

Lemma 2.6. Let ${PD}_{\lambda}$ is a structure of perimantanes diamondoid with ${\lambda} = 1.$ Then

Proof. The subset chosen in the Lemma 2.5 is a base case of this lemma. In the lemma, it is proved that ${R}_{m} = \{{\beta}_1, {\beta}_{4}, {\alpha}_{7}^1, {\alpha}_{13}^1\},$ is one of a candidate subset for the mixed-metric resolving set of structure of perimantanes diamondoid ${PD}_{1},$ and the structure shown in the Figure 1. Having four members in it, this proved that $dim_{m}\left({PD}_1\right)\leq4.$ For the twofold inequality, chose contrary method and we will proved that $dim_{m}\left({PD}_1\right)\neq3.$ Given below are some reasons on this assertion.

Let a chosen subset ${R}_{m}^\prime$ having three distinct elements, say ${R}_{m}^\prime = \{{\alpha}_{\xi}^1, {\alpha}_{1}^{j}\},$ with distinct ${\xi}, {j}.$ The contradiction will be arise due the either edges or edges which have two three and four distances to any of the chosen element of ${R}_{m}^\prime.$ Mathematically, it can be written as $r\left({\alpha}_{\alpha}^1|{R}_{m}^\prime\right) = r\left({\alpha}^1_{\beta}|{R}_{m}^\prime\right) = d\left({\alpha}_{\alpha}^1, {\alpha}_{\xi}^1\right)$ = $\left(2, 3, 4\right).$

All the chosen cases resulted in the same location of at least two edges or two vertices. If both the edges and vertices have unique position, then the inter positions are matched for the structure and our main assertion resulted in the contradiction. So, it is proved that $dim_{m}\left({PD}_1\right)\neq3.$

Hence, $dim_{m}\left({PD}_1\right) = 4.$

Theorem 2.3. Let ${PD}_{\lambda}$ is a structure of perimantanes diamondoid with ${\lambda}\geq2.$ Then

Proof. We will proved that the entire structure of perimantanes diamondoid have $dim_{m}\left({PD}_{\lambda}\right)$ = $2\left({\lambda} + 1\right)$ for every possible value of ${\lambda}\geq2.$ For this prove, we will employ the technique of mathematical induction and the base case, which is for $\lambda = 1$ and $dim_{m}\left({PD}_{\lambda}\right) = 4,$ is already proved in the Lemmas 2.5 and 2.6.

Now, suppose the assertion is true for ${\lambda} = {\chi},$ and the equation becomes

We will show that it is true for ${\lambda} = {\chi}+1.$ Suppose

Using Eqs 2.19 and 2.21 in Eq 2.22, we have

As a result, the conclusion holds for all positive integers ${\lambda}\geq1.$

Moreover, the generalized fault-tolerant edge resolving set for the generalized structure of perimantanes diamondoid, is in the set form is given by ${R}_{m} = \{{\beta}_1, {\beta}_{3{\lambda}+1}, {\alpha}_{7}^1, {\alpha}_{7}^2, {\alpha}_{7}^3, \dots, {\alpha}_{7}^{\lambda}, {\alpha}_{13}^1, {\alpha}_{13}^2, {\alpha}_{13}^3, \dots, {\alpha}_{13}^{\lambda}\}.$ This concludes.

3.   Conclusions

In this article, we have discussed the edge metric dimension and their generalizations for the generalized perimantanes diamondoid structure and proved that each parameter depends on the copies of original or base perimantanes diamondoid structure and changes with the parameter ${n}$ or its number of copies. Future direction can be considered as to discussed its other parameters which are also based on the metric of structure.

Conflict of interest

The authors declare no conflict of interest.