1.
Introduction
The majority of real-world MCDM problems include numerical value accumulation, which is where AOs come in. Many systems depend on data aggregation and fusion, including machine learning, decision-making, image processing and pattern recognition. The aggregation technique, in a wider sense, incorporates various pieces of knowledge to arrive at a conclusion or judgement. It has also been discovered that modeling working conditions in human cognition mechanisms using primitive data handling techniques based on crisp numbers is often difficult. As a consequence of these approaches, decision-makers (DMs) are left with hazy assumptions and uncertain decisions. As a result, in order to cope with unclear and fuzzy circumstances that occur in the real world, decision-makers need theories that allow them to comprehend ambiguous data values and sustain their decision-making requirements in accordance with the situation, whether it is pattern recognition or human cognition. In this direction, Zadeh [1] has pioneered the fuzzy set theory to describe vague information. After Zadeh, Atanassov [2] introduced intuitionistic fuzzy set (IFS), and Yager [3,4,5] introduced Pythagorean fuzzy set (PFS) which is an extension of IFS. Then Yager [6] further extended PFS to q-rung orthopair fuzzy set (q-ROFS) which is a strong model to describe vague information in the real-life problems. A q-rung orthopair fuzzy number (q-ROFN) is a pair of membership degrees (MDs) and non-membership degree (NMDs) with the property that the sum of qth power of both MDs and NMDs may be less than or identical to one. There is a parameter q involved, this increase in value of q provides an opportunity to select MDs and NMDs from a larger area. Initially, when q is increased from 1 to 2, there is a rapid increase in the area bounded between coordinate axes and the curve ˜ℵq+˘℘q=1. As the value of q increases (say after q = 2) increase in area gets slower and slower. Therefore for practical purposes, values from 2 to 10 are more useful. It can be seen that about 99% area of the unit square [0,1]×[0,1] is available for the selection of MD and NMDs when q=10. However no restriction other than q≥1 can be imposed on q. Although q is a real number, yet for an integral value it is bit easy to know about the area from where MD and NMDs are being selected [7].
AOs such as averaging and geometric operators for IFSs propsed by Xu et al. [8,9]. The notion of linear Diophantine fuzzy set (LDFS) introduced by Riaz and Hashmi [10]. A LDFS is new extension of fuzzy sets and new approach to decision making under vagueness. Riaz et al. introduced Einstein AOs [12] and prioritized AOs [11] related to LDFSs. They developed some interesting applications of these operators towards MCDM. Liu and Liu [13] initiated the idea of q-ROF Bonferroni mean AOs. Khan et al. [14] proposed the novel idea of axiomatically supported divergence measures for q-ROFS. Riaz et al. [15] introduced AOs based on generalized and group-generalized parameters related to q-RONs. Farid and Riaz [16] also introduced Einstein interactive geometric AOs with application to MCDM for q-ROFNs. Liu et al. [17] proposed the idea of q-ROF Heronian mean AOs and application related to MCDM. Saha et al. [22] proposed the novel idea of fairly AOs for q-ROFNs. Many researchers have a great work on different extensions of fuzzy set like, Sitara et al. [18] introduced q-rung picture fuzzy graph structures, Ashraf et al. [23] proposed the distance measure method with cubic picture fuzzy numbers, Chakraborty et al. [24] developed an intelligent decision model, Riaz et al. [25] discussed some properties of soft multi-set topology, Ali et al. [26] proposed Einstein geometric AOs for complex interval-valued PFS and Alosta et al. [27] solved the location selection problem by means of an integrated AHP-RAFSI technique. Akram et al. [19] proposed AOs related to complex spherical fuzzy set. Akram et al. [20] also introduced Dombi AOs for decision making under m-polar fuzzy information. Wang et al. [21] proposed Pythagorean fuzzy interactive Hamacher power AOs. Riaz et al. [28] introduced novel concept of bipolar picture fuzzy. Alcantud proposed the novel idea of softarisons [29] and characterization of soft topologies by crisp topologies [30]. Yager [31] introduced many prioritized AOs. Li and Xu [32] gave a novel idea of prioritized AOs based on the priority degrees.
In our daily lives, we come across numerous situations where a mathematical function capable of reducing a collection of numbers to a single number is needed. As a result, the AO inquiry plays a significant role in MCDM problems. Because of their broad use in fields, many researchers have recently focused on how to aggregate data. However, we often come across cases where the points to be aggregated have a strict prioritization relationship. For example, if we want to buy a plot of land to build a house based on the parameters of utility access (P1), location (P2), and cost (P3), we don't want to pay utility access for location and location for cost. That is, in this situation, there is strict prioritization among parameters, such as P1>P2>P3. To deal this type of problem Riaz et al. [33] introduced q-ROF prioritized AOs. The concept of deciding priority degrees among priority orders expands the versatility of the prioritized operators. The DM should choose the priority degree vector based on his or her preferences and the nature of the problem. Consider the preceding example of purchasing a plot to further illustrate the concept of priority degrees. Each priority level will be assigned a priority degree, which will be a true non-negative number. Since P1>P2>P3 in the preceding case. The first priority order P1>P2 is given a priority degree d1 where 0<d1<∞ and this prioritization relationship is written as P1>d1P2. Correspondingly, the priority degree d2 is allocated to the second priority order P2>d2P3 and 0<d2<∞. As a result, a two-dimensional vector d=(d1,d2) is assigned to the prioritized criteria P1>P2>P3, and the relationship is expressed as P1>d1P2>d2P3. Now, we'll look at three particular situations involving priority degrees:
1. If the first parameter is to be given top priority, the first priority degree d1 should be given a large value. Furthermore, we will illustrate in this paper that when d1→∞, the consolidated value is calculated solely by the first criterion, with the other criterion values being ignored.
2. If we consider the priority degree vector to be zero, we can see that all of the parameters become equally as important, and no prioritisation among the parameters remains.
3. There is natural prioritization among the parameters if each priority degree is equal to one. We will show Riaz et al. [33] proposed AOs and our proposed AOs based on priority degree is same.
Taking into consideration the superiority of the q-ROFs set over the other sets (as discussed above) for dealing with MCDM issues, there is a need to build some new prioritized AOs based on priority degrees. To the best of our knowledge, no work has been performed in the context of establishing such operators that take priority degrees into account among strict priority levels in a IFS, PFS and q-ROF framework. Motivated by this, the main objectives of this article are:
1. We propose some novel q-ROF prioritized averaging and geometric AOs based on the priority degrees model. Many other characteristics related to these proposed AOs and propositions are clarified in detail by providing different priority vectors, which are useful in fusing multiple q-ROF data.
2. Under the q-ROF setting, a group decision-making algorithm based on the presented prioritized AOs is presented. The defined algorithm's applicability is investigated on a real-world problem related to sustainable energy planning.
3. Ultimately, the proposed algorithm's validity is clarified by contrasting its findings to some previous techniques under q-ROFS. The mentioned algorithm has the significant strength that a decision maker could choose the priority degree vector based on his/her priorities and the nature of the problem, and thus can select the appropriate alternative(s) accordingly.
The remainder of this paper is set out as follows. Section 2 consists of a variety of basic principles related to q-ROFSs. The q-ROF prioritized AOs based on priority vector were addressed in Section 3. Based on new interaction operations, in Section 4, we present an algorithm to solve MCDM problems. Section 5 sets out an application relating to the selection of the sustainable energy source for Gwadar. Some concluding remarks and future directions are made in Section 6.
2.
Certain fundamental concepts
In this section of the paper, we keep in mind a few basics and operational principles of q-ROFNs.
Definition 2.1. [6] Assume q-ROF ˜T in Q is defines as
where ˜ℵ˜T,˘℘˜T:Q→[0,1] defines the MD and NMD of the alternative ς∈Q and ∀ς we have
Liu and Wang later proposed that q-ROFN data should be coupled with the preceding operational principles.
Definition 2.2. [34] Let ˘£1=⟨˜ℵ1,˘℘1⟩ and ˘£2=⟨˜ℵ2,˘℘2⟩ be q-ROFNs. σ>0, Then
(1) ˘£c1=⟨˘℘1,˜ℵ1⟩
(2) ˘£1∨˘£2=⟨max{˜ℵ1,˘℘1},min{˜ℵ2,˘℘2}⟩
(3) ˘£1∧˘£2=⟨min{˜ℵ1,˘℘1},max{˜ℵ2,˘℘2}⟩
(4) ˘£1⊕˘£2=⟨(˜ℵq1+˜ℵq2−˜ℵq1˜ℵq2)1/q,˘℘1˘℘2⟩
(5) ˘£1⊗˘£2=⟨˜ℵ1˜ℵ2,(˘℘q1+˘℘q2−˘℘q1˘℘q2)1/q⟩
(6) σ˘£1=⟨(1−(1−˜ℵq1)σ)1/q,˘℘σ1⟩
(7)˘£σ1=⟨˜ℵσ1,(1−(1−˘℘q1)σ)1/q⟩
Definition 2.3. [34] Let ˘£=⟨˜ℵ,˘℘⟩ be the q-ROFN, score function Ξ of ˘£ is defined as
Ξ(˘£)∈[−1,1]. The q-ROFN score shall decide its ranking, i.e. the maximum score shall determine the high q-ROFN priority. In certain situations, although, the score function isn't really beneficial for q-ROFN. It is therefore not sufficient to use the score function to evaluate the q-ROFNs. We're adding an additional function, i.e. an accuracy function.
Definition 2.4. [34] Let ˘£=⟨˜ℵ,˘℘⟩ be the q-ROFN, then an accuracy function H of ˘£ is defined as
H(˘£)∈[0,1].
Definition 2.5. Consider ˘£=⟨˜ℵ˘£,˘℘˘£⟩ and β=⟨˜ℵβ,˘℘β⟩ are two q-ROFN, and Ξ(˘£),Ξ(β) are the score function of ˘£ and β, and H(˘£),H(β) are the accuracy function of ˘£ and β, respectively, then
(a) If Ξ(˘£)>Ξ(β), then ˘£>β
(b) If Ξ(˘£)=Ξ(β), then
if H(˘£)>H(β) then ˘£>β,
if H(˘£)=H(β), then ˘£=β.
It should always be noticed that the value of score function is between 1 and 1. Riaz et al. [33] introduce another score function, to support this type of research, ˘Ξ(R)=1+˜ℵqR−˘℘qR2. We can see that 0≤˘Ξ(R)≤1. This new score function satisfy all properties of score function defined by Yager [6]. Liu and Wang [34] proposed basic weighted average and geometric AOs for q-ROFNs. Riaz et al. [33] introduced prioritized AOs for information aggregation related to q-ROFNs.
Supremacy of q-ROFNs and compared with some established theories
The superiority and comparison of ROFNs to certain systems is discussed as follows, such as fuzzy numbers (FNs), IFNs and PFNs. We can not argue about the dissatisfaction of part of the alternative in the decision-making dilemma of using FNs to take inputs.We can not consider MD and NMD quantities with a free option of operating requirements if we are using that both IFNs and PFNs. By laws, they were prohibited, e.g 0.96+0.45=1.41>1 and 0.962+0.452=1.1241>1, Which violates the particulars of IFNs and PFNs. But if we choose q=3, then the specification means 0.963+0.453=0.9759<1. The fuzzy criteria satisfy these standards so we can work with broad domain decision-making information. The Table 1 is a concise summary of q-ROFNs benefits and drawbacks with exiting theories.
3.
q-ROF prioritized aggregation operators with priority degrees
Within this section, we present the notion of q-rung orthopair fuzzy prioritized averaging (q-ROFPAd) operator with priority degrees and q-rung orthopair fuzzy prioritized geometric (q-ROFPGd) operator with priority degrees.
3.1. q-ROFPAd operator
Assume ˘£g=(˜ℵg,˘℘g)(g=1,2...,u) is the assemblage of q-ROFNs, there is a prioritization among these q-ROFNs expressed by the strict priority orders ˘£1≻d1˘£2≻d2…≻du−1˘£u−1, where ˘£u≻du˘£u+1 indicates that the q-ROFN ˘£u has du higher priority than ˘£u+1. d=(d1,d2,…,du−1) is the (u−1) dimensional vector of priority degrees. The assemblage of such q-ROFNs with strict priority orders and priority degrees is denoted by ℜd.
Definition 3.1. A q-ROFPAd operator is a mapping from ℜud to ℜd and defined as,
where ζ(d)g=T(d)g∑ug=1T(d)g, T(d)g=∏g−1q=1(˘Ξ(˘£q))dq, for each g=(2,3,…,u) and T1=1. Then q-ROFPAd is called q-rung orthopair fuzzy prioritized averaging operators with priority degrees.
Theorem 3.2. Assume ˘£g=(˜ℵg,˘℘g) is the assemblage of q-ROFNs, we can also find q-ROFPAd by
where ζ(d)g=T(d)g∑ug=1T(d)g, T(d)g=∏g−1q=1(˘Ξ(˘£q))dq, for each g=(2,3,…,u) and T1=1.
Proof. To prove this theorem, we use mathematical induction.
For u=2
Then
This shows that Eq 3.2 is true for u=2, now let that Eq 3.2 holds for u=b, i.e.,
Now u=b+1, by operational laws of q-ROFNs we have,
This shows that for u=b+1, Eq 3.2 holds. Then,
Example 3.3. Let ˘£1=(0.73,0.54), ˘£2=(0.53,0.75), ˘£3=(0.82,0.25) and ˘£4=(0.35,0.64) be the four q-ROFNs, there is strict prioritized relation in considered q-ROFNs, such that ˘£1≻d1˘£2≻d2˘£3≻d3˘£4. Priority vector d=(d1,d2,d3) is given as (5,1,1), by Eq 3.2, we take q=3 and get
and
Furthermore, the suggested q-ROFPAd operator is examined to ensure that it has idempotency and boundary properties. Their explanations are as follows:
Theorem 3.4. Assume that ˘£g=(˜ℵg,˘℘g) is the assemblage of q-ROFNs, and
Then,
where ζ(d)g=T(d)g∑ug=1T(d)g, T(d)g=∏g−1q=1(˘Ξ(˘£q))dq, for each g=(2,3,…,u) and T1=1.
Proof. Since,
and
From Eq 3.3 we have,
From Eq 3.4 we have,
Let
Then, ˘Ξ(˘£)=˜ℵq−˘℘q≤maxg(˜ℵ)q−minj(˘℘)q=˘Ξ(˘£max) So, ˘Ξ(˘£)≤˘Ξ(˘£max).
Again, ˘Ξ(˘£)=˜ℵq−˘℘q≥ming(˜ℵ)q−maxj(˘℘)q=˘Ξ(˘£min) So, ˘Ξ(˘£)≥˘Ξ(˘£min).
If, ˘Ξ(˘£)≤˘Ξ(˘£max) and ˘Ξ(˘£)≥˘Ξ(˘£min), then
If ˘Ξ(˘£)=˘Ξ(˘£max), then ˜ℵq−˘℘q=maxg(˜ℵ)q−minj(˘℘)q
Now, H(˘£)=˜ℵq+˘℘q=maxg(˜ℵ)q+ming(˘℘)q=H(˘£max)
If ˘Ξ(˘£)=˘Ξ(˘£min), then ˜ℵq−˘℘q=ming(˜ℵ)q−maxj(˘℘)q
Now, H(˘£)=˜ℵq+˘℘q=ming(˜ℵ)q+maxg(˘℘)q=H(˘£max)
Thus, from Eqs 3.5, 3.6 and 3.7, we get
Theorem 3.5. Assume that if ˘£⋄ is a q-ROFN satisfied the property, ˘£g=˘£⋄, ∀g then
Proof. Let ˘£⋄=(˜ℵ⋄,˘℘⋄) be the q-ROFN. Then by assumption, we have ˘£g=˘£⋄, ∀g gives ˜ℵg=˜ℵ⋄ and ˘℘g=˘℘⋄ ∀g. By Definition 3.1, we have ∑ug=1ζ(d)g. Then by using Theorem 3.2, we get
Corollary 3.6. If ˘£g=(˜ℵg,˘℘g) is the assemblage of largest q-ROFNs, i.e, . ˘£g=(1,0) for all g, then
Proof. We can easily obtain Corollary similar to the Theorem 3.5.
Corollary 3.7. If ˘£1=(˜ℵ1,˘℘1) is the smallest q-ROFN, i.e, . ˘£1=(0,1), then
Proof. Here, ˘£1=(0,1) then by definition of the score function, we have,
Since,
We have,
T(d)g=∏g−1q=1(˘Ξ(˘£q))dq=(˘Ξ(˘£1)d1)(˘Ξ(˘£2)d2)…(˘Ξ(˘£g−1)dg−1)=0
From Definition 3.1, we have
Theorem 3.8. Assume that ˘£g=(˜ℵg,˘℘g) and βg=(ϕg,φg) are two assemblages of q-ROFNs, if r>0 and β=(˜ℵβ,˘℘β) is a q-ROFN, then
1. q−ROFPAd(˘£1⊕β,˘£2⊕β,…˘£u⊕β)=q−ROFPAd(˘£1,˘£2,…˘£u)⊕β
2. q−ROFPAd(r˘£1,r˘£2,…r˘£u)=rq−ROFPAd(˘£1,˘£2,…˘£u)
3. q−ROFPAd(˘£1⊕β1,˘£2⊕β2,…˘£u⊕βn)=q−ROFPAd(˘£1,˘£2,…˘£n)⊕q−ROFPAd(β1,β2,…βu)
4. q−ROFPAd(r˘£1⊕β,r˘£2⊕β,…⊕r˘£u⊕β)=rq−ROFPAd(˘£1,˘£2,…˘£u)⊕β
Proof. This is trivial by definition.
q-ROFPAd operator satisfied following properties.
Property:1
Assume that ˘£g=(˜ℵg,˘℘g) is the assemblage of q-ROFNs, then we have
Proof. Given that, (d1,d2,…,du−1)→(1,1,…,1) from this we have,
by this we obtain, ζ(d)g→ζg
lim(d1,d2,…,du−1)→(1,1,…,1)q−ROFPAd(˘£1,˘£2,…˘£u)
Remark. When d1=d2=…,=du−1=1, Property:1 states that the existing q-ROFPWA operator is a particular situation of the suggested q-ROFPAd operator. As a result, q-ROFPAd operator is more generic than q-ROFPWA operator.
Property:2
Assume that ˘£g=(˜ℵg,˘℘g) is the assemblage of q-ROFNs and ˘Ξ(˘£g)≠0 for all g, then we have
Proof. Given that, (d1,d2,…,du−1)→(0,0,…,0) from this we have,
and ζ(d)g=T(d)g∑ug=1T(d)g=1n. Hence
Property:3
Assume that ˘£g=(˜ℵg,˘℘g) is the assemblage of q-ROFNs and ˘Ξ(˘£1)≠0 or 1, then we have
Proof. Here, d1→+∞ for each g=2,3,...,u we have
Because, 0<˘Ξ(˘£1)<1, ∑ug=1T(d)g=T(d)1=1 ⇒ζ(d)1=T(d)1∑ug=1T(d)1=1 and ζ(d)g=T(d)g∑ug=1T(d)g
for each g=2,3,...,u. Hence,
Remark. According to Property:3, when d1→+∞, the priority degree d1 of q-ROFN ˘£1 is very high in comparison to the priority degrees of other q-ROFNs. It indicates that q-ROFN ˘£1 is extremely essential. As a result, ˘£1 determines the aggregation result obtained by using the proposed operator q-ROFPAd in this case.
Example 3.9. Let ˘£1=(0.73,0.54), ˘£2=(0.53,0.75), ˘£3=(0.82,0.25) and ˘£4=(0.35,0.64) be the four q-ROFNs, it can easily compute that, ˘Ξ1=0.6158, ˘Ξ2=0.3635, ˘Ξ3=0.7679 and ˘Ξ4=0.3904. There is strict prioritized relation in considered q-ROFNs, such that ˘£1≻d1˘£2≻d2˘£3≻d3˘£4. In the corresponding portion we will aggregate the q-ROFNs for 4 distinct priority vectors d=(d1,d2,d3), keeping the values of priority degrees d2,d3 constant while varying the value of d1 and discussing its effect on the aggregated results, here we take q=3.
Case 1: when d=(1,1,1)
Case 2: when d=(5,1,1)
Case 3: when d=(8,1,1)
Case 4: when d=(13,1,1)
The consolidated findings from the preceding 4 cases show that as the priority degree d1 referring to q-ROFN ˘£1 rises, the aggregated value approaches the q-ROFN ˘£1 ranking values.
Property:4
Assume that ˘£g=(˜ℵg,˘℘g) is the assemblage of q-ROFNs and ˘Ξ(˘£g)≠0 for all g=1,2,...,k+1, and ˘Ξ(˘£k+1)≠1 then we have
Proof. Given that, (d1,d2,…,dk,dk+1)→(0,0,…,0,+∞). So,
for each g=2,3,…,k+1.
T(d)g=∏g−1q=1(˘Ξ(˘£q))dq=(˘Ξ(˘£1)d1)(˘Ξ(˘£2)d2)…(˘Ξ(˘£g−1)dg−1)→
(˘Ξ(˘£1))0(˘Ξ(˘£2))0…(˘Ξ(˘£k))0(˘Ξ(˘£k+1))+∞...(˘Ξ(˘£g−1))dg−1=0
∀g=k+2,k+3,…,u
So,
∑ug=1T(d)g=T(d)1=k+1 and ζ(d)g=T(d)g∑ug=1T(d)g→1k+1 for each g=1,2,3,...,k+1.
ζ(d)g=T(d)g∑ug=1T(d)g→0k+1=0 for each g=k+2,k+3,…,u.
Hence,
Remark. When (d1,d2,…,dk,dk+1)→(0,0,…,0,+∞), it means there's no prioritization association between the q-ROFNs ˘£1,˘£2,…,˘£k+1 and that all of these q-ROFNs ˘£1˘£2,…,˘£k+1 have a much higher priority than the q-ROFNs ˘£k+2˘£k+3,…,˘£u. As a result, the aggregated value is solely dependent on q-ROFNs ˘£1˘£2,…,˘£k+1, and these q-ROFNs ˘£1˘£2,…,˘£k+1 have similar weightage in the aggregation method.
Property:5
Assume that ˘£g=(˜ℵg,˘℘g) is the assemblage of q-ROFNs and ˘Ξ(˘£k+1)≠1 or 0 then we have
Proof. Given that, (d1,d2,…,dk,dk+1)→(1,1,…,1,+∞). So,
for each g=2,3,…,k+1.
T(d)g=∏g−1q=1(˘Ξ(˘£q))dq=(˘Ξ(˘£1)d1)(˘Ξ(˘£2)d2)…(˘Ξ(˘£g−1)dg−1)→
(˘Ξ(˘£1))(˘Ξ(˘£2))…(˘Ξ(˘£k))(˘Ξ(˘£k+1))+∞...(˘Ξ(˘£g−1))dg−1=0
∀g=k+2,k+3,…,u
So,
∑ug=1T(d)g→∑k+1g=1Tg and ζ(d)g=T(d)g∑ug=1T(d)g→Tg∑k+1g=1Tg for each g=1,2,3,...,k+1.
ζ(d)g=T(d)g∑ug=1T(d)g→0∑k+1g=1Tg=0 for each g=k+2,k+3,…,u.
Hence,
Remark. When (d1,d2,…,dk,dk+1)→(1,1,…,1,+∞), it means there's normal prioritization association between the q-ROFNs ˘£1,˘£2,…,˘£k+1 and that all of these q-ROFNs ˘£1˘£2,…,˘£k+1 have a much higher priority than the q-ROFNs ˘£k+2˘£k+3,…,˘£u. As a result, the aggregated value is solely dependent on q-ROFNs ˘£1˘£2,…,˘£k+1.
3.2. q-ROFPGd operator
Assume ˘£g=(˜ℵg,˘℘g)(g=1,2...,u) is the assemblage of q-ROFNs, there is a prioritization among these q-ROFNs expressed by the strict priority orders ˘£1≻d1˘£2≻d2…≻du−1˘£u−1, where ˘£u≻du˘£u+1 indicates that the q-ROFN ˘£u has du higher priority than ˘£u+1. d=(d1,d2,…,du−1) is the (u−1) dimensional vector of priority degrees. The assemblage of such q-ROFNs with strict priority orders and priority degrees is denoted by ℜd.
Definition 3.10. A q-ROFPGd operator is a mapping from ℜud to ℜd and defined as,
where ζ(d)g=T(d)g∑ug=1T(d)g, T(d)g=∏g−1q=1(˘Ξ(˘£q))dq, for each g=(2,3,…,u) and T1=1. Then q-ROFPGd is called q-rung orthopair fuzzy prioritized geometric operator with priority degrees.
Theorem 3.11. Assume ˘£g=(˜ℵg,˘℘g) is the assemblage of q-ROFNs, we can also find q-ROFPGd by
where ζ(d)g=T(d)g∑ug=1T(d)g, T(d)g=∏g−1q=1(˘Ξ(˘£q))dq, for each g=(2,3,…,u) and T1=1.
Proof. To prove this theorem, we use mathematical induction.
For u=2
Then
This shows that Eq 3.14 is true for u=2, now let that Eq 3.14 holds for u=b, i.e.,
Now u=b+1, by operational laws of q-ROFNs we have,
This shows that for u=b+1, Eq 3.14 holds. Then,
Example 3.12. Let ˘£1=(0.73,0.54), ˘£2=(0.53,0.75), ˘£3=(0.82,0.25) and ˘£4=(0.35,0.64) be the four q-ROFNs, there is strict prioritized relation in considered q-ROFNs, such that ˘£1≻d1˘£2≻d2˘£3≻d3˘£4. Priority vector d=(d1,d2,d3) is given as (5,1,1), by Eq 3.14, we take q=3 and get
and
Furthermore, the suggested q-ROFPGd operator is examined to ensure that it has idempotency and boundary properties. Their explanations are as follows:
Theorem 3.13. Assume that ˘£g=(˜ℵg,˘℘g) is the assemblage of q-ROFNs, and
Then,
where ζ(d)g=T(d)g∑ug=1T(d)g, T(d)g=∏g−1q=1(˘Ξ(˘£q))dq, for each g=(2,3,…,u) and T1=1.
Proof. Proof is same as Theorem 3.4.
Theorem 3.14. Assume that if ˘£⋄ is a q-ROFN satisfied the property, ˘£g=˘£⋄, ∀g then
Proof. Let ˘£⋄=(˜ℵ⋄,˘℘⋄) be the q-ROFN. Then by assumption, we have ˘£g=˘£⋄, ∀g gives ˜ℵg=˜ℵ⋄ and ˘℘g=˘℘⋄ ∀g. By Definition 3.10, we have ∑ug=1ζ(d)g. Then by using Theorem 3.11, we get
Corollary 3.15. If ˘£g=(˜ℵg,˘℘g) is the assemblage of largest q-ROFNs, i.e, . ˘£g=(1,0) for all g, then
Proof. We can easily obtain Corollary similar to the Theorem 3.14.
Corollary 3.16. If ˘£1=(˜ℵ1,˘℘1) is the smallest q-ROFN, i.e, . ˘£1=(0,1), then
Theorem 3.17. Assume that ˘£g=(˜ℵg,˘℘g) and βg=(ϕg,φg) are two assemblages of q-ROFNs, if r>0 and β=(˜ℵβ,˘℘β) is a q-ROFN, then
1. q−ROFPGd(˘£1⊕β,˘£2⊕β,…˘£u⊕β)=q−ROFPGd(˘£1,˘£2,…˘£u)⊕β
2. q−ROFPGd(r˘£1,r˘£2,…r˘£u)=rq−ROFPGd(˘£1,˘£2,…˘£u)
3. q−ROFPGd(˘£1⊕β1,˘£2⊕β2,…˘£u⊕βn)=q−ROFPGd(˘£1,˘£2,…˘£n)⊕q−ROFPGd(β1,β2,…βu)
4. q−ROFPGd(r˘£1⊕β,r˘£2⊕β,…⊕r˘£u⊕β)=rq−ROFPGd(˘£1,˘£2,…˘£u)⊕β
Proof. This is trivial by definition.
q-ROFPGd operator also satisfied following properties.
Property:1
Assume that ˘£g=(˜ℵg,˘℘g) is the assemblage of q-ROFNs, then we have
Property:2
Assume that ˘£g=(˜ℵg,˘℘g) is the assemblage of q-ROFNs and ˘Ξ(˘£g)≠0 for all g, then we have
Property:3
Assume that ˘£g=(˜ℵg,˘℘g) is the assemblage of q-ROFNs and ˘Ξ(˘£1)≠0 or 1, then we have
Property:4
Assume that ˘£g=(˜ℵg,˘℘g) is the assemblage of q-ROFNs and ˘Ξ(˘£g)≠0 for all g=1,2,...,k+1, and ˘Ξ(˘£k+1)≠1 then we have
Property:5
Assume that \breve{£}_{g} = (\widetilde{\aleph}_{g}, \breve{\wp}_{g}) is the assemblage of q-ROFNs and \breve{\Xi}(\breve{£}_{k+1}) \neq 1 or 0 then we have
4.
Methodology for MCDM using proposed AOs
Let \overline{\amalg} = \{\overline{\amalg}_1, \overline{\amalg}_2, \ldots, \overline{\amalg}_m \} be the assemblage of alternatives and \widehat{\amalg}' = \{\widehat{\amalg}'_1, \widehat{\amalg}'_2, \ldots, \widehat{\amalg}'_n \} is the assemblage of criterions, priorities are assigned between the criterions provided by strict priority orientation. \widehat{\amalg}'_1 \succ_{d_1} \widehat{\amalg}'_2 \succ_{d_2} \widehat{\amalg}'_3 \ldots \succ_{d_{n-1}} \widehat{\amalg}'_n , indicates criteria \widehat{\amalg}'_J has a high priority than \widehat{\amalg}'_{J+1} with degree d_q for q \in \{1, 2, \ldots, (n-1) \} . K = \{K_1, K_2, \ldots, K_p \} is a assemblage of decision-makers (DMs). Priorities are assigned between the DMs provided by strict priority orientation, K_1 \succ_{d_1'} K_2 \succ_{d_2'} K_3 \ldots \succ_{d_{p-1}'} K_p . DMs give a matrix according to their own standpoints D^{(p)} = (\mathscr{B}^{(p)}_{ij})_{m\times n } , where \mathscr{B}^{(p)}_{ij} is given for the alternatives \overline{\amalg}_i \in \overline{\amalg} with respect to the attribute \widehat{\amalg}'_j \in \widehat{\amalg}' by K_p DM. If all Performance criteria are the same kind, there is no need for normalization; however, since MCGDM has two different types of Evaluation criteria (benefit kind attributes \tau_b and cost kinds attributes \tau_c ), the matrix D_(p) has been transformed into a normalize matrix using the normalization formula Y^{(p)} = (\mathscr{P}^{(p)}_{ij})_{m\times n } ,
where (\mathscr{B}^{(p)}_{ij})^c show the compliment of \mathscr{B}^{(p)}_{ij} .
The suggested operators will be implemented to the MCGDM, which will require the preceding steps.
Step 1:
Obtain the decision matrix D^{(p)} = (\mathscr{B}^{(p)}_{ij})_{m\times n } in the format of q-ROFNs from DMs.
Step 2:
Two kinds of criterion are described in the decision matrix: (\tau_c) cost type indicators and (\tau_b) benefit type indicators. There is no need for normalisation if all indicators are of the same kind, but in MCGDM, there may be two types of criteria. The matrix was updated to the transforming response matrix in this case Y^{(p)} = (\mathscr{P}^{(p)}_{ij})_{m\times n } using the normalization formula Eq 4.1.
Step 3:
Using one of provided AOs to combine all of the independent q-ROF decision matrices Y^{(p)} = (\mathscr{P}^{(p)}_{ij})_{m\times n } into one combined evaluation matrix of the alternatives W^{(p)} = (\widetilde{\chi}_{ij})_{m\times n } .
\widetilde{\chi}_{ij} = {{\rm{q-ROFPA }}_d }(\mathscr{P}^{(1)}_{ij}, \mathscr{P}^{(2)}_{ij}, \ldots \mathscr{P}^{(p)}_{ij})
or
\widetilde{\chi}_{ij} = {{\rm{q-ROFPG}} _d }(\mathscr{P}^{(1)}_{ij}, \mathscr{P}^{(2)}_{ij}, \ldots \mathscr{P}^{(p)}_{ij})
Step 4:
Aggregate the q-ROF values \widetilde{\chi}_{ij} for each alternative \overline{\amalg}_{i} by the q-ROFPAd (or q-ROFPGd) operator.
\widetilde{\chi}_{ij} = {{\rm{q-ROFPA }}_d }(\mathscr{P}_{i1}, \mathscr{P}_{i2}, \ldots \mathscr{P}_{in})
or
\widetilde{\chi}_{ij} = {{\rm{q-ROFPG}} _d }(\mathscr{P}_{i1}, \mathscr{P}_{i2}, \ldots \mathscr{P}_{in})
Step 5:
Analyze the score for all cumulative alternative assessments.
Step 6:
The alternatives were classified by the score function and, eventually, the most suitable alternative was selected.
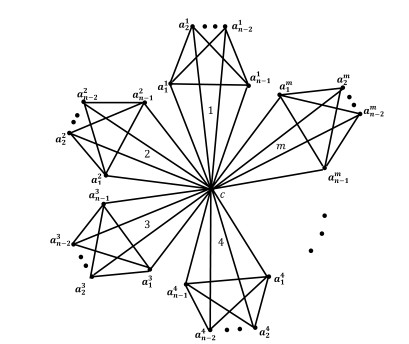
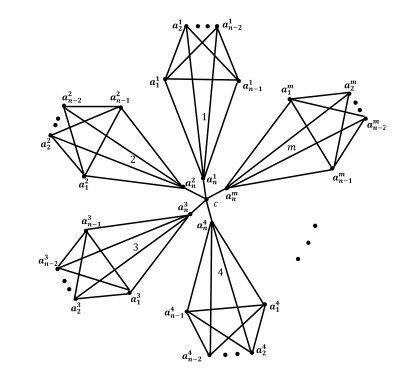
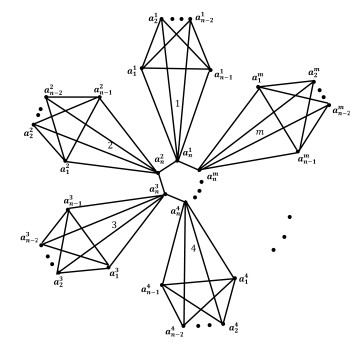
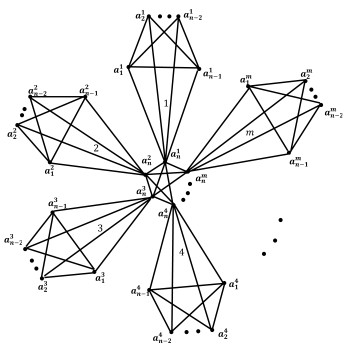
Pictorial view of Algorithm is given in Figure 1.
5.
Case study
Fossil fuel is the major source of global energy. Fossil fuels have very limited sources and it has been depleting with time. The dependence of energy generation on fossil fuel reached on its highest level. Similar exploitation of fossil fuel will diminish the resources in coming decades. This dependence caused environmental deterioration. Due to the excess use of fossil fuels, the concentration of CO_2 in the atmosphere has been increasing at unsafe level. This has polluted the atmosphere and air. Acid rain has been resulted due to the utilization of coal and nuclear energy plants. Air quality has been compromised. It is directly linked to health problems. Multiple diseases have been diagnosed and observed. Due to these severe causes, energy policy makers have been working to adopt novel techniques and technologies to reduce emissions and improve the quality of air. Therefore, it was and has still been dire need to exploit clean energy [35]. Pakistan has ranked poorly in pollution levels causing great amount of pollution such as haze, smoke, particulate matters and hazardous smog. Fossil fuels are the major sources of these pollution. Air Quality Index (AQI) results of Pakistan are very alarming in numbers [36]. Pakistan can improve AQI ranking by controlling and reducing the usage of fossil fuel in energy sector that is the main source of emissions. Pakistan electricity generation mix is consisted of 35,975 MWh till March 2020. Pakistan has only 2057 GWh share of Renewable Energy during 2019–2020 [37]. Pakistan has huge potential of renewable energy generation such as solar, wind, geothermal and tidal. Pakistan is needed to explore these kind of resources to meet the clean and green energy challenges. Renewable energy resources has become an alternative of fossil fuels curbing climate issues. Renewable energies have capability to meet the challenges of electricity supply and demand issues. RE produces clean and green electricity meeting the criterion of environmental challenges [38]. Pakistan holds the rich potential of renewable energy resources such as solar, wind and tidal etc. These resources can fulfil the energy requirement at national level. Pakistan is working to set renewable energy generation targets. Recently, renewable energy policy initiative has been taken at federal level. Energy policies can play vital role to install RE plants. Although, finance is major hurdle to start but Pakistan is strongly committed to achieve RE generation targets [39].
In this paper, we are targeting the energy generation, requirement and consumption scenario of Gwadar city located on the southwestern coastal area of an Arabian Sea province Balochistan. This area falls the rich amount of coastal winds and sunlight. Currently, electricity is generating from coal and wind at Gwadar at local level. Small capacity power plants have been installed. Coal power plants have also generating electricity at local level. In this study, we have proposed comprehensive analysis of RE energy generation such as solar, wind and tidal with coal energy generation. How the RE electricity can cover the electricity requirements of Gwadar area. How the RE meets the emissions targets. How it will create multiple jobs for local people. We have discussed the electricity generation from tidal source at coastal areas. Rich amount of natural resources can meet the energy crisis at national level. Coal power plant emits multiple gases into environment and deteriorate the environment. We have highlighted that solar, wind and tidal can replace coal power plants in near future. Gwadar region receives average sunlight of 3000 h per year and has capacity direct sunshine containing of 1800–2100 KW electricity production [40]. Therefore, the average sunlight hours are the highest across the Pakistan. This area can be the perfect electricity generation from solar technology. Pakistan government has issued several license to Independent Power Projects (IPPs) about wind electricity generation. Wind projects are working on Gwadar region. We have analyzed that wind can be more productive if it works with tidal energy production plants. The combination can produce better results. Tidal power plant can easily be installed at the shore side of Gwadar. It can generate the significant amount electricity that will cover the electricity needs of Balochistan rural areas. Electricity production from tidal is also clean reducing hazardous emission to the open air and environment. Coal is currently being generating electricity with the help of China. Coal projects are working with the partnership of China Pakistan Economic Corridor (CPEC) initiative. Although, coal power plants has adopted clean emission technology but emission has been produced during electricity generation. We have not been fulfilling the targets of AQI. Consequently, we have produced a comprehensive analysis among multiple electricity generating resources. We have observed from the data that Pakistan can generate clean electricity without consuming fossil fuel in any sector. National energy demand can be fulfilled with RE resources. Pollution can be controlled and we can reduce the emission level significantly. Health can be improved after the adopting and installation of RE resources. We still have a financial barrier that should be addressed with government policies. We still need strong policies that should support clean electricity production projects.
5.1. Tidal energy
In Pakistan, Gwadar has a huge potential of tidal energy generation at coastal areas. A study has estimated that tidal power plants can be installed in Gwadar coastal line [41]. It is needed to further investigate the potential of tidal electricity at coastal areas and deploy tidal energy plants to produce cheap and clean energy. Tidal energy is generated through high and low tides of water. Tidal turbines are installed in the barrage to harvest clean electricity from the water waves. It is clean and cheap energy [42]. The main advantage of tidal energy is combatting environmental pollution. It reduces CO_2 and GHG emissions to environment. Tidal electricity generation plant eliminates about 1000 g of CO_2 production from environment as compared to coal electricity plan. It also combats with other emissions effectively. It plays vital role in the reduction of methane ( CH_4 ), greenhouse gas emissions and nitrous oxide ( N_2O ). It does not emit particulate matters and other air emissions as well [43]. Tidal power generation is predictable and is easier to design and install. It will be efficient and long lasted as compared to solar and wind. Therefore, tidal energy is a contender of other renewable energy resources. Tidal energy generation plants are available commercially in China (5 MW), Canada (20 MW), Russia (0.4 MW) and France (240 MW). Recently, South Korea has installed tidal electricity plant of capacity (254 MW) [44]. The major of electricity in Pakistan is still from oil resources. Oil is less environmental friendly and emit multiple types of emissions to environmental. Pakistan's energy generation scenario can be seen in Figure 2. This oil based power industry has huge potential to shift towards renewable and clean energy. Pakistan can fulfil the demand of energy from renewable natural resources and it can even sale out to neighbor countries as well.
5.2. Wind energy
Renewable energy assessment resources show there are several wind corridors in various locations of southern, north western and central areas in Balochistan. The assessment of wind potential in Balochistan is shown in Table 2. Independent Power Projects (IPPs) are achieved license for wind electricity and have been working on 50 MW each wind energy generation projects shown in Table 3. Several feasibility reports have been submitted explaining the potential of wind energy in coastal areas [45]. There are various fundamental requirements which work for the deployment of wind electricity generation plants. The first one is wind speed to estimate potential and characteristics of other related parameters. The average wind speed indicates potential and efficiency of wind plant. The second one is easy access of transport and the third one is transmission lines from generation grid to the distribution grid. Balochistan has more wind energy potential than other provinces in Pakistan. This province has higher energy resources that are available in both renewable and non-renewable forms. The potential of total resources are yet be estimated and measured. Resources exploitations are still needed to be started in many areas of province. There are many areas that fulfil wind speed requirement and are needed to be used for small wind projects. This type of initiative can fulfil the electricity requirement of local community [46]. Although, several areas are still underdeveloped and are including strategically in the construction projects by the government of Pakistan. Construction of sea port will be the hub of traders and it could attract the new energy projects. It can be the hub of trade activities for Central Asia and China. Therefore, lack of infrastructure is the major issue to be solved. Transmission network is needed to be installed for renewable energy resources. Transportation network should be constructed for roadways which can create free accessibility towards remote areas. Pakistan has significant potential of wind energy. It has estimated installable potential of 346000 MW wind energy in Pakistan [47]. The total assessment of wind energy in various part of Pakistan is necessary to highlight the potential of investments avenues for both public and private sectors. The total assessment are estimated by the three major departments which are Pakistan Meteorological Department (PMD), National Renewable Energy Laboratory (NREL) and Alternative Energy Development Board (AEDB) [48]. The sources have reported that wind energy generation can fulfil the electricity demand at national. Wind energy exploitation is still underway due to lack of available resources and infrastructure in the country.
Pakistan has great potential of wind energy resources. It has reported that Pakistan has 346000 MW estimated potential of wind energy [49]. Coastal areas of Gwadar can be favorable areas for wind power projects. The deployment can cover the electricity needs of Gwadar region. Wind energy is environmentally clean and cost effective. It can be available in abundance with the installation of wind turbine at sites. Initial installation cost and transmission and distribution infrastructure are major hurdles and will require financial resources. This study highlights the advantages and disadvantages of installing and harvesting wind electricity at coastal areas of Gwadar. It provides an initial assessment about socially, environmentally and economically feasibility as compared to other renewable energy resources. This findings will help government to revise policy about conventional energy resources [50].
The environmental impacts of wind energy are positive as it reduces air pollution, water pollution and land pollution. Wind energy is relative competitive with other renewable resources in terms of social, political and cost. This renewable energy source is commercially profitable in most developed countries. Wind power plant impacts on ecosystem is less. Human and industrial activities are not effected mainly. It can have minor negative impacts on specific species on land. Efforts have been made to mitigate these negative impacts [51].
5.3. Solar energy
Pakistan is a rich country of sunlight. The capacity of sunlight reaches 3000 h per year. The estimated electrical potential from solar is around 1900–2200 MW. Solar power projects have been operational and generating electricity is shown in Table 4. Solar average global irradiation is 3 h per year which is predicted 1.9–2.3 MW h/ m^2 annually [52]. Gwadar is dry area with plenty of sunshine. The potential of solar energy is significantly high in Gwadar which is about 70%. This potential can cover the energy needs of rural areas. It is reported in [53] that average electricity usage at household in Gwadar is around 100 W. Solar electricity is long term cost benefit due to the availability of solar irradiation. Installation of solar units are socially, environmentally and economically acceptable to fulfill the electricity requirement in rural areas of Gwadar. Solar photovoltaic can cover the electricity needs reducing transmission and distribution cost in Gwadar. Social acceptance of solar electricity is favorable for middle class families in Gwadar as it covers electricity needs of low income households. Renewable energy policy 2006 dealt the potential and plan for renewable energies in the country. Policy highlights the installation of renewable energy projects in the country [54].
Solar energy is generated by the utilization of radiation of sun. It does not emit any particle, smoke and greenhouse gas (GHG) emission. Solar power is good role model to alleviating emission and promoting clean and green energy. Solar energy production technology can meet international climate change and global worming obligations. It reduces 20,000 tonnes per year emissions that have been emitted by fossil combustion fuel that has report in Table 5. The installation cost of solar power is less than other renewable and non-renewable energy resources. It does not demand expensive material like gas, coal or oil etc. Operation cost is also lower as compared to conventional power generation plants. Transportation cost is almost negligible than others that are transported to plants. Solar electricity panel's expectancy continues about 25–30 years and it can be increased to 40 years [55]. The availability of sun light is blessing in balochistan. It has potential to feed the electricity demand of Gwadar region. Solar power can be generated with both on or off grid options. On grid solar plants are connected to national grid and can compensate the additional demand. Decentralization is the major advantage of solar power. It works for local community and societies without the need of transmission and distribution infrastructures. It means it saves additional infrastructure cost. Although, decentralization has some limits and high initial installation cost but it is still viable to be utilized. This sector will create jobs for local's communities in installing, maintaining and monitoring of panels. This jobs opportunities can help to minimize unemployment in that region [56].
5.4. Coal energy
Coal, unlike other renewable energy resources, is easily source of energy. It cannot be affected as it is available without any delay like by darkness in solar, water scarcity in hydro. Currently, this source of energy has been widely used in world and Pakistan as well. It is cheapest and reliable in context of availability and utility. The performance of coal in Pakistan can be shown in Table 6. It is affordable energy and for that reason coal has no comparison. Therefore, it is most cost effective than other renewable energy sources. Electricity generation from coal is completely different from other renewable energy resources. The major issue attached to coal energy is smoke. Coal combustion is responsible of smoke and emission. The byproducts of coal can be used to manufacture various products. Heat extraction is easy than electricity generation of other energy sources [57].
This energy sector is labor dependent and it operates with the greater number of people. It creates direct jobs in huge number for the people of nearest community and society. Construction work creates jobs for drivers, cleaners, loaders. Technical jobs engineers are also created with capital incentives. It is imperative that Gwadar should be self-sufficient in electricity to fulfil the energy demand of local dwellers. Currently, 142.5 MW electricity is being supplied to Makran area in which 30 MW is being produced by domestic electricity generators along 8.5 MW in Gwadar Free Zone and major part of electricity is covered from electricity that is being imported from Iran. It is predicted that electricity needs of Gwadar region will raise to 778 MW in 2030 [58]. Coal energy projects are going to be installed at Gwadar that falls under CPEC. The capacity of the newly coal plant is 300 MW. It would cover the shortfall in Gwadar areas. Electricity generation from coal is still a threat to climate. There are several serious environmental impacts of coal power plants. Coal power plants emits pollutants. These pollutants contain sulphur dioxide, oxides of nigtrogen and oxides of carbon etc. Suspended particulate matters are also emitted from plants. Hot water is released from plants which is very dangerous for aquatic life and it also degrades the soils. Multiple health impacts are also observed. Coal is also produced radiation that are carcinogenic in nature. They are the main source of cancer along cell mutations. It has also reported that people living in the vicinity of coal power plants are suffered from asthma and lung cancer with multiple complications and disorder conditions. It is very disastrous for human health. Coal power plants extract heating liquids that are very hazardous for plants. Plants are being destroyed by the waste of coal plants. Similarly, it has compromised the groundwater mineral sources. It can disturb the harmony that nature provided to the species. It vanishes the natural habitants in ecosystem. Animals are being affected in the vicinity of power plants. It has changed the locality of the species with huge extraction as well [59]. It is the main source to bring in acid rain that can damage the whole infrastructure of habitant. Emissions are very harmful with undesirable consequences to health along whole environment. It has been reported [60] that coal is the major agent that has been responsible in the elevating of temperature level. Technological advancement has been introduced in coal technology to treat emissions. But it is still not environmental friendly. Coal power plants construction is the main source of vibration, noise, water and air pollution. Therefore, it is not socially accepted. It also disturbs the life of local people. It deforms the cultural heritage places with its pollutions. Politically acceptance is under threat due to introduction of recent renewable energy policy for Pakistan. Coal power plants in Gwadar areas are not suited for inhabited and humans as well. Coal extraction will have impact on environment, economic and society. Environmental and aquatic damage can be restored with installation of clean plants. Affected people can be relocated to in their areas and compensation can may also be adjusted in somehow [61]. It can be seen that coal has both potential positives and downsides negatives. Coal has been played many tremendous role in the development of modern structure. It cannot be ignored but with technological advancement it can meet the clean energy requirement. Therefore, it is needed to adopt the advanced steps for the mitigation of adverse impacts of coal to environment and humanity.
Coastal areas are rich potential wind and considered suitable for wind electricity generation and utilization. It is cost effective approach as compared to other renewable energy resources. High wind pressure is not sustainable for solar, coal and tidal. Several wind projects have been started their working and it will be become a hub of traders. CPEC projects show the political interest and willingness of Pakistan government as well as China government. Infrastructure work has been in progress. Coast areas wind energy production has less impacts on ecosystem. Local activities will not be harmed with the installation of wind turbines. It may have minor negative impacts on coastal areas. Wind turbines are environmental friendly and they generate clean energy reducing emissions. It does not emit CO_2 and hazardous gasses into the air, land, and water life. It complies environmental policy directives. It mitigates air pollution for the local community. It will improve health matters. Gwadar is located in the coastal areas. People are dwelling near the coastal lines. They are habitual to live in. Social barriers are not linked to wind electricity production. Although, it will fulfill their basic energy needs that can improve living standards of local community. Several wind projects will definitely increase the opportunities of job for local dwellers. Job opportunities will increase the living standards of local community. Trade options will be increased with locals.
5.5. Numerical illustration
Consider a decision making problem of finding out the most appropriate energy source. Assume the assemblage of alternatives, \overline{\amalg}_1 = wind energy, \overline{\amalg}_2 = solar energy, \overline{\amalg}_3 = tidal energy and \overline{\amalg}_4 = coal energy. There are five criterions for evaluation of these alternatives \widehat{\amalg}'_1 = impact on environment, \widehat{\amalg}'_2 = cost, \widehat{\amalg}'_3 = political acceptance, \widehat{\amalg}'_4 = job creation and \widehat{\amalg}'_5 = social acceptance. Assume that the criterions have been prioritized in strict priority order \widehat{\amalg}'_1 > _{d_1} \widehat{\amalg}'_2 > _{d_2} \widehat{\amalg}'_3 > _{d_3} \widehat{\amalg}'_4 > _{d_1} \widehat{\amalg}'_5 . The three dimensional vector of priority degrees is d = (2, 1, 3, 2). Here three DMs K _1 , K _2 and K _3 are involved, they have been prioritized in strict priority order K _1 > _{d_1'} K _2 > _{d_2'} K _3 , where d' = (3, 4).
Step 1:
Obtain the decision matrix D^{(p)} = (\mathscr{B}^{(p)}_{ij})_{m\times n } in the format of q-ROFNs from DMs. The judgement values, given by three DMs, are described in Table 7.
Step 2:
Normalize the decision matrixes acquired by DMs using Eq 4.1. In Table 7, there are two types of criterions. C_2 is cost type criteria and others are benefit type criterions. Normalized q-ROF decision matrix given in Table 8.
Step 3:
Using q-ROFPAd opeartor to combine all of the independent q-ROF decision matrices Y^{(p)} = (\mathscr{P}^{(p)}_{ij})_{m\times n } into one combined evaluation matrix of the alternatives W^{(p)} = (\widetilde{\chi}_{ij})_{m\times n } given in Table 9. First we find T_{ij}^{(1)} T_{ij}^{(2)} and T_{ij}^{(3)} , which are used in the calculation of q-ROFPAd operator.
Step 4:
Aggregate the q-ROF values \widetilde{\chi}_{ij} for each alternative \overline{\amalg}_{i} by the q-ROFPAd operator using Eq 4.4 given in Table 10.
Step 5:
Compute the score for all q-ROF aggregated values \widetilde{\chi}_{i} .
Step 6:
Ranks according to score values.
So,
\overline{\amalg}_1 = wind energy, is best alternative among all other alternatives. Wind energy output is determined by the average wind speed. It has been discovered that the chosen Gwadar wind area is suitable as compared to other renewable energy options that meet international standards. Gwadar's average wind speed corresponds to the best position and category. The Gwadar zone will meet the electricity demand with a high capacity factor. It has the potential to increase the share of renewable energy in the national energy mix. Local residents will be employed, lowering the unemployment rate. As a result, living standards can be raised by providing the best possible services and atmosphere.
5.6. Comparison analysis and advantages of the proposed AOs
We present a comparative review of recommended operators with some current AOs in this section. That both achieve the same final result is the excellence of our suggested AOs. By resolving the information data with some existing AOs, we compare our results and get the same optimal decision. This illustrates our proposed model's strength and consistency. The comparison can be made of the AOs presented with some current AOs is given in the Table 11. In our proposed AOs there is a parameter q involved, this increase in value of q provides an opportunity to select MDs and NMDs from a larger area. Initially, when q is increased from 1 to 2, there is a rapid increase in the area bounded between coordinate axes and the curve \widetilde{\aleph}^q + \breve{\wp}^q = 1 . As the value of q increases (say after q = 2) increase in area gets slower and slower. We obtain \overline{\amalg}_1 \succ \overline{\amalg}_2 \succ \overline{\amalg}_3\succ \overline{\amalg}_4 rating by our proposed aggregation operators; to validate our optimal option, we run this problem through other existing operators. The validity of our suggested aggregation operators is demonstrated by the fact that we obtain the same optimal decision.
6.
Conclusions
The current work employs q-ROFSs to handle ambiguity in the data using MDs and NMDs. The q-ROF framework is an extension of the IFS and PFS models. There is a parameter q involved in q-ROFS, increasing the value of q allows you to pick MDs and NMDs from a wider region. We introduced the notion of q-rung orthopair fuzzy prioritized averaging (q-ROFPAd) operator with priority degrees and q-rung orthopair fuzzy prioritized geometric (q-ROFPGd) operator with priority degrees by considering the strict priority orders. Many propositions concerning priority degree have been thoroughly investigated, and they will be useful in combining numerous q-ROF data. Under the q-ROF framework, a group MCDM approach based on the proposed prioritized AOs has been formed. An analogy is used to illustrate the proposed technique, and the methodology results are compared to several current AOs. Aside from that, the effect of priority degrees on aggregated outcomes is thoroughly explained. Furthermore, the impact of priority degrees on outcomes makes the proposed solution more robust since the DM can choose the priority degree vector based on his or her priorities and the complexity of the problem. We apply proposed group MCDM approach on a case study of Gwadar for sustainable energy planning, it has been investigated and evaluated that the Gwadar zone has a greater capacity for wind energy production than other RE sources. Electricity production costs will be kept to a bare minimum per device. Wind energy, like solar and tidal, emits no carbon, whereas coal does. The environmental advantage would boost the health of the local community. In Gwadar, it has the potential to replace traditional coal energy with renewable energy. Local grids have the potential to eliminate transmission losses as well as technical maintenance costs. As a result, transmission and distribution losses will be minimized, while energy quality and performance should improve.
In the future, some functional applications of the proposed work in fuzzy inferences could be addressed. We would also apply the suggested AOs and MCDM methodology to decision-making, medical diagnosis, pattern recognition, computational intelligence, and artificial intelligence. Aside from that, we will focus on developing some approaches for objectively acquiring priority degree in the future.
Acknowledgements
The authors extend their appreciation to the Deanship of Scientific Research at King Khalid University, Abha 61413, Saudi Arabia for funding this work through research groups program under grant number R.G. P-1/23/42.
Conflict of interest
The authors declare no conflict of interest.









 DownLoad:
DownLoad: