Sustainable thermal power equipment supplier selection by Einstein prioritized linear Diophantine fuzzy aggregation operators

1.   Introduction

The problem of ambiguous and deceptive information has been a serious concern for decades. One of the most exciting aspects of our daily lives is making decisions. Even though most reviewers use a variety of measurements to arrive at a result, some of them may be ambiguous. On the other hand, as the structure effects grow by the day, it becomes increasingly difficult for the decision maker (DM) to make a reasonable judgement in a fair amount of time using ambiguous, erroneous, and imprecise facts. MCDM is a classic cognitive activity tool whose main objective is to select from a limited set of options using preference knowledge provided by DMs. Unfortunately, because it combines the intricacies of human reasoning skills, the MCDM technique is ambiguous and imprecise, making it difficult for DMs in the assessment process to provide proper evaluation. It is vital to overcome this dilemma since, in addition to coping with uncertainty, Zadeh ^[1] invented the "fuzzy set" (FS) theory. Atanassov ^[2] developed the idea of "intuitionistic fuzzy set" (IFS). Yager ^[3,4] introduced "Pythagorean fuzzy set" (PFS). Yager established the q-rung orthopair fuzzy set (q-ROFS) after generalizing the IFS and PFS. The constraint of q-ROFS is that the sum of qth membership degree (MD) and the qth non membership degree (NMD) powers will be less than or equal to one. Clearly, the higher the rung q, the more orthopairs fulfil the constraining requirement, and hence the larger the universe of fuzzy data that may be defined by q-ROFSs. ^[5]. q-ROFs outperform IFS and PFS in terms of their capacity to deal with both vagueness and disregarded data. The q-ROFS knowledge, similarity, dissimilarity, and divergence measures were discussed in ^[6,7,8,9]. The concept of LDFS was introduced by Riaz and Hashmi ^[10]. In many real-life uncertain circumstances, the MD and NMD are not enough to analyze the objects/alternatives, so we need to add further assessment to the MD and NMDs. In fact reference/control parameters in "linear Diophantine fuzzy numbers" (LDFNs) associate additional ranking/grading/assessment to the DM's opinion. In this way the decision process will become more efficient and reliable.

Data aggregation is fundamental for decision-making in the sectors of business, organizational, economic, medicinal, technical, psychiatric, and autonomous systems. Generally, consciousness of the alternate has been viewed as a crisp number or linguistic number. The data, meanwhile, cannot be effectively consolidated owing to its ambiguity. In reality, aggregation operators (AOs) play an important role in the context of MCDM concerns, the main purpose of which is to combine a collection of inputs to a single figure. Peng et al. ^[11] proposed upgraded "single valued neutrosophic number" (SVNN) operations and established their associated AOs. Nancy and Garg ^[12] established AOs by employing Frank operations. Liu et al. ^[13] created some AOs for SVNNs based on "Hamacher operations". Zhang et al. ^[14] provided the AOs in the context of an "interval-valued neutrosophic set". Wu et al. ^[15] developed the prioritized AOs with SVNNs. Xu et al. ^[16,17] established geometric and averaging AOs for IFS. Wei et al. ^[19], Mahmood et al. ^[18], Akram et al. ^[20], Feng et al. ^[21], Wang and Liu ^[22] and Garg ^[23] developed several AOs. Wang et al. ^[24] introduced "Pythagorean fuzzy interactive Hamacher power" AOs. Wang and Li ^[25] developed "Pythagorean fuzzy interaction power Bonferroni mean" AOs. Riaz et al. ^[26] developed "linear Diophantine fuzzy prioritized AOs" and Iampan et al. ^[27] proposed Einstein AOs for LDFSs. Riaz et al. introduced the concepts of q-ROFS Einstein ^[28], prioritized ^[29], "Einstein prioritized" ^[30], Einstein interactive geometric ^[31], and AOs related to q-ROF soft set ^[32]. Liu and Liu ^[33] introduced "q-ROF bonferroni mean" AOs. Riaz et al. ^[34] developed the concept of "bipolar picture fuzzy set". Liu et al. ^[35] initiated the idea of "q-ROF Heronianmean AOs". Mesa et al. ^[36] presented the main contributions in the field of AOs by a bibliometric review approach. Mesa et al. ^[37] proposed bibliometric-based review for fuzzy decision-making.

Yager proposed a number of priority AOs. As per Yager, in such circumstances, if we choose a kid's bicycle depending on safety and economic factors, we should not allow the price advantage to impair the performance of protection. The two criteria then have a form of priority. The AOs in question, such as the average and geometric AOs, are significant because they allow us to evaluate higher priority criteria, such as safety in the case of the former. In this case, Yager ^[38] provided prioritized AOs by modeling attribute prioritization in terms of criterion weights based on fulfillment of the higher importance attributes. After Yager's ^[38] prioritized AOs many researchers developed hybrid operators, for example Gao ^[39] developed Hamacher prioritized averaging and geometric AOs for PFSs. Castro et al. ^[40] proposed novel "prioritized induced ordered weighted geometric average AOs". Arellano et al. ^[41] introduced "prioritized induced probabilistic ordered weighted average (PIPOWA) operator", "prioritized probabilistic weighted average (PPOWA) operator" and "prioritized induced ordered weighted average (PIOWA) operator". Arellano et al. ^[42] also offered new AOs to enhance the transparency index's evaluation. The "prioritised induced ordered weighted average weighted average (PIOWAWA) operator" is a new AOs. Ye ^[43] developed new hybrid AOs for interval-valued hesitant fuzzy set namely "interval-valued hesitant fuzzy prioritized weighted averaging (IVHFPWA) operator and an interval-valued hesitant fuzzy prioritized weighted geometric (IVHFPWG) operator".

To answer the question, why did we perform all of this research? We see that existing AOs do not provide a smooth approximation. There are several types of groups of t-norms and t-norms that can be used to construct intersections and unions. Einstein sums and products are a useful alternative to algebraic sums and products because they provide a pretty smooth approximation. We can apply the recommended AOs if we have a priority relationship in the criteria and a smooth approximation. Consistent with past study, we can conclude that decision-making issues in the modern environment are becoming increasingly complex. It is important to convey the unknown details in a more competitive fashion in order to choose the optimal alternative(s) for MCDM issues. Furthermore, it is essential to understand how to manage the prioritized relationship between various criteria. Having several of these characteristics in mind, and going to take benefit of the LDFS, we merge prioritized AOs and Einstein AOs and suggest prioritized Einstein AOs that takes advantage of both. As a result of these considerations, contributions of the manuscript given as follows:

1. Existing models such as IFSs, PFSs, and $q$-ROFSs all have severe restrictions on MDs and NMDs. LDFS is a novel, adaptable solution for overcoming these constraints. The DMs are free to choose any of these grades in $[0, 1]$. Furthermore, the reference or control parameters are employed as a weight vector such that their sum is less than unity. These characteristics classify the physical properties of items and aid in the management of unclear information about the objects in consideration.

2. Einstein aggregation operators are used to provide seamless information fusion, while prioritized operators are utilized to establish links between various criteria in a prioritized manner. To maximize the benefits of these operators, we design new hybrid AOs.

3. We proposed two hybrid AOs, to address the impact of DM's extremely high or excessively low values on the overall rankings, namely, "linear Diophantine fuzzy Einstein prioritized weighted average (LDFEPWA) operator" and the "linear Diophantine fuzzy Einstein prioritized weighted geometric (LDFEPWG) operator."

4. Some of the enticing characteristics of proposed AOs also discussed, such as boundary, idempotent and monotonicity.

5. To solve MCDM problems, a novel decision-making approach based on suggested operators is provided.

6. The proposed decision-making approach is illustrated through a practical application with proposed hybrid operators addressing issues related to green thermal power equipment providers.

The rest of the paper is arranged as follows. Section 2 provides basic principles relating to LDFSs and various AOs. Section 3 consists on a range of LDF "Einstein prioritized AOs". Section 4 gives an MCDM framework to the suggested AOs and Section 5 includes numerical examples and a comparison to current AOs. Section 6 outlines the important findings of the study.

2.   Preliminaries

Some fundamental concepts related to LDFSs have been presented in this section, over the universal set $\Theta$. For basic definitions related to fuzzy sets, one can see ^[1,2,3,5,10].

Definition 2.1. ^[10] A LDFS $\breve{\Psi}$ in $\Theta$ is defined as

where ${\mu}_{\breve{\Psi}}(\breve{x}), {\nu}_{\breve{\Psi}}(\breve{x}), {\beta}_{\breve{\Psi}}(\breve{x}), {\aleph}_{\breve{\Psi}}(\breve{x})\in[0, 1]$ are the MD, the NMD and the corresponding reference parameters (RPs), respectively. Moreover,

and

for all $\breve{x}\in\Theta$. The LDFS

is known the "absolute LDFS" in $\Theta$. The LDFS

is known the "null LDFS" in $\Theta$.

Specific structures can be modelled or classified using the RPs. We can categorize distinct systems by changing the logical interpretation of the RPs. In addition, $\eta_{\breve{\Psi}}(\breve{x})\pi_{\breve{\Psi}}(\breve{x}) = 1-({\beta}_{\breve{\Psi}}(\breve{x}){\mu}_{\breve{\Psi}}(\breve{x})+ {\aleph}_{\breve{\Psi}}(\breve{x}){\nu}_{\breve{\Psi}}(\breve{x}))$ is known as the "indeterminacy degree" and its corresponding RP of $\breve{x}$ to $\Psi$.

Definition 2.2. ^[10] A "linear Diophantine fuzzy number" (LDFN) is a tuple ${\breve{\rho}} = (\langle{\mu}_{{\breve{\rho}}}, {\nu}_{{\breve{\rho}}}\rangle, \langle{\beta}_{{\breve{\rho}}}, {\aleph}_{{\breve{\rho}}}\rangle)$ satisfying the following conditions:

$\text{(1)} \; 0\leq{\mu}_{{\breve{\rho}}}, {\nu}_{{\breve{\rho}}}, {\beta}_{{\breve{\rho}}}, {\aleph}_{{\breve{\rho}}}\leq 1$;

$\text{(2)} \; 0\leq{\beta}_{{\breve{\rho}}}+{\aleph}_{{\breve{\rho}}}\leq 1$;

$\text{(3)} \; 0\leq{\beta}_{{\breve{\rho}}}{\mu}_{{\breve{\rho}}}+{\aleph}_{{\breve{\rho}}}{\nu}_{{\breve{\rho}}}\leq 1$.

LDFS is a novel method to uncertainties and ambiguity that outperforms conventional methods such as IFSs, PFSs, and q-ROFSs. The distinguishing feature of LDFS is that there is a pair of RPs for each content and dissatisfaction degree, thus the valuation field of theoretical aspects they can represent is superior. It may be difficult for DMs to identify optimal or convincing alternatives due to the restrictions of certain existing techniques and their equivalent operators.

The set of RPs plays an important role in decision-making frameworks. They enable us to increase the assessment area of satisfaction and dissatisfaction functions and parameterize the model, which provides us with a range of taking options in various physical circumstances. The lack of parameterizations in IFS, PFS, and q-ROFS is a shortcoming. This innovative concept improves on previous approaches. Based on the conversation, it is evident that our presented solution is more appropriate and preferable to others, and it includes a variety of RPs. This method can be used in a variety of industrial, medicinal, cognitive computing, and MADM domains. In Table 1, we can see the comparison between proposed approach with the existing concepts.

Now we will presented some operational rules to aggregate the LDFNs.

Definition 2.3. ^[10] Let ${\breve{\rho}}_{1} = \left(\langle {\mu}_{1}, {\nu}_{1} \rangle, \langle {\beta}_{1}, {\aleph}_{1} \rangle \right)$ and ${\breve{\rho}}_{2} = \left(\langle {\mu}_{2}, {\nu}_{2} \rangle, \langle {\beta}_{2}, {\aleph}_{2} \rangle \right)$ be to LDFNs, then

Definition 2.4. ^[10] Let ${\breve{\rho}} = \left(\langle{\mu}_{{\breve{\rho}}}, {\nu}_{{\breve{\rho}}}\rangle, \langle{\mu}_{{\breve{\rho}}}, {\aleph}_{{\breve{\rho}}} \rangle \right)$ be the LDFN, then the expectation score function can be defined as follows.

if we have two LDFNs say ${\breve{\rho}}$ and ${\beta}$ if $\widehat{\daleth}({\breve{\rho}}) > \widehat{\daleth}({\beta})$, then ${\breve{\rho}} > {\beta}$.

From here $\sum_{h = 1}^{e} = \beth_{h}$ in whole manuscript for the sake of convenience.

Definition 2.5. ^[26] Let ${\breve{\rho}}_{h} = \left(\langle{\mu}_{h}, {\nu}_{h}\rangle, \langle {\beta}_{h}, {\aleph}_{h} \rangle \right)$ be the assemblage of LDFNs, and LDFPWA: $\Theta^n \rightarrow \Theta$, be a n dimension mapping. if

then the mapping LDFPWA is called "linear Diophantine fuzzy prioritized weighted averaging (LDFPWA) operator", where $\breve{\Gamma}_{h} = \prod_{h = 1}^{g-1} \widehat{\daleth}({\breve{\rho}}_h) \; (g = 2 \ldots, e)$, $\breve{\Gamma}_{1} = 1$ and $\widehat{\daleth}({\breve{\rho}}_{h})$ is the score of $h^{th}$ LDFN.

Theorem 2.6. ^[26] Let ${\breve{\rho}}_{h} = \left(\langle{\mu}_{h}, {\nu}_{h}\rangle, \langle {\beta}_{h}, {\aleph}_{h} \rangle \right)$ be the assemblage of LDFNs, we can also find LDFPWA by

Definition 2.7. ^[26] Let ${\breve{\rho}}_{h} = \left(\langle{\mu}_{h}, {\nu}_{h}\rangle, \langle {\beta}_{h}, {\aleph}_{h} \rangle \right)$ be the assemblage of LDFNs, and LDFPWG: $\Theta^n \rightarrow \Theta$, be a n dimension mapping. if

then the mapping LDFPWG is called "linear Diophantine fuzzy prioritized weighted geometric (LDFPWG) operator", where $\breve{\Gamma}_{h} = \prod_{h = 1}^{g-1} \widehat{\daleth}({\breve{\rho}}_h) \; (g = 2 \ldots, e)$, $\breve{\Gamma}_{1} = 1$ and $\widehat{\daleth}({\breve{\rho}}_{h})$ is the score of $h^{th}$ LDFN.

Theorem 2.8.^[26] Let ${\breve{\rho}}_{h} = \left(\langle{\mu}_{h}, {\nu}_{h}\rangle, \langle {\beta}_{h}, {\aleph}_{h} \rangle \right)$ be the assemblage of LDFNs, we can also find LDFPWG by

For LDFNs, Iampan et al. ^[27] presented the Einstein operation and investigated its attractive properties.

Definition 2.9. ^[27] If ${\breve{\rho}}_{1} = \left(\langle {\mu}_{1}, {\nu}_{1} \rangle, \langle {\beta}_{1}, {\aleph}_{1} \rangle \right)$ and ${\breve{\rho}}_{2} = \left(\langle {\mu}_{2}, {\nu}_{2} \rangle, \langle {\beta}_{2}, {\aleph}_{2} \rangle \right)$ be to LDFNs with $\mathfrak{T} > 0$ be a real number, then

Theorem 2.10. ^[27] Let ${\breve{\rho}}_{i} = (\langle{\mu}_{i}, {\nu}_{i}\rangle, \langle{\beta}_{i}, {\aleph}_{i}\rangle)$ be two LDFNs with $i = 1, 2$ and ${\vartheta} > 0$ be any real number, then

$(i)$ ${\breve{\rho}}_{1} \otimes_{\epsilon} {\breve{\rho}}_{2} = {\breve{\rho}}_{2} \otimes_{\epsilon} {\breve{\rho}}_{1}$

$(ii)$ ${\breve{\rho}}_{1} \oplus_{\epsilon} {\breve{\rho}}_{2} = {\breve{\rho}}_{2} \oplus_{\epsilon} {\breve{\rho}}_{1}$

$(iii)$ $({\breve{\rho}}_{1} \otimes_{\epsilon} {\breve{\rho}}_{2})^{{\vartheta}} = {\breve{\rho}}_{1}^{{\vartheta}} \otimes_{\epsilon} {\breve{\rho}}_{2}^{{\vartheta}}$

$(iv)$ ${\vartheta}._{\epsilon } ({\breve{\rho}}_{1} \oplus_{\epsilon} {\breve{\rho}}_{2}) = {\vartheta}._{\epsilon} {\breve{\rho}}_{1} \oplus_{\epsilon} {\vartheta}._{\epsilon}{\breve{\rho}}_{2}$

$(v)$ ${\breve{\rho}}_{1}^{{\vartheta}_1} \otimes_{\epsilon} {\breve{\rho}}_{1}^{{\vartheta}_2} = {\breve{\rho}}_{1}^{{\vartheta}_1 + {\vartheta}_2}$

$(vi) \; {\vartheta}_1._{\epsilon } ({\vartheta}_2._{\epsilon } {\breve{\rho}}_1) = ({\vartheta}_1._{\epsilon } {\vartheta}_2)._{\epsilon } {\breve{\rho}}_1$

$(vii)$ $({\breve{\rho}}_{1}^{{\vartheta}_1})^{{\vartheta}_2} = ({\breve{\rho}}_{1})^{{\vartheta}_1._{\epsilon } {\vartheta}_2}$

$(viii)$ ${\vartheta}_1._{\epsilon } {\breve{\rho}}_{1} \oplus_{\epsilon} {\vartheta}_2 = ({\vartheta}_1 + {\vartheta}_2)._{\epsilon } {\breve{\rho}}_{1}$.

Proof. Here, we omit the proof.

Definition 2.11. ^[27] Let $\Gamma_{\Lambda} = (\langle \mu_{\Lambda}, \nu_\Lambda\rangle, \langle{\beta_\Lambda}, {\aleph_\Lambda}\rangle)$ be a collection of LDFNs and $\Phi = (\Phi_1, \Phi_2, ..., \Phi_{n})^T$ be the weight vector (WV) with $\sum\limits^{n}_{\Lambda = 1}\Phi_{\Lambda} = 1$. Then $\mho:\Theta^n\rightarrow \Theta$ is called "linear Diophantine fuzzy Einstein weighted average (LDFEWA) operator" and defined as

$LDFEWA(\Gamma_{1}, \Gamma_{2}, \Gamma_{3}, ..., \Gamma_{n}) =$

$\sum\limits^{n}_{\Lambda = 1}{\Phi_\Lambda}\Gamma_{\Lambda} = {\Phi_1}._{\epsilon}\Gamma_{1}\oplus_{\epsilon} {\Phi_2}._{\epsilon} \Gamma_{2}\oplus_{\epsilon} \Phi_3._{\epsilon} \Gamma _{3}\oplus_{\epsilon}... \oplus_{\epsilon} \Phi_{n}._{\epsilon}\Gamma_{{n}}$.

In LDFEWA operator, we use $\Phi$ as a WV and $\Gamma_{\Lambda}$ are the LDFNs, where $\Lambda = 1, 2, ..., n$. $\Theta$ is the collection of all LDFNs.

Theorem 2.12. ^[27] Let $\Gamma_{\Lambda} = (\langle {\mu}_{\Lambda}, \nu_\Lambda\rangle, \langle{\beta_\Lambda}, {\aleph_\Lambda}\rangle)$ be a collection of LDFNs and $\Phi = (\Phi_1, \Phi_2, ..., \Phi_{n})^T$ be the WV with $\sum\limits^{n}_{\Lambda = 1}\Phi_{\Lambda} = 1$. Then the mapping $\mho:\Theta^n \rightarrow \Theta$ is called LDFEWA operator and can be written as

Definition 2.13. ^[27] Let $\Gamma_{\Lambda} = (\langle {\mu}_{\Lambda}, \nu_\Lambda\rangle, \langle{\beta_\Lambda}, {\aleph_\Lambda}\rangle)$ be a collection of LDFNs and $\Phi = (\Phi_1, \Phi_2, ..., \Phi_{n})^T$ be the WV with $\sum\limits^{n}_{\Lambda = 1}\Phi_{\Lambda} = 1$. Then the mapping $\mho:\Theta^n\rightarrow \Theta$ is called "linear Diophantine fuzzy Einstein weighted geometric (LDFEWG) operator" and defined as

$LDFEWG(\Gamma_{1}, \Gamma_{2}, \Gamma_{3}, ..., \Gamma_{n}) =$

$\prod\limits^{n}_{\Lambda = 1}{\Phi_\Lambda}\Gamma_{\Lambda} = {\Phi_1}._{\epsilon}\Gamma_{1}\otimes_{\epsilon} {\Phi_2}._{\epsilon} \Gamma_{2}\otimes_{\epsilon} \Phi_3._{\epsilon} \Gamma_{3}\otimes_{\epsilon}... \otimes_{\epsilon} \Phi_{n}._{\epsilon}\Gamma_{{n}}$.

In LDFEWG operator, we use $\Phi$ as a WV and $\Gamma_{\Lambda}$ are the LDFNs, where $\Lambda = 1, 2, ..., n$. $\Theta$ is the collection of all LDFNs.

Theorem 2.14. ^[27] Let $\Gamma_{\Lambda} = (\langle {\mu}_{\Lambda}, \nu_\Lambda\rangle, \langle{\beta_\Lambda}, {\aleph_\Lambda}\rangle)$ be the assemblage of LDFNs and $\Phi = (\Phi_1, \Phi_2, ..., \Phi_{n})^T$ be the WV with $\sum\limits^{n}_{\Lambda = 1}\Phi_{\Lambda} = 1$. Then $\mho:\Theta^n \rightarrow \Theta$ is called LDFEWG operator and can be written as

3.   Linear Diophantine fuzzy Einstein prioritized aggregation operators

Within this section, we present the notion of "linear Diophantine fuzzy Einstein prioritized weighted average (LDFEPWA) operator" and "linear Diophantine fuzzy Einstein prioritized weighted geometric (LDFEPWG) operator". Then we go over some of the other appealing characteristics of the prospective operators.

3.1.   LDFEPWA operator

Definition 3.1. Let ${\breve{\rho}}_{h} = \left(\langle{\mu}_{h}, {\nu}_{h}\rangle, \langle {\beta}_{h}, {\aleph}_{h} \rangle \right)$ be the assemblage of LDFNs, and LDFEPWA: $\Theta^n \rightarrow \Theta$, be a n dimension mapping. if

then the mapping LDFEPWA is called "linear Diophantine fuzzy Einstein prioritized weighted averaging (LDFEPWA) operator", where $\breve{\Gamma}_{h} = \prod_{h = 1}^{g-1} \widehat{\daleth}({\breve{\rho}}_h) \; (g = 2 \ldots, e)$, $\breve{\Gamma}_{1} = 1$ and $\widehat{\daleth}({\breve{\rho}}_{h})$ is the score of $h^{th}$ LDFN.

We may also simply consider LDFEPWA using Einstein operational laws, as shown in the theorem further below.

Theorem 3.2. Let ${\breve{\rho}}_{h} = \left(\langle{\mu}_{h}, {\nu}_{h}\rangle, \langle {\beta}_{h}, {\aleph}_{h} \rangle \right)$ be the assemblage of LDFNs, we can also find LDFEPWA by

$\text{LDFEPWA} ({\breve{\rho}}_1, {\breve{\rho}}_2, \ldots, {\breve{\rho}}_e) =$

where $\breve{\Gamma}_{h} = \prod_{h = 1}^{g-1} \widehat{\daleth}({\breve{\rho}}_h) \; (g = 2 \ldots, e)$, $\breve{\Gamma}_{1} = 1$ and $\widehat{\daleth}({\breve{\rho}}_{h})$ is the score of $h^{th}$ LDFN.

Proof. We will start this prove using mathematical induction.

For $h = 2$

As we know that both $\frac{\breve{\Gamma}_{1}}{\beth_{h} \breve{\Gamma}_{h} }._{\epsilon} {\breve{\rho}}_1$ and $\frac{\breve{\Gamma}_{2}}{\beth_{h} \breve{\Gamma}_{h} }._{\epsilon} {\breve{\rho}}_2$ are LDFNs, and also $\frac{\breve{\Gamma}_{1}}{\beth_{h} \breve{\Gamma}_{h} }._{\epsilon} {\breve{\rho}}_1 \oplus_{\epsilon} \frac{\breve{\Gamma}_{2}}{\beth_{h} \breve{\Gamma}_{h} }._{\epsilon} {\breve{\rho}}_2$ is LDFN.

Then

$\text{ LDFEPWA} ({\breve{\rho}}_1, {\breve{\rho}}_2) = \frac{\breve{\Gamma}_{1}}{\beth_{h} \breve{\Gamma}_{h} }._{\epsilon} {\breve{\rho}}_1 \oplus_{\epsilon} \frac{\breve{\Gamma}_{2}}{\beth_{h} \breve{\Gamma}_{h} }._{\epsilon} {\breve{\rho}}_2$

This holds true for $h = 2$.

Assuming the end result is valid for $h = b$, we have

$LDFEPWA ({\breve{\rho}}_1, {\breve{\rho}}_2, \ldots, {\breve{\rho}}_b)$

Now we will prove for $h = b+1$,

For $h = b+1$, the result is the same. This demonstrates that the desired outcome has been obtained.

Example 3.3. Let ${\breve{\rho}}_1 = (\langle0.60, 0.55\rangle, \langle0.15, 0.15\rangle)$, ${\breve{\rho}}_2 = (\langle0.65, 0.70\rangle, \langle 0.30, 0.65\rangle)$ and ${\breve{\rho}}_3 = (\langle0.45, 0.70\rangle, \langle 0.25, 0.45\rangle)$ be the LDFNs, prioritization between given LDFNs is given as ${\breve{\rho}}_1 \succ {\breve{\rho}}_2 \succ {\breve{\rho}}_3$ then we have,

and

Some of the LDFEPWA operator's enticing characteristics are described below.

Theorem 3.4. (Boundary) Assume that ${\breve{\rho}}_{h} = \left(\langle{\mu}_{h}, {\nu}_{h}\rangle, \langle {\beta}_{h}, {\aleph}_{h} \rangle \right)$ be the family of LDFNs, where $\breve{\Gamma}_{h} = \prod_{h = 1}^{g-1} \widehat{\daleth}({\breve{\rho}}_h) \; (g = 2 \ldots, e)$, $\breve{\Gamma}_{1} = 1$ and $\widehat{\daleth}({\breve{\rho}}_{h})$ is the score of $h^{th}$ LDFN, then

where,

Proof. Let $f(y) = \frac{2- y}{y}$, $y \in (0, 1]$ and $q\geq1$. Then $f'(y) < 0$. So, $f(y)$ is decreasing function on $(0, 1]$. Since ${\mu}_{{\breve{\rho}}_{min}} \leq{\mu}_{{\breve{\rho}}_{h}} \leq{\mu}_{{\breve{\rho}}_{max}}$, Then $f({\mu}_{{\breve{\rho}}_{max}}) \leq f({\mu}_{{\breve{\rho}}_{h}}) \leq f({\mu}_{{\breve{\rho}}_{min}})$, i.e, .

Let

be the prioritized WVs of ${\breve{\rho}}_{h} = \left(\langle{\mu}_{h}, {\nu}_{h}\rangle, \langle {\beta}_{h}, {\aleph}_{h} \rangle \right)$, s.t

Now,

Let $M(t) = \frac{1- t}{1+ t}$, $t \in [0, 1]$. Then $M'(t) < 0$. So, $M(t)$ is decreasing function on $(0, 1]$. Since ${\nu}_{{\breve{\rho}}_{max}} \leq{\nu}_{{\breve{\rho}}_{h}} \leq{\nu}_{{\breve{\rho}}_{min}}$, Then $M({\nu}_{{\breve{\rho}}_{min}}) \leq M({\nu}_{{\breve{\rho}}_{h}}) \leq M({\nu}_{{\breve{\rho}}_{max}})$, i.e, .

Let

be the prioritized WV of ${\breve{\rho}}_{h} = \left(\langle{\mu}_{h}, {\nu}_{h}\rangle, \langle {\beta}_{h}, {\aleph}_{h} \rangle \right)$, s.t

Now,

Let $f(y) = \frac{2- y}{y}$, $y \in (0, 1]$ and $q\geq1$. Then $f'(y) < 0$. So, $f(y)$ is decreasing function on $(0, 1]$. Since ${\beta}_{{\breve{\rho}}_{min}} \leq{\beta}_{{\breve{\rho}}_{h}} \leq{\beta}_{{\breve{\rho}}_{max}}$, Then $f({\beta}_{{\breve{\rho}}_{max}}) \leq f({\beta}_{{\breve{\rho}}_{h}}) \leq f({\beta}_{{\breve{\rho}}_{min}})$, i.e, .

Let

be the prioritized WVs of ${\breve{\rho}}_{h} = \left(\langle{\mu}_{h}, {\nu}_{h}\rangle, \langle {\beta}_{h}, {\aleph}_{h} \rangle \right)$, s.t

Now,

Let $M(t) = \frac{1- t}{1+ t}$, $t \in [0, 1]$. Then $M'(t) < 0$. So, $M(t)$ is decreasing function on $(0, 1]$. Since ${\aleph}_{{\breve{\rho}}_{max}} \leq{\aleph}_{{\breve{\rho}}_{h}} \leq{\aleph}_{{\breve{\rho}}_{min}}$, Then $M({\aleph}_{{\breve{\rho}}_{min}}) \leq M({\aleph}_{{\breve{\rho}}_{h}}) \leq M({\aleph}_{{\breve{\rho}}_{max}})$, i.e, .

Let

be the prioritized WV of ${\breve{\rho}}_{h} = \left(\langle{\mu}_{h}, {\nu}_{h}\rangle, \langle {\beta}_{h}, {\aleph}_{h} \rangle \right)$, s.t

Now,

Assume,

By Eqs 3.4, 3.6, 3.5 and 3.7 we can write ${\nu}_{{\breve{\rho}}_{max}}\: \leq \:{\nu}_{{\breve{\rho}}} \:\leq \: {\nu}_{{\breve{\rho}}_{min}}$, ${\aleph}_{{\breve{\rho}}_{max}}\: \leq \:{\aleph}_{{\breve{\rho}}} \:\leq \: {\aleph}_{{\breve{\rho}}_{min}}$, ${\beta}_{{\breve{\rho}}_{min}}\: \leq \:{\beta}_{{\breve{\rho}}} \:\leq \: {\beta}_{{\breve{\rho}}_{max}}$ and ${\mu}_{{\breve{\rho}}_{min}}\: \leq \:{\mu}_{{\breve{\rho}}} \:\leq \: {\mu}_{{\breve{\rho}}_{max}}$. Thus $\widehat{\daleth}({\breve{\rho}}) = \frac{1}{2} \left[\frac{{\mu}_{{\breve{\rho}}} +1 - {\nu}_{{\breve{\rho}}}}{2} + \frac{{\beta}_{{\breve{\rho}}} +1 - {\aleph}_{{\breve{\rho}}}}{2} \right] \: \leq \: \frac{1}{2} \left[\frac{{\mu}_{{\breve{\rho}}_{max}} +1 - {\nu}_{{\breve{\rho}}_{max}}}{2} + \frac{{\beta}_{{\breve{\rho}}_{max}} +1 - {\aleph}_{{\breve{\rho}}_{max}}}{2} \right] = \widehat{\daleth}({\breve{\rho}}_{max})$, similarly $\widehat{\daleth}({\breve{\rho}}) = \frac{1}{2} \left[\frac{{\mu}_{{\breve{\rho}}} +1 - {\nu}_{{\breve{\rho}}}}{2} + \frac{{\beta}_{{\breve{\rho}}} +1 - {\aleph}_{{\breve{\rho}}}}{2} \right] \: \geq \: \frac{1}{2} \left[\frac{{\mu}_{{\breve{\rho}}_{min}} +1 - {\nu}_{{\breve{\rho}}_{min}}}{2} + \frac{{\beta}_{{\breve{\rho}}_{min}} +1 - {\aleph}_{{\breve{\rho}}_{min}}}{2} \right]$. If $\bf{\widehat{\daleth}({\breve{ \pmb{\mathsf{ ρ}}}}) \: \leq \: \widehat{\daleth}({\breve{ \pmb{\mathsf{ ρ}}}}_{max}) } \quad$ and $\quad \bf{\widehat{\daleth}({\breve{ \pmb{\mathsf{ ρ}}}}) \: \geq \: \widehat{\daleth}({\breve{ \pmb{\mathsf{ ρ}}}}_{min}) }$, we have

Theorem 3.5. (Monotonicity) Assume that ${\breve{\rho}}_{h} = \left(\langle{\mu}_{h}, {\nu}_{h}\rangle, \langle {\beta}_{h}, {\aleph}_{h} \rangle \right)$ and ${\breve{\rho}}_{h^*} = \left(\langle{\mu}_{h^*}, {\nu}_{h^*}\rangle, \langle {\beta}_{h^*}, {\aleph}_{h^*} \rangle \right)$ are the families of LDFNs, where $\breve{\Gamma}_{h} = \prod_{h = 1}^{g-1} \widehat{\daleth}({\breve{\rho}}_h) \; (g = 2 \ldots, e)$, $\breve{\Gamma}_{1} = 1$, $\breve{\Gamma}_{h^*} = \prod_{h = 1}^{g-1} \widehat{\daleth}({\breve{\rho}}_{h^*}) \; (j = 2 \ldots, n)$, $\breve{\Gamma}_{1} = 1$, $\breve{\Gamma}_{1^*} = 1$, $\widehat{\daleth}({\breve{\rho}}_k)$ is the score of ${\breve{\rho}}_h$ LDFN, and $\widehat{\daleth}({\breve{\rho}}_{h^*})$ is the score of ${\breve{\rho}}_{h^*}$ LDFN. If ${\mu}_{h^*} \geq{\mu}_{h}$, ${\nu}_{h^*} \leq{\nu}_{h}$, ${\beta}_{h^*} \geq{\beta}_{h}$ and ${\aleph}_{h^*} \leq{\aleph}_{h}$for all $h$, then

Proof. Let $\varphi(t) = \frac{1-t}{1+t}$, $t\in [0, 1]$. Then $\varphi'(y) < 0$. So, $\varphi(y)$ is decreasing function on $(0, 1]$. If ${\nu}^*_{h} \leq{\nu}_{h}$ for all h. Then $\varphi({\nu}^*_{h}) \geq \varphi({\nu}_{h})$, i.e, .

Let $\breve{\mathcal{Z}} = \left(\frac{\breve{\Gamma}_{1}}{\beth_{h} \breve{\Gamma}_{h} }, \frac{\breve{\Gamma}_{2}}{\beth_{h} \breve{\Gamma}_{h} }, \ldots, \frac{\breve{\Gamma}_e}{\beth_{h} \breve{\Gamma}_{h} } \right)^T$ and $\breve{\mathcal{Z}}_* = \left(\frac{\breve{\Gamma}_{1^*}}{\beth_{h} \breve{\Gamma}_{s^*} }, \frac{\breve{\Gamma}_{2^*}}{\beth_{h} \breve{\Gamma}_{s^*} }, \ldots, \frac{\breve{\Gamma}_{e^*}}{\beth_{h} \breve{\Gamma}_{s^*} } \right)^T$ be the prioritized WVs of ${\breve{\rho}}_{h} = \left(\langle{\mu}_{h}, {\nu}_{h}\rangle, \langle {\beta}_{h}, {\aleph}_{h} \rangle \right)$ and ${\breve{\rho}}_{h^*} = \left(\langle{\mu}_{h^*}, {\nu}_{h^*}\rangle, \langle {\beta}_{h^*}, {\aleph}_{h^*} \rangle \right)$ respectively, s.t

$\beth_{h} \frac{\breve{\Gamma}_{h}}{\beth_{h} \breve{\Gamma}_{h}} = 1$ and $\beth_{h} \frac{\breve{\Gamma}_{h^*}}{\beth_{h} \breve{\Gamma}_{h^*}} = 1$.

Now,

Again, let $\pi(y) = \frac{2- y}{y}$. Then $\pi'(y) < 0$. So, $\pi(y)$ is decreasing function on $(0, 1]$. If ${\mu}^*_{h} \geq{\mu}_{h}$ for all h. Then $\pi({\mu}^*_{h}) \leq \pi({\mu}_{h})$, i.e, .

Let $\breve{\mathcal{Z}} = \left(\frac{\breve{\Gamma}_{1}}{\beth_{h} \breve{\Gamma}_{h} }, \frac{\breve{\Gamma}_{2}}{\beth_{h} \breve{\Gamma}_{h} }, \ldots, \frac{\breve{\Gamma}_e}{\beth_{h} \breve{\Gamma}_{h} } \right)^T$ and $\breve{\mathcal{Z}}_* = \left(\frac{\breve{\Gamma}_{1^*}}{\beth_{h} \breve{\Gamma}_{h^*} }, \frac{\breve{\Gamma}_{2^*}}{\beth_{h} \breve{\Gamma}_{h^*} }, \ldots, \frac{\breve{\Gamma}_{e^*}}{\beth_{h} \breve{\Gamma}_{h^*} } \right)^T$ be the prioritized WVs of ${\breve{\rho}}_{h} = \left(\langle{\mu}_{h}, {\nu}_{h}\rangle, \langle {\beta}_{h}, {\aleph}_{h} \rangle \right)$ and ${\breve{\rho}}_{h^*} = \left(\langle{\mu}_{h^*}, {\nu}_{h^*}\rangle, \langle {\beta}_{h^*}, {\aleph}_{h^*} \rangle \right)$ respectively, s.t $\beth_{h} \frac{\breve{\Gamma}_{h}}{\beth_{h} \breve{\Gamma}_{h}} = 1$ and $\beth_{h} \frac{\breve{\Gamma}_{h^*}}{\beth_{h} \breve{\Gamma}_{h^*}} = 1$.

Now,

Again, let $\xi(t) = \frac{1-t}{1+t}$, $t\in [0, 1]$. Then $\xi'(y) < 0$. So, $\xi(y)$ is decreasing function on $(0, 1]$. If ${\aleph}^*_{h} \leq{\aleph}_{h}$ for all h. Then $\xi({\aleph}^*_{h}) \geq \xi({\aleph}_{h})$, i.e, .

Let $\breve{\mathcal{Z}} = \left(\frac{\breve{\Gamma}_{1}}{\beth_{h} \breve{\Gamma}_{h} }, \frac{\breve{\Gamma}_{2}}{\beth_{h} \breve{\Gamma}_{h} }, \ldots, \frac{\breve{\Gamma}_e}{\beth_{h} \breve{\Gamma}_{h} } \right)^T$ and $\breve{\mathcal{Z}}_* = \left(\frac{\breve{\Gamma}_{1^*}}{\beth_{h} \breve{\Gamma}_{s^*} }, \frac{\breve{\Gamma}_{2^*}}{\beth_{h} \breve{\Gamma}_{s^*} }, \ldots, \frac{\breve{\Gamma}_{e^*}}{\beth_{h} \breve{\Gamma}_{s^*} } \right)^T$ be the prioritized WVs of ${\breve{\rho}}_{h} = \left(\langle{\mu}_{h}, {\nu}_{h}\rangle, \langle {\beta}_{h}, {\aleph}_{h} \rangle \right)$ and ${\breve{\rho}}_{h^*} = \left(\langle{\mu}_{h^*}, {\nu}_{h^*}\rangle, \langle {\beta}_{h^*}, {\aleph}_{h^*} \rangle \right)$ respectively, s.t

$\beth_{h} \frac{\breve{\Gamma}_{h}}{\beth_{h} \breve{\Gamma}_{h}} = 1$ and $\beth_{h} \frac{\breve{\Gamma}_{h^*}}{\beth_{h} \breve{\Gamma}_{h^*}} = 1$.

Now,

Again, let $G(y) = \frac{2- y}{y}$. Then $G'(y) < 0$. So, $G(y)$ is decreasing function on $(0, 1]$. If ${\beta}^*_{h} \geq{\beta}_{h}$ for all h. Then $G({\beta}^*_{h}) \leq G({\beta}_{h})$, i.e, .

Let $\breve{\mathcal{Z}} = \left(\frac{\breve{\Gamma}_{1}}{\beth_{h} \breve{\Gamma}_{h} }, \frac{\breve{\Gamma}_{2}}{\beth_{h} \breve{\Gamma}_{h} }, \ldots, \frac{\breve{\Gamma}_e}{\beth_{h} \breve{\Gamma}_{h} } \right)^T$ and $\breve{\mathcal{Z}}_* = \left(\frac{\breve{\Gamma}_{1^*}}{\beth_{h} \breve{\Gamma}_{h^*} }, \frac{\breve{\Gamma}_{2^*}}{\beth_{h} \breve{\Gamma}_{h^*} }, \ldots, \frac{\breve{\Gamma}_{e^*}}{\beth_{h} \breve{\Gamma}_{h^*} } \right)^T$ be the prioritized WVs of ${\breve{\rho}}_{h} = \left(\langle{\mu}_{h}, {\nu}_{h}\rangle, \langle {\beta}_{h}, {\aleph}_{h} \rangle \right)$ and ${\breve{\rho}}_{h^*} = \left(\langle{\mu}_{h^*}, {\nu}_{h^*}\rangle, \langle {\beta}_{h^*}, {\aleph}_{h^*} \rangle \right)$ respectively, s.t $\beth_{h} \frac{\breve{\Gamma}_{h}}{\beth_{h} \breve{\Gamma}_{h}} = 1$ and $\beth_{h} \frac{\breve{\Gamma}_{h^*}}{\beth_{h} \breve{\Gamma}_{h^*}} = 1.$

Now,

Again, Let,

and

Equations 3.9, 3.10, 3.11 and 3.12 can be written as ${\mu}_{{\breve{\rho}}} \leq{\mu}_{{\breve{\rho}}_{^*}}$, ${\nu}_{{\breve{\rho}}} \geq {\nu}_{{\breve{\rho}}_{^*}}$, ${\beta}_{{\breve{\rho}}} \leq{\beta}_{{\breve{\rho}}_{^*}}$ and ${\aleph}_{{\breve{\rho}}} \geq{\aleph}_{{\breve{\rho}}_{^*}}$. Thus $\widehat{\daleth}({\breve{\rho}}) = \frac{1}{2} \left[\frac{{\mu}_{{\breve{\rho}}} +1 - {\nu}_{{\breve{\rho}}}}{2} + \frac{{\beta}_{{\breve{\rho}}} +1 - {\aleph}_{{\breve{\rho}}}}{2} \right]$ $\leq \frac{1}{2} \left[\frac{{\mu}_{{\breve{\rho}}_{^*}} +1 - {\nu}_{{\breve{\rho}}_{^*}}}{2} + \frac{{\beta}_{{\breve{\rho}}_{^*}} +1 - {\aleph}_{{\breve{\rho}}_{^*}}}{2} \right]$ Therefore, $\widehat{\daleth}({\breve{\rho}}) \leq\widehat{\daleth}({\breve{\rho}}_{^*})$. we get

Theorem 3.6. (Idempotency) Assume that ${\breve{\rho}}_{h} = \left(\langle{\mu}_{h}, {\nu}_{h}\rangle, \langle {\beta}_{h}, {\aleph}_{h} \rangle \right)$ is the assemblage of LDFNs, where $\breve{\Gamma}_{h} = \prod_{h = 1}^{g-1} \widehat{\daleth}({\breve{\rho}}_h) \; (g = 2 \ldots, e)$, $\breve{\Gamma}_{1} = 1$ and $\widehat{\daleth}({\breve{\rho}}_h)$ is the score of ${\breve{\rho}}_h$ LDFN. If all ${\breve{\rho}}_{h}$ are equal, i.e, . ${\breve{\rho}}_{h} = {\breve{\rho}}$for all $h$, then

Proof.

Corollary 3.7. If ${\breve{\rho}}_{h} = \left(\langle{\mu}_{h}, {\nu}_{h}\rangle, \langle {\beta}_{h}, {\aleph}_{h} \rangle \right)$ is the assemblage of largest LDFNs, i.e, . ${\breve{\rho}}_{h} = (\langle 1, 0\rangle, \langle 1, 0\rangle)$$\forall j$, then

Proof. We can easily obtain Corollary similar to the Theorem 3.6.

Theorem 3.8. Let ${\breve{\rho}}_{h} = \left(\langle{\mu}_{h}, {\nu}_{h}\rangle, \langle {\beta}_{h}, {\aleph}_{h} \rangle \right)$ be the assemblage of LDFNs and let

be the WV of ${\breve{\rho}}_{h} = \left(\langle{\mu}_{h}, {\nu}_{h}\rangle, \langle {\beta}_{h}, {\aleph}_{h} \rangle \right)$. Then,

where $\breve{\Gamma}_{h} = \prod_{h = 1}^{g-1} \widehat{\daleth}({\breve{\rho}}_h) \; (g = 2 \ldots, e)$, $\breve{\Gamma}_{1} = 1$ and $\widehat{\daleth}({\breve{\rho}}_{h})$ is the score of $h^{th}$ LDFN.

Proof. Let $\text{LDFEPWA} ({\breve{\rho}}_1, {\breve{\rho}}_2, \ldots, {\breve{\rho}}_e) = \left(\langle{\mu}_{h}^E, {\nu}_{h}^E \rangle, \langle {\beta}_{h}^E, {\aleph}_{h}^E \rangle \right)$ and $LDFPWA ({\breve{\rho}}_1, {\breve{\rho}}_2, \ldots, {\breve{\rho}}_e) = \left(\langle{\mu}_{h}, {\nu}_{h}\rangle, \langle {\beta}_{h}, {\aleph}_{h} \rangle \right)$,

we have

From this we get,

These are equal iff ${\mu}_1 = {\mu}_2 = \ldots = {\mu}_e$.

Also,

Thus,

These are equal iff ${\nu}_1 = {\nu}_2 = \ldots = {\nu}_n$.

Also we have,

From this we get,

These are equal iff ${\beta}_1 = {\beta}_2 = \ldots = {\beta}_e$.

Also,

Thus,

These are equal iff ${\aleph}_1 = {\aleph}_2 = \ldots = {\aleph}_n$.

Equations 3.16, 3.17, 3.18 and 3.19 imply,

Thus we have the following relationship by defining the score function of LDFS.

3.2.   LDFEPWG operator

Definition 3.9. Let ${\breve{\rho}}_{h} = \left(\langle{\mu}_{h}, {\nu}_{h}\rangle, \langle {\beta}_{h}, {\aleph}_{h} \rangle \right)$ be the assemblage of LDFNs, and LDFEPWG: $\Theta^n \rightarrow \Theta$, be a n dimension mapping. if

then the mapping LDFEPWG is called "linear Diophantine fuzzy Einstein prioritized weighted geometric (LDFEPWG) operator", where $\breve{\Gamma}_{h} = \prod_{h = 1}^{g-1} \widehat{\daleth}({\breve{\rho}}_h) \; (g = 2 \ldots, e)$, $\breve{\Gamma}_{1} = 1$ and $\widehat{\daleth}({\breve{\rho}}_{h})$ is the score of $h^{th}$ LDFN.

We may also consider LDFEPWG operator based on Einstein operational principles using the theorem follows.

Theorem 3.10. ${\breve{\rho}}_{h} = \left(\langle{\mu}_{h}, {\nu}_{h}\rangle, \langle {\beta}_{h}, {\aleph}_{h} \rangle \right)$ be the assemblage of LDFNs, we can also find LDFEPWG by

where $\breve{\Gamma}_{h} = \prod_{h = 1}^{g-1} \widehat{\daleth}({\breve{\rho}}_h) \; (g = 2 \ldots, e)$, $\breve{\Gamma}_{1} = 1$ and $\widehat{\daleth}({\breve{\rho}}_{h})$ is the score of $h^{th}$ LDFN.

Proof. Proof is same as Theorem 3.2.

Example 3.11. Let ${\breve{\rho}}_1 = (\langle0.60, 0.55\rangle, \langle0.15, 0.15\rangle)$, ${\breve{\rho}}_2 = (\langle0.65, 0.70\rangle, \langle 0.30, 0.65\rangle)$ and ${\breve{\rho}}_3 = (\langle0.45, 0.70\rangle, \langle 0.25, 0.45\rangle)$ be the LDFNs, prioritization between given LDFNs is given as ${\breve{\rho}}_1 \succ {\breve{\rho}}_2 \succ {\breve{\rho}}_3$ then we have,

Below we define some of LDFEPWG operator's appealing properties.

Theorem 3.12. (Monotonicity) Assume that ${\breve{\rho}}_{h} = \left(\langle{\mu}_{h}, {\nu}_{h}\rangle, \langle {\beta}_{h}, {\aleph}_{h} \rangle \right)$ and ${\breve{\rho}}_{h^*} = \left(\langle{\mu}_{h^*}, {\nu}_{h^*}\rangle, \langle {\beta}_{h^*}, {\aleph}_{h^*} \rangle \right)$ are the families of LDFNs, where $\breve{\Gamma}_{h} = \prod_{h = 1}^{g-1} \widehat{\daleth}({\breve{\rho}}_h) \; (g = 2 \ldots, e)$, $\breve{\Gamma}_{1} = 1$, $\breve{\Gamma}_{h^*} = \prod_{h = 1}^{g-1} \widehat{\daleth}({\breve{\rho}}_{h^*}) \; (j = 2 \ldots, n)$, $\breve{\Gamma}_{1^*} = 1$, $\widehat{\daleth}({\breve{\rho}}_h)$ is the score of ${\breve{\rho}}_h$ LDFN and $\widehat{\daleth}({\breve{\rho}}_{h^*})$ is the score of ${\breve{\rho}}_{h^*}$ LDFN. If ${\mu}_{h^*} \geq{\mu}_{h}$, ${\nu}_{h^*} \leq{\nu}_{h}$ and ${\aleph}_{h^*} \leq{\aleph}_{h}$for all $h$, then

Proof. Proof is same as Theorem 3.5.

Theorem 3.13. (Boundary) Assume that ${\breve{\rho}}_{h} = \left(\langle{\mu}_{h}, {\nu}_{h}\rangle, \langle {\beta}_{h}, {\aleph}_{h} \rangle \right)$ be the assemblage of LDFNs, where $\breve{\Gamma}_{h} = \prod_{h = 1}^{g-1} \widehat{\daleth}({\breve{\rho}}_h) \; (g = 2 \ldots, e)$, $\breve{\Gamma}_{1} = 1$ and $\widehat{\daleth}({\breve{\rho}}_h)$ is the score of ${\breve{\rho}}_h$ LDFN, then

where, ${\breve{\rho}}_{min} = min({\breve{\rho}}_{h}) \quad \mathit{\text{and}} \quad {\breve{\rho}}_{max} = max ({\breve{\rho}}_{h})$.

Proof. Proof is same as Theorem 3.4.

Theorem 3.14. (Idempotency) Assume that ${\breve{\rho}}_{h} = \left(\langle{\mu}_{h}, {\nu}_{h}\rangle, \langle {\beta}_{h}, {\aleph}_{h} \rangle \right)$ is the assemblage of LDFNs, where $\breve{\Gamma}_{h} = \prod_{h = 1}^{g-1} \widehat{\daleth}({\breve{\rho}}_h) \; (g = 2 \ldots, e)$, $\breve{\Gamma}_{1} = 1$ and $\widehat{\daleth}({\breve{\rho}}_h)$ is the score of ${\breve{\rho}}_h$ LDFN. If all ${\breve{\rho}}_{h}$ are equal, i.e, . ${\breve{\rho}}_{h} = {\breve{\rho}}$for all $h$, then

Proof. Proof is same as Theorem 3.6.

Corollary 3.15. If ${\breve{\rho}}_{h} = \left(\langle{\mu}_{h}, {\nu}_{h}\rangle, \langle {\beta}_{h}, {\aleph}_{h} \rangle \right) \; j = (1, 2, \ldots n)$ is the assemblage of largest LDFNs, i.e, . ${\breve{\rho}}_{h} = (\langle 1, 0\rangle, \langle 1, 0\rangle)$$\forall j$, then

Proof. We can easily obtain Corollary similar to the Theorem 3.14.

Theorem 3.16. Let ${\breve{\rho}}_{h} = \left(\langle{\mu}_{h}, {\nu}_{h}\rangle, \langle {\beta}_{h}, {\aleph}_{h} \rangle \right)$ be the assemblage of LDFNs and let

be the WV of ${\breve{\rho}}_{h} = \left(\langle{\mu}_{h}, {\nu}_{h}\rangle, \langle {\beta}_{h}, {\aleph}_{h} \rangle \right)$. Then,

where $\breve{\Gamma}_{h} = \prod_{h = 1}^{g-1} \widehat{\daleth}({\breve{\rho}}_h) \; (g = 2 \ldots, e)$, $\breve{\Gamma}_{1} = 1$ and $\widehat{\daleth}({\breve{\rho}}_h)$ is the score of ${\breve{\rho}}_h$ LDFN.

Proof. Proof is similar to Theorem 3.8.

4.   Proposed methodology

Let $\widetilde{\mathfrak{R}}^{\beth} = \{\widetilde{\mathfrak{R}}^{\beth}_1, \widetilde{\mathfrak{R}}^{\beth}_2, \ldots, \widetilde{\mathfrak{R}}^{\beth}_m \}$ and $\breve{\Cup} = \{\breve{\Cup}_1, \breve{\Cup}_2, \ldots, \breve{\Cup}_n \}$ are the assemblages of alternatives and criterions respectively, priorities are assigned between the criteria provided by the linear orientation in this case. $\breve{\Cup}_1 \succ \breve{\Cup}_2 \succ \breve{\Cup}_3 \succ \ldots \succ \breve{\Cup}_n$ indicates criteria $\breve{\Cup}_j$ has a high priority than $\breve{\Cup}_i$ if $j > i$. $\tilde{\Phi} = \{\tilde{\Phi}_1, \tilde{\Phi}_2, \ldots, \tilde{\Phi}_p \}$ is a collection of DMs. Prioritization is provided by a linear pattern between the DMs given as, $\tilde{\Phi}_1 \succ \tilde{\Phi}_2 \succ \tilde{\Phi}_3 \succ \ldots \succ \tilde{\Phi}_p$ shows DM $\tilde{\Phi}_{\zeta}$ has a high importance than $\tilde{\Phi}_{\varrho}$ if $\zeta > \varrho$. DMs give a matrix according to their own standpoints $D^{(p)} = (\mathfrak{Y}^{(p)}_{ij})_{m\times n }$, where $\mathfrak{Y}^{(p)}_{ij}$ is given for the alternatives $\widetilde{\mathfrak{R}}^{\beth}_i \in \widetilde{\mathfrak{R}}^{\beth}$ with respect to the attribute $\breve{\Cup}_j \in \breve{\Cup}$ by $\tilde{\Phi}_p$ DM. If all performance criteria are the same kind, there is no need for normalization; however, since MCGDM has two different types of evaluation criteria (benefit kind attributes $\tau_b$ and cost kinds attributes $\tau_c$), the matrix $D_(p)$ has been transformed into a normalize matrix using the normalization formula $Y^{(p)} = (\aleph^{(p)}_{ij})_{m\times n }$,

where $(\mathfrak{Y}^{(p)}_{ij})^c$ show the compliment of $\mathfrak{Y}^{(p)}_{ij}$.

The suggested operators will be implemented to the MCGDM, which will require the preceding steps.

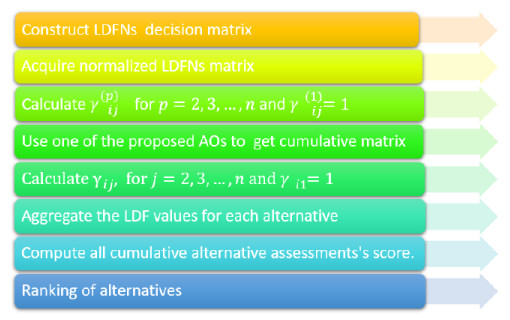
Pictorial view of Algorithm is shown in Figure 1.

5.   Case study

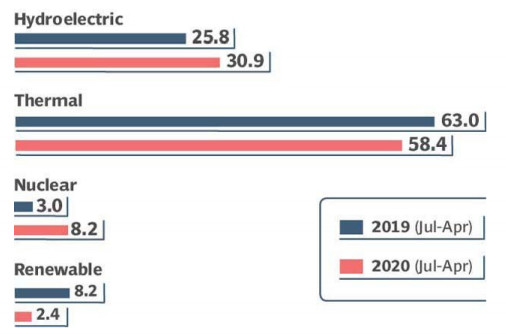
Following and opening up the Pakistan's reforms, a huge number of power plant projects are built to meet the need for electricity from social and economic progress. To meet this building requirement, the public bidding and tendering system has been employed since 1985 to buy thermal power equipment. Thermal power equipment supplier selection is an important component of thermal power equipment bidding and tendering management, which is also required for the smooth and long-term development of thermal power plants. Figure 2 depicts the share of electricity generated by various sources. With the tremendous increase in the use of fossil fuels and the ever-increasing pollution of our climate, the terms "green growth" and "sustainable development" have come to dominate world discourse.

A thermal power plant is a type of power plant that uses heat energy to generate electricity. Water is heated first in a thermal power plant, and then steam is generated from that heated water, spinning a steam turbine and driving a generator. In fact, high temperature and high pressure steam is responsible for the turbine spinning, which is then passed to the generator to generate power. In the ranking cycle, a condenser is used to condense the steam, which is then returned to where it was heated previously. Thermal power plants are classified into two types based on their fuel: fossil fuel power plants and biomass-fueled power plants. A fossil fuels thermal power plant generates electricity by burning fossil fuels such as coal, natural gas, or petroleum oil. They are built on a big scale to run indefinitely. A steam turbine is utilized in this sort of power plant, whereas a combustion turbine is used in natural gas-powered facilities. Currently, many biomass power facilities burn timber, agricultural, or construction wood waste. Biomass fuel is used in direct combustion power plant boilers, which supply energy to the same type of steam electric generators that use fossil fuels. This method turns biomass into methane gas, which is subsequently utilized to power steam generators, combustion turbines, and fuel cells.

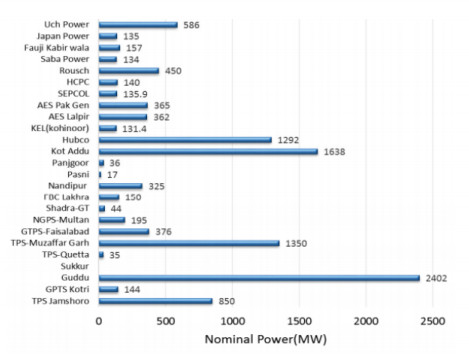
Based on prime mover, thermal power plants are classified into three types: steam turbine plants, gas turbine power plants, and combined cycle plants. In steam turbine power plants, the dynamic pressure produced by expanding steam is used to drive the turbine blades. Almost all non-hydro power plants use this approach. Approximately 80% of the world's electric power is generated using steam turbines. A gas turbine power plant is made up of three main parts: a compressor, a combustion system, and a turbine. Combination cycle power plants use both gas and steam engines to generate electricity. The waste heat from the gas turbine is directed to a nearby steam turbine, which generates extra energy. The majority of Pakistan's electricity is generated by thermal power plants, which use resources such as oil, gas, and coal. Some are combined-cycled, while others are steam and gas turbines. Pakistan has 49 thermal power plants located in the provinces of Punjab, Sindh, and Baluchistan. Thermal power generates 61 percent of Pakistan's electricity. Pakistan has 16599MW of installed thermal capacity. Guddu's capacity is 2402MW, TPS Muzaffargarh's capacity is 1350MW, Kot Addu's capacity is 1638MW, and HUBCO Baluchistan's capacity is 1200MW. The NGPS Multan, which was completed in 1960 and has a capacity of 195MW, is the oldest. Pakistan recently completed three biomass-powered power facilities with a combined capacity of 67MW ^[44]. Nominal power of different thermal power station are shown below in Figure 3.

Thermal power is the primary source of energy in Pakistan, and green supplier selection of thermal power equipment is critical to the smooth and long-term development of thermal power plants. As a result, in an environment that promotes the sustainable development of energy conservation and emissions reduction, choosing the right green supplier of thermal power equipment with green production consciousness is critical to the company's long-term development and the long-term viability of Pakistan's electric power industry.

The selection of environmentally friendly suppliers is an important MCDM problem ^[46]. The MCDM methodology ranks possible alternatives and chooses the best alternative by employing specific methods based on established decision-making knowledge derived from various parameters, and it has increasingly become a research subject in the fields of decision science, system science, and management science. Green supplier selection is an MCDM problem that must take into account a variety of factors, including cost, delivery time, environmental impact, and so on. Improving a company's environmental impact must be a major aspect of its management structure and business aim in order for it to succeed. What are the essential SCM principles? A article titled "The seven principles of supply chain management" was published in 1997 by Anderson et al. ^[45] in the supply chain management review. SCM was a relatively new concept at the time, but this paper did a fantastic job of conveying the fundamentals of SCM in a single round. After over 20 years, this work has become recognized as the "classic" manuscript and was republished in 2010. This manuscript has now earned over 300 citations in academic literature and business periodicals. Supplier selection studies can be divided into two categories: descriptive and analytical models. The descriptive studies look at the primary parameters for supplier assessment and selection. Dickson ^[47] presented 23 factors that contractors regarded to be relevant in various vendor selection difficulties. He discovered that the most critical metrics were time, performance, cost, and delivery. Wind et al. ^[48] discovered that many aspects were involved in various other supplier performance evaluations. Ho et al. ^[49] evaluated all techniques in a multi-criteria selection of international journals from 2000 to 2008 and determined that the most common characteristics used to measure the output of vendors were distribution, quality, cost, and so on. Weber et al. ^[50] analyzed 74 publications on supplier selection in empirical research models and identified a variety of strategies that have emerged in studies over the previous 25 years. They concluded that the majority of the approaches used were linear weighting, regression models, and certain optimization algorithms. A more recent examination of supplier recruitment and selection processes can be found here. In the supplier assessment paradigm, Amid et al. ^[51] examined fuzzy parameters. Jolaiet al. ^[52] suggested a fuzzy MCDM mechanism to get aggregated ratings from multiple vendors and then suggested the most appropriate ones utilizing the second-level objective programming (GP) technique. Sevkli et al. ^[53] tested the weights of their fuzzy linear programming model for supplier selection using an empirical hierarchical approach. Environmental challenges have become more relevant across businesses and areas as a result of climate change and global warming ^[54]. In recent decades, increasing emphasis has been paid to the research of the GSCM in an attempt to reduce atmospheric pollution raise awareness, and protect the environment ^[55]. The identifying and selection of relevant low-carbon suppliers is critical to the creation of a sustainable supply chain and, as a result, the resulting in ecological limitations. Simply expressed, the MCDM problem represents the vendor evaluation mechanism because, during the decision-making phase, cacophonic and various parameters must be analyzed and confirmed ^[56]. To date, the MCDM approaches have been used to assess and procure low carbon suppliers, but it has also been assumed that the attribute data are certain and correct ^[57]. Fortunately, the quick economic expansion and dynamic commercial atmosphere make it more difficult for decision-makers to deliver trustworthy analysis or preference specifics due to the inconsistency of human reasoning involved. Tong and Wang ^[58] employed the induced IF operator to tackle the low-carbon vendor selection problem lately. Zeng et al. ^[59] developed PF self-confidence AOs to address low-carbon supplier selection. As indicated in the introduction, the relaxation of limits on the MD and NMD of LDFSs allows for a wider range, making LDFS superior to IFS, PFS, and q-ROFS in the definition of unreliable and ambiguous information. In the framework of LDFS, it is therefore necessary and suitable to conduct a thorough investigation of the thermal power equipment provider selection issue.

5.1.   Illustrative example

To illustrate the proposed method below, we also provide a numerical example.

Consider a set of alternatives $\widetilde{\mathfrak{R}}^{\beth} = \{\widetilde{\mathfrak{R}}^{\beth}_1, \widetilde{\mathfrak{R}}^{\beth}_2, \widetilde{\mathfrak{R}}^{\beth}_3, \widetilde{\mathfrak{R}}^{\beth}_4 \}$ and $\breve{\Cup} = \{\breve{\Cup}_1, \breve{\Cup}_2, \breve{\Cup}_3, \breve{\Cup}_4 \}$ set of criterions, where $\breve{\Cup}_1$ = equipment quotation, $\breve{\Cup}_2$ = delivery accuracy rate, $\breve{\Cup}_3$ = equipment efficiency and $\breve{\Cup}_4$ = environmental consciousness. Priorities are assigned between the criteria provided by the linear orientation in this case. $\breve{\Cup}_1 \succ \breve{\Cup}_2 \succ \breve{\Cup}_3 \succ \ldots \succ \breve{\Cup}_5$ indicates criteria $\breve{\Cup}_J$ has a high priority than $\breve{\Cup}_i$ if $j > i$. In this example we use LDFNs as input data for ranking the given alternatives under the given attributes. Here three DMs are involved i.e $\tilde{\Phi}_1, \tilde{\Phi}_2$ and $\tilde{\Phi}_3$. DMs are not given the same priority. Prioritization is provided by a linear pattern between the DMs given as, $\tilde{\Phi}_1 \succ \tilde{\Phi}_2 \succ \tilde{\Phi}_3$ shows DM $\tilde{\Phi}_{\zeta}$ has a high imprtance than $\tilde{\Phi}_{\varrho}$ if $\zeta > \varrho$.

Step 1:

Compute the decision matrix $D^{(p)} = ({\aleph}^{(p)}_{ij})_{m\times n }$ in the form of LDFNs, given in Tables 2, 3 and 4.

Step 2:

Normalize the decision matrixes using Eq 4.1. First criteria is $\breve{\Cup}_1$ cost type and other is benefit type, given in Tables 5, 6 and 7.

Step 3:

Calculate the values of $\Gamma_{ij}^{(p)}$ by Eq 4.2.

Step 4:

Use LDFPWA to aggregate all individual LDF decision matrices $Y^{(p)} = (\mathcal{P}^{(p)}_{ij})_{m\times n }$ into one cumulative assessments matrix of the alternatives $W^{(p)} = (\mathcal{W}_{ij})_{m\times n }$ given below.

Step 5:

Evaluate the values of $\Gamma_{ij}$ by using Eq 4.3.

Step 6:

Aggregate the LDF values $\mathcal{W}_{ij}$ for each alternative $\widetilde{\mathfrak{R}}^{\beth}_{i}$ by the LDFPWA operator given in Table 8.

Step 7:

Calculate the score of all LDF aggregated values $\mathcal{W}_{i}$.

Step 8:

Rank by score function values.

So,

$\widetilde{\mathfrak{R}}^{\beth}_2$ is the best alternative.

5.2.   Comparison analysis

This section compares proposed AOs to some current AOs. Our proposed operators are distinct in that they both provide the same effect. By solving the information data with certain pre-existing operators and arriving at the same best option, we equate our findings. This indicates the robustness and validity of the models we proposed. The given methodologies on LDFNs are more effective and superior to some current theories due to their reference parameterizations. The benefit of this structure is that it separates MDs and NMDs and generates categorization criteria through parameterizations. The comparison of presented aggregation operators with some existing operators is given in Table 9.

6.   Conclusions

The evaluation of alternatives suggested by DMs is frequently accompanied by severe constraints that affect the decision making analysis. To alleviate these limits, an LDFS is a strong mathematical technique to expressing imprecise and uncertain information in real-world situations. The Einstein t-conorm and t-norm are frequently employed to provide smooth information fusion, and prioritized operators are effective for prioritized interactions between numerous criteria.

Main findings of this manuscript are listed as follows.

● To aggregate the LDF information and to use benefits of both Einstein operators and prioritized operators, we developed new AOs named as "linear Diophantine fuzzy Einstein prioritized weighted average (LDFEPWA) operator" and "linear Diophantine fuzzy Einstein prioritized weighted geometric (LDFEPWG) operator".

● We also investigated certain properties of proposed hybrid operators like, monotonicity, idempotency, etc.

● Furthermore, we suggested a robust MCDM approach to demonstrate the power and functionality of the developed operators. Furthermore, we used the newly established AOs to illustrate the decision-making problems.

● The proposed approach has the ability to evaluate and select the green suppliers of thermal power equipment with partial or a lack of quantitative information, and using the LDFNs can overcome the uncertainty. A numerical illustration has been proposed to demonstrate that the suggested operators have a more realistic way to solve decision making processes.

● Finally, we presented some comparisons with the existing operators to demonstrate the novel methodology's validity, practicability, and effectiveness.

Future directions of proposed AOs are listed as follows.

1. We extend the concept of these AOs to the other different extensions of fuzzy set, like picture fuzzy set, T-spherical fuzzy set, single-valued neutrosophic set and ets.

2. The concepts can be applied to effectively deal with ambiguity in a wide range of real scenarios, including business, machine intelligence, brand management, cognitive science, finding the shortest dilemma, representative democracy, pattern classification, deep learning, diagnostics, trade assessment, projections, agri-business assessment, mechatronics, cryptography, computer vision, hiring process issues, and so on.

3. We also extend this concept to different types of measures like, similarity measurers, divergence measures, entropy measures and knowledge measures.

Acknowledgments

This research was funded by National Science, Research and Innovation Fund (NSRF) and King Mongkut's University of Technology North Bangkok with Contract no. KMUTNB-FF-65-24.

Conflict of interest

The authors have no conflicts of interest to declare.