1.
Introduction
Studying the theory of fractional differential equations (FDEs) and fractional integral equations (FIEs) is crucial because they are used in many modeling applications. Fractional equations are essential for many areas of fundamental analysis and their applications in economics, physics, and other disciplines. Quadratic integral equations (QIE), in particular, tend to be helpful in describing a wide range of everyday issues, such as theory of radiative transfer, the theory of neutron transport, the kinetic theory of gases, the queuing theory, and the traffic theory (see, for example, [1,2,3,4]).
One of the studied QIEs is called the hybrid integral equation (HIE); see [5]. This issue has received great attention in the last few years; see [6,7,8,9]. As we see, the hybrid fixed point theory is used to develop the existing solution of the hybrid equations; see [10,11,12,13]. Other researchers focused on the analysis of QIEs in Orlicz spaces [14], equations of QIEs with fractional order arising in the queuing theory and biology [15], and the analysis of QIEs depending on both Schauder and Schauder–Tychonoff fixed point principles [16].
1.1. Chandrasekhar quadratic integral equation
The Chandrasekhar quadratic integral equation (CQIE) occurs in the theory of radiative transfer in a plane-parallel atmosphere [2]. The radiative transfer process and the integral equation for the scattering function and transmitted functions were developed by Chandrasekhar's work in the 1950s; see [14]. This work quickly turned into a major scientific topic in both mathematics and astrophysics, see [15,16]. The radiative transfer process and the simultaneous integral equation for the transmitted and scattering functions were developed in Chandrasekhar's seminal work from 1960. In [17], the simultaneous integral equation of Chandrasekhar was presented, along with the iteration scheme for the transmitted and scattering functions.
CQIE takes the form
where RLJμ denotes the Riemann-Liouville fractional integral (RLFI) of order μ, f1(ϱ,Φ(ϱ))∈C(ˆI×R,R−{0}), and ˆgκ(ϱ,Φ(ϱ))∈C(ˆI×R,R), for κ=1,2.
As a result of the applications of these equations, researchers were interested in studying them, and as a result of the difficulty of finding exact solutions, CQIEs are solved using the ADM and the PM. The ADM provides many advantages, including the ability to solve a variety of linear and nonlinear equations in deterministic or stochastic fields effectively and present an analytical solution for all of these equation types without requiring linearization or discretization. Additionally, it is reliable and provides faster convergence than other classical methods. Moreover, we use measures of weak noncompactness to study existence and uniqueness results. These results demonstrate that the two solutions provide nearly equal accuracy; however, when comparing the time required in each case, the ADM is found to take less time than the PM.
It can be summarized as follows: first, the second section introduces the basic concepts of the measure of noncompactness and the hypothesis. In the third section, we show that if the solution exists, then it will be unique and convergent. After that, we solve some nonlinear Chandraseker QIEs with fractional orders with a comparison between ADM and PM techniques. Finally, graphs are also constructed to illustrate the effectiveness of these two approaches and to compare them.
2.
Measure of noncompactness (MNC)
In the complement of this work, the classical Banach space C(ˆI)=C[0,b] is used, which contains all real continuous functions defined on ˆI having the norm
Let us recall the MNC definition in C(ˆI) which is used in this investigation and fix a bounded nonempty subset Ω of C(ˆI). For η∈Ω and ϵ>0, the modulus of continuity of the function η on the interval ˆI is defined by
and
It is well-known that ω0(Ω) is a measure of noncompactness inC(ˆI)such that the Hausdorff measure χ may be expressed by the formula
see [18]. We use the following theorem to prove our investigation.
Theorem 1. [18] Let Q be a bounded, nonempty, and closed convex subset in the space E. Also, let ˆH:Q→Q be a continuous operator such that χ(ˆHΩ)≤l χ(Ω) to any nonempty subset Ω of Q, as l∈[0,1) is constant, so ˆH has a fixed point in the set Q.
Considering the hypothesis:
i. φ :ˆI→R is continuous.
ii. f1:ˆI×R→R is continuous and the function f1(ϱ,0)∈ C(R),R is the space of all bounded continuous functions, and there exists a positive constant M=supϱ∈ˆI|f1(ϱ,0)|.
iii. |f1(ϱ,Φ)−f1(ϱ,ζ)|≤L|Φ−ζ| for any ϱ∈ˆI for all Φ,ζ∈R.
iv. ˆgκ:ˆI×R→R,κ =1,2 satisfies the Carathéodory condition (CC) so, it is measurable in ϱ for all Φ∈R and continuous in Φ for all ϱ∈ˆI, and there exist functions mκ, κ =1,2∈L1 such that:
v. RLJγm2(ϱ)<M2, γ≤μ, C≥0, and ∫b01ϱ+sm1(s)ds≤M1.
vi. There exists a number R0>0, such that
3.
Existence and uniqueness theorems
Define the ball BR0 as
Theorem 2. Using the hypotheses (i)−(vi), if [M2Lbμ−γΓ(μ−γ+1)+M1b2]<1, then there exists at least a solution Φ∈C(ˆI) for HIE (1.1).
Proof. Let the operator ˆH defined on C(ˆI) be
From the hypotheses (i)−(vi), the function ˆHΦ is continuous on ˆI for any Φ∈BR0. Further, applying the given hypothesis, we derive the following estimate:
So ˆHΦ is bounded on the interval ˆI. Also, we get
for the operator ˆH, which transforms the ball BR0 into itself, and R0 is:
Now, we are going to show that the operator ˆH is continuous on the ball BR0. So, we need to prove that the operator G2 defined by
is continuous on BR0. To do this, fix ϵ>0, let Φ0∈BR0, and from hypothesis (ii), we find δ>0 such that ||Φ−Φ0||≤δ, and then we have |ˆg2(s,Φ)−ˆg2(s,Φ0)|≤ϵ for s ∈ˆI, where Φ is any arbitrary element in BR0. For arbitrary fixed ϱ∈ˆI, we get
The operator f1Φ(ϱ)=f1(ϱ,Φ(ϱ)) is continuous, and then the operator f1.G2 is continuous on BR0, and similarly, we can prove that the operator
is continuous on BR0. This shows that ˆH is continuous on BR0. Let χ be a nonempty subset of BR0. Fix ϵ>0, and choose Φ∈χ and ϱ1,ϱ2∈ˆI such that |ϱ2−ϱ1|≤ϵ. Let ϱ1≤ϱ2, and then
Hence,
and so
Then
Then
We get
so,
where Ψ(f1,ϵ)=sup{|f1(ϱ2,Φ(ϱ1)−f1(ϱ1,Φ(ϱ1))|:ϱ1,ϱ2∈ˆI,|ϱ2−ϱ1|≤ϵ,Φ∈BR0}.
Knowing that f1 is uniformly continuous on the set ˆI ×BR0, we derive the inequality
Then
From (2.4), this inequality leads to
If [M2Lbμ−γΓ(μ−γ+1)+M1b2]<1 and using Theorem 1, there exists at least a solution Φ∈C(ˆI) for HIE (1.1).
The following hypotheses are satisfied if there exist ˆgκ, κ =1,2, such that (iv)∗ ˆgκ: ˆI×R→R, κ =1,2, satisfies CC, so it can be measurable in ϱ for all Φ∈R and continuous in Φ for all ϱ∈ˆI. Then
for all ϱ∈ˆI and Φ,ζ∈ R. Let Φ1 and Φ2 be two solutions for the HIE (1.1), and hence
where Υ=(b2M1+L1b2R0+LM2bμ−γΓ(μ−γ+1)+L2bμ(LR0+M)Γ(μ+1)). Then we get the theorem:
Theorem 3. Assume that the hypotheses (i)–(vi) are satisfied, and Υ<1. Then, the solution Φ∈C(ˆI) of (1.1) is unique.
4.
Methods for the solutions
4.1. Adomian decomposition method (ADM)
In the 1980s, Adomian presented the ADM [19,20,21], which is an analytical method used to solve a lot of different equations such as DEs, IEs, integro-differential equations, and partial DEs [22,23,24,25]. The obtained solution is an infinite series that converges to the exact solution. An important benefit of the ADM is that there is no linearization or perturbation that can change the main problem that has been solved, which is serious. A lot of researchers are interested in using the ADM, as it is successfully applied to many applications that appear in applied sciences [26,27,28]. In this research, the ADM is used as the first method to solve the HIE (1.1).
Applying the ADM to (1.1), the ADM solution algorithm is
where ˆAκ, and ˇDκ are Adomian polynomials of the nonlinear terms ˆg1(ϱ,Φ),f1(ϱ,Φ), and ˆg2(ϱ,Φ) which take the forms
Finally, the ADM solution is
4.1.1. Convergence analysis
Theorem 4. If hypotheses (i)−(vi) are satisfied, Υ2<1, and |Φ1(ϱ)|<k, where k is a positive constant, then the series solution (4.5) of (1.1) using the ADM is convergent.
Proof. Define the sequence {ˆSρ} such that ˆSρ=ρ∑κ=0Φκ(ϱ) is a sequence of partial sums taken from the series (4.5), and
Let ˆSρ and ˆSθ be two partial sums of the ADM series solution such that ρ>θ. We want to prove that {ˆSρ} is a Cauchy sequence (CS) in this Banach space (Bs).
hence,
Thus, by applying |⋅| to both sides, we find
where Υ2=[b2M1+LM2(b)μ−γΓ(μ−γ+1)]. Let ρ=θ+1, and we get
Using the triangle inequality, we arrive at
If 0<Υ2<1 and ρ>θ, this leads to (1−Υρ−θ2)≤1. Then
If |Φ1(ϱ)|<k, θ→∞, then ‖ˆSρ−ˆSθ‖→0, which leads to {ˆSρ} being a CS in this BS and the series (4.5) is convergent.
4.1.2. Error analysis
Theorem 5. The maximum absolute error of the ADM series solution (4.5) is
Proof. In Theorem 2, we see that
and ˆSρ=ρ∑κ=0Φκ(ϱ), ρ→∞. Then, ˆSρ→Φ(ϱ), and hence
and the maximum absolute error is written as
4.2. Picard method (PM)
The method of successive approximations (PM) was presented by Emile Picard in 1891. PM and ADM methods were first compared by Rach and Bellomo in 1987 [26,29]. In 1999, Golberg deduced that these two methods were equivalent for linear differential equations [30]. But this equivalence is not achieved in the nonlinear case. In 2010, El-Sayed et al. used them to solve QIE [31]. In 2012, El-Sayed et al. used them to solve a coupled system of fractional QIEs [32]. In 2014, El-Sayed et al. used them to solve FQIE [33]. In 2024, Ziada used them to solve a nonlinear FDE system containing the Atangana–Baleanu derivative [34]. In this research, we use them to get the solution for a nonlinear HDE and compare their results.
Applying the PM to the QIE (1.1), the solution is a sequence constructed by
All the functions Φκ(ϱ) are continuous functions and Φκ are the sum of successive differences
Therefore, the sequence Φκ convergence is the same as the infinite series ∑(Φκ−Φκ−1) convergence. The final PM solution takes the form
From the above relations, we can deduce that if the series ∑(Φκ−Φκ−1) is convergent, then the sequence Φκ(ϱ) is convergent to Φ(ϱ). To prove that the sequence {Φκ(ϱ)} is informally convergent, consider the associated series
From (4.7) for κ =1, we get
So, we have
Thus,
Now, we get an estimate for Φκ(ϱ)−Φκ−1(ϱ), κ ⩾2:
and
Thus,
From the hypotheses (ii) and (iii), we have
so,
where Υ1=[b2R0M1+M2bμ−η(LR0+M)Γ(μ−η+1)].
In the above relation, if we put κ =2 and use (4.13), we get
Doing the same for κ =3,4,⋯ gives us
Then the general form of this relation is
Since Υ1<1, then the series
is uniformly convergent. Hence, the sequence {Φκ(ϱ)} is uniformly convergent. Since ˆg1(ϱ,Φ(ϱ)),ˆg2(ϱ,Φ(ϱ)), and f1(ϱ,Φ(ϱ)) are continuous in Φ, then
Hence, the solution exists.
5.
Numerical examples
Example 1. For the HIE of Chandraseker type:
where
and its exact solution is Φ(ξ)=ξ2.
Applying the ADM to Eq (5.1), we get
Using the PM in Eq (5.1), the solution algorithm is
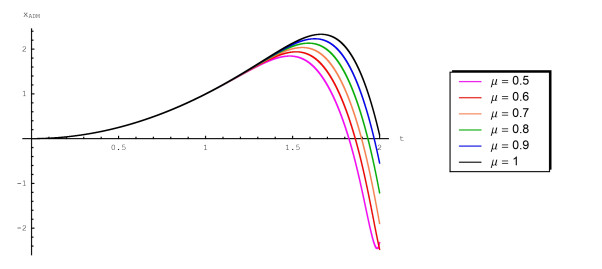
Figure 1 shows ADM solutions at different values of μ (μ=0.5, 0.6, 0.7, 0.8, 0.9, 1), and Figure 2 shows PM solutions at the same values.
Remark 1. A comparison between the absolute relative error (ARE) of ADM and PM solutions with the exact solution (where μ=0.5) is given in Table 1. It is clear from these results that the two solutions nearly give the same accuracy, but when a comparison is made between the time used in these two cases, it is found that the ADM takes less time than the PM (ADM time = 22 sec., PM time = 319.188 sec.). Figure 3(a) shows the ADM and the exact solution, while Figure 3(b) shows the PM with the exact solution.
Example 2. For the HIE of Chandraseker type:
where
and its exact solution is Φ(ξ)=ξ.
Applying the ADM to Eq (5.6), we get
Using the PM in Eq (5.6), we have
Figure 4(a) shows the ADM and exact solution, while Figure 4(b) shows the PM bwith the exact solution.
Remark 2. The absolute difference (AD) between ADM and PM solutions (where μ=0.9) is |ΦPM−ΦADM|=0 for ξ=0.2, 0.4, ...,2. It is clear from these results that the two solutions are nearly the same, but when a comparison is made between the time used in these two cases, it is found that the ADM takes less time than the PM (ADM time = 42 sec., PM time = 253.2 sec.). Figure 5 shows ADM and PM solutions at (μ=0.9).
Example 3. For the HIE of Chandraseker type:
where
and its exact solution is Φ(ξ)=ξ.
Applying the ADM to Eq (5.11), we have
Using the PM in Eq (5.11), we get
Remark 3. A comparison between the ARE of ADM and PM solutions with the exact solution is given in Table 2. It is clear from these results that the two solutions nearly give the same accuracy, but when a comparison is made between the time used in these two cases, it is found that the ADM takes less time than the PM (ADM time = 69.124 sec., PM time = 70.875 sec.). Figure 6 shows ADM solutions at different values of μ (μ=0.5, 0.6, 0.7, 0.8, 0.9, 1), and Figure 7 shows PM solutions at the same values.
6.
Conclusions
In this research, two analytical methods (ADM and PM) are used to solve the fractional CQIE that was found in the nonlinear analysis and its applications. The existence of a unique solution and its convergence to the two methods are proved (see Theorems 2, 4, and 5). This article focused on making a comparison between them with the exact solution (see the results in Tables 1 and 2). It is observed from the obtained results that the difference between their accuracy is too small to consider, but when we compare their used time, it was clear that the ADM takes less time than the PM (it is more clear in Example 1). These results showed that the two methods satisfied certain criteria that were provided by the solutions.
Use of AI tools declaration
The authors declare they have not used Artificial Intelligence (AI) tools in the creation of this article.
Acknowledgements
The authors would like to thank Princess Nourah bint Abdulrahman University Researchers Supporting Project number (PNURSP2024R406), Princess Nourah bint Abdulrahman University, Riyadh, Saudi Arabia.
Author contributions
Conceptualization, Eman A. A. Ziada and Hind Hashem; Formal analysis, Hind Hashem; Funding acquisition, Asma Al-Jaser; Investigation, Asma Al-Jaser and Osama Moaaz; Methodology, Eman A. A. Ziada and Monica Botros; Software, Asma Al-Jaser and Monica Botros; Writing—original draft, Eman A. A. Ziada and Monica Botros; Writing—review and editing, Osama Moaaz. All authors have read and agreed to the published version of the manuscript.
Conflict of interest
The authors declare there is no conflict of interest.









 DownLoad:
DownLoad:









