1.
Introduction
The theory of fixed points is considered to be the most delightful and energetic field of investigations in the development of mathematical analysis. In this scope, the notion of metric space [1] is one of pillars of not only mathematics but also physical sciences. Due to its noteworthy and remarkable contribution in different fields, it has been extended, improved and generalized in various ways.
In recent years, many interesting generalizations (or extensions) of the metric space concept appeared. The famous extensions of the concept of metric spaces have been done by Bakhtin [2] which was formally defined by Czerwik [3] in 1993. Czerwik [3] gave the idea of b -metric space which broaden the notion of metric space by improving the triangle equality metric axiom by putting a constant s≥1 multiplied to the right-hand side, is one of the enormous applied extensions for metric spaces. Khamsi et al. [4] reintroduced this notion under the name metric-type and proved some fixed point results in this newly introduced space. In [5], Branciari gave the notion of rectangular metric space and generalized the classical metric space by replacing the triangle inequality with more general inequality that is called rectangular inequality. This inequality involves distance of four points. In 2018, Jleli et al. [6] gave a compulsive extension of a metric space, b -metric space and rectangular metric space which is known as F -metric space. Later on, Al-Mazrooei et al. [7] utilized F -metric space and investigated some fixed point theorems for rational contraction which involves non-negative constants. For more details, we refer the researchers [8,9,10,11,12,13,14,15,16,17,18,19,20]
In this research article, we improve rational contraction of Al-Mazrooei et al. [7] by adding one more rational expression in it and replacing non-negative constants with control functions of two variables. We prove some common fixed point results which are generalizations of fixed point results in the context of F-metric spaces. As outcomes of our main results, we derive common fixed point theorems for rational contractions involving control functions of one variable. In this way, we derive the leading results of Jleli et al. [6] and Ahmad et al. [7]. We also establish some results in F-metric space equipped with a directed graph G. As an application, we investigate the solution to nonlinear neutral differential equation.
2.
Preliminaries
Let us recall some related material to be used to establish our main results. Recall that Czerwik [3] gave the notion of b-metric space as follows:
Definition 1. (see [3]) Let Θ≠∅ and s≥1 be a constant. A function ς:Θ×Θ→ [0,∞) is called a b-metric if the following assertions hold:
(b1) ς(ϱ,ℏ)≥0 and ς(ϱ,ℏ)=0 if and only if ϱ=ℏ;
(b2) ς(ϱ,ℏ)=ς(ℏ,ϱ);
(b3) ς(ϱ,φ)≤s[ς(ϱ,ℏ)+ς(ℏ,φ)];
for all ϱ,ℏ,φ∈Θ.
The pair (Θ,ς) is then said to be a b-metric space.
Jleli et al. [6] gave a fascinating extension of metric space and b -metric space as follows. Let F be a set of functions f:(0,+∞)→R satisfying
(F1) f is non-decreasing,
(F2) for each {ϱȷ}⊆R+, limȷ→∞ϱȷ=0 if and only if limȷ→∞f(ϱȷ)=−∞.
Definition 2. (see [6]) Let Θ≠∅ and ς:Θ×Θ→[0,+∞). Assume that there exists (f,h)∈F×[0,+∞) such that
(D1) (ϱ,ℏ)∈Θ×Θ, ς(ϱ,ℏ)=0 if and only if ϱ=ℏ,
(D2) ς(ϱ,ℏ)=ς(ℏ,ϱ), for all (ϱ,ℏ)∈Θ×Θ,
(D3) for every (ϱ,ℏ)∈Θ×Θ, for every N∈N, N≥2, and for every (ϱi)Ni=1⊂Θ, with
we have
Then (Θ,ς) is called a F-metric space.
Example 1. Let Θ=R and ς:Θ×Θ→[0,+∞) be defined by
with f(t)=ln(t) and h=ln(2), then (Θ,ς) is a F-metric space.
Definition 3. (see [6]) Let (Θ,ς) be F-metric space,
(i) a sequence {ϱȷ} in Θ is said to be F-convergent to ϱ∈Θ if {ϱȷ} is convergent to ϱ with respect to the F-metric ς;
(ii) a sequence {ϱȷ} is F-Cauchy, if
(iii) if every F-Cauchy sequence in Θ is F -convergent to a point of Θ, then (Θ,ς) is said to be a F-complete.
Theorem 1. (see [6]) Let (Θ,ς) be a F-complete F-metric space and ℜ:Θ→Θ. Assume that there exists α∈[0,1) such that
for all ϱ,ℏ∈Θ, then ℜ has a unique fixed point ϱ∗∈Θ. Moreover, for any ϱ0∈Θ, the sequence {ϱȷ}⊂Θ defined by
is F-convergent to ϱ∗.
Subsequently, Hussain et al. [14] defined α-ψ-contraction in the background of F-metric spaces and generalized the main result of Jleli et al. [6]. Later on, Ahmad et al. [7] defined a rational contraction in F-metric space and proved the following result as generalization of main theorem of Jleli et al. [6].
Theorem 2. (see [7]) Let (Θ,ς) be a F-complete F-metric space and ℜ:Θ→Θ. Assume that there exists α,β∈[0,1) such that
for all ϱ,ℏ∈Θ, then ℜ has a unique fixed point.
3.
Main result
We start this section with the following proposition which is helpful in proving our main result.
Proposition 1. Let (Θ,ς) be a F-metric space and ℜ1,ℜ2:(Θ,ς)→(Θ,ς). Let ϱ0∈ Θ. Define the sequence {ϱȷ} by
for all ȷ=0,1,2,....
Assume that there exist α:Θ×Θ→[0,1) satisfying
for all ϱ,ℏ∈Θ. Then
for all ϱ,ℏ∈Θ and ȷ=0,1,2,....
Proof. Let ϱ,ℏ∈Θ and ȷ=0,1,2,... Then we have
Similarly, we have
Hence, the proof is completed.
Lemma 1. Let (Θ,ς) be a F-metric space and α,β:Θ×Θ→[0,1). If ℜ1,ℜ2:Θ →Θ satisfy
and
for all ϱ,ℏ∈ Θ, then
and
Proof. Using the hypothesis, we have
Similarly, we have
Theorem 3. Let (Θ,ς) be a F-complete F-metric space and ℜ1,ℜ2:Θ →Θ. If there exist mappings α,β,γ:Θ×Θ→[0,1) such that
(a) α(ℜ2ℜ1ϱ,ℏ)≤α(ϱ,ℏ) and α(ϱ,ℜ1ℜ2ℏ)≤α(ϱ,ℏ)
β(ℜ2ℜ1ϱ,ℏ)≤β(ϱ,ℏ) and β(ϱ,ℜ1ℜ2ℏ)≤β(ϱ,ℏ)
γ(ℜ2ℜ1ϱ,ℏ)≤γ(ϱ,ℏ) and γ(ϱ,ℜ1ℜ2ℏ)≤γ(ϱ,ℏ),
(b) α(ϱ,ℏ)+β(ϱ,ℏ)+γ(ϱ,ℏ)<1,
(c)
for all ϱ,ℏ∈ Θ, then ℜ1 and ℜ2 have a unique common fixed point.
Proof. Let ϱ,ℏ∈Θ. From (3.2), we have
By Lemma 1, we get
Similarly, we have
By Lemma 1, we get
Let ϱ0 ∈Θ and the sequence {ϱȷ} be defined by (3.1). From Proposition 1, (3.3), (3.4) and for all ȷ=0,1,2,...
which implies that
Similarly, we have
which implies that
Let λ= α(ϱ0,ϱ1)1−β(ϱ0,ϱ1)<1. Then from (3.5) and (3.6), we have
for all ȷ∈N. Inductively, we can construct a sequence {ϱȷ} in Θ such that
for all ȷ∈N. Let (f,h)∈F×[0,+∞) be such that (D3) is satisfied. Let ϵ>0 be fixed. By (F2), there exists δ>0 such that
Hence, by (3.7), (F1) and (F2), we have
for m>ȷ≥ȷ(ϵ). Using (D3) and (3.8), we obtain ς(ϱȷ,ϱm)>0, m>ȷ≥ȷ(ϵ) implies
which yields by (F1) that ς(ϱȷ,ϱm)<ϵ, m>ȷ≥ȷ(ϵ). It shows that {ϱȷ} is F-Cauchy. As (Θ,ς) is F-complete, so there exists ϱ∗∈Θ such that {ϱȷ} is F -convergent to ϱ∗, i.e.,
Now, we show that ϱ∗ is fixed point of ℜ1. We contrary suppose that ς(ϱ∗,ℜ1ϱ∗)>0. Then from (3.2), (F1) and (D3), we have
Taking the limit as ȷ→∞ and using (F2) and (8), we have
which implies that ς(ϱ∗,ℜ1ϱ∗)=0, a contradiction. Thus ϱ∗=ℜ1ϱ∗. Now we prove that ϱ∗ is fixed point of ℜ2. Then from (3.2), (F1) and (D3), we have
Taking the limit as ȷ→∞ and using (F2) and (8), we have
which implies that ς(ϱ∗,ℜ1ϱ∗)=0, a contradiction. Thus ϱ∗=ℜ2ϱ∗.Thus ϱ∗ is a common fixed point of ℜ1 and ℜ2. Now we prove that ϱ∗ is unique. We suppose that
but ϱ∗≠ϱ/. Now from (3.2), we have
This implies that, we have
As α(ϱ∗,ϱ/)+γ(ϱ∗,ϱ/)<1, we have
Thus ϱ∗=ϱ/. Hence, the proof is completed.
Now, let us introduce the following example.
Example 2. Let Θ={Sȷ=2ȷ+1:ȷ∈N} be endowed with the F-metric
for all ϱ,ℏ∈Θ and f(t)=lnt. Then (Θ,ς) is an F-complete F-metric space. Define the mapping ℜ1,ℜ2:Θ→Θ by
and
Suppose that m≠ȷ, then
Thus all the assertions of Theorem 3 are satisfied with α:Θ×Θ→[0,1) defined by α(Sȷ,Sm)=e−1 and any β,γ:Θ×Θ→[0,1). Hence S1 is a unique common fixed point of ℜ1 and ℜ2.
Consequently, from Theorem 3, we have the following corollaries:
Corollary 1. Let (Θ,ς) be a F-complete F-metric space and ℜ1,ℜ2:Θ →Θ. If there exist mappings α,β:Θ×Θ→[0,1) such that
(a) α(ℜ2ℜ1ϱ,ℏ)≤α(ϱ,ℏ) and α(ϱ,ℜ1ℜ2ℏ)≤α(ϱ,ℏ),
β(ℜ2ℜ1ϱ,ℏ)≤β(ϱ,ℏ) and β(ϱ,ℜ1ℜ2ℏ)≤β(ϱ,ℏ);
(b) α(ϱ,ℏ)+β(ϱ,ℏ)<1;
(c)
for all ϱ,ℏ∈ Θ, then ℜ1 and ℜ2 have a unique common fixed point.
Proof. Setting γ:Θ×Θ→[0,1) by γ(ϱ,ℏ)=0 in Theorem 3.
Corollary 2. Let (Θ,ς) be a F-complete F-metric space and ℜ1,ℜ2:Θ→Θ. If there exist mappings α,γ:Θ×Θ→[0,1) such that
(a) α(ℜ2ℜ1ϱ,ℏ)≤α(ϱ,ℏ) and α(ϱ,ℜ1ℜ2ℏ)≤α(ϱ,ℏ),
γ(ℜ2ℜ1ϱ,ℏ)≤γ(ϱ,ℏ) and γ(ϱ,ℜ1ℜ2ℏ)≤γ(ϱ,ℏ);
(b) α(ϱ,ℏ)+γ(ϱ,ℏ)<1;
(c)
for all ϱ,ℏ∈ Θ, then ℜ1 and ℜ2 have a unique common fixed point.
Proof. Setting β:Θ×Θ→[0,1) by β(ϱ,ℏ)=0 in Theorem 3.
Corollary 3. Let (Θ,ς) be a F-complete F-metric space and ℜ1,ℜ2:Θ→Θ. If there exists a mapping α:Θ×Θ→[0,1) such that
(a) α(ℜ2ℜ1ϱ,ℏ)≤α(ϱ,ℏ) and α(ϱ,ℜ1ℜ2ℏ)≤α(ϱ,ℏ);
(b)
for all ϱ,ℏ∈ Θ, then ℜ1 and ℜ2 have a unique common fixed point.
Proof. Setting β,γ:Θ×Θ→[0,1) by β(ϱ,ℏ)=γ(ϱ,ℏ)=0 in Theorem 3.1.
Corollary 4. Let (Θ,ς) be a F -complete F-metric space and ℜ:Θ →Θ. If there exists mapping α:Θ×Θ→[0,1) such that
(a) α(ℜϱ,ℏ)≤α(ϱ,ℏ) and α(ϱ,ℜℏ)≤α(ϱ,ℏ);
(b)
for all ϱ,ℏ∈ Θ, then ℜ has a unique fixed point.
Corollary 5. Let (Θ,ς) be a F-complete F-metric space and ℜ:Θ →Θ. If there exist mappings α,β,γ:Θ×Θ→[0,1) such that
(a) α(ℜϱ,ℏ)≤α(ϱ,ℏ) and α(ϱ,ℜℏ)≤α(ϱ,ℏ),
β(ℜϱ,ℏ)≤β(ϱ,ℏ) and β(ϱ,ℜℏ)≤β(ϱ,ℏ),
γ(ℜϱ,ℏ)≤γ(ϱ,ℏ) and γ(ϱ,ℜℏ)≤γ(ϱ,ℏ);
(b) α(ϱ,ℏ)+β(ϱ,ℏ)+γ(ϱ,ℏ)<1;
(c)
for all ϱ,ℏ∈ Θ, then ℜ has a unique fixed point.
Proof. Setting ℜ1=ℜ2=ℜ in Theorem 3.
Corollary 6. Let (Θ,ς) be a F-complete F-metric space and ℜ:Θ →Θ. If there exist mappings α,β,γ:Θ×Θ→[0,1) such that
(a) α(ℜϱ,ℏ)≤α(ϱ,ℏ) and α(ϱ,ℜℏ)≤α(ϱ,ℏ),
β(ℜϱ,ℏ)≤β(ϱ,ℏ) and β(ϱ,ℜℏ)≤β(ϱ,ℏ),
γ(ℜϱ,ℏ)≤γ(ϱ,ℏ) and γ(ϱ,ℜℏ)≤γ(ϱ,ℏ);
(b) α(ϱ,ℏ)+β(ϱ,ℏ)+γ(ϱ,ℏ)<1;
(c)
for all ϱ,ℏ∈ Θ, then ℜ has a unique fixed point.
Proof. From the Corollary (5), we have ϱ∈Θ such that ℜnϱ=ϱ. Now from
which implies that
which is possible only whenever ς(ℜϱ,ϱ)=0. Thus ℜϱ=ϱ.
4.
Deduced results
Corollary 7. Let (Θ,ς) be a F-complete F-metric space and ℜ1,ℜ2:Θ →Θ. If there exist mappings α,β,γ:Θ→[0,1) such that
(a) α(ℜ2ℜ1ϱ)≤α(ϱ),
β(ℜ2ℜ1ϱ)≤β(ϱ),
γ(ℜ2ℜ1ϱ)≤γ(ϱ);
(b) α(ϱ)+β(ϱ)+γ(ϱ)<1;
(c)
for all ϱ,ℏ∈ Θ, then ℜ1 and ℜ2 have a unique common fixed point.
Proof. Define α,β,γ:Θ×Θ→[0,1) by
for all ϱ,ℏ∈Θ. Then for all ϱ,ℏ∈Θ, we have
(a) α(ℜ2ℜ1ϱ,ℏ)=α(ℜ2ℜ1ϱ)≤α(ϱ)=α(ϱ,ℏ) and α(ϱ,ℜ1ℜ2ℏ)=α(ϱ)=α(ϱ,ℏ),
β(ℜ2ℜ1ϱ,ℏ)=β(ℜ2ℜ1ϱ)≤β(ϱ)=β(ϱ,ℏ) and β(ϱ,ℜ1ℜ2ℏ)=β(ϱ)=β(ϱ,ℏ),
γ(ℜ2ℜ1ϱ,ℏ)=γ(ℜ2ℜ1ϱ)≤γ(ϱ)=γ(ϱ,ℏ) and γ(ϱ,ℜ1ℜ2ℏ)=γ(ϱ)=γ(ϱ,ℏ);
(b) α(ϱ,ℏ)+β(ϱ,ℏ)+γ(ϱ,ℏ)=α(ϱ)+β(ϱ)+γ(ϱ)<1;
(c)
(d) λ=α(ϱ0,ϱ1)1−β(ϱ0,ϱ1)=α(ϱ0)1−β(ϱ0)<1.
By Theorem 3, ℜ1 and ℜ2 have a unique common fixed point.
Corollary 8. Let ℜ1,ℜ2:Θ →Θ. If there exist α,β, γ∈[0,1) with α+β+γ<1 such that
for all ϱ,ℏ∈ Θ, then ℜ1 and ℜ2 have a unique common fixed point.
Proof. Taking α(⋅)=α, β(⋅)=β and γ(⋅)=γ in Corollary 7.
Corollary 9. Let (Θ,ς) be a F-complete F-metric space and ℜ1,ℜ2:Θ →Θ. If there exist α,β∈[0,1) with α+β<1 such that
for all ϱ,ℏ∈ Θ, then ℜ1 and ℜ2 have a unique common fixed point.
Proof. Taking γ=0 in Corollary 8.
Remark 1. If we set ℜ1=ℜ2=ℜ in the Corollary 9, the we get the main result of Al-Mazrooei et al. [7].
Corollary 10. Let (Θ,ς) be a F-complete F-metric space and ℜ1,ℜ2:Θ →Θ. If there exists α∈[0,1) such that
for all ϱ,ℏ∈ Θ, then ℜ1 and ℜ2 have a unique common fixed point.
Proof. Taking β=0 in Corollary 9.
Remark 2. If we set ℜ1=ℜ2=ℜ in the Corollary 10, the we get the main result of Samet et al. [6].
5.
Some results related to graphs
Let (Θ,ς) be an F-metric space and G be a directed graph. Let us represent by G−1 the graph generated from G by changing the direction of E(G). Hence,
Definition 4. An element ϱ∈ Θ is said to be a common fixed point of the pair (ℜ1,ℜ2), if ℜ1(ϱ)=ℜ2(ϱ)=ϱ. We denote by CFix(ℜ1,ℜ2), the family of all common fixed points of the pair (ℜ1,ℜ2), that is,
Definition 5. Let (Θ,ς) be an F-complete F-metric space equipped with a directed graph G and let ℜ1,ℜ2 :Θ→Θ. Then the pair (ℜ1,ℜ2) is called a G-orbital cyclic pair, if
for any ϱ∈ Θ. Let us consider the following sets
Remark 3. If (ℜ1,ℜ2) is a G-orbital-cyclic pair, then Θℜ1≠∅⟺Θℜ2≠∅.
Proof. Let ϱ0∈Θℜ1. Then (ϱ0,ℜ1ϱ0)∈E(G)⟹(ℜ1ϱ0,ℜ2(ℜ1ϱ0))∈E(G). If we represent by ϱ1=ℜ1ϱ0, then we get that (ϱ1,ℜ2(ϱ1))∈E(G), thus Θℜ2≠∅. Now let us prove the following main theorem.
Theorem 4. Let (Θ,ς) be a F-complete F-metric space equipped with a directed graph G and ℜ1,ℜ2:Θ→Θ is G-orbital cyclic pair. Assume that there exists α∈[0,1) such that
(ⅰ) Θℜ1≠∅,
(ⅱ) ∀ ϱ∈Θℜ1 and ℏ∈Θℜ2
(iii) ℜ1 and ℜ2 are continuous, or ∀ (ϱȷ)ȷ∈N⊂Θ, with ϱȷ→ϱ as  , and (ϱȷ,ϱȷ+1)∈E(G) for
, and (ϱȷ,ϱȷ+1)∈E(G) for  , we have ϱ∈Θℜ1∩Θℜ2. In these conditions CFix(ℜ1,ℜ2)≠∅,
, we have ϱ∈Θℜ1∩Θℜ2. In these conditions CFix(ℜ1,ℜ2)≠∅,
(iv) if (ϱ∗,ϱ/)∈CFix(ℜ1,ℜ2) implies ϱ∗∈Θℜ1 and ϱ/∈Θℜ2, then the pair (ℜ1,ℜ2) has a unique common fixed point.
Proof. Let ϱ0∈Θℜ1. Thus (ϱ0,ℜ1ϱ0)∈E(G). As the pair (ℜ1,ℜ2) is G-orbital cyclic, we get (ℜ1ϱ0,ℜ2ℜ1ϱ0)∈E(G). Construct ϱ1 by ϱ1=ℜ1ϱ0, we have (ϱ1,ℜ2ϱ1)∈E(G) and from here (ℜ2ϱ1,ℜ1ℜ2ϱ1)∈E(G). Denoting by ϱ2=ℜ2ϱ1, we have (ϱ2,ℜ1ϱ2)∈E(G). Pursuing along these lines, we generate a sequence (ϱȷ)ȷ∈N with ϱ2ȷ=ℜ2ϱ2ȷ−1 and ϱ2ȷ+1=ℜ1ϱ2ȷ, such that (ϱ2ȷ,ϱ2ȷ+1)∈E(G). We assume that ϱȷ≠ϱȷ+1. If, there exists  ∈N, such that ϱȷ0=ϱȷ0+1, then in the view of the fact that Δ⊂E(G), (ϱȷ0,ϱȷ0+1)∈E(G) and thus ϱ∗=ϱȷ0 is a fixed point of ℜ1. Now to manifest that ϱ∗∈CFix(ℜ1,ℜ2), we shall discuss these two cases for
∈N, such that ϱȷ0=ϱȷ0+1, then in the view of the fact that Δ⊂E(G), (ϱȷ0,ϱȷ0+1)∈E(G) and thus ϱ∗=ϱȷ0 is a fixed point of ℜ1. Now to manifest that ϱ∗∈CFix(ℜ1,ℜ2), we shall discuss these two cases for  . If
. If  is even, then
is even, then 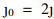 . Then, ϱ2ȷ=ϱ2ȷ+1=ℜ1ϱ2ȷ and thus, ϱ2ȷ is a fixed point of ℜ1. Assume that ϱ2ȷ=ϱ2ȷ+1=ℜ1ϱ2ȷ but ς(ℜ1ϱ2ȷ,ℜ2ϱ2ȷ+1)>0, and let ϱ=ϱ2ȷ∈Θℜ1 and ℏ=ϱ2ȷ+1∈Θℜ2. So
. Then, ϱ2ȷ=ϱ2ȷ+1=ℜ1ϱ2ȷ and thus, ϱ2ȷ is a fixed point of ℜ1. Assume that ϱ2ȷ=ϱ2ȷ+1=ℜ1ϱ2ȷ but ς(ℜ1ϱ2ȷ,ℜ2ϱ2ȷ+1)>0, and let ϱ=ϱ2ȷ∈Θℜ1 and ℏ=ϱ2ȷ+1∈Θℜ2. So
that is contradiction because α<1. Hence ϱ2ȷ is a fixed point of ℜ2 too. Likewise if 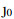 is odd number, then ∃ϱ∗∈Θ such that ℜ1ϱ∗∩ℜ2ϱ∗=ϱ∗. So we assume that ϱȷ≠ϱȷ+1 for all
is odd number, then ∃ϱ∗∈Θ such that ℜ1ϱ∗∩ℜ2ϱ∗=ϱ∗. So we assume that ϱȷ≠ϱȷ+1 for all 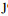 ∈N. Now we shall show that (ϱȷ)ȷ∈N is Cauchy sequence. We have these two possible cases to discuss:
∈N. Now we shall show that (ϱȷ)ȷ∈N is Cauchy sequence. We have these two possible cases to discuss:
Case 1. ϱ=ϱ2ȷ∈Θℜ1 and ℏ=ϱ2ȷ+1∈Θℜ2.
that is
which implies
Case 2. ϱ=ϱ2ȷ∈Θℜ1 and ℏ=ϱ2ȷ−1∈Θℜ2.
that is
which implies
Since τ=α1−α, so we have
Thus, we have
Let (f,h)∈F×[0,+∞) be such that (D3) is satisfied. Let ϵ>0 be fixed. By (F2), ∃δ>0 such that
Hence, by (5.6), (F1) and (F2), we have
for 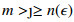 . By (D3) and (5.7), we get ς(ϱȷ,ϱm)>0,
. By (D3) and (5.7), we get ς(ϱȷ,ϱm)>0, 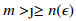 implies
implies
which yields by (F1) that ς(ϱȷ,ϱm)<ϵ, 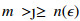 . It shows that {ϱȷ} is F-Cauchy. As (Θ,ς) is F-complete, so ∃ϱ∗∈Θ such that {ϱȷ} is F-convergent to ϱ∗, i.e.,
. It shows that {ϱȷ} is F-Cauchy. As (Θ,ς) is F-complete, so ∃ϱ∗∈Θ such that {ϱȷ} is F-convergent to ϱ∗, i.e.,
that is ϱȷ→ϱ∗ as 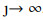 . It is obvious that
. It is obvious that
As ℜ1 and ℜ2 are continuous, so we have
Now letting ϱ=ϱ∗∈Θℜ1 and ℏ=ϱ2ȷ+2∈Θℜ2, we have We contrary suppose that ς(ϱ∗,ℜ1ϱ∗)>0. Then from (3.2), (F1) and (D3), we have
Taking 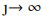 and using (F2), (5.10) and (5.11), we get
and using (F2), (5.10) and (5.11), we get
which is a contradiction. Thus, we have ς(ϱ∗,ℜ1ϱ∗)=0. This yields that ϱ∗=ℜ1ϱ∗. Similarly, suppose that ϱ=ϱ2ȷ+1∈Θℜ1 and ℏ=ϱ∗∈Θℜ2, we have
Taking the limit as 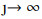 and using (F2), (5.10) and (5.11), we have
and using (F2), (5.10) and (5.11), we have
which is a contradiction. Thus, we have ς(ϱ∗,ℜ2ϱ∗)=0. This yields that ϱ∗=ℜ2ϱ∗.
Corollary 11. Let (Θ,ς) be a F-complete F-metric space equipped with a directed graph G and ℜ:Θ→Θ is a G-orbital-cyclic. Suppose that there exists α∈[0,1) such that
(i) Θℜ≠∅,
(ii) ∀ ϱ,ℏ∈Θℜ, we have
(iii) ℜ is continuous, or ∀ (ϱȷ)ȷ∈N⊂Θ, with ϱȷ→ϱ as ȷ→∞, and (ϱȷ,ϱȷ+1)∈E(G) for ȷ∈N, we have ϱ∈Θℜ.
Then ℜ has a unique fixed point.
6.
Applications in differential equations
A representative stability result based on fixed point theory arguments follows a number of basic arguments adapted to the special structure of the equation under consideration. It leads to large number of results in the literature for different classes of equations, see [21,22]. In the present section, we investigate the existence of solution to differential equation
We state a lemma of Djoudi et al.[23] which will be used in proving of our theorem.
Lemma 2. (see [23]) Assume that r/(t)≠1 ∀t∈R. Then ϱ(t) is a solution of (6.1) if and only if
where
Now suppose that ϑ:(−∞,0]→R is a bounded and continuous function, then ϱ(t)=ϱ(t,0,ϑ) is a solution of (6.1) if ϱ(t)=ϑ(t) for t≤0 and satisfies (6.1) for t≥0. Assume that C is the collection of ϱ:R→R which are continuous. Define ℵϑ by
Then ℵϑ is a Banach space endowed with ‖⋅‖.
Lemma 3. (see [14]) The space (ℵϑ,∥⋅∥) with the F-metric d defined by
for all t, t^{\ast }\in \aleph _{\vartheta }, is \mathcal{F} -metric space.
Theorem 5. Let \Re :\aleph _{\vartheta }\rightarrow \aleph _{\vartheta } be a mapping defined by
for all \varrho \in \aleph _{\vartheta } . Assume that there exists \alpha :\aleph _{\vartheta }\times \aleph _{\vartheta }\mathfrak{\rightarrow }[0, 1) such that
Then \Re has a fixed point.
Proof. It follows from (6.3) that \Re (\varrho), \Re (\hbar)\in \aleph _{\vartheta } . Now from (6.4), we have
Hence,
Thus all the assumptions of Corollary 4 are satisfied and \Re has a unique fixed point in \aleph _{\vartheta } which solves (6.1).
Open Problems
1) Can the notion of \mathcal{F} -metric space be extended to graphical \mathcal{F} -metric space?
2) Can the results proved in this article be extended to multivalued mappings and fuzzy set valued mappings?
3) Can differential inclusions can be solved as applications of fixed point results for multivalued mappings in the context of \mathcal{F} -metric space?
7.
Conclusion
In this article, we have utilized the notion of \mathcal{F} -metric spaces and obtained common fixed point results for generalized rational contractions involving control functions of two variables. We have derived common fixed points and fixed points of single valued mappings for contractions involving control functions of one variable and constants. We also have established some common fixed point theorems in \mathcal{F} -metric spaces endowed with graph. We expect that the obtained theorems in this article will make new relations for those people who are employing in \mathcal{F} -metric spaces.
Acknowledgments
The second author Ahmad Al-Rawashdeh is financially supported by the Grants: UPAR-2019, Fund No. 31S397, and the Post-Doc-2019, Fund No. 31S404.
Conflict of interest
The authors declare that they have no conflicts of interest.









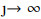 , and (
, and (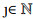 , we have
, we have 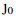
 . If
. If 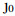 is even, then
is even, then 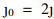 . Then,
. Then, 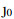 is odd number, then
is odd number, then 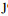
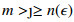 . By (D
. By (D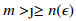 implies
implies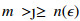 . It shows that
. It shows that 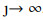 . It is obvious that
. It is obvious that and using (
and using ( and using (
and using (



 DownLoad:
DownLoad: