On geometry of isophote curves in Galilean space

1.   Introduction

The isophote curve method is one of the most efficient methods that can be used to analyze and visualize surfaces by lines of equal light intensity. Isophote curve whose normal vectors make a constant angle with a fixed vector (the axis) is one of the curves to characterize surfaces such as parameter, geodesics, and asymptotic curves or lines of curvature. Moreover, this curve is used in computer graphics and it is also interesting to study for geometry.

The isophote curve of a given surface is calculated in two steps: firstly the normal vector field $n(s, t)$ of the surface is computed, and secondly the surface point is traced as

where $\beta$ is a constant angle ($0\leq \beta \leq \frac{\pi }{2}$).

Isophote curve is called a silhouette curve when

where $d$ is the unit fixed vector.

From past to present, there have been a lot of researches about isophote curves and their characterizations in ^[3,4,6,7].

In this paper, our aim is to investigate isophote curves on surfaces in Galilean space and find its axis $d$ such that it is an isotropic and a non-isotropic vector through the Galilean Darboux frame. According to the axis $d$, we split our studies into two cases to find the axis of isophote curves lying on a surface in Galilean space. Moreover, we give a method to compute isophote curves of surfaces of revolution obtained by revolving a curve by Euclidean and isotropic rotations.

2.   Preliminaries

Following the Erlangen Program, due to F. Klein, each geometry is associated with a group of transformations, and hence there are as many geometries as groups of transformations. Associated with group of transformations that in physics guarantees the invariance of many mechanical systems, the Galilei group, is the so-called Galilean geometry ${G}_{3}$. That is, Galilean geometry is one of the nine Cayley-Klein geometries with projective signature $(0, 0, +, +)$. The absolute of the Galilean geometry is an ordered triple $\{ \omega, f, I \}$, where $\omega$ is the ideal (absolute) plane, $f$ the line in $\omega$ and $I$ the fixed elliptic involution of $f$ as in ^[11].

A plane is called Euclidean if it contains $f$, otherwise it is called isotropic or i.e., plane $x$ = constant is Euclidean, and so is the plane $\omega$. Other planes are isotropic. In other words, an isotropic plane does not involve any isotropic direction. A vector $u = (u_{1}, u_{2}, u_{3})$ is called non-isotropic if $u_{1}\neq0$, otherwise it is called isotropic vector. All unit non-isotropic vectors are of the form $u = (1, u_{2}, u_{3})$ ^[11].

A Galilean scalar product of two vectors $u = (u_{1}, u_{2}, u_{3})$ and $v = (v_{1}, v_{2}, v_{3})$ in the Galilean 3-space $G_{3}$ is defined as

and a Galilean norm of $u$ is given by

A Galilean cross product of $u$ and $v$ on $G_{3}$ is defined by

where $e_{2} = (0, 1, 0)$ and $e_{3} = (0, 0, 1)$ ^[8,10].

A curve given in parametric form $\alpha (t) = \left(f(t), g(t), h(t)\right)$ in the Galilean 3-space $G_{3}$ is called admissible if nowhere its tangent vector is isotropic, that is $f^{\prime }(t) = \frac{df}{dt}\neq 0.$

Let $\alpha$ be a unit-speed admissible curve of the class ${C}^{\infty }$ in ${G}_{3},$ and parametrized by the invariant parameter $s$, defined by

Then the Frenet frame fields of $\alpha (s)$ are given by

where the curvature $\kappa (s)$ and the torsion $\tau (s)$ of $\alpha (s)$ are written as, respectively,

Here $T,$ $N$ and $B$ are the tangent, principal normal and binormal vectors of $\alpha (s)$.

On the other hand, the Frenet formula of the curve is given by (cf. ^[9])

Consider a $C^{r}$-regular surface $M$, $r\geq 1$, in $G_{3}$ parameterized by

We denote by $x_{u_{i}}$, $y_{u_{i}}$ and $z_{u_{i}}$ the partial derivatives of the functions $x$, $y$ and $z$ with respect to $u_{i}$ ($i = 1, 2$), respectively. Besides $\mathbf{X}$ is said to be admissible if nowhere it has Euclidean tangent planes, i.e., $x_{u_{i}}\neq0$ for some $i = 1, 2$.

On the other hand, the matrix of the first fundamental form $ds^{2}$ of a surface $M$ in $G_{3}$ is given by

where $ds_{1}^{2} = (g_{1}du_{1}+g_{2}du_{2})^{2}$ and $ds_{2}^{2} = h_{11}du_{1}^{2}+2h_{12}du_{1}du_{2}+h_{22}du_{2}^{2}$. Here $g_{i} = x_{u_{i}}$ and $h_{ij} = \langle \tilde{\mathbf{X}}_{u_{i}}, \tilde{ \mathbf{X}}_{u_{j}}\rangle$ $(i, j = 1, 2)$ means the Euclidean scalar product of the projections $\tilde{\mathbf{X}}_{u_{i}}$ of vectors $\mathbf{X} _{u_{i}}$ onto the $yz$-plane.

The unit normal vector field $n$ of a surface $M$ is defined by

where the positive function $\omega$ is given by

Let $\{T, Q, n\}$ be a Galilean Darboux frame of $\alpha (s)$ with $T$ as the tangent vector of a curve $\alpha (s)$ in $G_{3}$ and $n$ be the unit normal to a surface and $Q = n\times T$. Then the Galilean Darboux frame is expressed as

where $k_{g}$, $k_{n}$ and $\tau _{g}$ are the geodesic curvature, normal curvature and geodesic torsion of $\alpha (s)$ on $M$, respectively. Also, (2.2) implies

where $\phi$ is an angle between the surface normal vector $n$ and the binormal vector $B$ of $\alpha$ ^[12]. A curve $\alpha (s)$ is a geodesic (an asymptotic curve or a line of curvature) if and only if $k_{g}$ ($k_{n}$ or $\tau _{g}$) vanishes, respectively.

On the other hand, the usual transformation between the Galilean Frenet frames and the Darboux frames takes the form

Artykbaev introduced an angle between two vectors in Galilean space as follows:

Definition 2.1. (^[1]) Let $x = (1, x_{2}, x_{3})$ and $y = (1, y_{2}, y_{3})$ be two unit non-isotropic vectors in $G{_{3}}$. Then an angle $\vartheta$ between $x$ and $y$ is defined by

Definition 2.2. (^[1]) An angle between a unit non-isotropic vector $x = (1, x_{2}, x_{3})$ and an isotropic vector $y = (0, y_{2}, y_{3})$ in $G{_{3}}$ is defined by

Definition 2.3. (^[1]) An angle $\theta$ between two isotropic vectors $x = (0, x_{2}, x_{3})$ and $y = (0, y_{2}, y_{3})$ parallel to the Euclidean plane in $G{_{3}}$ is equal to the Euclidean angle between them. That is,

3.   The axis of an isophote curve in Galilean space

The starting point of this section is to get the unit fixed vector $d$ of an isophote curve via its Galilean Darboux frame.

Let $M$ be an admissible regular surface and $\alpha :I\subset R\rightarrow M$ be an unit-speed curve parametrized by $\alpha(s) = (s, \alpha_2(s), \alpha_3(s))$ as an isophote curve for some $s \in I$.

In order to prove the results, we split it into two cases according to the fixed vector $d$.

Case 1. Considering $d$ is a unit fixed isotropic vector.

Since $n$ is the unit isotropic normal vector of a surface $M$, using the Definition 2.3, we have

differentiating the above equation, we get

That is, $\tau _{g} = 0$ or $\langle Q, d\rangle = 0$. By differentiating $\langle Q, d\rangle = 0,$ we get

It means that $\tau _{g} = 0.$ So

On the other hand, if $k_{g}\neq 0,$ using the differentiating $\langle T, d\rangle = 0$ with respect to $s$, we get

Then $d$ can be written as

Since $\left\Vert d\right\Vert = 1$, we get

In this situation, we conclude that $\phi = \pm \theta$ or $\phi = \pi \pm \theta.$

From (2.3) and (2.4) in terms of the Galilean Frenet frame, we get

If we differentiate (3.6) using (3.3) and (3.3), we get $d^{\prime } = 0,$ that is, $d$ is a constant isotropic vector. From now on, we suppose if $\alpha$ is a unit-speed isophote curve, then $\alpha$ is also a line of curvature.

Theorem 3.1. Let $\alpha$ be a unit-speed isophote curve on a surface $M$ in $G_3$ with a unit fixed isotropic vector $d$ as the axis of the isophote curve. In that case, we have the following:

$i)$ If $\alpha$ is a geodesic curve, then $\alpha$ is a straight line.

$ii)$ If $\alpha$ is an asymptotic curve on $M$, then it is a plane curve, and the fixed vector $d$ is parallel to $B.$

Proof. $i)$ If $k_{g} = 0$, then $\tau = -\tau _{g} = 0$ from (2.3) and (3.3). Also from (3.4), $k_{n} = 0$. Then from (2.3), we get $\kappa = 0$. Hence $\alpha$ is a straight line.

$ii)$ If $\alpha$ is an asymptotic curve, then $k_{n} = 0.$ Trivially, if $k_{g} = 0$, it is trivial that $\alpha$ is a plane curve. If $k_{g}\neq0$, then from (3.6), we get

So from (2.3), $\tau = 0$. That is, $\alpha$ is a plane curve. Also using (2.3) and (2.4) into (3.6), it can be easily shown that $d$ is parallel to $B.$

Theorem 3.2. Let $\alpha$ be a unit-speed isophote curve on a surface $M$ in $G_3$ with a unit fixed isotropic vector $d$ as the axis of the isophote curve. The axis $d$ is perpendicular to the principal normal line of $\alpha$ if and only if either $\alpha$ is a straight line or an asymptotic curve on $M$ or $\alpha$ is a curve with $\frac{k_{n}}{k_{g}} = -\tan \theta.$

Proof. If $\alpha$ is a unit-speed isophote curve, then from (3.8), we get

from this equation, we have $k_{g} = 0$ or $\sin \theta = 0$.

If $k_{g} = 0$ then, from Theorem 3.1, $\alpha$ is a straight line.

If $\sin \theta = 0$, then $k_{n} = 0,$ that is, $\alpha$ is an asymptotic curve.

If we take $\frac{k_{n}}{k_{g}} = -\tan \theta$, then we can easily get $\langle N, d\rangle = 0.$

Theorem 3.3. Let $\alpha$ be a unit-speed isophote curve on a surface $M$ in $G_3$ with a unit fixed isotropic vector $d$ as the axis of the isophote curve. The axis $d$ is perpendicular to the principal binormal line of $\alpha$ such that $\dfrac{k_{n}}{k_{g}} = \tan \theta$ if and only if $k_{n} = k_{g}$

Proof. If $\alpha$ is a unit-speed isophote curve with $\frac{k_{n}}{k_{g} } = \tan \theta$, then from (3.8), we get

Since $\alpha$ is a non-geodesic curve, $-\sin ^{2}\theta +\cos ^{2}\theta = 0.$ So, $\tan \theta = 1$. We know that $0\leq\theta \leq \dfrac{\pi }{2},$ then we get $\theta = \dfrac{\pi }{4}.$

Theorem 3.4. If $\alpha$ is a silhouette curve on $M,$ and $d$ is a unit isotropic vector such that it is parallel to $Q$, then the curve $\alpha$ is a plane curve.

Proof. If a fixed vector $d$ is a unit isotropic vector and is parallel to $Q$, then we have

By differentiating above equations with respect to $s$, we obtain

Since $\alpha$ is a silhouette curve with $\langle n, d\rangle = 0$, we get

from this, we have $\tau = 0.$ It means that $\alpha$ is a plane curve.

Case 2. Considering $d$ is a unit fixed non-isotropic vector.

Since $n$ is the unit isotropic normal vector of a surface $M$, using the Definition 2.2, we have

where $0\leq \varphi \leq 1$.

By differentiating the above equation, we get

That is, $\tau _{g} = 0$ or $\langle Q, d\rangle = 0$. By differentiating $\langle Q, d\rangle = 0,$ we get

That means $\tau _{g} = 0.$ So

On the other hand, if $k_{g}\neq 0,$ using the differentiating $\langle T, d\rangle = 1$ with respect to $s$, we get

Then the unit non-isotropic vector $d$ can be written as

Since $d$ is a constant vector, we get $k_{g} = k_{n} = 0.$ Trivially, it is a conflict.

On the other hand considering $k_{n} = 0,$ from (3.13) and using (3.12), $d$ can be written as

Since $d$ is a constant vector, we can easily get$\ k_{g} = 0.$

Thus, we have the following result:

Theorem 3.5. Let $\alpha$ be a unit-speed isophote curve on a surface $M$ in $G_{3}$ with a unit fixed non-isotropic vector $d$ as the axis of the isophote curve. Then $\alpha$ is a straight line.

Theorem 3.6. Let $\alpha$ be a silhouette curve on $M$ and $d$ be a unit non-isotropic vector.

$i)$ If $d$ lies in the plane spanned by $T$ and $Q$, then $\alpha$ is a plane curve. $ii)$ If the axis $d$ is parallel to $T$, then $\alpha$ is a geodesic curve.

Proof. $i)$ Since $\alpha$ is a silhouette curve and $d$ is a unit non-isotropic vector, we get

If we differentiate (3.16) with respect to $s$, then we get

Since $d$ is lied in the plane spanned by $T$ and $Q,$ we get $k_{g} = 0$. Also, if we differentiate $\langle n, d\rangle = 0$ with respect to $s$, we get

it follows that $\tau _{g} = 0.$

Also, by substituting $\tau _{g} = 0$ and $k_{g} = 0$ into (2.3), we get $\tau = 0.$ Thus, $\alpha$ is a plane curve.

$ii)$ If $d$ is parallel to $T$, then we get

If we differentiate the above equation, then $d^{\prime } = k_{g}Q$, it follows that $k_{g} = 0$, that is, the curve is a geodesic curve.

4.   Applications for isophote curves

We investigate an isophote curve among surfaces of revolution in Galilean space and give some characterization for isophote curves on these surfaces. To see this, notice that in $G_{3}$ surfaces of revolution are obtained by revolving a curve by Euclidean or isotropic rotations as follows, respectively,

where $t$ is the Euclidean angle and

where $t\in \mathbb{R}$ and $c = \text{constant} > 0$.

The trajectory of a single point under a Euclidean rotation is a Euclidean circle

The invariant $r$ is the radius of the circle.

The trajectory of a point under isotropic rotation is an isotropic circle whose normal form is

The invariant $c$ is the radius of the circle. The fixed line of the isotropic rotation is the absolute line $f\ $^[11]. For some more studies, see ^[2,5].

If a curve $\alpha (s) = (f(s), 0, g(s)),$ $\left(g(s) > 0\right)$ is rotated by Euclidean rotations, then a surface of revolution is parametrized by

If a curve $\alpha (s)$ is parametrized by the arc-length, then we take $f(s) = s.$ In this case, the unit isotropic normal vector field $n(s, t)$ of $S$ is defined by

where $S_{s}$ and $S_{t}$ are the partial differentiations with respect to $s$ and $t,$ respectively. Then, the isotropic normal vector is given by

it becomes in terms of the Frenet frame as follows:

Proposition 4.1. Let $\alpha (s)$ be a general helix with the isotropic axis $d$. Then, for $t_{0} = (\frac{2k+1}{2})\pi$ $(k\in \mathbb{Z}),$ the curve $\alpha (s)$ on surfaces of revolution given by (4.3) is an isophote curve with the axis $d$.

Proof. Substituting $t_{0}$ into (4.5), we get

If $\alpha(s)$ is a general helix with the axis $d$, then $\langle B, d\rangle =$constant. Therefore, we get

Thus $\alpha(s)$ is an isophote curve with the axis $d$ on the surfaces of revolution.

Proposition 4.2. Let $\alpha (s)$ be a slant helix with the isotropic axis $d$. Then, for $t_{0} = k\pi$ $(k\in \mathbb{Z}),$ the curve $\alpha (s)$ on surfaces of revolution given by (4.3) is an isophote curve with the axis $d$.

Proof. Substituting $t_{0}$ into (4.5), we get

If $\alpha(s)$ is a slant helix with the axis $d$, then $\langle N, d\rangle =$constant. Therefore, we get

Thus $\alpha(s)$ is an isophote curve with the axis $d$ on the surfaces of revolution.

If a curve $\alpha (s) = (f(s), 0, g(s)),$ $\left(g(s) > 0\right)$ is rotated by isotropic rotations, then a surface of revolution is parametrized by

If a curve $\alpha (s)$ is parametrized by the arc-length, then we take $f(s) = s.$ In this case, the isotropic surface normal is given by

it becomes in terms of the Frenet frame as follows:

Theorem 4.3. Let $d$ be an isotropic axis given by (0, $\mathit{d}_{y}, \mathit{d}_{z}$).

$i)$ If $\mathit{d}_{y} = 0$ and $g(s) = \frac{s^{2}}{2c}+b_{1},$ ($b_{1}\in \mathit{ \mathbb{R} })$, then the curve $\alpha (s)$ on surfaces of revolution given by (4.6) is an isophote curve.

$ii)$ If $\mathit{d}_{z} = 0$ and $g(s) = \frac{s^{2}}{2c}+b_{1},$ ($b_{1}\in \mathit{ \mathbb{R} })$, then the curve $\alpha (s)$ on surfaces of revolution given by (4.6) is an isophote curve.

Proof. $i)$ If $\mathit{d}_{y} = 0,$ then we get $d = \lambda_1N, (\lambda_1\in \mathbb{R} -\{0\}$, it folllows that (4.7) implies

If we take $g(s) = \frac{s^{2}}{2c}+b_{1},$ $(b_{1}\in \mathbb{R}),$ then we obtain $\langle n, d\rangle = \frac{\lambda_1}{\sqrt2}.$ So the curve $\alpha$ is an isophote curve.

$ii)$ If $\mathit{d}_{z} = 0,$ then we get $d = -\lambda_2B, (\lambda_2\in \mathbb{R} -\{0\}).$ From (4.7) we get

If we consider $g(s) = \frac{s^{2}}{2c}+b_{1},$ $(b_{1}\in \mathbb{R})$, then we obtain $\langle n, d\rangle = \frac{\lambda_2}{\sqrt2},$ It means that $\alpha$ is an isophote curve.

Corollary 4.4. The generating curve $\alpha (s) = (f(s), 0, g(s))$ on surfaces of revolution given by (4.5) becomes both a general helix and a slant helix when the axis $d = (0, \mathit{d}_{y}, d_{z})$.

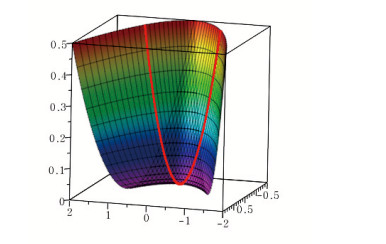
Remark 4.5. The isophote curve $\alpha(s)$ in Theorem 4.3 is an isotropic circle on surfaces of revolution given by (4.5). Figure 1 is shown an isophote curve on the isotropic surface of revolution with $c = 1$ and $b_1 = 0$ in Theorem 4.3 ii).

5.   Conclusion

In this paper, we investigated isophote curves on surfaces in Galilean space ${G}_{3}$ and obtained its axis $d$ such that it is an isotropic and a non-isotropic vector. Furthermore, we presented some characterizations for isophote curves lying on surfaces of revolution.

Acknowledgments

The second author was supported by Basic Science Research Program through the National Research Foundation of Korea (NRF) funded by the Ministry of Education (NRF-2018R1D1A1B07046979).

Conflict of interest

The authors declare no conflicts of interest in this paper.