Normalized solutions to nonautonomous Kirchhoff equation

1.   Introduction

In the past four decades, Hopfield neural networks (HNNs), were firstly proposed by Hopfield ^[1,2], have drawn much attention and in-depth research since their potential applications in many fields such as object recognition ^[3], signal and image processing ^[4], mathematical programming ^[5], etc. It is widely recognized that these engineering applications rely heavily on the dynamic behaviors of neural networks. Therefore, the study of their dynamics became a hot research topic and significant progress has been made in recent years, see ^[6,7,8] and the references therein.

In the hardware implementation of neural networks, however, time delays are inevitable since the finite switching speed of amplifiers, and a neural network has much more complicated dynamics due to the incorporation of time delays. For example, it has been shown that oscillation and bifurcation phenomena will appear when a discrete delay was introduced into HNNs ^[9,10], which are of great significance to practical applications of neural networks. Particularly, in a real nervous system, there perpetually encounters a representative time delay, which is essentially different from the conventional delays, named leakage delay, and it broadly exists in the negative feedback terms of the system which are identified as leakage terms, the leakage delay is usually incorporated in the study of network modeling, such a type of time delay often has a tendency to destabilize the neural networks and is difficult to handle ^[11]. Nevertheless, just as the traditional time delays, the leakage delay also has great influence on the dynamics of many different kinds of NNs, see, e.g., ^{[12,13,14,15,16]}. On the other hand, diffusion phenomenon inevitably appears in NNs and electric circuits once electrons transmit in a inhomogeneous electromagnetic field, which means that the whole structure and dynamic behavior of NNs are not only dependent on the time evolution but also on the spatial location ^[17,18]. Therefore, it is of great importance and significance to investigate the dynamics of leakage and discrete delayed HNNs with diffusion effects.

Synchronization, as an important class of neurodynamics, has been extensively studied since its potential applications in such as secure communication ^[19], image processing ^[20] and so on. Among many control strategies in practical applications, finite-time and fixed-time control is an important and effective tool to realize synchronization of network systems, and it is shown that such a control technique has better robustness and disturbance rejection properties ^[22]. Because of these merits, the finite-/fixed-time synchronization problem of kinds of neural networks has been deeply studied in recent years (see for example, ^{[23,24,25,26,27,28,29,30]} and the references therein). It is notable, however, that few works have considered simultaneously the leakage delay, discrete delay and diffusion effects in FFTS dynamics of neural networks under consideration.

Motivated by the above discussions, we study the FFTS problem of leakage and discrete delayed HNNs with diffusion effects. Firstly, based on the finite-/fixed-time convergence theorem, a novel negative exponential state feedback controller is designed. Then, by applying the inequality technique, and Lyapunov-Krasovskii functional method, some new sufficient conditions are established to realize the FFTS for the considered network model. Finally, a numerical example is given to verify the theoretical results established in this paper.

The plan of the paper is organized as follows. In Section 2, the model description and some necessary preliminaries are presented. Section 3 is devoted to designing a negative exponential controller and the FFTS criteria are established. In Section 4, a numerical example is given to illustrate the effectiveness of the obtained results. Finally, a brief conclusion is drawn in Section 5.

2.   Model description and preliminaries

In this paper, we are interested in the following diffusive Hopfield neural networks with leakage and discrete delays:

where $n$ is the number of neurons in the networks, $x = (x_{1}, x_{2}, ..., x_{m})^{T}\in\Omega$ is the space vector, in which $\Omega\subseteq R^{m}$ represents a bounded set with smooth boundary $\partial\Omega$, and ${\rm mes}\; \Omega > 0$ means the measure of $\Omega$; $u_{i}(t, x)$ denotes the state of $i$th neuron at time $t$ and in space $x,$ $D_{ik}\geq0$ represents the transmission diffusion coefficient along the $i$th neuron; $\sigma$ and $\tau$, respectively, stand for the leakage delay and transmission delay; $a_{i} > 0$ indicates the rate with which the $i$th neuron will reset its potential to the resting state in isolation when disconnected from the networks and external inputs; $b_{ij}$ is the strength of the $j$th neuron on the $i$th neuron at time $t$ and in space $x$; $c_{ij}$ is the strength of the $j$th neuron on the $i$th neuron at time $t-\tau$ and in space $x$; $f_{j}(\cdot)$ and $g_{j}(\cdot)$, respectively, denotes the activation functions of the $j$th neuron without and with the time delay, and $I_{i}$ denotes a constant external input.

As for system (2.1), the initial-boundary value conditions are supplemented with

and

in which $\varphi_{i}\in C([-\max\{\sigma, \tau\}, 0]\times\Omega, \mathbb{R})$ representing the Banach space of continuous functions defined on the region $[-\max\{\sigma, \tau\}, 0]\times\Omega, \; i = 1, 2, ..., n.$

To achieve the FFTS goal, we refer to system (2.1) as the drive system, the response system is given as follows:

where $v_{i}(t, x)$ represents the response state, $U_{i}(t, x)$ means the control input. Associated with (2.3), the initial-boundary value conditions are shown as follows:

and

in which $\phi_{i}\in C([-\max\{\sigma, \tau\}, 0]\times\Omega, \mathbb{R}), \; i = 1, 2, ..., n.$

Denote $e_{i}(t, x) = v_{i}(t, x)-u_{i}(t, x)$, and then subtract (2.1) from (2.3), we deduce that the error system can be written as follows:

In view of (2.2) and (2.4), the initial-boundary value conditions of system (2.5) are equipped as follows:

and

We are now in a position to give some preliminary preparations.

Definition 2.1. If for a suitable designed controller and any initial state $e_{i}(s, x) = \phi_{i}(s, x)-\varphi_{i}(s, x), \ s\in [-\max\{\sigma, \tau\}, 0]$, there is a time $\mathbb{T}$ that depends (or does not depend) on the initial values such that

and

Then the drive system (2.1) and response system (2.3) are said to achieve finite-time (or fixed-time) synchronization.

Lemma 1 (see ^[31]). Suppose that $V(x):\mathbb{R}^{n}\to \mathbb{R}$ is a C-regular, positive definite and radially unbounded function and $x(\cdot): [0, \infty) \to \mathbb{R}^{n}$ is absolutely continuous on any compact subinterval of $[0, \infty)$. Let $\mathscr{K}(V) = k_{1}V^{\alpha}+k_{2}V^{\beta}$ satisfy

and

Then,

1) if $0\leq \alpha, \beta < 1$, $V(t)$ will reach zero in a finite time and the settling time is bounded by $\mathbb{T}\leq \min\Big\{\frac{V^{1-\alpha}(0)}{k_{1}(1-\alpha)}, \frac{V^{1-\beta}(0)}{k_{2}(1-\beta)}\Big\}$;

2) if $\alpha > 1, 0\leq \beta < 1$, $V(t)$ will reach zero in a fixed time and the settling time is bounded by $\mathbb{T}\leq \frac{1}{k_{1}}\frac{1}{\alpha-1} +\frac{1}{k_{2}}\frac{1}{1-\beta}$.

Lemma 2 (Poincaré's inequality, see ^[32]). Suppose that $u(x)\in H_{0}^{1}(\Omega)$, then we have the following inequality

where $\eta$ is a constant independent of $u$, and $H_{0}^{1}(\Omega)$ is a Sobolev space expressed by

Remark 2.1. As pointed out by Chen et al. in ^[33], the constant $\eta$ can be calculated as follows: $\eta = d^{2}$, where

Lemma 3 (see ^[34]). If $a_1, a_2, ..., a_n$ are positive numbers and $0 < r < p$, then

To realize the FFTS, the following assumption is given.

Assumption 1. For any $u, v\in \mathbb{R}$, there exist positive constants $p_{j}$ and $q_{j}$, such that

3.   Main results

In this section, the FFTS problem of drive-response systems (2.1)–(2.3) is discussed under a unified framework and the corresponding sufficient criteria are established.

Firstly, we design the controller by

where

and $\lambda_{1i},$ $\lambda_{2i} > 0$ are feedback control parameters to be determined, $i = 1, 2, ..., n.$

Our main result can be stated as follows.

Theorem 3.1. Suppose that Assumption 1 holds. If the control parameters in (3.1) satisfy

where

Then, $(I)$ the drive-response systems (2.1) and (2.3) can achieve finite-time synchronization when $0 < \alpha < 1$; $(II)$ the drive-response systems (2.1) and (2.3) can achieve fixed-time synchronization when $\alpha > 1.$

Proof. Define a Lyapunov-Krasovskii functional as follows:

with

and

We differentiate $V(t)$ along solutions of system (2.5), and obtain from Assumption 1 that

Recalling from Lemma 2, we have

On the other hand, one can easily deduce that

and

Therefore, substituting (3.5)–(3.8) into (3.4), we deduce from (3.2) that

Case I: When $0 < \alpha < 1$, with the definition of $E_{i}(t)$ and Lemma 3, we can see that

where

Putting (3.10) into (3.9), it follows that

Based on Case I in Lemma 1, we arrive at the conclusion that systems (2.1)–(2.3) can achieve the finite-time synchronization and the settling time is exactly estimated by

Case II: When $\alpha > 1$, a similar procedure as applied to derive (3.10) gives

Substituting (3.13) into (3.9), we find from Lemma 3 that

According to Case II in Lemma 1, it follows that the response system (2.3) can fixed-timely synchronize to the drive system (2.1). Moreover, the settling time can be precisely bounded by

This ends the proof of Theorem 3.1.

Remark 3.1. It is readily seen from (3.12) and (3.14) that the settling time is closely related not only to the controller parameters but also to the leakage and discrete delays, the finite or fixed time synchronization goal can be realized by adjusting the related parameters flexibly. One the other side, the synchronization criteria established in this paper are independent on the leakage and discrete delays, which are easier to check than the delay dependent ones. Therefore, the obtained theoretical results show that the control parameters and time delays including both leakage and discrete delys affect the synchronization mechanism from different aspects.

Remark 3.2. In ^{[23,25,27,28]}, the authors considered other types of neural networks with diffusion effects, some novel criteria have been established to realize the FFTS synchronization, but the leakage delay is not considered. In ^[27], by designing new negative exponential controllers, the finite-/fixed-time anti-synchronization problem of discontinuous neural networks with leakage delays is well studied. Unfortunately, the diffusion effects are still not considered. As we all know that network system with a diffusion term can be described by a partial differential equations which is a infinite dimensional dynamical system, which undoubtedly increases the complexity of the model. On the other hand, the leakage delay in negative feedback term also enhances the nonlinearity of the model itself, these undoubtedly bring many theoretical difficulties. To the best of our knowledge, there is no research on FFTS of neural networks considering both diffusion and delay effects until now. Thus, the obtained theoretical results are completely new and can be seen as a continuation work of the aforementioned references.

4.   A numerical example

In this section, we provide an example and its numerical simulations to support the theoretical result.

Example 4.1. For $n = 2, \; m = 1$, the parameters of the drive-response systems (2.1)–(2.3) are chosen as follows: $A = diag(a_{1}, a_{2}) = \left(\begin{array}{ccc} 0.2 & 0\\ 0 & 0.4 \\ \end{array} \right)$, $B = (b_{ij})_{2\times2} = \left(\begin{array}{ccc} 3.12 & -2.34\\ -4.2 & -1.68 \\ \end{array} \right)$, $C = (c_{ij})_{2\times2} = \left(\begin{array}{ccc} -2.1 & 1.4\\ -3.2 & 2.6\\ \end{array} \right)$, $D_{1} = 0.15, \quad D_{2} = 0.35, \ {\rm and}\ I_{1} = 1, \ I_{2} = -1, \; \sigma = 0.5, \; \tau = 0.2.$

To realize the FFTS between the drive-response systems (2.1)–(2.3), we design the following feedback controllers:

Case I (Finite-time synchronization):

Let $\Omega = [-8, 8]$, and choose the following activation functions

Clearly, $f_{j}(\cdot)$ and $g_{j}(\cdot)$ satisfy Assumption 1 with $p_{j} = 1, \; q_{j} = 1.6, \quad j = 1, 2.$

Since $\Omega = [-8, 8]$, we obtain $\eta = 4.$ Choosing $\lambda_{11} = 15$, $\lambda_{12} = 15$, $\lambda_{21} = 1, \; \lambda_{22} = 1, \alpha = 0.5$ in (4.1), we have

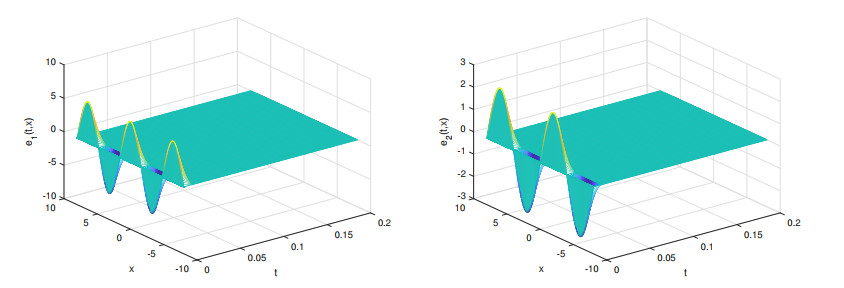
From Theorem 3.1, we can conclude that error system (2.5) is finite-time stable, and thus, the drive system (2.1) and response system (2.3) realize the finite-time synchronization under the designed controller (4.1) with $\alpha = 0.5$.

The spatiotemporal evolution trajectories of the error system are shown in Figure 1, which strongly support the desired finite-time synchronization results.

Case II (Fixed-time synchronization):

Take activation functions as follows:

It is easy to verify that Assumption 1 is satisfied with $p_{j} = 2, \; q_{j} = 2.5, j = 1, 2.$

Let $\Omega = [-\frac{9}{2}, \frac{9}{2}]$ and design the controller (4.1) with $\lambda_{11} = 25, \lambda_{12} = 24, \lambda_{21} = 1, \; \lambda_{22} = 1, \alpha = 1.5.$ By simple calculation, we obtain

It has been verified that all of the conditions in Theorem 3.1 are valid. Then the fixed-time synchronization can be realized for systems (2.1) and (2.3) under the feedback controller (4.1). Simulation results of the error system are shown in Figure 2, which demonstrate that the synchronization errors $e_{1}(t, x)$ and $e_{2}(t, x)$ converge to zero in a fixed time.

5.   Conclusions

In this paper, we studied the FFTS problem for leakage and discrete delayed HNNs with diffusions. By designing a novel negative exponential state feedback controller and constructing a Lyapunov-Krasovskii functional, and then by the aid of finite-/fixed-time convergence theorem, we obtained some novel and useful FFTS criteria under a unified framework, which can ensure the FFTS of the considered systems. Moreover, a numerical example is given to support the effectiveness and feasibility of the proposed approach. Compared with the asymptotical or exponential synchronization results on the leakage delayed NNs with diffusion effects, the established results greatly shorten the convergence time and hence have a better applicability. In the future work, we intend to study the dynamic behaviors of other kinds of network models such discontinuous HNNs or stochastic HNNs with leakage and diffusive effects.

Data Availability

The data used to support the findings of this study is available from the corresponding author upon request.

Acknowledgment

This work was jointly supported by the Anhui Provincial Natural Science Foundation (2208085MA04), Key Program of University Natural Science Research Fund of Anhui Province (KJ2021A0451), Key Program of Anhui Province University Outstanding Young Talents Support Plan (gxyqZD2022035), Open Research Fund of Anhui Province Engineering Laboratory for Big Data Analysis and Early Warning Technology of Coal Mine Safety (CSBD2022-ZD02).

Conflict of interest

The authors declare that they have no conflict of interest.