1.
Introduction
Floods are a frequently occurring and pervasive form of natural disaster, on par with earthquakes and cyclones. They are the most prevalent type of disaster and present significant hazards to individuals' and communities' economic and social progress [1]. According to the World Disaster Report by the International Federation of Red Cross and Red Crescent Societies, flooding accounted for the highest proportion of recorded disasters between 2008 and 2017, making up 41% of all disasters. Floods can be caused by heavy rainfall, melting snow, hurricanes, and other factors [2]. By enabling communities to plan and respond more skillfully, good flood forecasting and early warning systems can help lessen the effects of floods [3].
Floods present major challenges. Floods can seriously damage buildings, infrastructure, and houses, resulting in monetary losses for both people and communities [4]. Floods have the potential to be life-threatening, leading to injury or fatalities through drowning, illness, or other causes. Effective flood forecasting and early warning systems must be used in concert to address the problem [5]. A long-term flood risk management plan focusing on prevention, protection, and preparedness should be created, along with reliable and accurate flood risk maps [6]. Giving early and reliable information about a flood's likelihood and possible effects, it can assist in increased flood preparation. Emergency responders and the public can use this information to reduce a flood's impact by evacuating at-risk areas, sandbagging, and preparing emergency supplies [7]. Continuous improvement and refinement of flood forecasting and prediction methods are critical to ensure their accuracy and effectiveness. This can involve incorporating new data sources, developing more sophisticated models, and improving communication strategies to ensure that forecasts and predictions are communicated effectively to those who need them [8].
Floods are inherently unpredictable regarding their scale, timing, location, and how they interact with geography. Thus, complete control over them is often impossible [9]. Therefore, traditional flood management approaches that rely on structural measures like dams and levees to alter the flood characteristics and decrease their impact are not always viable options [10]. Although these measures can reduce flood risk to some extent, they cannot eliminate it and may have negative environmental consequences in some regions [9]. On the other hand, non-structural measures, which are more affordable and reversible, can effectively reduce flood risk without the need for expensive infrastructure [11].
Available flood modeling techniques are broadly classified into deterministic models (empirical, conceptual, and physics-based models), semi-distributed models (stochastic models), and data-driven models [such as machine learning (ML), artificial neural network (ANN), etc.] [12]. The first two categories necessitate some understanding of the problem's underlying physics, which can be expressed using simplified relations or partial differential equations in one or two dimensions [13]. Empirical flood models are based on statistical relationships between observed data and flood events. Empirical models use historical data and other sources to quantify the relationship between key variables such as rainfall, river discharge, and flood height. Empirical models are relatively simple to develop and use. Still, their accuracy depends on the quality and availability of data. They may not be applicable in regions with limited data or significant variability in flood events [9]. Conceptual flood models are based on a qualitative understanding of the physical processes that control the behavior of a river system. Conceptual models simplify the interactions between the catchment, channels, and floodplains, which are some of the distinct parts of the river system. When there is a lack of data, conceptual models are frequently utilized because they can explain the general behavior of a river system. However, according to Kratzert F et al report [14], it might not be enough to predict individual flood episodes. Physical processes that control the behavior of a river system are represented mathematically in flood models that are based on physics. Physics-based models consider variables like friction, turbulence, and water levels when describing how water flows in a river using intricate mathematical calculations. Although physics-based flood models are the most precise, they are also the most difficult and time-consuming to create and utilize. They require a lot of data and computing resources, which might not be feasible in places with scarce data [15]. In addition, using these models to analyze floods requires a predetermined set of hydrological and meteorological data that could not be accessible [16].
To represent floods, stochastic models also known as semi-distributed models incorporate features from both distributed and lumped models. They divide a river basin into many sub-catchments, simulating the water flow in each one separately [17]. To calculate the total discharge at the catchment outlet, the output discharge from each sub-catchment is combined. When compared to fully distributed models, this modeling strategy gives a more accurate representation of the geographical variability in the watershed while maintaining computational efficiency [18]. A probabilistic study of flood risk is possible by combining semi-distributed models with stochastic components to represent uncertainty in the model parameters. A completely distributed model may not be feasible in catchments with complicated hydrology, and a simple lumped model may not adequately capture hydrological processes [15]. In these situations, semi-distributed models are frequently used for flood risk assessments, floodplain mapping, and floodplain management because they offer a reasonable compromise between accuracy and computing complexity.
Contrarily, data-driven models are those that are created using statistical techniques, also referred to as "black box models", such as machine learning algorithms or artificial neural networks, to model the relationship between various factors that affect floods, such as rainfall, river discharge, and flood height [19]. These models develop a mathematical representation of the connections between the variables using historical data. Data-driven models are easier to create and use than physics-based models and do not need a thorough comprehension of the physical mechanisms that govern the behavior of a river system [20]. However, the quality and availability of the data determine its accuracy. They might not be useful in areas with scant data or a great deal of variation in flood events. Data-driven models are widely used for short-term flood forecasting, flood risk assessments, and floodplain mapping.
Machine learning (ML) has attracted significant attention recently as a means of researching and predicting floods [20]. Artificial intelligence's ML branch makes computers more adept at activities like data analysis, prediction, and classification without requiring explicit programming [21]. Machine learning algorithms may be trained on past flood data to forecast future floods, including the likelihood and intensity of a flood in a certain area. Additionally, ML can map the extent of floods using remote sensing data, including satellite photos, aerial photography, and other data. The creation of early warning flood systems, which seek to provide decision-makers with timely information on the likelihood that a flood will occur in a certain location, can also benefit from ML [22]. It may be applied to assess the likelihood of flooding in specific areas while taking infrastructure, land use, and population density into consideration. Furthermore, ML may be used to assess the magnitude of flood damage, including damage to houses, roads, and other infrastructure.
Over the past 20 years, ML techniques have continued to advance, demonstrating their suitability for flood-related problems and their reasonable pace of improvement over conventional approaches [23]. The use of ML techniques for flood forecasting has been the subject of a substantial amount of study in recent years. For instance, Ighile EH et al. [24] used historical flood data to anticipate flood-prone locations in Nigeria using logistic regression (LR) and artificial neural network (ANN) models. According to the results, the ANN model outperformed the LR model, and both models classified low-lying areas as being extremely susceptible to floods. Nayak et al. [25] provided flood forecasting using teaching learning-based optimization (TLBO) and deep belief network (DBN) for the Daya and Bhargavi rivers in India. The study compared the impact of barrage construction and evaluated the performance of DBN against TLBO in terms of root mean square error (RMSE) and mean absolute percentage error (MAPE) for forecasting periods of 1 day, 1 week, and 2 weeks. The study emphasized the importance of using ML for flood mitigation planning. Based on temperature and rainfall intensity, Sankaranarayanan S et al. [26] utilized deep neural networks to forecast floods in Kerala, India. The model outperformed other ML models in terms of accuracy and error, showing potential for efficient flood forecasting. Jabbari and Bae [27] evaluated the effectiveness of using ANN for bias correction in real-time precipitation forecasting to improve the accuracy of hydrometeorological models for flood forecasting in the Imjin River, resulting in a significant reduction in statistical error and improved flood forecasting performance. Elsafi [28] developed an ANN model to forecast the flood hazard in River Nile at Dongola Station, Sudan, using upstream flow data and showed that the model is reliable for detecting flood risks.
Several studies have combined multiple ML models to improve the accuracy of flood forecasting. For example, Chen et al. [29] proposed a two-stage probability analysis for estimating flood probability using satellite data involving decision trees and ANN, which can mitigate flooding damage in the urban drainage of Kaohsiung City. ML models can incorporate multiple data sources, such as meteorological observations, remote sensing data, and historical records, to produce more accurate forecasts [30,31]. ML models can make real-time forecasts, providing critical information to decision-makers during a flood event [32]. Identification of key elements that influence flood prediction is necessary for flood forecasting models. In several research works [33,34,35], the efficacy of various ML models for flood forecasting, such as ANNs, support vector machines (SVMs), random forests (RFs), and k-nearest neighbors (k-NNs), has been compared. The relative performances of the models under various conditions as well as the factors that affect that performance were analyzed in this study. Overall, past research on ML for flood forecasting and prediction has demonstrated that ML models may be useful for these tasks and could potentially increase the precision and effectiveness of flood forecasting and prediction systems. To overcome some of the drawbacks of the present strategies, further studies are required, particularly in the areas of model validation and the creation of interpretable models.
This review article aims to provide an overview of the state of the field and to compile the most recent research and advancements in this subject regarding the use of ML algorithms in flood forecasting and prediction. The following are the goals of the paper:
a) Review the literature on the use of ML for flood forecasting and prediction, looking at different methods and scenarios.
b) Evaluate the problems that need to be resolved while highlighting the benefits and drawbacks of the available ML-based solutions.
c) Highlight recent developments in the field and explore the use of ML for flood forecasting and prediction.
d) Offer suggestions for further research in this field, highlighting potential directions for development and prospective study topics.
This review paper follows the following fundamental structure: The definition of machine learning (ML), its terminology, learning obstacles, and an overview of the most popular learning models and techniques are covered in Section 2. In Section 3, the topic of machine learning models' use, effectiveness, and limits in forecasting and predicting floods is covered. The challenges and future steps for utilizing machine learning to estimate and predict floods are covered in Section 4. Section 5 discusses future directions and opportunities for machine learning in floods. Finally, in Section 6, the key takeaways from the analysis of the current state of ML in flood prediction and forecasting are concluded and summarized.
2.
Machine learning overview and techniques
Machine learning (ML) is a subset of artificial intelligence (AI) that uses statistical models and algorithms to train computer systems to learn from data and make predictions or decisions without requiring explicit programming (Liakos et al., 2018). Several basic terms and concepts are commonly used in ML (Section 2.1) and its classification (Section 2.2), which are important for understanding how these systems work.
2.1. Basic machine learning terminology
In the domain of data analysis and computing, AI particularly ML has witnessed significant growth in recent years, enabling the intelligent functioning of various applications [20]. ML, as a technology, allows systems to learn and enhance themselves through experience without relying on explicit programming [36]. The methodologies of ML encompass a learning process that aims to achieve a given task by learning from past experiences [21]. A performance metric that enhances experience is employed to evaluate the ML model's performance for a specific task [37]. Table 1 presents a summary of the evolution and advancement of ML. Xu and Liang [38] noted that various statistical and mathematical models are employed to assess the efficacy of machine learning models and algorithms. Once the training phase is complete, the trained model can leverage its acquired knowledge to categorize, forecast, or group new instances [21]. Pattern recognition, regression, and functional approximation are the three problems that can be solved using machine learning technology [8]. In addition, supervised learning, unsupervised learning, semi-supervised learning, and reinforcement learning are the four categories into which ML technology based on learning methods can be divided [39]. A general flowchart for a typical machine-learning process is shown below.
1: Start.
2: Define the problem and determine the goal.
3: Collect and pre-process data.
4: Split the data into training and testing sets.
5: Select a suitable model and train it using the training data.
6: Assess the model's effectiveness by testing it on independent testing data.
7: Refine the model by modifying its hyperparameters and architecture or adding more data.
8: Employ the model to make predictions on new and unseen data.
9: Continuously monitor the model's performance and update it as needed.
10: End.
2.2. Classification of machine learning techniques
ML allows machines to construct problem-solving models by discovering data patterns rather than through user intervention. Learning refers to specifying relations among variables using various algorithms and then utilizing those similarities to change the model to deliver more accurate output in the shortest possible time. There are four forms of machine learning: supervised, unsupervised, semi-supervised, and reinforcement learning.
2.2.1. Supervised learning
Supervised learning (SL) is a method of training algorithms that provides data and corresponding labels to a problem. The aim is to use the sample input–output pairs to train a model that can translate inputs into outputs [8]. SL uses a labeled dataset consisting of both a test set and instructional data to infer a mapping function. SL can then take place when a set of information and objectives has been defined. The two most common applications of SL are classification and regression [20]. Classification separates data into different categories, while regression predicts the output based on a set of input–output data combinations [40]. A model trained through SL can separate data from different sources and label them accordingly through classification, and regression can estimate results for the response variable through statistical models such as linear regression, logistic regression, multivariate regression, and decision trees [41]. One of the challenges of SL is finding labeled data, which can be difficult in cases with many options. For example, speech recognition has an endless number of possible combinations of words, making it impossible to account for all of them. The same problem arises with extensive unstructured data, where labeled data may not always be readily available. However, one advantage of AI systems is that they frequently gather a large amount of data, which provides abundant opportunities for labeled data to be created spontaneously [42].
2.2.2. Unsupervised learning
ML techniques such as unsupervised learning (UL) may find patterns in data without supervision or labeling. When working with unlabeled data, where the machine must independently determine the structure, this approach proves helpful.. To forecast the outcome, UL can uncover hidden patterns and trends [43]. A well-liked UL technique that determines the number of groups in data by contrasting data observations is the K-means classifier. The algorithm consists of two steps: first, it predicts how the model thinks the world will look, and second, it evaluates the environment and corrects or learns from its evaluations. UL is helpful in a variety of applications, including spotting outliers in the data or detecting anomalies. Based on qualities, it can sort data into comparable categories [44]. Since it is simpler to get unlabeled than labeled data, UL is frequently preferred to SL. The absence of labeled data, which makes it more difficult to connect the output with the desired output, is one of UL's disadvantages. Since the information gathered may not always correlate with the desired results, data cleansing is also essential in UL [45].
2.2.3. Semi-supervised learning
Semi-supervised learning (SSL) is an ML technique that trains models using both labeled and unlabeled data. It is employed when labeled data is hard to get or expensive. SSL strikes a balance between supervised and unsupervised learning to solve the drawbacks of both SL and UL methods. According to Gnecco et al. reported [41], SSL uses a combination of labeled and untagged data throughout the training process. Little labeled data is used, but a sizable amount of unlabeled data is accessible. The UL technique is first used to categorize the unlabeled data into relevant groups, and then labeling is done on this untagged data of a similar nature. This approach reduces the need for extensive data labeling, which can be time-consuming and costly. It also increases the applicability of unlabeled data, which is often challenging to use in most cases. SSL is used in various applications, including voice and face recognition, biometric reading, webpage ranking, web content classification, and protein sequence classification in DNA and text document classifiers that require active human interaction [20].
SSL makes various hypotheses to comprehend the link between the items in an unenforced dataset. It assumes continuity, examining items in the same group or label that are close to each other. The cluster hypothesis asserts that information is arranged into different clusters, where all location points in the same cluster share the same output tag. The final supposition is that ranges and concentrations may be applied to a lower dimensional surface than the input vector. SSL uses faux tagging to construct the model with less tagged training data, and several types of neural networks and instructional strategies can be merged throughout the procedure. The procedure of learning is continued until the system gives the expected outputs. The methods then use the unmarked dataset with virtual labels, and the outcome may no longer be valid. Labels from marked training data and data with faux labels are now linked. Finally, the first step is repeated by training the model with the new combined input, minimizing inaccuracies and raising the precision [36].
2.2.4. Reinforcement learning
Reinforcement learning (RL) is a type of ML that involves selecting the best set of actions in each environment to optimize the performance of various models. RL is often used to find the optimal path or trajectory to follow in data from a system. The learning algorithm in RL includes a response variable, allowing the machine to be trained with the correct response, and the reinforcing agent chooses where to go to perform the official task [46]. In the absence of training examples, an ML task is forced to learn from its own experience. The key to RL is making decisions sequentially and implementing change incrementally. The results depend on the configuration of the current input, and the outcome of the previous input determines the next input. In some cases, such as playing chess with a computer, RL's decision-making process is interdependent and needs to consider fully dependent response chains. To maximize the performance of multiple models, RL entails picking the best feasible set of actions in each environment. RL entails learning from experience and making judgments in a sequential manner [48]. In RL, the two types of reinforcement are positive and negative feedback systems. Positive feedback improves model responses, whereas negative feedback improves model behavior. RL has a wide range of practical applications, including robotics, education, healthcare, marketing, and home automation.
Table 2 outlines the advantages and disadvantages of the different types of machine learning.
3.
Latest developments in machine learning models for flood forecasting and prediction
In the last few decades, ML has become a strong tool for making flood predictions and forecasts more accurate. ML models that use both supervised and unsupervised learning methods have been developed and used to solve these problems. Researchers are also becoming more interested in using deep learning models to identify and project floods, in addition to standard machine learning models. ML models are advancing rapidly, with new methods and approaches being developed and tested for flood prediction and forecasting.
3.1. Elements of a flood forecasting and prediction system
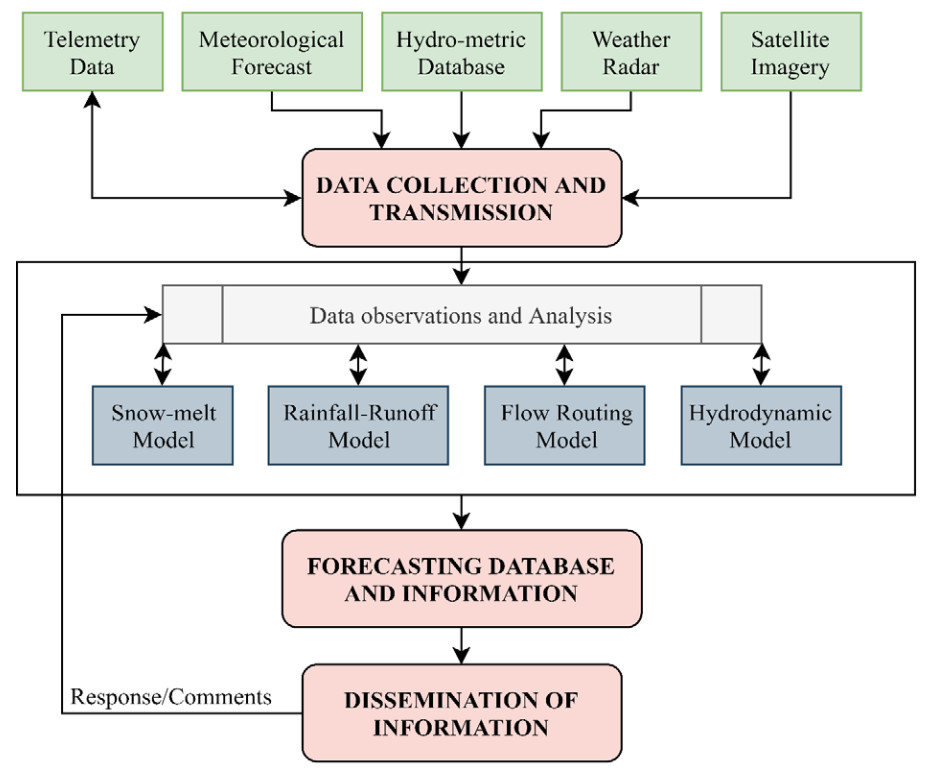
A flood analysis and warning system's main goal is to promptly and accurately alert the public and pertinent authorities of the impending arrival of a flood. A typical flood forecasting and prediction system is depicted in Figure 1 along with the data collecting, forecasting, and dissemination processes. The system's main components were the information sources that were utilized to forecast floods. These might contain things like stream flow measurements, soil moisture levels, and weather forecasts, among other data types. The next step is the data collecting and management component, which can accurately and quickly gather data from a variety of sources. This can entail the use of sensors, automated data-collecting methods, or hand-written data input. Data interpretation and analysis make up the third element [49]. To evaluate and understand the data to produce flood forecasts, ML algorithms and other methods are frequently utilized. For example, to achieve this, models that anticipate flood levels based on historical data may be created, or real-time data may be analyzed to produce short-term flood forecasts. The fourth component makes use of data analysis and interpretation to produce flood predictions and forecasts that are shown in the form of maps, graphs, or other visualizations. Dissemination of information to relevant parties, including first responders and the public, constitutes the fifth component. Utilizing multiple communication channels, including social media, SMS alerts, and open websites, may be necessary [50]. The last element is reaction and mitigation, which relates to taking appropriate steps based on flood predictions and forecasts to lessen the effects of flooding occurrences, such as evacuating at-risk regions or putting in place flood control measures [51].
3.2. Application of ML methods in flood prediction and forecasting models
ML techniques have been used in a variety of ways to solve forecasting and flood prediction problems. Typical strategies include:
1. Time-series forecasting: This technique forecasts future water levels in a river or other bodies of water by analyzing previous data on water levels, rainfall, and other pertinent variables.
2. Hydrological models: To calculate water flow and foresee probable floods, the method uses physical water cycle models. Integrating ML approaches improves the accuracy of these models.
3. Remote sensing: This entails mapping and monitoring land use, vegetation, and other elements that may influence flood risk using satellite data and aerial images. To evaluate the data and forecast upcoming floods, ML techniques like random forests, decision trees, or neural networks can be utilized.
4. Social media and web scraping: This involves collecting and analyzing data from social media and other online sources to gain insights into local weather patterns and flooding conditions. Natural language processing techniques are utilized to extract essential data and forecast future floods.
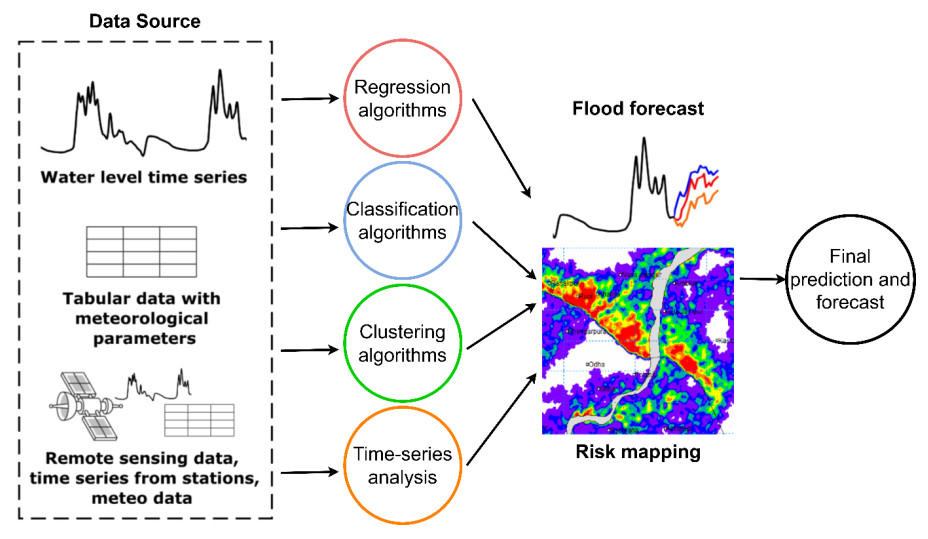
The ML techniques typically employed for flood prediction and forecasting frameworks are depicted in Figure 2. The process involves three major steps: 1) data collection, 2) machine learning model selection, and 3) flood forecasting, prediction, and risk mapping.. The first stage entails gathering several sorts of data, such as water level time series, tabular data, and remote sensing data. The second stage involves selecting acceptable machine learning models depending on the features of the data and the prediction task. Regression methods, such as linear regression and support vector regression, can be used to estimate flood levels based on historical data. These algorithms develop a mathematical link between input factors (such as weather and soil moisture levels) and output variables (such as flood levels) [52]. Based on input characteristics such as topography, soil type, and previous flood history, classification algorithms such as decision trees and random forests may be used to categorize locations as high, medium, or low risk of flooding [53]. Clustering approaches such as hierarchical clustering and k-means can be used to group together areas with common flood risk indicators. This can help identify places that are more vulnerable to floods to prioritize them for flood control and mitigation measures [54]. Short-term flood predictions may be created using time series analysis methods like long short-term memory (LSTM) and autoregressive integrated moving average (ARIMA). These methods can aid in identifying patterns and trends in the data that can be utilized to provide forecasts with a higher degree of accuracy [55,56]. Finally, in the third step, risk maps are created, and flood predictions and forecasts are made using the chosen machine learning models. Risk maps are produced to assist disaster response teams and policymakers in making decisions regarding flood mitigation and preparedness strategies. The main ML algorithms used in flood prediction and forecasting are k-nearest neighbor [57], deep convolutional neural network models [58], decision trees [59], support vector regression models [13,60], random forest (RF) [61], and cluster [62] and artificial neural networks [27,63]. The detailed applications of these ML techniques in different components are described in the following subsections.
4.
Time series analysis techniques
4.1. Artificial neural networks (ANNs)
Flood models have commonly utilized ANN algorithms more than any other method [64]. ANNs are a type of ML model that is modeled after the structure and function of the human brain. They are composed of interconnected nodes, which are artificial neurons that process data and make decisions based on that data [28]. By being trained on large datasets, ANNs can recognize patterns and make predictions, being widely used for a variety of tasks including image recognition, natural language processing, and game playing. ANN can handle enormously complex relationships, learn, and make intelligent decisions on its own through multiple layers [34,65]. A typical ANN has three primary layers: input, hidden, and output. The three major ANN parameters are weight, bias, and activation functions. ANNs derive meaning from historical data rather than from the physical characteristics of a catchment [20]. Thus, ANNs are regarded as reliable data-driven tools for developing black-box models of complex and nonlinear rainfall and flood relationships [66]. Furthermore, compared to most conventional models, many studies have shown that ANN is one of the finest modeling techniques, offering an acceptable level of generalization ability and speed [67]. ANNs have been successfully used for a variety of flood applications [63,68,69,70]. ANNs have demonstrated superior capabilities when interacting with nonlinear systems [71]. Elsafi [28] used ANN to forecast the River Nile flow and discovered that the results were accurate at detecting flood hazards. Feng and Lu [72] used ANN for flood forecasting and found that the model provided improved results in terms of performance and efficiency. Dtissibe [73] employed ANN to forecast floods, and the results of extensive experiments suggested that the proposed model is effective.
Although ANNs offer several advantages, they also have some limitations that should be considered. These include challenges in selecting appropriate network architecture, managing and preparing large amounts of data, difficulties in interpreting the physical meaning of the modeled system, the potential for lower accuracy compared to other methods, and the need for iterative fine-tuning of parameters [74]. ANNs are good at handling complex, nonlinear relationships; they may still struggle with highly nonlinear or chaotic systems, such as those found in some flood prediction scenarios. Varieties of ANN models for predicting floods are frequently used by researchers, such as multilayer perceptron (MLR) [75] or wavelet neural network [76], to overcome these limitations. Deep artificial neural networks (DNNs), also referred to as deep learning (DL) or deep neural networks, represent a relatively recent field of machine learning research that leverages multi-layer processing models to learn intricate data representations through multiple levels of abstraction. A basic definition of a DNN is an artificial neural network that contains multiple hidden layers situated between the input and output layers. These hidden layers may be supervised, partially supervised, or unsupervised in their operation [21].
4.2. Deep learning models
Deep learning models are a type of artificial neural network with multiple layers that perform hierarchical feature extraction. These models can learn complex representations of data by stacking multiple nonlinear transformations, allowing them to perform highly accurate predictions on tasks such as image and speech recognition [77]. Deep learning models are trained using large amounts of data and algorithms such as backpropagation, which allow them to adjust their parameters to minimize prediction error. In the hydrology and water resources areas, convolutional neural networks (CNNs) and recurrent neural networks (RNNs) are two types of deep learning models that are often used in flood forecasting and prediction [78,79]. CNNs are a special class of neural networks that excel at tasks requiring image or spatial data. They have been used in projects like flood inundation prediction utilizing radar data and high-resolution digital elevation models (DEMs) [80]. For instance, using a dataset of previous flood occurrences to train the model, researchers have utilized CNNs to forecast flood inundation in real time. The model was then used to predict future flood events. According to Kabir S et al reported [58], who used CNN for fluvial flood inundation prediction, CNN offers a lot of potential for real-time flood modeling and forecasting because of its simplicity, excellent performance, and computational efficiency. When it comes to jobs containing sequential data, such as time series data, RNNs are a particular sort of neural network that excels. By combining information on meteorological and hydrological conditions with information on land use and land cover, they have been employed for tasks including forecasting flood risk [55].
A specific kind of RNN models called long short-term memory (LSTM) was created to handle jobs requiring long-term dependencies in sequential data, such as time series data [14]. The employment of gating mechanisms by LSTM models makes them unique in that they can selectively remember or forget information from the past. In the study by Song T et al. [79], LSTM was employed for forecasting flash floods, and the study provided incredibly precise forecasts that help with disaster mitigation and preparedness. A special kind of RNN called a gated recurrent unit (GRU) was created to handle jobs requiring long-term dependencies in sequential input. GRU models employ gating methods to control the information flow, like LSTM models. However, compared to LSTM models, they are often thought to be easier and more effective to train [81]. An RNN model called echo state network (ESN) was created expressly to tackle tasks involving chaotic systems, including weather forecasting. The use of a fixed recurrent layer, a distinguishing characteristic of ESN models, enables them to learn intricate patterns in the data without the need for backpropagation, improving their computing efficiency [82]. The optimum model for the job at hand may need to be found via some trial and error depending on the unique qualities of the data and the prediction task. Both CNNs and RNNs have the potential to considerably increase the precision and dependability of flood prediction and forecasting systems and are expected to play a large role in the future. The benefits and drawbacks of various deep learning models are displayed in Table 3.
This section covers key machine learning methods for flood prediction. ANNs are widely used due to their ability to model complex, nonlinear relationships, though they face challenges such as architecture selection and data handling. Deep learning models include CNNs for spatial data and RNN, such as LSTM and GRU, for sequential and time series data. ESNs handle chaotic systems efficiently. These techniques enhance the accuracy and reliability of flood forecasting by leveraging sophisticated data analysis methods.
5.
Classification algorithms
5.1. Support vector machine (SVM)
Support vector machines (SVMs), which are based on the structural risk reduction concept, were created using statistical learning theory. To improve generalization performance, SVMs work to reduce both empirical risk and the learning machine's confidence interval [83]. Consequently, SVMs have been shown to be very trustworthy and effective algorithms for performing classification and regression tasks [83]. The SVM has been enhanced during the past 20 years to include more resources for classification and regression applications. Support vector regression (SVR) is a regression tool [84], whereas support vector classification (SVC) is a classification tool [85]. SVMs have several applications and may be used to solve both linear and nonlinear classification issues. They have earned a reputation as reliable and efficient machine learning algorithms for flood prediction. Hydrologists are increasingly using SVMs and SVR to predict floods [86]. Several models have been developed by various academics to predict floods using SVM [35,87,88,89,90,91]. Using SVM models for flash flood forecasting, according to Yan et al. [92], can be a helpful tool for boosting emergency response efforts and decreasing the loss of lives and property caused by urban floods. Similarly, Bermúdez et al. [93] utilized support vector regression (SVR) for spatial flood hazard mapping, and their results indicate that this regression technique has the potential to quickly and accurately compute flood extent and hazard maps. These findings suggest that SVM-based approaches can be effective tools in addressing flood-related challenges.
Despite their ability to generalize well and other numerous advantages, some shortcomings should be considered when using SVMs for machine learning tasks. These include challenges with selecting appropriate parameters, issues with algorithmic complexity that can result in longer training times for large datasets, difficulties in developing optimal classifiers for multi-class problems, and suboptimal performance in unbalanced datasets [94]. Some limitations should be considered when using SVMs for flood prediction, namely, the limited ability to handle missing data. SVMs are not well-suited for handling missing data, which can be a common problem in flood prediction scenarios where data may be difficult to obtain. Also, training time for SVMs can be computationally intensive, especially for large datasets. This can restrict its usefulness in instances involving real-time prediction. SVMs, which are adept at managing complex nonlinear relationships, may effectively handle extremely nonlinear or chaotic systems, such as those encountered in some flood prediction scenarios. To overcome a few of the limitations, researchers modified the original SVM and applied it to flood predictions. Li et al. [95] modified the SVM using a genetic algorithm (GA) to obtain better stream flow predictions than a simple SVM. Sahoo et al. [96] combined SVM with radial basis function neural network (RBFNN) and firefly algorithm (FA) to predict floods in Barak River and discovered that hybrid models outperform RBFNN, SVM, and ANN.
5.2. Decision tree (DT)
A decision tree is a machine-learning model that utilizes a tree-like structure to perform both regression and classification tasks. This supervised learning algorithm recursively splits the input data into smaller subsets based on the values of the input features. At each node of the tree, a decision is made by evaluating a specific input feature, and the data is partitioned into two or more branches based on the possible outcomes of the decision. This process is repeated until a leaf node is reached, which represents the final prediction of the model for the given input data [97]. During the construction of a decision tree, the recursive splitting process continues until the subsets become pure enough, which means that they only contain data points that belong to the same class or have similar output values. When the splitting process is finished, the resultant structure is a tree-like model that can be used to forecast new input data by traversing the tree from the root node to the leaf nodes while paying attention to the choices made at each internal node [29]. By using this strategy, the decision tree model can produce precise predictions for brand-new, unobserved data points and effectively capture the underlying connections between the input characteristics and the target variable [98]. Decision trees have been shown to be an effective tool in a range of machine-learning applications. In flood modeling, DT is often used. The two DT types that have been effectively used in flood modeling are regression and classification. In classification trees (CT), the leaves stand in for class labels and the branches for feature label conjunctions in a discrete collection of values that represent the final variables in a DT. When an ensemble of trees is utilized, however, and the target variable in a DT has continuous values, regression trees (RT) are used [99]. The DT method has been used by a few researchers to forecast and predict floods [30,97,100,101].
To forecast floods, Lawal ZK et al. [98] utilized SVR, DT, and logistic regression (LR); it was discovered that DT performed better. Another popular DT methodology for flood prediction is the random forests (RF) method. This method entails building an ensemble of decision trees, where each tree is constructed using a bootstrapped sample of the training data and a randomly selected subset of the input characteristics. To decrease the possibility of overfitting and increase the model's overall accuracy, the predictions of each individual tree in the ensemble are combined to get the final forecast. Due to its capacity to handle high-dimensional data and noisy input characteristics, the RF technique has been proven to be successful in a number of ML applications, including flood prediction. In the study by Costache R et al. [59], authors used six distinct ML techniques to forecast floods: decision tree, SVM, RF, adaptive neuro-fuzzy inference system (ANFIS), alternating decision tree (ADT), and ANN. It was found that the RF performed better when compared to other models. An additional crucial DT model is the M5 algorithm. The M5 algorithm is a decision tree–based model that is commonly used for regression tasks. It builds a decision tree by recursively partitioning the input space into smaller, more homogeneous regions. Once the tree is constructed, linear regression models are fitted to the data within each leaf node, thus improving the prediction accuracy by modeling continuous variables. The M5 algorithm is particularly useful because it combines the strengths of both decision trees and linear regression, offering a robust and interpretable approach for handling regression tasks with high accuracy.
According to the study by Zahiri A et al. [102], the M5 method is especially helpful for regression tasks because it can efficiently capture nonlinear correlations between the input characteristics and the target variable using a combination of linear models and decision trees. Singh KK et al. [103] employed a back propagation neural network (BNN) and M5 model tree-based regression technique to compute the mean annual flood. The results show that the M5 model tree performs better than the BNN. Significant improvements in flood forecasting and prediction have been accomplished using DTs. The ability of DTs to manage nonlinear relationships between the predictor variables and the result (flood prediction) is one of its key benefits. DTs can effectively manage missing data and work with big datasets. Furthermore, the interpretability of DTs makes it simple to visualize the decision-making process, which can help with comprehending the elements that affect the risk of flooding. DTs can also handle numerous outputs, which makes them an excellent choice for multi-class classification problems like estimating the severity of a flood occurrence.
5.3. Clustering algorithms
A common supervised machine learning technique used for both classification and regression problems is K-nearest neighbor (KNN). The KNN algorithm operates on the premise that data points with comparable characteristics are probably members of the same class or have comparable output values [20]. To make predictions for a new data point, the algorithm finds the K-nearest neighbors in the training data and aggregates their class labels or outputs. The value of K determines the number of neighbors to consider and can be chosen through cross-validation or other methods. KNN can handle binary and multi-class classification problems as well as regression tasks [57]. The algorithm is relatively easy to implement and computationally efficient, although it can be sensitive to the choice of K and the scaling of the data. The KNN technique has one possible limitation in that it needs to store the complete training set in memory, which may become difficult for very big datasets. Several researchers have employed the KNN algorithm for flood forecasting and prediction [104,105,106]. Alizadeh et al. [105] utilized several ML models, including radial basis neural networks (RBFNNs), feedforward neural networks (FFNNs), RNN, time delay neural networks (TDNNs), a grasshopper optimization algorithm (GOA)-based support vector machine (SVM), and the KNN model, to predict monthly flow. The results showed that the KNN model is well-suited for short-term predictions with more input features, while the RBFNN model is more appropriate for cases with fewer input features but more training observations. Sankaranarayanan et al. [107] used SVM, KNN, Naive Bayes, and deep learning to assess the accuracy and inaccuracy of flood prediction. According to the findings, the deep neural network can properly anticipate floods based on monsoon characteristics just before the flood happens. For the prediction of flash floods, El-Magd SAA et al. [108] combined KNN and extreme gradient boosting (XGBoost), with the results demonstrating that the XGBoost algorithm outperformed KNN in terms of accuracy.
The KNN algorithm has made several key advancements in flood forecasting and prediction. One of its key advantages is its ability to capture complex, nonlinear relationships between predictor variables and the outcome (flood prediction). KNN also does not make any assumptions about the underlying data distribution, making it a robust method for handling diverse datasets [109]. KNN also works well with big datasets and can manage missing data. The algorithm is a great tool for comprehending the variables that affect flood risk because its interpretability makes it simple to visualize the decision-making process. KNN is adaptable for a range of flood prediction issues since it can be utilized for both regression and classification tasks [32]. However, KNN has limits that should be considered when utilizing it for flood prediction. Particularly for big datasets or for prediction tasks with numerous characteristics, KNN has a high computational cost. This may reduce its applicability in situations involving real-time prediction. The KNN model's performance may be significantly impacted by the choice of the k parameter, which establishes the number of nearest neighbors utilized for prediction. Selecting an acceptable k value may be challenging and demands significant thought. The existence of noisy or duplicated features in the data might have a substantial influence on KNN since it is sensitive to irrelevant characteristics. In some flood prediction scenarios where the data may be complicated or noisy, this may restrict its effectiveness. The benefits and drawbacks of various ML models and applications in flood are shown in Table 4.
This section explores key classification algorithms for flood prediction. SVMs are effective for both linear and nonlinear tasks but face challenges such as parameter selection and handling large datasets. DTs use a tree-like structure for regression and classification, with variations like RF and M5 models improving performance. KNNs classify data based on proximity to other data points and handle complex, nonlinear relationships but can be computationally intensive and sensitive to the choice of parameters. These techniques are widely applied in flood forecasting to enhance accuracy and handle diverse datasets.
6.
Unsupervised learning
Unsupervised learning is a machine learning category in which a model is trained on an unlabeled dataset. The advent of hierarchical learning, clustering algorithms, dimensionality reduction approaches, latent models, and outlier detection techniques have all contributed to considerable improvements in recent years [45]. Unsupervised learning algorithms can be used to find hidden patterns and correlations in the data that might not be immediately obvious in the field of flood forecasting and prediction [43]. These algorithms can aid in finding obscure factors, including weather patterns or soil moisture levels, which are crucial for flood prediction. Unsupervised learning algorithms, such as clustering algorithms like k-means [54] and hierarchical clustering [115], and dimensionality reduction techniques like principal component analysis [116] have been successfully used to predict floods. These algorithms are helpful for organizing comparable data points into groups, finding abnormalities in the data, and simplifying the data to make it easier to model and analyze. It is important to note that unsupervised learning algorithms do not assume any specific relationship between predictor variables and the outcome of flood prediction. Therefore, they should be combined with other supervised or semi-supervised methods to achieve more accurate and reliable results [117].
Inyang et al. [118] used an unlabeled dataset of flood events to predict flood risks using a two-stage unsupervised learning approach based on k-means clustering and self-organizing maps (SOM). The results indicated a significant enhancement in the classification and prediction of flood risks using a single machine-learning tool. Oppel and Fischer [43] used a new clustering technique based on unsupervised learning to identify recurrent temporal patterns in rainfall and investigate flood types. The results revealed that the temporal distribution of rainfall intensities has shifted from early peaks to a more uniform distribution. Devi et al. [119] proposed unsupervised deep learning approaches, stacked auto encoder (SAE) connected with tapped delay line (TDL), for the frequency and prediction of a flood. When compared to historical records, the proposed approach demonstrates improved performance and yields better results than traditional approaches. Unsupervised learning has some interesting advantages, but there are also many drawbacks, such as inappropriate technique selection, lack of interpretability, lack of operational success, ignoring simple non-machine-learning-based tools, overfitting, data quality issues, and inaccurate model building [45]. It is important to consider these limitations carefully and to choose the appropriate model for the task at hand. Supervised learning algorithms, which use labeled data, are generally more suitable for prediction tasks, including flood prediction.
7.
Semi-supervised learning
Semi-supervised learning is a ML technique that combines aspects of both supervised and unsupervised learning. By utilizing a small set of labeled data along with a large amount of unlabeled data, the model can be trained to improve its accuracy. In the context of flood prediction, where labeled data may be scarce, semi-supervised learning can be particularly useful since it leverages the abundance of unlabeled data to enhance the model's performance [120]. Semi-supervised learning is a relatively new technique for flood prediction. For the detection of flood-prone areas, Gnecco G et al. [41] used supervised and semi-supervised machine-learning techniques. The results show that semi-supervised techniques outperform supervised techniques. Zhao et al. [121] used semi-supervised ML, i.e., weakly labeled support vector machine (WELLSVM), for urban flood susceptibility. The results show that WELLSVM outperformed and can better utilize spatial information (unlabeled data). However, semi-supervised learning also has limitations that should be considered when applied to flood prediction: difficulty in labeling data, limited ability to handle nonlinear relationships, limited interpretability, and reliance on the quality of the unlabeled data.
8.
Reinforcement learning
Reinforcement learning (RFL) is a category of machine learning in which an agent learns to make decisions by taking actions in an environment to maximize a reward signal. The agent's primary objective is to acquire a policy that can map states to actions, with the aim of maximizing the cumulative reward over time [20]. Reinforcement learning algorithms use trial-and-error experiences to learn, updating their policy based on the results of their actions. This method is employed in a variety of applications, including control systems, robotics, and gaming [122]. The benefits include adaptability, versatility, end-to-end learning, and optimum solutions; nevertheless, there are several drawbacks, including sample inefficiency, difficulty in designing reward signals, difficulties in the exploration vs. exploitation trade-off, and lack of interpretability [123].
It is feasible to employ reinforcement learning in flood forecasting and prediction. In this situation, the agent's goal would be to decide how to manage water resources, such as opening floodgates or releasing water from dams, to lessen the chance of flooding. A multitude of factors, such as rainfall data, river flow rates, and historical floods, may influence the agent's conclusions. The incentive signal would be developed with the purpose of lowering damage and flooding danger. Reinforcement learning systems might learn to make judgments based on past knowledge and environmental data. The agent might learn to optimize its decision-making over time to reduce the danger of floods. This method has the potential to be more flexible and dynamic than standard flood prediction approaches, which are frequently based on predefined rules or algorithms. However, to ensure their usefulness and dependability in a real-world flood prediction situation, reinforcement learning algorithms would need to be properly constructed and verified. Bowes et al. [124] used RFL for real-time stormwater system control and flood mitigation and monitoring [125].
9.
Challenges and way forward
There are several challenges associated with ML-based methods for flood prediction and forecasting. Some of these challenges and potential solutions are discussed in the following subsections.
9.1. Data availability and quality
Accurate flood prediction and forecasting require reliable high-resolution spatial-temporal data inputs, including meteorological data, hydrological data, topographic data, and land use/land cover data [126]. However, such datasets may not always be readily available or may have missing data and noise [127]. To address the challenge of data availability and quality, it may be necessary to gather and process additional data sources or to improve the quality of existing data. In the absence of ground-based observations, satellite imagery and remote sensing-based datasets have recently shown promising results for providing a more comprehensive view of the flood event [128,129]. However, there are several key challenges to utilizing remote sensing datasets, such as lower spatial and temporal resolutions, missing data due to cloud covers, and data integration and fusion [130]. Also, remote sensing-based datasets often have errors and biases that need to be corrected [131]. Although the advances in remote sensing technology, such as the development of higher resolution sensors and more frequent data collection, can help to improve the spatial and temporal resolution of remote sensing data [132], significant efforts are required to develop new algorithms and data processing tools to utilize these products for accurate flood predictions and forecasting [133].
9.2. Model complexity
Flood prediction and forecasting models can be complex [134], involving interdependent static and dynamic physio-meteorological variables as inputs and outputs, and may require sophisticated ML algorithms that can handle complex and nonlinear dynamic systems [135]. This can make it difficult to develop and tune the models for optimal performance [136]. To address the challenge of model complexity, advanced ML algorithms, such as deep learning or reinforcement learning, have recently emerged to handle the complexity of the interdependent variables for different prediction tasks in hydrology, including flood prediction and forecasting [40,135,137,138,]. Additionally, by merging the predictions from several models, model ensemble approaches have been developed to enhance the overall performance of the hydrological prediction [139]. To completely comprehend the benefits and drawbacks of different ensemble methodologies, more research is necessary, particularly around hydrological forecasting and flood prediction.
9.3. Temporal and spatial variability
Being a complicated natural phenomenon with a wide variety of geographical and temporal features, floods make it challenging to construct generic models that can reliably anticipate their impacts [140]. The breadth of the flooded region, the duration of the event, the kind of terrain and land cover, and the intensity of the rainfall are the features that distinguish one flood event from another, and that can have a significant impact on the temporal and geographical variability of the flood event [141]. Flood models must be tailored to the characteristics of each site and flood occurrence to address the issue of temporal and geographical variability in floods.
To completely comprehend the spatial and temporal variability of the flood, this strategy necessitates gathering data from a variety of sources, such as hydrological models, topographical maps, meteorological records, and satellite pictures. This aids in making informed decisions about flood preparedness, response, and recovery [142,143]. By including this data into the flood model, more accurate predictions and simulations of the behavior and impacts of the flood may be produced. This supports informed decisions on flood preparedness, response, and recovery [144,145,146].
9.4. Limited generalization
ML models are often developed through the training process, which involves modifying the model's parameters to perform a job using a particular dataset. Because these models are only trained on data that they have already seen, they may not be able to manage differences in the distribution of data outside of the training set, which might prevent them from effectively generalizing to new situations or places [147]. As a result, the models may only have limited practical application since they may not function effectively with fresh, untested data. Transfer learning approaches can be used to get over this problem of low generalizability [148]. Transfer learning is a subset of ML that deals with taking a model that has already been trained and changing it to apply it to a different issue or situation [149]. This is accomplished by either fine-tuning the pre-trained model on a new dataset, which may have a different distribution than the original training data, or by changing the model architecture to better suit the new task or location. Transfer learning seeks to overcome the issue of limited generalizability by utilizing the information acquired from the pre-trained model and applying it to enhance performance on a new assignment.
9.5. Uncertainty and sensitivity
Models for forecasting and predicting floods are essential tools for reducing the consequences of flooding on people and infrastructure. However, these models are inherently imprecise and could produce false forecasts because of the complexity of the underlying physical processes involved in flood generation and spread as well as the lack of data [150]. Accurate flood prediction might be difficult due to the limited data availability and sensitivity to small changes in the input data or parameters [151]. Probabilistic ML models may be utilized to solve the issue of uncertainty and sensitivity in flood prediction models [152,153]. The inherent uncertainty in the prediction task may be captured by probabilistic ML models, such as Bayesian neural networks, by modeling the probability distribution of the output rather than offering a single-point estimate [154]. This makes it possible to communicate the prediction's uncertainty in a more complex way, enabling more thoughtful decision-making. Additionally, sensitivity analysis methods may be applied to find any variables that significantly affect model performance [155,156]. Sensitivity analysis entails changing the model's input parameters over time and evaluating how that affects the model's output, which enables the most important factors to be identified. By concentrating on the most crucial inputs, the model's accuracy may be increased. Alternatively, this knowledge can be utilized to better understand the model's constraints and enhance the data gathering procedure to more accurately capture the underlying processes.
9.6. Lack of interpretability
The interpretability of ML models is a crucial factor to consider since it can affect the accuracy of the predictions the model makes as well as the capacity to see any biases or flaws in the model [157]. Due to the numerous nonlinear transformations and hundreds of thousands of parameters included in certain ML models, especially those built using deep learning approaches, they can be particularly challenging to comprehend [158]. Interpretable ML models [159] can be used to solve the problem of the lack of interpretability in ML models, such as decision trees or linear regression. These models are more transparent in their decision-making because they clearly reveal the relationship between the input features and the output. To further pinpoint the most crucial elements influencing model performance, feature selection and dimensionality reduction approaches may be applied [160]. These methods can help the model employ fewer input characteristics, which enables a more in-depth examination of the connections between inputs and outputs. This can aid in increasing the model's interpretability by drawing attention to the most crucial elements and enabling a more in-depth analysis of the model's predictions.
Emerging solutions for model interpretability: To overcome the interpretability challenge, recent advancements in interpretable machine learning have provided practical solutions. Two widely used approaches are SHAP (Shapley Additive Explanations) values and LIME (local interpretable model-agnostic explanations).
SHAP values: Based on game theory, SHAP assigns an importance value to each feature by considering the contribution of a feature to a prediction in various possible scenarios. This allows users to see how much each feature contributes to an individual prediction and helps build trust in complex models like neural networks or ensemble methods.
LIME: LIME is a model-agnostic tool that explains individual predictions by approximating the complex model with an interpretable surrogate model. By perturbing the input data and observing changes in the output, LIME can provide insights into how the original model is making its decisions.
Both methods allow users to better understand machine learning models without compromising their accuracy. For flood prediction tasks, SHAP and LIME can help decision-makers grasp how inputs like rainfall, soil moisture, and terrain contribute to the forecasted flood risk, improving the model's transparency and usefulness in practical applications.
10.
Future directions
ML is increasingly being integrated into flood forecasting, offering promising advancements in prediction accuracy, real-time data processing, and decision-making support [161]. However, the development and implementation of ML models in flood forecasting require further research and innovation to address existing gaps and fully harness their potential. One of the key areas for improvement is the computational efficiency of ML models. While current models can handle vast datasets, there is a need for more computationally efficient algorithms that can process data in real time without compromising accuracy. Future research could focus on developing lightweight models or optimizing existing models to minimize processing time and resource consumption. Moreover, basin-specific models that consider the unique hydrological, geographical, and meteorological characteristics of each basin are necessary. Although general ML models exist, they often fail to capture the specific complexities of different regions. Future efforts should focus on creating models that account for basin diversity, soil moisture variability, and other local factors. This would enhance prediction accuracy for different terrains and climates [162].
Model update frequency is another area that warrants attention. Rapidly evolving flood conditions, influenced by real-time data such as rainfall, river flow, and ground saturation, necessitate continuous updates to forecasting models. The future of ML in flood forecasting should include adaptive models that can integrate real-time data and update predictions dynamically, ensuring that forecasts remain accurate as conditions change. The explainability and transparency of ML models are also critical. Many advanced ML algorithms, particularly deep learning models, are often perceived as black boxes due to their complexity. To build trust among stakeholders such as flood management authorities and local communities, ML models need to be more interpretable [163]. Future research could explore the development of interpretable models or tools that provide clear explanations for how predictions are generated. This would help decision-makers understand the reasoning behind the forecasts and improve the adoption of these models.
Furthermore, the integration of remote sensing and IoT technologies with ML holds immense potential. Internet of Things based ML in flood forecasting refers to a system that uses IOT devices to collect data about the physical environment and meteorological conditions to anticipate the likelihood of floods. Sensors, cameras, weather stations, and other Internet of Thing's devices may collect real-time data on precipitation, river flow, soil moisture, and other pertinent factors. The machine learning model may then be trained and improved in accuracy using this data. IOT and ML may be used to develop an end-to-end flood forecasting system that provides real-time data and forecasts. This will make it possible for authorities and communities to prepare for and respond to anticipated flood events. IOT-based ML for flood forecasting can provide more accurate and up-to-date information than traditional techniques, reducing the damage caused by floods to people and communities. Satellite data, radar, and LiDAR provide crucial information about precipitation, water levels, and land use changes. However, the effective fusion of these datasets with ML models remains an open research question. Future research should focus on creating more robust data fusion techniques, enabling ML models to incorporate remote sensing data with ground observations for more comprehensive flood predictions.
Another exciting area of development is hybrid modeling, where ML models are combined with physical or hydrological models. Such approaches could help leverage the strengths of both data-driven and physics-based techniques, offering a more holistic view of flood dynamics. Future research should explore how hybrid models can be used to simulate complex hydrological processes while maintaining the predictive power of ML. Ethical considerations also need to be at the forefront of future developments in ML-based flood forecasting. The collection and use of large datasets, especially from IoT devices and social media, raise concerns about privacy, data ownership, and potential biases in the data. Researchers and developers should ensure that ML models are built and deployed in an ethical manner, considering the social, legal, and ethical implications of their use.
11.
Conclusions
ML can considerably increase the accuracy and reliability of flood predictions. ML systems can analyze massive volumes of data and uncover specific linkages and patterns to provide a more comprehensive picture of flood risk. However, when employing ML for flood forecasting, it is critical to approach with caution and consider the flaws and constraints of these models. High-quality data, regular updates, minimizing model bias, and not overfitting the training data are all required to maintain ML models reliable. Despite these challenges, it is expected that future developments in machine learning will lead to applications that are more complex and successful. It is crucial to stay updated on developments and continue exploring the potential of ML across various domains and applications. This literature analysis provides a thorough summary of the state of the art in ML for flood prediction, emphasizing the advantages and disadvantages of the various ML algorithms. This review serves as a starting point for researchers and professionals aiming to utilize ML for forecasting floods and developing more precise prediction models.
Use of AI tools declaration
The authors declare they have not used Artificial Intelligence (AI) tools in the creation of this article.
Acknowledgments
No external funding was granted for this work. The datasets are available from the corresponding author on reasonable request.
Conflicts of interest
No potential conflict of interest was reported by the authors.









 DownLoad:
DownLoad: