Stability analysis on the post-quantum structure of a boundary value problem: application on the new fractional $(p, q)$-thermostat system

1.   Introduction

Fractional calculus, which deals with special operators of arbitrary order, has led to enormous advances in the theoretical and practical development of various fields of engineering science. In order to understand the reasons for this importance and extent, we can refer to useful mathematical results that include the investigation of the existence of solutions to fractional differential equations and the design of different numerical algorithms for simulating fractional processes. For example, the trace of the fractional calculus can be seen recently in a variety of clinical disciplines, economics and analysis of economic models, medical models, image processing, engineering, physics etc. ^[1,2,3]. In the part of existence of solution and study of approximate solutions, fractional calculus has shown its simulation power well. In most classical mathematical models, standard integer-order operators were used, but nowadays it has been proven to everyone that for existing models, fractional operators can also provide better results with the least simulation error. Examples of these fractional order redesigns of mathematical models can be seen in the articles published by ^{[4,5,6,7,8,9,10,11,12,13,14,15,16,17]}.

In 1910, quantum calculus, which is also called $q$-calculus in some sources, was proposed and introduced by Jackson ^[18,19]. Along with the advances in modern analysis, $q$-calculus paved the way for more applicability with the definitions of new concepts offered. Gradually, $q$-calculus found its way into some physical and mathematical problems, so that Fock ^[20] defined special $q$-difference equations in which he could study the symmetry of hydrogen atoms. Later, using the concepts and properties in $q$-calculus, researchers took help from $q$-operators and $q$-series to analyze the theory of quantum mechanics, number theory, hypergeometric functions, orthogonal polynomials and combinations ^[21,22]. For more studies in this regard, see ^{[23,24,25,26,27]}.

Along with the evolution of $q$-calculus, a new extension of this field has recently been generalized by two parametric operators and notions in $(p, q)$-calculus. The starting point of this theory is related to a resaerch done by Chakrabarti and Jagannathan ^[28]. The trace of $(p, q)$-operators can be followed in some theories such as approximation theorey ^[29,30], Lie groups, B{é}zier curves and surfaces ^[31], physical sciences ^[32] and hypergeometric series ^[33]. Later, Sadjang ^[34] proved several basic theorems in $(p, q)$-calculus and also established the $(p, q)$-Taylor formula. The $(p, q)$-gamma and $(p, q)$-Beta functions are obtained by Cheng et al. and Milovanovic et al. ^[35,36], respectively. Next, some researchers imported $(p, q)$-calculus in the boundary value problem (BOVP) theory and derived the existence properties for solutions of $(p, q)$-difference equations. After that, in 2020, Soontharanon et al. ^[37] derived and proved some useful properties on $(p, q)$-calculus.

In 2020, Soontharanon and Sitthiwirattham ^[38] studied a Riemann-Liouville-like $(p, q)$-integro-difference $w^{th}$-order BOVP under the $(p, q)$-Robin boundary conditions with the fixed point theory, which takes the form

where ${\rm{\mathsf{ρ}}} \in \mathbf{J}_{p, q}^a - \Big\{ 0, \dfrac{a}{p}\Big\}$ and $\mathbf{J}_{p, q}^a : = \Big\{ \dfrac{q^j}{p^{j+1}} a:j \in \mathbb{N}_0 \Big\} \cup \{ 0\}$. Also, $\mathbb{F} \in \mathcal{C} (\mathbf{J}_{p, q}^a \times \mathbb{R}^3, \mathbb{R})$ and $G_1, G_2 : \mathcal{C} (\mathbf{J}_{p, q}^a, \mathbb{R}) \to \mathbb{R}$. Note that $\varphi \in \mathcal{C} (\mathbf{J}_{p, q}^a \times \mathbf{J}_{p, q}^a, [0, \infty))$ and

and also ${}^{R}\mathbb{D}_{p, q}^{w^*}$ is the fractional Riemann-Liouville-like $(p, q)$-derivative of ${w^*}^{th}$-order so that $w^* = w_i(i = 0, 2, 3)$.

In 2021, Neang et al. ^[39] studied the existence results with the help of the same techniques used in ^[38] for another nonlinear Caputo-like $(p, q)$-difference $w^{th}$-order BOVP under the separated boundary conditions, given by

with the first order $(p, q)$-difference $\mathbb{D}_{p, q}$, the ${w}^{th}$-order Caputo-like $(p, q)$-derivative ${}^{c}\mathbb{D}_{p, q}^{w}$ and the continuous function $\mathbb{F}: [0, \frac{a}{p^w}] \times \mathbb{R} \to \mathbb{R}$.

In 2022, Neang et al. ^[40] completed their study by defining a new continuous function $\mathbb{F}: [0, a] \times \mathbb{R} \to \mathbb{R}$ to reduce the complication of the domain of function and proved their results for solutions of the Caputo-like $(p, q)$-difference BOVP

with the first order $(p, q)$-difference $\mathbb{D}_{p, q}$ and the ${w}^{th}$-order Caputo-like $(p, q)$-derivative ${}^{c}\mathbb{D}_{p, q}^{w}$. For more studies based on $(p, q)$-calculus, see ^{[41,42,43,44,45,46]}.

In the real world, to study the dynamics of some phenomena, we need to have a mathematical model of them. In fact, mathematical models are the basis of studying all kinds of physical, mechanical and engineering processes and phenomena. One of these mathematical models in which various studies have been done recently is the mathematical model presented for the thermostat system. So far, different versions of this physical equation have been defined and specific research objectives have been studied in each article. The above new structures inspired us in this paper to continue our study by introducing a new two-parametric structure of a $(p, q)$-thermostat equation with the Caputo-like $(p, q)$-derivative. In fact, we will manipulate the standard second-order thermostat system formulated by Infante et al. ^[47], given by

and transform it into a new framework in the context of $(p, q)$-calculus (will be introduced in Section 3).

To the best of our knowledge, the Caputo-like $(p, q)$-difference BOVP of the thermostat has not yet been studied in the context of the $(p, q)$-derivatives. In this study, we will generalize the aforesaid standard BOVP (1.1) to a Caputo-like $(p, q)$-BOVP with the help of the first order $(p, q)$-difference $\mathbb{D}_{p, q}$ and the ${w}^{th}$-order Caputo-like $(p, q)$-derivative ${}^{c}\mathbb{D}_{p, q}^{w}$ so that $w \in (1, 2]$ is the order of the new $(p, q)$-system. Also, we will show that if $p = 1$ and $q\to 1$, then our suggested $(p, q)$-model reduces to a Caputo-like $q$-BOVP and a standard Caputo BOVP of the thermostat equation, respectively. To fill this gap, we first define our desired model by concepts of $(p, q)$-calculus and find its fractional Riemann-Liouville-like $(p, q)$-integral-based solution, and then we prove the existence and stability results.

It is notable that the $q$-derivatives and $(p, q)$-derivatives are based on the finite difference re-scaling, working with these methods are simple and fast and computational softwares run the commands quickly. Moreover, note that another main advantage of $q$-calculus and $(p, q)$-calculus is that they deal with nondifferentiable functions. Therefore, these theories are very much suitable to deal with any physical phenomena, which are described by equations involving nondifferentiable functions.

The organization of this paper is as follows. Some needed notions and definitions of $(p, q)$-calculus are collected as the preliminaries in Section 2. Next, in Section 3, the Caputo-like $(p, q)$-difference BOVP of thermostat and its special cases are introduced. Also in Section 3, we obtain the integral structure of the solution and prove the existence results. In Section 4, we investigate stabilities for the post quantum structure of the thermostat model. Numerical examples are given and investigated graphically in Section 5. The paper is concluded with conclusions in Section 6.

2.   Preliminaries

In two subsections, we review some definitions about $q$-calculus and $(p, q)$-calculus. Throughout the paper, we take $q\in (0, 1)$ and $0 < q < p \leq 1$.

2.1.   $q$-calculus

The $q$-analogue of $({\rm{\mathsf{ρ}}}_1 - {\rm{\mathsf{ρ}}}_2)^m$ [$q$-power function] is formulated as ^[48]

Generally, let $m = w \in \mathbb{R}$, then

Clearly, we have $({\rm{\mathsf{ρ}}}_1)_q^{(w)} = {\rm{\mathsf{ρ}}}_1^{w}$ if ${\rm{\mathsf{ρ}}}_2 = 0$ ^[48]. Also, the $q$-number $[{\rm{\mathsf{ρ}}}]_q$ and $q$-gamma function $\Gamma_q(\cdot)$, for ${\rm{\mathsf{ρ}}} \in \mathbb{R}$ and $w \in \mathbb{R} \backslash \mathbb{Z}^{\leq 0}$, are defined as

so that $\Gamma_q(w +1) = [w]_q \Gamma_q (w)$ ^[48].

Definition 2.1 (^[49]). The $q$-difference of the function $\Psi$ is given by

Note that $\mathbb{D}_{q}^m \Psi (t) = \mathbb{D}_{q} (\mathbb{D}_{q}^{m-1} \Psi (t)), \, \forall\, m \in \mathbb{N}$ and $\mathbb{D}_{q}^0 \Psi (t) = \Psi(t)$ ^[49].

Definition 2.2 (^[50,51]). The $w^{th}$-order fractional Riemann-Liouville-like $q$-integral of the function $\Psi \in \mathcal{C}([0, + \infty), \mathbb{R})$ is defined by

if the integral exists.

Definition 2.3 (^[50,51]). Let ${\rm{\mathsf{λ}}} = [w]+1$. The $w^{th}$-order Caputo-like $q$-derivative of $\Psi\in \mathcal{C}^{({\rm{\mathsf{λ}}})}([0, + \infty), \mathbb{R})$ is defined by

if the integral converges.

2.2.   $(p, q)$-calculus

Here, we provide some definitions about $(p, q)$-calculus. It is sufficient to put $p = 1$, then all definitions of this subsection reduce to the existing definitions in the previous subsection.

The $(p, q)$-analogue of $({\rm{\mathsf{ρ}}}_1 - {\rm{\mathsf{ρ}}}_2)^m$ [$(p, q)$-power function] is defined by ^[37]

Generally, let $m = w \in \mathbb{R}$, then

Also, $({\rm{\mathsf{ρ}}}_1)_{p, q}^{(w)} = \frac{1}{p^w}{\rm{\mathsf{ρ}}}_1^{w}$ if ${\rm{\mathsf{ρ}}}_2 = 0$ ^[37]. In the sequel, the $(p, q)$-number $[{\rm{\mathsf{ρ}}}]_{p, q}$ and $(p, q)$-gamma function $\Gamma_{p, q}(\cdot)$, for ${\rm{\mathsf{ρ}}} \in \mathbb{R}$ and $w \in \mathbb{R} \backslash \mathbb{Z}^{\leq 0}$, are defined by

so that $\Gamma_{p, q}(w +1) = [w]_{p, q} \Gamma_{p, q} (w)$ ^[37]. Moreover, the $(p, q)$-Beta function $\mathbb{B}_{p, q}(\cdot, \cdot)$ is defined by

Definition 2.4 (^[37]). The $(p, q)$-difference of the function $\Psi$ is defined by

Note that if $p = 1$, then $\mathbb{D}_{1, q} \Psi (t) = \mathbb{D}_{q} \Psi (t)$, and also if $q\to 1$, then $\mathbb{D}_{1, q\to 1} \Psi (t) = \Psi' (t)$.

Definition 2.5 (^[37]). Let $\Psi : [0, a] \to \mathbb{R}$ be continuous. The $(p, q)$-integral of $\Psi$ is defined by

Definition 2.6 (^[37]). The $w^{th}$-order fractional Riemann-Liouville-like $(p, q)$-integral of the function $\Psi \in \mathcal{C}([0, a], \mathbb{R})$ is defined by

if the integral exists.

Note that if $p = 1$, then ${}^{R}\mathbb{I}_{1, q}^w \Psi (t) = {}^{R}\mathbb{I}_{q}^w\Psi (t)$, which is defined in Definition 2.2.

Definition 2.7 (^[37]). Let ${\rm{\mathsf{λ}}} = [w]+1$. The $w^{th}$-order Caputo-like $(p, q)$-derivative of $\Psi\in \mathcal{C}^{({\rm{\mathsf{λ}}})}([0, a], \mathbb{R})$ is defined by

if the integral converges.

Note that if $p = 1$, then ${}^{c}\mathbb{D}_{1, q}^w \Psi (t) = {}^{c}\mathbb{D}_{q}^w\Psi (t)$, which is defined in Definition 2.3.

The following properties are important and are proved in ^[37].

Lemma 2.8. ^[37] Let $w, \tilde{w} > 0$, then

$(A_{p, q})$ $^{R}\mathbb{I}_{p, q}^{ w } \big[^{R}\mathbb{I}_{p, q}^{ \tilde{w} } \Psi (t) \big] = ^{R}\mathbb{I}_{p, q}^{ \tilde{w} } \big[^{R}\mathbb{I}_{p, q}^{ w } \Psi (t) \big] = ^{R}\mathbb{I}_{p, q}^{ w + \tilde{w} } \Psi (t)$.

$(B_{p, q})$ $^{c}\mathbb{D}_{p, q}^w \big[^{R}\mathbb{I}_{p, q}^{ w } \Psi(t) \big] = \Psi (t)$.

Lemma 2.9. ^[37] Let $w, \tilde{w} > 0$ and $\Psi(t) = t^{\tilde{w}}$, then

$(C_{p, q})$ $^{R}\mathbb{I}_{p, q}^{ w } \Psi(t) = \dfrac{\Gamma_{p, q}(\tilde{w}+1)}{\Gamma_{p, q}(\tilde{w}+w +1)}t^{\tilde{w}+w}$.

$(D_{p, q})$ $^{c}\mathbb{D}_{p, q}^{ w } \Psi(t) = p^w \dfrac{\Gamma_{p, q}(\tilde{w}+1)}{\Gamma_{p, q}(\tilde{w} - w +1)}t^{\tilde{w} - w}, \, \, \tilde{w} > w$.

$(E_{p, q})$ $\int_0^t (t-qv)_{p, q}^{(w-1)} v^{\tilde{w}} \mathrm{d}_{p, q}v = \mathbb{B}_{p, q} (\tilde{w}+1, w) t^{w + \tilde{w}}.$

The results of the following example will be used in the paper.

Example 2.10. Let $\beta \in \mathbb{R}$, then

(i) $\int_0^\beta (\beta - qv)_{p, q}^{(w-1)} v^5 \mathrm{d}_{p, q}v = \beta^{w+5} \mathbb{B}_{p, q}(6, w).$

(ii) $\int_0^{pa} (pa - qv)_{p, q}^{(w-2)} v^5 \mathrm{d}_{p, q}v = (pa)^{w+4} \mathbb{B}_{p, q}(6, w-1).$

Proof. We just proved the $(p, q)$-integral (i). The second one is similar. By Definition 2.5, we can write

This ends the proof. □

Theorem 2.11 (^[37]). Let ${\rm{\mathsf{λ}}} = [w]+1$, then

Shortly, we have

where $k_i^* \in \mathbb{R}; \, i = 0, 1, \dots, {\rm{\mathsf{λ}}}-1$.

Finally, we recall the Brouwer fixed point theorem, which will be used in the sequel.

Theorem 2.12 (^[52]). If $G$ is a nonempty, closed, bounded and convex set and $g:G\to G$ is continuous, then there exists $s \in G$ such that $g(s) = s$.

3.   The Caputo-like ${(p, q)}$-BOVP of thermostat model

In the present section, we intend to introduce our Caputo-like $(p, q)$-difference $w^{th}$-order BOVP of the thermostat system inspired by the standard second-order BOVP (1.1). For $w \in (1, 2]$, the fractional $(p, q)$-difference thermostat BOVP is formulated by

with the first order $(p, q)$-difference $\mathbb{D}_{p, q}$ and the ${w}^{th}$-order Caputo-like $(p, q)$-derivative ${}^{c}\mathbb{D}_{p, q}^{w}$ defined in Definitions 2.4 and 2.7, respectively. $\mathbb{F}: [0, a] \times \mathbb{R} \to \mathbb{R}$ is continuous and $0 < q < p\leq 1$.

It is natural that if $p = 1$, then the above Caputo-like $(p, q)$-difference $w^{th}$-order BOVP of the thermostat system reduces to the Caputo-like $q$-difference $w^{th}$-order BOVP, which takes the form

with the first order $q$-difference $\mathbb{D}_{q}$ and the ${w}^{th}$-order Caputo-like $q$-derivative ${}^{c}\mathbb{D}_{q}^{w}$ defined in Definitions 2.1 and 2.3, respectively. Moreover, if $p = 1$ and $q\to 1$, then the Caputo-like $(p, q)$-difference $w^{th}$-order BOVP (3.1) reduces to the Caputo $w^{th}$-order BOVP, which takes the form

with the first order derivative $\mathbb{D}^1 = \dfrac{\mathrm{d}}{\mathrm{d}t}$ and the ${w}^{th}$-order Caputo derivative ${}^{c}\mathbb{D}^{w}$ ^[1,3]. Finally, if $p = 1$, $q\to 1$, $w = 2$ and $M_1 = M_2 = 0$, then our Caputo-like $(p, q)$-difference $w^{th}$-order BOVP (3.1) reduces to the standard second-order thermostat system (1.1).

In this paper, we focus on the main Caputo-like $(p, q)$-difference $w^{th}$-order BOVP (3.1) and then, we will compare our numerical solutions to solutions of the $q$-difference, fractional and second-order BOVPs (3.2), (3.3) and (1.1), respectively. For the next computations, the Banach space $\mathbb{H}$ is considered under the norm $\Vert \Psi\Vert = \sup \vert \Psi(t)\vert$ for all $t\in [0, a]$.

3.1.   The structure of solutions for (3.1)

In this subsection, by considering the given Caputo-like $(p, q)$-difference $w^{th}$-order BOVP (3.1) of thermostat system, we first find the fractional Riemann-Liouville-like $(p, q)$-integral-based structure of the solutions.

Theorem 3.1. Let $b > 0$, $a \geq 1$, $w\in (1, 2]$, $\beta \in [0, a]$, $M_1, M_2 \in \mathbb{R}$ and $t \in [0, a]$. For the given function $A: [0, a] \to \mathbb{R}$, $\Psi$ satisfies (as a solution) the linear Caputo-like $(p, q)$-BOVP of thermostat

if and only if $\Psi (t)$ for all $t\in [0, a]$ satisfies (as a solution) the $(p, q)$-integral equation

so that the unique solution $\psi$ satisfies the homogeneous Caputo-like $(p, q)$-BOVP

Proof. At first, suppose that $\psi$ satisfies (3.6). With the help of the fractional Riemann-Liouville-like $(p, q)$-integral ${}^{R}\mathbb{I}_{p, q}^{ w }$ (given in Definition 2.6) and by applying it on the Caputo-like $(p, q)$-difference equation (3.6), we get

with $k_0^*, k_1^* \in \mathbb{R}$ (by Theorem 2.11). If we apply $\mathbb{D}_{p, q}$ on both sides of (3.7), then $\mathbb{D}_{p, q} \psi(t) = k_1^*$. Now, from the condition $\mathbb{D}_{p, q} \psi (0) = M_1$, we get $k_1^* = M_1$. On the other hand, the second condition of the homogeneous Caputo-like $(p, q)$-BOVP (3.6), i.e., $b\mathbb{D}_{p, q}\psi (pa) + \psi (\beta) = M_2$, gives $k_0^* = M_2 -M_1(b + \beta)$. We put $k_0^*$ and $k_1^*$ in $\psi(t)$ given by (3.7), and we have

In another step, suppose that $\Psi(t)$ satisfies (as a solution) the linear Caputo-like $(p, q)$-difference $w^{th}$-order BOVP (3.4) of thermostat system. By Theorem 2.11, the general solution of (3.4) admits the following form

with $k_0^{**}, k_1^{**} \in \mathbb{R}$. Definition 2.6 immediately gives

If we apply $\mathbb{D}_{p, q}$ on both sides of (3.9), then

From $\mathbb{D}_{p, q} \Psi (0) = M_1$, we find that $k_1^{**} = M_1$. Also, the second condition of (3.4) gives

Now, we put the obtained values for $k_0^{**}$, $k_1^{**}$ and $\psi(t)$ in $\Psi(t)$, given by (3.9), and get

Thus, (by (3.8)) we have

which is the same desired Eq (3.5). On the other hand, the solution of (3.10) is proved easily and satisfies the linear Caputo-like $(p, q)$-difference $w^{th}$-order BOVP (3.4) of the thermostat system. Therefore, the proof is completed. □

3.2.   Existence analysis of solutions

To conduct the existence analysis of solutions related to the Caputo-like $w^{th}$-order $(p, q)$-BOVP (3.1) of the thermostat system, we get help from Theorem 3.1 and define an operator for $t\in [0, a]$ as

Notice that a fixed point of $\mathcal{A}$ is considered as a solution for the given Caputo-like $w^{th}$-order $(p, q)$-BOVP (3.1) of the thermostat system. To confirm such a claim, the Brouwer fixed point theorem ^[52] will be useful.

Theorem 3.2. Let $\mathbb{F} \in C([0, a]\times \mathbb{R}, \mathbb{R})$ and $\sup \{ \vert \psi (t) \vert: \; t \in [0, a] \} \leq \lambda$ so that the unique solution $\psi$ satisfies the homogeneous Caputo-like $w^{th}$-order $(p, q)$-BOVP (3.6) of thermostat system. Set

If

then the Caputo-like $w^{th}$-order $(p, q)$-BOVP (3.1) of the thermostat system has a solution.

Proof. First of all, since $\mathbb{F}$ is continuous, $\mathcal{A}$ is continuous. Next, we intend to prove the theorem by establishing $\mathcal{A} G \subset G$, where $G: = \{ \Psi (t) \in \mathbb{H}:\; \Vert \Psi \Vert \leq 2\lambda \}$ is a nonempty, closed, bounded and convex set for $\lambda > 0$. In other words, this will show that $\mathcal{A}$ maps $G$ into $G$. For $\Psi(t) \in G$, we have

In view of the upper bound of all variables $t$, we get

by considering $\Vert \cdot \Vert$ as the supremum norm. If we pay attention to (3.12), then the inequality (3.13) becomes

Therefore, $\mathcal{A}\Psi \in G$ or $\mathcal{A}G \subset G$. The Brouwer fixed point theorem (Theorem 2.12) confirms the existence of at least a fixed point for $\mathcal{A}$ as a solution of the Caputo-like $w^{th}$-order $(p, q)$-BOVP (3.1) of thermostat system, and this ends the proof. □

Theorem 3.3. Let ($J_{\mathbb{F}}$) $\exists\, K > 0$ such that $\vert \mathbb{F}(t, \Psi) - \mathbb{F}(t, \Psi') \vert \leq K \vert \Psi -\Psi' \vert, \, \, \forall\, t\in [0, a]$ and $\forall\, \Psi, \Psi' \in \mathbb{H}$.

If

then the Caputo-like $w^{th}$-order $(p, q)$-BOVP (3.1) of the thermostat system admits a solution on $\mathbb{H}$ uniquely.

Proof. We begin our proof by considering arbitrary elements $\Psi, \Psi' \in \mathbb{H}$ and $t\in [0, a]$. In this case, by (3.11), we have

This gives

The inequality condition (3.14) proves this fact that $\mathcal{A}$ is a contraction on the Banach space $\mathbb{H}$. Therefore, $\mathcal{A}$ possesses a fixed point uniquely via the (Banach) contraction principle, and this is equivalent to the existence of a unique solution for the Caputo-like $w^{th}$-order $(p, q)$-BOVP (3.1) of the thermostat system. The proof is complete. □

4.   Ulam stable solutions

In the current situation, the Ulam stable solutions are defined for the given Caputo-like $w^{th}$-order $(p, q)$-BOVP (3.1) of thermostat system based on the existing sources in which the authors use different stability cases for different structures of problems ^[9,53,54]. For more detailed information about the Hyers-Ulam and Hyers-Ulam-Rassias stability, we refer the redaers to ^[55,56].

Definition 4.1. If for all $V\in \mathbb{H}$ satisfying

there is $\Psi \in \mathbb{H}$ as a solution of (3.1) and there is $\delta > 0$, such that for each $t\in [0, a]$,

then it is said to be that the Caputo-like $w^{th}$-order $(p, q)$-BOVP (3.1) of the thermostat system is $\mathbf{HU}$-stable (in the sense of Hyers-Ulam).

Definition 4.2. If for every $V\in \mathbb{H}$ satisfying

there is $\Psi \in \mathbb{H}$ as a solution of (3.1) and there is $\delta^* > 0$, such that for all $t\in [0, a]$,

then it is said to be that the Caputo-like $w^{th}$-order $(p, q)$-BOVP (3.1) of the thermostat system is $\mathbf{HUR}$-stable (in the sense of Hyers-Ulam-Rassias).

We call $V\in \mathbb{H}$ as a solution of (4.1) if and only if there is $\mathbb{G}: [0, a] \to \mathbb{R}$ satisfying

($S_{A1}$) $\vert \mathbb{G} (p^wt) \vert \leq \zeta$;

($S_{A2}$) ${}^{c}\mathbb{D}_{p, q}^w V (t) = \mathbb{F} \big(p^wt, V (p^wt)\big) + \mathbb{G} (p^wt)$.

This fact is similar for inequality (4.2).

Lemma 4.3. If $V$ solves the inequality (4.1), then

Proof. Since $V$ solves the inequality (4.1), then from the above definitions, the solution to ($S_{A2}$) satisfies

Hence, we have

and this completes the proof. □

Theorem 4.4. Let the condition ($J_{\mathbb{F}}$) be satisfied. Also, assume that the inequality (4.1) holds. If

then the Caputo-like $w^{th}$-order $(p, q)$-BOVP (3.1) of the thermostat system is $\mathbf{HU}$-stable.

Proof. By considering the solution (3.5) together with Lemma 4.3, for each $t\in [0, a]$, we get

It gives that

Therefore,

In view of the above relation, we can derive

where

So, the Caputo-like $w^{th}$-order $(p, q)$-BOVP (3.1) of the thermostat system is $\mathbf{HU}$-stable. □

For the $\mathbf{HUR}$-stabiliy analysis, another condition is needed as follows.

($S_{A3}$) Assume an increasing function $\hbar : [0, a] \to \mathbb{R}^+$. Also, $\exists\, \ell > 0$ such that

Based on the next lemma and the above condition ($S_{A3}$), we shall establish the $\mathbf{HUR}$-stability for the Caputo-like $w^{th}$-order $(p, q)$-BOVP (3.1) of the thermostat system.

Lemma 4.5. Let $V$ solves the inequality (4.2) and ($S_{A3}$) is to be held. In this case, we have the following estimate:

Proof. By the hypothesis, we know that the function $V$ satisfies the inequality (4.2). Thus, for $t\in [0, a]$, the function $\mathbb{G}: [0, a] \to \mathbb{R}$ can be found and it satisfies $\vert \mathbb{G} (p^wt) \vert \leq \zeta \hbar(p^wt)$ and

In this case, according to Theorem 2.11, the solution of the latter Caputo-like $w^{th}$-order $(p, q)$-difference equation is formulated by

In view of the structure of this solution and by ($S_{A3}$), we can estimate

This completes the proof. □

Theorem 4.6. Assume that the Lipschitz condition ($J_{\mathbb{F}}$) along with the condition ($S_{A3}$) are to be held and inequality (4.3) is fulfilled, then the Caputo-like $w^{th}$-order $(p, q)$-BOVP (3.1) of the thermostat system is $\mathbf{HUR}$-stable

Proof. By considering the solution (3.5) together with Lemma 4.5 for $t\in [0, a]$, we get

It gives that

Therefore,

In view of the above relation, we can derive

where

So, the Caputo-like $w^{th}$-order $(p, q)$-BOVP (3.1) of the thermostat system is $\mathbf{HUR}$-stable. □

5.   Example

Our examples help us to see the structure and dynamics of solutions in some special cases of the Caputo-like $q$-model, $(p, q)$-model and standard model of the thermostat system.

Example 5.1. We intend to provide an illustration of numerical solutions of the given Caputo-like $w^{th}$-order BOVP of thermostat system graphically. For this purpose, we consider some initial and fixed data as follows: $M_1 = 2$, $M_2 = 3$, $\beta = 0.75$, $b = 0.002$, $a = 10$ and $A(t) = t^5$. With this data, we formulate the general form of the Caputo-like $w^{th}$-order BOVP of thermostat system

where different values can be determined for the order $w \in (1, 2]$. In (5.1), we consider three cases for derivation operator ${}^{c}\mathbf{D}_{\mathbf{\bullet}}^w$ as follows:

so that these operators denote the Caputo-like $w^{th}$-order $(p, q)$-difference derivative, Caputo-like $w^{th}$-order $q$-difference derivative and Caputo $w^{th}$-order derivative, respectively.

In Section 3, we discussed on the solution function of the Caputo-like $w^{th}$-order $(p, q)$-BOVP (5.1) of the thermostat system when ${}^{c}\mathbf{D}_{\mathbf{\bullet}}^w \Psi (t) = {}^{c}\mathbb{D}_{p, q}^w \Psi (t)$. For the case ${}^{c}\mathbf{D}_{\mathbf{\bullet}}^w \Psi (t) = {}^{c}\mathbb{D}_{p, q}^w \Psi (t)$, by using arbitrary values for the order $w$ and by (3.5), the solution function of (5.1) is formulated by

Lemma 2.9, the relations (2.7) and (2.8) and Definition 2.5 about the $(p, q)$-integral of the function $p^5t^5$ give

Since

we have

Similarly, by Lemma 2.9, Example 2.10, the relations (2.7) and (2.8) and Definition 2.5 about the $(p, q)$-integral of the function $p^{5}t^5$, we obtain

Finally, by following the above computations and formulas, we have

Therefore,

From (5.3)–(5.6), the solution function (5.2) becomes

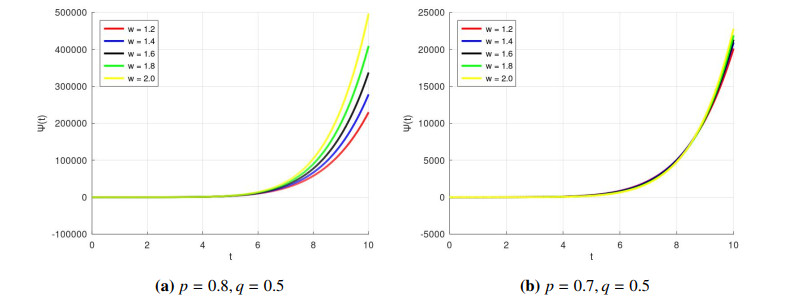
In Figure 1, we plot the smooth graphs of the solution function given by (5.7) for orders $w = 1.2, 1.4, 1.6.1.8, 2.0$ and the parameters (a) $p = 0.8$ and $q = 0.5$ and (b) $p = 0.7$ and $0.5$. We see that as the fractional orders tend to the integer value $w = 2.0$, the graphs overlap together and have the same behavior. Also, as the parameter $p$ tends to $q$, this overlap is much greater. In fact, based on these graphs, we can be sure that $(p, q)$-solutions tend to the standard classical solutions of the system and this confirms that these $(p, q)$-derivatives (defined without the notion of the limit) give the better results in the simulations.

In Figure 2, we plot the 3D-surface graphs of the solution function $\Psi(t)$ (given in (5.7)) with respect to the parameters $0\leq t\leq 10$ and $0 < p \leq 1$ with (a) $w = 1.15$ and $q = 0.5$, (b) $w = 1.45$ and $q = 0.5$, (c) $w = 1.75$ and $q = 0.5$, and (d) $w = 1.95$ and $q = 0.5$.

In the given thermostat system, it discharges and adds an amount of heat based on the detected temperature by a sensor by varying the value of the constant $\beta$ between zero to $10$. In this simulation, we change the values of $\beta$ as $\beta = 0.5$, $\beta = 1.5$, $\beta = 2.5$, $\beta = 3.5$, $\beta = 4.5$, $\beta = 5.5$, $\beta = 6.5$, $\beta = 7.5$, $\beta = 8.5$, and $\beta = 9.5$, respectively. In Table 1, in six different positions, we see that by increasing the value of $\beta$ and tending it to $10$, the system discharges the heat $\Psi(t_i)$ at specefic times $t_i, \, i = 0, 2, 4, 6, 8, 10$; in fact, the released heat decreases by the thermostat when $\beta$ increases.

Now, we consider two special cases for ${}^{c}\mathbf{D}_{\mathbf{\bullet}}^w \Psi (t)$.

Case (1) We put $p = 1$ and ${}^{c}\mathbf{D}_{\mathbf{\bullet}}^w \Psi (t) = {}^{c}\mathbb{D}_{q}^w \Psi (t)$. In this case, the Caputo-like $w^{th}$-order BOVP (5.1) reduces to a Caputo-like $w^{th}$-order $q$-BOVP of thermostat system as follows

with the first order $q$-difference $\mathbb{D}_{q}$ and the ${w}^{th}$-order Caputo-like $q$-derivative ${}^{c}\mathbb{D}_{q}^{w}$. Easily, one can find that the solution function of the above $q$-thermostat system is given by

Case (2) We put $p = 1$, $q\to 1$ and ${}^{c}\mathbf{D}_{\mathbf{\bullet}}^w \Psi (t) = {}^{c}\mathbb{D}_0^w \Psi (t)$. In this case, the Caputo-like $w^{th}$-order BOVP (5.1) reduces to a standard Caputo $w^{th}$-order BOVP of thermostat system as follows

with the first order derivative $\mathbb{D}^1 = \dfrac{\mathrm{d}}{\mathrm{d}t}$ and the ${w}^{th}$-order Caputo derivative ${}^{c}\mathbb{D}^{w}$. As a result, the solution function of the above fractional thermostat system is given by

Example 5.2. In the present example, we determine some numerical values like $M_1 = 2$, $M_2 = 3$, $\beta = 0.75$, $b = 0.002$, $a = 10$, $p = 0.7$ and $q = 0.25$. Also, the fractional order is chosen as $w = 1.45$. Now, the nonlinear form of the Caputo-like $w^{th}$-order $(p, q)$-BOVP of the thermostat system is assumed by

with

In Figure 3, we can see a 3D-suface plot of the continuous function $\mathbb{F}$ with respect to $\Psi$ and $t\in [0, 10]$. In virtue of the condition ($J_{\mathbb{F}}$) in Theorem 3.3, we find that $K = 0.00085 > 0$. Therefore, by the inequality (3.14), we get

The final result of Theorem 3.3 ensures the existence of a unique solution for the given Caputo-like $w^{th}$-order $(p, q)$-BOVP (5.12) of the thermostat system.

On the other side, we have

As $K = 0.00085 < 0.05861$, by the condition (4.3), if the inequality

is satisfied for each $V\in \mathbb{H}$, then the Caputo-like $w^{th}$-order $(p, q)$-BOVP (5.12) of the thermostat system is $\mathbf{HU}$-stable by Theorem 4.4.

6.   Conclusions

This paper investigated the qualitative and analytical results for a new fractional $(p, q)$-model of the thermostat system. In fact, we applied $(p, q)$-difference and also the Caputo-like $w^{th}$-order $(p, q)$-derivative for modeling the thermostat system. The existence results were established and the stability property was satisfied from the Ulam point of view. In two numerical examples, the behavior of solutions in the given Caputo-like $(p, q)$-model was examined carefully. In these examples, we considered three cases: The Caputo-like $q$-model, $(p, q)$-model and standard model of the thermostat system. In every case, we obtained the corresponding solution and observed the graph of these solutions by varying some parameters of the models. In fact, we were going to investigate the structure of the solutions in the context of a new field of mathematics like post-quantum calculus, which is well-known to the calculus without the limit. The obtained numerical results and the graphical illustrations showed that these three models yield the closer results and we can remodel other systems by using the $(p, q)$-derivatives, because these operators and other notions of $(p, q)$-calculus have less complexity in the computations and computers can run the commands in the fast manner. In future studies, we will try to expand our results to other real systems equipped with $(p, q)$-derivatives and symmetric $(p, q)$-derivatives. Moreover, we will find a new numerical algorithm for finding approximate solutions of the $(p, q)$-difference equations.

Use of AI tools declaration

The authors declare they have not used Artificial Intelligence (AI) tools in the creation of this article.

Acknowledgments

The authors extend their appreciation to the Deputyship for Research & Innovation, Ministry of Education in Saudi Arabia for funding this research work through the project number (IF2/PSAU/2022/01/22923). Also, all authors would like to thank dear reviewers for their constructive and useful comments to improve the quality of the paper.

Conflict of interest

The authors declare no conflicts of interest.