1.
Introduction
In 1977 and 1984, Freedman and Waltman ([1,2]) studied the following two-predator one-prey system:
where xi(t) stands for the size of the ith population and all the parameters are positive constants.
However, the deterministic system has its limitation in mathematical modeling of ecosystems since the parameters involved in the system are unable to capture the influence of environmental noises [3,4,5]. Therefore, it is of enormous importance to study the effects of environmental noises on the dynamics of population systems. Assume that the parameters ri are affected by white noises, i.e., r1↪r1+σ1˙B1(t), −r2↪−r2+σ2˙B2(t), −r3↪−r3+σ3˙B3(t), where Bi(t) are mutually independent standard Wiener processes defined on a complete probability space (Ω,F,P) with a filtration {Ft}t≥0 satisfying the usual conditions. Then, the stochastic two-predator one-prey system with white noises can be expressed as follows:
On the other hand, "all species should exhibit time delay" in the real world, and incorporating time delays in biological systems makes the systems much more realistic than those without time delays ([6,7,8,9,10]). Hence, in this paper we concern the dynamics of the following stochastic two-predator one-prey system with S-type distributed time delays:
where Dji(xi)(t)=ajixi(t)+∫0−τjixi(t+θ)dμji(θ), ∫0−τjixi(t+θ)dμji(θ) are Lebesgue-Stieltjes integrals, τji>0 are time delays, μji(θ) are nondecreasing bounded variation functions defined on [−τ,0], τ=maxi,j=1,2,3{τji}.
2.
Persistence in mean and Extinction
Denote Aij=aij+∫0−τijdμij(θ), D1=r1−σ212, Di=ri+σ2i2 (i=2,3) and
Assume that Θ>0. For the matrix corresponding to Θ (respectively, Θk), denote by MΘij (respectively, MΘkij) the complement minor of the element at the i-th row and the j-th column (i,j,k=1,2,3).
Theorem 2.1. For any (ξ1,ξ2,ξ3)T∈C([−τ,0],R3+), system (1.3) has a unique global solution (x1(t),x2(t),x3(t))T∈R3+ on t∈[0,+∞) a.s. Moreover, for any constant p>0, there are Ki(p)>0 such that
Proof. The proof is rather standard and here is omitted (see, e.g., [11] and [12]).
Lemma 2.1. ([13]) Suppose Z(t)∈C(Ω×[0,+∞),R+) and limt→+∞o(t)t=0.
(i) If there exists constant δ0>0 such that for t≫1,
then
(ii) If there exist constants δ>0 and δ0>0 such that for t≫1,
then
Lemma 2.2. Consider the following auxiliary system:
(a) If D1<0, then limt→+∞Xi(t)=0 a.s. (i=1,2,3).
(b) If D1≥0, −Di+Ai1D1A11<0, then
(c) If D1≥0, −Di+Ai1D1A11≥0, then
Proof. By Itô's formula, we have
where
Case(i): D1<0. Then limt→+∞X1(t)=0 a.s. Hence, for ∀ϵ∈(0,Di2) and t≫1,
So, limt→+∞Xi(t)=0 a.s. (i=2,3).
Case(ii): D1≥0. Then,
Consider the following SDDE:
Then, Xi(t)≤~Xi(t) a.s. (i=2,3). By Itô's formula,
In view of Lemma 2.1, we obtain:
(1)† If D1≥0, −Di+Ai1D1A11<0, then limt→+∞~Xi(t)=0 a.s. (i=2,3).
(2)† If D1≥0, −Di+Ai1D1A11≥0, then
Therefore, for arbitrary constant γ>0,
Based on (2.10) and system (2.7), for i=2,3,
Thanks to Lemma 2.1, we obtain:
(1)‡ If D1≥0, −Di+Ai1D1A11<0, then limt→+∞Xi(t)=0 a.s. (i=2,3).
(2)‡ If D1≥0, −Di+Ai1D1A11≥0, then
Therefore, the desired assertion (b) follows from combining (2.9) with (1)‡, and (c) follows from combining (2.9) with (2)‡.
Lemma 2.3. For system (1.3), lim supt→+∞t−1lnxi(t)≤0 a.s. (i=1,2,3).
Proof. Thanks to Lemma 2.2 and (2.7), system (2.6) satisfies limt→+∞t−1lnXi(t)=0 a.s. (i=1,2,3). From the stochastic comparison theorem, we obtain the desired assertion.
Theorem 2.2. For system (1.3):
(i) If D1<0, then limt→+∞xi(t)=0 a.s. (i=1,2,3).
(ii) If D1≥0, MΘ233<0, MΘ322<0, then
(iii) If D1≥0, MΘ13≤0, Θ3<0, MΘ233>0, then
(iv) If Θ1>0, Θ2>0, Θ3>0, MΘ11>0, then
(v) If D1≥0, MΘ12≥0, Θ2<0, MΘ322<0, then
(vi) If D1≥0, MΘ12≥0, Θ2<0, MΘ322≥0, then
Proof. According to (2.10), for arbitrary constant γ>0,
By Itô's formula and (2.11), we derive
Case(i): D1<0. From Lemma 2.2 (a), limt→+∞xi(t)=0 a.s. (i=1,2,3).
Case(ii): D1≥0, MΘ233<0, MΘ322<0. Based on system (2.12), for ∀ϵ∈(0,1) and t≫1,
By Lemma 2.1 and the arbitrariness of ϵ, we obtain
Hence, for ∀ϵ∈(0,1) and t≫1,
According to Lemma 2.1 and the arbitrariness of ϵ, we have
Based on (2.16) and system (2.12), for ∀ϵ∈(0,1) and t≫1,
In view of Lemma 2.1 and the arbitrariness of ϵ, we obtain
Case(iii): D1≥0, MΘ13≤0, Θ3<0, MΘ233>0. Compute
By Lemma 2.3, for ∀ϵ∈(0,1) and t≫1,
From Lemma 2.1 and the arbitrariness of ϵ, we deduce
By (2.20) and system (2.12), for ∀ϵ∈(0,1) and t≫1,
According to (2.21), for ∀ϵ∈(0,1) and t≫1,
Thanks to Lemma 2.3, for ∀ϵ∈(0,1) and t≫1,
In view of Lemma 2.1 and the arbitrariness of ϵ, we obtain
Thanks to (2.21) and (2.22b), for ∀ϵ∈(0,1) and t≫1,
Based on Lemma 2.1 and the arbitrariness of ϵ, we obtain
Combining (2.22-1) with (2.24) yields
From (2.21) and (2.25), for ∀ϵ∈(0,1) and t≫1,
Thanks to Lemma 2.1 and the arbitrariness of ϵ, we have
Combining (2.22-2) with (2.27) yields
Case(iv): Θ1>0, Θ2>0, Θ3>0, MΘ11>0. According to Lemma 2.1, (2.19) and the arbitrariness of ϵ, we obtain
In view of system (2.12), we compute
Then, for ∀ϵ∈(0,1) and t≫1,
According to Lemma 2.1 and the arbitrariness of ϵ, we deduce
Therefore, for ∀ϵ∈(0,1) and t≫1,
Based on (2.31) and system (2.12), for ∀ϵ∈(0,1) and t≫1,
Thanks to Lemma 2.1 and the arbitrariness of ϵ, we obtain
Combining (2.30-1) with (2.33) yields
According to (2.31), (2.33) and system (2.12), for ∀ϵ∈(0,1) and t≫1,
From Lemma 2.1 and the arbitrariness of ϵ, we have
Combining (2.30-2) with (2.36) yields
Similarly, for ∀ϵ∈(0,1) and t≫1,
Thanks to Lemma 2.1 and the arbitrariness of ϵ, we obtain
Combining (2.29) with (2.39) yields
Case(v): D1≥0, MΘ12≥0, Θ2<0, MΘ322<0. By Lemma 2.1, (2.30) and the arbitrariness of ϵ, we have
Hence, for ∀ϵ∈(0,1) and t≫1,
Based on (2.42), we deduce
Therefore, for ∀ϵ∈(0,1) and t≫1,
In view of Lemma 2.1 and the arbitrariness of ϵ, we deduce
According to (2.42) and (2.45), for ∀ϵ∈(0,1) and t≫1,
Clearly, (2.20) is true. From (2.20) and (2.42), for ∀ϵ∈(0,1) and t≫1,
In view of Lemma 2.1 and the arbitrariness of ϵ, we obtain (2.18).
Case(vi): D1≥0, MΘ12≥0, Θ2<0, MΘ322≥0. Thanks to Lemma 2.1, (2.46) and the arbitrariness of ϵ,
According to (2.42) and (2.48), for ∀ϵ∈(0,1) and t≫1,
Based on Lemma 2.1 and the arbitrariness of ϵ, we have
Combining (2.45) with (2.50) yields
By (2.42) and (2.50), for ∀ϵ∈(0,1) and t≫1,
Thanks to Lemma 2.1 and the arbitrariness of ϵ, we deduce
Combining (2.48) with (2.53) yields
The proof is complete.
3.
Global attractivity
Theorem 3.1. Assume that 2ajj>∑3i=1Aij (j=1,2,3). Let X(t;ϕ)=:(x1(t;ϕ),x2(t;ϕ),x3(t;ϕ))T be the solution to system (1.3) with initial condition ϕ∈C([−τ,0],R3+). Then, for any ϕ and ϕ∗∈C([−τ,0],R3+),
Proof. We only need to show
Define
From Itô's formula, we derive
According to (3.3), we have
which implies
Define Gi(t)=E[|xi(t;ϕ∗)−xi(t;ϕ)|] (i=1,2,3). Then,
Based on Hölder's inequality, for t2>t1 and p>1,
In view of Theorem 7.1 in [14], for p \geq 2 , we obtain
From Hölder's inequality, we derive
According to Hölder's inequality, we get
Based on (3.6)-(3.9), for p \geq 2 and |t_{2}-t_{1}| \leq \delta ,
where
Combining (3.5) with (3.10) yields
Therefore, (3.2) follows from (3.4), (3.11) and Barbalat's conclusion in [15].
4.
Numerical simulations
In this section we provide some numerical simulations to show the effectiveness of our main theoretical results by using the Milstein approach mentioned in [16]. Let \tau_{ji} = \ln 2 , \mu_{ji}(\theta) = \mu_{ji}{\mathrm{e}}^{\theta} . Denote
4.1. Example 1
Let
subject to x_{1}(\theta) = 0.7 {\mathrm{e}}^{\theta} , x_{2}(\theta) = 0.6 {\mathrm{e}}^{\theta} , x_{3}(\theta) = 0.5 {\mathrm{e}}^{\theta} , \theta \in [-\ln 2, 0] .
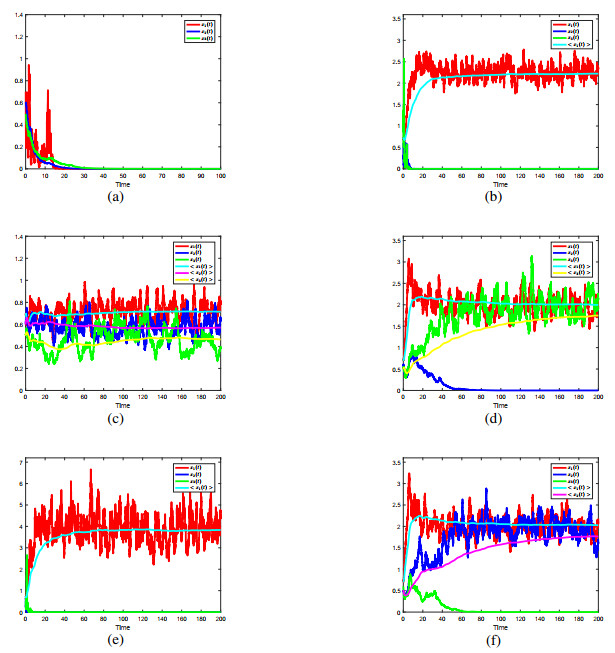
\bf{Case \, \, 1.} \sigma_{1} = 1.4 , \sigma_{2} = 0.1 , \sigma_{3} = 0.1 . Then, D_{1} = -0.08 . By Theorem 2.2 \mathfrak{(i)} , all three species are extinctive. See Figure 1(a).
\bf{Case \, \, 2.} \sigma_{1} = 0.1 , \sigma_{2} = 2.0 , \sigma_{3} = 1.9 . Then, D_{1} = 0.895 , M_{33}^{\Theta_{2}} = -0.0745 , M_{22}^{\Theta_{3}} = -0.046 . From Theorem 2.2 \mathfrak{(ii)} , x_{1}(t) is persistent in mean, while x_{2}(t) and x_{3}(t) are extinctive. See Figure 1(b).
\bf{Case \, \, 3.} \sigma_{1} = 0.1 , \sigma_{2} = 0.1 , \sigma_{3} = 0.1 . Then, \Theta = 0.225 , \Theta_{1} = 0.16225 , \Theta_{2} = 0.13375 , \Theta_{3} = 0.09825 , M_{11}^{\Theta} = 0.14 . In view of Theorem 2.2 \mathfrak{(iv)} , all three species are persistent in mean. See Figure 1(c).
4.2. Example 2
Let
subject to x_{1}(\theta) = 0.7 {\mathrm{e}}^{\theta} , x_{2}(\theta) = 0.6 {\mathrm{e}}^{\theta} , x_{3}(\theta) = 0.5 {\mathrm{e}}^{\theta} , \theta \in [-\ln 2, 0] .
\bf{Case \, \, 4.} \sigma_{1} = 0.1 , \sigma_{2} = 0.1 , \sigma_{3} = 0.1 . Then, \Theta = 0.001 , D_{1} = 0.795 , M_{12}^{\Theta} = 0.01 , \Theta_{2} = -0.00975 , M_{22}^{\Theta_{3}} = 0.1975 . According to Theorem 2.2 \mathfrak{(vi)} , both x_{1}(t) and x_{3}(t) are persistent in mean, while x_{2}(t) is extinctive. See Figure 1(d).
\bf{Case \, \, 5.} \sigma_{1} = 0.2 , \sigma_{2} = 1.8 , \sigma_{3} = 1.5 . Then, \Theta = 0.001 , D_{1} = 0.78 , M_{12}^{\Theta} = 0.01 , \Theta_{2} = -0.0143 , M_{22}^{\Theta_{3}} = -0.031 . Based on Theorem 2.2 \mathfrak{(v)} , x_{1}(t) is persistent in mean, while both x_{2}(t) and x_{3}(t) are extinctive. See Figure 1(e).
4.3. Example 3
Let
subject to x_{1}(\theta) = 0.7 {\mathrm{e}}^{\theta} , x_{2}(\theta) = 0.5 {\mathrm{e}}^{\theta} , x_{3}(\theta) = 0.6 {\mathrm{e}}^{\theta} , \theta \in [-\ln 2, 0] .
\bf{Case \, \, 6.} \sigma_{1} = 0.1 , \sigma_{2} = 0.1 , \sigma_{3} = 0.1 . Then, \Theta = 0.001 , D_{1} = 0.795 , M_{13}^{\Theta} = -0.01 , \Theta_{3} = -0.00975 , M_{33}^{\Theta_{2}} = 0.1975 . Thanks to Theorem 2.2 \mathfrak{(iii)} , both x_{1}(t) and x_{2}(t) are persistent in mean, while x_{3}(t) is extinctive. See Figure 1(f).
All mentioned above can be confirmed by Figure 1.
Acknowledgement
The work is supported by National Natural Science Foundation of China (No.11901166).
Conflict of interest
The authors declare that there are no conflicts of interest.









 DownLoad:
DownLoad:


