Persistence and periodicity of survival red blood cells model with time-varying delays and impulses

1.   Introduction

The scalar delay differential equation

where $\alpha, \beta, \gamma, \tau\in \mathbb{R}_+,$ was proposed by Wazewska-Czyzewska and Lasota ^[1] as an appropriate model to describe the survival of red blood cells in an animal. In this model, it is assumed that the cells are lost from the circulation at a rate $\alpha$, $N(t)$ denotes the density of mature red blood cells in blood circulation at time $t,$ $\beta$ and $\gamma$ denote the production of red blood cells per unit time and $\tau$ is the time delay between the production of immature cells in the bone marrow and their maturation for release in circulating bloodstreams. More detailed information about system (1.1) can be found in ^{[2,3,4,5,6,7,8]}, where ^[2,3,4,5,6] deals with the periodic solutions for system, ^[7] deals with the automorphic solutions for system, ^[8] deals with the global attractivity, and ^[9,10,11] deals with the dynamics of discrete case.

The variation of the environment plays an important role in many biological and ecological dynamical systems. Thus, the system parameters are not fixed constants and often vary within a certain range and the assumption of parameters fluctuation in the system is necessary. In particular, due to the effects of a periodically varying environment such as seasonal fluctuations, the system parameters often exhibit periodicity. As pointed out by Nicholson ^[12] that any periodic change of climate tends to impose its period upon oscillations of internal origin or to cause such oscillations to have a harmonic relation to periodic climatic changes. Hence, it is more realistic to consider the nonautonomous case of system (1.1) as follows (^[13,14,15]):

or its special and extensive cases (^{[2,3,6,16,17]}):

where $\alpha, \beta, \beta_i, \gamma, \gamma_i, \tau, \tau_i$ are all positive $\omega$-periodic functions, $\omega$ is a positive constant and $m$ is a non-negative integer. In particular, Li and Wang ^[14] studied the existence and global attractivity of positive periodic solutions of system (1.2) by employing the continuation theorem developed by Gaines and Mawhim ^[18]. However, these results can only be applied to system (1.2) when $0 < \alpha(t) < 1$ and $\dot{\tau}(t)\leq1,$ which is too restrictive in real applications.

In ^[16], Saker and Agarwal investigated the oscillation and global attractivity of system (1.3) when $\gamma(t) = \gamma,$ where $\gamma$ is a real constant, and obtained that system (1.3) has a unique positive $\omega$-periodic solution if

Obviously, it leads to

where $\alpha^I, \beta^I$ denote the minimum values of $\alpha(t)$ and $\beta(t),$ respectively. It implies that the criteria in ^[16] are only valid for the special time delay $\tau = m\omega,$ where $m\omega$ satisfies the inequality (1.5).

In ^[17], Liu et al. investigated the existence and global attractivity of unique positive periodic solution of system (1.4) and obtained that system (1.4) has a unique positive $\omega$-periodic solution if $Mpq\leq1,$ where

Obviously, it leads to

which implies that the criteria in ^[17] are only valid for some special periodic constants $\omega$ and $m$. Hence, techniques and methods for dynamical analysis of red blood cells models (1.2)-(1.4) should be further developed and explored.

Recently, dynamical analysis of impulsive nonlinear systems has attracted the attention of many researchers ^{[19,20,21,22,23,24,25,26,27,28,29]}. For instance, based on the concept of periodic time scales, Wang ^[19] studied the periodic solution for a new type of neutral impulsive stochastic Lasota-Wazewska model. Modeling by Fractional Mathematics, Stamov ^[20] investigated uncertain impulsive fractional order Lasota-Wazewska model on the survival of red blood cells. In addition, taking into account the effects of both delays and impulses such as weather change, resource availability, food supplies, etc, Yan ^[23] considered the following red blood cells model with impulsive effects:

where $b_k > -1$ denotes the possible measure of an impulsive effect on cell $x$ at time $t_k, k\in \mathbb{Z}_+.$ The author obtained some sufficient conditions for existence and global attractivity of positive periodic solution of system (1.6) under the assumption that

Then Liu and Takeuchi ^[24] pointed out that the $\omega$-periodicity of $\Gamma$ in ^[23] implies that $\Gamma(\omega) = 1$ which is a more restrictive condition and so some new sufficient conditions were derived in ^[24] for the existence and global attractivity of positive periodic solution of system (1.6), which removed the restriction that $\Gamma(\omega) = 1$ and extended and improved the results in ^[23]. Unfortunately, one may observe that the methods used in ^{[21,22,23,24]} are only valid for red blood cells models with time-invariant delays ^[21,22] or some special time delays ^[23,24], i.e., $\tau_i = m_i\omega.$ In other words, it is necessary that $\frac{\tau_i}{\omega}\in \mathbb{Z}_+.$

Motivated by the above discussions, our aim in this paper is to study the dynamics of the following red blood cells model with time-varying delays and impulsive effects:

The paper is organized as follows. In Section 2, we introduce some necessary notations, definitions and prove that the solutions are positive and ultimately bounded. In Section 3, we present some results on persistence of system (1.7) based on those ultimately bounded conditions. In our model the persistence describes the persistent survival of the mature red blood cells under delay and impulsive perturbations. In Section 4, some sufficient conditions ensuring the existence and global attractivity of unique positive periodic solution are presented under the assumption that the coefficients in the model are $\omega$-periodic, which are suitable for Lasota-Wazewska model with any $\omega\in \mathbb{R}_+.$ These criteria describe the nonexistence of any dynamic diseases in the mammal. Two examples and their computer simulations are offered to show the effectiveness and advantages of our new results in Section 5. Finally, we draw conclusions in Section 6.

2.   Preliminaries

Notations. Let $\mathbb{R}$ denotes the set of real numbers, $\mathbb{R}_{+}$ the set of positive real numbers and $\mathbb{Z}_+$ the set of positive integers. $[\bullet]^\ast$ denotes the integer function. $\Lambda = \{1, 2, \cdots, m\}.$ For any interval $J\subseteq \mathbb{R}$, set $S\subseteq \mathbb{R}^k (1\leq k\leq N), C(J, S) = \{\varphi:J\rightarrow\ S$ is continuous$\}$ and $PC(J, S) = \{\varphi:J\rightarrow\ S$ is continuous everywhere except at finite number of points $t$, at which $\varphi(t^{+})$, $\varphi(t^{-})$ exist and $\varphi(t^{+}) = \varphi(t)\}.$ In particular, let $\mathbb{PC}_\tau$ be an open set in $PC([-\tau, 0], \mathbb{R}_+).$ Given a continuous function $f$ which is defined on $J\in \mathbb{R},$ we set

Consider the red blood cells model (1.7) with initial value:

where $\phi \in \mathbb{PC}_\tau, \ 0\leq \tau_{i}(t)\leq \tau, i\in\Lambda,$ where $\tau$ is a given constant. For each $t\geq t_0, \ x_t\in \mathbb{PC}_\tau$ is defined by $x_t(s) = x(t+s), s\in [-\tau, 0].$

In this paper we need the following assumptions:

$(H_1)$ $\alpha, \beta_i$ and $\gamma_i: \mathbb{R}_+\rightarrow\mathbb{R}_+, i\in\Lambda,$ are all continuous functions with positive lower and upper bounds.

$(H_2)$ The impulse times $t_{k}, k\in \mathbb{Z}_+,$ satisfy $0\leq t_{0} < t_{1} < \ldots < t_{k} \rightarrow +\infty$ as $k\rightarrow+\infty.$

$(H_3)$ $I_{k}: \mathbb{R}_+\times \mathbb{R}_+\rightarrow\mathbb{R}_+$ are continuous functions which satisfies $\rho^I_{k}u\leq I_{k}(t, u) \leq \rho^S_{k}u, \ u\in \mathbb{R}_+, \ t\in \mathbb{R}_+, \ k\in \mathbb{Z}_+,$ where $\rho^I_{k}$ and $\rho^S_{k}$ are some positive constants.

Definition 2.1. System (2.1) is said to be persistent, if there exist constants $M > 0$ and $m > 0$ such that each positive solution $x(t)$ of model (2.1) satisfies

Definition 2.2. A map $x: \mathbb{R}_+\rightarrow \mathbb{R}_+$ is said to be an $\omega$-periodic solution of system (2.1), if

(1) $x(t)$ is a piecewise continuous map with first-class discontinuity points and satisfies (1);

(2) $x(t)$ satisfies $x(t+\omega) = x(t), t\neq t_k$ and $x(t_k+\omega^{+}) = x(t_k^{+}), k\in \mathbb{Z}_+.$

Definition 2.3. Let $x^\ast = x^\ast(t, t_0, \phi^\ast)$ be a solution of system (2.1) with initial value $(t_0, \phi^\ast),$ where $\phi^\ast \in \mathbb{PC}_\tau.$ Then the solution $x^\ast$ is said to be a positive stationary oscillation of system (2.1), if

(1) $x^\ast$ is the unique positive $\omega$-periodic solution of system (2.1);

(2) For any other solution $x = x(t, t_0, \phi)$ of system (2.1) through $(t_0, \phi)$, it holds that

To derive the main results, we need to introduce some Lemmas and their Corollaries.

Lemma 2.1. $\mathbb{R}_+$ is the positively invariant set of system (2.1).

Proof. Let $x(t) = x(t, t_0, \phi)$ be a solution of system (2.1) with initial value $(t_0, \phi),$ where $\phi \in \mathbb{PC}_\tau.$ First, we prove that $x(t) > 0$ for $t\in [t_0, t_1).$ Suppose on the contrary, in view of the continuous of $x$ on interval $[t_0, t_1)$ and $\phi(0) > 0,$ then there exists a $\hat{t}\in [t_0, t_1)$ such that $x(\hat{t}) = 0$ and $x(t) > 0$ for $t\in [t_0, \hat{t}).$ Thus it follows from system (2.1) that

which leads to

where

Since $x$ is continuous on interval $[t_0, t_1),$ it can be deduced that

Obviously, this is a contradiction and so $x(t) > 0$ for $t\in [t_0, t_1).$ Note that $x(t_1) = I_{1}(x(t^{-}_1)) > 0,$ we can similarly prove that $x(t) > 0$ for $t\in [t_1, t_2).$ In this way, it can be finally deduced that $x(t) > 0$ for $t\in [t_0, \infty).$ The proof is complete.

Lemma 2.2. (^[30]) Assume that there exist functions $m\in PC(\mathbb{R}_+, \mathbb{R}_+), p, q\in C(\mathbb{R}_+, \mathbb{R})$ and constants $d_k\geq0$ such that

Then

Remark 2.1. It should be noted that the above assertion still holds if the sign $''\leq''$ in Lemma 2.2 are replaced by $''\geq''.$

Lemma 2.3. Assume that $(H_1)-(H_3)$ hold. If there exist some real constants $\eta_1 > 0, \eta_2 > 0, \theta_1\geq0$ and $\theta_2\geq0$ such that

for any $t_0\leq s\leq t$, , where

Then the set $\Omega = \{x\in \mathbb{R}_+:\ 0 < m\leq x \leq M \}$ is the ultimately bounded set of system (2.1), where $M$ and $m$ are any positive constants that satisfy

Proof. Let $x(t) = x(t, t_0, \phi)$ be a solution of system (2.1) with initial value $(t_0, \phi),$ where $\phi \in \mathbb{PC}_\tau.$ By Lemma 2.1, we know that $x(t) > 0$ for $t\in [t_0, \infty).$ Then it follows from system (2.1) that

By Lemma 2.2 and (2.2), it can be deduced that

which implies that there exists a constant $T_1\geq t_0$ such that $x(t) \leq M, \ t\geq T_1.$

Next we show that there exists a constant $T_2\geq T_1+\tau$ such that $m\leq x(t), \ t\geq T_2.$ First, from system (2.1) we know that

Without loss of generality, one may suppose that $T_1+\tau \neq t_k, k\in \mathbb{Z}_+.$ Then by Remark 2.1 and (2.2), it can be deduced that

where $\nu_i = \exp(-\gamma^S_i M)$ which implies that there exists a constant $T_2\geq T_1+\tau$ such that $m\leq x(t), \ t\geq T_2.$ The proof is therefore complete.

Suppose that

then the following result can be derived.

Corollary 2.1. Assume that $(H_1)-(H_3)$ hold. If there exists a constant $\mu > 0$ such that $t_k-t_{k-1}\geq\mu > \frac{\ln \rho^S}{\alpha^I}, k\in \mathbb{Z}_+.$ Then the set $\Omega = \{x\in \mathbb{R}_+:\ 0 < m\leq x \leq M \}$ is the ultimately bounded set of system (2.1), where $M$ and $m$ are any real constants that satisfy

Proof. For any given $t_0\leq s\leq t,$ if there exist some impulsive points on the interval $[s, t],$ then assume without loss of generality that $t_m\leq s < t_{m+1} < \cdots < t_{m+j}\leq t < t_{m+j+1},$ where $t_{m+k}, k = 1, \cdots, j,$ are the impulsive points on the interval $[s, t].$ Then note that $t_k-t_{k-1}\geq\mu,$ one may derive that $t-s\geq t_{m+j}-t_{m+1}\geq (j-1)\mu,$ which implies that $\frac{t-s}{\mu}+1\geq j.$ In this case, it can be deduced from the definition of $\Psi_1$ and $\Psi_2$ that

Obviously, if there is no impulsive point on the interval $[s, t],$ the above assertions also hold. Hence, let $\eta_1 = { } \alpha^I- \frac{\ln\rho^S}{\mu}, \eta_2 = \alpha^S- \frac{\ln\rho^I}{\mu}, \theta_1 = \ln\rho^S$ and $\theta_2 = -\ln\rho^I$ and by Lemma 2.3, we can obtain Corollary 2.1.

If

then we have

Corollary 2.2. Assume that $(H_1)-(H_3)$ hold. If there exists a constant $\mu > 0$ such that $t_k-t_{k-1}\geq\mu > \frac{\ln \rho^S}{\alpha^I}, k\in \mathbb{Z}_+.$ Then the set $\Omega = \{x\in \mathbb{R}_+:\ 0 < m\leq x \leq M \}$ is the ultimately bounded set of system (2.1), where $M$ and $m$ are any real constants that satisfy

Proof. The proof is similar to Corollary 2.1 and we only need notice that

which implies that $\eta_2 = \alpha^S$ and $\theta_2 = 0.$

In addition, if

then we have

Corollary 2.3. Assume that $(H_1)-(H_3)$ hold. Then the set $\Omega = \{x\in \mathbb{R}_+:\ 0 < m\leq x \leq M \}$ is the ultimately bounded set of system (2.1), where $M$ and $m$ are any real constants that satisfy

Proof. Notice that

which implies that $\eta_1 = \alpha^I$ and $\theta_1 = 0.$ By the proof of Corollary 2.1, we can obtain the above result.

In particular, when there is no impulsive effects, i.e., $I_{k}(t, u) = u,$ the following result can be directly derived by Corollary 2.3.

Corollary 2.4. Assume that $(H_1)$ and $(H_2)$ hold. Then the set $\Omega = \{x\in \mathbb{R}_+:\ 0 < m\leq x \leq M \}$ is the ultimately bounded set of system (2.1), where $M$ and $m$ are any real constants that satisfy

3.   Persistence of Lasota-Wazewska model

We are now in a position to state our main results on persistence of system (2.1).

Theorem 3.1. Assume that $(H_1)-(H_3)$ hold. Then system (2.1) is persistent if there exist some constants $\eta_1 > 0, \eta_2 > 0, \theta_1\geq0$ and $\theta_2\geq0$ such that

for any $t_0\leq s\leq t$, where

Corollary 3.1. Assume that $(H_1)-(H_3)$ and (2.3) hold. Then system (2.1) is persistent if there exists a constant $\mu > 0$ such that $t_k-t_{k-1}\geq\mu > \frac{\ln \rho^S}{\alpha^I}, k\in \mathbb{Z}_+.$

Corollary 3.2. Assume that $(H_1)-(H_3)$ and (2.4) hold. Then system (2.1) is persistent if there exists a constant $\mu > 0$ such that $t_k-t_{k-1}\geq\mu > \frac{\ln \rho^S}{\alpha^I}, k\in \mathbb{Z}_+.$

Corollary 3.3. Assume that $(H_1)-(H_3)$ and (2.5) hold, then system (2.1) is persistent.

Corollary 3.4. Assume that $(H_1)$ holds, then system (2.1) without impulsive effects is persistent.

Remark 3.1. Based on the results (Lemma 2.3 and Corollaries 2.1–2.3) in Section 2, the above conclusions can be obtained easily and the detailed proofs are omitted here.

Remark 3.2. One may observe from Corollaries 3.1–3.3 that there exists a necessary restriction on the lower bound of impulsive intervals $[t_{k-1}, t_k)$ to guarantee the persistence when $\rho^S > 1.$ But the restriction can be removed when $\rho^S\leq1.$ The ideas behind it is that the encountered impulsive perturbation can be large enough provided the impulsive intervals are larger than a special value which is related to the perturbation scopes. But the restriction on impulsive intervals can be removed when the impulsive perturbation is small.

4.   Periodicity of Lasota-Wazewska model

In this section, we shall investigate the stationary oscillation of system (2.1). First, to derive the results we need introduce some assumptions that are more restrictive than $(H_1)-(H_3)$ as follows:

$(P_1)$ $\alpha, \beta_i, \gamma_i$ and $\tau_i: \mathbb{R}_+\rightarrow\mathbb{R}_+, i\in\Lambda,$ are all positive continuous $\omega$-periodic functions, where $\omega > 0$ is a real constant.

$(P_2)$ $I_{k}(t, u) = \rho_k u, u\in \mathbb{R}_+, k\in \mathbb{Z}_+.$

$(P_3)$ For given $\omega > 0$, there exists an integer $q\in \mathbb{Z}_+$ such that $t_k+\omega = t_{k+q}$ and $\rho_{k+q} = \rho_{k}, k\in \mathbb{Z}_+.$

Lemma 4.1.(^[31]) Assume that $(P_1)-(P_3)$ hold. Then system (2.1) has an $\omega$-periodic solution if there exists a $\phi\in \mathbb{PC}_\tau$ such that $x_{t_0+\omega}(t_0, \phi) = \phi,$ where $x(t, t_0, \phi)$ is the solution of system (2.1) through $(t_0, \phi).$

Theorem 4.1. Assume that $(P_1)-(P_3)$ hold. Then system (2.1) admits a positive stationary oscillation if there exist constants $\mathbb{M} > 0, \delta\geq0$ such that

and

Proof. First, we prove that the following inequality holds:

In fact, let $E = e^{-\gamma_i(t)},$ then it holds that $|e^{-\gamma_i(t)u}-e^{-\gamma_i(t)v}| = |E^{u}-E^{v}| = E^{\xi}|\ln E||u-v|\leq E^{\xi}\gamma^S_i|u-v|,$ where $\xi$ is a real value between $u$ and $v.$ Since $u, v\in \mathbb{R}_+,$ we know that $\xi > 0,$ which implies that (4.3) holds.

Let $x = x(t, t_0, \phi)$ and $y = y(t, t_0, \varphi)$ be two arbitrary solutions of system (2.1) with initial values $(t_0, \phi)$ and $(t_0, \varphi),$ respectively, where $\phi, \varphi\in \mathbb{PC}_\tau.$ Consider an auxiliary function $V(t) = |x-y|.$ Obviously, $V\in PC(\mathbb{R}, \mathbb{R}_+).$ Calculating the upper right derivative of function $V,$ it can be deduced from (4.3) that

where $V_\tau(t) = \sup_{t-\tau\leq s \leq t }V(s).$

On the other hand, it follows from $(P_2)$ that

From (4.1)–(4.5) and using the Lemma 2.1 in ^[32], we get

where $\lambda > 0$ satisfies $\lambda < \alpha^I-{ }\sum\limits_{i = 1}^m \beta_i^S\gamma^S_i e^{\lambda\tau}.$

By (4.2), one may choose a ${\varepsilon} > 0$ small enough such that

Choose $\lambda = \delta+{\varepsilon},$ then (4.6) becomes

i.e.,

Thus there exists a $T\geq t_0$ such that

Define an operator

Obviously, operator $\mathscr{F}$ maps the set $\mathbb{PC}_\tau$ into itself. By induction, it can be deduced that

Let $k$ large enough such that $t_0+k\omega-2\tau\geq T,$ then it follows from (4.7) that

Hence, operator $\mathscr{F}$ is a contraction mapping in Banach space $\mathbb{PC}_\tau.$ Using Banach fixed point theorem, there exists a unique $\phi^\star\in\mathbb{PC}_\tau$ such that $\mathscr{F}\phi^\star = \phi^\star.$ By Lemma 4.1, we know that system (2.1) has a positive $\omega$-periodic solution $x(t, t_0, \phi^\star).$

Furthermore, we show that $x(t) = x(t, t_0, \phi^\star)$ is the unique $\omega$-periodic solution of system (2.1) and all other solutions converge exponentially to it. Suppose on the contrary that there exists another $\omega$-periodic solution $y(t) = y(t, t_0, \varphi^\star)$ where $\varphi^\star\in \mathbb{PC}_\tau.$ Then similar to the proof of (4.7), we get that for $t\geq 0$

which implies that $x(t)\equiv y(t), \ t\geq0.$ Hence, $x(t)$ is the unique positive $\omega$-periodic solution of system (2.1) and all other solutions converge exponentially to it, i.e., system (2.1) admits a positive stationary oscillation. The proof is thus complete.

If

then we have

Corollary 4.1. Assume that $(P_1)-(P_3)$ hold. Then system (2.1) admits a positive stationary oscillation if there exist constants $\mu > 0, \delta > 0$ such that $t_k-t_{k-1}\geq\mu > \frac{\ln \rho^S}{\delta}, k\in \mathbb{Z}_+$ and

Proof. Notice that $\max\{1, \ \ \rho_k \}\leq \rho^S, \ k\in \mathbb{Z}_+$ and $t_n-t_0\geq n\mu, n\in \mathbb{Z}_+,$ by Theorem 4.1 we can obtain the above result.

Remark 4.1. Compared Corollary 4.1 with Corollaries 3.1 and 3.2, one may observe that $t_k-t_{k-1}\geq\mu > \frac{\ln \rho^S}{\delta} > \frac{\ln \rho^S}{\alpha^I}$ implies that more restrictive condition on impulsive interval is needed to guarantee the existence of stationary oscillation.

In addition, if

then we have

Corollary 4.2. Assume that $(P_1)-(P_3)$ hold. Then system (2.1) admits a positive stationary oscillation if $\ \alpha^I > { }\sum\limits_{i = 1}^m \beta_i^S\gamma^S_i.$

Proof. Notice that $\max\{1, \ \ \rho_k \}\leq 1, \ k\in \mathbb{Z}_+$ and let $\mathbb{M} = 1, \delta = 0,$ by Theorem 4.1 we can obtain the above result.

Corollary 4.3. Assume that $(P_1)$ holds, then system (2.1) without impulsive effects admits a positive stationary oscillation if $\ \alpha^I > { }\sum\limits_{i = 1}^m \beta_i^S\gamma^S_i.$

Remark 4.2. In ^[15,17,18], the authors investigated the stationary oscillation of system (2.1) with/without impulsive effects under the assumption that $\tau(t)$ is a constant delay that satisfies $\frac{\tau}{\omega}\in \mathbb{Z}_+.$ Note in our results, the restriction is completely removed and the time-varying delay $\tau(t)$ may be large enough or small enough provided that it is positive $\omega$-periodic.

Remark 4.3. When there is no impulsive effects, i.e., $\rho_k\equiv1,$ the stationary oscillation of system (2.1) has been studied by Li and Wang ^[13] under the assumptions that $0 < \alpha(t) < 1$ and $\dot{\tau}(t)\leq1$ and Liu et al. ^[16] under the assumption that $Mpq\leq1,$ where

It is obvious that those assumptions are greatly relaxed in Corollary 4.3. Moreover, one may note from Corollary 4.3 that there is nothing restriction on periodic constant $\omega.$ In other words, the development results in this paper are suitable for any $\omega\in \mathbb{R}_+.$

5.   Applications

In this section, we shall give two examples and theirs computer simulations to show the effectiveness of the proposed results.

Example 5.1. Consider a delayed red blood cells model with impulses as follows:

where $\rho > 0$ and $\tau > 0$ are some real constants.

Property 5.1. Case: $\rho > 1.$ System (5.1) is persistent if there exists a constant $\mu > 0$ such that ${ } t_{k+1}-t_k\geq \mu > \ln \rho, \ k\in \mathbb{Z}_+.$

Property 5.2. Case: $\rho\leq 1.$ System (5.1) is persistent for any impulsive sequence $\{t_k\}_{k\in \mathbb{Z}_+}$ satisfying $(H_2).$

Proof. It is easy to check that system (5.1) satisfies the conditions $(H_1)-(H_3)$ and by Corollaries 3.2 and 3.3, we can obtain the above properties, respectively.

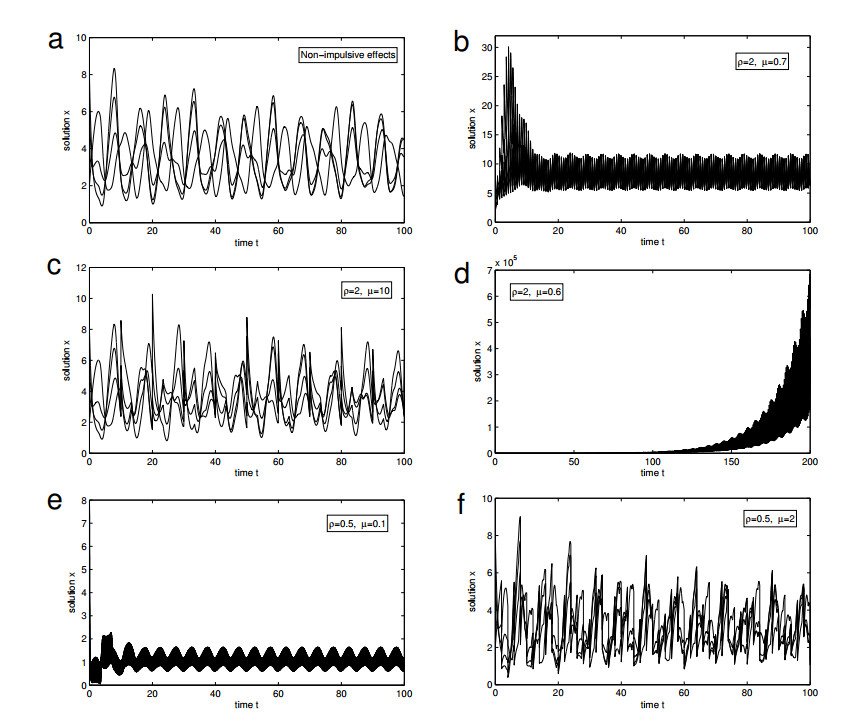
Remark 5.1. When there is no impulsive effects, i.e., $\rho = 1,$ the state trajectories of system (5.1) are given in Figure 1.$(a).$ In this case, obviously, system (5.1) is persistent. If we consider the impulsive effects such as $\rho = 2,$ then by Property 5.1, we know that system (5.1) is persistent if $t_{k+1}-t_k\geq 0.6931.$ Figure 1.$(b, c)$ show the state trajectories of system (5.1) with $t_k = 0.7k$ and $10k,$ respectively. However, when $t_k = 0.6k$ which violates the Property 5.1, it is interesting to see from Figure 1.$(d)$ that system (5.1) is non-persistent. It confirms that the proposed condition in Property 5.1 is feasible and effective to guarantee the persistence of system (5.1).

In addition, if $\rho = 0.5,$ then by Property 5.2, we know that system (5.1) is persistent for any impulsive sequence $\{t_k\}_{k\in \mathbb{Z}_+}$ in $(H_2).$ Figure 1.$(e, f)$ show the state trajectories of system (5.1) with $t_k = 0.1k$ and $2k,$ respectively.

Remark 5.2. In the simulations of Example 4.1, we choose the time delay $\tau = 3.4,$ time step $h = 0.01$ and initial values $\phi = 2m, m = 1, \cdots, 4.$

Example 5.2. Consider a simple delayed red blood cells model with impulses:

where $\tau(t) = 0.2-0.1\Big[\sin { }\frac{2\pi}{\omega} t\Big]^\ast$ and $\omega > 0, \rho > 0$ are two real constants.

Property 5.3. Case: $\rho > 1.$ System (5.2) admits a positive stationary oscillation if there exist constants $q\in \mathbb{Z}_+, \delta > 0, \mu > 0$ such that $t_k+\omega = t_{k+q}$ and

Corollary 5.1. Case: $\rho > 1.$ System (5.2) with $t_k = \mu k, k\in \mathbb{Z}_+$ admits a positive stationary oscillation if there exist constants $\delta > 0, \mu > 0$ such that

Property 5.4. Case: $\rho\leq1.$ System (5.2) admits a positive stationary oscillation if there exists a constant $q\in \mathbb{Z}_+$ such that $t_k+\omega = t_{k+q}.$

Corollary 5.2. Case: $\rho\leq1.$ System (5.2) with $t_k = \mu k, k\in \mathbb{Z}_+$ admits a positive stationary oscillation if ${ } \frac{\omega}{\mu}\in \mathbb{Z}_+.$

Proof. It is easy to check that system (5.2) satisfies the conditions $(P_1)-(P_3)$ and by Corollaries 4.1 and 4.2, we can obtain the above properties, respectively.

Remark 5.3. From Property 5.4, one may note that system (5.2) without impulsive effects admits a positive stationary oscillation for any $\omega\in \mathbb{Z}_+.$

Remark 5.4. When there is no impulsive effects, i.e., $\rho = 1,$ by Corollary 5.2, we know that system (5.2) admits a positive stationary oscillation for any $\omega > 0.$ The corresponding simulations for $\omega = 2$ and 8 are shown in Figure 2.$(a, b).$ If we consider the impulsive effects such as $\rho = 2$ or $4.8,$ then by Corollary 5.1, we know that system (5.2) admits a positive stationary oscillation if

Thus it can be deduced that system (5.2) admits a positive stationary oscillation when (I) $\omega = \rho = 2$ and $t_k = 2k;$ (II) $\omega = 8, \rho = 4.8$ and $t_k = 4k,$ which is shown in Figure 2.$(c, d).$ In addition, if $\rho = 0.8,$ then by Corollary 5.2, we know that system (5.2) $t_k = \mu k, k\in \mathbb{Z}_+$ admits a positive stationary oscillation if ${ } \frac{\omega}{\mu}\in \mathbb{Z}_+.$ Figure 2.$(e, f)$ show the state trajectories of system (5.2) with $t_k = 0.1k, w = 2$ and $t_k = 0.8k, w = 8,$ respectively. Those simulations match our development results perfectly.

Remark 5.5. In the simulations of Example 4.2, we choose the time step $h = 0.01$ and initial values $\phi = 0.2m, m = 1, \cdots, 4.$

Remark 5.6. Obviously, all of the criteria in ^[15,17,18] are invalid for system (5.2) since $\frac{\tau}{\omega}\bar{\in}\mathbb{Z}_+$. In particular, when there is no impulsive effects, i.e., $\rho = 1,$ the criteria in ^[16] can be applied to guarantee the stationary oscillation of system (5.2) under the assumption that

However, from Remark 5.3, we know that system (5.2) without impulsive effects admits a positive stationary oscillation for any $\omega \in \mathbb{R}_+.$ Thus our development results are more general than those ^{[15,16,17,18]}.

6.   Conclusions

This paper was dedicated to the dynamical analysis of survival red blood cells model with time-varying delays and impulsive effects. By use of the theory on impulsive differential equations, some sufficient conditions for the persistence have been presented. Then assuming that the coefficients in the model are common periodic, some criteria ensuring the existence-uniqueness and global attractivity of positive periodic solution were obtained, which extended and improved some recent works in the literature. Two examples and their computer simulations have been given to show the effectiveness and advantages of the results. In addition, the ideas used in this paper can be developed to study some other dynamical systems.

Acknowledgments

This work was supported by Outstanding Youth Innovation Team in Shandong Higher Education Institutions (2019KJI008).

Conflict of interest

All authors declare no conflicts of interest in this paper.