Input-to-state stability of delayed systems with bounded-delay impulses

1.   Introduction

There have been extensive studies characterizing the effects of external inputs on the dynamical behaviors of a control system, and many important notions have been proposed. One of them is the concept of input-to-state stability (ISS), proposed in ^[2], which is widely studied by numerous investigators. In past decades, substantial progress has been achieved on ISS for various systems, such as discrete systems, networked control systems and hybrid systems ^{[3,4,5,6,7,8,9,10]}.

Thousands of impulsive systems, a type of hybrid systems, have been formulated to naturally describe systems subject to abrupt changes and have attracted much attention during last decades ^{[11,12,13,14,15]}. Up to now, many interesting results on impulsive systems have been reported ^{[12,16,17,18,19,20]}. With more and more applications of impulsive systems with external inputs, the ISS concept of impulsive systems was introduced in ^[21], and further investigated in ^[1,22,23]. Recently, the ISS concept was generalized to more nonlinear systems and the effect of delayed impulses on ISS property was investigated in ^[1]. In addition, it is known that the time delay should be considered in engineering and biological control systems to describe delayed feedbacks, samplings and outputs ^[24]. Especially, more and more investigators are interested in studying the dynamic behaviors of delayed systems and numerous significant results have been derived ^{[12,25,26,27]}. Sometimes delay plays a significant role on system dynamics and false inference would be obtained if ignoring it ^[28]. Considering this aspect, impulsive control systems with delay are formulated. Correspondingly, the ISS concept has been extended and extensive studies have been reported for this type of systems ^[29,30].

It is worth noting that in many practical cases such as neural networks and biological systems, the delay may be time-varying and cannot be accurately measured, and the bound of the delay may be a priori unknown due to some uncertainties ^[13,31]. Many interesting results have been obtained for the case that both continuous dynamics and discrete dynamics incorporates delays ^[30,32], and especially, the effect of arbitrarily bounded delay in continuous dynamics has been studied, such as ^[22,33]. However, very few results have been reported for systems with bounded-delay impulses, where the delays in impulses are time-varying and arbitrarily large but bounded. Here we briefly mention some studies on ISS of nonlinear systems with delayed impulses, which are closely related to the current study. It was shown in ^[1] that for the case that delay-free continuous dynamics are ISS, original stabilizing impulses may turn to be destabilizing if delays in impulses are considered, and correspondingly, it may lead to instability of the whole system if the impulses occur too frequently. Further, ISS of systems with delays occurring in both continuous dynamics and impulses were well investigated in ^[30], and particularly, the case of bounded-delay impulses can be addressed by using the method of Lyapunov-Krasovskii functionals (see Theorem 4 in ^[30]).

Technically speaking, the construction of a valid Lyapunov-Krasovskii functional often requires certain experience. In comparison with this method, the method of Halanay-type inequality firstly proposed in ^[34], may derive tractable and concise conditions applicable in many cases (see ^[35,36]). As we know, Halanay-type inequality has been extensively developed in the past decades, and it has also been verified to be a useful technique in the stability analysis of impulsive delayed differential systems ^[13,36]. Thus, it is our belief that an insightful extension of Halanay-type inequality with delayed impulses and external inputs, for new ISS stability criteria, is worthy of investigation. In addition, for some specific delays, such as fast varying delay and discontinuous delay, it is more effective to handle by using the method of Halanay-type inequality than that of Lyapunov-Krasovskii functional ^[37]. Hence, our objective is to establish some simple ISS criteria for systems with bounded-delay impulses by applying a Halanay-type inequality with delayed impulses and external inputs. Furthermore, we note that further investigation can be performed on the effect of delayed impulses on ISS property of the system in ^[1] with the aid of new analytic methods. In particular, by using the proposed Halanay-type inequality, some tractable ISS criteria for systems with bounded-delay impulses can be established and the details will be discussed in Section 3.

We aim to analyze the ISS property of delayed systems with bounded-delay impulses, where the delays in impulses are arbitrarily large but bounded. In terms of a new Halanay-type inequality and a more relaxed assumption, some sufficient conditions under which the ISS property of the impulsive delayed system can be achieved are proposed. Compared with existing work, our obtained results further reveal the essential effect of delayed impulses on ISS property and the main contributions of this study are listed below.

$1.$ A new Halanay-type inequality involving delayed impulses and external inputs is proposed. By using this inequality, we investigate the ISS of delayed systems with bounded-delay impulses and further derive some simple ISS criteria, which are easy to check to some degree. We present an example to show that both the continuous dynamics and discrete dynamics should be ISS when concerning the effect of bounded-delay impulses.

$2.$ For the case that the continuous dynamics are ISS and impulses are stabilizing when the delays in impulses are equal to zero, it is shown that the impulses may become destabilizing if these delays become larger. More interestingly, we prove that in this case, ISS property of the whole system can be ensured for arbitrary impulsive instant sequence provided the delays in impulses are bounded, which greatly extends the conclusions in ^[1].

$3.$ Note that in existing literatures, the delays in impulses are often assumed to be constant or satisfy some restrictive conditions. In fact, the current study shows that only the boundedness of the delays in impulses is sufficient, greatly relaxing the assumptions for ISS. Compared with relevant results in ^[30], our conditions for ISS are simple and easy to check in a sense due to the avoidance of the construction of Lyapunov-Krasovskii functional.

The remainder of this paper is arranged as follows. In Section 2, the problem is formulated, and necessary notations and definitions are presented. The main results are derived in Section 3. Two numerical examples and a brief conclusion are presented in Section 4 and Section 5, respectively.

2.   Preliminaries

In this paper, let $\mathbb{R}$, $\mathbb{R}_+$ and $\mathbb{Z}_+$, stand for the set of real numbers, nonnegative real numbers and positive integers, respectively. In addition, $\mathbb{R}^n$ and $\mathbb{R}^{n\times m}$ denote the $n$-dimensional and $n\times m$-dimensional real spaces, respectively. We denote Euclidean norm by $|\cdot|$. The notation $\max \{a, b\}$ stands for the maximum of real numbers $a$ and $b$. Let $PC([a, b], \mathbb{R}^n) = \{\phi:[a, b]\rightarrow \mathbb{R}^n$ is continuous everywhere except at finite number of instants $t$, at which $\phi(t^+), \; \phi(t^-)$ exist and $\phi(t^+) = \phi(t) \}$, where $\phi(t^+) = \lim_{s\rightarrow t^+}\phi(s)$ and $\phi(t^-) = \lim_{s\rightarrow t^-}\phi(s)$, and the norm is defined by $\|\phi\|_{[a, b]} = \sup_{a\leq\theta\leq b}|\phi(\theta)|$, where $a, b \in \mathbb{R}$, $a < b$. Further, denote by $PC([a, \infty), \mathbb{R}^n)$ the set of functions $\psi:[a, \infty)\rightarrow \mathbb{R}^n$ such that $\psi|_{[a, b]}\in PC([a, b], \mathbb{R}^n)$ for all $b > a$, where $\psi|_{[a, b]}$ is a restriction of $\psi$ on interval $[a, b]$. For convenience, let $\|\phi\|_\nu$ denote $\|\phi\|_{[-\nu, 0]}$, for $\phi\in PC([-\nu, 0 ], \mathbb{R}^n)$ and given $\nu > 0$. Suppose that $x\in PC([-\nu, \infty), \mathbb{R}^n)$, and for every $t\geq t_0$, we define $x_t\in PC([-h, 0 ], \mathbb{R}^n)$ by $x_t(s): = x(t+s)$ for $-h\leq s\leq 0$; $x_{t^-}\in PC([-\tau, 0 ], \mathbb{R}^n)$ is defined as $x_{t^-}(s) = x((t+s)^-)$, for $s\in[-\tau, 0]$, where $\nu = \max\{h, \tau\}$.

Consider following impulsive delayed system:

where $x(t)$ is the system state and its right-hand derivative is denoted by $\dot{x}(t)$; $u\in PC([t_0, \infty), \mathbb{R}^m)$ is the locally bounded external input; $\phi\in PC([-\nu, 0 ], \mathbb{R}^n)$ is the initial condition. Denote $f: \mathbb{R}_+\times PC([-h, 0 ], \mathbb{R}^n)\times \mathbb{R}^m\rightarrow \mathbb{R}^n$ and $g:\; \mathbb{R}_+\times PC([-\tau, 0 ], \mathbb{R}^n)\times \mathbb{R}^m\rightarrow \mathbb{R}^n$, which satisfies $f(t, 0, 0) = g(t, 0, 0) = 0$. One may observe that the delay bounds in continuous dynamics and impulses are different, and it is of significance to investigate the effects of these two type of delays, respectively (see ^[32]). Particularly, $Zeno$ phenomenon means that there exist an accumulation point by which an infinite number of impulses persistently occur. To avoid $Zeno$ phenomenon, we denote by $t_0$ the initial instant, and assume that impulsive instant sequence $\{t_k\}$ satisfies $0\leq t_0 < t_1 < \cdots < t_k\rightarrow +\infty$, as $k\rightarrow +\infty$. Given $u(t)\in PC([t_0, \infty), \mathbb{R}^m)$, define $f^*(t, \psi) = f(t, \psi, u(t))$ and suppose $f^*$ meets all the necessary conditions in ^[38] to guarantee that system (2.1) admits a unique solution $x(t, t_0, \phi)$ in a maximal interval $[t_0-\nu, t_0+b^*)$ for every initial condition $\phi\in PC([-\nu, 0 ], \mathbb{R}^n)$, where $b^*\in(0, +\infty]$.

Definition 2.1. Suppose that

I) $V: [t_0-\nu, \infty)\times \mathbb{R}^n\rightarrow \mathbb{R}_+$ is continuous in the intervals $[t_{k-1}, t_k)\times \mathbb{R}^n$ and $V(t, v)\rightarrow V(t_k^-, u)$ as $(t, v)\rightarrow (t_k^-, u)$, where $k\in \mathbb{Z}_+$;

II) $V(t, x)$ is locally Lipschitzian in $x$ and $V(t, 0)\equiv0$, $\forall\; t\in\mathbb{R}_+$.

Then, such a function $V$ is said to be of the class $\mathcal{V}_0$.

Definition 2.2. (^[29,39]). Let $V\in\mathcal{V}_0$. Its upper right-hand derivative of $V$along with state trajectories of system (2.1) is defined by:

for $\psi\in PC([-\nu, 0], \mathbb{R}^n)$.

In this study, note that impulsive instant sequences $\{t_k\}$ can be arbitrary and the delay in impulses depends on the state evolution over some previous time period that is only assumed to be bounded. Note that in the previous results, the impulsive instants and delays in impulses are assumed to satisfy some conditions, such as average impulsive interval condition and constant delay in impulses (see ^[1,19]). To be specific, it assumes that there exist positive numbers $T_a$ and $N_0$ such that

where $N(T, t)$ stands for the number of impulsive instants of sequence $\{t_k\}$ in the interval $(t, T]$. Clearly, compared with such conditions in the previous results, the condition in current study is weaker and ISS analysis for system (2.1) is more challenging. For convenience, we call such delayed impulses the bounded-delay impulses and denote $\mathfrak{F}_{ab}$ the set of delayed impulses such that $\{t_k\}$ are arbitrary and the delay bound $\tau < +\infty$.

We call a function $\alpha:\mathbb{R}_+\rightarrow \mathbb{R}_+$ is of the class $\mathcal{K}$, provided it is continuous, strict increasing and satisfies $\alpha(0) = 0$. Further, we call $\alpha$ is of the class $\mathcal{K}_\infty$ if it is of the class $\mathcal{K}$ and satisfies $\lim\limits_{t\rightarrow +\infty}\alpha(t) = +\infty$. In addition, a function $\beta:\mathbb{R}_+\times \mathbb{R}_+\rightarrow \mathbb{R}_+$ is of the class $\mathcal{KL}$ provided $\beta(r, t)\in \mathcal{K}$ for every fixed $t\geq0$ and $\beta(r, t)$ is strictly decreasing to zero as $t\rightarrow + \infty$ for every fixed $r\geq 0$.

Definition 2.3. (^[30]). Given an impulsive instant sequence $\{t_k\}$ and the delay bound $\tau$, system (2.1) is said to be input-to-state stable if there exist functions $\beta\in \mathcal{KL}$ and $\gamma\in \mathcal{K}_\infty$ such that, for every initial condition $\phi\in PC([-\nu, 0 ], \mathbb{R}^n)$ and every input $u(t)\in PC([t_0, \infty), \mathbb{R}^m)$, the solution to system (2.1) satisfies

Moreover, we call system (2.1) uniformly input-to-state stable over the class $\mathfrak{F}_{ab}$ provided that it is input-to-state stable for each sequence $\{t_k\}$ and delay bound $\tau$ in $\mathfrak{F}_{ab}$, and functions $\beta, \gamma$ are independent on the choices of these sequences.

3.   Main results

In this section, we will firstly propose a new Halanay-type inequality involving delayed impulses and external inputs. Then, based on this inequality, some valid criteria for ISS of impulsive delayed system (2.1) are obtained. Moreover, some comparisons with existing results are presented in detail.

First, consider the following Halanay-type inequality with delayed impulses and external inputs:

where $V\in PC([t_0-\nu, \infty), \mathbb{R}_+)$, $u(t)\in PC([t_0, \infty), \mathbb{R}^m)$, $\varphi\in \mathcal{K}$ and parameters $\lambda_1, \; \lambda_2, \; h, \; \omega_1, \; \omega_2, \; \tau\in \mathbb{R}_+$. Particularly, $\varphi(|u(t)|)$ describes the potential impact of external inputs on the decay of function $V$.

Especially, a function $V\in PC([t_0-\nu, \infty), \mathbb{R}_+)$ is called a solution of (3.1) if $V$ satisfies inequality (3.1) for all $t\geq t_0$.

Before giving the new ISS criteria, we firstly propose two useful lemmas in this study by applying a new analysis technique.

Lemma 3.1. Suppose that $\lambda_1 > \lambda_2 > 0$. Construct $\mathcal{F}(t) = V(t)e^{\epsilon(t-t_0)}$, for $t\in[t_0-\nu, \infty)$, where $V\in PC([t_0-\nu, \infty), \mathbb{R}_+)$ is a solution of (3.1) and $\epsilon\in(0, \hat{\epsilon})$ with $\hat{\epsilon}$ satisfying that

If there exists $t^*\in[t_{k-1}, t_k)$ for certain $k\in \mathbb{Z}_+$ such that

then it follows that

where $\zeta = -\frac{1}{2}(-\lambda_1+\lambda_2e^{\epsilon h}+\epsilon)$.

Proof. Using (3.2) and (3.3), we can observe that

This completes the proof.

Lemma 3.2. Assume that $\lambda_1 > \lambda_2 > 0$, $\omega_1 > 0$, $\omega_2 > 0$ and $\omega_1+\omega_2: = \omega\in(0, 1)$. Select proper $\epsilon > 0$, $\zeta > 0$ such that (3.2) is satisfied. Further, let $\delta\geq 1$ satisfy that $\delta\geq\frac{1-\omega}{\epsilon+\zeta}$, which leads to

Then, any solution $V\in PC([t_0-\nu, \infty), \mathbb{R}_+)$ of (3.1) satisfies that

for $t\in[t_{k-1}, t_k)$, $k\in\mathbb{Z}_+$, where $\bar{V}(t_0): = \sup\limits_{s\in[t_0-\nu, t_0]}V(s)$ and $\varpi: = \max\{1, \omega_1+\omega_2 e^{\epsilon \tau}\}$.

Proof. Clearly, it is equivalent to show that

for $t\in[t_{k-1}, t_k)$, $k\in\mathbb{Z}_+$. To prove (3.6), we define

and an auxiliary function

for all $t\in[t_{k-1}, t_k), \; k\in \mathbb{Z}_+$. Hence, we turn to prove that $\Xi(t)\leq\Gamma_k$, for all $t\in[t_{k-1}, t_k)$, $k\in \mathbb{Z}_+$. To begin with, when $k = 1$, we shall prove $\Xi(t)\leq\Gamma_1 = \bar{V}(t_0)$, $t\in[t_0, t_1)$. Clearly, we can check that $\Xi(t_0)\leq\Gamma_1$. If the afore-mentioned inference for $k = 1$ is not correct, then there emerges $t^*\in[t_0, t_1)$, such that $\Xi(t^*) = \Gamma_1$, $\Xi(\theta)\leq\Gamma_1$, for all $\theta\in[t_0-\nu, t^*)$ and $D^+\Xi(t^*)\geq0$. Due to $\Xi(t^*) = \Gamma_1\geq0$, it yields that

Further, from

one can observe that for $\theta\in[t_0-\nu, t^*)$,

which implies

since function $\varphi(\|u\|_{[t_0, t]})$ is monotonically increasing with respect to time $t$. Hence, it follows that $\mathcal{F}(t^*)\geq \mathcal{F}(\theta)$, $\theta\in[t_0-\nu, t^*)$ and by using Lemma 3.1, we can further conclude that (3.4) holds. Recall the monotonically increasing property of function $\varphi(\|u\|_{[t_0, t]})$, it leads to $D^+\varphi(\|u\|_{[t_0, t]})\geq 0$ (or $D^+\varphi(\|u\|_{[t_0, t]}) = +\infty$) for all $t\geq t_0$. Then, by utilizing (3.4), (3.5) and (3.7), it follows that

Thus, it derives a contradiction to $D^+\Xi(t^*)\geq0$. Next, we assume that (3.6) holds for all $k\leq N$ for certain $N\in \mathbb{Z}_+$. Then, we shall prove that (3.6) still holds for $k = N+1$, i.e., $\Xi(t)\leq\Gamma_{N+1}$, $t\in[t_{N}, t_{N+1})$. First, when $t = t_N$, due to the monotonically increasing property of $\Gamma_k$ on $k\in \mathbb{Z}_+$, it follows from the assumption that

Since $\delta\geq 1$ and $0 < \omega_1+\omega_2 = \omega < 1$, we can conclude that

Then, due to (3.9) and the fact $\varphi(|u(t_N^-)|)\leq\varphi(\|u\|_{[t_0, t_N]})$, estimate (3.8) can be further deduced that

If there emerges some $t\in[t_N, t_{N+1})$ satisfying that $\Xi(t) > \Gamma_{N+1}$, then we can choose a proper $\hat{t}\in[t_N, t_{N+1})$ such that $\Xi(\hat{t}) = \Gamma_{N+1}$, $\Xi(\theta)\leq\Gamma_{N+1}$, $\theta\in[t_0-\nu, \hat{t})$, and $D^+\Xi(\hat{t})\geq0$. Now, by applying Lemma 3.1 again, we can conclude that $D^+\Xi(\hat{t}) < 0$, and this is a contradiction to $D^+\Xi(\hat{t})\geq0$. Therefore, by the method of mathematical induction, (3.6) is shown to be true for all $k\in\mathbb{Z}_+$. This concludes the proof.

Next, based on Halanay-type inequality (3.1) and Lemma 3.2, some tractable ISS criteria for system (2.1) are derived.

Theorem 3.1. Assume that there exist function $\mathrm{V}\in\mathcal{V}_0$, functions $\alpha_1, \alpha_2\in \mathcal{K}_\infty$, $\varphi\in \mathcal{K}$, and parameters $\lambda_1 > \lambda_2 > 0$, $\omega_1 > 0$, $\omega_2 > 0$ with $\omega_1+\omega_2 = \omega\in (0, 1)$ such that the following conditions hold

for all $t\geq t_0$, $x\in\mathbb{R}^n$, $u\in\mathbb{R}^m$ and $\psi\in PC([-\nu, 0 ], \mathbb{R}^n)$, where $k\in \mathbb{Z}_+$.Then, system (2.1) is uniformly input-to-state stable over the class $\mathfrak{F}_{ab}$.

Proof. For simplicity, we set $\mathrm{V}(t) = \mathrm{V}(t, x(t))$. For arbitrary given $h\in\mathbb{R}_+$ and $\tau\in\mathbb{R}_+$, which are bounded, we can select sufficiently small $\epsilon > 0$, $\zeta > 0$ and sufficiently large $\delta\geq 1$ such that (3.2), (3.5) and $\omega_1+\omega_2 e^{\epsilon \tau}\leq1$ are satisfied. Clearly, it follows from conditions $\bf{H}_2$ and $\bf{H}_3$ that all conditions of Lemma 3.2 hold. Hence, by using Halanay-type inequality (3.1) and Lemma 3.2, it holds that

for all $t\geq t_0$, where $\bar{\mathrm{V}}(t_0): = \sup\limits_{s\in[t_0-\nu, t_0]}\mathrm{V}(s, x(s))$. Then, condition $\bf{H}_1$ together with (3.10) imply that

for every initial condition $\phi\in PC([-\nu, 0 ], \mathbb{R}^n)$. Thus, system (2.1) is uniformly input-to-state stable over the class $\mathfrak{F}_{ab}$. This completes the proof.

Remark 3.1. Clearly, it follows from (3.5) and (3.11) that the ultimate bound of system state depends on coefficient $\omega$ and the size of external input to some degree. To be specific, the ultimate bound of system state may become smaller when either $\omega$ or the size of external input becomes less. Furthermore, when there is no external input, the sufficient condition for Lyapunov stability of system (2.1) with $u(t)\equiv0$ can be derived. Hence, the obtained results enrich the work on Lyapunov stability of nonlinear systems with delayed impulses to a certain degree.

In particular, in order to better compare with the results in ^[1], we consider the case that there is no delay in continuous dynamics and derive the following result.

Corollary 3.1. Assume that there exist function $\mathrm{V}\in\mathcal{V}_0$, functions $\alpha_1, \alpha_2\in \mathcal{K}_\infty$, $\varphi\in \mathcal{K}$, and parameters $\lambda_1 > 0$, $\omega_1 > 0$, $\omega_2 > 0$ with $\omega_1+\omega_2 = \omega\in (0, 1)$ such that $\bf{H}_1$, $\bf{H}_3$ and the following condition hold

for all $t\geq t_0$, $x\in\mathbb{R}^n$, $u\in\mathbb{R}^m$ and $\psi\in PC([-\nu, 0 ], \mathbb{R}^n)$, where $k\in \mathbb{Z}_+$.Then, system (2.1) is uniformly input-to-state stable over the class $\mathfrak{F}_{ab}$.

Remark 3.2. One may observe from Theorem 3.1 and Corollary 3.1 that both continuous dynamics and discrete dynamics are required to be ISS. However, note that due to the occurrence of delays in impulses, the original stabilizing impulses may turn to be destabilizing ones if the sizes of delays and impulsive intervals break through certain constraints, which can be also found in ^[1]. More importantly, when the delays in impulses are arbitrarily finite, the destabilizing effect of such delayed impulses, i.e., the bounded-delay impulses, may be more dramatic. In fact, it is necessary to consider the conditions $\bf{H}_2$ and $\bf{H}_3$ with $\lambda_1 > \lambda_2 > 0$, $\omega\in (0, 1)$ for the case of bounded-delay impulses. In particular, we take the following simple system without external input as an example to show the influence of such delayed impulses. Consider system

with initial condition $x(t_0) = x_0\neq0$, where $a > 0$, $b > 0$ and the delays in impulses can be regarded as $\tau_k\equiv (t_k-t_{k-1})^-$. Clearly, such delayed impulses is of bounded-delay impulses if $\sup_{k\in\mathbb{Z}_+}\{t_k-t_{k-1}\} < \infty$. One can check that the solution to system (3.12) satisfies

It follows that $x(t_k) = x_0b^{k}$, which implies that system (3.12) is unstable for arbitrary $b > 1$. Especially, system (3.12) is stable but not asymptotically stable if $b = 1$. Hence, in order to overcome the difficulty that the delays in impulses are arbitrarily finite and guarantee the system achieve asymptotical stability, we need to impose the condition $0 < b < 1$. Based on the above analysis, one may conclude that when investigating the ISS of delayed systems with bounded-delay impulses by Halanay-type inequality (3.1), conditions $\lambda_1 > \lambda_2 > 0$ and $\omega\in (0, 1)$ in Theorem 3.1 are necessary to some degree.

Remark 3.3. Recently, some significant work on ISS of nonlinear systems with delayed impulses has been reported, such as ^[1,30]. Note that ^[1] has studied the effect of delayed impulses on ISS, but the effect of delayed impulses was not well revealed. To be specific, it was shown in ^[1] that the occurrence of delay in impulses may make impulses destabilizing even if coefficient $\omega\in(0, 1)$. Moreover, ^[1] claimed that in this case, system (2.1) may become unstable if the impulses occur too frequently. In the same situation, we conclude that these delayed impulses do not destroy the ISS property of system (2.1) provided that the delays in impulses are bounded, irrespective of the frequency of impulses via our results. Different from the previous results (e.g., ^[1,20]), the current study mainly focuses on the case of bounded-delay impulses, where the delay bound can be an any finite value; and further, the addressed impulsive instant sequence can be arbitrary. Thus, the obtained results may be conservative for the case of delay that is very small or satisfies some specific conditions.

Remark 3.4. By using Lyapunov-Krasovskii functional method, ^[30] has derived some useful ISS criteria for time-delay systems with delayed impulses, and the case of bounded-delay impulses can be addressed as well (see Theorem 4 in ^[30]). For one thing, however, the construction of Lyapunov-Krasovskii functionals is critical in utilizing these results in ^[30] and there exists no rule on how to select such functionals. For another thing, our results admit different bounds for the delays in continuous dynamics and impulses, which is more general than that considered in ^[30]. Particularly, these results remain valid for delay-free systems with delayed impulses when $h = 0$, such as ^[1,23]; delayed systems with delay-free impulses when $\tau = 0$, such as ^[22,33].

4.   Numerical examples

To demonstrate the effectiveness and less restrictiveness of the obtained results, two numerical examples are presented in this section. Especially, the first example is an extension of that considered in ^[1].

Example 4.1. Discuss the following system

where $|a|+|b| < 1$, $|\varrho|+|\beta| < 1$, $2(1-|a|-|b|) > |a|$; $\rm{sat}(\cdot)$ stands for the well-known saturation function, that is ${\rm sat}(x) = \frac{1}{2}(|x+1|-|x-1|)$. Select Lyapunov function

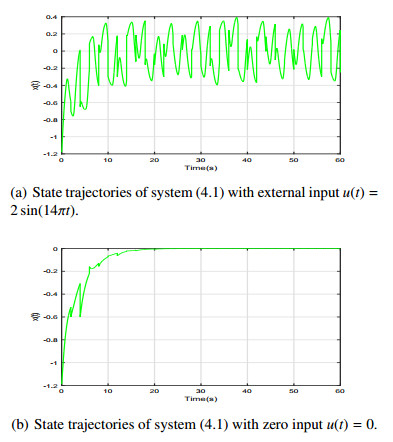
If $|x|\leq1$, we can see $\nabla V(x)\cdot f\leq-(2-|a|-|b|)V(x)+|a|V(x(t-h))+|b|u^2$. If $|x| > 1$, $\nabla V(x)\cdot f\leq-2(1-|a|-|b|)V(x)$. It follows from above two cases that $\nabla V(x)\cdot f\leq-2(1-|a|-|b|)V(x)+|a|V(x(t-h))+|b|u^2$, $\forall\; x$ a.e., $\forall u$, which leads to $\bf{H}_2$ with $\lambda_1 = 2(1-|a|-|b|)$, $\lambda_2 = |a|$. At impulsive instants, if $|\varrho x+\beta\; {\rm sat}(u)|\leq1$, $V(g(x, u)) = (\varrho x+\beta\; {\rm sat}(u))^2\leq \varrho^2V+2|\beta||u|+3\beta^2u^2$; If $|\varrho x+\beta\; {\rm sat}(u)| > 1$, we can check that $|x| > (1-|\beta|)/|\varrho| > 1$. Hence, $V(g(x, u)) = {\rm exp}(2(|\varrho x+\beta\; {\rm sat}(u)|-1))\leq{\rm exp}(2|\varrho| |x|+2|\beta|-2))\leq \omega_0 V(x)$, where $\omega_0: = \rm{exp}(-2(1-|\beta|-|\varrho|))$. Denote $\varphi(s) = 2|\beta|s+(3\beta^2+|b|)s^2$, and then $\bf{H}_3$ holds with $\omega_1 = 0$ and $\omega_2 = \omega_0\vee \varrho^2$. By utilizing Theorem 3.1, we can conclude that system (4.1) is uniformly input-to-state stable over the class $\mathfrak{F}_{ab}$. Specifically, choose parameters $a = 0.4$, $b = 0.5$, $\varrho = 0.5$, $\beta = 0.1$, $h = 2$ and $\tau_k = 3+(-1)^k+\frac{1}{k^2}$, $t_k = k$, $k\in \mathbb{Z}_+$. Clearly, all conditions of Theorem 3.1 hold, and hence we can conclude that system (4.1) is uniformly input-to-state stable over the class $\mathfrak{F}_{ab}$. Dynamical behaviors of system (4.1) with external input $u(t) = 2\sin(14\pi t)$ are shown in Figure 1(a). In particular, dynamical behaviors of system (4.1) with input $u(t) = 0$ are shown in Figure 1(b).

Remark 4.1. Actually, this example with $a = 0$ is the one considered in ^[1]. It is worth noting that in order to acquire the uniform ISS of system (4.1) for arbitrary impulsive instant sequence, conditions $\tau_k\equiv\tau$ and $2\tau(1-|b|)\leq -\ln \omega_2$ should be satisfied in ^[1]. While in this case, our results only require that $\sup_{k\in\mathbb{Z}_+}\{\tau_k\} < +\infty$, i.e., the delays in impulses are bounded. Hence, this example shows that our results require milder condition to some degree.

The second example studies the ISS property of time-varying neural networks with distributed delayed impulses, which is adopted from ^[30,33] with slight changes.

Example 4.2. Consider following neural networks

subject to impulses

where $h(t) = 2-\sin(t^2)$, $J = (u_1(t), u_2(t))^T$ and $\tau = 1$. Consider Lyapunov function $V(t, x(t)) = |x_1(t)|+|x_2(t)|$, and set $V(t): = V(t, x(t))$ for convenience. Then, we can derive the derivative of $V$ along the system trajectories of system (4.2)-(4.3) and the change at impulsive instants as follows:

where $\varphi(|J(\cdot)|) = |u_1(\cdot)|+|u_2(\cdot)|$.

It follows that $\lambda_1 = 3.2$, $\lambda_2 = 1.4$, $\omega_1 = \frac{1}{2}$, $\omega_2 = \frac{1}{4}$ and all conditions in Theorem 3.1 are satisfied. Hence, we can conclude that system (4.2)-(4.3) is input-to-state stable for arbitrary impulsive instant sequences. Especially, we set $t_k = 0.2k$ and simulation results for system (4.2)-(4.3) with $J(t) = (\sin(-16\pi t), \cos(-16\pi t))^T$ and $J(t) = (0, 0)^T$ are shown in Figure 2(a) and Figure 2(b), respectively.

Compared with the results in ^[23] and ^[33], both the delay effects in continuous dynamics and impulses are considered in our results. Different from the method of Lyapunov-Krasovskii functional in ^[30], a new Halanay-type inequality (3.1) is proposed to analyze the ISS property of nonlinear systems with bounded-delay impulses. To some extent, our results are simple and easy to verify since they avoid constructing a Lyapunov-Krasovskii functional. In addition, when the delay in continuous dynamics is fast varying (e.g., $h(t) = 2-\sin(t^2)$ in this example), the method of Halanay inequality shows more effectiveness than the method of Lyapunov-Krasovskii functional ^[37]. Hence, compared with the relevant results in ^[30], the conditions in our results are easier to check in this sense, and can be applied to deal with more complicated time-varying delays.

5.   Conclusions

In this paper, the ISS property of delayed systems with bounded-delay impulses was explored, where the delays in impulses may be arbitrarily large but bounded. Particularly, a new Halanay-type inequality subject to bounded-delay impulses and external inputs was proposed as a theoretical tool to establish the results. It was shown that under certain conditions, the ISS property can be guaranteed for delayed systems with bounded-delay impulses regardless of both the size of this bound and the frequency of impulses. Finally, theoretical results were validated by two numerical examples. An interesting topic is to apply the theoretical results to the synchronization problem of time-delay complex networks with delayed impulses.

Acknowledgments

This work was partially supported by the National Natural Science Foundation of China under Grant No. 61973078, the Natural Science Foundation of Jiangsu Province of China under Grant No. BK20170019, the Postgraduate Research and Practice Innovation Program of Jiangsu Province under Grant No. KYCX20_0073, and the Fundamental Research Funds for the Central Universities under Grant 3207012103D.

Conflict of interest

All authors declare no conflicts of interest in this paper.