Stability analysis of delayed switched cascade nonlinear systems with uniform switching signals

1.   Introduction

Switched cascade nonlinear systems (SCNSs) combine both features of switched systems ^[1,2,3,4,5] and cascade systems ^[6,7,8,9] and are characterized by complex dynamics and broad applications ^[10,11,12]. On this ground, a great number of researchers have intensively investigated dynamics, especially stability and control issues, of SCNSs, see ^{[13,14,15,16]} and the references therein.

Consider the following SCNS with delays:

where $d(t)$ is delay, $\sigma(t)$ is a switching signal. System (1.1a) and

are called separate systems 1 and 2 of cascade system (1.1), respectively, and the term $\boldsymbol{g}_{\sigma(t)}$ is the coupling term. Clearly, evolution of (1.1a) is completely determined by (1.1a) itself and it affects that of system (1.1b) via $\boldsymbol{g}_{\sigma(t)}$.

Lyapunov theory is a powerful and popular tool to study stability of cascade systems ^[17,18,19]. Since cascade systems have particular structure, a widely employed method is composite Lyapunov function (functional) ^[20] which is based on separate Lyapunov function (functional) constructed for each separate system. This method uses an intuitive idea: The property of a cascade system is related to all separate systems and the coupling terms among them.

Note that (1.1b) can be viewed as perturbed system of (1.2) with perturbation $\boldsymbol{g}_{\sigma(t)}$. It would be desirable that one can draw a stability conclusion for system (1.1) from stability properties of systems (1.1a) and (1.2) and property of perturbation $\boldsymbol{g}_{\sigma(t)}$. Actually, several papers have been reported on stability of delayed SCNSs using the intuitive point of view. Suppose always that coupling term $\boldsymbol{g}_{\sigma(t)}$ has a linear growth bound ^[21]. With the assumption that system (1.2) is exponentially stable, it was revealed that system (1.1) is exponentially stable if and only if so is system (1.1a), and that it is asymptotically stable if so is system (1.1a) ^[14,16]. These results essentially rely on the following key observation: For a nominal delayed switched nonlinear system being exponentially stable, trajectories of the corresponding perturbed system exponentially (asymptotically) decay to origin if the perturbation can be upper bounded by a function exponentially (asymptotically) converging to zero. Note that many systems are asymptotically stable but not exponentially stable since asymptotic stability is weaker than exponential one. Therefore, it is more significant to study the situation where the nominal systems are asymptotically stable. Recently, it was proposed in Ref. ^[22] that for a nominal discrete-time switched system being asymptotically stable, trajectories of the corresponding perturbed system asymptotically converge to zero if the perturbation exponentially approaches zero. Based on this fact and with assumption that separate system 2 is asymptotically stable, it was shown that a discrete-time SCNS is asymptotically stable if separate system 1 is exponentially stable ^[22].

Our motivations are as follows: (ⅰ) The key assumption in Ref. ^[14] is that separate system 2 is exponentially stable. The stability property keeps unknown in the situation where separate system 2 is asymptotically stable and will be investigated in the present paper. (ⅱ) Explore the robust convergence of an asymptotically stable nominal system with perturbations. Since asymptotic stability is much weaker than exponential one, the issue discussed here is of more importance than that in ^[14]. (ⅲ) Provide a counterpart of Ref. ^[22] for continuous-time SCNSs, which is more challenging.

The main contribution lies in three aspects: (ⅰ) The concept of uniform switching signals is proposed, which makes main results in the present paper applicable to many classes of switched systems. (ⅱ) It is shown that, with the assumption that the nominal delayed switched nonlinear system is asymptotically stable, trajectories of the perturbed system asymptotically approach zero if the perturbation can be upper bounded by an exponentially decaying function. (ⅲ) Some sufficient asymptotic stability conditions are presented for continuous-time delayed SCNSs. These results can be applied to stability analysis and controller design for SCNSs in a decomposition way.

The rest is organized as follows. Preliminaries are presented in Section 2. Convergence property of delayed switched nonlinear systems subject to perturbations is discussed in Subsection 3.1, and stability conditions of SCNSs are proposed in Subsection 3.2. A numerical example is provided in Section 4. Finally, Section 5 concludes this paper.

Notation. $\mathbb{R}^{n\times m}$ is the set of $n\times m$-dimensional real matrices and $\mathbb{R}^{n} = \mathbb{R}^{n\times 1}$. $\mathbb{R}_{0, +} = \left[0, \infty \right)$ and $\mathbb{R}_{+} = \left(0, \infty \right)$. $\mathbb{N}_0 = \left\{0, 1, 2, \ldots\right\}$ and $\mathbb{N} = \left\{1, 2, \ldots\right\}$. $A^{\text{T}}$ is the transpose of matrix $A$. Given vectors $\boldsymbol{x}, \boldsymbol{y}$, $\mathrm{col}\left(\boldsymbol{x}, \boldsymbol{y} \right) = \left[\boldsymbol{x}^{\text{T}}\, \boldsymbol{y}^{\text{T}}\right]^{\text{T}}$. For a fixed real number $a$, $\left\lceil a\right\rceil$ is the minimum integer greater than or equal to $a$ and $\left\lvert {a} \right\rvert$ is its absolute value. $\left\|\boldsymbol{x}\right\|_\infty = \max \left\{\left\lvert {x_1} \right\rvert, \ldots, \left\lvert {x_n} \right\rvert\right\}$ is the $l_\infty$ norm of $\boldsymbol{x}\in \mathbb{R}^n$ and is denoted by $\left\|\boldsymbol{x}\right\|$. For any continuous function $\boldsymbol{x}\left(s\right)$ on $\left[-d, a\right)$ with scalars $a > 0$, $d > 0$ and any $t\in \left[0, a\right)$, $\boldsymbol{x}_t$ denotes a continuous function on $\left[t-d, t\right]$ defined by $\boldsymbol{x}_t \left(\theta\right) =$ $\boldsymbol{x} \left(t+\theta\right)$ for each $\theta\in [-d, 0]$. $\left\|\boldsymbol{x}_t\right\| =$ $\sup_{t-d\leq s\leq t}\left\{\left\|\boldsymbol{x}(s)\right\|\right\}$. $\mathcal{C}\left(\left[a, b\right], \mathbb{R}^n\right)$ is the set of continuous functions from interval $\left[a, b\right]$ to $\mathbb{R}^n$, and $\mathcal{C}_\vartheta(\left[a, b\right], \mathbb{R}^n) = \left\{\boldsymbol{x}\in \mathcal{C}(\left[a, b\right], \mathbb{R}^n): \left\|\boldsymbol{x}\right\| < \vartheta\right\}$ with $\vartheta > 0$. Let $\kappa\in \left(0, \infty\right]$. A continuous function $\alpha:\left[0, \kappa\right)\rightarrow \left[0, \infty\right)$ belongs to class $\mathcal{K}$ if it is strictly increasing and $\alpha(0) = 0$, and a continuous function $\beta:\left[0, \kappa\right)\times \left[0, \infty\right)\rightarrow \left[0, \infty\right)$ belongs to class $\mathcal{KL},$ if for each fixed $s$, $\beta\left(r, s\right)$ belongs to class $\mathcal{K}$ with respect to $r$, and for each fixed $r$, $\beta\left(r, s\right)$ is decreasing with respect to $s$ and $\beta\left(r, s\right)\rightarrow 0$ as $s\rightarrow \infty$ ^[21]. Throughout this paper, the dimensions of matrices and vectors will not be explicitly mentioned if clear from context.

2.   Preliminaries

Consider the following switched nonlinear system:

where $t_0\geq0$, $\boldsymbol{x}(t)\in \mathbb{R}^n$ is the state, $\sigma: \left[t_0, \infty\right)\rightarrow \left\{1, \ldots, m\right\}$ is a switching signal with $m$ being the number of subsystems and is piecewise constant and continuous from the right. It is assumed in the sequel that $\sigma$ is with switching sequence $\left\{t_i\right\}_{i = 0}^\infty$. $\boldsymbol{d}(t) = \mathrm{col}\left(d_1(t), \ldots, d_\iota(t) \right)\in \mathbb{D}_\iota\triangleq\left[d_{11}, d_{12}\right]\times \cdots\times\left[d_{\iota1}, d_{\iota2}\right]$, where $d_{i2}\geq d_{i1}\geq 0, \forall i\in \left\{1, \ldots, \iota \right\}$, and $d_i(t)\in \left[d_{i1}, d_{i2}\right]$ is piecewise continuous ^[23]. $d = \max\limits_{i\in \left\{1, \ldots, \iota \right\}}\left\{d_{i2}\right\}$. For each $l\in \left\{1, \ldots, m\right\}$, $\boldsymbol{f}_l$ maps $\left[t_0, \infty\right)\times \mathcal{C}(\left[-d, 0\right], \mathbb{R}^n)\times\mathbb{D}_\iota$ into $\mathbb{R}^n$. $\boldsymbol{\varphi}\in \mathcal{C}(\left[t_0-d, t_0\right], \mathbb{R}^n)$ is an initial vector-valued function.

Remark 2.1. By definition, $\boldsymbol{x}_t$ has implicitly included the information of $\boldsymbol{d}(t)$ since $\boldsymbol{x}_t \left(\theta\right) =$ $\boldsymbol{x} \left(t+\theta\right), \forall\theta\in [-d, 0]$. Thus, the parameter $\boldsymbol{d}(t)$ in system (2.1) is somewhat redundant in some sense. However, due to the fact that several vectors of delays will be introduced in Subsection 3.2, we prefer to write $\boldsymbol{d}(t)$ explicitly in order to distinguish them. The vector $\boldsymbol{d}(t)$ can describe multiple delays including constant, time-varying, distributed, and interval ones ^[23].

The following assumption is made for system (2.1).

Assumption 2.1. $\boldsymbol{f}_l\left(\cdot, \boldsymbol{0}, \cdot\right) = \boldsymbol{0}$. $\boldsymbol{f}_l$ is continuous on $\left[t_0, \infty\right)\times \mathcal{C}(\left[-d, 0\right], \mathbb{R}^n)\times\mathbb{D}_\iota$ and is (locally) Lipschitz in the second argument, uniformly in $\left[t_0, \infty\right)\times\mathbb{D}_\iota$, that is, there exist positive scalars $L, \vartheta$ such that

Note that $\boldsymbol{f}_l$ is globally Lipschitz if $\vartheta = \infty$.

There are many kinds of switching signals in literature which play a fundamental role on system dynamics. To motivate our new concept, let us recall some kinds of familiar switching signals in literature.

1. Periodically switching signals: There exists a scalar $\kappa > 0$ such that $\sigma\left(t+\kappa\right) = \sigma\left(t\right), \forall t\geq t_0$, which clearly implies that $\sigma\left(t+\kappa\right) = \sigma\left(t\right), \forall t\geq t_0+c$ with $c > 0$.

2. Switching signals having property of finite discontinuities on finite interval: $\sigma$ has finite discontinuities on any finite interval contained in $\left[t_0, \infty\right)$. Clearly, $\sigma$ also has finite discontinuities on any finite interval contained in $\left[t_0+c, \infty\right)$ with $c > 0$.

Now introduce the concept of uniform switching signal which includes most switching signals frequently encountered in literature.

Definition 2.1. (Uniform switching signals) A switching property $\text{P}$ is said to uniform (with respect to $t$) if $\text{P}$ is valid on $\left[t_0+c, \infty\right)$ for any $c > 0$. A switching signal $\sigma$ satisfying a uniform property $\text{P}$ is said to be a uniform one (with $\text{P}$). The set $\mathbb{S}(\text{P}) = \left\{\sigma: \sigma \text{ has uniform property }\text{P}\right\}$ is said to be uniform with $\text{P}$.

One can easily check the following properties are uniform. (ⅰ). Property satisfying average dwell time constraint: There exist positive numbers $N_0$ and $\tau_a$ such that $N_\sigma(T, t)\leq N_0+\frac{T-t}{\tau_a}$ with $N_\sigma\left(T, t\right)$ being the switching number of $\sigma$ on the open interval $\left(t, T\right), \forall T > t\geq t_0$ ^[24]. (ⅱ). Property satisfying dwell time constraint (which is actually a special case of (ⅰ) with $N_0 = 1$ [24,page 58]). (ⅲ). Fast switching property ^[25,26]. (ⅳ). Homogeneous Markov switching property ^[27]. (ⅴ). Property satisfying mode-dependent average dwell time constraint ^[28].

In the sequel, it is always assumed that the underlying set $\mathbb{S}$ is uniform with certain given $\text{P}$. Since we do not discuss any specific $\text{P}$, $\text{P}$ will not be mentioned explicitly. Moreover, when speaking of "a switched system has some dynamic property", we mean that the property holds for any $\sigma\in \mathbb{S}$.

It is worth noting that there may exist some relationship between different uniform $\text{P}$'s and that for a given switched system with specified subsystems, its dynamic property may vary with $\text{P}$. If $\text{P}_1$ is "arbitrary switching" and $\text{P}_2$ has a dwell time $\tau_\text{d}$, then it may occur that a system is asymptotically stable over $\mathbb{S}(\text{P}_2)$ but diverges over $\mathbb{S}(\text{P}_1)$. Conversely, if a system is asymptotically stable over $\mathbb{S}(\text{P}_1)$ then it is also asymptotically stable over $\mathbb{S}(\text{P}_2)$ since $\mathbb{S}(\text{P}_2)\subseteq \mathbb{S}(\text{P}_1)$.

Though most switching signals possess uniformity, there do exist exceptions one of which is the so-called Zeno behavior admitting an infinite number of switches in a finite interval ^[29].

To make notation concise, from now on we denote the solution to (2.1) by $\boldsymbol{x}\left(t; \boldsymbol{\varphi} \right)$ rather than $\boldsymbol{x}\left(t; t_0, \boldsymbol{\varphi} \right)$ with starting time $t_0$ and initial function $\boldsymbol{\varphi}$ defined on $\left[t_0-d, t_0\right]$, since $t_0$ is indicated by $\boldsymbol{\varphi}$. $\boldsymbol{x}_c$ is the solution to (2.1) on interval $\left[c-d, c\right]$ for $c\geq t_0$. This convention will be applied to systems (3.1) and (3.40) without further statement.

Definition 2.2. (^[30]) Given a set of switching signals $\mathbb{S}$. System (2.1) is locally uniformly exponentially stable (LUES) if there exist positive scalars $\alpha, \gamma, \delta$ satisfying $\left\|\boldsymbol{x}\left(t; \boldsymbol{\varphi} \right)\right\|\leq\alpha \exp\left(-\gamma(t-t_0) \right)\left\|\boldsymbol{\varphi}\right\|, \forall t_0\geq 0, t\geq t_0, \left\|\boldsymbol{\varphi}\right\|\leq \delta, \boldsymbol{d}(t)\in \mathbb{D}_\iota, \sigma\in \mathbb{S}$; if $\delta$ can be arbitrarily large, then it is globally uniformly exponentially stable (GUES). (2.1) is locally uniformly asymptotically stable (LUAS) if there exists a function $\beta$ of class $\mathcal{KL}$ and a scalar $\delta$ such that $\left\|\boldsymbol{x}\left(t; \boldsymbol{\varphi} \right)\right\|\leq \beta\left(\left\|\boldsymbol{\varphi}\right\|, t-t_0\right), \forall t_0\geq 0, t\geq t_0, \left\|\boldsymbol{\varphi}\right\|\leq \delta, \boldsymbol{d}(t)\in \mathbb{D}_\iota, \sigma\in \mathbb{S}$; if $\delta$ can be arbitrarily large, then it is globally uniformly asymptotically stable (GUAS). The scalar $\delta$ is called the radius of attraction if (2.1) is LUES or LUAS.

Now introduce the following lemma:

Lemma 2.1. Let $(2.1)$ be GUAS, that is, there exists a function $\beta\in \mathcal{KL}$ satisfying $\left\|\boldsymbol{x}\left(t; \boldsymbol{\varphi}\right)\right\|\leq \beta\left(\left\|\boldsymbol{\varphi}\right\|, t-t_0\right), \forall t\geq t_0$. Fix positive scalars $a, b, \nu$ satisfying $a\leq b$. Then there exists a scalar $\tau > 0$ such that $\beta\left(\left\|\boldsymbol{\varphi}\right\|, t-t_0\right)\leq \nu\left\|\boldsymbol{\varphi}\right\|$ and $\left\|\boldsymbol{x}\left(t; \boldsymbol{\varphi}\right)\right\|\leq \nu\left\|\boldsymbol{\varphi}\right\|$, $\forall t\geq t_0+\tau, a\leq \left\|\boldsymbol{\varphi}\right\| \leq b$.

Proof. Fix $a, b, \nu$. Since (2.1) is GUAS, there exists $\beta\in \mathcal{KL}$ such that $\left\|\boldsymbol{x}\left(t; \boldsymbol{\varphi}\right)\right\|\leq \beta\left(\left\|\boldsymbol{\varphi}\right\|, t-t_0\right)$. Suppose by contradiction that for any $\tau > 0$, there exists a pair $\left(\boldsymbol{\varphi}, c\right)$ with $a \leq \left\|\boldsymbol{\varphi}\right\| \leq b$ and $c \geq \tau$ such that $\beta\left(\left\|\boldsymbol{\varphi}\right\|, c\right) > \nu\left\|\boldsymbol{\varphi}\right\|$. Take an increasing positive sequence $\left\{\tau _i \right\}_{i = 1}^\infty$ with $\tau _1\geq t_0, \lim\limits_{i\rightarrow \infty}\tau _i = \infty$, and there exists a sequence of pairs $\left\{\left(\boldsymbol{\varphi}_i, c_i \right) \right\}_{i = 1}^\infty$ satisfying $a\leq \left\|\boldsymbol{\varphi}_i\right\| \leq b, \tau _i \leq c_i$, and $\beta\left(\left\|\boldsymbol{\varphi}_i\right\|, c_i\right) > \nu\left\|\boldsymbol{\varphi}\right\|$. $\left\|\boldsymbol{\varphi}_i\right\| \leq b$ means $\lim\limits_{i\rightarrow \infty}\beta\left(b, c_i\right)\geq \lim\limits_{i\rightarrow \infty}\beta\left(\left\|\boldsymbol{\varphi}_i\right\|, c_i\right)\geq \nu\left\|\boldsymbol{\varphi}\right\|\geq \nu a > 0$, contradicting the fact $\lim\limits_{i\rightarrow \infty}\beta\left(b, c_i\right) = 0$. Therefore, there necessarily exists $\tau > 0$ satisfying $\beta\left(\left\|\boldsymbol{\varphi}\right\|, t-t_0\right)\leq \nu\left\|\boldsymbol{\varphi}\right\|, \forall t\geq t_0+\tau, a\leq \left\|\boldsymbol{\varphi}\right\| \leq b$. Since $\left\|\boldsymbol{x}\left(t; \boldsymbol{\varphi}\right)\right\|\leq \beta\left(\left\|\boldsymbol{\varphi}\right\|, t-t_0\right)$, it is clear that $\left\|\boldsymbol{x}\left(t; \boldsymbol{\varphi}\right)\right\|\leq \nu\left\|\boldsymbol{\varphi}\right\|, \forall t\geq t_0+\tau, a\leq \left\|\boldsymbol{\varphi}\right\| \leq b.$

A similar proof immediately yields the next corollary:

Corollary 2.1. Assume that $(2.1)$ is LUAS with radius of attraction $\delta$, that is, there exists a function $\beta\in \mathcal{KL}$ satisfying $\left\|\boldsymbol{x}\left(t; \boldsymbol{\varphi}\right)\right\|\leq \beta\left(\left\|\boldsymbol{\varphi}\right\|, t-t_0\right), \forall t\geq t_0, \left\|\boldsymbol{\varphi}\right\|\leq \delta$. Fix positive scalars $a, b, \nu$ such that $a\leq b\leq \delta$. Then there exists a scalar $\tau > 0$ such that $\beta\left(\left\|\boldsymbol{\varphi}\right\|, t-t_0\right)\leq \nu\left\|\boldsymbol{\varphi}\right\|$ and $\left\|\boldsymbol{x}\left(t; \boldsymbol{\varphi}\right)\right\| \leq \nu\left\|\boldsymbol{\varphi}\right\|$, $\forall t\geq t_0+\tau, a\leq \left\|\boldsymbol{\varphi}\right\| \leq b$.

3.   Main results

This section first investigates the convergence of delayed switched systems with perturbations, and then analyzes stability of switched cascade systems with delays.

3.1.   Convergence analysis of perturbed switched systems

Consider the following perturbed system of (2.1)

with $\boldsymbol{u}(t)$ being perturbation. The next assumption is always imposed on $\boldsymbol{u}(t)$:

Assumption 3.1. $\left\|\boldsymbol{u}(t)\right\|\leq \alpha_1\exp\left(-\gamma_1\left(t-t_0 \right) \right), \forall t \geq t_0$, for some $\alpha_1 > 0, \gamma_1 > 0$.

The following lemma estimates the error between the trajectories of (2.1) and (3.1) with the assumption that both systems are with a same initial function.

Lemma 3.1. Assume that systems (2.1) and (3.1) evolve from $c\geq t_0$ and are with a same initial function $\boldsymbol{\phi}$ defined on $[c-d, c]$. Let $\boldsymbol{x}(t; \boldsymbol{\phi}), \boldsymbol{y}(t; \boldsymbol{\phi})$ be solutions to (2.1) and (3.1), respectively. Define

The following statements hold:

1. Suppose that Assumption 2.1 holds with $\vartheta = \infty$. Then

2. Suppose that Assumption 2.1 holds with $\vartheta\in \mathbb{R}_+$. If $\boldsymbol{x}_s, \boldsymbol{y}_s\in \mathcal{C}_\vartheta(\left[-d, 0\right], \mathbb{R}^n)$ hold for any $s\in \left[c, t\right]$, then $(3.2)$ is true.

Proof. Item 2 is exactly [14,Lemma 7], and Item 1 naturally holds for $\vartheta = \infty$.

Note that the above lemma provides an estimate of difference between solutions to (2.1) and (3.1) on $\left[c_{i-1}, c_i\right)$, which is completely determined by perturbation $\boldsymbol{u}(t)$ and is not affected by initial condition. Item 1 is a global version and Item 2 is a local one.

Define

Clearly, $\mu(t)$ monotonically increases. It follows from (3.2) that

which, by Assumption 3.1, further means that

The identity $\boldsymbol{y}\left(t; \boldsymbol{\varphi}\right) = \boldsymbol{y}\left(t; \boldsymbol{y}_c\right)$ clearly holds for $t\geq c\geq t_0$. If (2.1) is GUAS, then there exists a $\beta\in \mathcal{KL}$ satisfying $\left\|\boldsymbol{x}\left(t; \boldsymbol{\varphi}\right)\right\| \leq \beta\left(\left\|\boldsymbol{\varphi}\right\|, t-t_0\right), \forall t_0\geq 0, t\geq t_0$, and thus

Hence,

(3.5) and (3.6), together with (3.4), indicate that

Fix $\vartheta$ in Assumption 2.1. If (2.1) is LUAS with the radius of attraction $\delta$ and if $\left\|\boldsymbol{y}_s\right\|\leq \min\left\{\delta, \vartheta\right\}$ for $s\in \left[c, t\right]$, then (3.5)-(3.8) also hold.

Lemma 3.2. Fix a uniform $\mathbb{S}$ⁱ. Suppose that system $(2.1)$ is LUAS. Then the solution to system $(3.1)$ is bounded on $\left[t_0, \infty\right)$ with $\left\|\boldsymbol{\varphi}\right\|\leq \delta_1, \alpha_1\leq \delta_1$ for some $\delta_1$.

ⁱHere we drop the uniform property $\text{P}$ from $\mathbb{S}(\text{P})$. In the sequel, $\mathbb{S}(\text{P})$ will also be denoted by $\mathbb{S}$.

Proof. The importance of uniformity of $\mathbb{S}$ is demonstrated first. We are concerned with the dynamics of system (3.1) over given set $\mathbb{S}$ and will exploit evolution of system (2.1) with different starting time $c > t_0$ and initial function $\boldsymbol{y}_{c}$ defined on $\left[c-d, c\right]$. Define $\mathbb{S}_{c} = \left\{\sigma:\left[c, \infty\right)\rightarrow \left\{1, \ldots, m\right\}, \sigma\in\mathbb{S}\right\}$. Since $\mathbb{S}$ is uniform with given uniform $\text{P}$, $\mathbb{S}_{c}$ is necessarily uniform with $\text{P}$. System (2.1) is LUAS implies that there exists a constant $\varepsilon > 0$ and a function $\beta\in \mathcal{KL}$ such that $\left\|\boldsymbol{x}\left(t; \boldsymbol{\varphi} \right)\right\| \leq \beta\left(\left\|\boldsymbol{\varphi}\right\|, t-t_0\right), \forall t_0\geq 0, t\geq t_0, \left\|\boldsymbol{\varphi}\right\|\leq \varepsilon$, where $\boldsymbol{\varphi}\in \mathcal{C}(\left[t_0-d, t_0\right], \mathbb{R}^n)$. It also holds that $\left\|\boldsymbol{x}\left(t; \boldsymbol{\phi}\right)\right\| \leq \beta\left(\left\|\boldsymbol{\phi}\right\|, t-c\right), \forall c > t_0, t\geq c, \left\|\boldsymbol{\phi}\right\|\leq \varepsilon$ with $\boldsymbol{\phi}\in \mathcal{C}(\left[c-d, c\right], \mathbb{R}^n)$ since both $\mathbb{S}$ and $\mathbb{S}_{c}$ are uniform with $\text{P}$.

$\beta\in \mathcal{KL}$ implies existence of $\tau > 0$ such that

Let $\zeta = \frac{0.5L\varepsilon}{2^{\left\lceil (\tau+d)/L\right\rceil}-1}$ and take $\delta_1 = \min \left\{\varepsilon, \zeta\right\}$. Suppose that $\boldsymbol{\varphi}, \alpha_1$ satisfy $\left\|\boldsymbol{\varphi}\right\|\leq \delta_1, \alpha_1\leq \delta_1$. By definition of $\mu(t)$,

Inequality (3.7) with $c = t_0$ indicates that

(3.9) and the definition of $\delta_1$ mean that $\beta\left(\delta_1, t-t_0\right)\leq \beta\left(\varepsilon, t-t_0\right)$, which, together with (3.10), (3.11), and the monotonicity of $\mu$, gives the following estimate:

By (3.7),

The consequence of (3.12) is $\left\|\boldsymbol{y}_{t_0+\tau+d}\right\|\leq \varepsilon$. Clearly, $\exp\left(-\gamma_1\left(\tau+d\right) \right) < 1$. Therefore, (3.13) implies that

Following a similar process, one can show that for any $i\in \mathbb{N}_0$, the following inequality holds:

By (3.8) and (3.15), it is not difficult to see that

In a word, if $\left\|\boldsymbol{\varphi}\right\|\leq \delta_1, \alpha_1\leq \delta_1$, then

The proof is completed.

We are in a position to present the following result which shows that the solution to system (3.1) approaches zero asymptotically provided that system (2.1) is LUAS.

Theorem 3.1. Fix a uniform $\mathbb{S}$ and consider system (3.1). Suppose that system (2.1) is LUAS and that Assumption 2.1 holds. Then there exists a $\bar{\beta}\in \mathcal{KL}$ and a scalar $\delta_1 > 0$ such that

for any $\left\|\boldsymbol{\varphi}\right\| < \delta_1, \theta < \delta_1$, where $\theta = \max\left\{\left\|\boldsymbol{\varphi}\right\|, \alpha_1\right\}$.

Proof. Since system (2.1) is LUAS, there exists a $\beta\in \mathcal{KL}$ and $\vartheta$ such that Assumption 2.1 is satisfied and that

It is easy to see that there exists a unique constant $\varepsilon > 0$ such that $\beta\left(\varepsilon, 0\right)+0.5\varepsilon = \vartheta$. According to (3.17) and Lemma 3.2, one can fix $\delta_1 > 0$ such that

which means that $\delta\triangleq\sup_{\left\|\boldsymbol{\varphi}\right\|\leq \delta_1, \alpha_1\leq \delta_1, t\geq t_0}\left\{\left\|\boldsymbol{y}\left(t; \boldsymbol{\varphi}\right)\right\|\right\} \leq \vartheta$ exists. Fix $\boldsymbol{\varphi}, \alpha_1$ with $\left\|\boldsymbol{\varphi}\right\| < \delta_1, \alpha_1 < \delta_1$ and let $\theta = \max\left\{\left\|\boldsymbol{\varphi}\right\|, \alpha_1\right\}$. Thus,

Since $\beta\left(\delta q, 0\right)+0.5\delta q^2 = 0$ if $q = 0$ and $\beta\left(\delta q, 0\right)+0.5\delta q^2$ is strictly increasing with respect to $q$, we can pick the unique $q\in (0, 1)$ satisfying $\beta\left(\delta q, 0\right)+0.5\delta q^2 = \delta$ (note that $\beta\left(\delta, 0\right)\geq \delta$).

By Corollary 2.1, there exists a positive increasing sequence $\left\{ \tau_i\right\}_{i = 1}^\infty$ such that

which further means

Denote $\mu_i = \mu(\tau_i+d)$ with $\mu$ defined in (3.3). Choose $a_1 > 0$ satisfying $\mu_1 \theta \exp\left(-a_1 \gamma_1\right) \leq 0.5\delta q$. Note that such an $a_1$ does exist, since $\mu_1, \theta, \gamma_1, \delta$ and $q$ are all known positive constants. Then, pick the minimal $b_1\in\mathbb{N}$ and $a_2 > 0$ such that $\mu_2 \theta\exp\left(-a_2\gamma_1\right) \leq 0.5\delta q^2$ and $a_2 = a_1 +b_1\left(\tau_1+d \right)$. Construct in the same manner a positive sequence $\left\{a_i\right\}_{i = 1}^\infty$ and a positive integer sequence $\left\{b_i\right\}_{i = 1}^\infty$ which are minimal in the sense that $a_i(i > 1), b_i(i\geq 1)$ are minimal values satisfying

Note that $a_i, b_i$ are independent of $t_0$. The following symbols will be used repeatedly.

It is clear that

$a_{i_0} = a_i, a_{i_{b_i}} = a_{i+1}, c_{i_0} = c_i, c_{i_{b_i}} = c_{i+1}, i\in\mathbb{N}$.

Taking $c = c_{i_{l-1}}$, it follows form (3.4) that

Since $\mu(s)$ is increasing in $s$, it holds that $\mu(t-c_{i_{l-1}})\leq \mu(c_{i_l}-c_{i_{l-1}}) = \mu(\tau_i+d) = \mu_i, \forall t\in \mathbb{I}_{i_{l}}$. This, together with (3.26), implies that

By definition of $a_{i_l}$ in (3.25), $a_i = a_{i_0} < a_{i_1} < \cdots < a_{i_{b_i}}$. The above inequality means that

which, by considering the inequality in (3.24), results in

First prove that

Condition (3.21) clearly means that

By the definition of $\tau_1$, it holds that $\beta\left(s, t-t_0\right)\leq 0.5qs, t\geq t_0+\tau_1, \delta q\leq s \leq\delta$. This inequality, combining (3.27) (with $i = l = 1$), (3.29) (with $i = 1$), and (3.5), yields that

If $b_1 = 1$ then (3.28) holds; otherwise suppose that $\left\|\boldsymbol{y}\left(t; \boldsymbol{\varphi}\right)\right\|\leq \delta q (t\in \bar{\mathbb{I}}_{1_l})$ with $l\in \left\{1, \ldots, b_1-1 \right\}$. (3.7) and (with $c = c_{1_1}$) and (3.27) and (with $i = 1, l = l+1$) imply that

By induction, $\left\|\boldsymbol{y}\left(t; \boldsymbol{\varphi}\right)\right\|\leq \delta q, t\in \bar{\mathbb{I}}_{1_{l+1}}$ holds for any $l\in \left\{1, \ldots, b_i \right\}$. Therefore (3.28) is true.

Now show that for $i\in\mathbb{N}\setminus \left\{1\right\}$, it holds that

Consider (3.31) and (3.32) for the case $i = 2$. By condition (3.28) and the definitions of $b_1, c_2$, we have

By the definition of $\tau_2$ and considering (3.23) (with $i = 2$), (3.33) further means that

If $b_2 = 1,$ then (3.31) and (3.32) hold for $i = 2$. Otherwise, following a reasoning similar to the process from (3.33) to (3.34), and using the mathematical induction principle, it yields that

Since $\beta$ is decreasing in the second argument, (3.35) and (3.36) respectively imply that (3.31) and (3.32) hold for $i = 2$. Moreover, in a very similar manner proving the case $i = 2$, it is straightforward to verify that (3.31) and (3.32) hold for $i+1$ provided that they hold for $i\geq 2$. Therefore, by the mathematical induction principle, (3.31) and (3.32) hold for any $i\in \mathbb{N}\setminus \left\{1\right\}$.

Conditions (3.21), (3.31) and the fact $\beta\left(\delta q, 0\right)+0.5\delta q^2 = \delta$ produce that

Fix a scalar $\epsilon > 0$ and introduce

Since $\delta = \sup_{\left\|\boldsymbol{\varphi}\right\|\leq \theta, \alpha_1\leq \theta, t\geq t_0}\left\{\left\|\boldsymbol{y}\left(t; \boldsymbol{\varphi}\right)\right\|\right\}$, $\delta$ is increasing in $\theta$. Fix $0\leq \theta_1 < \theta_2, t\geq 0$. If $t\in \left[0, a_2\right]$, then $\bar{\beta}\left(\theta_2, t\right)-\bar{\beta}\left(\theta_1, t\right)\geq \epsilon\theta_2-\epsilon\theta_1 > 0$; if $t\in \left(a_i, a_{i+1}\right]$ for some $i\in\mathbb{N}\setminus \left\{1\right\}$, then $\bar{\beta}\left(\theta_2, t\right)-\bar{\beta}\left(\theta_1, t\right) > 0$ also holds. That is, $\bar{\beta}\left(\theta, t\right)$ strictly monotonically increases in $\theta$. Moreover, $\bar{\beta}\left(\theta, t\right)$ monotonically decreases in $t$, and approaches zero as $t\rightarrow \infty$. Therefore, $\bar{\beta}$ belongs to $\mathcal{KL}$ and is independent of $t_0$.

(3.37) and (3.38) indicate that $\left\|\boldsymbol{y}\left(t; \boldsymbol{\varphi}\right)\right\| \leq\bar{\beta}\left(\theta, t-t_0\right)$. The proof is completed.

It seems that the constraint $\left\|\boldsymbol{u}(t)\right\|\leq \alpha_1\exp\left(-\gamma_1\left(t-t_0 \right) \right)$ in Assumption 3.1 is too restrictive. Actually, in our context, if $\left\|\boldsymbol{u}(t)\right\|$ is upper bounded by a function asymptotically rather than exponentially decaying to zero, then the perturbed system may diverge, as indicated in the next example.

Example 3.1. Consider the following scalar system:

Let $a(t) = \ln0.5 < 0, u(t) = 1$ for $t\in [0, 1)$, $a(t) = -0.6\ln0.5 > 0$ for $t\in [1, 2), a(t) = \ln0.5$ for $t\in [2, 3), u(t) = 0.6$ for $t\in [1, 3)$. Define for $i\in \mathbb{N}\setminus \left\{1\right\}$ the interval $\Omega_i = \left[2^i-1, 2^{i+1}-1\right)$ and $u(t) = 0.6^i, \forall t\in \Omega_i$. Thus, $u(t)\rightarrow 0$ as $t\rightarrow 0$. Note that $u(t)$ does not satisfy Assumption 3.1. Further define $\Omega_{i_l} = \left[2^i+l-1, 2^i+l\right), \forall l\in \left\{0, 1, \ldots, 2^i-1 \right\}$. $a(t)$ is defined on $\Omega_i, i\geq 2,$ in the following way:

It is not difficult to show that (3.39a) is asymptotically rather than exponentially stable and that (3.39b) diverges. This fact is shown in Figure 1.

3.2.   Stability of SCNSs with delays

Consider the following cascade system

$\begin{align} \mathrm{col}\left(\boldsymbol{x}(t), \boldsymbol{y}(t)\right) = \; & \boldsymbol{\varphi}(t), \quad t\in \left[t_0-d, t_0\right], \end{align}$

where $\boldsymbol{x}(t)\in \mathbb{R}^{n_1}, \boldsymbol{y}(t)\in \mathbb{R}^{n_2}$, and $\boldsymbol{\varphi} = \mathrm{col}\left(\boldsymbol{\varphi}_1, \boldsymbol{\varphi}_2\right)$, which means that for each $t\in \left[t_0-d, t_0\right]$, $\boldsymbol{\varphi}(t) = \mathrm{col}\left(\boldsymbol{\varphi}_1(t), \boldsymbol{\varphi}_2(t) \right)$ with $\boldsymbol{\varphi}_1(t)\in \mathbb{R}^{n_1}, \boldsymbol{\varphi}_2(t)\in \mathbb{R}^{n_2}$. $\tilde{\boldsymbol{d}}(t), \boldsymbol{d}(t), \bar{\boldsymbol{d}}(t)$ are different delay vectors, and $d$ is upper bound of all delays. (3.40a) and

are two separate systems of system (3.40), and $\boldsymbol{g}_{\sigma(t)}\left(t, \boldsymbol{x}_{t}, \bar{\boldsymbol{d}}(t)\right)$ in (3.40b) is the coupling term. Note that the state of system (3.40) is $\mathrm{col}\left(\boldsymbol{x}(t), \boldsymbol{y}(t)\right)$. As usual, it is assumed that $\tilde{\boldsymbol{f}}_l\left(\cdot, \boldsymbol{0}, \cdot\right) = \boldsymbol{0}, \boldsymbol{f}_l\left(\cdot, \boldsymbol{0}, \cdot\right) = \boldsymbol{0}, l\in \left\{1, \ldots, m\right\}$.

Assumption 3.2. There exist two positive scalars $L, \vartheta$ such that

The following result is a consequence of Theorem 3.1.

Theorem 3.2. Fix a uniform $\mathbb{S}$. Suppose that Assumption 3.2 holds with $\vartheta \in \mathbb{R}_+$ and that $\tilde{\boldsymbol{f}}_l, \boldsymbol{f}_l$ are continuous and are locally Lipschitz in the second argument, uniformly in the first and third ones. System (3.40) is LUAS if (3.40a) is LUES and (3.41) is LUAS.

Proof. Let us view (3.41) and (3.40b) as the nominal system (2.1) and the perturbed system (3.1) with perturbation $\boldsymbol{u}(t) = \boldsymbol{g}_{\sigma(t)}\left(t, \boldsymbol{x}_{t}, \bar{\boldsymbol{d}}(t)\right)$ in Theorem 3.1, respectively.

Let $\vartheta, L$ be as in Assumption 3.2. System (3.40a) being LUES means that there exist $\delta_1 > 0, \alpha\geq 1$\footnoteⁱⁱ and $\gamma > 0$ such that $\left\|\boldsymbol{x}(t; \boldsymbol{\varphi}_1)\right\|\leq \alpha\exp \left(-\gamma\left(t-t_0\right)\right)\left\|\boldsymbol{\varphi}_1\right\|, \forall t \geq t_0, \left\|\boldsymbol{\varphi}_1\right\|\leq \delta_1$. If we choose $\boldsymbol{\varphi}_1$ satisfying $\left\|\boldsymbol{\varphi}_1\right\|\leq \min\left\{\frac{\vartheta}{\alpha\exp \left(\gamma d\right)}, \frac{\delta_1}{\alpha}\right\}$ then $\left\|\boldsymbol{x}(t; \boldsymbol{\varphi}_1)\right\|\leq \delta_1, \forall t \geq t_0$. As a result, $\left\|\boldsymbol{x}_{t}\right\|\leq \alpha\exp \left(-\gamma\left(t-t_0-d\right)\right)\left\|\boldsymbol{\varphi}_1\right\| \leq \alpha\exp \left(\gamma d\right)\left\|\boldsymbol{\varphi}_1\right\|\leq \vartheta,$ $\forall t \geq t_0$. By Assumption 3.2,

ⁱⁱBy definition of exponential stability, $\alpha > 0$. By the continuous dependence of solution on initial function, it is easy to that $\alpha\geq 1$ holds (for example, take $\boldsymbol{\varphi}$ as a nonzero constant function).

where $\varsigma = L\alpha\exp \left(\gamma d\right).$

Note that (3.41) is LUAS. It follows from (3.43) and Theorem 3.1 that there exists a constant $\delta_2$ and a $\beta\in \mathcal{KL}$ such that $\left\|\boldsymbol{y}(t; \boldsymbol{\varphi}_2) \right\|\leq \beta\left(\max\left\{ \left\|\boldsymbol{\varphi}_2\right\|, \varsigma \left\|\boldsymbol{\varphi}_1\right\| \right\}, t-t_0\right)$ for $\left\|\boldsymbol{\varphi}_2\right\| < \delta_2, \varsigma \left\|\boldsymbol{\varphi}_1\right\| < \delta_2, \forall t \geq t_0$, where $\boldsymbol{y}(t; \boldsymbol{\varphi}_2)$ is the solution to (3.40b).

Now take $\delta = \min\left\{\delta_2, \frac{\delta_2}{\varsigma}\right\}$. Fix $\boldsymbol{\varphi} = \mathrm{col}\left(\boldsymbol{\varphi}_1, \boldsymbol{\varphi}_2 \right)$ with $\left\|\boldsymbol{\varphi}\right\| < \delta$. It follows that

Function $\alpha\exp \left(-\gamma\left(t-t_0\right)\right)\left\|\boldsymbol{\varphi}\right\|+ \beta\left(\max\left\{1, \varsigma \right\}\left\|\boldsymbol{\varphi}\right\|, t-t_0\right)$ belongs to $\mathcal{KL}$. The proof is completed.

It is worth pointing out that [14,Corollary 18] also holds for system (3.40) with uniform $\mathbb{S}$. This conclusion follows from a proof line similar to Theorem 3.2. Combining this conclusion and Theorem 3.2 above, one has the next corollary:

Corollary 3.1. Fix a uniform $\mathbb{S}$. Suppose that Assumption 3.2 holds and that $\tilde{\boldsymbol{f}}_l, \boldsymbol{f}_l$ are continuous and are locally Lipschitz in the second argument, uniformly in the first and third ones. System $(3.40)$ is LUAS if one of the following statements holds:

1. $(3.40 \rm a)$ is LUES and $(3.41)$ is LUAS.

2. $(3.40 \rm a)$ is LUAS and $(3.41)$ is LUES.

Remark 3.1. One special case of switched systems is non-switched systems, that is, there exists only one subsystem. All the main results in the present paper are valid for non-switched systems.

Remark 3.2. The main results presented in Corollary 3.1, can be easily employed for designing controller. Consider the following control system

with $\tilde{\boldsymbol{v}}(t), \boldsymbol{v}(t)$ being control inputs and $\boldsymbol{g}_{\sigma(t)}$ satisfying Assumption 3.2. To design a controller making (3.45) asymptotically stable, it suffices to design $\tilde{\boldsymbol{v}}(t), \boldsymbol{v}(t)$ such that (3.45a) is uniformly asymptotically (exponentially) stable and $\dot{\boldsymbol{y}}(t) = \boldsymbol{f}_{\sigma(t)}\left(t, \boldsymbol{y}_{t}, \boldsymbol{d}(t)\right)+\boldsymbol{v}(t)$ is uniformly exponentially (asymptotically) stable, without paying any attention to the term $\boldsymbol{g}_{\sigma(t)}\left(t, \boldsymbol{x}_{t}, \bar{\boldsymbol{d}}(t)\right)$.

Remark 3.3. It is assumed in Theorem 3.2 that system (3.41) is uniformly asymptotically stable. In this case, Theorem 3.2 can only provide a local stability condition, leaving the global one open. If system (3.41) is uniformly exponentially stable, then both local and global stability conditions can be established, for details see [14,Corollary 18].

4.   Example

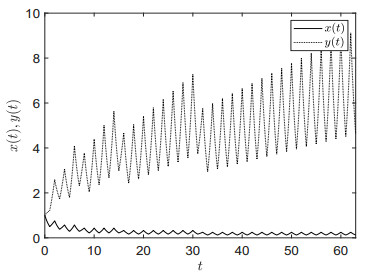
This section provides an example to demonstrate Theorem 3.2.

Example 4.1. Consider the following system which is a reduced version of (3.40):

where $\sigma: \left[t_0, \infty\right)\rightarrow \left\{1, 2\right\}$, $\boldsymbol{x}(t) = \mathrm{col}\left(x _1(t), x _2(t)\right)\in\mathbb{R}^2, \boldsymbol{y}(t) = \mathrm{col}\left(y_1(t), y_2(t)\right)\in\mathbb{R}^2,$ and

By [31,Theorem 1], system (4.1a) is exponentially stable with $d = 2$ under arbitrary switching signals. Moreover, it follows from ^[32] that system $\dot{\boldsymbol{y}}(t) = \boldsymbol{f}_{\sigma(t)}\left(\boldsymbol{y}(t), \boldsymbol{y}(t-d)\right)$ is asymptotically stable with $d = 2$ under arbitrary switching signals.

By Theorem 3.2, system (4.1) is asymptotically stable under arbitrary switching signals; this fact is shown in Figures 2 and the involved switching signal is plotted in Figures 3.

5.   Conclusions

This paper has addressed the asymptotic stability issue of continuous-time switched cascade nonlinear systems with delays. Technically, the main results rely on the robust convergence property of delayed switched nonlinear systems with perturbations. It was shown that trajectory of the perturbed system asymptotically approaches origin if the perturbation can be upper bounded by an exponentially decaying function and if the nominal system is asymptotically stable. This property was then employed to analyse the asymptotic stability of switched cascade nonlinear systems with delays, and some stability conditions have been proposed. Two points should be pointed out: (ⅰ) The considered delays are bounded. (ⅱ) Perturbations in this paper are additive. Therefore, more challenging work in the future is to investigate dynamics of systems involving unbounded delays and multiplicative perturbations.

Acknowledgments

This work was partially supported by National Nature Science Foundation (62073270), State Ethnic Affairs Commission Innovation Research Team, Innovative Research Team of the Education Department of Sichuan Province (15TD0050), and Fundamental Research Funds for the Central Universities (2021HQZZ02).

Conflict of interest

The authors declare there is no conflict of interests.