1.
Introduction
Automatic Fruit Disease Detection (FDD) refers to a process of identifying the presence of diseases in fruit crops. Image analysis, sensor technology, and Machine Learning (ML) are some of the techniques through which the diseases can be detected. The ML techniques remain the most common method for automatic detection of fruit disease while this technique analyzes the images of the fruits [1]. For this purpose, a large set of images of both diseased as well as healthy fruits are collected and utilized to train the ML method. Then, the technique detects the patterns in these images that are indicative of diseases [2]. Sensor technology is another method used to collect the data on fruit crops. This includes humidity, other environmental conditions, temperature measurements and readings from sensors that confirm the presence of particular diseases [3]. The collected data can be used for fruit classification i.e., either diseased or healthy. There exist numerous benefits when utilizing an automated FDD system. One of the most important advantages is its accuracy and quick disease detection [4], which helps in the prevention of disease and protection of crop health. Automatic disease detection systems can be utilized for monitoring vast areas of crops, thus reducing the requirement for manual inspection. Also, these automatic systems are unbiased and highly objective than the human analysis, which in turn helps in minimizing the errors and enriching the precision of disease detection process [5]. However, there exists a few difficulties too when utilizing an automatic FDD system [6]. The necessity for high-quality and accurate data is one of the most challenging issues faced in automatic FDD system. The method cannot detect the disease accurately, if the data used to train the model is of poor quality or incomplete [7]. Further, another difficulty is the demand for robust algorithms that can manage variations in the dataset. For instance, the method should be aware of different kinds of fruits that may have diverse features. At last, the automatic systems necessitate reliable software and hardware infrastructure for proper functioning [8].
There has been a significant surge in the application of image processing and machine vision technologies for enriching the quality of fruit surface images. This is attributed to the reason that such technologies provide huge benefits in areas, where the human eye is not sensitive. Thus, the application of image processing and Computer Vision (CV) methods overcomes the challenges through subjective industrial quality control approaches [9]. Although the performance of the ML algorithms developed earlier is good enough, these methods mostly rely upon the image types (of fruit crops) used during testing and training periods. Further, the outcomes depend on the handcrafted feature extraction techniques, which are again a labor-intensive one. Additionally, such methods have been tested and trained only for smaller datasets, which raises the risk of biased estimations. An alternate method to overcome the abovementioned issues is to leverage the Deep Learning (DL) methods and develop a fruit classification and grading mechanism [10]. Such DL-based technique should be capable of deriving the appropriate features mechanically without any need for manual intervention [11].
In this background, the current research paper introduces a novel Battle Royale Optimization with a Feature Fusion Based Fruit Disease Grading and Classification (BROFF-FDGC) approach. In the proposed BROFF-FDGC approach, the Bilateral Filtering (BF) model is initially implemented for noise elimination. Besides, a compendium of DL methods such as the Inception v3, NASNet, and Xception methods is also utilized for feature extraction. In addition to this, the Bayesian optimization (BO) algorithm is used as a hyperparameter optimizer. Moreover, the BROFF-FDGC model utilizes the stacked sparse-AE(SSAE) technique for classification purpose. Furthermore, the BRO technique is also utilized for optimum hyperparameter tuning of the SSAE technique. The proposed BROFF-FDGC model was extensively experimented through simulation while the outcomes showcase the enriched performance of the model.
The remaining sections of the article are arranged as briefed herewith. Section 2 offers the literature review and Section 3 discusses about the proposed method. Then, Section 4 elaborates on the evaluation results and Section 5 concludes the work.
2.
Related works
Majid et al. [12] examined an integrated DL architecture for fruit disease classification. At first, the researchers applied a data increase and two different kinds of features were derived. In the initial feature type, both color and texture features were derived while in the next type, the DL features were extracted through a pre-trained process. In general, the pre-trained process can be reused with Transfer Learning (TL). Then, both the features were fused through the maximal mean value of the serial technique after which a harmonic threshold-based genetic algorithm was used for the optimization of the resultant fused vector.
Shah et al. [13] proposed a new computerized technique featuring Ant Colony Optimization (ACO)-oriented choice with the help of DL technique. The presented technique had four basic steps; a combination of the derived deep features through matrix length, data augmentation for solving the imbalanced data set, selection of better features utilizing ACO and hybrid Neighbourhood Component Analysis (NCA) methods, and fine-tuned pre-trained DL techniques (such as MobileNet-V2 and NasNet Mobile). The best-selective features were then passed onto multiple classifiers for ultimate recognition. Mostafa et al. [14] leveraged a deep CNN (DCNN)-related data enhancement method by utilizing the unsharp masking technique and color-histogram equalization in order to identify distinct guava plant species. In the presented technique, the data was first preprocessed and normalized. The presented study utilized five NN frameworks such as ResNet-50, AlexNet, GoogLeNet, ResNet-101, and SqueezeNet for identifying distinct guava plant species.
The authors in the study conducted earlier [15] employed a two-phase DCNN methodology for citrus disease classification and plant disease detection by exploiting the leaf images. The proposed methodology had two key stages; categorization of the majorly targeted area to a particular disease; and its classification utilizing a classifier, thus suggesting the effectively-targeted diseased areas in the RPN. In literature [16], the authors examined novel DL-related citrus disease recognition and classification models. An innovative DL-related AlexNet architecture was used in this study for effectual disease detection. The Otsu method was executed in this study for image segmentation after which the application of Alex-Net architecture was executed as an extraction procedure. Afterwards, the Random Forest (RF) method was utilized for categorizing different types of citrus diseases. In addition to this, the Adaptive Gamma Correction (AGC) method was also enforced to enhance the contrast of citrus images. Nikhitha et al. [17] emphasized on formulating a user-friendly device that can detect and grade the disease levels. The inception model utilized CNNs for the classification while the latter was retrained through the TL method. The presented mechanism even ranked the fruit depending on the infection percentage.
In literature [18], the author focused on grape diseases and presented a new structure for identification and classification of selective diseases at the initial phase. A DL-related solution was entrenched into a typical architecture for optimum performance. In this model, three major stages were involved (a) Fusion of stronger features utilizing the presented approach after which the features were subjected to classifier step utilizing the Least Squared Support Vector Machine (LS-SVM), (b) feature extraction after the application of TL on pretrained deep methods such as ResNet101 and AlexNet, and (c) selecting the optimal features through the proposed Yager Entropy along with Kurtosis (YEaK) method. Kejriwal et al. [19] intended to identify and detect the foliar diseases with the help of apple leaf images. Involving a professional to detect the infections is not only time-consuming, but also inefficient, and expensive for large orchards. So, this technique was presented in which a group of three pre-trained DCNNs such as InceptionResNetV2, ResNet101V2, and Xception was used to categorize the apple tree leaves as either infected or healthy based on five disease classes.
3.
The proposed model
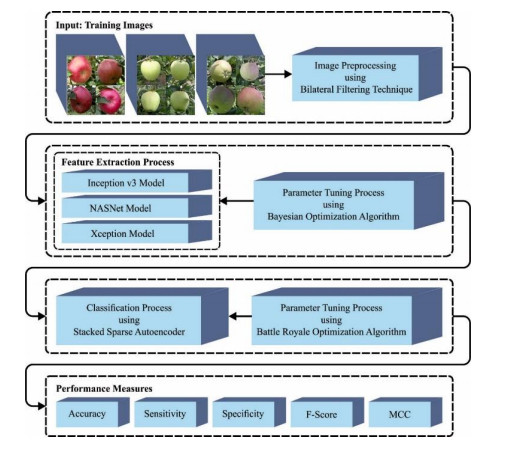
In the current study, an innovative BROFF-FDGC method has been introduced for identification and classification of the fruit disease. The proposed BROFF-FDGC technique incorporates the following processes namely, BF-based noise removal, fusion-based feature extraction, BO-based hyperparameter tuning, SSAE classification, and BRO-based parameter optimization. Figure 1 represents the working flow of the BROFF-FDGC system.
3.1. BF-based noise removal process
The BF process is used to smoothen the images, while at the same time, preserving the rest of the fine details and edges in the image [20]. BF is used in image processing techniques to reduce the number of details and noise in the image and also preserve the overall structure of the image. The BF functions employ a weighted average for all the pixels in the image while the closest pixel is used as weight. The weight is measured based on the intensity difference and spatial distance between the pixel and its neighbors. This enables the filter to preserve other fine details and edges in the image, as the pixel with the largest spatial distance or highest intensity difference from the neighbor is given lower weight and remains less affected by the filter. BF is wisely applied in medical images for smoothening the images, while preserving the significant structures like tissue boundaries and blood vessels.
3.2. Fusion-based feature extraction process
In this study, a fusion of DL architectures like Inception v3, NASNet, and Xception models is executed for the feature extraction process. Data fusion technique has been employed in a diverse range of CV and ML applications [21]. Feature fusion is a crucial task that incorporates multiple feature vectors. This technique depends on feature fusion using the formulated entropy.
The three vectors are defined as follows
Furthermore, the extracted feature is combined in a single vector.
Here, f refers to the fused vector (1 × 1186). The entropy is exploited to feature the vectors for the optimum Feature Selection (FSs) outcomes as per the score. The FS technique can be expressed mathematically as shown in the Eqs (1) and (4). Entropy is used for selecting 1186 score-assisted features in 7835 features.
In these expressions, p denotes the feature probability and He signifies the entropy. The last selected feature is then fed into the classifiers for differentiating the glioma and healthy images.
3.2.1. Inceptionv3 model
InceptionV3 model outperforms the preceding inception architecture through cost-effective computational outcomes [22]. Inception module remains the core component of the inception architecture. The inception model allows for deep networking and effective computation through a reduction dimension with a stacked 1x1 convolutional layer. The key aim of the proposed technique is to address the problems of computation cost and overfitting among various other problems. The fundamental concept behind the inception model is to generate different filters of various dimensions that can run in parallel mode instead of series mode. The network in the inception module has an additional 1x1 convolutional layer before the 3×3 and 5x5 convolutional layers and this feature makes the procedure, a highly robust and computationally inexpensive one. A dense 128x1 layer exchanges the classifier part of the models, viz., head of the model, 3x1 and 128x, and 12x1 for ternary and binary classifications, correspondingly. Afterwards, the model is fine-tuned upon the input images for extracting the best features. For training purposes, InceptionV3 model is fed with input images sized at 224x224x3 while the input passes through different inception models in later stages and it might assist in preventing over-fitting as well as decreasing the computation cost. After being passed over the Inception module, the inputs are then passed to the dense layer of different sizes such as 128x1 and 3x1 or 2x1 for the classifier model.
3.2.2. NASNet model
NASNet is a framework created with the help of a neural structural search method [23]. The search system called the Neural Architecture Search (NAS) as it exploits a control NN to develop a better CNN structure for the given data. The type of NASNet that was exploited in InstaCovNet19 was originally intended for the data named ImageNet. In this design, two classes of convolution cells are exploited, viz., the standard cell, and the reduction cell. Particularly, NASNet has been increased for the ImageNet data that comprises of images from every walk of life. In this case, a pre-trained NASNet infrastructure has been presented. The lack of huge datasets needs the usage of a pre-trained module. During the fine-tuning process, NASNet is provided with input images sized 224x224x3. Then, the input images pass over various reduction and normal layers that extract better features from these images. At last, the attained features are provided as two Dense layers sized at 128x1 and 3x1 for the purpose of classification.
3.2.3. Xception model
Xception refers to 'extreme inception' and it comprises of 36 deep layers apart from the FC layer. Xception contains depthwise convolution layers including the MobileNet model and it comprises of 'shortcuts', in which the outcome of a certain layer is added to the output from the preceding layers. Unlike the InceptionV3, the Xception model combines the input records with compacted lumps, thus mapping the spatial connection for every channel autonomously; later, 1x1 depthwise convolutional layer is implemented to capture the cross-channel connections. The Xception model overtakes the InceptionV3 model with ImageNet dataset classification. In this case, a pre-trained Xception module (trained on ImageNet data) is developed. The pre-trained method is utilized due to the lack of a huge database for detection. The classification models, viz., head of the architecture, are later exchanged with dense layers sized at 128x1and 2x1 for binary classification and 128x1and 2x1 for ternary classification, correspondingly.
3.2.4. BO-based hyperparameter tuning
The BO technique is exploited for optimum hyperparameter adjustment process. BO functions by creating a posterior distribution of the function viz., Gaussian procedure that better describes the operation to be optimized [24]. When the observation counts increase, the posterior distribution also increases. Then, it becomes more apparent, especially at the region in parameter space that is worth computing and exploring. BO technique comprises of two key elements: A statistical method for modeling the objective function and an acquisition function for determining the next set of samples. Alternatively, the acquisition function offers sample points in the searching region as well. The function drives the tradeoff exploration and exploitation processes. Exploitation is a sample whereas the statistical models predict the highest objective scores while exploration refers to the return of a solution: The point estimate is either with a large posterior mean or large (x). The sampling is done at the location where the forecast uncertainty is higher. Then, the objective is estimated based on the initial space‐filling analysis model. This model frequently contains only those points that are selected randomly and iteratively used for allocating the remainder of the N function's budget evaluation, demonstrated in Algorithm 1. For the BO algorithm, the time complexity is (n3) in which n represents the observation counts. The time complexity for DL is (w⋅m⋅e), where w represents the weight counts, m indicates the learning instance counts, and e indicates the running epochs. In this study, hyperparameter tuning was conducted by the researchers based on the attention module. The filter counts exploited in every attention layer can be enhanced. The optimization technique defines a better integration of the filter numbers for every attention layer.
3.3. Optimal fruit classification process
In order to identify the fruit diseases, the SSAE classifier is used. AE is a NN with multiple Hidden Layers (HLs) of unsupervised feature learning [25]. The basic design is to apply more than one layer of NN for mapping the input dataset to attain the output vectors. Furthermore, the AE is applied to reduce the data feature sizes that could characterize both linear and non-linear conversions. The unlabeled input vector is exposed to weight mapping so as to attain the values of HL resultant vector as formulated below.
Let yi be the activation value of the HL, Wij indicates the weight coefficient, bi represents the offset vector of HL and S(x) represents the activation function and the mathematical expression is:
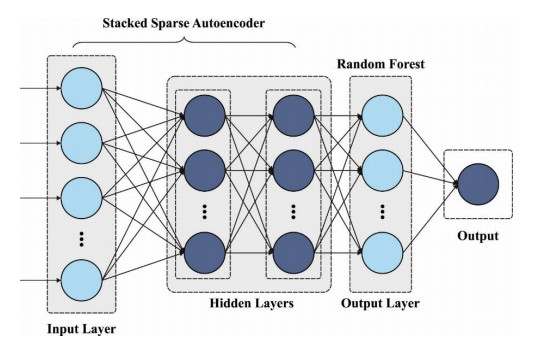
The weight parameter from the input layer to the HL is θ={W,b}, and the weight parameter in the HL to the resultant layer is represented as θ'={W',b'}. Figure 2 shows the architecture of the SSAE model.
Once a certain number of neurons is acquired from the HL, then they are considered as features so as to reduce the data size. Due to the existence of various neurons from the HL, a sparsity constraint is added for training the network and extracting valuable features.
here, ρ indicates the sparsity parameter; S2 denotes the number of neurons from the HL; β characterizes the penalty factor to control sparsity; and KL(ρ‖ρj) indicates the difference between ρ and ˆρj.
Typically, a simple SAE is not suitable for training purposes. So, the current study adopts a stacking technique in which every HL is separately trained with unsupervised learning of SAE. Then, these layers are linked to produce a stacked network. Every sensor extracts 31 feature parameters in the vibration signal which are signified as 31xN input feature vector as SSAE.
To raise the classification effectiveness of the SSAE system, the BRO technique is utilized in the current study. Rahkar Farshi (2020) introduced the BRO algorithm based on battle royal video game. In this game, the player needs to make use of and explore the surroundings to survive [26]. All the players outside the safer region get affected in two ways while the former is exposed to the risk of being removed from the battle.
The early population of the BRO technique is made up of n randomly-produced individuals in D-dimensional region. xti(i∈[1,n]) and xti,dam represent the location data and damage level of the ith player in tthiteration amongst the population, correspondingly. The player from the best location who stayed back in the original location is termed as the winner. However, the loss level is fixed as 0. The player in a worse position gets damaged during a mutual attack and so, they are named as loser. The loss levels of the loser can be upgraded, as demonstrated in Eq (10). Furthermore, due to the global best place and the present place, the location of the loser is upgraded, as demonstrated in Eq (11) and it aims to defend itself and attack the enemy from another side.
In this expression, r indicates the coefficient (r∈[0,1]). Once a player becomes the winner, then the damage levels return to 0. In order to emphasize the exploration process, once the player's cumulative damage levels exceed the predetermined value (thre=3), the player dies and respawns from the present safer region. In this scenario, the damage level returns to 0. The average player respawns from the present safer region, as given below.
In Eq (12), ubd and lbd denote the lower and upper boundaries of the safer region. Furthermore, during the iteration procedure, if the iteration count is better than the area update threshold (Δ), then the safer region gets reduced by the global optimum location while the center, the upper and lower limits are demonstrated as follows:
here, SD(¯xd) denotes the standard deviation, xbest indicates the existing optimum solution. lbd and ubd exceed the lower and upper boundary of the solution space as given below.
The initial value Δ is log10(Tmax),andTmaxrepresents the maximal iteration counts. The pseudocode of the BRO algorithm is shown in Algorithm 2.
The BRO algorithm grows an FF to realize a better effectiveness of the classifier and finds a positive integer to represent the optimal outcomes of the candidate. Here, FF corresponds to a reduced classifier error rate.
4.
Performance validation
The performance of the proposed BROFF-FDGC system, in terms of FDD, was validated using the the CASC IFW Database [27]. Table 1 provides the details about the dataset. Figure 3 shows some of the sample images. The images in the dataset were collected from the Internal Feeding Worm (IFW) dataset of Comprehensive Automation for Specialty Crops (CASC) research work after obtaining previous consent from Purdue University. The dataset contains images for four cultivars of apples: Golden Delicious, Fuji, York, and Red Delicious. Every cultivar has images in different phases of ripening of defective and healthy apples. The images of the damaged apples show noticeable dark spots on the outside skin as a result of damage from internal feeding worms. Individual apple images were clipped to a size range of 120 × 120 pixels for the purpose of image processing.
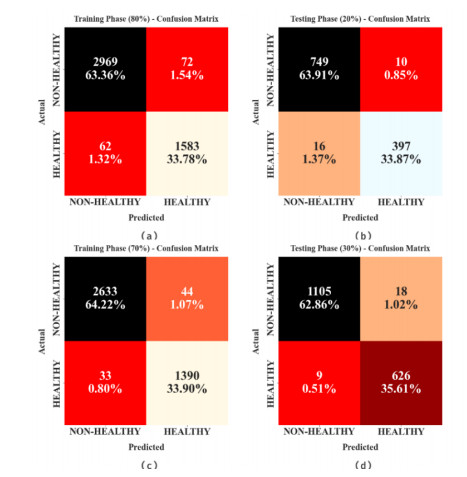
The confusion matrices generated by the BROFF-FDGC methodology on the FDD process are shown in Figure 4. The simulation values specify that the BROFF-FDGC approach correctly classified the healthy and damaged apples.
Table 2 offers a brief overview on the FDD results accomplished by the BROFF-FDGC methodology with 80:20 of TR phase and TS phase. Figure 5 exhibits the overall FDD performance of the BROFF-FDGC system for 80% of the TR phase. The simulation values indicate that the BROFF-FDGC technique classified non-healthy and healthy apple images properly. In addition, it is also observed that the BROFF-FDGC technique attained an average accubal of 96.93%, sensy of 96.93%, specy of 96.93%, Fscoreof 96.87%, and an MCC of 93.73%.
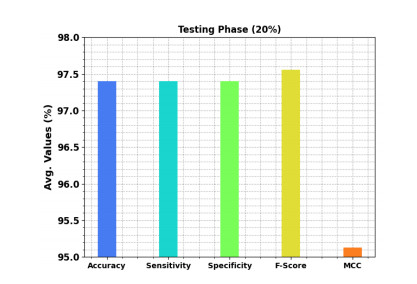
Figure 6 demonstrates the overall FDD examination outcomes achieved by the BROFF-FDGC technique on 20% of the TS set. The results point out that the BROFF-FDGC model can recognize non-healthy and healthy apple images accurately. Furthermore, it is also observed that the BROFF-FDGC technique accomplished an average accubal of 97.40%, sensy of 97.40%, specy of 97.40%, Fscoreof 97.56%, and an MCC of 95.13%.
Table 3 shows the comprehensive FDD analytical outcomes attained by the BROFF-FDGC technique with 70:30 of the TR set and TS set. Figure 7 shows the overall FDD analysis outcomes of the BROFF-FDGC system at 70% of the TR set. The obtained outcomes infer that the BROFF-FDGC technique identified non-healthy and healthy apple images accurately. Furthermore, it is also noted that the BROFF-FDGC approach accomplished an average accubal of 98.02%, sensy of 98.02%, specy of 98.02%, Fscoreof 97.93%, and an MCC of 95.87%.
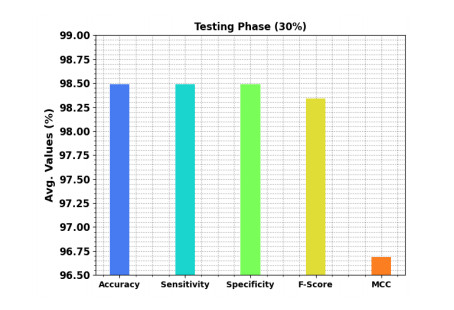
Figure 8 shows the overall FDD examination outcomes produced by the BROFF-FDGC method on 30% of the TS set. The outcomes indicate that the BROFF-FDGC method detected non-healthy as well as healthy apple images appropriately. Besides, it is observed that the BROFF-FDGC methodology accomplished an average accubal of 98.49%, sensy of 98.49%, specy of 98.49%, Fscoreof 98.34%, and an MCC of 96.69%.
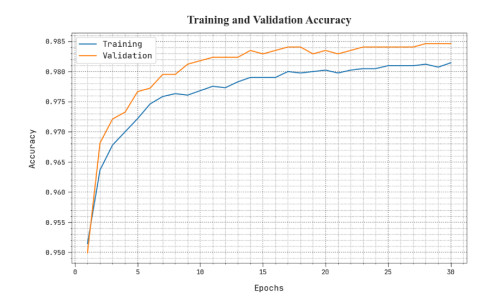
The TRAC and VDAC values achieved by the BROFF-FDGC system for the FDD solution are shown in Figure 9. The outcomes exhibit that the BROFF-FDGC method produced excellent outcomes with high TRAC and VDAC values. The BROFF-FDGC system attained the highest TRAC performance.
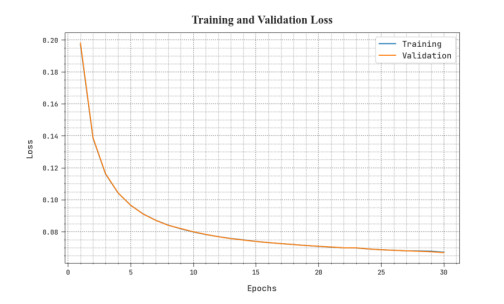
The TRLS and VDLS values, accomplished by the BROFF-FDGC technique in terms of FDD solution, are shown in Figure 10. The outcomes infer that the BROFF-FDGC system achieved better outcomes with lesser TRLS and VDLS values. This is obvious due to the fact that the BROFF-FDGC technique achieved the least VDLS performance.
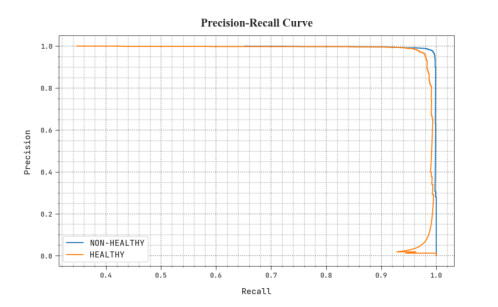
Figure 11 shows an apparent PR curve plotted with the values produced by the BROFF-FDGC system on the test database. The outcomes denote that the BROFF-FDGC method improved the PR outcomes on both the classes.
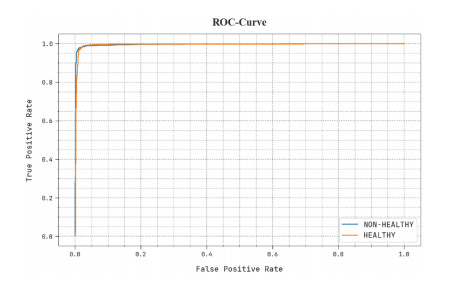
A comprehensive ROC inspection was conducted upon the BROFF-FDGC technique using the test database and the results are shown in Figure 12. The simulation values imply that the BROFF-FDGC methodology established its ability on both the classes.
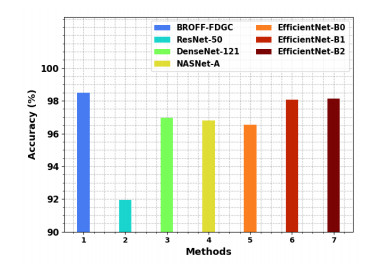
Table 4 shows the comprehensive analysis outcomes of the BROFF-FDGC system with recent models [28]. Figure 13 exhibits the comparative analysis results achieved by the BROFF-FDGC approach in terms of accuy. The simulation values indicate that the BROFF-FDGC approach gained an optimal solution with the maximal values of accuy. in terms of accuy, the BROFF-FDGC technique yielded a maximum accuy of 98.49% while the ResNet-50, DenseNet-121, NASNetA, EfficientNetB0, EfficientNetB1, and the EfficientNetB2 models accomplished the least accuy values such as 91.94%, 96.96%, 96.79%, 96.53%, 98.06%, and 98.15% correspondingly.
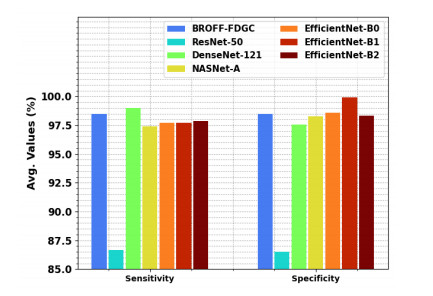
Figure 14 exhibits the comparative analytical outcomes of the BROFF-FDGC methodology in terms of sensy and specy. The outcomes demonstrate that the BROFF-FDGC system attained better performance with the maximum values of sensy and specy. With regards to sensy, the BROFF-FDGC technique produced a high sensy of 98.49% whereas the ResNet-50, DenseNet-121, NASNetA, EfficientNetB0, EfficientNetB1, and the EfficientNetB2 techniques achieved the least sensy values such as 86.65%, 99.01%, 97.39%, 97.71%, 97.69%, and 97.88% correspondingly.
Also, in terms of specy, the BROFF-FDGC method accomplished a maximum specy of 98.49% while the ResNet-50, DenseNet-121, NASNetA, EfficientNetB0, EfficientNetB1, and the EfficientNetB2 techniques achieved the least specy values such as 86.53%, 97.54%, 98.26%, 98.59%, 99.91%, and 98.34% correspondingly. Therefore, it can be inferred that the BROFF-FDGC technique shows maximum performance on FDD over other DL models.
5.
Conclusions
In the current study, a novel BROFF-FDGC methodology has been presented for detection and classification of the fruit diseases. The proposed BROFF-FDGC method integrates the BF-based noise removal, fusion-based feature extraction, BO-based hyperparameter tuning, SSAE classification, and BRO-based parameter optimization processes. A fusion of DL models namely Inception v3, NASNet, and Xception models is executed for the purpose of feature extraction. Besides, the BRO algorithm is also employed for optimum hyperparameter tuning of the SSAE technique. The BROFF-FDGC algorithm was extensively validated through simulation and the outcomes exhibit the greater performance. The obtained outcomes display the superior performance of the BROFF-FDGC approach compared to the rest of the methods. In the future, hybrid metaheuristic optimization algorithms can be designed to improve the FDD performance.
Use of AI tools declaration
The authors declare they have not used Artificial Intelligence (AI) tools in the creation of this article.
Conflict of interest
The authors declare that they have no conflicts of interest. The manuscript was written through the contributions of all authors. All authors have approved the final version of the manuscript.









 DownLoad:
DownLoad: