Fractional operators on the bounded symmetric domains of the Bergman spaces

1.   Introduction

The Atangana-Baleanu fractional differential operator (ABFDO) ^[1] has recently been used to define fractional derivatives (see ^[2,3,4]). Additionally, fractional derivatives with nonsingular kernels are crucial because some models of dissipation processes cannot be properly represented by the conventional fractional operators (see^[5,6,7]). Applications are presented using ABFDO together with different types of polynomials, such as the Chebyshev polynomial, B-spline polynomials and Alexander polynomials ^{[8,9,10,11,12]}.

For decades, classical fractional calculus based on the Riemann-Liouville fractional differential and integral operators has been used to define many classes of fractional analytic functions in an open unit disk. The recent work has demonstrated the ability to modify these classes and it has offered a combination between the most important special functions, called the generalized Mittag-Leffler function (the queen of special functions) and the formula for the fractional integral operator. This work can be suggested to develop linear operators (convolution operators), as well as the integral formula for the Bulboaca integral operator, Breaz integral operator and their generalizations. In addition, since the ABFDO involves the Mittag-Leffler function, it can be extended to k-calculus and q-calculus.

In a recent effort, we have extended the ABFDO to a complex domain (an open unit disk) to obtain the ABFDOs of a complex variable. To explore the geometric properties of the main operators, we have acted the operators on a special type of class of analytic functions with the $m$-fold symmetry characteristic in a bounded symmetric domain. This class of analytic functions is a natural generalization of the normalized analytic functions, when m = 1. The most discoveries in this direction involve demonstrating that the operators are convex and have starlike shapes in the open unit disk under some conditions. Moreover, the boundedness in the weighted Bergman and the convex Bergman spaces associated with a bounded symmetric domain is investigated. Duality relations are presented for these spaces. Our method is based on Young's convolution inequality.

The paper is divided into the following sections. Section 2 deals with the definition of the $m$-fold symmetric class of analytic functions and the formula that will be studied. Section 3 involves the preliminaries that will be utilized in the proof of our results. Section 4 includes the extended ABFDO and it contains the study of its geometrical characteristics. Sections 5 and 6 discuss the Bergman spaces for a bounded symmetric domain with applications. Section 7 presents the conclusion of the results and the future work.

2.   $m$-fold symmetric class

In this section, we deduce the meaning of the $m$-fold symmetric class of analytic functions in the open unit disk $\mathbb{D}: = \left\{\zeta\in\mathbb{C}:|\zeta| < 1\right\}$ (see ^[13]). In this investigation, we consider the class of $m$-fold symmetric functions $\Omega_m$ (see ^[14,15,16]), as follows:

Akgul ^[14] modified the class of $m$-fold symmetric functions in ^[13] to determine some coefficient results and complex inequalities. Seker and Taymur ^[15] described two new subclasses of bivalent functions, which are both $m$-fold symmetric analytic functions. In their study, they determined the upper bounds for the coefficients. Hamzat ^[16] has analyzed various features of fractional analytic functions belonging to two novel subclasses of $m$-fold symmetric starlike and convex functions in an open unit disk. Furthermore, features of a new subclass of $m$-fold symmetric bi-Bazilevic functions associated with modified sigmoid functions are addressed, as are numerous related minor repercussions.

Note that for $m = 1,$ we have the normalized function (the class is denoted by $\Omega$)

Corresponding to $\varphi_m(\zeta),$ we have the following class of function denoted by $\mathcal{T}\Omega_m$

where $\mathcal{T}\Omega$ is a special class of $\mathcal{T}\Omega_m, m = 1$ with

Definition 2.1. Functions $\varphi_m\in \Omega_m$ are considered to belong to the class of $(\kappa, m)$-Janowski starlike functions symbolized by $(\kappa, m)-\mathcal{ST}(u, v), \, -1\leq v < u\leq 1, \kappa\geq 0,$ whenever the following inequality is true

where $\Re$ indicates the symbol of the real part.

Also, we have the following class of convex functions:

Definition 2.2. Functions $\varphi_m\in \Omega_m$ are supposed to belong to the class of $(\kappa, m)$-Janowski convex functions symbolized by $(\kappa, m)-\mathcal{CT}(u, v), \, -1\leq v < u\leq 1, \kappa\geq 0,$ whenever the following inequality is true

When $m = 1,$ we have the Noor-Malik class described in ^[17].

3.   Lemmas

This section deals with the supplement results.

Lemma 3.1. (^[18], Theorem 2.4] or ^[19]HY__HY, Theorem 11.2]) If $\sigma, \varsigma, \tau \in \mathbb{C}$ with $\Re(\sigma) > 0, \Re(\varsigma) > 0, \Re(\tau) > 0$, then

Lemma 3.2. ^[20] For the function $\varphi(\zeta) = \zeta+\sum_{n = 2}^{\infty}a_{n}\zeta^{n},$ $\zeta \in \mathbb{D}$, if $\sum_{n = 2}^{\infty}(n-q)|a_{n}|\zeta^{n}\leq 1-q$ then $\varphi$ is starlike of order $q.$ Moreover, if $\sum_{n = 2}^{\infty}n(n-q)|a_{n}|\zeta^{n}\leq 1-q$ then $\varphi$ is convex of order $q.$

Lemma 3.3. ^[21] Let $\rho_1\geq 0,$ $\rho_2 > 0,$ and $\omega > 1/2$. If $f$ is starlike and $g$ is convex then the integral

is starlike of order $(2\omega-1)/2\omega.$

Lemma 3.4. ^[22] For some integer $m\geq 1,$ let

be analytic in $\mathbb{D}$ with its nonpositive real part in $\mathbb{D}.$ Then, there exists a point $\zeta_0\in \mathbb{D}$ with $\rho(\zeta_0) = i \xi$ and $\zeta_0\rho'(\zeta_0) = \vartheta,$ where $\vartheta \leq -m(1+\xi^2)/2.$

4.   Fractional differential operator

Numerous mathematical, physical and engineering fields make use of the Mittag-Leffler function, particularly in relation to fractional calculus, fractional differential equations and fractional order systems. It appears in issues with anomalous diffusion, viscoelasticity and memory effects. Recursive relations, integral representations and linkages to other special functions are only a few of the Mittag-Leffler function's intriguing characteristics. It is essential to fractional calculus and serves as a potent tool for comprehending and resolving issues involving fractional derivatives and integrals.

A branch of fractional calculus, which is an extension of ordinary calculus, is the fractional operator based on the Mittag-Leffler function. It has been applied to simulate a variety of physical events and is especially helpful when representing non-local or memory effects-based systems. There are numerous scientific and engineering domains for which the Mittag-Leffler function and the fractional operator it defines are applied, including physics, biology, economics and signal processing. These methods offer a more thorough framework for comprehending and examining intricate systems involving fractional order dynamics. The advantages of using the Mittag-Leffler function include, but are not limited to the following observations. It is simpler to deal with the Mittag-Leffler function in theoretical analysis and modeling since it has good features. It makes the exploration of fractional operators more approachable by allowing mathematicians and scientists to find closed-form solutions to fractional differential equations. In order to ensure that numerical simulations and approximations are well-behaved and accurately converge to the true solution, it gives stable solutions to fractional differential equations. Regarding its relationship to practical applications, in numerous real-world applications, such as the modeling of biological systems, financial mathematics, control systems, and diffusion operations in porous media, fractional calculus with the Mittag-Leffler function has shown great potential.

In this section, we proceed to extend the ABFDO in $\mathbb{D}$.

Definition 4.1. The generalized Mittag-Leffler function is defined by

where $(\alpha)_n$ represents the Pochhammer symbol.

We will employ double Mittage-Leffler functions in definition to display the modified ABFDOs of a complex variable.

Definition 4.2. For $\varphi_m \in \Omega_m,$ the extended fractional operators are given, as follows:

and

where $\omega$ indicates the power of $\zeta$ in the power series of $\varphi_m(\zeta).$

Example 4.3. Suppose that $\varphi_m(\zeta) = \zeta.$ By Lemma 3.1, we have

where $(y)_n = y(y+1)...(y+n-1).$ And,

As a result, we obtain the relation $^{ABC}\Delta_\zeta^\nu (\zeta) = \, ^{ABR}\Delta_\zeta^\nu (\zeta).$ In general, we get

We have the following result:

Proposition 4.4. Let $\varphi_ m \in \Omega_m$ and $b(\nu): = \dfrac{w(\nu)}{(1-\nu)}.$ Then,

and

Proof. Let $\varphi_m \in \Omega_m.$ A calculation implies that

where $\sigma_{mn}^{\nu, \mu}: = (nm+1)\left(\dfrac{E^2_{\nu, 2+mn}\left(-\mu_\nu\right)}{E^2_{\nu, 2}\left(-\mu_\nu\right)}\right),$ and the notation "$*$" indicates the convolution product. Thus, we conclude that $^{\mathfrak{ABC}} \Delta_\zeta^\nu\varphi_m(\zeta) \in \Omega_m.$ Similarly, we have that $^{\mathfrak{ABR}} \Delta_\zeta^\nu\varphi_m(\zeta) \in \Omega_m.$ □ Note that the integral corresponding to $^{\mathfrak{ABC}} \Delta_\zeta^\nu\varphi_m(\zeta)$ is given by the following series:

satisfying

A modification of the ABFDO is given for the normalized univalent functions and quantum analytic functions described in ^[23].

4.1.   Properties of the operator

In this part, we shall investigate the most important geometric properties of the operator $^{\mathfrak{ABC}} \Delta_\zeta^\nu\varphi_m(\zeta) \in \Omega_m.$

Theorem 4.5. The operator $^{\mathfrak{ABC}} \Delta_\zeta^\nu\varphi_m(\zeta) \in \Omega_m$ can be included in the class $(\kappa, m)-\mathcal{ST}(u, v), \, -1\leq v < u\leq 1, \kappa\geq 0,$ if it satisfies the following condition:

Proof. We aim to show that

A computation yields

If the condition (4.3) is true, the last assertion is bounded by 1, which completes the proof. □

For a comparison with other works, we have the following observations:

● $m = 1$ and $\sigma_{nm+1}^{\nu, \mu} = 1 \Rightarrow$ ^[17];

● $m = 1, u = 1, v = -1$ and $\sigma_{nm+1}^{\nu, \mu} = 1 \Rightarrow$ ^[24];

● $m = 1, u = 1-2a, a \in [0, 1), v = -1$ and $\sigma_{nm+1}^{\nu, \mu} = 1 \Rightarrow$ ^[25];

● $m = 1, u = 1-2a, a \in [0, 1), v = -1, \kappa = 0$ and $\sigma_{nm+1}^{\nu, \mu} = 1 \Rightarrow$ ^[20].

In a similar proof of Theorem 4.5, we have the following result:

Theorem 4.6. The operator $^{\mathfrak{ABC}} \Delta_\zeta^\nu\varphi_m(\zeta) \in \Omega_m$ is included in the class $(\kappa, m)-\mathcal{CT}(u, v), \, -1\leq v < u\leq 1, \kappa\geq 0,$ if it satisfies the following condition:

Theorem 4.7. Let $\varphi_m \in \Omega_m$ be starlike of order $\wp, \wp \in [0, 1)$ with non-positive coefficients ($a_{nm+1}\leq 0$). Moreover, let

Then,

(1) $[\, ^{\mathfrak{ABC}} \Delta_\zeta^\nu\varphi_m(\zeta)]$ achieves the starlikeness under the order $\wp$.

(2) It satisfies the boundedness inequality

(3) Its derivative reaches the following maximum bound and minimum bound:

(4) The maximal function is given by the formula

$(5)$ If $\, \sigma_m (\zeta)$ and $\varphi_m(\xi)$ are starlike of order $\wp$, then $[^{\mathfrak{ABC}} \Delta_\zeta^\nu\varphi_m(\zeta)]$ is starlike of order $q,$ where

Proof. By the positivity of the connections, $\varphi_m$ can be represented by the power series

In addition, since $\varphi_m$ is starlike of order $\wp,$ where $\wp \in [0, 1)$ and the following inequality is satisfied:

then, according to Lemma 3.2, we get the starlikeness of $[^{\mathfrak{ABC}} \Delta_\zeta^\nu\varphi_m(\zeta)]$ under the order $\wp$.

Using the leader component, we can obtain

which yields

Consequently, we get

and

We get the second portion by combining the two inequalities above.

Using the information below,

we have

and

We get the third item when we combine the above inequalities. The maximal function obtained from a direct calculation is as follows:

which completes part four.

Using the definition of the convolution product, we get

where $\sigma _{m}$ and $\varphi _{m}$ are starlike of order $\wp.$ To prove the starlikeness of $^{\mathfrak{ABC}} \Delta_\zeta^\nu\varphi_m(\zeta),$ it is sufficient to show that

Since

and

the Cauchy-Schwarz inequality implies that

where

But,

or, equivalently,

But the above fraction is an increasing function; thus, by letting $n = 1,$ the inequality of the above conclusion yields

Hence, according to Lemma 3.2, we have that $[^{\mathfrak{ABC}} \Delta_\zeta^\nu\varphi_m(\zeta)]$ is starlike of order $q.$ □

Theorem 4.8. Assume the convexity of $\varphi_m \in \Omega_m$ with order $\wp, \wp \in [0, 1)$ and non-positive coefficients ($a_{nm} \leq 0$). Moreover, suppose that

Then,

(1) $[^{\mathfrak{ABC}} \Delta_\zeta^\nu\varphi_m(\zeta)]$ achieves convexity under order $\wp$.

(2) It satisfies the boundedness inequality

(3) Its derivative admits the following boundedness inequality:

(4) The maximal function is given by the formula

$(5)$ If $\sigma_m$ and $\varphi_m$ are convex of order $\wp$, then $[^{\mathfrak{ABC}} \Delta_\zeta^\nu\varphi_m(\zeta)]$ is convex of order $\wp,$ where

Proof. Assume that

satisfies the inequality

And in view of Lemma 3.2 (the second part), we have that $[^{\mathfrak{ABC}} \Delta_\zeta^\nu\varphi_m(\zeta)]$ admits a convexity under order $\wp$.

As a consequence of the above conclusion, we get

which yields

Moreover, we have

Thus, we are left with the second and third sections, respectively. Clearly, the formula gives the greatest sharp function, as follows:

A convolution property implies that

where $\sigma _m$ and $\varphi_m$ are convex of order $\wp.$ To obtain that $[^{\mathfrak{ABC}} \Delta_\zeta^\nu\varphi_m(\zeta)]$ is convex of order $q,$ we obtain that

Since

and

the Cauchy-Schwarz inequality yields

where

But,

or, equivalently, we have the increasing inequality

By assuming that $n = 1,$ computation yields

Hence, $[^{\mathfrak{ABC}} \Delta_\zeta^\nu\varphi_m(\zeta)]$ addresses the convexity under order $q.$ □

Theorem 4.9. Consider the operator $[^{\mathfrak{ABC}} \Delta_\zeta^\nu\varphi_m(\zeta)].$ Then,

where $g_m$ is a convex univalent function, $\omega > 1/2,$ $\rho_1\geq0$ and $\rho_2 > 0.$

Moreover, if

then

where $g$ is a convex univalent function and $\rho_1\geq0$ and $\rho_2 > 0.$

Proof. Let

First, we must show that

By the definition of $[^{\mathfrak{ABC}} \Delta_\zeta^\nu\varphi_m(\zeta)],$ we have

As a consequence, we obtain (4.5). By the convexity of $g_m,$ we attain that it is in the class $\mathcal{S}^* (1/2)$. Since the multiplication of starlike functions implies starlikeness, $I[F, G]_m(\zeta)$ admits starlikeness of order $(2\omega-1)/2\omega$ (see Lemma 3.3). The second part of the theorem is valid when $g_m(\zeta) = \zeta/(1-\zeta^ m)$ and $\rho_2 = 1.$

□ We have the following outcome for some geometric inequalities:

Theorem 4.10. Consider the operator $[^{\mathfrak{ABC}} \Delta_\zeta^\nu\varphi_m(\zeta)].$ If

then

(1) $\Re \left(\dfrac{\zeta[^{\mathfrak{ABC}} \Delta_\zeta^\nu\varphi_m(\zeta)]'}{[^{\mathfrak{ABC}} \Delta_\zeta^\nu\varphi_m(\zeta)]} \right) < \dfrac{1}{2};$

(2) or, equivalently, $\left|\dfrac{\zeta [^{\mathfrak{ABC}} \Delta_\zeta^\nu\varphi_m(\zeta)]' }{[^{\mathfrak{ABC}} \Delta_\zeta^\nu\varphi_m(\zeta)]}-1\right| < 1.$

Proof. Note that $\Re \left(\dfrac{\zeta[^{\mathfrak{ABC}} \Delta_\zeta^\nu\varphi_m(\zeta)]'}{[^{\mathfrak{ABC}} \Delta_\zeta^\nu\varphi_m(\zeta)]} \right) < \dfrac{1}{2}$ is equivalent to $\Re \left(\dfrac{[^{\mathfrak{ABC}} \Delta_\zeta^\nu\varphi_m(\zeta)]}{\zeta[^{\mathfrak{ABC}} \Delta_\zeta^\nu\varphi_m(\zeta)]'} \right) > \dfrac{1}{2}.$ Based on the above inequality, we define the following function:

Then one can write the formula series $\rho(\zeta) = 1+\rho_m\zeta^m+\rho_{m+1}\zeta^{m+1}+...\,$ by using

Assume that $\rho(\zeta)$ does not have a positive real component. Lemma 3.4 states that a point $\zeta_0$ belongs to $\mathbb{D},$ where $\rho(\zeta_0) = i \xi$ and $\zeta_0\rho(\zeta_0) = \vartheta,$ where $\vartheta \leq-m(1+\xi^2)/2.$ Direct computation yields

This leads to the assertion made by this theorem. □

Not that, when $m = 1$ and $\sigma_{nm+1}^{\nu, \mu} = 1,$ we obtain the result presented in ^[26], and when $\sigma_{nm}^{\nu, \mu} = 1,$ we have the result presented in ^[13]. Moreover, Theorem 4.10 can be considered for the integral operators in Theorem 4.9 and the fractional operator corresponds to $[^{\mathfrak{ABC}} \Delta_\zeta^\nu\varphi_m(\zeta)].$

Theorem 4.11. Consider the operator $[^{\mathfrak{ABC}} \Delta_\zeta^\nu\varphi_m(\zeta)].$ If

for $k \in \mathbb{Z}_{+}$ and

then

Proof. By the assumption of the theorem, we have

and

Direct computation yields

and

Combining the above inequalities, we obtain

We proceed to show that $\Re \left(\dfrac{\zeta[^{\mathfrak{ABC}} \Delta_\zeta^\nu\varphi_m(\zeta)]'}{[^{\mathfrak{ABC}} \Delta_\zeta^\nu\varphi_m(\zeta)]_k }\right) > 0.$ Let $\rho(\zeta) = \dfrac{\zeta[^{\mathfrak{ABC}} \Delta_\zeta^\nu\varphi_m(\zeta)]'}{[^{\mathfrak{ABC}} \Delta_\zeta^\nu\varphi_m(\zeta)]_k}.$ Then, by the formula series $\rho(\zeta) = 1+\rho_m\zeta^m+\rho_{m+1}\zeta^{m+1}+...\,$ can be applied

Assume that $\rho(\zeta)$ does not have a positive real component. Lemma 3.4 states that $\zeta_0$ belongs to $\mathbb{D}$ with $\rho(\zeta_0) = i \xi$ and $\zeta_0\rho(\zeta_0) = \vartheta,$ where $\vartheta \leq-m(1+\xi^2)/2.$ Direct computation yields

This contradicts the assertion made by this theorem. Hence, $\Re\left(\dfrac{\zeta[^{\mathfrak{ABC}} \Delta_\zeta^\nu\varphi_m(\varpi^n \zeta)]'}{[^{\mathfrak{ABC}} \Delta_\zeta^\nu\varphi_m(\varpi^n \zeta)]_k}\right) > 0.$ □

The above theorem is valid for the integrals in Theorem 4.9 and the integral corresponds to $[^{\mathfrak{ABC}} \Delta_\zeta^\nu\varphi_m(\varpi^n \zeta)].$

Example 4.12. Consider the fractional differential equation

Equation (4.6) has the following expression:

which satisfies

thus, it is starlike. Let

then, according to Theorem 4.9,

where $g_m$ is a convex univalent function, $\rho_1\geq 0$ and $\rho_2 > 0.$

5.   Bergman spaces for a bounded symmetric domain

In this part, we study the boundedness of the operator $^{\mathfrak{ABC}} \Delta_\zeta^\nu\varphi_m(\zeta)$ in some well-known spaces. We shall use the Bergman space of analytic functions in $\mathbb{D}$ (bounded symmetric domain). The weighted Bergman space is a modification of the Bergman space in which functions are not only square integrable, but they also have integrability that can be quantified in terms of a specific weight function. The space of functions that are square-integrable with respect to this weight is affected by this weight function, which imposes varying weights on various places in the bounded symmetric domain. Weighted Bergman spaces have been utilized to investigate diverse analytic function qualities for certain domains and particular actions selected by the weight function. Alongside other mathematical disciplines these spaces have uses in complex analysis, potential theory, and harmonic analysis. The characteristics of the related weighted Bergman space might be very different for a given domain and weight function. Understanding how analytic functions behave in relation to the domain's underlying geometry and weight distribution can be accomplished by looking at the properties of functions in these spaces. These realizations may then contribute to a deeper comprehension of sophisticated analysis and associated mathematical ideas.

The weighted Bergman space is a set of all analytic functions in $\mathbb{D}$ (bounded symmetric domain) with the norm ^[27]

The convex structure is formulated when $\gamma \in (0, 1/2],$ as follows:

where $d \Lambda = d\zeta/\pi$ is the area measure. Note that, when $\beta = \frac{1-2 \gamma}{\gamma},$ we obtain the weighted space. In addition, the non-normal weighted logarithmic Bergman space is defined as follows ^[28]:

The two parameter normal weighted logarithmic Bergman space is defined as follows ^[29]:

Finally, the general weighted Bergman spaces have the following structure:

Alternatively, they have the following parametric structure ^[30]:

We have the following result of this section:

Theorem 5.1. Consider the operator $^{\mathfrak{ABC}} \Delta_\zeta^\nu\varphi_m(\zeta),$ where $\varphi_m \in \Omega_m.$ Then,

(1) $\|\varphi_m\|_{\mathfrak{B}_p^\beta} \Leftrightarrow \|^{\mathfrak{ABC}} \Delta_\zeta^\nu\varphi_m\|_{\mathfrak{B}_p^\beta }, \quad \beta > -1;$

(2) $\|\varphi_m\|_{\mathfrak{B}_p^\gamma } \Leftrightarrow \|^{\mathfrak{ABC}} \Delta_\zeta^\nu\varphi_m\|_{\mathfrak{B}_p^\gamma }, \quad \gamma \in (0, 1/2];$

(3) $\|\varphi_m\|_{\mathfrak{B}_{p, \log}^\beta} \Leftrightarrow \|^{\mathfrak{ABC}} \Delta_\zeta^\nu\varphi_m\|_{\mathfrak{B}_{p, \log}^\beta }, \quad \beta > 1;$

(4) $\|\varphi_m\|_{\mathfrak{B}_{p, \log}^{\beta, \gamma}} \Leftrightarrow \|^{\mathfrak{ABC}} \Delta_\zeta^\nu\varphi_m\|_{\mathfrak{B}_{p, \log}^{\beta, \gamma} }, \quad \beta \leq 0, \gamma > -1;$

(5) $\|\varphi_m\|_{\mathfrak{B}^{p }_{ \omega}} \Leftrightarrow \|^{\mathfrak{ABC}} \Delta_\zeta^\nu\varphi_m\|_{\mathfrak{B}^{p }_{ \omega}}, \quad \omega \in L^1(\mathbb{D});$

(6) $\|\varphi_m\|_{\mathfrak{B}^{p }_{ \omega^\sharp}} \Leftrightarrow \|^{\mathfrak{ABC}} \Delta_\zeta^\nu\varphi_m\|_{\mathfrak{B}^{p }_{ \omega^\sharp}}, \quad \omega^\sharp(\zeta) = \omega(\zeta) \varpi ^\alpha(\zeta)\in L^1(\mathbb{D}), \, \alpha \in \mathbb{R}.$

Proof. Let $\varphi_m \, \in \mathfrak{B}_p^\beta.$ Assume that

Then, for $p\geq 1$, Young's inequality of the convoluted functions implies that

Thus, $^{\mathfrak{ABC}} \Delta_\zeta^\nu\varphi_m \in {\mathfrak{B}_p^\beta }.$ Conversely, let

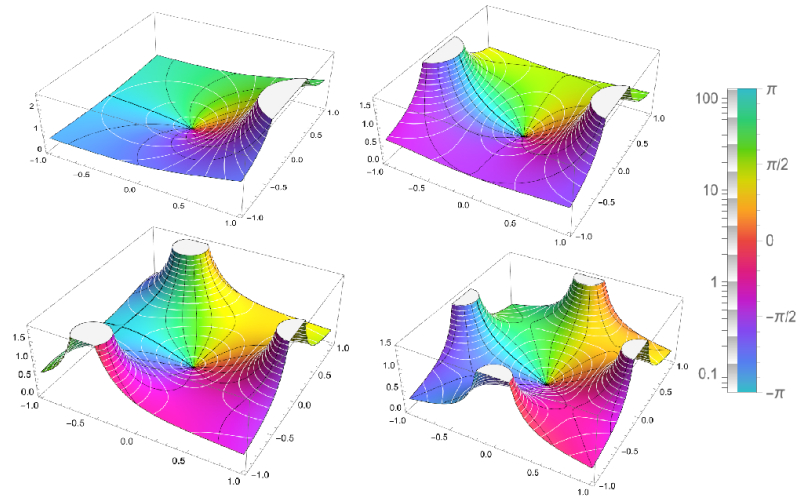
and assume that $\|^{\mathfrak{ABC}} \Delta_\zeta^\nu\varphi_m\|_{\mathfrak{B}_p^\beta } < \infty.$ Analogous to $\sigma_m(\varsigma),$ define the function $\varsigma_m(\zeta)$ as follows (see Figures 1 and 2):

Then, Young's inequality yields

Thus, $\varphi_m \in \mathfrak{B}_p^\beta.$

The process is similar for the other above listed cases. □

Remark 5.2. Figures 1 and 2 present the $m$-symmetrical behavior of the Koebe function, which is the extreme function of the convexity in an open unit disk. The Koebe function is an extreme function in a number of univalent function problems. The $m$-symmetric Koebe function is a useful mathematical tool for complex analysis and conformal mapping theory. It helps mathematicians and scientists to understand and work with conformal mappings, which have numerous applications in physics and engineering. While it lacks a direct physical interpretation, the characteristics and theorems it references can be used to solve real-world problems involving complicated shapes and locations.

In the same manner of Theorem 5.1, we have the following result regarding the operator $^{\mathfrak{ABR}} \Delta_\zeta^\nu\varphi_m(\zeta).$

Theorem 5.3. Consider the operator $^{\mathfrak{ABR}} \Delta_\zeta^\nu\varphi_m(\zeta),$ where $\varphi_m \in \Omega_m.$ Then, consider the following:

(1) $\|\varphi_m\|_{\mathfrak{B}_p^\beta} \Leftrightarrow \|^{\mathfrak{ABR}} \Delta_\zeta^\nu\varphi_m\|_{\mathfrak{B}_p^\beta }, \quad \beta > -1;$

(2) $\|\varphi_m\|_{\mathfrak{B}_p^\gamma } \Leftrightarrow \|^{\mathfrak{ABR}} \Delta_\zeta^\nu\varphi_m\|_{\mathfrak{B}_p^\gamma }, \quad \gamma \in (0, 1/2];$

(3) $\|\varphi_m\|_{\mathfrak{B}_{p, \log}^\beta} \Leftrightarrow \|^{\mathfrak{ABR}} \Delta_\zeta^\nu\varphi_m\|_{\mathfrak{B}_{p, \log}^\beta }, \quad \beta > 1;$

(4) $\|\varphi_m\|_{\mathfrak{B}_{p, \log}^{\beta, \gamma}} \Leftrightarrow \|^{\mathfrak{ABR}} \Delta_\zeta^\nu\varphi_m\|_{\mathfrak{B}_{p, \log}^{\beta, \gamma} }, \quad \beta \leq 0, \gamma > -1;$

(5) $\|\varphi_m\|_{\mathfrak{B}^{p }_{ \omega}} \Leftrightarrow \|^{\mathfrak{ABR}} \Delta_\zeta^\nu\varphi_m\|_{\mathfrak{B}^{p }_{ \omega}}, \quad \omega \in L^1(\mathbb{D});$

(6) $\|\varphi_m\|_{\mathfrak{B}^{p }_{ \omega^\sharp}} \Leftrightarrow \|^{\mathfrak{ABR}} \Delta_\zeta^\nu\varphi_m\|_{\mathfrak{B}^{p }_{ \omega^\sharp}}, \quad \omega^\sharp(\zeta) = \omega(\zeta) \varpi ^\alpha(\zeta)\in L^1(\mathbb{D}), \, \alpha \in \mathbb{R}.$

6.   Applications

Numerous mathematical disciplines, such as complex analysis, functional analysis, harmonic analysis, operator theory and others, all make use of the Bergman space. It is an invaluable instrument for comprehending and resolving issues in these sectors because of its adaptability and connections to diverse mathematical disciplines. A symmetric function is one that does not change when its variables are permuted. An $m$-fold symmetric function is a special form of symmetric function in which the variables are permuted by separating them into $m$ distinct subgroups and permuting the variables within each subset. The physical meaning of an $m$-fold symmetric function is determined by the situation. Here are a handful of samples to demonstrate its significance in many fields:

● In physics, $m$-fold symmetric functions can describe the action of material properties that maintain some kind of symmetry when seen from multiple locations or orientations inside the crystal lattice, particularly in the study of solid-state materials and crystals. When investigating the electronic band structure of crystals, for example, $m$-fold symmetric functions can help scholars to describe the energy levels and wave functions of electrons in the crystal while taking the crystal's symmetry into account (see ^[31]).

● In chemistry, symmetry is important for the classification of molecular structures and their spectroscopic properties. The symmetry of molecular vibrations, electronic states and other features can be described by using $m$-fold symmetric functions. For instance, when evaluating a molecule's vibration modes, $m$-fold symmetric functions can aid in the determination of which modes are Raman-active or infrared-active, as predicated on their symmetry-related features (see ^[32]).

● In engineering, $m$-fold symmetric functions can be utilized to examine signals or systems that display specified symmetries, particularly in signal processing and control theory. By taking advantage of the underlying symmetries, this may simplify system analysis and implementation (see ^[33]). Other applications are discussed in ^{[34,35,36,37]}.

In this part, we study the estimate of the fractional equation

when

It is well known that the $\overline{\partial}(.) = \frac{\partial(.)}{ \partial \overline{\zeta}} d \overline{\zeta}$ equation has many applications in different fields, including mathematical physics and fluids.

Theorem 6.1. Consider the Eq (6.1). Then, it admits a solution satisfying the finite inequality

where $\omega$ is the decreasing weight. Furthermore,

where $C_1, C_2$ are positive constants.

Proof. It is enough to prove that

Define an analytic function on the disk $\mathbb{D}(r_0),$ where

as follows:

Let $\chi_n$ be a partition covering the disk $\mathbb{D}(r_n), r_n \leq r < 1$ with $|\chi_n(\xi)| < 1.$ Suppose that

According to the Cauchy-Pompeiu formula, we get

Then, it can be extended by the power series

Thus, we obtain

Assume that

and

We aim to show that

According to Hölder's inequality, we obtain

Now, in view of Fubini's theorem, and by using the inequalities (6.4) and (6.5), we have

where

and $\chi_n(\xi)$ is considered for $\mathbb{D}(r_n), r_n < 1$ with $|\chi_n(\xi)| < 1.$ Then, we obtain (6.2). Since $\varphi_m \in \mathfrak{B}^{p}_{ \omega^ \sharp},$ Theorem 5.1 yields (6.3).

The proof is completed. □

7.   Conclusions

Working on a specific kind of class of analytic functions with the $m$-fold symmetry feature in a complex domain, we expanded the fractional differential operator. We have illustrated a set of geometric properties of this operator including the uniform starlike and uniform convex shapes (Theorem 4.5). Sufficient conditions on this operator are presented to be starlike in terms of double $(\kappa, m)$-symmetric-conjugate points (Theorems 4.10 and 4.11). Under some conditions, the operator preserves some integral formulas (Theorem 4.9). Sharpness for some geometric properties has been indicated. Applications in the field of fractional differential equations are presented to determine the geometric behavior of the solutions in an open unit disk (Example 4.12). The final aim of this work was to study the symmetry of fractional differential operator in Bergman spaces for a symmetric domain. We suggest that the applications can to find the solution of the $\overline{\partial}$-equation whenever $\varphi_m \in \mathfrak{B}^{p}_{ \omega^ \sharp}.$ To summarize, the use of fractional derivatives of complex variables is a particular mathematical technique that involves applying fractional calculus to complex functions. They are applied in a variety of scientific and technical disciplines whereby complex systems or events must be investigated and simulated. A fractional derivative in the complex plane can be converted to a fractional Laplacian operator in some instances, which is a generalization of the Laplacian operator for real variables. Other properties can be considered in the future by using different classes of analytic functions, including the class of meromorphic functions, multi-valent functions and harmonic functions.

Use of AI tools declaration

The authors declare that they have not used Artificial Intelligence (AI) tools in the creation of this article.

Conflict of interest

The authors declare no conflict of interest.