A faster fixed point iterative algorithm and its application to optimization problems

1.   Introduction

Mathematicians are always interested in finding the solutions of nonlinear problems but some problems may not be approached by analytical methods to solve such problems, though the fixed theory plays a key role ^[1]. In this area, two major directions for research: One is for the existence of fixed points, and to establish a broad class of mappings and conditions; and the other is to define iterative processes to find those fixed points.

A prominent result of fixed point theory is due to the famous functional analysis founder Banach ^[2]. This prominent result provides the fixed point (FP) existence for a special class of nonlinear operators called contractions and comes up with an iterative approach to approximate the FP using an algorithm called the Picard iterative algorithm. By retaining the convergence property and by weaking the contraction hypothesis, the researchers attempted to generalize Banach's contraction.

Nonexpansive mappings are generalizations of contraction mappings. A selfmap $\eta$ on a nonempty subset $E$ of a Banach space $B$(from now to onward $B$ will represent Banach space) is called nonexpansive, if for all $x, y \in E\ \text{and}||\eta(x)-\eta(y)||\leq||x-y||$ holds. If there is atleast one FP for $\eta$, then we will denote and define the set of all FPs of $\eta$ by $F(\eta) = \{x:\eta(x) = x\}.$ If $\eta$ is nonexpansive and $F(\eta)$ is nonempty, and for all $x \in E$ and $p \in F(\eta)$, the inequaltiy $||\eta(x)-p||\leq||x-p||$ holds, and we regard such a selfmap as a quasi-nonexpansive on $E$ ^[3]. The nonexpansive selfmap $\eta$ of $E$ has a FP when the domain of the map is convex bounded and closed in any provided reflexive Banach space $B$ ^[4]. The existence of FPs for nonexpansive maps were also studied by Browder ^[5] and G{ö}hde ^[6], who obtain a result similar to the result in ^[4].

Suzuki ^[7] introduced a weaker notion for nonexpansive mappings known as Condition(C). A selfmap $\eta$ on subest $E$ of $B$ is regarded as mappings with Condition (C), if for any pair of points, $x, y \in E$ whenever $\frac{1}{2}||x-\eta(x)||\leq||x-y||, \ \text{then}\ ||\eta(x)-\eta(y)||\leq||x-y||\ \text{holds.}$ The class of mappings introduced by Suzuki ^[7] was a significant advancement because these mappings effectively extended the concept of nonexpansive nonlinear operators in a novel and straightforward manner. It is also important that the class of mappings that are enriched with the Condition(C) are also called as Suzuki's generalized nonexpansive mapping. Although Suzuki mappings with fixed points are quasi-nonexpansive and hence these mappings are not general than the class of quasi-nonexpansive maps. For proving that the Suzuki mappings properly includes nonlinear nonexpansive maps, some examples are constructed in ^[7,8] and other papers.

Aoyama and Koshaka ^[9] introduced the class of $\alpha$-nonexpansive maps in 2011, which are described as follows: Let $E$ be a subset of $B$. A selfmap $\eta$ on $E$ is said to be $\alpha$-nonexpansive, if $\alpha \in [0, 1)$ can be found such that the following inequality holds for each pair of $x, y \in E$.

Clearly, for $\alpha = 0$, we have again nonexpainsive mapping i-e., every nonexpansive mapping is a $\alpha$-nonexpansive but the converse is not valid. For $\alpha > 0$ an example in ^[9], which reveals the discountitnuity for $\alpha$-nonexpansive mappings, and shows the fact that the class of $\alpha$-nonexpanisve mappings is vast than the class of nonexpansive mappings. For more details, see ^[10].

In 2017, Pant and Shukla ^[11] studied a new type of extension of nonlinear nonexpansive maps in Banach spaces and suggested the concept of generalized $\alpha$-nonexpansive maps: The selfmap $\eta$ will be called a generalized $\alpha$-nonexpansive whenever one has

then,

for any choice of $x, y\in E$ and a real number $\alpha$, which is fixed and $0\leq \alpha < 1$.

In other words, the class of generalized $\alpha$-nonexpansive mappings contains nonexpansive mappings; however, the opposite is not true ^[11]. It is evident that for $\alpha = 0$, the generalized $\alpha$-nonexpansive mapping again becomes Suzuki's nonexpainsive mapping. In the context of Banach space, numerous mathematicians have attempted to approximate the FP of generalized $\alpha$-nonexapnsive mappings ^[12].

In 2019, Pant and Pandey ^[13] studied a new type of extension of nonlinear nonexpansive maps in Banach spaces and suggested the concept of $\beta$-nonexpansive maps: The selfmap $\eta$ will be called a Reich-Suzuki nonexpansive whenever one has

then,

for any choice of $x, y\in E$ and a real number $\beta$, which is fixed and $0\leq \beta < 1$.

Clearly, for $\beta = 0$, the $\beta$-Reich-Suzuki nonexpansive mapping becomes Suzuki's nonexpansive mapping i-e., every Suzuki map can be regarded as a Reich-Suzuki map but the converse is not true ^[13].

In 2020, Ullah et al. ^[14] enriched the study of nonexpansive mappings with a novel class of generalized mappings: The selfmap $\eta$ will be called a generalized $(\alpha, \beta)$-nonexpansive provided that

then,

for any choice of $x, y\in E$ and real numbers $\alpha, \beta$ which are fixed and $0\leq \alpha, \beta < 1$ and $\alpha+\beta\leq1$.

The authors in ^[14] compared this new class of maps with the already existing classes of mappings given above. More study on these mappings were carried out in ^[14,15], which further explored the importance of these mappings.

Numerous iterative algorithms for numerical solutions have been studied by various authors, with their applications extending to a wide range of applied sciences problems ^[16,17]. Banach result ^[2] shows that for any contraction in a complete spaces, the fixed point is essentially the limit point for the Picard algorithm. The iterative sequence $\{{x_n}\}$ can be produced using Picard iterations as:

The Picard algorithm generates the above sequence, which converges to the fixed point of contractive mapping but not of nonexpansive mapping in general.

Mann ^[18] presented an iterative approach to approximate FPs for nonexpansive mappings. For an appropriate sequence $\{\sigma_{n}\}$ in $(0, 1)$, the sequence $\{x_{n}\}$ obtained by Mann is:

If $\eta$ is pseudo-contractive mapping, the Mann algorithm fails to converge the FP of $\eta$. Ishikawa^[19] overcomes this problem by defining a two-steps iterative algorithm. For two appropriate sequences $\{\sigma_{n}\}$ and $\{\lambda_{n}\}$ in $(0, 1)$, then the sequence $\{x_{n}\}$ obtained by the Ishikawa algorithm is given as:

Noor^[20], the pioneer of three-steps iterative algorithms, introduced a three-steps iteration process in $2000$, which is faster than Ishikawa's two-steps iterative algorithm. For an arbitrary $x_{1}$ in $E$ and for three sequences of real numbers $\{\sigma_{n}\}$, $\{\lambda_{n}\}$, and $\{\xi_{n}\}$ in $(0, 1)$, then the sequence $\{x_{n}\}$ obtained by this algorithm is given as:

In $2007$, Agarwal^[21] introduced the following iterative algorithm:

where, $\{\sigma_{n}\}$ and $\{\lambda_{n}\}$ are sequences in $(0, 1)$.

Abbas and Nazir^[22] come with another three-step iterative algorithm in $2014$. For an arbitrary $x_{1}$ in $E$ and for three sequences of real numbers $\{\sigma_{n}\}$, $\{\lambda_{n}\}$, and $\{\xi_{n}\}$ in $(0, 1)$, then the sequence $\{x_{n}\}$ obtained by them is given as:

Thakur et al.^[8] proposed another three-step iterative algorithm in $2016$. For an arbitrary $\{x_{1}\}$ in $E$ and for three sequences of real numbers $\{\sigma_{n}\}$, $\{\lambda_{n}\}$, and $\{\xi_{n}\}$ in $(0, 1)$, then the sequence $\{x_{n}\}$ obtained by Thukar is given as:

Ullah and Arshad ^[23] introduced a new iterative algorithm in $2018$ known as $M$-Iteration. For an arbitrary sequence $x_{1}$ in $E$ and for appropriate sequence of real number $\{\sigma_{n}\}$ in $(0, 1)$, then the sequence $\{x_{n}\}$ obatained by the M-Iterative algorithm is:

Ullah et al.^[24] proposed a new iterative algorithm in 2022 known as the $KF$-Iteration. For an arbitrary $x_{1}$ in $E$ and for two appropriate sequences of real number $\{\sigma_{n}\}$ and $\{\xi_{n}\}$ in $(0, 1)$, then the sequence $\{x_{n}\}$ obatained by the KF-Iterative algorithm is:

In 2022, Abbas et al. ^[25] proposed the $AA$-Iterative algorithm. For the class of contractive mappings and enhanced contractive mappings, the $AA$-Iterative algorithm converges faster than the approaches outlined before. For an arbitrary $x_{1}$ in $E$ and for three sequences of real numbers $\{\sigma_{n}\}$, $\{\lambda_{n}\}$, and $\{\xi_{n}\}$ in $(0, 1)$, then the sequence $\{x_{n}\}$ obtained with the help of the $AA$-iterative algorithm is given as:

In 2019, Ali et al. ^[26] proved the convergence results for Suzuki's-type generalized nonexpansive mappings. To approximate the FP of a more generalized nonexpansive mapping as fast as possible is of great interest of mathematicians due to the theoratical and practical applications of fixed point theory in nonlinear equations. Motivated by ^{[14,15,25,26,27]}, we will prove some weak and strong convergence theorems using the $AA$-Iterative algorithm (1.10) for the class of generalized$(\alpha, \beta)$-nonexpasive mappings in uniformly convex Banach space $B$.

2.   Preliminaries

The following concepts are needed in the main outcome.

Definition 2.1. ^[28] Suppose $B$ represents a norm space which satisfies the condition: For each selected $\epsilon$ in the interval $(0, 2]$, one has a real number namely $0 < \delta < \infty$ such that $\|v-s\|\geq\epsilon$; then, for each $v, s \in B$ satisfying $\|v\|\leq1, \|s\|\leq1$, it follows that

If any norm space $B$ satisfying the above condition, we call it a uniformly convex norm space.

Definition 2.2. ^[29] For a given norm space $B$, we say that $B$ is endowed with the has Opial Property whenever for any sequence $\{s_n\}$ in the space $B$ if it is weakly convergent to $v$, it is the case that;

where $v\neq u$.

Definition 2.3. ^[30] Take a point, namely $s$, in a norm space $B$ and assume that $\{s_{n}\}$ is a bounded sequence composed of points of $B$. Consider the functional.

● Then, the asymptotic radius, which we denote here as $\Upsilon(E, \{s_n\})$, shows that $E$ is any subset of $B$ it reads as follows:

● Similarly, the asymptotic center is denoted by $A(E, \{x_n\})$ and reads as follows:

● In the case when $B$ is Banach space and uniformly convex, then the asymptotic center admits a unique point.

Definition 2.4. ^[29] Suppose $E \ne \emptyset$ be a closed convex subset of Banach space $B$ then, the mapping $\eta:E\to B$ is demiclosed, if for every $\{x_n\} \in E$ which converge weakly to some $x_{0}\in E$ and the strong convergence of sequence $\{\eta(x_n)\}$ to $y_{0} \in B$ $\implies$ $\eta(x_{0}) = y_{0}$.

Definition 2.5. ^[31] Assume that $E \ne \emptyset$ is a subset in a norm space $B$. The map $\eta:E\to E$ is called map with condition (I) whenever one has a funtion $\Upsilon:[0, \infty)\to[0, \infty)$ such that $\Upsilon(0) = 0$ and $\Upsilon(t) > 0, \forall\ t > 0$, one has

here, $d(s, F(\eta) = \text{inf} \{d(s, p): p \in F(\eta)\}.$

The following proposition is due to Ullah et al. ^[14].

Proposition 2.6. For a selfmap $\eta$ over the nonempty closed convex subset $E$ of $B$, then the following results can be obtained directly:

a. The Suzuki nonexpansiveness of $\eta$ leads us to the fact that $\eta$ is nonexpansive on the set $E$.

b. The generalized nonexpansiveness of $\eta$ leads us to the fact that $\eta$ is $\alpha$-nonexpansive on the set $E$.

c. The generalized nonexpansiveness of $\eta$ leads us to the fact that $\eta$ is $\beta$-Reich-Suzuki nonexpansive on the set $E$.

Lemma 2.7. ^[14] Any generalized $(\alpha, \beta)$- nonexpansive selfmap $\eta$ on any subset namely $E$ of a norm space $B$ form a quasi-nonexpansive map on $E$.

Lemma 2.8. ^[14] The fixed point set associated with a $(\alpha, \beta)$- nonexpansive selfmap $\eta$ is always closed in the setting of Banach space.

Lemma 2.9. ^[14] Any generalized $(\alpha, \beta)$- nonexpansive selfmap $\eta$ on any subset namely $E$ of a norm space $B$ satisfies the following:

for any choice of $v, s\in E$.

Lemma 2.10. ^[14] Assume that $\eta$ denotes any map that is essentially defined on a closed subset $E$ of any given complete norm space $B$. Suppose $E$ is endowed with the Opial Property and the map is generalized $(\alpha, \beta)$-nonexpansive on the set $E$. Consider $\{s_{n}\}$ as being convergent to any element $s\in B$ in the weak sense with $\lim\limits_{n\to\infty}\|\eta(s_{n})-s_{n}\| = 0$. In this case, $(I-\eta)$ is demiclosed at zero, i.e., $\eta s = s$.

Lemma 2.11. ^[32] (Property of uniform convexity) For Banach space $B$, which is uniformly convex, and take any sequence $0 < \zeta_{t} < 1, \forall t\in\mathbb{N}.$ Consider $\{x_{t}\}$ and $\{y_{t}\}$ that form two sequences of elements of $B$ satisfying $\limsup\limits_{t\to\infty}\|x_{t}\|, \limsup\limits_{t\to\infty}\|y_{t}\|\leq\rho, \ {\text{and}}\ \limsup\limits_{t\to\infty}\|\zeta_{t}x_{t}+(1-\zeta_{t})y_{t}\| = \rho$ where $\rho$ is a positive constant. Eventually, it follows that $\lim\limits_{t \to \infty} \|x_{t}-y_{t}\| = 0.$

3.   Weak and strong convergence results

In recent years, we note that different iterative methods are used for the fixed point construction of nonlinear maps. This section proposes new fixed point results for the faster iterative scheme (1.10) under mild conditions in a Banach space context. In all the major results, we write simply $B$ for a unifomly convex Banach space. We consider the following result to start the section.

Lemma 3.1. Define a selfmap $\eta$ on any subset $E$ that is closed and convex in $B$. If $\eta$ forms a generalized $(\alpha, \beta)$-nonexpansive map having nonempty fixed point $F(\eta)$, then, for the sequence of iterations in (1.10), we have $\lim\limits_{n\to\infty}\|x_{n}-p\|$, which exists by taking any point $p$ in the set $F(\eta)$.

Proof. Taking any point, namely $p\in F(\eta)$, and suppose $s\in E$, then according to Lemma 2.7, $\eta$ is quasi-nonexpansive on the set $E$, that is,

Therefore, keeping the above fact in mind, it follows from (1.10) that

From the generalized $(\alpha, \beta)$-nonexpansiveness and nonexpansiveness of $\eta$, one has

Keeping in mind (3.2) and (3.1), one concludes that

If $c_{n} = (1-\lambda_{n})h_{n}+\lambda_{n}\eta(h_{n})$, we have

Now,

Now, by using $c_{n} = (1-\lambda_{n})h_{n}+\lambda_{n}\eta(h_{n})$, we have

Now,

Now, by using (3.3) and (3.7) in (3.6), we have

It follows from (3.4), (3.5), and (3.8)

Now, by taking $d_{n} = (1-\sigma_{n})\eta(h_{n})+\sigma_{n}\eta(z_{n})$, we have

Now,

Thus,

Hence, by using (3.7), (3.12) in (3.11), we have

By (3.3), (3.9), and (3.13), we obtain

It follows from (3.10) and (3.13)

Now,

Using (3.9) in (3.16), one has

Eventually, we see that $\{\|x_{n+1}-p\|\}$ has the property that for any $p\in E$, it does not increases and is bounded. From the basic concept of analysis, we conclude that $\lim\limits_{n\to\infty}\|x_{n}-p\|$ exists. □

Lemma 3.2. Define a selfmap $\eta$ on any subset $E$ that is closed and convex in $B$. If $\eta$ forms a generalized $(\alpha, \beta)$-nonexpansive map having fixed point $F(\eta)$. Then, for the sequence of iterations in (1.10), we have $\lim\limits_{n\to\infty}\|\eta x_{n}-x_{n}\| = 0$ if and only if $F(\eta)$ is nonempty in $E$.

Proof. If $F(\eta)$ contains at-least one element, then we can assume that $p$ is a point of $F(\eta)$. It immediately follows from 3.1 that $\lim\limits_{n\to\infty}\|x_{n}-p\|$ exists, and the sequence of iterations $\{x_{n}\}$ is essentially bounded in $E$. Thus, we can assume that

From (3.9), (3.12), and (3.14), we have

It follows from (3.2) that we have

So,

Considering limit on (3.22) as follows, we have

It follows from (3.20) and (3.23), that

Regarding (3.22), (3.10), (3.11), and (3.12), one has

Considering limit on (3.25) as follows, we have

By (3.20) and (3.26), we obtain

From (3.25), we have

Considering limit on (3.27) as follows, we have

By (3.19) and (3.28), we obtain

Morover,

Hence,

By (3.17), (3.21), (3.29), and Lemma 2.11, we have

Conversly, by letting $\{x_n\}$ bounded and $\lim\limits_{n\to\infty}\|x_{n}-\eta(x_{n})\| = 0$, let $p\in A(E, \{x_n\})$, and then by Lemma 2.9, we have

This indicates that $\eta(p)\in A(E, \{x_{n}\})$. Since $B$ is uniformly convex Banach space, $A(E, \{x_{n}\})$ is a singleton. Thus, we obtain $\eta(x) = x$.

□

Theorem 3.3. Define a selfmap $\eta$ on any subset $E$ that is closed and convex in $B$. If $\eta$ forms a generalized $(\alpha, \beta)$-nonexpansive map having nonempty fixed point $F(\eta)$, then, for the sequence of iterations in (1.10), we have $\{x_{n}\}$, which is weakly convergent to a FP of $\eta$.

Proof. Consider any point $p\in F(\eta)$ so it follows from Lemma 3.1 that $\lim\limits{n\to\infty}\|x_{n} -p\|$ exists. We need to establish the fact that $\{x_{n}\}$ admits one and only one weak subsequential limit in the set $F(\eta)$. To show this, we assume that $\kappa_{1}$ and $\kappa_{2}$ form two different weak limits for the subsequences, namely $\{x_{n_{i}}\}$ and $\{x_{n_{j}}\}$ of the given sequence, respectively. It follows now from Lemma 3.2 that $\lim\limits_{n\to\infty}\|x_{n}-\eta(x_{n})\| = 0$. Similarly, by Lemma 2.10, one can conclude that $(I-\eta)$ is demiclosed on zero, that is, $(I-\eta)\kappa_{1} = 0$ and hence $\eta(\kappa_1) = \kappa_1$. In the same steps, we can show that $\eta(\kappa_2) = \kappa_2$.

Furthermore, we assume that $\kappa_1\ne\kappa_2$. Using Opial Property, we have

This contradicts our suppostion. $\kappa_1 = \kappa_{2}\implies\{x_n\}$ converges weakly to a $p\in F(\eta).$ □

Theorem 3.4. Define a selfmap $\eta$ on any subset $E$ that is closed and convex in $B$. If $\eta$ forms a generalized $(\alpha, \beta)$-nonexpansive map having a nonempty fixed point $F(\eta)$, then, for the sequence of iterations in (1.10), we have that $\{x_{n}\}$ is weakly convergent to a FP of $\eta$ $\iff \liminf\limits_{n\to\infty}d(x_n, F(\eta)) = 0$.

Proof. Notice that if $\{x_n\}$ is convergent to some FP $p$ of $\eta$, then it follows that $\liminf\limits_{n\to\infty}d(x_n, F(\eta)) = 0$, which proves the result.

On the other hand, consider that $\liminf\limits_{n\to\infty}d(x_n, F(\eta)) = 0$ and we want to prove that $\{x_{n}\}$ is convergent to a FP of $\eta$. For this purpose, we notice from Lemma 3.1 that $\lim\limits_{n\to\infty}\|x_n-p\| = 0$ exists even for each FP $p$ of $\eta$. Hence, from the given condition, it follows that $\liminf\limits_{n\to\infty}d(x_n, F(\eta)) = 0.$

It is the aim to establish that $\{x_n\}$ is a Cauchy sequence in the closed set $E$. Notice that $\liminf\limits_{n\to\infty}d(x_n, F(\eta)) = 0$, which suggests that for all $\epsilon > 0$ there must be a number $n_{o}\in \mathbb{N}$ with the property that $\forall n\geq n_{o}$ with $d(x_{n}, F(\eta)) < \dfrac{\epsilon}{2}$. If follows that

Accordingly, we conclude that inf$\{\|x_{n}-p\|:p\in F(\eta)\} < \dfrac{\epsilon}{2}.$ Hence, for any $p$ that forms a FP for $\eta$, one has $\|x_{n_{o}}-p\| < \dfrac{\epsilon}{2}.$ Thus, for any choice of $m, n\geq n_{o}$, one has

The last conclusion suggests that $\{x_{n}\}$ forms a Cauchy sequence $E$. By closeness of $E$, we can find an element, namely $\ell\in E$, with the fact that $\lim\limits_{n\to\infty}x_{n} = \ell$. However, we have $\lim\limits_{n\to\infty}d(x_{n}, F(\eta)) = 0\implies d(\ell, F(\eta)) = 0$. This proves that $\ell\in F(\eta)$, which completes the proof. □

Theorem 3.5. Define a selfmap $\eta$ on any subset $E$ that is closed and convex in $B$. If $\eta$ forms a generalized $(\alpha, \beta)$-nonexpansive map having a nonempty fixed point $F(\eta)$, then, for the sequence of iterations in (1.10), we have that $\{x_{n}\}$ is weakly convergent to a FP of $\eta$ if $E$ is compact in $B$.

Proof. In the view of Lemma 3.2, we have

Since $E$ is compact, one has a subsequence $\{x_{n{i}}\}$ of $\{x_n\}$, such that $x_{n{i}}\to p$ for some $p\in E$. Then, by Lemma 2.9, we obtained

By applying the limit, we obtained $x_{n_{i}}\to\eta(p)\ \text{as}\ i\to\infty$. This shows that $\eta(p) = p,$ which is $p\in F(\eta).$ In addition, $\lim\limits_{n\to\infty}\|x_{n}-p\|$ exists by Lemma 3.1. Thus, $\{x_n\}\to p$ as $n\to\infty$. □

The next result is established using the condition (Ⅰ).

Theorem 3.6. Define a selfmap $\eta$ on any subset $E$ that is closed and convex in $B$. If $\eta$ forms a generalized $(\alpha, \beta)$-nonexpansive map having a nonempty fixed point $F(\eta)$, then, for the sequence of iterations in (1.10), we have that $\{x_{n}\}$ is weakly convergent to a FP of $\eta$ if $\eta$ is endowed with the condition (Ⅰ).

Proof. As we already did in Lemma 3.2,

From Condition (Ⅰ) and (3.23), we got

Since, $\Upsilon:[0, 1)\to[0, 1)$ is increasing with $\Upsilon(0) = 0, \Upsilon(t) > 0\ \forall t > 0$, we have

One can now conclude from 3.4 that the given sequence is convergent to a FP of $\eta$. □

4.   Examples and compartive study

We aim to construct various numerical examples to test our scheme on the considered class of mappings. One example is simple and constructed in one-dimensional space, while the other is two-dimensional. Graphical representations and numerical comparisons clearly show the superior accuracy of our main outcome.

Example 4.1. Define $\eta:[0, \infty)\to[0, \infty)$ by

Here, $\eta$ does not posseses the Condition(C). However, $\eta$ is generalized $\alpha, \beta$-nonexpansive mapping. Let $x = \dfrac{5}{2}$ and $y = \dfrac{3}{2}$ then $\eta(x) = \dfrac{25}{12}$. So,

Moreover, $|x-y| = \left|\dfrac{5}{2}-\dfrac{3}{2}\right| = 1\ \implies\dfrac{1}{2}\left|x-\eta(x)\right|\leq|x-y|.$

However, $|\eta(x)-\eta(y)| = \left|\dfrac{24}{12}-0\right| = \dfrac{25}{12},$ $\implies$ $|\eta(x)-\eta(y)|\geq|x-y|$.

Hence, $\eta$ does not posses the Condition(C).

Now, take $\alpha = \dfrac{5}{11}$ and $\beta = \dfrac{1}{22}$. Clearly, $\alpha+\beta = \dfrac{1}{2} < 1$, which causes the following cases to arise.

Case 1: If $x, y\in[0, 2)$, then

Case 2: If $y\in[0, 2)$, and $x\in[0, \infty),$ then we have

Case 3: If $x, y\in[0, 2)$, then we have

Hence, $\eta$ is generalized $\left(\dfrac{5}{11}, \dfrac{1}{22}\right)$-nonexpansive mapping. However, for $x = \dfrac{5}{2}$, $y = \dfrac{3}{2}$, $\alpha = \dfrac{5}{11}$ and $\beta = \dfrac{1}{22}$ $\eta$ is neither generalized $\dfrac{5}{11}$-nonexpansive nor $\dfrac{1}{22}$-Reich-Suzuki type map.

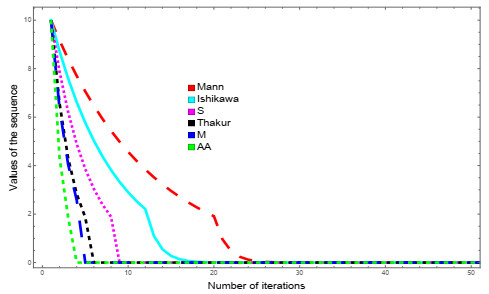
Now, we will draw graphs and tables to show that the sequence $\{x_n\}$ of the $AA$-Iterative Algorithm (1.10) moves faster to the FP of example 4.1 as compared to the Mann iteration (1.2), Ishkawa iteration (1.3), S iteration (1.5), Thakur (1.7), and M-iteration (1.8). By assuming $\alpha_{n} = 0.50, \lambda_{n} = 0.65$ and $\xi_{n} = 0.85$ and by taking the initial guess $10.0$, the observations are provided in Table 1 and Figure 1, which show that $AA$-Iterative Algorithm (1.10) is faster than mentioned above.

Now, assuming $\sigma_{n} = 0.60, \lambda_{n} = 0.43$, and $\xi_{n} = 0.67$ and by taking the initial guess $26.0$, the observations are provided in Table 2 and Figure 2.

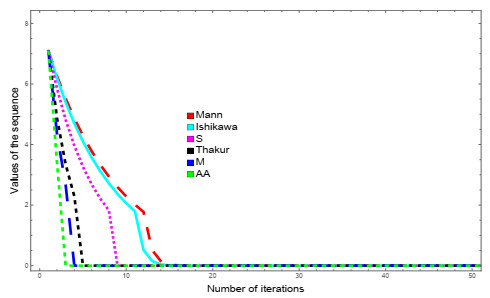
Now, assuming $\sigma_{n} = 0.89, \lambda_{n} = 0.74$, and $\xi_{n} = 0.17$ and by taking the initial guess $13.3$, the observations are provided in Table 3 and Figure 3.

Now, assuming $\sigma_{n} = 0.71, \lambda_{n} = 0.1$, and $\xi_{n} = 0.3$ and by taking the initial guess $7.1$, the observations are provided in Table 4 and Figure 4.

Now, assuming $\sigma_{n} = 0.791, \lambda_{n} = 0.545$, and $\xi_{n} = 0.023$ and by taking the initial guess $6.853$, the observations are provided in Table 5 and Figure 5.

Example 4.2. Let $E = [0, 1]$. Consider a mapping $\eta:E\times E\to E\times E$ defined by

We assume that the norm here is taxicab norm. Here, $\eta$ is generalized $(\alpha, \beta)$-nonexpansive mapping.

For $(x_{1}, y_{1})$ and $(x_{2}, y_{2})$ in $E\times E$, whenever $\dfrac{1}{2}\|(x_{1}, y_{1})-\eta(x_{1}, y_{1})\|\leq\|(x_{1}, y_{1})-(x_{2}, y_{2})\|$. For $\alpha = \dfrac{1}{2}$ and $\beta = \dfrac{1}{4}$, we have

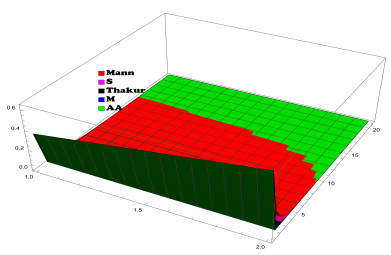
Now, we will draw graphs and tables to show that the sequence $\{x_n\}$ of the $AA$-Iterative Algorithm (1.10) moves faster to FP from example 4.2 as compared to the Mann iteration (1.2), S iteration (1.5), Thakur (1.7), and M-iteration (1.8). By assuming $\alpha_{n} = 0.34, \lambda_{n} = 0.68$, and $\xi_{n} = 0.19$ and by taking the initial guess $(0.8, 0.8)$, the observations are provided in Table 6 and Figure 6, which show that the $AA$-Iterative Algorithm (1.10) is faster than mentioned above.

Now, assuming $\sigma_{n} = 0.91, \lambda_{n} = 0.55$, and $\xi_{n} = 0.73$ and by taking the initial guess $(0.36, 0.64)$, the observations are provided in Table 7 and Figure 7.

5.   Applications

In this part, we will apply our findings to Fractional differential equations and Convex minimization problems.

5.1.   Application to fractional differential equations

Fractional differential equations (FDEs), unlike traditional integer order differential equations, involve derivatives of the non-integer order, offering a more accurate description of processes exhibiting memory and long-range dependencies. FDEs have a powerful mathematical framework for modeling complex phenomena in various scientific disciplines and is becoming an active field of interest. In recent years, it has been shown by many authors that the concept of FDEs is an appropriate way to solve nonlinear problems of mathematical modeling and engineering (see, e.g., ^[33] and others). On the one hand, the approximate and exact solutions for these FDEs are comparatively difficult to the ordinary differential. The purpose of this is work is to explore the notion of FDEs using the concept of fixed points and the class of generalized nonexpansive mappings via our AA-iterative algorithm.

Now, to achieve our main objective, we consider a very general class of FDEs of fractional order as follows:

Here, the notations $1\leq\zeta\leq2$, $0\leq\upsilon\leq1$ and eventually $\mathcal{D}^\zeta$ denotes the well-known notion of the fraction order derivative in the sense of Caputo having order $\zeta$ and $\varphi$ as an appropriate function on $[0, 1]\times\mathbb{R}$.

Assume that $\mathcal{S}$ is the set of solutions for our Problem (5.1). To establish the main result of our paper, we need to express the solution as a fixed point of suitable mapping. To do this, we need the following function known as Green's function of (5.1) as follows:

Now we want obtain the major results of this section.

Accordingly, our main result that proves the convergence of the AA-iteration approach for the given problem is the following theorem.

Theorem 5.1. Assume that the Banach space $B$ is the space $\mathcal{C}[0, 1]$, and $\eta:\mathcal{C}[0, 1]\to\mathcal{C}[0, 1]$ is a mapping that reads as follows:

If

where $\alpha, \beta\in [0, 1)$ with $\alpha+\beta\leq1$. Consequently, the $AA$-Iterative Algorithm (1.10) converges to some point of solution set "$\mathcal{S}$" of (5.1) provided that, $\liminf\limits_{n\to\infty}d(x_n, \mathcal{S}) = 0$.

Proof. $h\in C[0, 1]$ solves (5.1), if and only if it solves

The aims is to prove that the above selfmap forms a generalized mapping for some $\alpha$ and $\beta$. Hence, selecting any For $h, g\in C[0, 1]$ such that $0\leq\upsilon\leq1$, we see that

Hence, $\eta$ is generalized $(\alpha, \beta)$-nonexpansive mapping. By Theorem 3.4, the sequence obtained by the $AA$-iterative algorithm (1.10) converges to the FP of $\eta$ and to the solution of a given equation. □

5.2.   Application to 2D Volterra integral equations

Now, we will solve 2D Volterra integral equations in the setting of generalized ($\alpha, \beta$)-nonexpansive mapping. Instead of other iterative algorithms, we use the $AA$-Iterative Algorithm to approximate the solution of following the 2D Volterra integral equation:

for all $r, \xi\lambda, v\in[0, 1]$, where $h\in\mathcal{M}\times\mathcal{M}$, $\kappa:[0, 1]\times[0, 1]\to\mathbb{R}^{2}$, $\Lambda_{i}(i = 1, 2, 3):[0, 1]\times[0, 1]\times\mathbb{R}^{2}, \delta, \gamma\geq 0$ and $\mathcal{M} = \mathcal{C}[0, 1]$ is Banach space with the maximum norm

We are now in a position to present a new application of the algorithm we have studied. This result is obtained under some mild conditions, which are as follows:

Theorem 5.2. Consider $\Omega$ as closed convex subset of $\mathcal{M}$ such that $\eta:\Omega\to\Omega$ is a map with

Assume the following assertions below are true

($\mathcal{A}_{1}$) the function $h:\mathcal{M}\times\mathcal{M}\to\mathbb{R}^{2}$ is continuous;

($\mathcal{A}_{2}$) the function $\Lambda_{i}(i = 1, 2, 3):[0, 1]\times [0, 1]\times\mathbb{R}^{2}\to\mathbb{R}^{2}$ is continuous and there are the constants $\ell_{1}, \ell_{2}, \ell_{3} > 0$, such that for all $\tau_{1}, \tau_{2}\in\mathbb{R}^{2}$

($\mathcal{A}_{3}$) for $\delta, \gamma\geq 0, \ \ell_{1}+\delta\ell_{2}+\gamma\ell_{3}\leq\wp$, where $\wp\in(0, 1)$.

Consequently, the $AA$-Iterative Algorithm (1.10) converges to some point of solution set "$\mathcal{S}$" of (5.2) provided that, $\liminf\limits_{n\to\infty}d(x_n, \mathcal{S}) = 0$.

Proof. Let $h, g\in\mathcal{M}\times\mathcal{M}$, then

which implies that

Hence, by Lemma 2.9, $\eta$ is generalized $(\alpha, \beta)$-nonexpansive mapping becuase it satisfies $\|x-\eta(y)\| \leq \left(\frac{3+\alpha+\beta}{1-\alpha-\beta} \right)\|x-\eta(x)\|+\|x-y\|$ for $\left(\frac{3+\alpha+\beta}{1-\alpha-\beta} \right) = 1$. As all conditions for Lemma 3.2 are satisfied, the AA-iteration converges to the solution. □

5.3.   Application to the convex minimization problem

In this section, we are concerned with finding a solution to the convex minimization problem using the $AA$-Iterative algorithm (1.10). Assume $g:\mathcal{C}\to \mathbb{R}$, where $\mathcal{C}$ is closed and a convex subset of a real Hilbert space $\mathcal{H}$, and $g$ is a convex mapping. Consider the convex minimization problem

Assmue that $P_\mathcal{C}:\mathcal{H}\to\mathcal{C}$ is a projection map and $g$ is a Fréchet differentiable. Consider that $\nabla g$ represents a gradient of $g$. It is obvious that $\dot{y}\in\mathcal{C}$ solves (5.3) if it solves the variational inequality:

that is, $\dot{y}\in\Omega(\mathcal{C}, \mathcal{A})$. Here, $\Omega(\mathcal{C}, \mathcal{A}) = \{y\in\mathcal{C}:\langle\mathcal{A}y, y-x\rangle\geq0\forall x\in\mathcal{C}\}$ and $\mathcal{A}:\mathcal{H}\to\mathcal{H}$ is a nonlinear operator. Adding more $\dot{y}$ solves (5.3) if $\dot{y} = P_{\mathcal{C}}(\dot{y}-\gamma\nabla g(x_{n})),$ where $x_{1}\in\mathcal{C}$ and $0 < \gamma < \dfrac{2}{L^2}$. To solve (5.3), the gradient project algorithm is used and is defend by

where $x_{0}\in\mathcal{C}$ and $\gamma$ is a step size.

Lemma 5.3. Let $\eta$ be a generalized $(\alpha, \beta)$-nonexpanisve mapping, and if $\dot{y}\in F(\eta)\cap\Omega(\mathcal{C}, \mathcal{A})$, then $\eta = P_{\mathcal{C}}(I-\gamma\nabla g)\ \mathrm{for\ identinty\ mapping}\ I.$

Proof. Since $\dot{y}\in F(\eta)\cap\Omega(\mathcal{C}, \mathcal{A})$, we have $\dot{y}\in F(\eta)$ and $\dot{y}\in\Omega(\mathcal{C}, \mathcal{A})$. This implies that

and

It follows form (5.5) and (5.6)

Hence, $\eta = P_{\mathcal{C}}(I-\gamma\nabla g)\ \mathrm{for\ identinty\ mapping}\ I$. □

For an arbitrary $\{x_{1}\}$ in $\mathcal{C}$ and for three sequences of real numbers $\{\sigma_{n}\}$, $\{\lambda_{n}\}$, and $\{\xi_{n}\}$ in $(0, 1)$, then the sequence $\{x_{n}\}$ obtained by the following algorithm converges to the solution of a convex minimization problem (5.3);

Theorem 5.4. Suppose that the convex minimization problem (5.3) has a solution, then the sequence obtained by algorithm (5.7) converges weakly to the solution of (5.3).

Proof. By Lemma 5.3 $\eta = P_{\mathcal{C}}(I-\gamma\nabla g)\ \mathrm{for\ identinty\ mapping}\ I$, then the conclusion follows from Theorem 3.3. □

Theorem 5.5. Suppose that the convex minimization promlem (5.3) has a solution. Then, the sequence obtained by algorithm (5.7) converges strongly to the solution of (5.3) if $\liminf\limits_{n\to\infty}d(x_n, \Omega)) = 0$, where $\lim\limits_{n\to\infty}d(x_n, \Omega) = \mathit{\text{inf}}\{\|x_{n}-p\|:p\in \Omega\}.$

Proof. The proof follows from Theorem 3.4. □

6.   Conclusions and future plan

In this study, we used an $AA$-iterative algorithm to approximate the FP of generalized $(\alpha, \beta)$-nonexpansive mappings. We proved weak convergence and strong convergence results for mappings in uniformly convex Banach spaces for generalized $(\alpha, \beta)$-nonexpansive. We showed that the $AA$-iterative algorithm for generalized $(\alpha, \beta)$-nonexpansive mappings converged more quickly than other existing algorithms, as demonstrated by a numerical example. We proved in the setting of generalized $(\alpha, \beta)$-nonexpansive mappings that the iterative scheme $AA$ can be used to solve fractional differential equations, the 2D voltera differential equation, and a convex minimization problem.

In the future, we will utilize the AA-iterative algorithm and the results presented in this paper to find optimal solutions for machine learning problems. We also aim to extend our study to the setting of multi-valued mappings. Since we used Hilbert and Banach spaces, which are linear spaces, we will also try to extend the study to the setting of nonlinear CAT(0) and hyperbolic spaces.

Author contributions

Hamza Bashir: Writing–original draft; Junaid Ahmad: Investigation; Walid Emam: Software; Muhammad Arshad: Supervision; Zhenhua Ma: Writing–review & editing. All authors agree to publish this version.

Use of AI tools declaration

The authors declare they have not used Artificial Intelligence (AI) tools in the creation of this article.

Funding

The study was funded by Researchers Supporting Project number (RSPD2024R749), King Saud University, Riyadh, Saudi Arabia.

Acknowledgments

The study was funded by Researchers Supporting Project number (RSPD2024R749), King Saud University, Riyadh, Saudi Arabia. The work in this paper is also supported by HEC, Pakistan under the NRPU research project No 15548 entitled "Fixed Point Based Machine Learning Optimization Algorithms and RealWorld Applications".

Conflict of interest

No competing interests are disclosed by the writers.