A novel algorithm with an inertial technique for fixed points of nonexpansive mappings and zeros of accretive operators in Banach spaces

1.   Introduction

Let $\mathcal{C}$ be a nonempty, closed, and convex subset of a real Banach space $\mathcal{B}$ with dual $\mathcal{B}^*$. Let $J:\mathcal{B}\rightarrow2^{{\mathcal{B}}^{*}}$ denote the normalized duality mapping given by

where $\langle \cdot, \cdot \rangle$ denotes the generalized duality pairing (see, for example, ^[1]). $J$ is single valued if $\mathcal{B}^*$ is strictly convex. In what follows, we denote single valued normalized duality mapping by $J$. A Banach space $\mathcal{B}$ is said to be uniformly convex ^[2,3] if, for any sequences $\left\{v_{m}\right\}$ and $\left\{\wp_{m}\right\}$, in $\mathcal{B}$ with $\left\|v_{m}\right\| = \left\|\wp_{m}\right\| = 1$ and $\lim\limits_{m\rightarrow \infty}\left\|v_{m} + \wp_{m}\right\| = 2$ imply $\lim\limits_{m\rightarrow \infty}\left\|v_{m} - \wp_{m}\right\| = 0.$ The modulus of smoothness $\rho_\mathcal{B}(\cdot)$ of $\mathcal{B}$ is the function $\rho_\mathcal{B} : \left[0, +\infty\right) \rightarrow \left[0, +\infty\right)$ defined by

It is well known that $\mathcal{B}$ is uniformly smooth if, and only if, $\frac{\rho_\mathcal{B}(\tau)}{\tau} \rightarrow 0,$ as $\tau \rightarrow 0.$ Let $q > 1$ be a real number. A Banach space $\mathcal{B}$ is said to be $q$-uniformly smooth if there exists a positive constant $K_q$ such that $\rho_\mathcal{B}(\tau) \leq K_{q}\tau^{q}$ for any $\tau > 0$. It is obvious that a $q$-uniformly smooth Banach space must be uniformly smooth. A mapping $\mathcal{T} : \mathcal{C} \rightarrow \mathcal{C}$ is said to be $L-Lipschitzian$ if there exits $L\ \geq 0$ such that

$\mathcal{T}$ is said to be a contraction if $L\in[0, 1)$ and $\mathcal{T}$ is said to be nonexpansive if $L = 1$ (see ^{[1,2,3,4,5,6]}).

In this paper, we are interested in formulating a numerical method for solving the fixed point problem

where $\mathcal{T} : \mathcal{C} \rightarrow \mathcal{C}$ is a nonexpansive mapping. We consider $\mathcal{F}(\mathcal{T})\neq \emptyset$ and designate $\mathcal{F}(\mathcal{T})$ by the set of all fixed points of $\mathcal{T}$, which is $\mathcal{F}(\mathcal{T}) \ : = \ \{ v \in \mathcal{C} \ | \ v = \mathcal{T}(v) \}.$

The most naive approach when looking for a fixed point of a contraction mapping $\mathcal{T} : \hbar \rightarrow \hbar$ defined on a complete metric space $(\hbar, \mathcal{L})$ has a unique fixed point, where $\mathcal{L}$ is the distance that describes the mapping $\mathcal{T}$ as the following process, also called Banach-Picard iteration,

where $v_0 \in \hbar$ is a starting point.

According to the Banach-Picard fixed point theorem, if $\mathcal{T}$ is a contraction, namely, $\mathcal{T}$ is Lipschitz continuous with modulus $\delta \in [0, 1)$, then the sequence $\{v_m\}_{m \geq 0}$ generated by (1.2) converges strongly to the unique fixed point of $\mathcal{T}$ with linear convergence rate.

If $\mathcal{T}$ is just nonexpansive, then this statement is no longer true. To illustrate this, it is enough to choose $\mathcal{T} = - Id$, where $Id$ denotes the identity mapping, and $v_0 \neq 0$, in which case the Banach-Picard iteration fails an approach to a fixed point of $\mathcal{T}$.

In order to overcome the restrictive contraction assumption on $\mathcal{T}$, Krasnoselskii proposed in ^[7] to apply the Banach-Picard iteration (1.2) to the operator $\frac{1}{2}Id+\frac{1}{2}\mathcal{T}$ instead of $\mathcal{T}$. The Krasnoselskii-Mann iteration is written as follows:

where $\{\eta_m\}$ is a sequence in $(0, 1)$. This iteration is often said to be a segmenting Mann iteration (see ^[8,9,10]) or to be of the Krasnoselskii-type (see e.g., ^{[11,12,13,14,15,16,17]}). It was found that the sequence $\left\{v_m\right\}$ created by (1.3) weakly converges to a fixed point of $\mathcal{T}$ under the conditions of $\mathcal{F}(\mathcal{T})\neq \emptyset$ and mild assumptions imposed on $\left\{\eta_m\right\}.$

It turned out that a fundamental step in proving the convergence of the iterates of (1.3) is to show that $v_m - \mathcal{T}(v_m) \rightarrow 0$ as $m \rightarrow +\infty$, as it was done by Browder and Petryshyn in ^[18] in the constant case $\eta_m\equiv \eta \in (0, 1).$ The weak convergence of the iterates was then studied in various settings in ^{[9,19,20,21,22]}.

It should be noted that, even in real Hilbert spaces, all previous modifications to the Krasnoselskii-Mann method for nonexpansive mappings only provide weak convergence; for further information, see ^[23].

Bot et al. ^[24] recently presented a new form for Mann's method to address the previously mentioned issues. Let $v_0$ be arbitrary in a real Hilbert space $\mathcal{H}$, $\forall m\geq 0$,

They proved that the iterative sequence $\{v_m\}$ produced by (1.4) is strongly convergent using appropriate $\{\eta_m\}$ and $\{\zeta_m\}$ assumptions. Sequence $\{\zeta_m\}$, also known as the Tikhonov regularization sequence, plays a significant role in acceleration (1.4). Dong et al. ^[25], Fan et al. ^[26], and Polyak ^[27] have cited several theoretical and numerical conversations to examine strong convergence utilizing the Tikhonov regularization algorithm.

Recent years have seen the development and introduction of additional algorithms, such as the inertial algorithm initially presented by Polyak ^[27]. He minimized a smooth convex function by use of inertial extrapolation. It is important to note that these simple adjustments improved the efficiency and efficacy of these algorithms. Researchers have been able to study several vital applications after adopting this concept. For example, see ^{[25,26,28,29,30,31,32,33,34,35,36]}.

An operator $\Upsilon : D(\Upsilon) \subseteq \mathcal{B} \rightarrow R(\Upsilon) \subseteq \mathcal{B}$ is called accretive (see ^[1]) if for all $t > 0$ and for all $v, \wp \in D(\Upsilon)$, where $D(\Upsilon)$ denotes the domain of $\Upsilon$. We have

Furthermore, $\Upsilon$ is accretive if, and only if, for each $v, \wp \in D(\Upsilon)$, there exists $j(v-\wp) \in J(v-\wp)$ such that

An accretive operetor $\Upsilon$ is said to be $m$-accretive (see, for example, ^[1]) if $R(I+e \Upsilon) = \mathcal{B}$ for all $e > 0$, where $R(I+e \Upsilon)$ is the range of $(I+e\Upsilon)$. $\Upsilon$ is said to satisfy the range condition if $\overline{D(\Upsilon)} \subseteq R(I+e\Upsilon)$ for all $e > 0$, where $\overline{D(\Upsilon)}$ is the closure of the domain of $\Upsilon$. Moreover, if $\Upsilon$ is accretive ^[37], then $J_{\Upsilon} : R(I+ \Upsilon) \rightarrow D(\Upsilon)$, which, defined by $J_{\Upsilon} = {(I+\Upsilon)}^{-1}$, is a single-valued nonexpansive and $\mathcal{F}(J_{\Upsilon}) = N(\Upsilon)$, where $N(\Upsilon) = \left\{v \in D(\Upsilon) : 0 \in\Upsilon v \right\}$ and $\mathcal{F}(J_{\Upsilon}) = \left\{ v\in \mathcal{B} : J_{\Upsilon}v = v \right\}$.

Browder ^[38] and Kato ^[39] independently introduced the accretive operators. Due to their close relation to the existence theory for nonlinear equations of evolving in Banach spaces, the study of such mappings is very fascinating.

Under suitable Banach spaces, accretive operators play a crucial role in many physically relevant situations that may be characterized as initial boundary value problems as follows:

Many embedded models of evolution equations exist, including the Schrodinger, heat, and wave equations ^[40]. According to Browder ^[38], (1.5) has a solution if $\Upsilon$ is locally Lipschitzian and accretive on $\mathcal{B}$. He also proved that $\Upsilon$ is $m$-accretive and there is a solution to the equation below

Ray ^[40] uses the fixed point theory of Caristi ^[41] to elegantly and precisely improve Browder's conclusions. Robert and Martin ^[42] show that the problem (1.5) is solved in the space $\mathcal{B}$ if $\Upsilon$ is continuous and accretive. Utilizing this result, Martin ^[43] proved that if $\Upsilon$ is continuous and accretive, then $\Upsilon$ is $m$-accretive.

See Browder ^[44] and Deimling ^[45] for further information on the theorems for zeros of accretive operators.

One should note that, if $\mu$ is independent of $\tau$ in (1.5), then $\frac{d\mu}{d\tau} = 0$. Because of this, (1.5) simplifies to (1.6), whose solution illustrates the problem's stable or equilibrium state. This in turn is tremendously fascinating in a variety of beautiful applications, including, but not limited to, economics, physics, and ecology. Significant efforts have been undertaken to solve (1.6) when $\Upsilon$ is accretive. Researchers were interested in investigating the fixed point and approximate iterative approaches for zeros of $m$-accretive mappings since $\Upsilon$, in general, is nonlinear and there is no known process to discover a close solution to this equation. As a result, research in the field has flourished up to the present. Some of the related work can be found in ^[46,47] and the references therein.

Based on the previous research, the sequence was created iteratively by a novel algorithm with an inertial technique, and a strong convergence using the proposed algorithm is also discussed in a real uniformly convex Banach space with a Gâteaux differentiable norm. In addition, we find zeros of accretive mappings. Moreover, a numerical example is presented to illustrate the behavior of our algorithm.

2.   Preliminaries

In this section, we summarize notations and lemmas that play a significant role in the convergence analysis of our algorithm.

A real normed linear space $\mathcal{B}$ is said to have a Gâteaux differentiable norm if the limit

exists for all $v, \wp\in\aleph$, where $\aleph$ denotes the unit sphere of $\mathcal{B}$ (i.e., $\aleph = \left\{v \in{\mathcal{B}} : \|v\| = 1 \right\}$). In this case, $\mathcal{B}$ is called smooth. It is also said to be uniformly smooth if the limit is attained uniformly for $v, \wp\in\aleph$, and $\mathcal{B}$ is said to have a uniformly Gâteaux differentiable norm.

If $\mathcal{B}$ is smooth, it is clear that every duality mapping on $\mathcal{B}$ is a single-valued mapping. If $\mathcal{B}$ has a uniformly Gâteaux differentiable norm, then the duality mapping is norm-to-weak* uniformly continuous on bounded subsets of $\mathcal{B}$.

Let ${\Delta}$ be a nonempty, closed, convex, and bounded subset of a real Banach space $\mathcal{B}$ and the diameter of ${\Delta}$ defined by $d\left(\Delta\right) = \sup\left\{\|v-\wp\|, v, \wp\in\Delta\!\right\}.$ The Chebyshev radius of ${\Delta}$ is given by $w\left(\Delta\right) = \inf\left\{w\left(v, \wp\right), v\in\Delta\right\},$ where $v\in\Delta$,

Bynum ^[48] proposed the normal structural coefficient $N(\mathcal{B})$ of $\mathcal{B}$ as follows:

If $N(\mathcal{B}) > 1$, then $\mathcal{B}$ has a uniform normal structure.

Every space with a uniform normal structure is reflexive, which means that all uniformly convex and uniformly smooth Banach spaces have a uniform normal structure. See ^[1,49] for more details.

In the sequel, the following lemmas are needed to prove our main results.

Lemma 1. ^[50] Suppose that $\mathcal{B}$ is a real uniformly convex Banach space. For arbitrary $u > 0$, ${\aleph_u\left(0\right)}$ = $\left\{v\in \mathcal{B} : \|v\| \leq u \right\}$ and $\alpha\in\left[0, 1\right]$, then there is a continuous strictly increasing convex function $r:\left[0, 2u\right]\rightarrow \mathbb{R}, \ r\left(0\right) = 0$ such that

Lemma 2. ^[45] Suppose that $\mathcal{B}$ is a real normed linear space, then for any $v, \wp\in\mathcal{B}, \ j(v+\wp)\in J(v+\wp)$, we have that the following inequality holds

Lemma 3. ^[5] Let $\mathcal{B}$ be a uniformly convex Banach space and ${\Delta}$ a nonempty, closed, and convex subset of ${\mathcal{B}}.$ Suppose that ${\mathcal{T}:\Delta\rightarrow \Delta}$ is a nonexpansive mapping with fixed points. Let $\left\{v_m\right\}$ be a sequence in ${\Delta}$ such that $v_m\rightharpoonup v$ and $v_m-\mathcal{T}v_m\longrightarrow\wp$, then $v-\mathcal{T}v = \wp$.

Lemma 4. ^[49] Let $\mathcal{B}$ be a Banach space with uniform normal structure and ${\Delta}$ a nonempty, bounded subset of $\mathcal{B}$. Suppose that $\mathcal{T}:\Delta\longrightarrow\Delta$ is a uniformly L-Lipschitzian mapping with $L < N\left(\mathcal{B}\right)^{\frac{1}{2}}$. If there is a nonempty, bounded, closed, convex subset ${\mathfrak{R}}$ of ${\Delta}$ with the property (D), that is,

then $\mathcal{T}$ has a fixed point in ${\Delta}$.

Note that $\varpi_w(v) = \{\wp\in\mathcal{B}:y = weak\ \varpi-lim\mathcal{T}^{n_j}v, \exists\ n_j\rightarrow \infty\}$; here is the ${\varpi}$-limit set of $\mathcal{T}$ at $v$.

Lemma 5. ^[51] Suppose that $(v_0, \ v_1, v_2, ...)\in l_\infty$, is so that $\delta_m v_m\le0$ for all Banach limits $\delta$. If $\limsup\limits_{m\rightarrow \infty}\left(v_{m+1}-v_m\right)\le0$, then $\limsup\limits_{m\rightarrow \infty}v_m\le0.$

Lemma 6. ^[52] Let $\left\{{e_m}\right\}$ be a sequence of nonnegative real numbers such that

If

(i) $\left\{c_m\right\} \subset[0, 1], \sum{c_m} = \infty$, $\limsup\limits_{m\rightarrow \infty}$ $\sigma_m\le\ 0$,

( ii ) for each $m \geq 0$, $\pi_m \geq 0, \sum {\pi_m} < \infty,$ then $\lim\limits_{m\rightarrow \infty}e_m = 0.$

3.   Main results

We now prove the following strong convergence results.

Theorem 1. Let $\mathcal{C}$ be a nonempty, closed, convex subset of a real uniformly convex Banach space $\mathcal{B}$, which has a uniformly Gâteaux differentiable norm, and $\mathcal{T} : \mathcal{C} \rightarrow \mathcal{C}$ is a nonexpansive mapping such that $\mathcal{F}(\mathcal{T}) \neq \emptyset.$ Consider that the following assumptions hold:

(i) $\lim\limits_{m\rightarrow \infty}\xi_m = 0,$ $\lim\limits_{m\rightarrow \infty}\sigma_m = 0,$ $\sum\limits_{m = 1}^{\infty}\sigma_m = \infty,$ $\xi_m, \sigma_m\in{(0, 1)}, \rho_m\in[l_1, l_2]\subset{(0, 1)},$

(ii) $\pi_m \geq 0,$ $\forall m\in \mathbb{N}$ and $\sum\limits_{m = 1}^{\infty} \pi_m < \infty$.

For arbitrary $\nu_0, \nu_1 \in \mathcal{C}$, let $\{{v_m}\}$ be the sequence generated by

then $\left\{v_m\right\}$ converges strongly to a point in $\mathcal{F}(\mathcal{T})$.

Proof. Let $d \in\mathcal{F}(\mathcal{T})$. Set $\wp_m = \left(1-\sigma_m\right)\hbar_m$. Using (3.1), we have

By mathematical induction, one can obtain

This shows that $\left\{v_m\right\}$ is bounded, so $\left\{\hbar_m\right\}$, $\left\{\wp_m\right\}$, and $\left\{\psi_m\right\}$ are also bounded. By condition $(ii),$ this implies $\sum\limits_{m = 1}^{\infty} \pi_m\|\nu_m-\nu_{m-1}\| < \infty.$ Using Lemmas 1 and 2 and (3.1), we have

On the other hand, one can write

The boundedness of $\left\{v_m\right\}$, $\left\{\hbar_m\right\}$, $\left\{\wp_m\right\}$, and $\left\{\psi_m\right\}$ leads to constants $\Lambda_1, \Lambda_2, \Lambda_3 > 0$ so that for all $m\geq 1$,

Applying (3.3) in (3.2), we have

This implies that $\left\{v_m\right\}$ converges to $d.$ We consider the following cases in order to achieve strong convergence:

Case (a). If the sequence $\left\{ \|v_m -d \| \right\}$ is monotonically decreasing, then $\left\{ \|v_m -d \| \right\}$ is convergent. We see that

as $m \rightarrow \infty$. By (3.4), we have

Using the property of $r$ and $\rho_m \in \big[{l_1, l_2}\big]\subset (0, 1),$ we have

Combining (3.1) and (3.5), we find that

Using (3.1) and condition ($i$), we have

From (3.1) and condition ($i$), we get

It follows from (3.7) and (3.8) that

From $\sum\limits_{m = 1}^{\infty} \pi_m\|\nu_m-\nu_{m-1}\| < \infty$, we get

Based on (3.9) and (3.10), we can write

Using (3.6) and (3.11), we have

Using (3.5), (3.9), and (3.10), we have

Since $\left\{ v_m \right\}$ is bounded, there exists a subsequence $\left\{ v_{m_{b}} \right\} \subset \left\{ v_m \right\}$ such that it converges weakly to $d \in \mathcal{B}.$ In addition, using Lemma 3, we have $d \in \mathcal{F}(\mathcal{T}).$ Now, we prove that

Suppose that $\chi : \mathcal{B} \rightarrow \mathbb{R}$ is given by

then $\chi(v) \rightarrow \infty$ as $\|v\|\rightarrow \infty$ and $\chi$ is convex and continuous. Since $\mathcal{B}$ is reflexive, then there exists $\wp^* \in \mathcal{B}$ such that $\chi(\wp^*) = \min\limits_{a\in \mathcal{B}} \chi(a)$. Hence, the set $\hat{\mathfrak{R}}$ $\neq\emptyset$, where

It follows from $\lim\limits_{m\rightarrow \infty} \|\mathcal{T}\psi_m -\psi_m \| = 0$ and $\lim\limits_{m\rightarrow \infty} \|\psi_m -\wp_m \| = 0$ that

Since $\lim\limits_{m\rightarrow \infty}\|\mathcal{T}\wp_m -\wp_m \| = 0,$ it follows from induction that $\lim\limits_{m\rightarrow \infty} \|\mathcal{T}^n \wp_m -\wp_m \| = 0$ for all ${n \geq 1}$. Thus, using Lemma 4, if $v \in \mathfrak{R}$ and $\wp = \varpi- \lim\limits_{j \rightarrow \infty}\mathcal{T}^{n_{j}} v$, then from weak lower semicontinuity of $\chi$ and $\lim\limits_{m\rightarrow \infty} \|\mathcal{T}\wp_m -\wp_m \| = 0,$ we get

Hence, $\wp^* \in \hat{\mathfrak{R}}$. It follows from Lemma 4 that $\mathcal{T}$ has a fixed point in $\hat{\mathfrak{R}}$, so $\hat{\mathfrak{R}} \cap \mathcal{F}(\mathcal{T})\neq \emptyset$. Without losing the general case, as a particular instance, suppose that $\wp^* = d \in \hat{\mathfrak{R}}\cap \mathcal{F}(\mathcal{T}).$ Consider $\tau \in (0, 1)$, then it is easy to see that $\chi(d) \leq \chi(d- \tau d)$. With the help of Lemma 2, we have

By the properties of $\chi$, we can write

By arranging the above inequality, we have

This leads to

In addition,

Since the normalized duality mapping is norm-to-weak* uniformly continuous on bounded subsets of $\mathcal{B},$ we have, as $\tau\rightarrow 0$ and for fixed $n$,

Thus, for each $\epsilon > 0,$ there is $\varsigma_\epsilon > 0$ such that for all $\tau\in (0, \varsigma_\epsilon),$

Thus,

Since $\epsilon$ is an arbitrary, using (3.8), we obtain

By the triangle inequality, we have

Using (3.4)–(3.6) and (3.8), we have

Again, since the normalized duality mapping is norm-to-weak* uniformly continuous on bounded subsets of $\mathcal{B}$, we have

Using Lemma 5, we have

From (3.1), we obtain

Thus,

Since

using (3.1), (3.13), Lemma 2, and $\sum\limits_{m = 1}^{\infty} \pi_m\|\nu_m-\nu_{m-1}\| < \infty$, we have

Applying Lemma 6, we conclude that $\left\{v_m\right\}$ converges to $d.$

Case (b). Suppose the sequence $\{\|v_m-d\|\}$ is not monotonically decreasing. Let $\Xi_m = {\|v_m-d\|}^{2}$. Suppose that $\Pi : \mathbb{N} \rightarrow \mathbb{N}$, defined by

Obviously, $\Pi$ is a nonincreasing sequence so that $\lim\limits_{m\rightarrow \infty}\Pi(m) = \infty$ and $\Xi_{\Pi(m)} \leq \Xi_{\Pi(m)+1}$ for $m \geq m_0$ (for some $m_0$ large enough). Using (3.4), we have

In addition, we get

Using the same circumstances as in Case (a), we can show that $v_{\Pi(m)} \rightharpoonup d$ as $\Pi(m) \rightarrow \infty$ and $\limsup\limits_{\Pi(m)\rightarrow \infty} \langle -d, j (\wp_{\Pi(m)}-d)\rangle \leq 0.$ For all $m \geq m_0$, we obtain by (3.14) that

This implies that

Since $\limsup\limits_{\Pi(m)\rightarrow \infty} \langle -d, j (\wp_{\Pi(m)}-d)\rangle \leq 0,$ taking the limit as $m \rightarrow \infty$ in the above inequality, we have

Thus,

Moreover, for all $m \geq m_0$, it is easy to notice that $\Xi_m \leq \Xi_{\Pi(m)+1}$ if $m \neq \Pi(m)$, that is, $\Pi(m) < m,$ since $\Xi_i > \Xi_{i+1}$ for $\Pi(m) + 1 \leq i \leq m.$ As a result, for all $m \geq m_0,$ we get

Hence, $\lim\limits_{m\rightarrow \infty}\Xi_m = 0,$ which concludes that $\left\{v_m\right\}$ converges strongly to a point $d$. This finishes the proof. □

Since every uniformly convex Banach space has a uniformly Gâteaux differentiable norm, our theorem can be stated in a uniformly convex Banach space, which is also uniformly smooth. Therefore, we can also obtain the following result without proof.

Corollary 1. Let $\mathcal{C}$ be a nonempty, closed, convex subset of a real uniformly convex Banach space $\mathcal{B}$, which is also uniformly smooth, and $\mathcal{T} : \mathcal{C} \rightarrow \mathcal{C}$ is a nonexpansive mapping such that $\mathcal{F}(\mathcal{T}) \neq \emptyset.$ Let $\left\{v_m\right\}$ be a sequence generated iteratively by (3.1), then $\left\{v_m\right\}$ converges strongly to a point in $\mathcal{F}(\mathcal{T})$.

In the remainder of this section, we prove the following theorem for finding zeros of accretive mappings.

Theorem 2. Let $\mathcal{C}$ be a nonempty, closed, convex subset of a real uniformly convex Banach space $\mathcal{B}$, which has a uniformly Gâteaux differentiable norm, and $\Upsilon : \mathcal{C} \rightarrow \mathcal{C}$ is a continuous and accretive mapping such that $N(\Upsilon) \neq\emptyset$. For arbitrary $v_0, v_1\in\mho$, let $\left\{v_m \right\}$ be the sequence generated by

where $J_{\Upsilon} = {(I+\Upsilon)^{-1}}$. Consider that the following assumptions hold:

(i) $\lim\limits_{m \rightarrow \infty}\xi_m = 0, \lim\limits_{m\rightarrow \infty} \sigma_m = 0, \sum\limits_{m = 1}^{\infty}\sigma_m = \infty, \xi_m, \sigma_m\in{(0, 1)}, \rho_m\in[l_1, l_2]\subset{(0, 1)}$,

(ii) $\pi_m \geq 0,$ $\forall m\in \mathbb{N}$ and $\sum\limits_{m = 1}^{\infty} \pi_m < \infty$,

then $\left\{v_m \right\}$ converges strongly to a point in $N(\Upsilon)$.

Proof. According to the results of Martin ^[42,43,44] and Cioranescu ^[37], $\Upsilon$ is $m$-accretive. This implies that $J_{\Upsilon} = {(I+\Upsilon)}^{-1}$ is nonexpansive and $\mathcal{F}(J_{\Upsilon}) = N(\Upsilon).$ Setting $J_{\Upsilon} = \mathcal{T}$ in Theorem 1 and using the same approach going forward, we obtain the desired result. □

4.   Numerical examples

Using the following experiment, we examine the algorithm's behavior (${3.1}$) for approximating the fixed point. We show the convergence results discussed in this study graphically and with a table of numerical values.

Example 1. Consider that a fixed point problem taken from ^[53] in which $\mathcal{B} = \mathbb{R}$ through the usual real number space $\mathbb{R}$ with the usual norm. A mapping $\mathcal{T}:\mathcal{B} \rightarrow \mathcal{B}$ is defined by

where $A = \left\{v: 0 \leq v \leq 50 \right\}$.

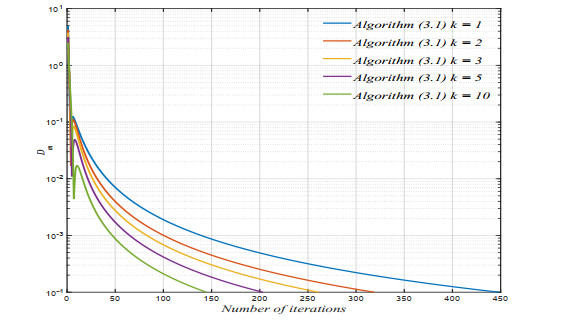
Experiment 1. For the control parameter $\xi_m = \sigma_m = \frac{1}{(km+2)}$ in this experiment, we used several values for $k = 1, 2, 3, 5, 10.$ Consider $\rho_m = 0.80, v_0 = v_1 = 10,$ $\pi_m = \frac{10}{(m+1)^2}$, and $D_m = \|v_{m}-v_{m-1}\|$ (see on Table 1 and Figure 1).

Experiment 2. We used several values for $k = 0.15, 0.35, 0.55, 0.75, 0.95$ for the control parameter $\rho_m = k.$ Also, consider $\xi_m = \sigma_m = \frac{1}{(2m+2)}, v_0 = v_1 = 10,$ $\pi_m = \frac{10}{(m+1)^2}$, and $D_m = \|v_{m}-v_{m-1}\|$ (see on Table 2 and Figure 2).

Symmetry considerations can be related to signal processing, especially when signals satisfy certain symmetries. Now, we focus on applying Algorithm (3.1) to signal recovery problems. In signal processing, compressed sensing can be modeled as the following under the determined linear equation system:

where $v \in \mathbb{R}^{n}$ is the original signal with $n$ components to be recovered, $\nu, y \in \mathbb{R}^{m}$ are noise and the observed signal with noise for $m$ components, respectively, and $A \in \mathbb{R}^{m \times n}$ is a degraded matrix. Finding the solutions of the previous underdetermined linear equation system can be viewed as solving the least absolute shrinkage and selection operator problem (LASSO problem):

where $\lambda > 0$. Various techniques and iterative schemes have been developed to solve the LASSO problem. Our method for solving the LASSO problem can be applied by setting $\mathcal{T}v = \text{prox}_{\mu g}(v - \mu \nabla f(v))$, where $f(v) = \|y - Av\|_2^2/2$, $g(v) = \lambda\|v\|_1$, and $\nabla f(v) = A^T(Av - y)$.

Next, we provide an example of applying our algorithm to signal recovery problems.

Example 2. Let $A \in \mathbb{R}^{m \times n} (m < n)$ be a degraded matrix and $y, \mathbf{\nu} \in \mathbb{R}^{m}$. We propose the following method to find the solution of the signal recovery problem:

where $\mathcal{T}v = \text{prox}_{\mu g}\left(v - \mu A^T \left(Av - y \right) \right)$, $\mu = 1.8/{\|A^T A\|}_2$. Moreover, we randomly choose vectors $v_0$ and $v_1$ and apply them to the proposed method, where $\sigma_m = \xi_m = \tfrac{1}{10(m+1)}$, $\rho_m = \frac{m}{m+1}$, and $\pi_m = \frac{1}{(m+100)^2}$ for each $m \in \mathbb{N}$.

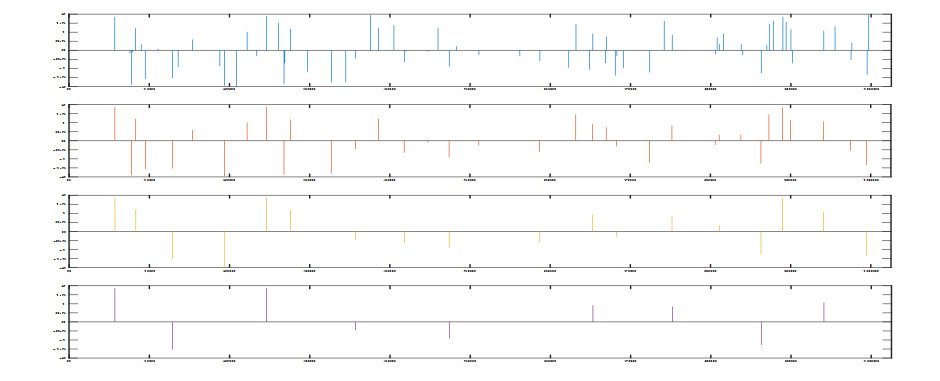
A straightforward observation confirms the satisfaction of all conditions in Theorem 1. Next, we conduct experiments to showcase the convergence and effectiveness of the proposed algorithm in recovering the $k$-sparse signal $v_k$ recovery problem with $k = 70, 35, 18, 9$ (see Figure 3).

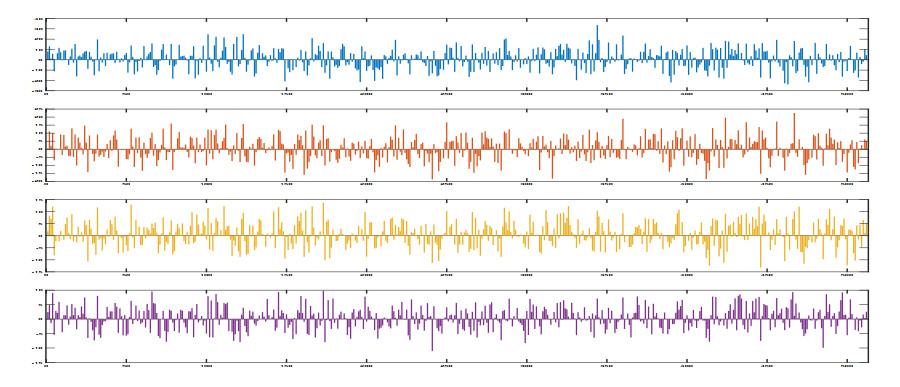
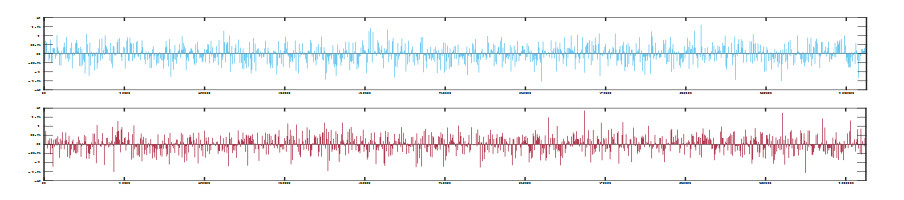
A signal of size $n = 1024$ elements, generated uniformly within the interval $[-2, 2]$, is utilized to produce observation signals $y_k = Av_k + \nu$, where $m = 512$ (see on Figure 4).

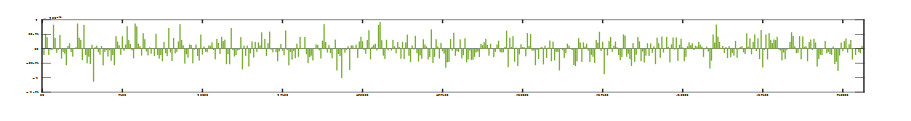
The white Gaussian noise $\nu$ is depicted in Figure 5.

The process starts with randomly selected initial signal data $v_0$ and $v_1$, each comprising $n = 1024$ randomly chosen elements (see Figure 6).

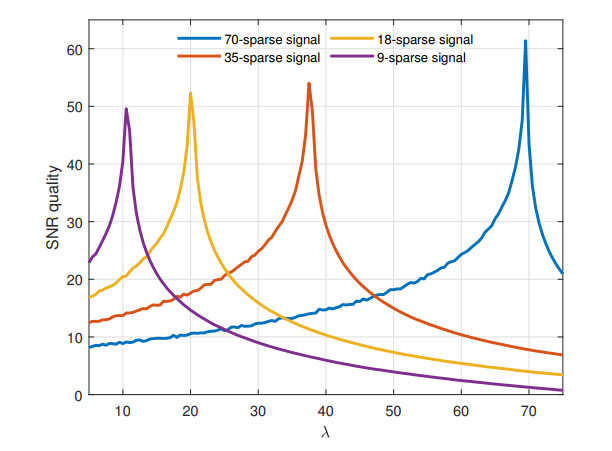
In addressing the challenge of recovering $k$-sparse signals, we reconstructed the observed signals depicted in Figure 4 to obtain the $k$-nonzero signal shown in Figure 3. Throughout this recovery process, we carefully considered the optimal regularization parameter, denoted as $\lambda$, to maximize the signal-to-noise ratio (SNR). The performance of the proposed method at $m^{th}$ iteration is measured quantitatively by means of the SNR, which is defined by

where $v_{m}$ is the recovered signal at the $m^{th}$ iteration using the proposed method. The SNR quality influenced by the regularization parameter $\lambda$ within the range $\left[5, 75\right]$ are visualized in Figure 7.

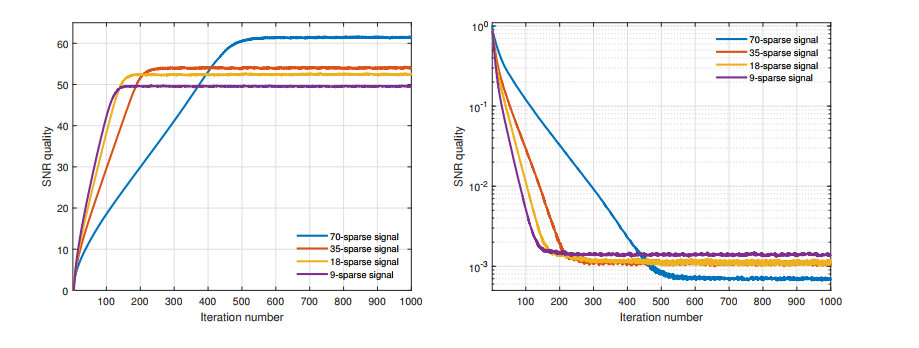
The most recent figure illustrates that the proposed algorithms can solve the sparse signal recovery challlenge. Moreover, we present the evolution of the SNR and relative error plot using max-norm over the number of iterations during the recovery of $k$-sparse signals with $k = 70, 35, 18, 9$ (see Figure 8). This is done while identifying the optimal regularization parameter, denoted as $\lambda$, to achieve the highest SNR quality, as illustrated in the figure above.

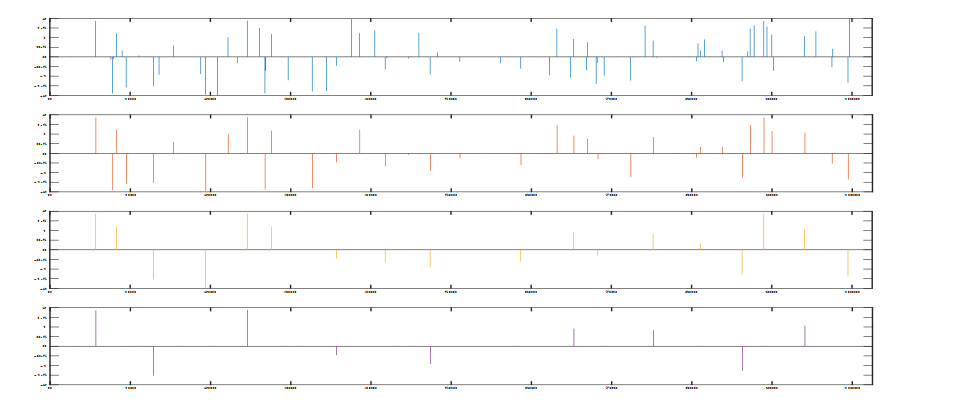
Notably, the plot of the signal's relative error exhibits a continuous decrease until it reaches convergence to a constant value. In the SNR quality plot, it is evident that the SNR value progressively rises until it stabilizes at a constant value. Additionally, Figure 9 demonstrates the best recovery of $k$-sparse signals with $k = 70, 35, 18, 9$ during 400 iterations using the proposed algorithm along with its optimal regularization parameter $\lambda$.

Based on these findings, it can be inferred that the proposed algorithm successfully enhances the quality of the recovered signal in solving the signal recovery problem.

5.   Conclusions

We constructed a novel algorithm with an inertial technique to approximate a fixed point of a nonexpansive mapping in a real uniformly convex Banach space with a Gâteaux differentiable norm. Furthermore, we found zeros of accretive mappings. An illustrative example was also provided as Example 1. Moreover, an application of the algorithm to a signal recovery problem was presented. We proved a strong convergence result, which is stronger than a weak convergence result.

Use of AI tools declaration

The authors declare they have not used Artificial Intelligence (AI) tools in the creation of this article.

Acknowledgments

This project is funded by the National Research Council of Thailand (NRCT) and University of Phayao Grant No. N42A660382.

Conflict of interest

The authors declare that they have no known competing financial interests or personal relationships that could have appeared to influence the work reported in this paper.