Finite-time stabilization of stochastic systems with varying parameters

1.   Introduction

In the last few decades, the theory of stochastic systems has been used and studied in the fields of mathematics and engineering. It has also been extensively employed to analyze and design stochastic problems with uncertain parameters. Since the efficiency of a control system is influenced and troubled by uncertainties, such as parameter variables, environmental noise and measurement accuracy ^[1], undesired and disordered behaviors can lead to the failure of the stability, and even the collapse of the system. Consequently, a control is designed and taking out the disturbance behaviors are developed to obtain the robustness analysis of the nonlinear stochastic system ^[2]. In previous research works, new sufficient conditions ^[3] for exponential stability and stabilization via parameter- dependent state feedback controllers were mentioned based on a time-varying version of the Lyapunov stability theorem. In 2006, Moulay and Perruquetti ^[4] studied the particular property of the finite stability, which is (settling time) required to reach the equilibrium that became efficient in many situations and that need an accelerated convergence of the trajectories to the target reference. Moreover, Bhat and Bernstein ^[3] provides a necessary condition involving the continuity of the settling-time function at the origin. Therefore, the main approach introduced in ^[4] was applied to prove the asymptotic stability by means of a Lyapunov function and an integral property condition equivalent to the finite time convergence was proposed. Some properties of finite-time stable stochastic nonlinear systems was derived in ^[5]. A recent paper given by ^[6] provide a backstepping technique and reduced-order observer in order to obtain the preassigned finite-time control scheme. In ^[7], the authors studied the stabilization via a finite-time optimal feedback control in order to obtain finite-time convergence. Hence, they provided an optimal feedback control for finite-time stability and finite-time stabilization.

Despite their importance, all the above-mentioned results are limited to the deterministic systems. The study of feedback stabilization of nonlinear deterministic systems has been intensively examined by the research community ^[3,8,9]. Afterwards, the obtained results were extended to a wide class of stochastic systems where equations were driven by the Wiener process.

Meanwhile, the homogeneous stochastic systems have also received considerable attention ^[10,11]. The theory of homogeneous dynamical systems has been explored for ordinary differential equations in recent years. In fact, the feature of homogeneous systems is the equivalence of the local and global properties. It was introduced by ^[12] and further developed by many authors ^[4,13]. In ^[10], the researchers noticed the existence of a homogeneous stabilizing feedback for a homogeneous control stochastic nonlinear system using the standard dilation. One of the important results given by Rosier ^[14] is the existence of a homogeneous Lyapunov function for any asymptotically stable homogeneous system. After determining the homogeneity of the stochastic systems as seen in the literature on homogeneous deterministic systems, the different stability properties of the nonlinear stochastic systems can be described in the same manner as deterministic systems. Indeed, in several works, the homogeneous systems were extended to stochastic systems. These ideas have been pursued by ^[15]. In ^[16], the authors considered the stability of the homogeneous stochastic systems and presented their stabilization method. They proved that a stable homogeneous system can be almost finite-time stable or surely $\rho$-exponentially stable in probability depending on the homogeneity degree. Moreover, in ^[17], it was shown that a stochastic nonlinear system is finite-time stable if its drift coefficient has a negative degree of homogeneity. The study presented in ^[15] discussed three kinds of stabilization of the stochastic homogeneous systems (rational, exponential or finite time convergence), depending on the sign's degree of homogeneity.

Besides, a control Lyapunov function (CLF) was proposed in ^[18,19,20] to stabilize an affine nonlinear system with uncertain parameters. In ^[9], the stabilization of affine nonlinear systems with bounded parameters was expressed as an extension of Sontag's control. However, this control failed to maintain the closed loop system's homogeneity. Motivated by the aforementioned discussions, this present study gives an extension to some results given in ^[9] on the deterministic systems to the stochastic systems driven by a Wiener process.

The authors in ^[15] investigated the stabilization of a control system

and provided a homogeneous feedback law inspired by ^[10]. Although this system guarantees the convergence of the system, this control unfortunately has no advantage if some perturbation affects the deterministic part of the system. In ^[2], the finite time stability of homogeneous stochastic systems was studied, while the stabilization issue of the stochastic system was neglected. Lyapunov-like techniques for stochastic stability was introduced in ^[21]. A preassigned-time controller with a novel performance function was developed in ^[22,23].

The applicability of the stabilization with varying parameters has been explored in many directions, and numerous extensions have been developed to tackle a wide range of problems. For instance, Baklouti et al. ^[24] developed an optimal preventive maintenance policy for a solar photovoltaic system, emphasizing the importance of efficient maintenance strategies in sustainable energy systems. Furthermore, the decision-making process for selling or leasing used vehicles was investigated in ^[25], considering factors such as their energetic type, potential leasing demand, and expected maintenance costs. Additionally, the authors in ^[26,27,28] explored quadratic Hom-Lie triple systems, contributing to the field of geometry and physics.

The objectives of this study are to design a feedback law that preserves the homogeneity of the system and maintain the robustness of the stability even if some perturbation affect the deterministic part of the system. Moreover, the finite time stability is attained by employing the proposed control.

This article is organized as follows: After presenting certain preliminaries in Section 2, a modified Sontag's control coherent to the homogeneous stochastic nonlinear systems is developed. Then, in Section 4, some results related to the stabilization of the finite time stochastic stability depending on a bounded parameter, are given.

2.   Preliminaries

Consider a stochastic nonlinear system:

where $t > 0$, $x \in \mathbb{R}^{n}$, $u \in \mathbb{R}^{m}$ are the system state, single control input, $G(x) = \left(G_{1}(x), \ldots, G_{m}(x)\right)^T$, $\sigma(x) = \left(\sigma_{1}(x), \ldots, \sigma_{m}(x)\right)^T$, $w$ denotes m-dimensional Wiener process (Brownian motion) and $\alpha \in \mathbb{R}^{d}$ represents the unknown time invariant parameter vector. We assume that the parameter $\alpha$ varies in a compact $\Omega \subset \mathbb{R}^{d}$.

The functions $F: {{{\rm{I\mkern-3.5mu R}}}}^n\times \Omega\rightarrow {{{\rm{I\mkern-3.5mu R}}}}^n$ and $G: {{{\rm{I\mkern-3.5mu R}}}}^n\rightarrow {{{\rm{I\mkern-3.5mu R}}}}^{n\times m}$ and $\sigma: {{{\rm{I\mkern-3.5mu R}}}}^n\rightarrow {{{\rm{I\mkern-3.5mu R}}}}^{n\times m}$ are smooth vector fields with $F(0, \alpha) = 0, \ G(0) = 0$ and $\sigma(0) = 0$ for all $\alpha \in \Omega$ which implies that the system (2.1) has a trivial zero solution.

2.1.   Homogeneity

In this subsection, the definitions of homogeneity cited in ^[12] are adopted.

Definition 2.1. Let $x\in{{{\rm{I\mkern-3.5mu R}}}}^n$, $\lambda > 0$ and $\{r_{1}, ..., r_n\}$ are n-tuple positive real numbers. The mapping $\delta_{\lambda}^{r}:{{{\rm{I\mkern-3.5mu R}}}}^n \rightarrow {{{\rm{I\mkern-3.5mu R}}}}^n$ where $\delta_{\lambda}^{r} x = \left(\lambda^{r_{1}} x_{1}, \ldots, \lambda^{r_{\pi}} x_{n}\right)$ is called weighted dilation.

Definition 2.2. ⅰ) A function $h: \mathbb{R}^{n} \times \Omega \rightarrow \mathbb{R},$ is homogeneous function of degree $\tau$ if

ⅱ) A vector field $f: \mathbb{R}^{n}\times \Omega \rightarrow \mathbb{R}^{n}$ is homogeneous of degree $\tau$ if each

$f_{i}$, where $j = 1, \ldots, n$, is a homogeneous function of degree $\tau+r_{j}$, i.e.,

Definition 2.3. (^[14]) Let $V:{{{\rm{I\mkern-3.5mu R}}}}^n \rightarrow {{{\rm{I\mkern-3.5mu R}}}}$, suppose that $V$ is a smooth homogeneous function of degree $k$, then:

Definition 2.4. The system (2.1) is said to be a homogeneous Itô stochastic system of degree $(k_0, k_1)$ if the vector fields $F$, $G$ and $\sigma$ are homogeneous functions of degree $k_0$, $k_1$ and $k_{0} / 2$, respectively.

We omit the modifier (with respect to a dilation $\delta_{\lambda}^{r}$) if no confusion arises.

3.   Construction of a stabilizing feedback

In this section, a homogeneous feedback control is constructed using homogeneous control Lyapunov functions that stabilize the control system (2.1).

Definition 3.1. The control system (2.1) is said to be stabilizable (respectively continuously stabilizable) if there exist a nonempty neighborhood of the origin $\mathcal{V}$ in $\mathbb{R}^{n}$ and a feedback control law $u \in C^{0}\left(\mathcal{V} \backslash\{0\}, \mathbb{R}^{m}\right)$ (respectively $\left.u \in C^{0}\left(\mathcal{V}, \mathbb{R}^{m}\right)\right)$ such that:

1) $u(0) = 0$,

2) the origin of the closed loop-system (2.1) is asymptotically stable in probability for all $\alpha \in \Omega$.

Notations 1. Let $\Omega$ be a compact subset of $\mathbb{R}^{d}, \mathcal{V}$ a neighborhood of the origin and $V: \mathcal{V} \rightarrow \mathbb{R}_{+}$ a continuously differentiable function. Let $(x, \alpha) \in \mathcal{V} \times \Omega$. ${\mathcal{L}}$ denotes the infinitesimal generator.

Where $\frac{\partial V}{\partial x} = (\frac{\partial V}{\partial x_1}, ..., \frac{\partial V}{\partial x_n})$ and $\frac{\partial^{2}V}{\partial x^2} = (\frac{\partial^{2}V}{\partial x_i\partial x_j})_{n\times n}$ we define:

With a minor change in hypothesis (Sontag's small control property), the concept of CLF is presented as follows:

Definition 3.2. 1) A $C^2$ positive definite function $V: \mathcal{V} \rightarrow \mathbb{R}_{+}$ where $\mathcal{V}$ corresponds to a neighborhood of 0 in ${{{\rm{I\mkern-3.5mu R}}}}^n$ is said to be a CLF for the stochastic system (2.1) if the following condition is satisfied: $\forall x \in \mathcal{V} \backslash\{0\}$ and $\forall \alpha \in \Omega$ one has

2) A CLF $V$ for the stochastic system (2.1) is said to satisfy the small control property, if for all $\epsilon > 0,$ there exists $\delta > 0$ such that if $\|x\| < \delta,$ there exists a control $u$ ($\|u\| < \epsilon$), verifying ${\mathcal{A}}(x, \alpha)+\langle B(x), u\rangle < 0$ for all $\alpha \in \Omega$.

The other version of the last definition is presented as follows:

Definition 3.3. ^[15] A function $V:{{{\rm{I\mkern-3.5mu R}}}}^n\rightarrow {{{\rm{I\mkern-3.5mu R}}}}$ is a stochastic homogeneous control Lyapunov function of the homogeneous control system (2.1) if the function $V(x)$ is twice continuously differentiable on ${{{\rm{I\mkern-3.5mu R}}}}^n$, positive definite, radially unbounded and homogeneous with respect to the dilation $\delta$, and that is for any $x\in{{{\rm{I\mkern-3.5mu R}}}}^n\setminus\{0\}$

Remark 1. If $m = 1,$ the last condition is equivalent to

Lemma 3.1. Let $H: \mathbb{R}^{n} \times \Omega \rightarrow \mathbb{R}^{n}$. $\bar{H}(x): = \max _{\alpha \in \Omega} H(x, \alpha)$ is continuous on $\mathbb{R}^{n}$ if $H$ is continuous on $\mathbb{R}^{n} \times \Omega,$ where $\Omega$ is a compact set of $\mathbb{R}^{d}$.

3.1.   Homogeneous feedback stabilization depending on a parameter

In the present section, we provide a result of stabilization of the homogeneous stochastic control system (2.1), when $m = 1$.

Consider the single input system described by

Lemma 3.2. Let $V: \mathbb{R}^{n} \rightarrow \mathbb{R}$ of class $C^{1}$, be homogeneous of degree $k$. Let $y \in \mathbb{R}^{n} \backslash\{0\}$ and $\lambda > 0$; by setting $x = \delta_{\lambda}(y)$, one has for all $\alpha \in \Omega$

and

Proof. For $y \in \mathbb{R}^{n} \backslash\{0\}$ and $\lambda > 0;$ let $x = \delta_{\lambda}(y)$, so

where

So for $\alpha \in \Omega$, one has

A similar computation gives

Theorem 3.1. If there exists a CLF $V: \mathbb{R}^{n} \rightarrow \mathbb{R}_{+}$ which is continuously differentiable for the homogeneous system (3.3) of degree $k$ then

stabilizes the system (3.3) and it is homogeneous of degree $k_{0}-k_{1}$, where $p = \tfrac{2 q\left(k+k_{1}\right)}{k+k_{0}}$.

Additionally, if $V$ satisfies the small control property, then the controller (3.4) stabilizes continuously the system (3.3).

Proof. Let $H = \{(x, y)\in{{{\rm{I\mkern-3.5mu R}}}}^2: x < 0, \mbox{ or } y > 0\}$, and the function $\theta$ defined on $H$ by

Using ^[13], $\theta$ is continuous on $E$. We can verify that $(\bar{{\mathcal{A}}}(x), {\mathcal{B}}(x))\in E$. Therefore, the control

is continuous.

Let $V: \mathbb{R}^{n} \rightarrow \mathbb{R}$ be an homogeneous CLF for the system (3.3).

Then, the equilibrium point of the closed loop system (3.3) is stochastic asymptotically stable, and the controller (3.4) continuously stabilizes the system (3.3).

Afterwards, we verify that the controller $u$ is homogeneous of degree $k_{0}-k_{1}$. We set $x = \delta_{\lambda}(y)$, by lemma 3.2, $\forall \alpha \in \Omega$ we get

So

In addition, we have

using $p = \tfrac{2 q\left(k+k_{1}\right)}{k+k_{0}}$, then $u$ given by (3.4) is homogeneous of degree $k_{0}-k_{1}.$

3.2.   Stabilization of affine system depending on a parameter

Consider a stochastic nonlinear system:

The following result is an extension of Theorem 3.1, where $f$ (and $\sigma$) are homogeneous of degree $k_0$ (and $\frac{k_0}{2}$ resp) and all $G_i$ are homogeneous of same degree $k_1, \ \ \forall i\in\{1, ..., m\}$.

Theorem 3.2. Suppose that for a stochastic homogeneous control system (2.1) there exists a stochastic homogeneous control Lyapunov function $V: \mathcal{V} \rightarrow \mathbb{R}_{+}$, then it is stabilizable using the controller

for $i \in\{1, \ldots, m\}$ where $p > 1$, and $q > 1$ are real positive numbers. Furthermore, the control (3.5) is continuous at the origin if $V$ satisfies the small control property.

Proof. Using the same assumptions presented in Theorem 3.1, the controller $u$ is continuous and homogeneous of degree $k_0-k_1$.

On the other hand,

Then, the equilibrium point of the closed loop system (2.1) is stochastic asymptotically stable.

An illustrative example is given to show the effectiveness and applicability of the proposed controller.

Example 1. Consider a stochastic control system given by

where

Taking into account the dilation $\delta_{\lambda}^{r}(x) = (\lambda^3x_1, \lambda x_2)$. The functions F and G are of degree 0 and $-1$. The Lyapunov function is defined as follow

$V$ is homogeneous of degree 4. We have

and ${\mathcal{B}}(x) = -2x_{1}+16x_{2}^{3}$. Let $(p, q) = (2, \frac{4}{3})$, by Theorem 3.1, the feedback

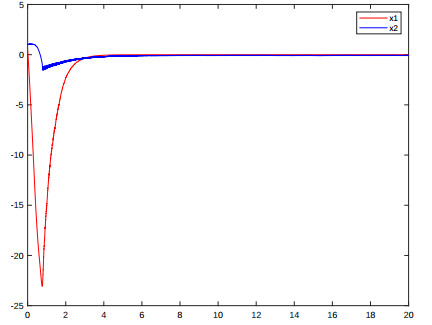
is homogeneous of degree 1 and stabilizes the system (3.6). Figures 1 and 2 insured the effectiveness of the controller.

Remark 2. The authors in ^[15] dealt with the stabilization of homogeneous systems and provided a controller $u(x) = -K \|x\|^{\eta}L_{G}V(x)$ (where $\eta = k_0-k-2k_1$) using the homogeneous norm. This controller succeeded to preserve the homogeneity of the system but failed to achieve the stabilization if some perturbation affected the deterministic part of the system. It can be seen (Figures 3 and 4) using the controller injected in the Example 1 that the trajectory of the system (3.6) diverge.

4.   Finite control Lyapunov function

We shall look into a few characteristics of a stochastic system with a finite-time stability.

Definition 4.1. ^[17] The trivial zero solution of (2.1) is said to be finite-time stochastically stable, if the equation admits a unique solution for any initial value $x_0\in{{{\rm{I\mkern-3.5mu R}}}}^n$, denoted by $x(t, x_0)$ moreover, the following properties hold:

a) Finite-time attractiveness in probability: for any initial value $x_0\in{{{\rm{I\mkern-3.5mu R}}}}^n\setminus\{0\}$, the first hitting time $\tau(x_0) = \inf \{t, x(t, x_0) = 0\}$, called the stochastic settling time, is finite almost surely, that is $P\{\tau (x_0) < \infty\} = 1$.

b) Stability in probability: for each pair of $\epsilon\in (0, 1)$ and $r > 0$ there exists a $\delta = \delta(\epsilon, r)$ such that

Definition 4.2. Let $c > 0$ and $0 < \beta < 1$. A function $V: \mathbb{R}^{n} \rightarrow \mathbb{R}_{+}$ positive definite is a f-CLF for (2.1) if it is proper and satisfies for $x \neq 0$

Theorem 4.1. Suppose that the system (2.1) has a f-CLF then the controller

makes the solution finite time stable in probability.

Besides, the setting time verifies

Proof. Let $x \in \mathbb{R}^{n} \backslash\{0\}\ \ i\in\{1, ..., m\}$, there are two cases:

1) If $L_{G_{i}}V(x) = 0, \ \ \forall i \Rightarrow {\mathcal{B}}(x) = 0$, yields to

2) If there exists $1 \leq i \leq m$, such that $L_{G_{i}}V(x) \neq 0$, that means ${\mathcal{B}}(x) \neq 0$, we get

This implies that the system (2.1) is finite time stable in probability under the control (4.2).

We prove now that

Suppose that $x_0$ verifies $V(x_0) > \dfrac{1}{k}$.

Consider $\tau_k = \inf\{t\backslash V(x(t, x_0)) < \dfrac{1}{k}\}$. According to (^[16], p 89), $\mathcal{P}[\tau_k < \infty] = 1$.

Let $\tilde{V}(x) = V(x)^{1-\beta}$,

So $\forall x\neq 0$ we have $\dot{\tilde{V}}(x) = \dfrac{1-\beta}{V^{\beta}(x)}L_0V(x)-\dfrac{\beta(\beta-1)}{2V^{\beta+1}(x)}trace [\sigma^{T}.\dfrac{\partial^{2}V}{\partial x^2}.\sigma]$

Using (4.1), we obtain:

According to Dynkin's formula and (4.4),

As conformed by Fatou's lemma, (4.5) yields to

It can be seen from the following illustrative example how the system's state trajectories converge to zero in finite time.

Example 2. Consider a stochastic control system given by

where

Let $\alpha (t) = \dfrac{1}{\sqrt{t}+2}+1\in [1, \frac{3}{2}]$ and define Lyapunov function as $V(x) = \frac{1}{2}(x_{1}^2+x_{2}^2)$.

We have

and ${\mathcal{B}}(x) = x_{2}^2$. Since ${\mathcal{B}}(x) = 0 \Rightarrow x_{2} = 0$ then

By Theorem 4.1, the feedback

stabilizes the system in finite time in probability and Figure 5 ensured the effectiveness of the method.

5.   Conclusions

The efficiency of a control system is influenced and troubled by uncertainties, such as environmental noise and measurement accuracy. In consequence, to maintain the stochastic stability of the system, a homogeneous controller was proposed in this manuscript. Some works in the literature, such as ^[15], developed a controller to stabilize a stochastic input for affine control systems. The authors dealt with the stabilization of homogeneous systems and provided a controller $u_i(x) = -K \|x\|^{\eta_i}L_{g_i}V(x)$ using the homogeneous norm. This controller succeeded to preserve the homogeneity of the system but failed to make it stable if some perturbation affects the deterministic part of the system. Therefore, the objective of using the controller introduced in the present work is to maintain both homogeneity of the system as well as robustness stochastic stability, even if some perturbations disturb the deterministic part of the system. On the other hand, based on the finite time stability theory for stochastic systems, a controller was developed.

Use of AI tools declaration

The authors declare they have not used Artificial Intelligence (AI) tools in the creation of this article.

Acknowledgments

The authors would like to thank the Deanship of Scientific Research at Umm Al-Qura University for supporting this work grant code: 23UQU4331225DSR001.

Conflict of interest

The authors declare no conflict of interest.