Numerical investigations of nonlinear Maxwell fluid flow in the presence of non-Fourier heat flux theory: Keller box-based simulations

Afraz Hussain Majeed; Sadia Irshad; Bagh Ali; Ahmed Kadhim Hussein; Nehad Ali Shah; Thongchai Botmart; Afraz Hussain Majeed; Sadia Irshad; Bagh Ali; Ahmed Kadhim Hussein; Nehad Ali Shah; Thongchai Botmart

doi:10.3934/math.2023631

AIMS Mathematics

2023, Volume 8, Issue 5: 12559-12575. doi: 10.3934/math.2023631

Previous Article Next Article

Research article

Numerical investigations of nonlinear Maxwell fluid flow in the presence of non-Fourier heat flux theory: Keller box-based simulations

1.
Department of Mathematics, Air University, PAF Complex E-9, Islamabad 44000, Pakistan
2.
Institute of Mathematics, Khwaja Fareed University of Engineering and Information Technology, Rahim Yar Khan, Punjab 64200, Pakistan
3.
Faculty of Computer Science and Information Technology, Superior University, Lahore 54000, Pakistan
4.
Mechanical Engineering Department, College of Engineering, University of Babylon, Hilla 00964, Iraq
5.
College of Engineering, University of Warith Al-Anbiyaa, Karbala 56001, Iraq
6.
Department of Mechanical Engineering, Sejong University, Seoul 05006, South Korea
7.
Department of Mathematics, Faculty of Science, Khon Kaen University, Khon Kaen 40002, Thailand
† These authors contributed equally to this work and are co-first authors

Received: 18 October 2022 Revised: 17 November 2022 Accepted: 18 December 2022 Published: 27 March 2023
MSC : 76-10, 76R10

We investigate the thermal flow of Maxwell fluid in a rotating frame using a numerical approach. The fluid has been considered a temperature-dependent thermal conductivity. A non-Fourier heat flux term that accurately reflects the effects of thermal relaxation is incorporated into the model that is used to simulate the heat transfer process. In order to simplify the governing system of partial differential equations, boundary layer approximations are used. These approximations are then transformed into forms that are self-similar with the help of similarity transformations. The mathematical model includes notable quantities such as the rotation parameter $\lambda$ , Deborah number $\beta$ , Prandtl number Pr, parameter $ϵ$ and the dimensionless thermal relaxation times $\gamma$ . These are approximately uniformly convergent. The Keller box method is used to find approximate solutions to ODEs. We observed due to the addition of elastic factors, the hydrodynamic boundary layer gets thinner. The thickness of the boundary layer can be reduced with the use of the k rotation parameter as well. When Pr increases, the wall slope of the temperature increases as well and approaches zero, which is an indication that Pr is decreasing. In addition, a comparison of the Cattaneo-Christov (CC) and Fourier models are provided and discussed.

Keywords:

Citation: Afraz Hussain Majeed, Sadia Irshad, Bagh Ali, Ahmed Kadhim Hussein, Nehad Ali Shah, Thongchai Botmart. Numerical investigations of nonlinear Maxwell fluid flow in the presence of non-Fourier heat flux theory: Keller box-based simulations[J]. AIMS Mathematics, 2023, 8(5): 12559-12575. doi: 10.3934/math.2023631

Related Papers:

[1]	Sheheryar Shah, M. N. Abrar, Kamran Akhtar, Aziz Khan, Thabet Abdeljawad . Entropy formation analysis for magnetized UCM fluid over an exponentially stretching surface with PST and PSHF wall conditions. AIMS Mathematics, 2023, 8(5): 11666-11683. doi: 10.3934/math.2023591
[2]	S. R. Mishra, Subhajit Panda, Mansoor Alshehri, Nehad Ali Shah, Jae Dong Chung . Sensitivity analysis on optimizing heat transfer rate in hybrid nanofluid flow over a permeable surface for the power law heat flux model: Response surface methodology with ANOVA test. AIMS Mathematics, 2024, 9(5): 12700-12725. doi: 10.3934/math.2024621
[3]	Yasir Khan, Sohaib Abdal, Sajjad Hussain, Imran Siddique . Numerical simulation for thermal enhancement of $ H_2O $ + Ethyl Glycol base hybrid nanofluid comprising $ GO + (Ag, AA7072, MoS_2) $ nano entities due to a stretched sheet. AIMS Mathematics, 2023, 8(5): 11221-11237. doi: 10.3934/math.2023568
[4]	Haifaa Alrihieli, Musaad S. Aldhabani, Ghadeer M. Surrati . Enhancing the characteristics of MHD squeezed Maxwell nanofluids via viscous dissipation impact. AIMS Mathematics, 2023, 8(8): 18948-18963. doi: 10.3934/math.2023965
[5]	Jean-Paul Pascal, Serge D’Alessio, Syeda Rubaida Zafar . The instability of liquid films with temperature-dependent properties flowing down a heated incline. AIMS Mathematics, 2019, 4(6): 1700-1720. doi: 10.3934/math.2019.6.1700
[6]	Khalil Ur Rehman, Wasfi Shatanawi, Zeeshan Asghar, Haitham M. S. Bahaidarah . Neural networking analysis for MHD mixed convection Casson flow past a multiple surfaces: A numerical solution. AIMS Mathematics, 2023, 8(7): 15805-15823. doi: 10.3934/math.2023807
[7]	Asifa, Poom Kumam, Talha Anwar, Zahir Shah, Wiboonsak Watthayu . Analysis and modeling of fractional electro-osmotic ramped flow of chemically reactive and heat absorptive/generative Walters'B fluid with ramped heat and mass transfer rates. AIMS Mathematics, 2021, 6(6): 5942-5976. doi: 10.3934/math.2021352
[8]	Yellamma, N. Manjunatha, Umair Khan, Samia Elattar, Sayed M. Eldin, Jasgurpreet Singh Chohan, R. Sumithra, K. Sarada . Onset of triple-diffusive convective stability in the presence of a heat source and temperature gradients: An exact method. AIMS Mathematics, 2023, 8(6): 13432-13453. doi: 10.3934/math.2023681
[9]	Kiran Sajjan, Nehad Ali Shah, N. Ameer Ahammad, C.S.K. Raju, M. Dinesh Kumar, Wajaree Weera . Nonlinear Boussinesq and Rosseland approximations on 3D flow in an interruption of Ternary nanoparticles with various shapes of densities and conductivity properties. AIMS Mathematics, 2022, 7(10): 18416-18449. doi: 10.3934/math.20221014
[10]	W. Abbas, Ahmed M. Megahed, Osama M. Morsy, M. A. Ibrahim, Ahmed A. M. Said . Dissipative Williamson fluid flow with double diffusive Cattaneo-Christov model due to a slippery stretching sheet embedded in a porous medium. AIMS Mathematics, 2022, 7(12): 20781-20796. doi: 10.3934/math.20221139

Abstract

1. Introduction

Generalized Newtonian fluid mechanics has been a inspirational field as it describes such central problems from food processing, chemical and petroleum industries. Its models are valuable to find the effects of fluid flow in nature and industries such as biological fluid, pastes, polymeric liquids and numerous complicated mixtures. Viscoelastic fluids are exclusive generalized Newtonian fluids in which the shear-stress memory function of deformation rate. In these types of fluids, the deformation rate generally decreases when the shear stress is eliminated. This phenomenon is called stress relaxation. Many researchers have given special concentrate to the boundary layer flows of Maxwell fluid in the current background. The coupled flow and heat transfer of an upper-convected Maxwell fluid over a stretching plate with a velocity slip barrier was investigated by Han et al. ^[1]. The work under consideration obtained an analytical solution. The rotating flow of upper-convected Maxwell fluid was studied using the CC heat flux model by Mustafa ^[2], and it was discovered that the fluid velocity significantly rises. Bhattacharyya et al. ^[3] have introduced multiple results for Maxwell fluid with a porous shrinking surface by shooting numerical scheme. Fetecau et al. ^[4,5] have introduced the unsteady flows in Maxwell fluid with oscillation and acceleration mature in the rigid body in flow. Mahsud et al. ^[6] studied the Influence of time-fractional derivatives on the boundary layer flow of Maxwell fluids. It introduced that the Maxwell fluid is reasonable for relaxation effects which can't be forecast in other various kinds of non-Newtonian fluid. Khan et al. ^[7] give a scientific report on heat transfer analysis in mixed convection flow of Maxwell fluid over an oscillating vertical plate. S. K. Nandy ^[8] investigated the heat transfer assessment over a decreasing sheet with the Navier slip effect and unsteady upper-convected Maxwell nanofluid flow, and they found that the quantities of the Nusselt number increased with increasing values of the Maxwell variable. Shah et al. ^[9] provided an idea of Maxwell fluid flow between vertical plates with damped shear and thermal flux a free convection phenomenon. Cao et al. ^[10] investigated the steady flow of Maxwell fluid over a porous medium using an interpolation methodology that is equivalent to the implicit RK. scheme. The expanding subject of engineering known as hybrid nano-liquid has captured the attention of a large number of researchers who were seeking for ways to increase the efficiency of cooling processes used in industry. These researchers were looking for ways to improve the effectiveness of cooling processes. A nano-liquid is a type of fluid that is created when powerful particles with sizes less than 100 nm in diameter are propagated in other fluids. One of the noticeable properties of a nano-liquid is its low thermal conductivity, which is one of the characteristics that can hinder the effectiveness of heat transport. Karim and Samad ^[11] examined the effect of Brownian diffusion with CC heat flux on viscoelastic squeeze nanofluid flow and double slip impact in a channel. Specifically, they focused on the effects of these two factors. The effect of thermal radiation on the transmission of heat and mass through an unstable stretched surface was examined by Shateyi and Motsa ^[12]. Additionally, they noticed that when the values of the unsteadiness parameter grew, the velocity profiles and concentration distributions decreased. Abel et al. ^[13] used a numerical approximation to study the flow of a Maxwell material close to a stretchy surface. The temperature of the fluid medium above the sheet decreases when the radiation parameter is increased. When necessary, this effect might be employed to quicken the sheet's cooling process. The Maxwell effect's implications in relation to issues with molecular transport were examined by C. I. Christov ^[14]. To the best of authors' knowledge, no attempt has yet been made to include the Maxwell delay in the closure models for turbulent fluxes, and this potential is explored in the current study. B. Straughan ^[15] used a Cattaneo-type constitutive energy equation to investigate the heat flux caused by thermal convection in an incompressible viscous fluid. They showed that when the Cattaneo number is high enough and the convection mechanism flips from stationary convection to oscillatory convection for smaller magnitudes, thermal relaxation exhibits a significant influence. Tibullo et al. ^[16] introduced the uniqueness of solutions for heat transfer in an incompressible fluid by Cattaneo-Shristov law. In the framework of the CC model, S. Hadded ^[17] analyzes the thermal instability of flow through a porous media.

Aims of this paper are the numerical study of viscoelastic fluid flow in rotating surfaces with variable thermal conductivity. In this, firstly, mathematical modeling for laminar flow of Maxwell fluid with a stretchable rotating surface. Secondly, to investigate the effects of heat transfer with thermal conductivity. Finally, to solve numerical results by using the Keller box based on a finite difference scheme. Furthermore, the following strategy is being utilized for the current communication: The equation that describes the characteristics of fluid flow can be found in Section 2 of the document. Section 3 disclosure includes the procedure for the numerical system that was implemented. In Section 4, the primary conclusions are presented in the form of rough sketches of a few of the involved quantities. In Section 5, the results are discussed.

2. Mathematical modeling

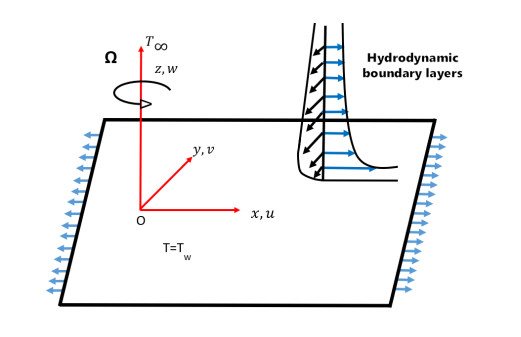
Let's imagine a stretchy surface as an incompressible Maxwell fluid laminar flow (see Figure 1).

Figure 1. Schematic diagram of the problem.

DownLoad: Full-Size Img PowerPoint

We choose the Cartesian coordinate system so that the fluid is taken into account in space $z\ge 0$ and the surface is aligned with the xy-plane. The fluid is considered to spin continuously about the z-axis with constant angular velocity $\varOmega$ while the surface is assumed to stretch in the x-direction at a greater rate. We investigate a non-Fourier heat conduction model because the temperature of the sheet, denoted by the symbol ${T}_{W}$ , is constant and is thought to be higher than the ambient temperature ${T}_{\infty }$ .

Below are given the pertinent equations that represent the Maxwell fluid flow in a rotating frame ^[6].

$\nabla \cdot \mathbf{V} = \mathbf{0} \text {, }$

(2.1)

$\left\{\left(\boldsymbol{V}.\nabla \right)\boldsymbol{V}+\left(\mathbf{\Omega }\times \left(\mathbf{\Omega }\times \boldsymbol{r}\right)\right)+\left(2\mathbf{\Omega }\times \boldsymbol{V}\right)\right\} = -\nabla p+\nabla .\boldsymbol{S},$

(2.2)

where the pressure and fluid density are denoted by $p \ \mathrm{a}\mathrm{n}\mathrm{d} \ \rho ,$ while the angular velocity is $\mathbf{\Omega } = \left[0, 0, \mathrm{\Omega }\right].$ The stress tensor S for upper-convected Maxwell fluid follows the relation

$\left(1+{\lambda }_{1}\frac{D}{Dt}\right)\boldsymbol{S} = \mu {\mathbf{A}}_{1},$

(2.3)

where the fluid relaxation time is ${\lambda }_{1},$ first Rivlin-Ericksen tensor is ${\mathbf{A}}_{1} = \left(\nabla \boldsymbol{V}\right)+{\left(\nabla \boldsymbol{V}\right)}^{T}$ and the derivative of upper-convection with time is $\frac{D}{Dt}.$ For second rank tensor S and vector a, as

$\frac{D\boldsymbol{S}}{Dt} = \frac{\partial \boldsymbol{S}}{\partial t}+\left(\mathbf{V}.\nabla \right)\boldsymbol{S}-\boldsymbol{L}\boldsymbol{S}-\boldsymbol{S}{\boldsymbol{L}}^{\boldsymbol{T}},$

(2.4)

$\frac{D\boldsymbol{a}}{Dt} = \frac{\partial \boldsymbol{a}}{\partial t}+\left(\mathbf{V}.\nabla \right)\boldsymbol{a}-\boldsymbol{L}\boldsymbol{a},$

(2.5)

Now, consider $\left(1+{\lambda }_{1}\frac{D}{Dt}\right)$ operator to applying in Eq (2.3), then compute the component forms after implementation of boundary layer approximation while the resulting equations are obtained as ^[18]

$\text{u}\frac{{\partial u}}{{\partial x}} + v\frac{{\partial u}}{{\partial y}} + w\frac{{\partial u}}{{\partial z}} - 2\Omega v + {\lambda _1}\left[ {\begin{array}{*{20}{l}} {u^2}\frac{{{\partial ^2}u}}{{\partial {x^2}}} + {v^2}\frac{{{\partial ^2}u}}{{\partial {y^2}}} + {w^2}\frac{{{\partial ^2}u}}{{\partial {z^2}}} + 2uv\frac{{{\partial ^2}u}}{{\partial x\partial y}} + 2vw\frac{{{\partial ^2}u}}{{\partial y\partial z}} \hfill \\ + 2uw\frac{{{\partial ^2}u}}{{\partial x\partial z}} - 2\Omega \left( {u\frac{{\partial v}}{{\partial x}} + v\frac{{\partial v}}{{\partial y}} + w\frac{{\partial v}}{{\partial z}}} \right) \hfill \\ + 2\Omega \left( {v\frac{{\partial u}}{{\partial x}} - u\frac{{\partial u}}{{\partial y}}} \right) \hfill \\ \end{array}} \right] = v\frac{{{\partial ^2}u}}{{\partial {z^2}}},$

(2.6)

$\text{u}\frac{{\partial v}}{{\partial x}} + v\frac{{\partial u}}{{\partial y}} + w\frac{{\partial v}}{{\partial z}} + 2\Omega u + {\lambda _1} \left[ {\begin{array}{*{20}{l}} {u^2}\frac{{{\partial ^2}u}}{{\partial {x^2}}} + {v^2}\frac{{{\partial ^2}u}}{{\partial {y^2}}} + {w^2}\frac{{{\partial ^2}u}}{{\partial {z^2}}} + 2uv\frac{{{\partial ^2}u}}{{\partial x\partial y}} + 2vw\frac{{{\partial ^2}u}}{{\partial y\partial z}} \hfill \\ + 2uw\frac{{{\partial ^2}u}}{{\partial x\partial z}} + 2\Omega \left( {u\frac{{\partial v}}{{\partial x}} + v\frac{{\partial v}}{{\partial y}} + w\frac{{\partial v}}{{\partial z}}} \right) \hfill \\ + 2\Omega \left( {v\frac{{\partial u}}{{\partial x}} - u\frac{{\partial u}}{{\partial y}}} \right) \hfill \\ \end{array}} \right] = v\frac{{{\partial ^2}v}}{{\partial {z^2}}},$

(2.7)

The energy balance equation is provided by under the supposition that viscous dissipation and heat generation/absorption effects are zero.

$\rho {c_p}({\mathbf{V}} \cdot \nabla T) = - \nabla \cdot {\mathbf{q}},$

(2.8)

${\mathbf{q}} = - {\lambda _3}\left( {\frac{{\partial {\mathbf{q}}}}{{\partial t}} + {\mathbf{V}} \cdot \nabla {\mathbf{q}} - {\mathbf{q}} \cdot \nabla {\mathbf{V}} + (\nabla \cdot {\mathbf{V}}){\mathbf{q}}} \right) - k\nabla T,$

(2.9)

where $k$ is the thermal conductivity and ${\lambda }_{3}$ is the thermal relaxation time. The time delay required for the start of a heat flow after a temperature gradient has been introduced at a certain place is known as the thermal relaxation time. The heat can be transported via thermal waves that travel at a finite rate according to the non-Fourier heat flux phenomenon. We eliminate q from Eqs (2.8) and (2.9) to achieve the following:

$\begin{gathered} \ \ \text{u }\frac{{\partial T}}{{\partial x}} + v\frac{{\partial u}}{{\partial y}} + w\frac{{\partial T}}{{\partial z}} \hfill \\ = \frac{1}{{\rho {c_p}}}\frac{\partial }{{\partial z}}\left( {k\frac{{\partial T}}{{\partial z}}} \right) - {\lambda _3}\left( \begin{gathered} {u^2}\frac{{{\partial ^2}u}}{{\partial {x^2}}} + {v^2}\frac{{{\partial ^2}u}}{{\partial {y^2}}} + {w^2}\frac{{{\partial ^2}u}}{{\partial {z^2}}} + 2uv\frac{{{\partial ^2}u}}{{\partial x\partial y}} + 2vw\frac{{{\partial ^2}u}}{{\partial y\partial z}} + \hfill \\ 2uw\frac{{{\partial ^2}u}}{{\partial x\partial z}} + \left( {u\frac{{\partial v}}{{\partial x}} + v\frac{{\partial v}}{{\partial y}} + w\frac{{\partial v}}{{\partial z}}} \right) + \left( {v\frac{{\partial u}}{{\partial x}} - u\frac{{\partial u}}{{\partial y}}} \right) \hfill \\ \end{gathered} \right){\text{.}} \hfill \\ \end{gathered}$

(2.10)

The flow is subjected to the following conditions:

$\left\{ {\begin{array}{*{20}{l}} {u = ax, \ \ v = 0, \ \ w = o, \ \ T = {T_w}, \ \ \ \ {\text{ at }}z = 0, } \\ {u \to 0, \ \ v \to 0, \ \ T \to {T_\infty }, \ \ \ \ {\text{ at }}z \to \infty .} \end{array}} \right.$

(2.11)

We seek the similarity solution of the problem in the following form:

$f'\left( \eta \right) = \frac{u}{{ax}}, \ \ g\left( \eta \right) = \frac{v}{{ax}}, \ \ \text{w} = - \sqrt {av} f\left( \eta \right), \ \ \theta \left( {{T_w} - {T_\infty }} \right) = \left( {T - {T_\infty }} \right), \ \ \eta = \sqrt {\frac{a}{v}} z{\text{ , }}$

(2.12)

where prime denotes a change in differentiation with regard to $\eta$ . The equation $k = {k}_{\infty }\left[1+\frac{ϵ\left(T-{T}_{\infty }\right)}{\Delta T}\right]$ , where $ϵ > 0$ is a positive constant and ${k}_{\infty }$ specifies the thermal conductivity at ambient temperature, further assumes that the thermal conductivity varies with temperature $T$ . Equation (2.1) is automatically satisfied by transformation (2.12), and Eqs (2.6), (2.7) and (2.10) are transformed into the following differential equations:

$f''' + ff'' - f{'^2}{\text{ + 2}}\lambda g - 2\lambda \beta fg' + 2\beta ff'f'' - \beta {f^2}f''' = 0{\text{, }}$

(2.13)

$g'' + fg' - f'\text{g} - {\text{2}}\lambda f' - 2\lambda \beta \left( {f{'^2} - ff'' + {g^2}} \right) + \beta \left( {2ff'\text{g}' - {f^2}\text{g}''} \right) = 0,$

(2.14)

$\left[ {1 + \in \theta } \right]\theta '' + \in \theta {'^2} + Prf\theta ' - \gamma Pr\left[ {ff'\theta ' + {f^2}\theta ''} \right] = 0,$

(2.15)

assuming to the transformed conditions:

$\left\{ {\begin{array}{*{20}{l}} {f = 0, \ \ g = 0, \ \ f' = 1, \ \ \theta = 1, \ \ \ \ {\text{ at }}\eta \to 0, } \\ {f' \to 0, \ \ g \to 0, \ \ \theta \to 0, \ \ \ \ {\text{ at }}\eta \to \infty , } \end{array}} \right.$

(2.16)

where $\beta = {\lambda }_{1}a$ is relaxation time, $\gamma = {\lambda }_{3}a$ is thermal relaxation, $P r=\mu c_p / k$ is the Prandtl number, $\lambda=\Omega / a$ is the Ratio of rotation and stretching rates.

3. Numerical approach

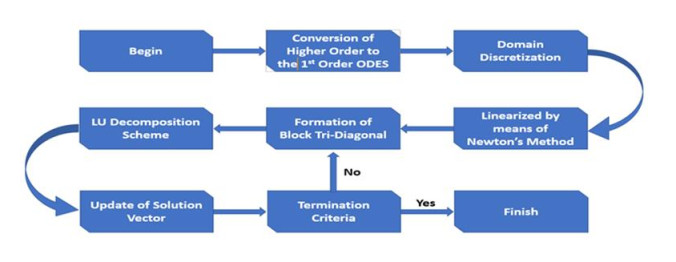
The physical perception of the current work requires the manipulation of an accurate solution. Karman approximation is used to model the equations at first, and then we arrive at the nonlinear system of ordinary differential equations (2.13)–(2.15) along with boundary conditions in Eq (2.16). Therefore, we used the implicit finite difference Keller-box approach that is mentioned in ^[19,20,21]. As follows are the details of the Keller Box Method:

Figure 2. Keller box method.

DownLoad: Full-Size Img PowerPoint

In order to use this strategy, we must first convert to a system of first-order equations and establish new variables $u\left(z, \eta \right), v\left(z, \eta \right), g\left(z, \eta \right), w\left(z, \eta \right), p\left(z, \eta \right)$ and $\theta \left(z, \eta \right)$ are

${f}^{'} = u, {u}^{'} = v, {g}^{'} = w, {\theta }^{'} = p,$

(3.1)

${v}^{'}+fv-{u}^{2}+2\lambda \left(g-\beta +w\right)+\beta \left(2fuv-{f}^{2}{v}^{'}\right) = 0,$

(3.2)

${w}^{'}+fw-ug-2\lambda \left(u+\beta \left[{u}^{2}-fv+{g}^{2}\right]\right)+\beta \left(2fuv-{f}^{2}{w}^{'}\right) = 0,$

(3.3)

$\left(1+ϵ\theta \right){p}^{'}+{P}_{r}fp-{P}_{r}\gamma \left(fup+{f}^{2}{p}^{'}\right) = 0,$

(3.4)

Implementing the Newton iteration ${f}_{j+1} = {f}_{j}+\delta {f}_{j}$ for all dependent variables included in the linearized nonlinear algebraic equations, substituting these formulations in the differential systems, and ignoring quadratic and higher order terms in $\delta$ the following linear tridiagonal arrangement:

$\delta {f}_{j}-\delta {f}_{j-1}-\frac{1}{2}h\left(\delta {u}_{j}+\delta {u}_{j-1}\right) = {\left({r}_{1}\right)}_{j-\frac{1}{2}} ,$

$\delta {u}_{j}-\delta {u}_{j-1}-\frac{1}{2}h\left(\delta {v}_{j}+\delta {v}_{j-1}\right) = {\left({r}_{2}\right)}_{j-\frac{1}{2}} ,$

$\delta {g}_{j}-\delta {g}_{j-1}-\frac{1}{2}h\left(\delta {w}_{j}+\delta {w}_{j-1}\right) = {\left({r}_{3}\right)}_{j-\frac{1}{2}} ,$

$\delta {\theta }_{j}-\delta {\theta }_{j-1}-\frac{1}{2}h\left(\delta {p}_{j}+\delta {p}_{j-1}\right) = {\left({r}_{4}\right)}_{j-\frac{1}{2}} ,$

where

${f}_{j-1}-{f}_{j}+\frac{h}{2}\left({u}_{j}+{u}_{j-1}\right) = {\left({r}_{1}\right)}_{j-\frac{1}{2}} ,$

${u}_{j-1}-{u}_{j}+\frac{h}{2}\left({v}_{j}+{v}_{j-1}\right) = {\left({r}_{2}\right)}_{j-\frac{1}{2}} ,$

${g}_{j-1}-{g}_{j}+\frac{h}{2}\left({w}_{j}+{w}_{j-1}\right) = {\left({r}_{3}\right)}_{j-\frac{1}{2}} ,$

${\theta }_{j-1}-{\theta }_{j}+\frac{h}{2}\left({p}_{j}+{p}_{j-1}\right) = {\left({r}_{4}\right)}_{j-\frac{1}{2}} ,$

${\psi }_{1} = {\psi }_{2} = \frac{1}{4}\left({v}_{j}+{v}_{j-1}\right)-\frac{\lambda \beta h}{2}\left({w}_{j}+{w}_{j-1}\right)+\frac{\beta h}{4}\left({u}_{j}+{u}_{j-1}\right)\left({v}_{j}+{v}_{j-1}\right)$

$-\frac{\beta }{2}\left({f}_{j}+{f}_{j-1}\right)\left({v}_{j}+{v}_{j-1}\right) ,$

${\psi }_{3} = {\psi }_{4} = -\frac{h}{2}\left({u}_{j}+{u}_{j-1}\right)+\frac{\beta h}{4}\left({v}_{j}+{v}_{j-1}\right)\left({f}_{j}+{f}_{j-1}\right) ,$

${\psi }_{5} = 1+\frac{h}{4}\left({f}_{j}+{f}_{j-1}\right)+\frac{\beta h}{4}\left(u+{u}_{j-1}\right)\left({f}_{j}+{f}_{j-1}\right)-\frac{\beta }{4}{\left({f}_{j}+{f}_{j-1}\right)}^{2} ,$

${\psi }_{6} = -1+\frac{h}{4}\left({f}_{j}+{f}_{j-1}\right)+\frac{\beta h}{4}\left(u+{u}_{j-1}\right)\left({f}_{j}+{f}_{j-1}\right)-\frac{\beta }{4}{\left({f}_{j}+{f}_{j-1}\right)}^{2} ,$

${\psi }_{7} = {\psi }_{8} = \lambda h ,$

${\psi }_{9} = {\psi }_{10} = \frac{\lambda h}{2}\left({f}_{i}+{f}_{j-1}\right) .$

Also,

${{(r}_{5})}_{j+\frac{1}{2}} = {v}_{j+1}-{v}_{j}-\frac{h}{4}\left({f}_{j}+{f}_{j-1}\right)\left({v}_{j}+{v}_{j-1}\right)+\frac{h}{4}{\left({v}_{j}+{v}_{j-1}\right)}^{2}$

$-h\lambda \left({g}_{j}-{g}_{j-1}\right)+\frac{h{\beta }_{1}}{2}\left({f}_{j}+{f}_{j-1}\right)\left({v}_{j}+{v}_{j-2}\right) ,$

${\alpha }_{1}\delta {f}_{j}+{\alpha }_{2}\delta {f}_{j-1}+{\alpha }_{3}\delta {u}_{j}+{\alpha }_{4}\delta {u}_{j-1}+{\alpha }_{5}\delta {v}_{j}+{\alpha }_{6}\delta {v}_{j-1}$

$+{\alpha }_{7}\delta {g}_{j}+{\alpha }_{8}\delta {g}_{j-1}+{\alpha }_{9}\delta {w}_{j}++{\alpha }_{10}\delta {g}_{j-1} = {\left({r}_{6}\right)}_{j-1} ,$

${\alpha }_{1} = {\alpha }_{2} = \frac{h}{4}{(w}_{j}+{w}_{j-1})+\frac{\lambda \beta h}{2}\left({v}_{j}+{v}_{j-1}\right)-\frac{\beta h}{4}\left({u}_{j}+{u}_{j-1}\right)\left({w}_{j}+{w}_{j-1}\right)$

$-\frac{\beta }{2}({f}_{j}+{f}_{j-1})\left({w}_{j}+{w}_{j-1}\right) ,$

${\alpha }_{3} = {\alpha }_{4} = \frac{\beta h}{4}\left({f}_{j}+{f}_{j-1}\right)\left({w}_{j}+{w}_{j-1}\right)-\frac{h}{4}\left({g}_{j}+{g}_{j-1}\right)-\lambda h-\lambda \beta h({f}_{j}+{f}_{j-1})\left({u}_{j}+{u}_{j-1}\right) ,$

${\alpha }_{5} = {\alpha }_{6} = \frac{\lambda \beta h}{2}\left({f}_{j}+{f}_{j-1}\right) ,$

${\alpha }_{7} = {\alpha }_{8} = -\frac{h}{4}\left({u}_{j}+{u}_{j-1}\right)-2h\lambda \beta ({g}_{j}+{g}_{j-1}) ,$

${\alpha }_{9} = 1+\frac{h}{4}\left({f}_{j}+{f}_{j-1}\right)+\frac{\beta h}{4}\left({f}_{j}+{f}_{j-1}\right)\left({u}_{j}+{u}_{j-1}\right)-\frac{\beta }{4}{\left({f}_{j}+{f}_{j-1}\right)}^{2} ,$

${\alpha }_{10} = -1+\frac{h}{4}\left({f}_{j}+{f}_{j-1}\right)+\frac{\beta h}{4}\left({f}_{j}+{f}_{j-1}\right)\left({u}_{j}+{u}_{j-1}\right)-\frac{\beta }{4}{\left({f}_{j}+{f}_{j-1}\right)}^{2} .$

Also,

${\left({r}_{6}\right)}_{j-1} = {w}_{j-1} – {w}_{j}-\frac{h}{4}\left({f}_{j}+{f}_{j-1}\right)\left({w}_{j}+{w}_{j-1}\right)+\frac{h}{4}\left({f}_{j}+{f}_{j-1}\right)\left({g}_{j}+{g}_{j-1}\right)+2h\lambda \left(\frac{{u}_{j}-{u}_{j-1}}{\begin{array}{c}2\\ \end{array}}\right)$

$+\beta \left[\right(\frac{{u}_{j}-{u}_{j-1}}{2}{)}^{2}-\left(\frac{{f}_{j}-{f}_{j-1}}{2}\right)\left(\frac{{v}_{j}-{v}_{j-1}}{2}\right)+\left(\frac{{g}_{j}-{g}_{j-1}}{2}{)}^{2}\right]$

$-\beta h\left[2\right(\frac{{f}_{j}-{f}_{j-1}}{2}\left)\right(\frac{{u}_{j}-{u}_{j-1}}{2}\left)\right(\frac{{w}_{j}-{w}_{j-1}}{2})-(\frac{{f}_{j}-{f}_{j-1}}{2}{)}^{2}\left(\frac{{w}_{j}-{w}_{j-1}}{h}\right)] ,$

${\beta }_{1}\delta {f}_{j}+{\beta }_{2}\delta {f}_{j-1}+{\beta }_{3}\delta {u}_{j}+{\beta }_{4}\delta {u}_{j-1}+{\beta }_{5}\delta {\theta }_{j}+{\beta }_{6}\delta {\theta }_{j-1}+{\beta }_{7}\delta {p}_{j}+{\beta }_{8}\delta {p}_{8} = {\left({r}_{7}\right)}_{j-1} ,$

${\beta }_{1} = {\beta }_{2} = \frac{{P}_{r}h}{{{4}_{j}}_{j-1}\frac{{P}_{r}rh}{\theta }\left({{u}_{j}+}_{j-1}\right)\frac{{P}_{r}r}{2}\left({{f}_{j}+}_{j-1}\right)} ,$

${\beta }_{3} = {\beta }_{4} = -\frac{{P}_{r}rh}{8}\left({f}_{j}+{f}_{j-1}\right)({p}_{j}+{p}_{j-1}) ,$

${\beta }_{5} = {\beta }_{6} = \varepsilon ({p}_{j}-{p}_{j-1}) ,$

${\beta }_{7} = (1+\varepsilon \theta )+\frac{\varepsilon h}{2}({p}_{j}+{p}_{j-1})+\frac{{P}_{r}h}{4{{f}_{j}+}_{j-1}\frac{{P}_{r}rh}{8}{{u}_{j}+}_{j-1}{{f}_{j}+}_{j-1}\frac{{P}_{r}r}{4}{{{f}_{j}+}_{j-1}}^{2}} ,$

${\beta }_{8} = -(1+\varepsilon \theta )+\frac{\varepsilon h}{2}({p}_{j}+{p}_{j-1})+\frac{{P}_{r}h}{4{{f}_{j}+}_{j-1}\frac{{P}_{r}rh}{8}{{u}_{j}+}_{j-1}{{f}_{j}+}_{j-1}\frac{{P}_{r}r}{4}{{{f}_{j}-}_{j-1}}^{2}} ,$

$({r}_{7}{)}_{j-\frac{1}{2}} = \left(1+\varepsilon \left(\frac{{\theta }_{j}+{\theta }_{j-1}}{2}\right)\right)({p}_{j-1}-{p}_{j})-\varepsilon h{\left(\frac{{p}_{j}+{p}_{j-1}}{2}\right)}^{2}-{P}_{r}h\left(\frac{{f}_{j}+{f}_{j-1}}{2}\right)\left(\frac{{p}_{j}+{p}_{j-1}}{2}\right)$

$+{P}_{r}rh\left[\left(\frac{{f}_{j}+{f}_{j-1}}{2}\right)\left(\frac{{u}_{j}+{u}_{j-1}}{2}\right)\left(\frac{{p}_{j}+{p}_{j-1}}{2}\right)+{\left(\frac{{f}_{j}+{f}_{j-1}}{2}\right)}^{2}\left(\frac{{p}_{j}+{p}_{j-1}}{h}\right)\right] .$

The block tridiagonal structure of the linearized difference equation often consists of variables or constants, but in this case, it consists of block matrices. The matrix elements in our scenario are defined as follows:

$\left[\begin{array}{cccccc}\left[{\mathbf{A}}_{1}\right]& \left[{\mathbf{C}}_{2}\right]& \cdot & \cdot & \cdot & \cdot \\ \left[{\mathbf{B}}_{2}\right]& \left[{\mathbf{A}}_{2}\right]& \left[{\mathbf{C}}_{2}\right]& \cdot & \cdot & \cdot \\ \cdot & \cdot & \cdot & \cdot & \cdot & \cdot \\ \cdot & \cdot & \cdot & \cdot & \cdot & \cdot \\ \cdot & \cdot & \cdot & \cdot & \cdot & \cdot \\ \cdot & \cdot & \cdot & \left[{\mathbf{B}}_{{\mathbf{j}}_{-1}}\right]& \left[{\mathbf{A}}_{{\mathbf{j}}_{-1}}\right]& \left[{\mathbf{C}}_{\mathbf{j}-1}\right]\\ \cdot & \cdot & \cdot & \cdot & {[\mathbf{B}}_{\mathbf{j}}]& \left[{\mathbf{A}}_{\mathbf{j}}\right]\end{array}\right]\left[\begin{array}{c}\left[{\delta }_{1}\right]\\ \left[{\delta }_{2}\right]\\ \cdot \\ \cdot \\ \cdot \\ \left[{\delta }_{j-1}\right]\\ \left[{\delta }_{j}\right]\end{array}\right] = \left[\begin{array}{c}\left[{r}_{1}\right]\\ \left[{r}_{2}\right]\\ \cdot \\ \cdot \\ \cdot \\ \left[{r}_{j-1}\right]\\ \left[{r}_{j}\right]\end{array}\right] .$

That is $\left[A\right]\left[\delta \right] = \left[r\right]$ , where

$\text{ A}_{1 = } \left[\begin{array}{ccccccc}1& 0& 0& 0& 0& 0& 0\\ 0& 1& 0& 0& 0& 0& 0\\ 0& 0& 0& 1& 0& 0& 0\\ 0& 0& 0& 0& 0& 1& 0\\ 0& 1& \frac{-h}{2}& 0& 0& 0& 0\\ 0& 0& 0& 1& \frac{-h}{2}& 0& 0\\ 0& 0& 0& 0& 0& 1& \frac{-h}{2}\end{array}\right] {, }$

$\text{B}_{2 = } \left[\begin{array}{ccccccc}1& \frac{-h}{2}& 0& 0& 0& 0& 0\\ {\varPsi }_{1}& {\varPsi }_{3}& {\varPsi }_{5}& {\varPsi }_{7}& {\varPsi }_{9}& 0& 0\\ {\alpha }_{1}& {\alpha }_{3}& {\alpha }_{5}& {\alpha }_{7}& {\alpha }_{9}& 0& 0\\ {\beta }_{1}& {\beta }_{3}& 0& 0& 0& {\beta }_{5}& {\beta }_{7}\\ 0& 0& 0& 0& 0& 0& 0\\ 0& 0& 0& 0& 0& 0& 0\\ 0& 0& 0& 0& 0& 0& 0\end{array}\right] {, }$

$\mathrm{C}2 = \left[\begin{array}{ccccccc}0& 0& 0& 0& 0& 0& 0\\ 0& 0& 0& 0& 0& 0& 0\\ 0& 0& 0& 0& 0& 0& 0\\ 0& 0& 0& 0& 0& 0& 0\\ 0& -1& \frac{-h}{2}& 0& 0& 0& 0\\ 0& 0& 0& -1& \frac{-h}{2}& 0& 0\\ 0& 0& 0& 0& 0& -1& \frac{-h}{2}\end{array}\right] {, }$

$\text{ A}_{2 = } \left[\begin{array}{ccccccc}-1& \frac{-h}{2}& 0& 0& 0& 0& 0\\ {\varPsi }_{1}& {\varPsi }_{4}& {\varPsi }_{6}& {\varPsi }_{8}& \varPsi & 0& 0\\ {\alpha }_{2}& {\alpha }_{1}& {\alpha }_{6}& {\alpha }_{8}& {\alpha }_{10}& 0& 0\\ {\beta }_{2}& {\beta }_{4}& 0& 0& 0& {\beta }_{6}& {\beta }_{8}\\ 0& 1& \frac{-h}{2}& 0& 0& 0& 0\\ 0& 0& 0& -1& \frac{-h}{2}& 0& 0\\ 0& 0& 0& 0& 0& 1& \frac{h}{2}\end{array}\right] {.}$

We assume that A is nonsingular and it can be factored into $\left[A\right] = \left[L\right]\left[U\right]$ , where

$\left[L\right] = \left[\begin{array}{cccccc}\left[{\mathbf{ \pmb{\mathsf{ α}} }}_{1}\right]& \cdot & \cdot & \cdot & \cdot & \cdot \\ \left[{\mathbf{ \pmb{\mathsf{ β}} }}_{2}\right]& \left[{\mathbf{ \pmb{\mathsf{ α}} }}_{2}\right]& \cdot & \cdot & \cdot & \cdot \\ \cdot & \cdot & \cdot & \cdot & \cdot & \cdot \\ \cdot & \cdot & \cdot & \cdot & \cdot & \cdot \\ \cdot & \cdot & \cdot & \cdot & \cdot & \cdot \\ \cdot & \cdot & \cdot & \cdot & \left[{\mathbf{ \pmb{\mathsf{ α}} }}_{{\mathbf{j}}_{-1}}\right]& \cdot \\ \cdot & \cdot & \cdot & \cdot & {[\mathbf{ \pmb{\mathsf{ β}} }}_{\mathbf{j}}]& \left[{\mathbf{ \pmb{\mathsf{ α}} }}_{\mathbf{j}}\right]\end{array}\right] \text{ and } \left[U\right] = \left[\begin{array}{cccccc}\left[\mathbf{I}\right]& \left[{\mathbf{\Gamma }}_{1}\right]& \cdot & \cdot & \cdot & \cdot \\ \cdot & \left[\mathbf{I}\right]& \left[{\mathbf{\Gamma }}_{2}\right]& \cdot & \cdot & \cdot \\ \cdot & \cdot & \cdot & \cdot & \cdot & \cdot \\ \cdot & \cdot & \cdot & \cdot & \cdot & \cdot \\ \cdot & \cdot & \cdot & \cdot & \cdot & \cdot \\ \cdot & \cdot & \cdot & \cdot & \left[\mathbf{I}\right]& \left[{\mathbf{ \pmb{\mathsf{ α}} }}_{{\mathbf{j}}_{-1}}\right]\\ \cdot & \cdot & \cdot & \cdot & \cdot & \left[\mathbf{I}\right]\end{array}\right] ,$

where $\left[I\right]$ is the matrix of order 7 and $\left[{{\rm{ \mathsf{ α} }}}_{\mathrm{i}}\right]$ and $\left[{\mathrm{\Gamma }}_{\mathrm{i}}\right]$ are 7x7 matrices which elements determined by the following equation:

$\left[{{\rm{ \mathsf{ α} }}}_{1}\right] = \left[{\mathrm{A}}_{1}\right] ,$

$\left[{\mathrm{A}}_{1}\right] = \left[{\mathrm{\Gamma }}_{1}\right]\left[{\mathrm{C}}_{1}\right] ,$

$\left[{\mathrm{A}}_{\mathrm{J}}\right] = \left[{\mathrm{A}}_{\mathrm{J}-1}\right]-\left[{\mathrm{B}}_{\mathrm{J}}\right]\left[{\mathrm{\Gamma }}_{\mathrm{J}-1}\right] , \text{J = 2, 3, 4, ..., J,}$

$\left[{\mathrm{A}}_{\mathrm{J}}\right]\left[{\mathrm{\Gamma }}_{\mathrm{J}}\right] = \left[{\mathrm{C}}_{\mathrm{J}}\right] ,\text{ J = 2, 3, 4, …, J-1,}$

$\left[\mathrm{L}\right]\left[\mathrm{U}\right]\left[\delta \right] = \left[\mathrm{r}\right] .$

If we define $\left[\mathrm{U}\right]\left[\delta \right] = \left[\mathrm{W}\right]$ , then equation becomes $\left[\mathrm{L}\right]\left[W\right] = \left[\mathrm{r}\right]$ , where

$\left[W\right] = \left[\begin{array}{c}\left[{W}_{1}\right]\\ \left[{W}_{2}\right]\\ \cdot \\ \cdot \\ \cdot \\ \left[{W}_{j-1}\right]\\ \left[{W}_{j}\right]\end{array}\right] , 1 \le j\le J ,$

and the $\left[{W}_{j}\right]$ are 7x1 column matrices. The elements W can be solved from

$\left[{{\rm{ \mathsf{ α} }}}_{1}\right]\left[{W}_{1}\right] = \left[{\mathrm{r}}_{1}\right] ,$

$\left[{{\rm{ \mathsf{ α} }}}_{2}\right]\lfloor {W}_{j}\rfloor = \lfloor {r}_{j}\rfloor - \lfloor {B}_{j}\perp {W}_{j}\rfloor , 2 \le j\le J .$

The step in which ${\mathrm{\Gamma }}_{\mathrm{J}}$ , ${\mathbf{ \pmb{\mathsf{ α}} }}_{\mathbf{j}}$ and ${W}_{j}$ are calculated is usually referred to as the forward sweep. Once the elements of W are found, the solution $\delta$ in the so-called backward sweep in which the elements are obtained by the following relations:

$\left[{\delta }_{j}\right] = \left[{W}_{j}\right] ,$

$\left[{\delta }_{j}\right] = \left[{W}_{j}\right]-\lfloor {\Gamma }_{j}\rfloor \lfloor {\delta }_{j+1}\rfloor , 1 \le j\le J -1.$

These calculations are repeated until some convergence criterion is satisfied and calculations are stopped where $\left|\delta {v}_{0}^{\left(i\right)}\right|\le {\varepsilon }_{1},$ where ${\varepsilon }_{1}$ is a small, prescribed value.

4. Results and discussion

We were able to find a solution to the problem of laminar boundary layer flow in rotating Maxwell fluid that was induced by uniform elastic stretching material. In addition, the CC model is put to use in the study of the characteristics of heat transmission. The primary objective of this section is to present and analyze the impacts that physical parameters have on the solutions, and this will be accomplished through the use of instances. To do this, we depict the temperature and velocity profiles in Figures 3–8.

Figure 3. Impact of

$\beta$ on

$f\mathrm{'}\left(\eta \right)$ .

DownLoad: Full-Size Img PowerPoint

Figure 4. Impact of

$\lambda$ on

$f'\left(\eta \right)$ .

DownLoad: Full-Size Img PowerPoint

Figure 5. Impact of

$\beta$ on

$g\left(\eta \right)$ .

DownLoad: Full-Size Img PowerPoint

Figure 6. Impact of

$\lambda$ on

$g\left(\eta \right)$ .

DownLoad: Full-Size Img PowerPoint

Figure 7. Impact of

$ϵ$ on

$\theta \left(\eta \right)$ .

DownLoad: Full-Size Img PowerPoint

Figure 8. Impact of

$\gamma$ on

$\theta \left(\eta \right)$ .

DownLoad: Full-Size Img PowerPoint

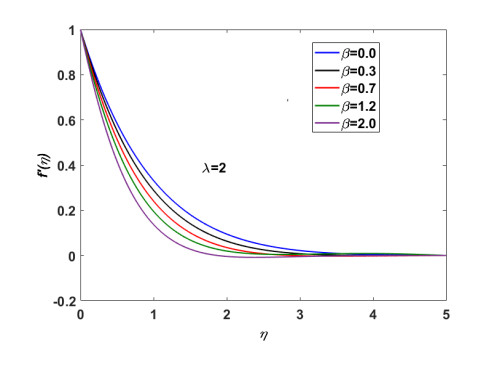
When $\lambda$ = 0.2, we draw conclusions about the profiles of the non-dimensional x-component of velocity $f'\left(\eta \right)$ at various values of the Deborah's number $\beta$ is shown in . Deborah's number contrasts the observation time scale with the fluid relaxation time of memory distortion. When Deborah number is low, the material reacts like a completely viscous fluid and the recovery time is quick. When $\beta$ increases, the horizontal velocity of the vehicle tends to decrease. A greater value of $\beta$ implies a more physically significant viscous force, which resists the flow and causes the velocity to drop. When the value of $\beta$ is increased, we observe that the patterns are skewed in the direction of the border. This suggests that the thickness of the boundary layer decreases as $\beta$ increases. A substance with a high Deborah number, however, receives a strong like response. When $\eta$ is increasing and it diminishes beyond the boundary layer, the function $f'\left(\eta \right)$ gradually decreases. It is evident that the velocity fluid $f'\left(\eta \right)$ and boundary layer thickness decrease as the parameter $\beta$ , or the parameter associated to fluid relaxation time, increases.

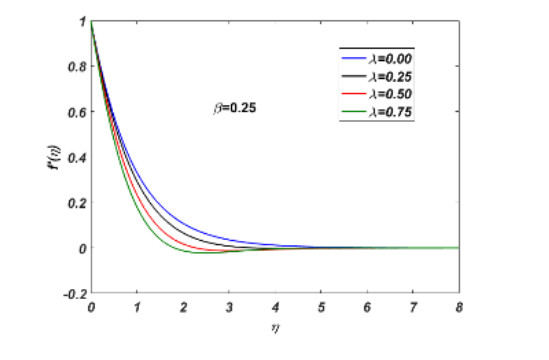
reveals that the behavior of $f'\left(\eta \right)$ affects the rotational parameter $\lambda$ . $\lambda$ = 0 refers to a condition where the frame is not rotating. The ratio of rotation rate to stretching rate rises as $\lambda$ grows. From a physical perspective, when $\lambda$ grows, the rotation rate increases more quickly than the stretching rate. Due to rotational effects, the value of $f'$ for bigger values of $\lambda$ turns negative close to the border.

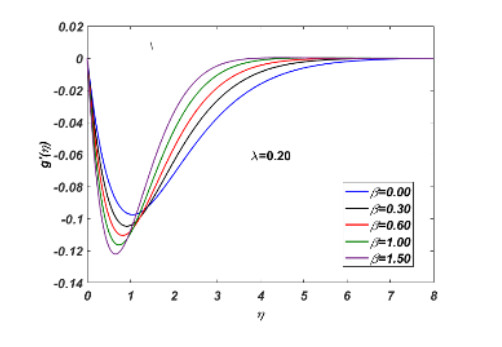
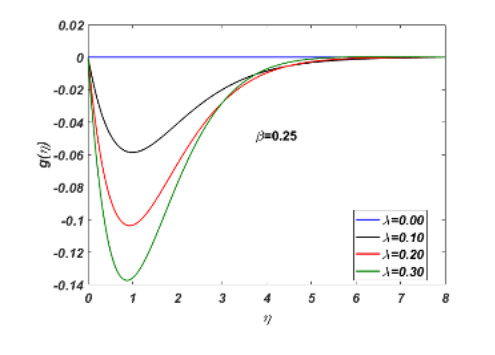
reveal that the features of Deborah number $\beta$ on the $g\left(\eta \right)$ , the sheet is only stretched in the x-direction, therefore flow in the y-direction is only anticipated as a result of the rotating frame, where the magnitude of the velocity field $g\left(\eta \right)$ , which is proportional to the y-component of velocity, decreases further from the stretching surface. Because of the rotating effect, flow only occurs in the negative y-direction in this case, as indicated by the negative value of $g\left(\eta \right)$ . The velocity profile $g\left(\eta \right)$ as a function of the rotational constant λ is depicts in . As can be observed, the rotation parameter helps the flow move in the opposite direction of y. The profile $g\left(\eta \right)$ is comparable to the function - $\eta$ exp(- $\eta$ ) for lower lambda. However, an oscillating pattern in the profile of $g\left(\eta \right)$ is seen for large values of the rotation parameter λ.

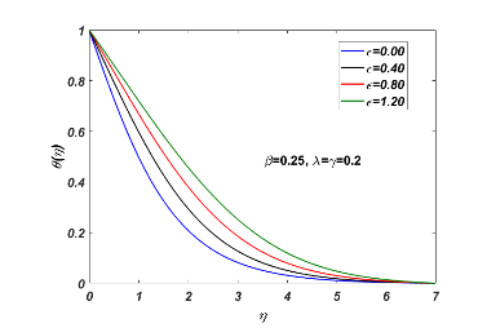
shows that the on $\theta \left(\eta \right)$ for different values of $\varepsilon$ with given values of other factors. In this study, variable thermal conductivity is taken into account, and its expression implies that the parameter $\varepsilon$ has a direct relationship to thermal conductivity. A bigger value of $\varepsilon$ indicates a larger thermal diffusivity, which results in a thicker temperature penetration depth.

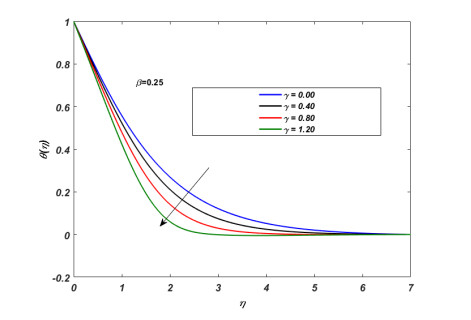
includes the $\theta$ curve with non-dimensionality for different thermal relaxation times. The curve tends to move closer to the stretching border when parameter $\gamma$ is directly proportional to the increase in thermal relaxation time. When a longer thermal relaxation time is taken into account, it suggests that the temperature penetration depth is shortened.

In order to verify the accuracy of our calculations, we compare the results for $f''\left(0\right)$ to those found in the prior literature in the non-rotating frame $\lambda = 0,$ is shown in . contain a numerical summary of the results for the wall velocity gradients $f''\left(0\right)$ and $g'\left(0\right)$ .

Table 1. Code validation test for

${f}^{''}\left(0\right)$ for

$\lambda = 0$ .

$\beta$	Abel et al. ^[13]	1.000000
0.0	0.999962	1.101903
0.4	1.101850	1.196711
0.8	1.196692	1.285363
1.2	1.285257	1.368758
1.6	1.368641	1.368758
2.0	0.999962	1.000000

| Show Table

DownLoad: CSV

Table 2. Numeric data for

${f}^{''}\left(0\right)$ and

$g'\left(0\right)$ for

$\lambda = 0.2$ .

$\beta$	$-f''\left(0\right)$	$-g'\left(0\right)$
0.0	1.01091	1.01091
0.4	1.13091	1.13091
0.8	1.19832	1.19832
1.0	1.25312	1.25312
1.2	1.30912	1.30912

| Show Table

DownLoad: CSV

5. Conclusions

A numerical investigation of Maxwell thermal fluid flow in a rotating frame with variable thermal conductivity and Cattaneo-Christov heat flux is carried out. A Keller box finite difference-based approach is used to solve nonlinear equations governing self-similar flow. The following summarizes the main features of this work:

● Viscoelastic effects provide flow resistance in both the x and y directions. However, when a larger value of the viscoelastic component $\beta$ is considered, the temperature $\theta$ rises slightly.

● As $Pr$ rises, the wall slope of the temperature rises and becomes closer to zero, which indicates that $Pr$ is vanishing.

● As expected, increasing $ϵ$ increases the thickness of the thermal boundary layer while decreasing the gradient of the wall temperature.

● By putting $\beta = 0$ , the current model reduces to the Newtonian fluid condition. The setting $\gamma = 0$ also makes it possible to retrieve the Fourier-law aspect.

● The consideration of rotational effects reduces the thickness of the hydrodynamic boundary layer.

These findings could be extended to include the unsteady dynamics of the incompressible UCM fluids that are caused by body forces.

Conflict of interest

The authors declare no conflict of interest.

References

[1]	S. H. Han, L. C. Zheng, C. R. Li, X. X. Zhang, Coupled flow and heat transfer in viscoelastic fluid with Cattaneo-Christov heat flux model, Appl. Math. Lett., 38 (2014), 87–93. https://doi.org/10.1016/j.aml.2014.07.013 doi: 10.1016/j.aml.2014.07.013
[2]	M. Mustafa, Cattaneo-Christov heat flux model for rotating flow and heat transfer of upper-convected Maxwell fluid, AIP Adv., 5 (2015), 047109. https://doi.org/10.1063/1.4917306 doi: 10.1063/1.4917306
[3]	B. Krishnendu, H. Krishnendu, A. Ahmed, Dual solutions in boundary layer flow of Maxwell fluid over a porous shrinking sheet, Chinese Phys. B, 23 (2014), 124701. https://doi.org/10.1088/1674-1056/23/12/124701 doi: 10.1088/1674-1056/23/12/124701
[4]	C. Fetecau, M. Jamil, C. Fetecau, I. Siddique, A note on the second problem of Stokes for Maxwell fluids, Int. J. Non-Linear Mech., 44 (2009), 1085–1090. https://doi.org/10.1016/j.ijnonlinmec.2009.08.003 doi: 10.1016/j.ijnonlinmec.2009.08.003
[5]	C. Fetecau, M. Athar, C. Fetecau, Unsteady flow of generalized Maxwell fluid with fractional derivative due to a constantly accelerating plate, Comput. Math. Appl., 57 (2009), 596–603. https://doi.org/10.1016/j.camwa.2008.09.052 doi: 10.1016/j.camwa.2008.09.052
[6]	Y. Mahsud, N. A. Shah, D. Vieru, Influence of time-fractional derivatives on the boundary layer flow of Maxwell fluids, Chinese J. Phys., 55 (2017), 1340–1351. https://doi.org/10.1016/j.cjph.2017.07.006 doi: 10.1016/j.cjph.2017.07.006
[7]	I. Khan, N. A. Shah, L. C. C. Dennis, A scientific report on heat transfer analysis in mixed convection flow of Maxwell fluid over an oscillating vertical plate, Sci. Rep., 7 (2017), 40147. https://doi.org/10.1038/srep40147 doi: 10.1038/srep40147
[8]	S. K. Nandy, Unsteady flow of Maxwell fluid in the presence of nanoparticles toward a permeable shrinking surface with Navier slip, J. Taiwan Inst. Chem. Eng., 52 (2015) 22–30. https://doi.org/10.1016/j.jtice.2015.01.025 doi: 10.1016/j.jtice.2015.01.025
[9]	W. Na, N. A. Shah, I. Tlili, I. Siddique, Maxwell fluid flow between vertical plates with damped shear and thermal flux: free convection, Chinese J. Phys., 65 (2020), 367–376. https://doi.org/10.1016/j.cjph.2020.03.005 doi: 10.1016/j.cjph.2020.03.005
[10]	L. M. Cao, X. H. Si, L. C. Zheng, Convection of Maxwell fluid over stretching porous surface with heat source/sink in presence of nanoparticles: Lie group analysis, Appl. Math. Mech., 37 (2016), 433–442. https://doi.org/10.1007/s10483-016-2052-9 doi: 10.1007/s10483-016-2052-9
[11]	M. E. Karim, M. A. Samad, Effect of Brownian diffusion on squeezing elastico-viscous nanofluid flow with Cattaneo-Christov heat flux model in a channel with double slip effect, Appl. Math., 11 (2020), 277–291. https://doi.org/10.4236/am.2020.114021 doi: 10.4236/am.2020.114021
[12]	S. Shateyi, S. S. Motsa, Thermal radiation effects on heat and mass transfer over an unsteady stretching surface, Math. Probl. Eng., 2009 (2009), 1–13. https://doi.org/10.1155/2009/965603 doi: 10.1155/2009/965603
[13]	M. S. Abel, J. V. Tawade, J. N. Shinde, The effects of MHD flow and heat transfer for the UCM fluid over a stretching surface in presence of thermal radiation, Adv. Math. Phys., 2012 (2012), 1–21. https://doi.org/10.1155/2012/702681 doi: 10.1155/2012/702681
[14]	C. I. Christov, On frame indifferent formulation of the Maxwell-Cattaneo model of finite-speed heat conduction, Mech. Research Commun., 36 (2009), 481–486. https://doi.org/10.1016/j.mechrescom.2008.11.003 doi: 10.1016/j.mechrescom.2008.11.003
[15]	B. Straughan, Thermal convection with the Cattaneo-Christov model, Int. J. Heat Mass Transfer, 53 (2010), 95–98. https://doi.org/10.1016/j.ijheatmasstransfer.2009.10.001 doi: 10.1016/j.ijheatmasstransfer.2009.10.001
[16]	V. Tibullo, V. Zampoli, A uniqueness result for the Cattaneo-Christov heat conduction model applied to incompressible fluids, Mech. Research Commun., 38 (2011), 77–79. https://doi.org/10.1016/j.mechrescom.2010.10.008 doi: 10.1016/j.mechrescom.2010.10.008
[17]	S. A. M. Haddad, Thermal instability in Brinkman porous media with Cattaneo-Christov heat flux, Int. J. Heat Mass Transfer, 68 (2014), 659–668. https://doi.org/10.1016/j.ijheatmasstransfer.2013.09.039 doi: 10.1016/j.ijheatmasstransfer.2013.09.039
[18]	R. M. Khan, N. Imran, Z. Mehmood, M. Sohail, A Petrov-Galerkin finite element approach for the unsteady boundary layer upper-convected rotating Maxwell fluid flow and heat transfer analysis, Waves Random Complex Media, 2022 (2022), 1–18. https://doi.org/10.1080/17455030.2022.2055201 doi: 10.1080/17455030.2022.2055201
[19]	S. Bilal, A. H. Majeed, R. Mahmood, I. Khan, A. H. Seikh, E. S. M. Sherif, Heat and mass transfer in hydromagnetic second-grade fluid past a porous inclined cylinder under the effects of thermal dissipation, diffusion and radiative heat flux, Energies, 13 (2020), 1–17. https://doi.org/10.3390/en13010278 doi: 10.3390/en13010278
[20]	A. H. Majeed, S. Bilal, R. Mahmood, M. Y. Malik, Heat transfer analysis of viscous fluid flow between two coaxially rotated disks embedded in permeable media by capitalizing non-Fourier heat flux model, Phys. A, 540 (2020), 1231182 https://doi.org/10.1016/j.physa.2019.123182 doi: 10.1016/j.physa.2019.123182
[21]	S. Bilal, A. Tassaddiq, A. H. Majeed, K. S. Nisar, F. Ali, M. Y. Malik, Computational and physical examination about the aspects of fluid flow between two coaxially rotated disks by capitalizing non-Fourier heat flux theory: finite difference approach, Front. Phys., 7 (2019), 209. https://doi.org/10.3389/fphy.2019.00209 doi: 10.3389/fphy.2019.00209

This article has been cited by:

1.	Xingyang Li, Guokun Zhang, Deao Li, Qiang Li, Weiwei Xu, Muming Hao, Influence of mass conservation cavitation boundary on transient performance of water-lubricated bearings, 2024, 36, 1070-6631, 10.1063/5.0215294
2.	Karim K. Ahmed, Hisham H. Hussein, Hamdy M. Ahmed, Wafaa B. Rabie, Wassim Alexan, Analysis of the dynamical behaviors for the generalized Bogoyavlvensky–Konopelchenko equation and its analytical solutions occurring in mathematical physics, 2024, 15, 20904479, 103000, 10.1016/j.asej.2024.103000
3.	Qasim Ali, Muhammad Amir, Ahmed Sayed M. Metwally, Usman Younas, Ahmed Zubair Jan, Ayesha Amjad, Investigation of MHD fractionalized viscous fluid and thermal memory with slip and Newtonian heating effect: a fractional model based on Mittag-Leffler kernel, 2024, 149, 1388-6150, 8257, 10.1007/s10973-024-13205-5
4.	Xiangning Zhou, Muhammad Amer Qureshi, Nargis Khan, Wasim Jamshed, Siti Suzilliana Putri Mohamed Isa, Nanthini Balakrishnan, Syed M. Hussain, Thermosolutal Marangoni convective flow of MHD tangent hyperbolic hybrid nanofluids with elastic deformation and heat source, 2024, 22, 2391-5471, 10.1515/phys-2024-0082
5.	Adil Darvesh, Manuel Sánchez-Chero, Carla Angélica Reyes Reyes, Pedro Aníbal Solís Céspedes, Marcos Timina Alvarez, Max Fernando Urbina Cárdenas, Carmen Elvira Rosas-Prado, Mathematical Analysis of Bio-nanofluid Flow over a Nonlinear Tapering Artery with Stenosis Conditions Using Cross Fluid Viscosity Model, 2023, 13, 2191-1630, 2082, 10.1007/s12668-023-01210-1
6.	Sadia Irshad, Shah Jahan, Afraz Hussain Majeed, Ehab Ghith, Mehdi Tlija, Atif Asghar, Nusrat Rehman, Numerical investigation of stagnation point heat transfer with MHD effects using finite difference method: Analysis of thermal absorption and generation, 2024, 14, 2158-3226, 10.1063/5.0219843
7.	Salman Zeb, Awais Adnan, Waqar Ahmad, Shafiq Ahmad, Inna Samuilik, Thermal stratification and heat generation/absorption impacts on stagnation point flow of MHD UCM fluid through a permeable medium, 2024, 10, 26668181, 100692, 10.1016/j.padiff.2024.100692
8.	Sadia Irshad, Shah Jahan, José Mendes Machado, Paweł Śliwiński, Krzysztof kedzia, Ahmed Zubair Jan, MHD Casson flow across a stretched surface in a porous material: a numerical study, 2025, 8, 2520-8160, 10.1007/s41939-024-00628-8
9.	Afraz Hussain Majeed, Ahmed Zubair Jan, Atif M. Alamri, Salman A. AlQahtani, Mohamed R. Ali, Ahmed S. Hendy, Recent developments in the Darcy-Forchheimer model of magnetized tetra hybrid nanofluid activation energy/joule heating in a stenotic artery, 2024, 59, 2214157X, 104346, 10.1016/j.csite.2024.104346
10.	Mohamed Boujelbene, Sohail Rehman, Mohamed Balegh, Entropy degradation in a dual diffusion flow of a non‐Newtonian fluid in inclined channel using Keller‐Box approach, 2024, 0170-4214, 10.1002/mma.9843
11.	Feng Ding, Gang Tao, Peng Wen, High plateau drift characteristics of spin-stabilized projectile from individual recoilless rifle with rifling, 2024, 36, 1070-6631, 10.1063/5.0203055
12.	Issa El Glili, Abdelmajid Saidi, Youness Foukhari, Mohamed Driouich, Mohamed Sammouda, Numerical simulation of unsteady blood based Carreau nanofluid flow in an inclined porous saturated artery having overlapping stenosis with electro-osmosis, 2024, 1040-7782, 1, 10.1080/10407782.2024.2353886
13.	R. Madan Kumar, R. Srinivasa Raju, M. Anil Kumar, Effects of chemical reaction, Soret and Dufour parameters on MHD dissipative Williamson nanofluid flow over a slippery stretching sheet through a porous medium, 2023, 0228-6203, 1, 10.1080/02286203.2023.2261812

Reader Comments

Your name:*

Email:*
© 2023 the Author(s), licensee AIMS Press. This is an open access article distributed under the terms of the Creative Commons Attribution License (http://creativecommons.org/licenses/by/4.0)

通讯作者: 陈斌, bchen63@163.com

1.
沈阳化工大学材料科学与工程学院沈阳 110142

AIMS Mathematics

1.8 3.4

Metrics

Article views(1494) PDF downloads(73) Cited by(13)

Preview PDF

Download XML

Export Citation

Article outline

Show full outline

Figures and Tables

Figures(8) / Tables(2)

AIMS Mathematics

Numerical investigations of nonlinear Maxwell fluid flow in the presence of non-Fourier heat flux theory: Keller box-based simulations

Related Papers:

Abstract

1. Introduction

2. Mathematical modeling

3. Numerical approach

4. Results and discussion

5. Conclusions

Conflict of interest

References

This article has been cited by:

Reader Comments

通讯作者: 陈斌, bchen63@163.com

Metrics

Figures and Tables

Other Articles By Authors

Catalog

AIMS Mathematics

Numerical investigations of nonlinear Maxwell fluid flow in the presence of non-Fourier heat flux theory: Keller box-based simulations

Related Papers:

Abstract

1. Introduction

2. Mathematical modeling

3. Numerical approach

4. Results and discussion

5. Conclusions

Conflict of interest

References

This article has been cited by:

Reader Comments

通讯作者: 陈斌, bchen63@163.com

Metrics

Figures and Tables

Other Articles By Authors

Related pages

Tools

Export File

Citation

Format

Content

Catalog